Characterization of the Mixing Flow Structure of Molten Steel in a Single Snorkel Vacuum Refining Furnace
Abstract
:1. Introduction
2. Mathematical Modeling
2.1. Euler-Euler Two-Fluid Model
2.2. Momentum Transfer
2.3. User-Defined Scalar (UDS) Transport Model
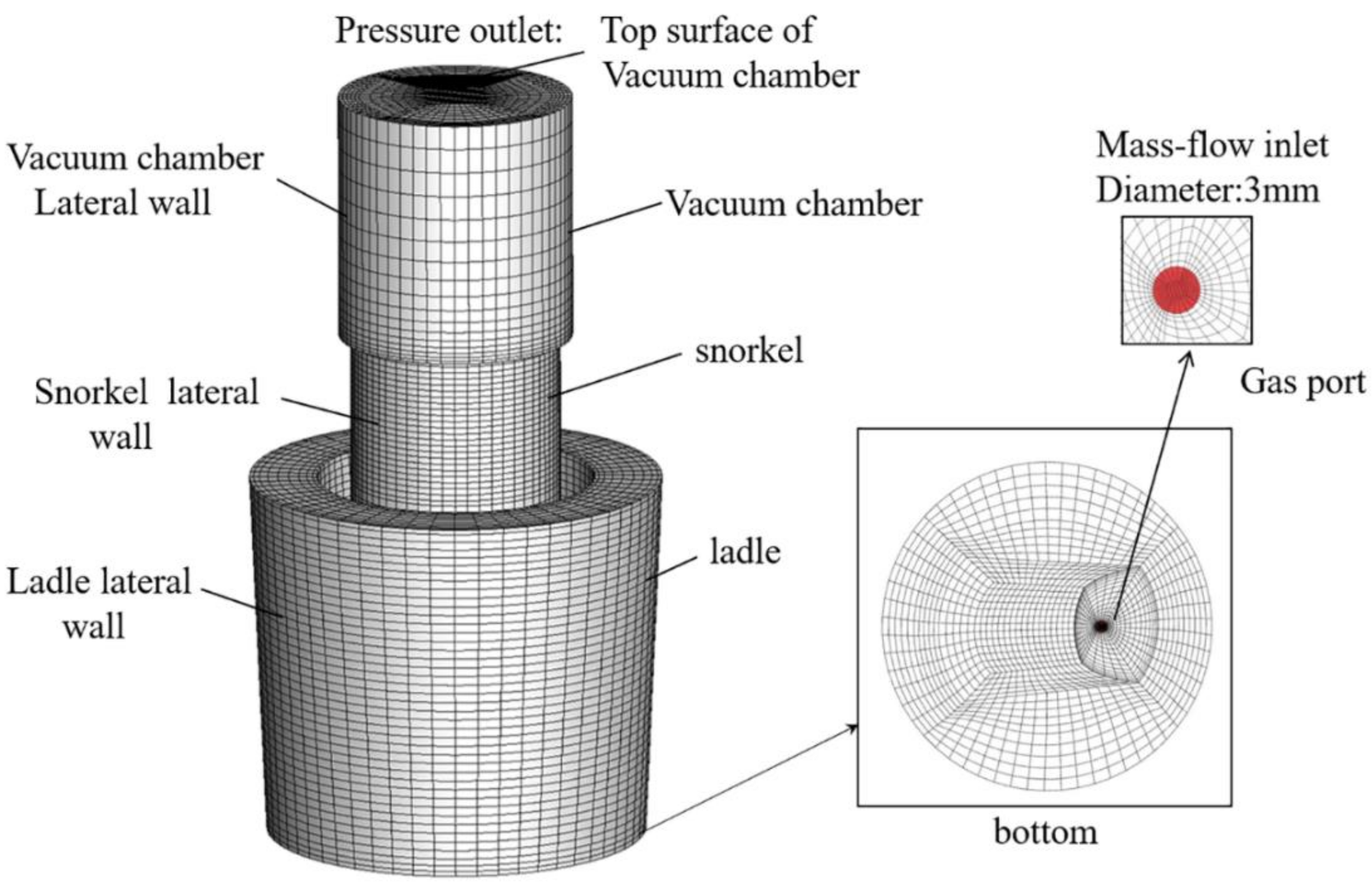
2.4. Experimental Setup
2.5. Numerical Details
3. Results and Discussion
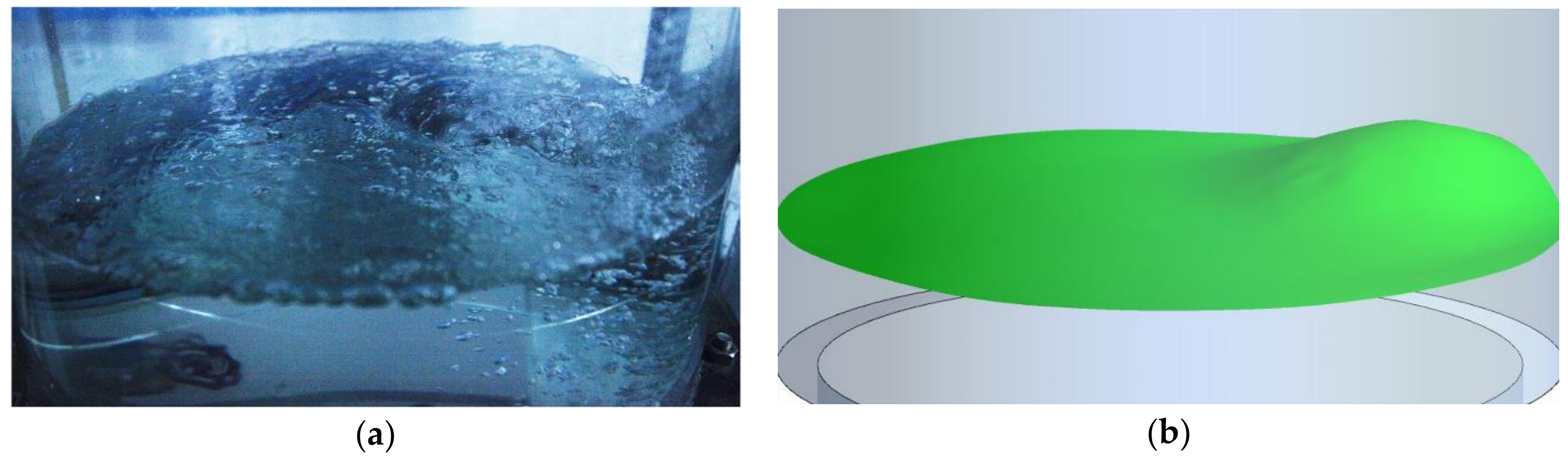
3.1. Validation of Numerical Model
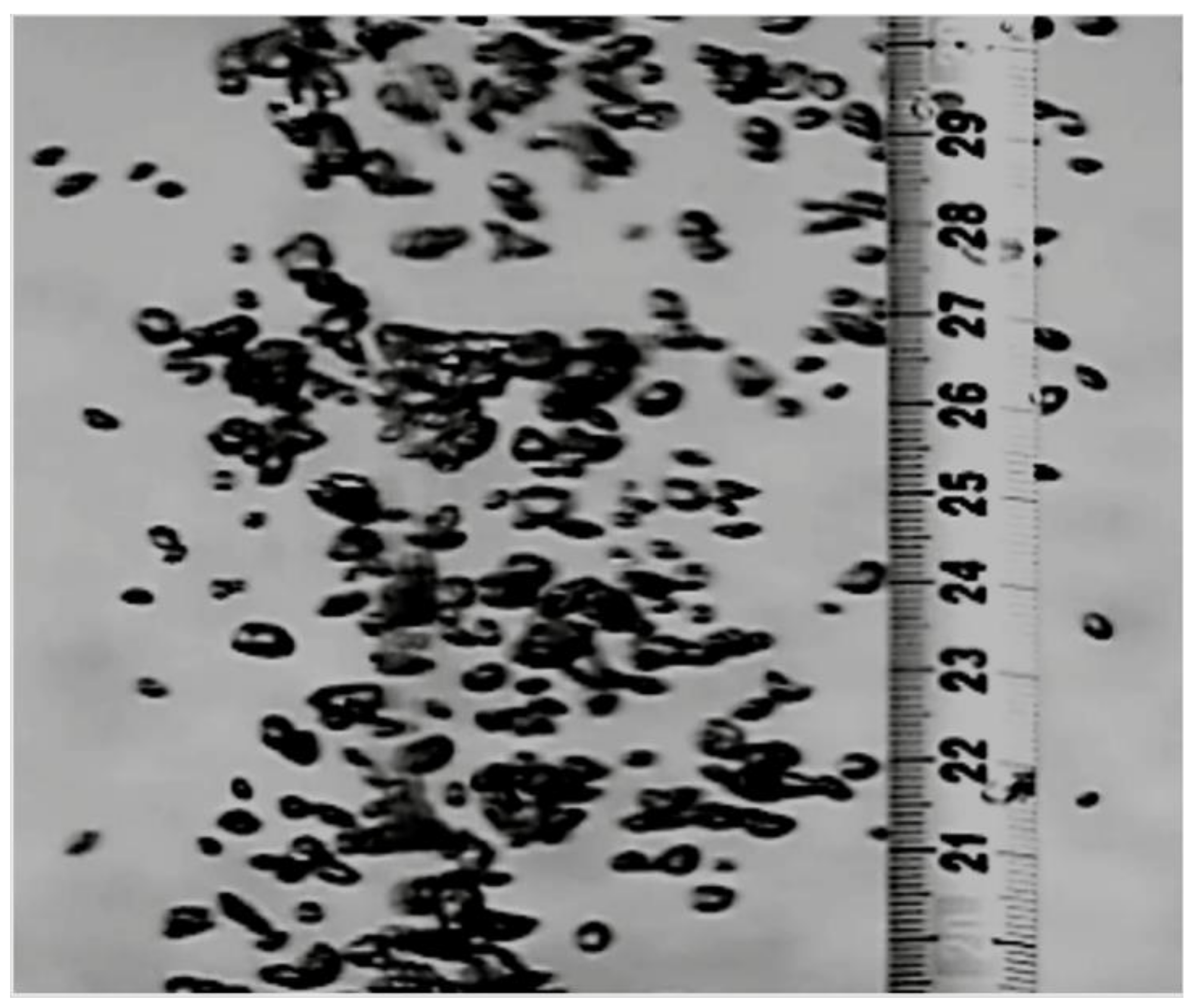
3.1.1. Fluctuation
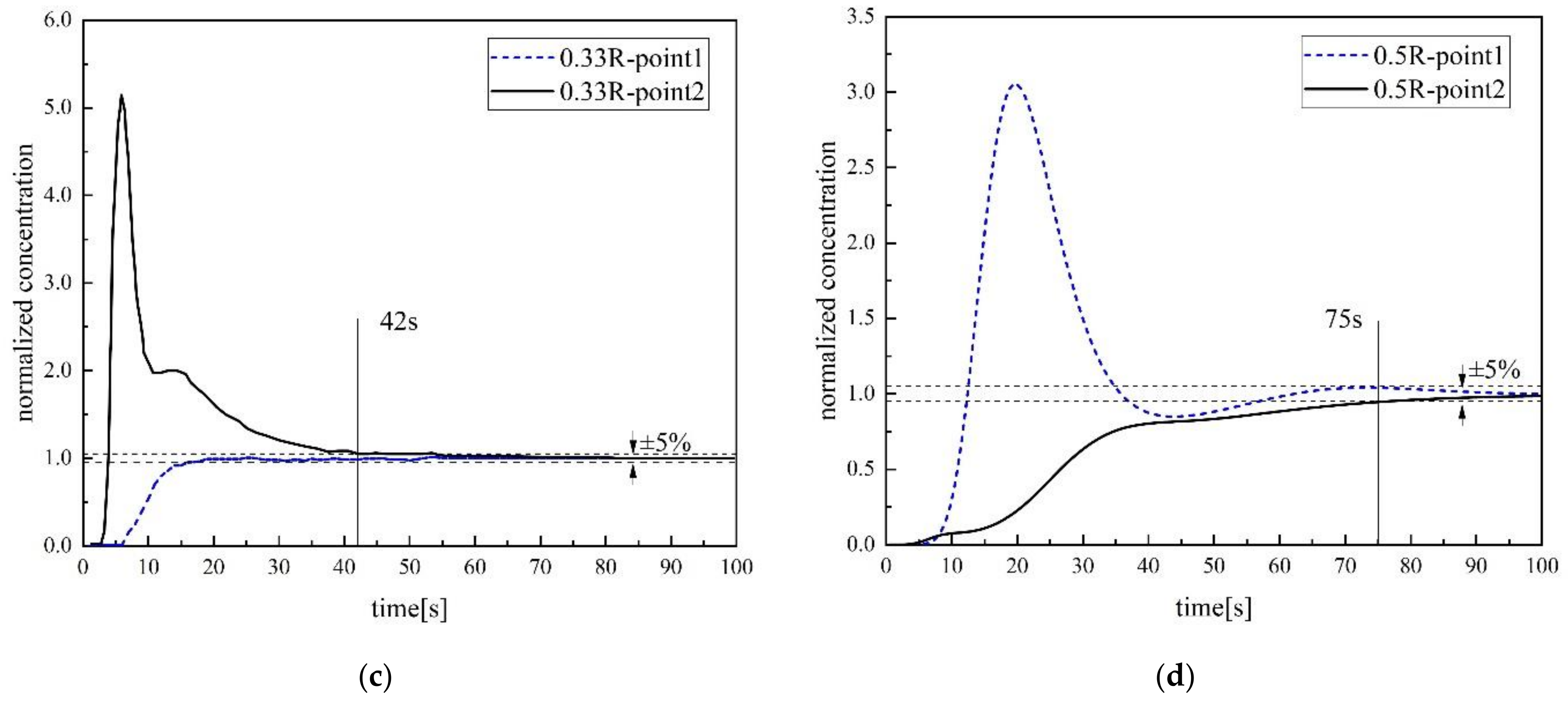
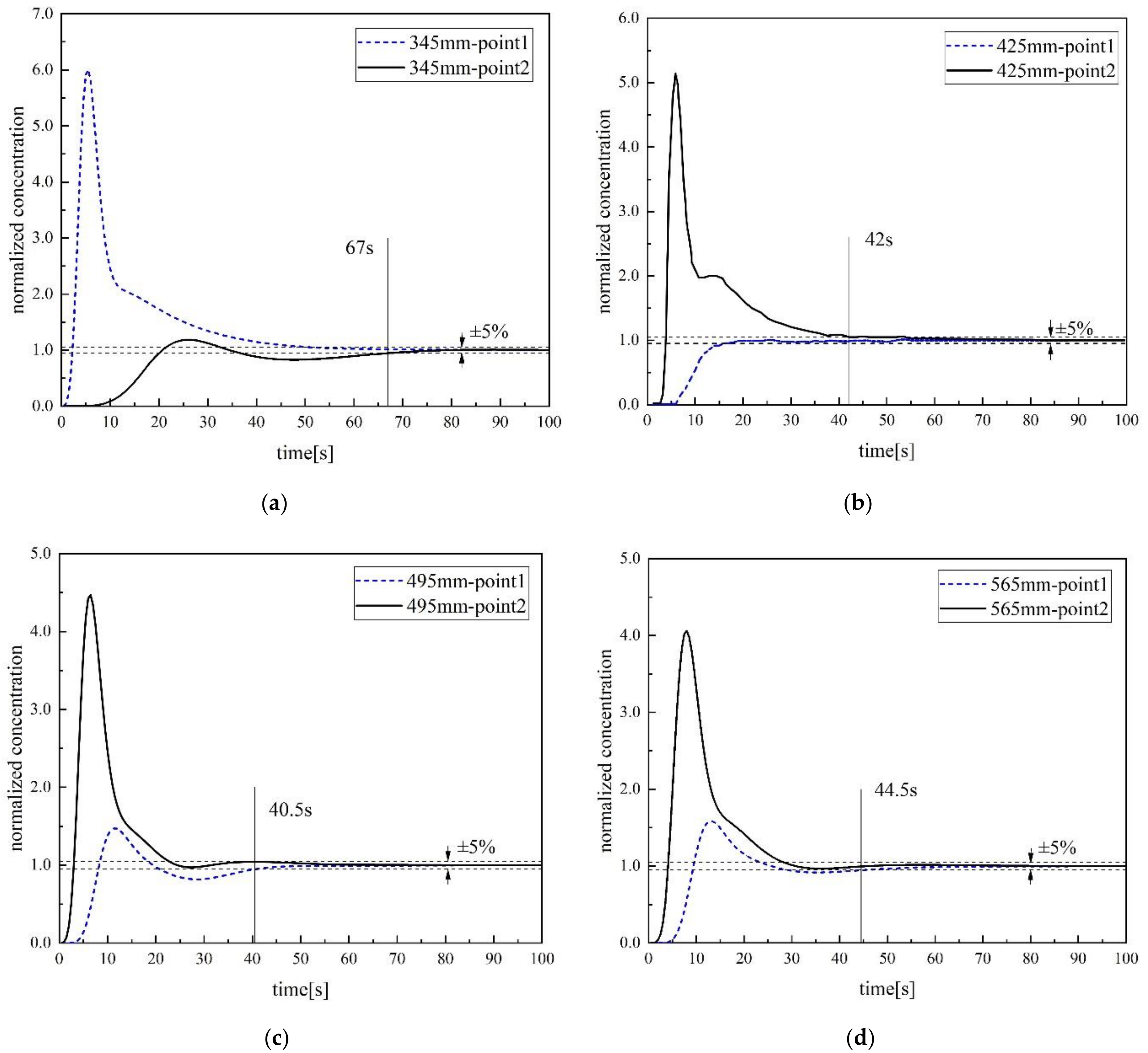
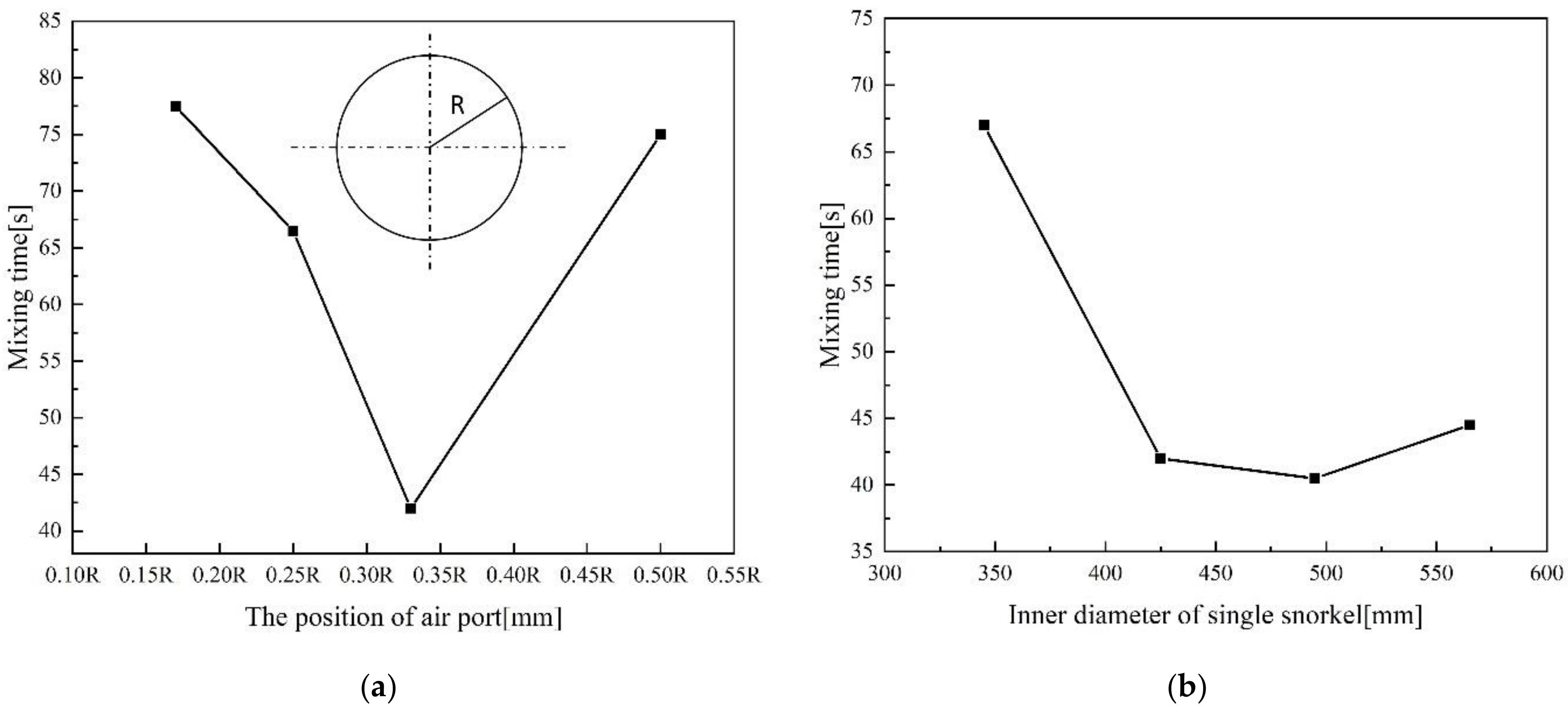
3.1.2. Comparison of Mixing Time
3.2. Flow Field
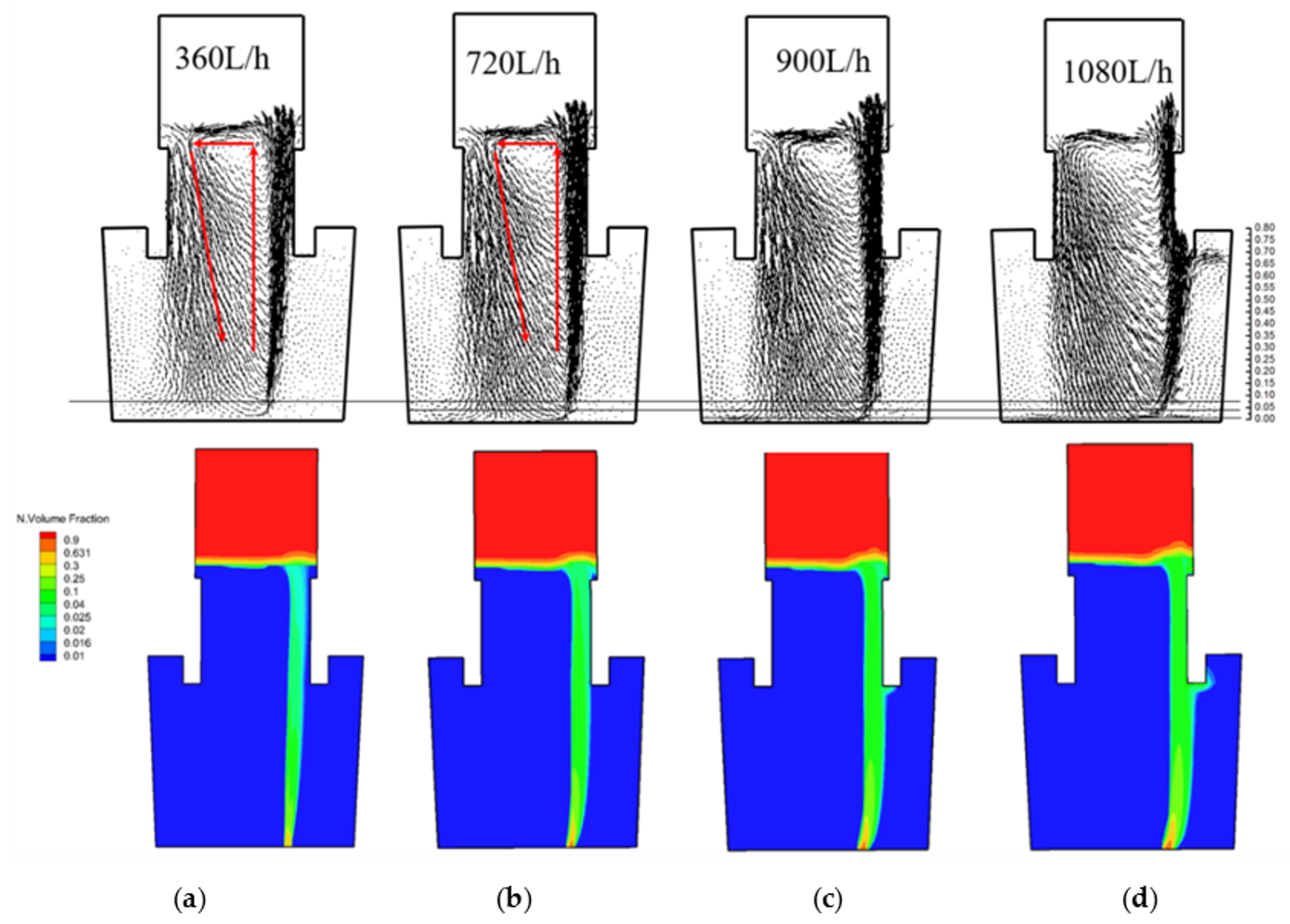
3.2.1. Comparison of Flow Field
3.2.2. Flow Field in SSF
3.3. Mixing Time of SSF
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, J. Research and Development Group of Single Snorkel Vacuum Refining Furnace with Ar Blowing: Experimental results of a Single Snorkel Vacuum Refining Furnace with Ar Blowing. Dalian Spec. Steel 1978, 1, 5–26. [Google Scholar]
- Cheng, G.G.; Zhang, J.; Yang, N.Z. Dynamic Model of Deoxidation for Bearing Steel Treated with Single–snorkel Refining Furnace. Spec. Steel 1994, 15, 22–25. [Google Scholar]
- Cheng, G.G.; Zhang, J.; Tong, F.S. An Oil–water Model for Slag Entrainment in Melting Bath of Vacuum Refining Unit with Single Snorkel. Spec. Steel 1994, 15, 27–30. [Google Scholar]
- Wang, C.; Zhang, J.; Yang, N.Z. Investigation on Water Modelling of Flow Field and Circulation Flow Rate for Single Snorkel Refining Furnace. Spec. Steel 1998, 19, 12–15. [Google Scholar]
- Qin, Z.; Zhu, M.T.; Cheng, G.G. A Study on Water Model for Alloy Adding Pattern and Mixing Characteristics of Single Snorkel Refining Furnace. Spec. Steel 2010, 19, 5–7. [Google Scholar]
- Zhao, J.L.; Zhang, J.; Cheng, G.G. Water Model Experiment on the Single Nozzle Oxygen–blowing Refining Furnace. Res. Iron Steel 1992, 20, 10–14. [Google Scholar]
- Wang, H.; Zhao, J.; Cheng, G.G. Water Model of Decarburization for Refining Furnace with a Single Snorkel. J. Anhui Univ. Technol. 2005, 22, 623–626. [Google Scholar] [CrossRef]
- Duan, J.P.; Zhang, Y.L.; Yang, X.M. Experiment Research on Decarburization Technology of 80t Single Snorkel Vacuum Refining Equipment. Iron Steel 2011, 46, 21–25. [Google Scholar]
- Duan, J.P.; Zhang, Y.L.; Yang, X.M. Desulphurization Behavior of an 80t Multifunctional Single Snorkel Vacuum Refining Furnace. Steelmaking 2011, 27, 44–48. [Google Scholar]
- Duan, J.P.; Zhang, Y.L.; Yang, X.M. Analysis of Commercial production Test on Metallurgical Functions of an 80t Single Snorkel Vacuum Refining Furnace. Spec. Steel 2012, 19, 26–29. [Google Scholar]
- Qin, Z.; Pan, H.W.; Zhu, M.T. Modeling Study on Flow Behaviors of Molten Steel in Single Snorkel Refining Furnace. Iron Steel 2011, 46, 22–25. [Google Scholar]
- Yang, X.M.; Zhang, M.; Wang, F. Mathematical Simulation of Flow Field for Molten Steel in an 80–ton Single Snorkel Vacuum Refining Furnace. Steel Res. Int. 2012, 83, 52–82. [Google Scholar] [CrossRef]
- Aoki, H.; Kitamura, S.; Miyamoto, K. Development of New Secondary Refining Process–READ. Ironsteelmaker 1999, 26, 17–21. [Google Scholar]
- Kitamura, S.; Aoki, H.; Miyamoto, K.; Furuta, H.; Yamashita, K.; Yonezawa, K. Development of a Novel Degassing Process Consisting with Single Large Immersion Snorkel and a Bottom bubbling ladle. ISIJ Int. 2000, 40, 455–459. [Google Scholar] [CrossRef]
- Miyamoto, K.; Aoki, H.; Kitamura, S. Comparison of the Vacuum Degasser Characteristics by Water Model. CAMP–ISIJ 1998, 11, 756. [Google Scholar]
- Aoki, H.; Furuta, H.; Hirashims, N. Experimental Result of 175ton Vacuum Degasser by Large Snorkel with Bottom Gas Bubbling. CAMP–ISIJ 1998, 11, 757. [Google Scholar]
- Aoki, H.; Furuta, H.; Fujiwara, K. Improvement of 350ton DH to New Type Vacuum Degasser by Large Snorkel with Bottom Gas bubbling. CAMP–ISIJ 1998, 11, 758. [Google Scholar]
- Sugano, H.; Shinkai, A.; Kato, K. Steelmaking of Stainless Steel by New Type Vacuum Degasser. CAMP–ISIJ 1999, 12, 747. [Google Scholar]
- Miyamoto, K.; Sugano, H.; Shinkai, A.; Kato, K.; Kitamura, S.; Yamashita, K. Reaction Behavior Stainless Steel in New Type Vacuum Degasser (Development of New Type Vacuum Degasser (REDA–5)). CAMP–ISIJ 1999, 12, 748. [Google Scholar]
- Mondal, M.K.; Maruoka, N.; Gupta, G.S.; Nogami, H.; Kitamura, S.; Shibata, H. Study of Fluid Flow and Mixing Behaviour of a Vacuum Degasser. Trans. Indian Inst. Met. 2012, 65, 321–331. [Google Scholar] [CrossRef]
- Schiller, L.; Naumann, A. A drag coefficient correlation. Z. Ver. Deutsch. Ing. 1935, 77, 318–320. [Google Scholar]
- Diaz, M.E.; Montes, F.J.; Galan, M.A. Influence of the lift force closures on the numerical simulation of bubble plumes in a rectangular bubble column. Chem. Eng. Sci. 2009, 64, 930–944. [Google Scholar] [CrossRef]
- Tomiyama, A.; Shimada, N.; Galan, M. A numerical method for bubbly flow simulation based on a multi-fluid model. J. Press. Vessel Technol. 2001, 123, 510–516. [Google Scholar] [CrossRef]
- Tomiyama, A.; Tamai, H.; Zun, I.; Hosokawa, S. Transverse migration of single bubbles in simple shear flows. Chem. Eng. Sci. 2002, 57, 1849–1858. [Google Scholar] [CrossRef]
- Frank, T.; Zwart, P.J.; Krepper, E.; Prasser, H.-M.; Lucas, D. Validation of CFD models for mono- and polydisperse air–water two-phase flows in pipes. Nucl. Eng. Des. 2008, 238, 647–659. [Google Scholar] [CrossRef]
- Wellek, R.M.; Agrawal, A.K.; Skelland, A.H.P. Shape of liquid drops moving in liquid media. AIChE J. 1966, 12, 834–1032. [Google Scholar] [CrossRef]
- Cheung, C.P.; Yeoh, G.H.; Tu, J.Y. On the modelling of population balance in isothermal vertical bubbly flows-Average bubble number density approach. Chem. Eng. Process. 2007, 46, 742–756. [Google Scholar] [CrossRef]
- Yakhot, V.; Orszag, S.A.; Thangam, S.; Gatski, T.B.; Speziale, C.G. Development of turbulence models for shear flows by a double expansion technique. Phys. Fluids A Fluid Dyn. 1992, 4, 1510–1520. [Google Scholar] [CrossRef]
- Zhu, M.Y.; Sha, J.; Huang, Z.Z. Numerical Simulation of Molten Steel Flow in a RH Degasser. Acta Metall. Sin. 2000, 36, 1175–1178. [Google Scholar]







| Vacuum Vessel | Submerged Pipe | Ladle | ||||
|---|---|---|---|---|---|---|
| D/m | H/m | D/m | H/m | D1/m | D2/m | H/m |
| 0.475 | 0.500 | 0.425 | 0.412 | 0.770 | 0.860 | 0.992 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, F.; Liu, J.; Liu, Z.; Cheung, S.C.P.; Li, B. Characterization of the Mixing Flow Structure of Molten Steel in a Single Snorkel Vacuum Refining Furnace. Metals 2019, 9, 400. https://doi.org/10.3390/met9040400
Qi F, Liu J, Liu Z, Cheung SCP, Li B. Characterization of the Mixing Flow Structure of Molten Steel in a Single Snorkel Vacuum Refining Furnace. Metals. 2019; 9(4):400. https://doi.org/10.3390/met9040400
Chicago/Turabian StyleQi, Fengsheng, Jinxin Liu, Zhongqiu Liu, Sherman C. P. Cheung, and Baokuan Li. 2019. "Characterization of the Mixing Flow Structure of Molten Steel in a Single Snorkel Vacuum Refining Furnace" Metals 9, no. 4: 400. https://doi.org/10.3390/met9040400
APA StyleQi, F., Liu, J., Liu, Z., Cheung, S. C. P., & Li, B. (2019). Characterization of the Mixing Flow Structure of Molten Steel in a Single Snorkel Vacuum Refining Furnace. Metals, 9(4), 400. https://doi.org/10.3390/met9040400






