Nanoindentation Investigation on the Size-Dependent Creep Behavior in a Zr-Cu-Ag-Al Bulk Metallic Glass
Abstract
:1. Introduction
2. Materials and Methods
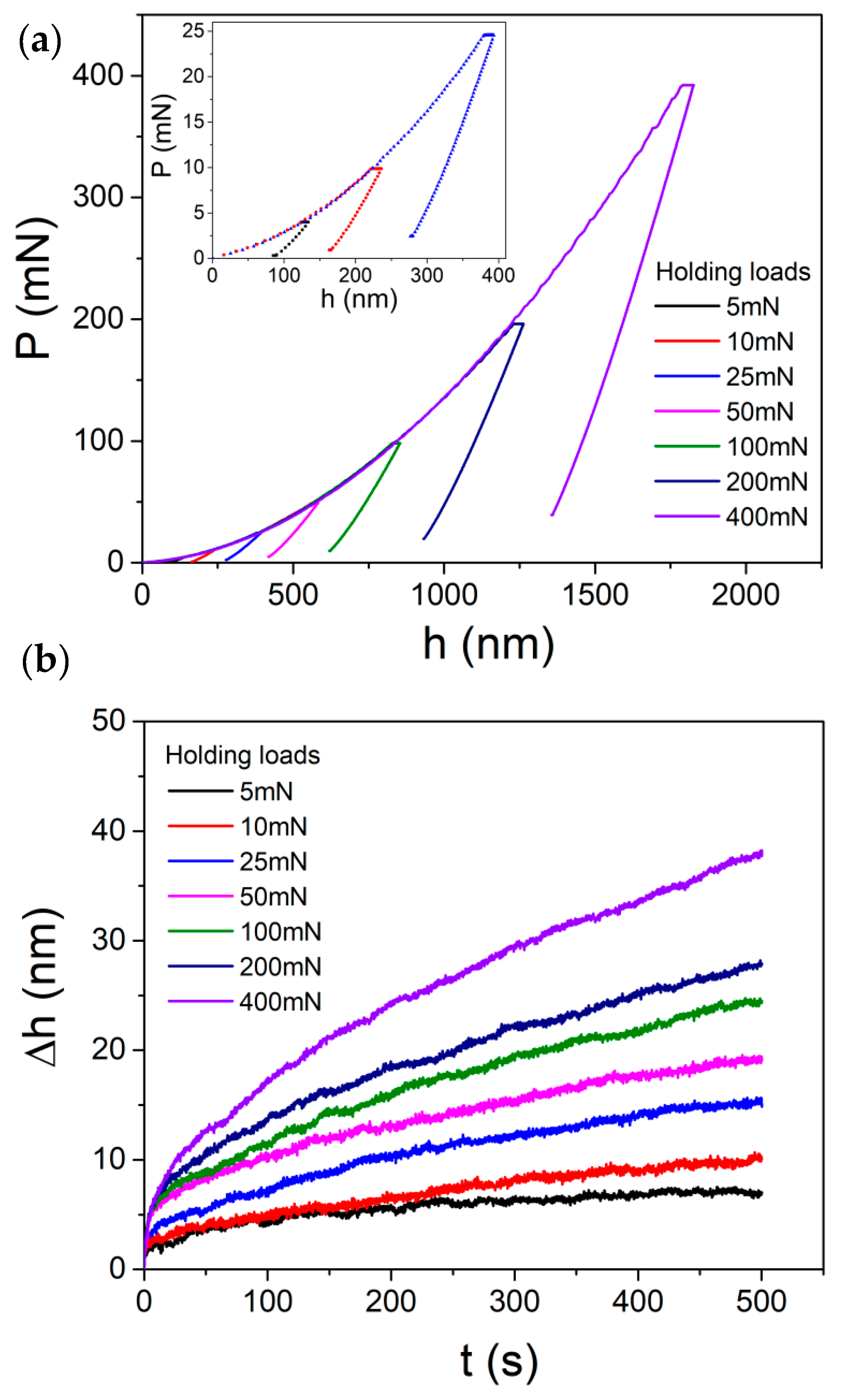
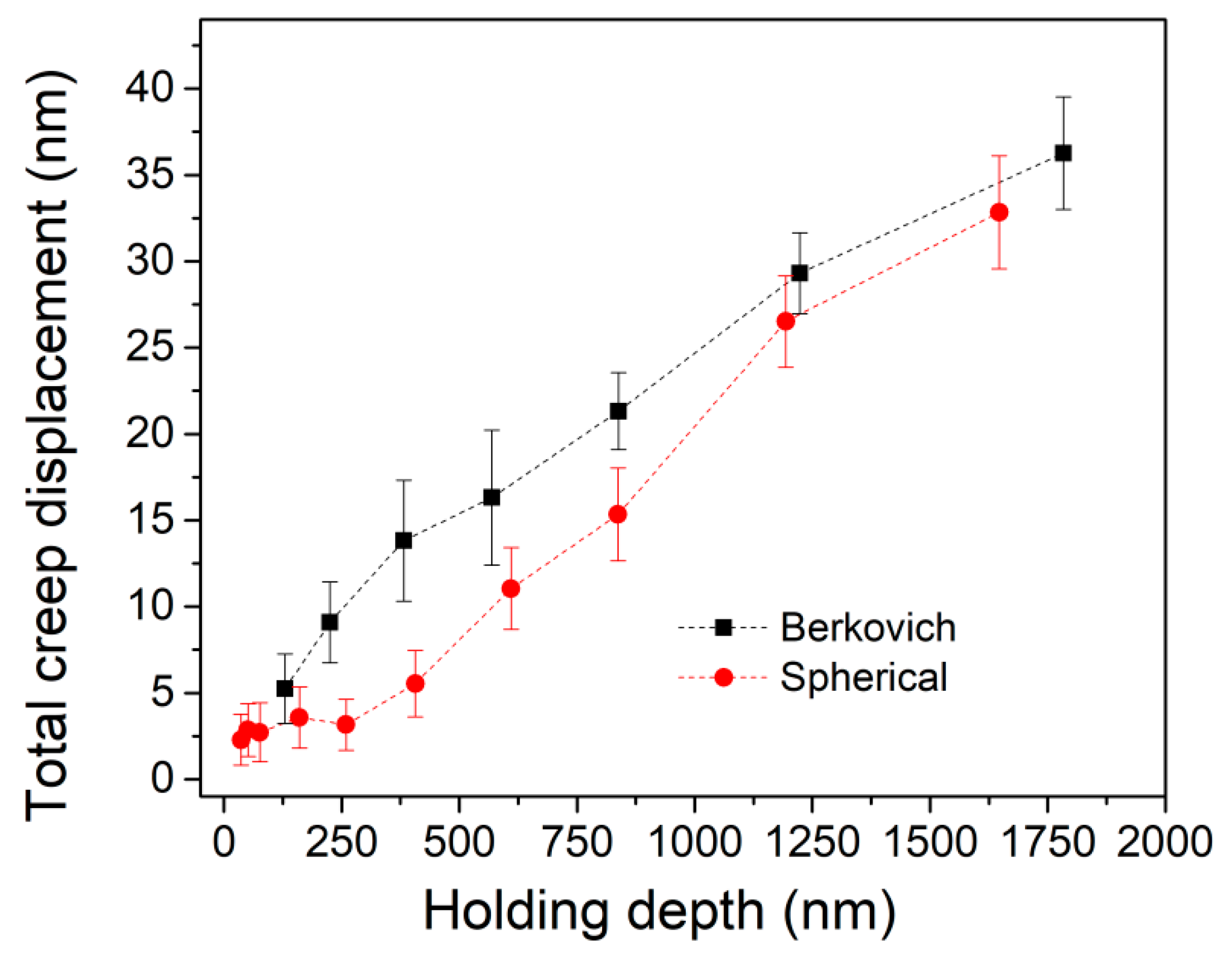
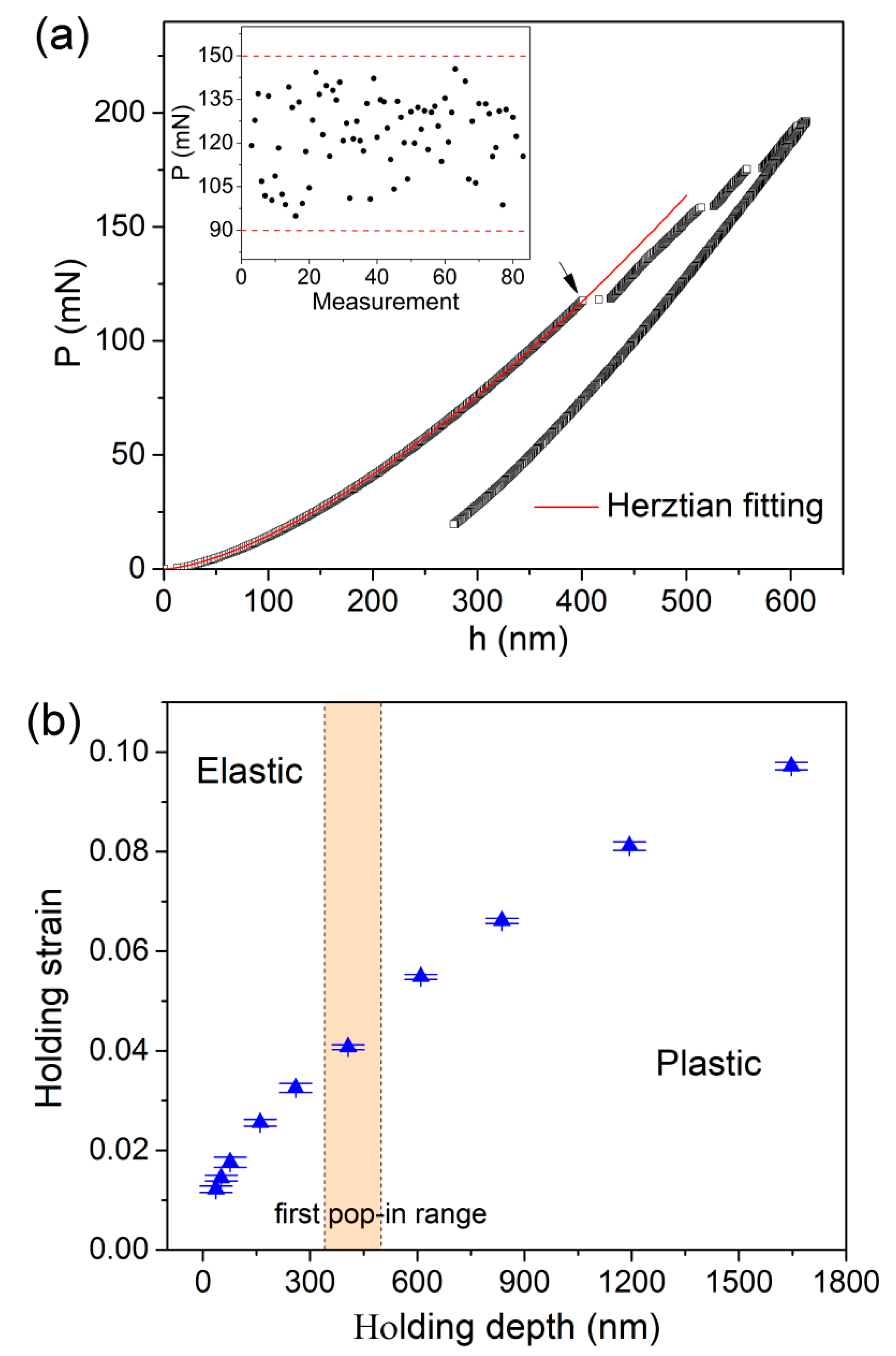
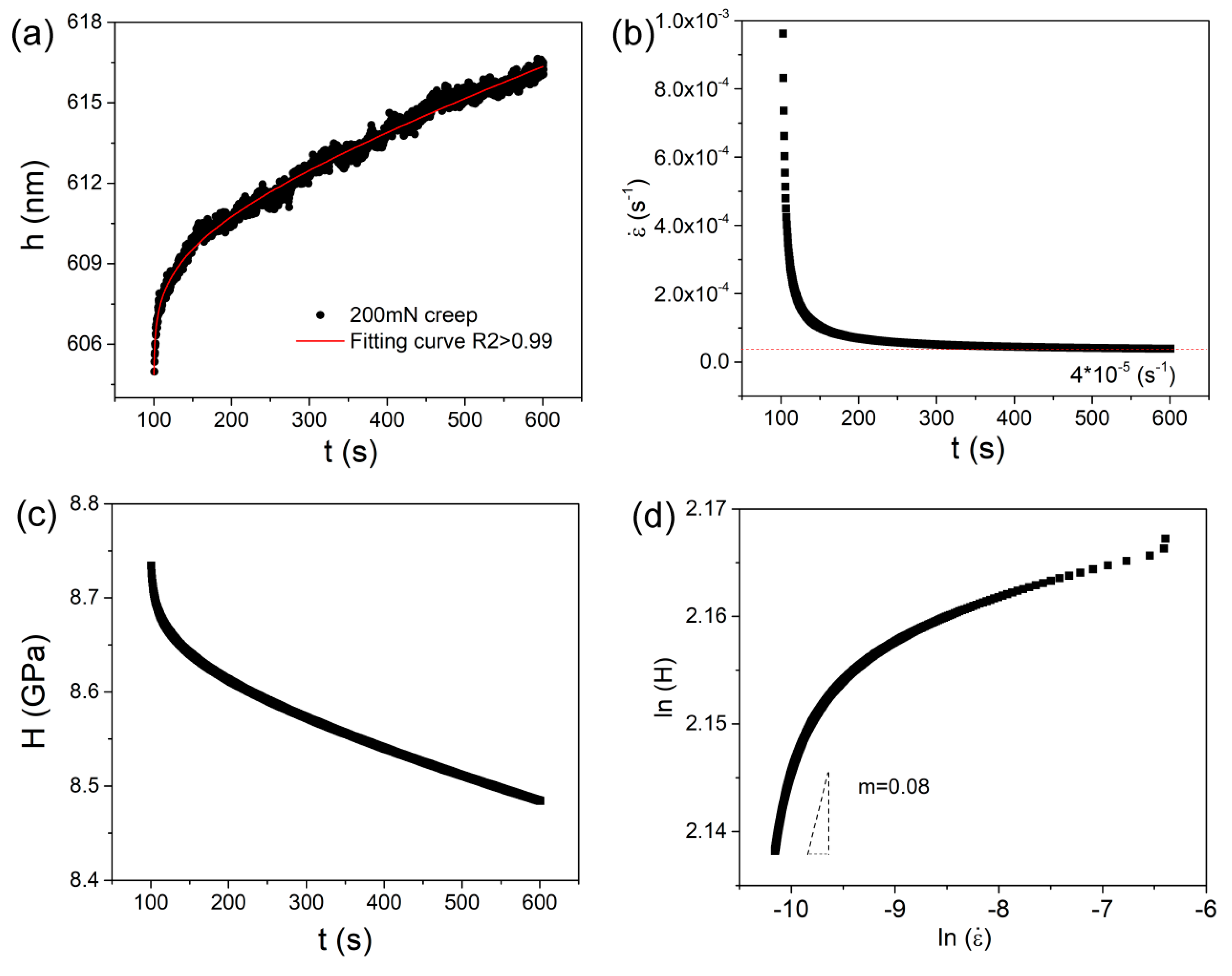
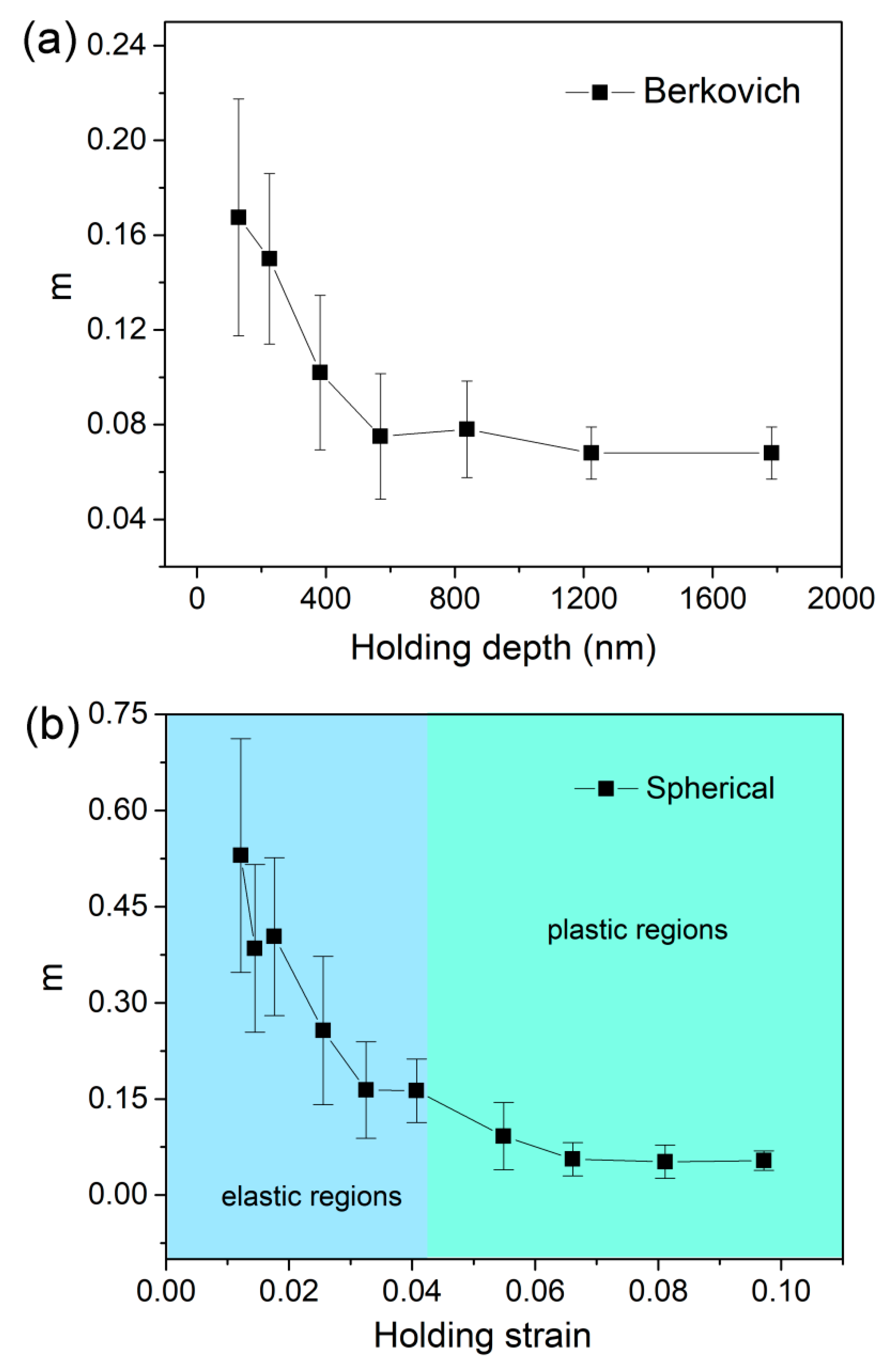
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Inoue, A.; Shen, B.; Koshiba, H.; Kato, H.; Yavari, A.R. Cobalt-based bulk glassy alloy with ultrahigh strength and soft magnetic properties. Nat. Mater. 2003, 2, 661–663. [Google Scholar] [CrossRef]
- Jun, W.K.; Willens, R.H.; Duwez, P.O.L. Non-crystalline structure in solidified gold–silicon alloys. Nature 1960, 187, 869–870. [Google Scholar]
- Schuh, C.A.; Hufnagel, T.C.; Ramamurty, U. Mechanical behavior of amorphous alloys. Acta Mater. 2007, 55, 4067–4109. [Google Scholar] [CrossRef]
- Loffler, J.F. Bulk metallic glasses. Intermetallics 2003, 11, 529–540. [Google Scholar] [CrossRef]
- Argon, A.S. Plastic deformation in metallic glasses. Acta Metall. 1979, 27, 47–58. [Google Scholar] [CrossRef]
- Mayr, S.G. Activation energy of shear transformation zones: A key for understanding rheology of glasses and liquids. Phys. Rev. Lett. 2006, 97, 195501. [Google Scholar] [CrossRef] [PubMed]
- Jang, D.; Greer, J.R. Transition from a strong-yet-brittle to a stronger-and-ductile state by size reduction of metallic glasses. Nat. Mater. 2010, 3, 215–219. [Google Scholar] [CrossRef]
- Guo, H.; Yan, P.F.; Wang, Y.B.; Tan, J.; Zhang, Z.F.; Sui, M.L.; Ma, E. Tensile Ductility and Necking of Metallic Glass. Nat. Mater. 2007, 10, 735–739. [Google Scholar] [CrossRef]
- Ma, Y.; Cao, Q.P.; Qu, S.X.; Jiang, J.Z. Stress-state-dependent deformation behavior in Ni–Nb metallic glassy film. Acta Mater. 2012, 60, 4136–4143. [Google Scholar] [CrossRef]
- Schuh, C.A. Nanoindentation studies of materials. Mater. Today 2006, 9, 32–40. [Google Scholar] [CrossRef]
- Nabarro, F.R. Creep in commercially pure metals. Acta Mater. 2006, 54, 263–295. [Google Scholar] [CrossRef]
- Ma, Y.; Peng, G.J.; Wen, D.H.; Zhang, T.H. Nanoindentation creep behavior in a CoCrFeCuNi high-entropy alloy film with two different structure states. Mat. Sci. Eng. A 2015, 621, 111–117. [Google Scholar] [CrossRef]
- Ma, Y.; Feng, Y.H.; Debela, T.T.; Zhang, T.H. Nanoindentation study on the creep characteristics of high-entropy alloy films: Fcc versus bcc structures. Int. J. Refract. Met. H 2016, 54, 395–400. [Google Scholar] [CrossRef]
- Chen, H.; Zhang, T.H.; Ma, Y. Effect of Applied Stress on the Mechanical Properties of a Zr-Cu-Ag-Al Bulk Metallic Glass with Two Different Structure States. Materials 2017, 10, 711. [Google Scholar] [CrossRef] [PubMed]
- Zhang, T.H.; Ye, J.H.; Feng, Y.H.; Ma, Y. On the spherical nanoindentation creep of metallic glassy thin films at room temperature. Mat. Sci. Eng. A 2017, 685, 294–299. [Google Scholar] [CrossRef] [Green Version]
- Ma, Y.; Ye, J.H.; Peng, G.J.; Zhang, T.H. Loading rate effect on the creep behavior of metallic glassy films and its correlation with the shear transformation zone. Mat. Sci. Eng. A 2015, 622, 76–81. [Google Scholar] [CrossRef]
- Yoo, B.G.; Kim, J.Y.; Kim, Y.J.; Choi, I.C.; Shim, S.; Tsui, T.Y.; Jang, J.I. Increased time-dependent room temperature plasticity in metallic glass nanopillars and its size-dependency. Int. J. Plasticity 2012, 37, 108–118. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, J.; Wu, K.; Liu, G.; Kiener, D.; Sun, J. Nanoindentation creep behavior of Cu–Zr metallic glass films. Mater. Res. Lett 2018, 6, 22–28. [Google Scholar] [CrossRef]
- Ma, Y.; Peng, G.J.; Jiang, W.F.; Chen, H.; Zhang, T.H. Nanoindentation study on shear transformation zone in a Cu-Zr-Al metallic glassy film with different thickness. J. Non-Cryst. Solids 2016, 442, 67–72. [Google Scholar] [CrossRef]
- Ma, Y.; Ye, J.H.; Peng, G.J.; Zhang, T.H. Nanoindentation study of size effect on shear transformation zone size in a Ni–Nb metallic glass. Mat. Sci. Eng. A 2015, 627, 153–160. [Google Scholar] [CrossRef]
- Yoo, B.G.; Oh, J.H.; Kim, Y.J.; Park, K.W.; Lee, J.C.; Jang, J.I. Nanoindentation analysis of time-dependent deformation in as-cast and annealed Cu–Zr bulk metallic glass. Intermetallics 2010, 18, 1898–1901. [Google Scholar] [CrossRef]
- Wang, F.; Li, J.M.; Huang, P.; Wang, W.L.; Lu, T.J.; Xu, K.W. Nanoscale creep deformation in Zr-based metallic glass. Intermetallics 2013, 38, 156–160. [Google Scholar] [CrossRef]
- Ma, Y.; Peng, G.J.; Feng, Y.H.; Zhang, T.H. Nanoindentation investigation on the creep mechanism in metallic glassy films. Mat. Sci. Eng. A 2016, 651, 548–555. [Google Scholar] [CrossRef] [Green Version]
- Yoo, B.G.; Kim, K.S.; Oh, J.H.; Ramamurty, U.; Jang, J.I. Room temperature creep in amorphous alloys: Influence of initial strain and free volume. Scr. Mater. 2010, 63, 1205–1208. [Google Scholar] [CrossRef]
- Bei, H.; Lu, Z.P.; George, E.P. Theoretical strength and the onset of plasticity in bulk metallic glasses investigated by nanoindentation with a spherical indenter. Phys. Rev. Lett. 2004, 93, 125504. [Google Scholar] [CrossRef] [PubMed]
- Johnson, K.L. Contact Mechanics; Cambridge University Press: Cambridge, UK, 1987. [Google Scholar]
- Jiang, Q.K.; Wang, X.D.; Nie, X.P.; Zhang, G.Q.; Ma, H.; Fecht, H.-J.; Bendnarcik, J.; Franz, H.; Liu, Y.G.; Cao, Q.P.; et al. Zr–(Cu,Ag)–Al bulk metallic glasses. Acta Mater. 2008, 56, 1785–1796. [Google Scholar] [CrossRef]
- Packard, C.E.; Homer, E.R.; Al-Aqeeli, N.; Schuh, C.A. Cyclic hardening of metallic glasses under Hertzian contacts: Experiments and STZ dynamics simulations. Philos. Mag. 2010, 90, 1373–1390. [Google Scholar] [CrossRef]
- Packard, C.E.; Schuh, C.A. Initiation of shear bands near a stress concentration in metallic glass. Acta Mater. 2007, 55, 5348–5358. [Google Scholar] [CrossRef]
- Tabor, D. The hardness of solids. Rev. Phys. Technol. 1970, 1, 145–179. [Google Scholar] [CrossRef]
- Su, C.; Herbert, E.G.; Sohn, S.; LaManna, J.A.; Oliver, W.C.; Pharr, G.M. Measurement of power-law creep parameters by instrumented indentation methods. J. Mech. Phys. Solids 2012, 61, 517–536. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, Z.Y.; Song, Y.X.; Ma, Y.; Huang, X.W.; Zhang, T.H. Nanoindentation Investigation on the Size-Dependent Creep Behavior in a Zr-Cu-Ag-Al Bulk Metallic Glass. Metals 2019, 9, 613. https://doi.org/10.3390/met9050613
Ding ZY, Song YX, Ma Y, Huang XW, Zhang TH. Nanoindentation Investigation on the Size-Dependent Creep Behavior in a Zr-Cu-Ag-Al Bulk Metallic Glass. Metals. 2019; 9(5):613. https://doi.org/10.3390/met9050613
Chicago/Turabian StyleDing, Z. Y., Y. X. Song, Y. Ma, X. W. Huang, and T. H. Zhang. 2019. "Nanoindentation Investigation on the Size-Dependent Creep Behavior in a Zr-Cu-Ag-Al Bulk Metallic Glass" Metals 9, no. 5: 613. https://doi.org/10.3390/met9050613
APA StyleDing, Z. Y., Song, Y. X., Ma, Y., Huang, X. W., & Zhang, T. H. (2019). Nanoindentation Investigation on the Size-Dependent Creep Behavior in a Zr-Cu-Ag-Al Bulk Metallic Glass. Metals, 9(5), 613. https://doi.org/10.3390/met9050613




