Hydrogen Diffusion Mechanism around a Crack Tip in Type 304L Austenite Stainless Steel Considering the Influence of the Volume Expansion of Strain-Induced Martensite Transformation
Abstract
:1. Introduction
2. Investigation Procedure
2.1. Hydrogen Dependent Material Properties
2.2. Hydrogen Diffusion Analysis
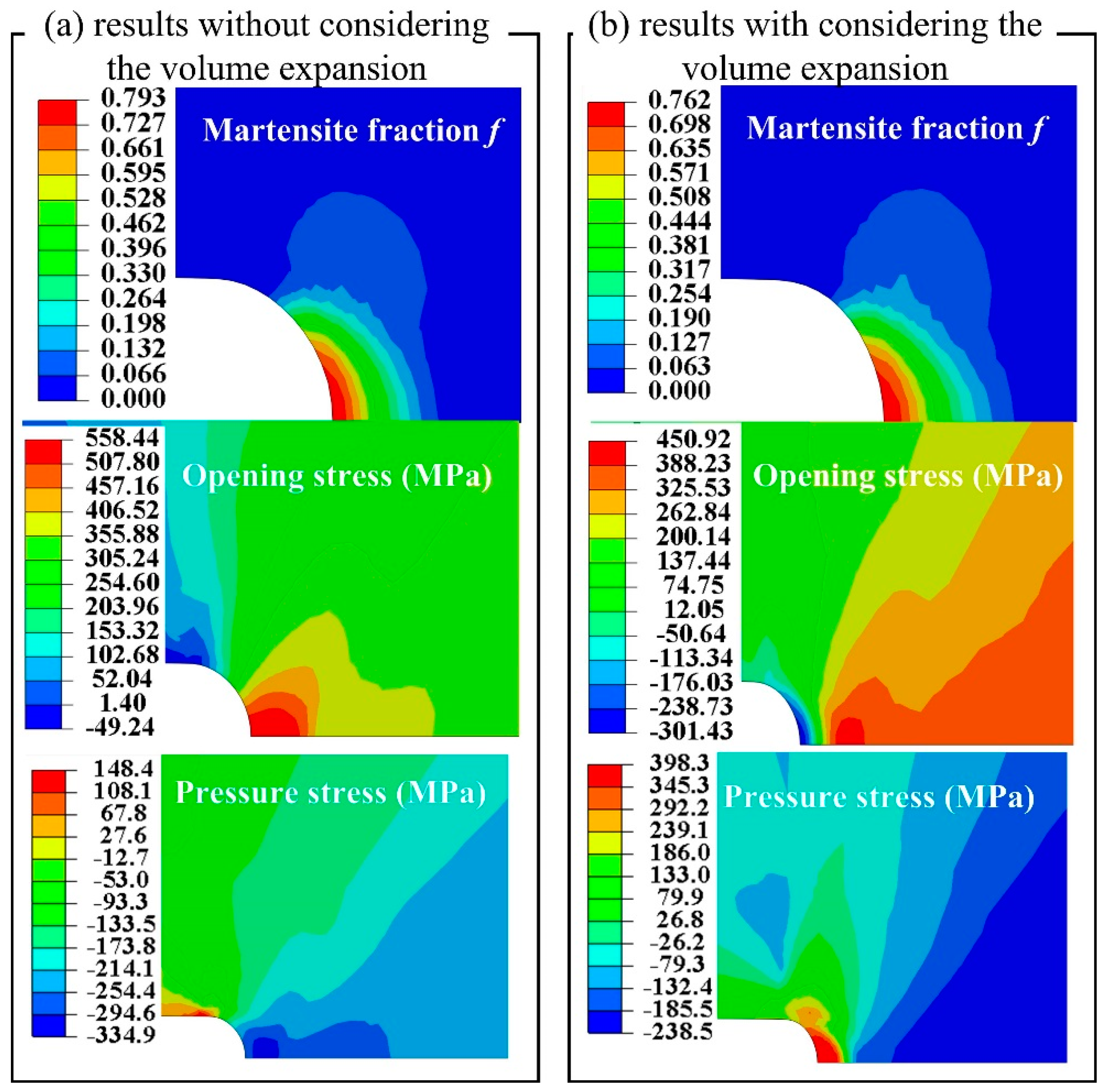
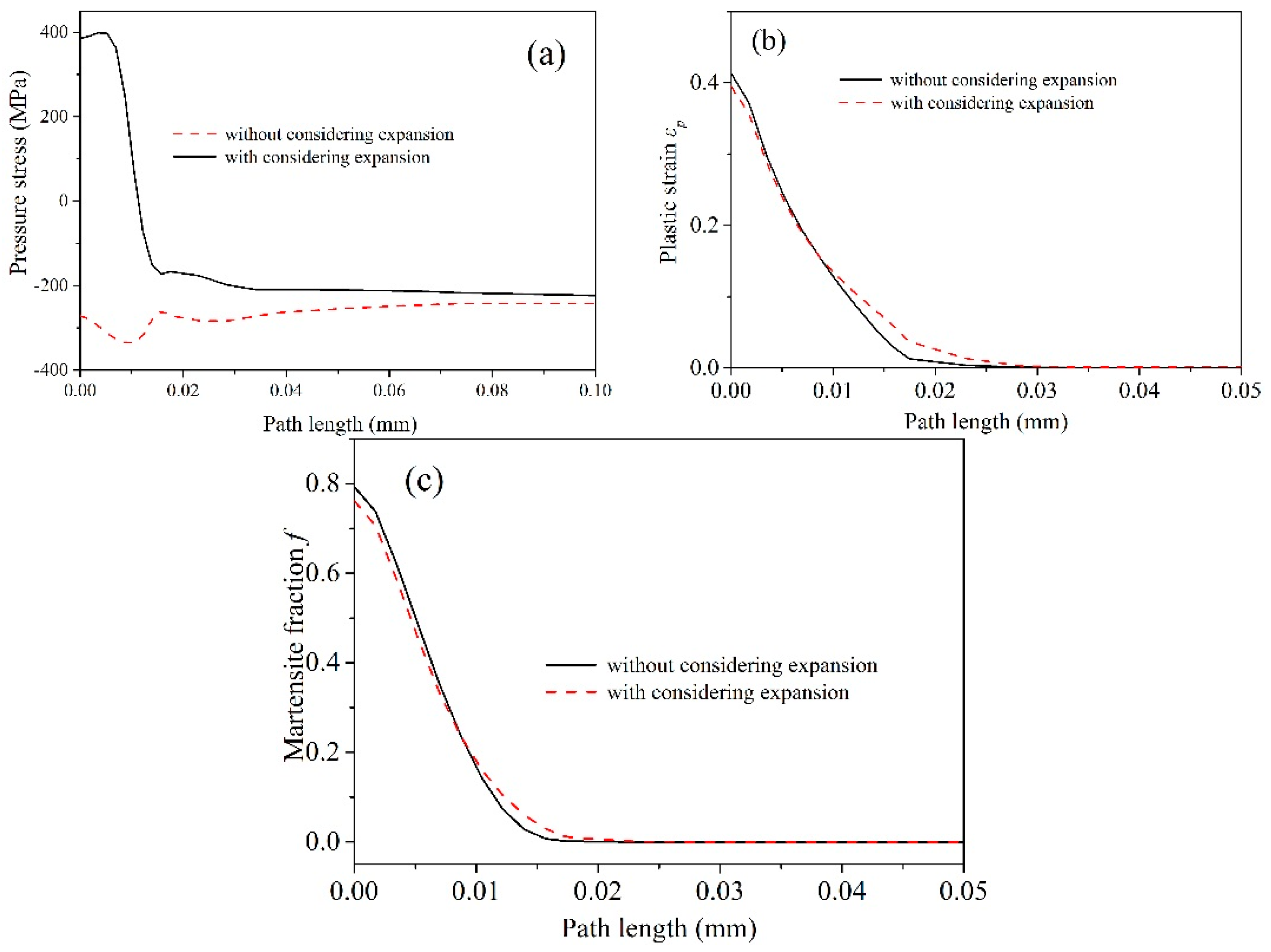
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Garcia, C.; Martin, F.; De Tiedra, P.; Alonso, S.; Aparicio, M.L. Stress corrosion cracking behavior of cold-worked and sensitized type 304 stainless steel using the slow strain rate test. Corrosion 2002, 58, 849–857. [Google Scholar] [CrossRef]
- Lai, C.L.; Tsay, L.W.; Kai, W.; Chen, C. Notched tensile tests of cold-rolled 304L stainless steel in 40 wt.% 80 °C MgCl2 solution. Corros. Sci. 2009, 51, 380–386. [Google Scholar] [CrossRef]
- Chen, T.C.; Chen, S.T.; Tsay, L.W. The role of induced α′-martensite on the hydrogen-assisted fatigue crack growth of austenitic stainless steels. Int. J. Hydrogen Energy 2014, 39, 10293–10302. [Google Scholar] [CrossRef]
- Ueki, S.; Mine, Y.; Takashima, K. Crystallographic study of hydrogen-induced twin boundary separation in type 304 stainless steel under cyclic loading. Corros. Sci. 2017, 129, 205–213. [Google Scholar] [CrossRef]
- Jackson, H.; San Marchi, C.; Balch, D.; Somerday, B.; Michael, J. Effects of low temperature on hydrogen-assisted crack growth in forged 304L austenitic stainless steel. Metall. Mater. Trans. A 2016, 47A, 4334–4350. [Google Scholar] [CrossRef]
- Zhang, L.; An, B.; Fukuyama, S.; Iijima, T.; Yokogawa, K. Characterization of hydrogen-induced crack initiation in metastable austenitic stainless steels during deformation. J. Appl. Phys. 2010, 108, 063526. [Google Scholar] [CrossRef]
- Chen, X.; Zhou, C.; Cai, X.; Zheng, J.; Zhang, L. Effects of external hydrogen on hydrogen transportation and distribution around the fatigue crack tip in type 304 stainless steel. J. Mater. Eng. Perform. 2017, 26, 4990–4996. [Google Scholar] [CrossRef]
- Sofronis, P.; McMeeking, R.M. Numerical analysis of hydrogen transport near a blunting crack tip. J. Mech. Phys. Solids 1989, 37, 317–350. [Google Scholar] [CrossRef]
- Toshimitsu, Y.J.A.; Takenao, N.; Koji, S.; Tetsuya, Y. Numerical analysis on hydrogen diffusion and concentration in solid with emission around the crack tip. Eng. Fract. Mech. 1996, 55, 47–60. [Google Scholar]
- Liang, Y.; Sofronis, P. Toward a phenomenological description of hydrogen-induced decohesion at particle/matrix interfaces. J. Mech. Phys. Solids 2003, 51, 1509–1531. [Google Scholar] [CrossRef]
- Takakuwa, O.; Nishikawa, M.; Soyama, H. Numerical simulation of the effects of residual stress on the concentration of hydrogen around a crack tip. Surf. Coat. Technol. 2012, 206, 2892–2898. [Google Scholar] [CrossRef]
- Martínez-Pañeda, E.; del Busto, S.; Niordson, C.F.; Betegón, C. Strain gradient plasticity modeling of hydrogen diffusion to the crack tip. Int. J. Hydrogen Energy 2016, 41, 10265–10274. [Google Scholar] [CrossRef] [Green Version]
- Mayer, H.R.; Stanzl-Tschegg, S.E.; Sawaki, Y.; Huhner, M.; Hornbogen, E. Influence of transformation-induced crack closure on slow fatigue crack growth under variable amplitude loading. Fatigue Fract. Engngy Mater. Struct. 1995, 19, 935–948. [Google Scholar] [CrossRef]
- Olden, V.; Saai, A.; Jemblie, L.; Johnsen, R. FE simulation of hydrogen diffusion in duplex stainless steel. Int. J. Hydrogen Energy 2014, 39, 1156–1163. [Google Scholar] [CrossRef]
- Wang, Y.; Li, X.; Dou, D.; Shen, L.; Gong, J. FE analysis of hydrogen diffusion around a crack tip in an austenitic stainless steel. Int. J. Hydrogen Energy 2016, 41, 6053–6063. [Google Scholar] [CrossRef]
- Kanezaki, T.; Narazaki, C.; Mine, Y.; Matsuoka, S.; Murakami, Y. Effects of hydrogen on fatigue crack growth behavior of austenitic stainless steels. Int. J. Hydrogen Energy 2008, 33, 2604–2619. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, X.; Gong, J.; Shen, L.; Dong, W. Hydrogen embrittlement of catholically hydrogen-precharged 304L austenitic stainless steel: Effect of plastic pre-strain. Int. J. Hydrogen Energy 2014, 39, 13909–13918. [Google Scholar] [CrossRef]
- Hallberg, H.; Banks-Sills, L.; Ristinmaa, M. Crack tip transformation zones in austenitic stainless steel. Eng. Fract. Mech. 2012, 79, 266–280. [Google Scholar] [CrossRef] [Green Version]
- Jiang, W.; Chen, W.; Woo, W.; Tu, S.-T.; Zhang, X.-C.; Em, V. Effects of low-temperature transformation and transformation-induced plasticity on weld residual stresses: Numerical study and neutron diffraction measurement. Mater. Design 2018, 147, 65–79. [Google Scholar] [CrossRef]
- Wang, Q.; Liu, X.S.; Wang, P.; Xiong, X.; Fang, H.Y. Numerical simulation of residual stress in 10Ni5CrMoV steel weldments. J. Mater. Process. Tech. 2017, 240, 77–86. [Google Scholar] [CrossRef]
- Zheng, W.J.; He, Y.M.; Yang, J.G.; Gao, Z.L. Hydrogen diffusion mechanism of the single-pass welded joint in welding considering the phase transformation effects. J. Manuf. Process. 2018, 36, 126–137. [Google Scholar] [CrossRef]
- Kim, Y.S.; Kim, S.S.; Choe, B.H. The role of hydrogen in hydrogen embrittlement of metals: the case of stainless steel. Metals 2019, 9, 406. [Google Scholar] [CrossRef]
- Martinez, B.A.; Laso, J.A.A.; Gutierrez-Solana, F.; Martinez, A.C.; Martinez, Y.J.J.; Aparicio, A.R.S. A proposal for the application of failure assessment diagrams to subcritical hydrogen induced cracking propagation processes. Metals 2019, 9, 670. [Google Scholar] [CrossRef]
- Zheng, W.J.; Liu, Y.; Gao, Z.L.; Yang, J.G. Just-in-time semi-supervised soft sensor for quality prediction in industrial rubber mixers. Chemom. Intell. Lab. Syst. 2018, 180, 36–41. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, C.; Gao, Z.; Yao, Y. Ensemble deep kernel learning with application to quality prediction in industrial polymerization processes. Chemom. Intell. Lab. Syst. 2018, 174, 15–21. [Google Scholar] [CrossRef]
- Xuan, Q.; Chen, Z.; Liu, Y.; Huang, H.; Bao, G.; Zhang, D. Multiview generative adversarial network and its application in pearl classification. IEEE Trans. Ind. Electron. 2019, 66, 8244–8252. [Google Scholar] [CrossRef]
- Zheng, W.; He, Y.; Yang, J.; Gao, Z. Influence of crystallographic orientation of epitaxial solidification on the initial instability during the solidification of welding pool. J. Manuf. Process. 2019, 38, 298–307. [Google Scholar] [CrossRef]
- Beldowski, P.; Mazurkiewicz, A.; Topolinski, T.; Malek, T. Hydrogen and water bonding between glycosaminoglycans and phospholipids in the synovial fluid: Molecular dynamics study. Materials 2019, 12, 2060. [Google Scholar] [CrossRef]
- Deng, D. FEM prediction of welding residual stress and distortion in carbon steel considering phase transformation effects. Mater. Design 2009, 30, 359–366. [Google Scholar] [CrossRef]
- Olden, V.; Alvaro, A.; Odd, M.A. Hydrogen diffusion and hydrogen influenced critical stress intensity in an API X70 pipeline steel welded joint—Experiments and FE simulations. Int. J. Hydrogen Energy 2012, 27, 11474–11486. [Google Scholar] [CrossRef]
- Alvaro, A.; Olden, V.; Akselsen, O.M. 3D cohesive modelling of hydrogen embrittlement in the heat affected zone of an X70 pipeline steel. Int. J. Hydrogen Energy 2013, 38, 7539–7549. [Google Scholar] [CrossRef]
- Yan, C.; Liu, C.; Yan, B. 3D modeling of the hydrogen distribution in X80 pipeline steel welded joints. Comp. Mater. Sci. 2014, 83, 158–163. [Google Scholar] [CrossRef]
- Toribio, J.; Kharin, V.; Vergara, D.; Lorenzo, M. Two-dimensional numerical modelling of hydrogen diffusion in metals assisted by both stress and strain. Adv. Mater. Res. 2010, 138, 117–126. [Google Scholar] [CrossRef]
- Serebrinsky, S.; Carter, E.A.; Ortiz, M. A quantum-mechanically informed continuum model of hydrogen embrittlement. J. Mech. Phys. Solids 2004, 52, 2403–2430. [Google Scholar] [CrossRef]
- Mine, Y.; Koga, K.; Kraft, O.; Takashima, K. Mechanical characterisation of hydrogen-induced quasi-cleavage in a metastable austenitic steel using micro-tensile testing. Scr. Mater. 2016, 113, 176–179. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiong, Z.; Zheng, W.; Liu, Y.; Kuang, Y.; Yang, J. Hydrogen Diffusion Mechanism around a Crack Tip in Type 304L Austenite Stainless Steel Considering the Influence of the Volume Expansion of Strain-Induced Martensite Transformation. Metals 2019, 9, 977. https://doi.org/10.3390/met9090977
Xiong Z, Zheng W, Liu Y, Kuang Y, Yang J. Hydrogen Diffusion Mechanism around a Crack Tip in Type 304L Austenite Stainless Steel Considering the Influence of the Volume Expansion of Strain-Induced Martensite Transformation. Metals. 2019; 9(9):977. https://doi.org/10.3390/met9090977
Chicago/Turabian StyleXiong, Zhiliang, Wenjian Zheng, Yanzhang Liu, Yanjun Kuang, and Jianguo Yang. 2019. "Hydrogen Diffusion Mechanism around a Crack Tip in Type 304L Austenite Stainless Steel Considering the Influence of the Volume Expansion of Strain-Induced Martensite Transformation" Metals 9, no. 9: 977. https://doi.org/10.3390/met9090977
APA StyleXiong, Z., Zheng, W., Liu, Y., Kuang, Y., & Yang, J. (2019). Hydrogen Diffusion Mechanism around a Crack Tip in Type 304L Austenite Stainless Steel Considering the Influence of the Volume Expansion of Strain-Induced Martensite Transformation. Metals, 9(9), 977. https://doi.org/10.3390/met9090977




