Advances and Challenges in Palaeoenvironmental Studies Based on Oxygen Isotope Composition of Skeletal Carbonates and Phosphates
Abstract
:1. Introduction
2. Diagenetic Alteration
2.1. Calcareous Fossils
| Mn (ppm) | Fe (ppm) | Sr (ppm) | |
|---|---|---|---|
| Anderson et al. [46] | <100 | <1000 | - |
| Jones et al. [47] | <50 | <150 | - |
| Wierzbowski and Joachimski [78], Wierzbowski [79] | <100 | <250 | >490 |
| Korte and Hesselbo [60] | <250 | - | >400 |
| Zuo et al. [69] | <100 | <700 | >600 |
| Danise et al. [80] | <250 | <250 | >350 |
2.2. Apatite Fossils
3. Temperature Equations
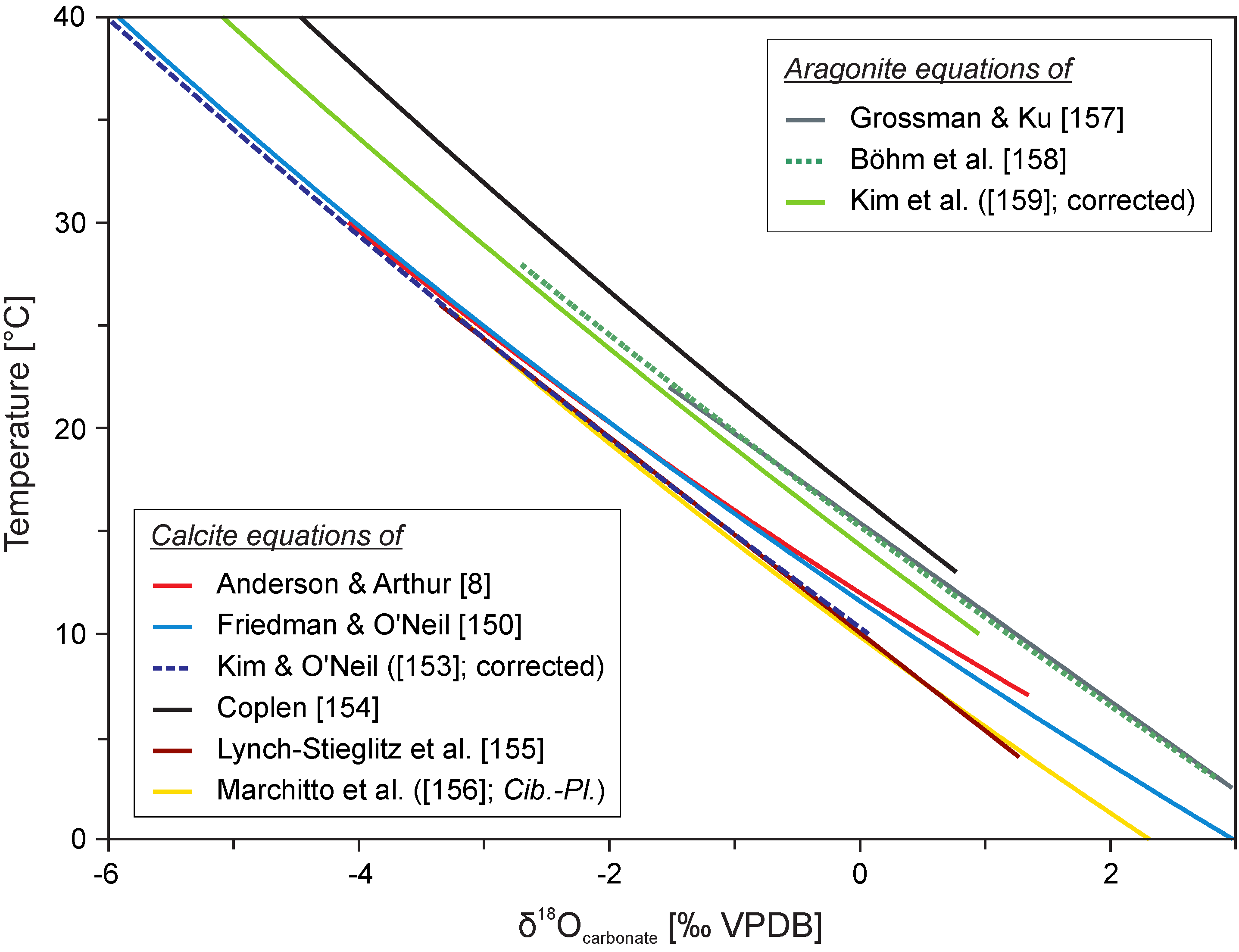
3.1. Calcium Carbonate
3.2. Calcium Phosphate
4. Vital and Habitat Effects
4.1. Calcareous Skeletons
4.2. Apatite Skeletons
5. Oxygen Isotope Composition of Seawater and Habitat Depth
6. Precise Sampling Methods
6.1. Microsampling Techniques
6.2. Ion Microprobe
7. Clumped Isotopes
8. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Urey, H.C. The thermodynamic properties of isotopic substances. J. Chem. Soc. 1947, 562–581. [Google Scholar] [CrossRef]
- McCrea, J.M. On the isotopic chemistry of carbonates and a paleotemperature scale. J. Chem. Phys. 1950, 18, 849–857. [Google Scholar] [CrossRef]
- Urey, H.C.; Lowenstam, H.A.; Epstein, S.; McKinney, C.R. Measurement of paleotemperatures and temperatures of the upper cretaceous of England, Denmark, and the southeastern United States. GSA Bull. 1951, 62, 399–416. [Google Scholar] [CrossRef]
- Epstein, S.; Buchsbaum, R.; Lowenstam, H.; Urey, H.C. Carbonate-water isotopic temperature scale. GSA Bull. 1951, 62, 417–425. [Google Scholar] [CrossRef]
- Epstein, S.; Buchsbaum, R.; Lowenstam, H.A.; Urey, H.C. Revised carbonate-water isotopic temperature scalE. GSA Bull. 1953, 64, 1315–1326. [Google Scholar] [CrossRef]
- Longinelli, A.; Nuti, S. Revised phosphate-water isotopic temperature scale. Earth Planet. Sci. Lett. 1973, 19, 373–376. [Google Scholar] [CrossRef]
- Kolodny, Y.; Luz, B.; Navon, O. Oxygen isotope variations in phosphate of biogenic apatites, I. Fish bone apatite—rechecking the rules of the game. Earth Planet. Sci. Lett. 1983, 64, 398–404. [Google Scholar] [CrossRef]
- Anderson, T.F.; Arthur, M.A. Stable isotopes of oxygen and carbon and their application to sedimentologic and paleoenvironmental problems. In Stable Isotopes in Sedimentary Geology: SEPM Short Course No. 10; Arthur, M.A., Anderson, T.F., Kaplan, I.R., Veizer, J., Land, L.S., Eds.; Society of Economic Paleontologists and Mineralogists: Tulsa, OK, USA, 1983; pp. 1-1–1-151. [Google Scholar]
- Veizer, J. Chemical diagenesis of carbonates: Theory and trace element technique. In Stable Isotopes in Sedimentary Geology, SEPM Short Course No. 10; Arthur, M.A., Anderson, T.F., Kaplan, I.R., Veizer, J., Land, L.S., Eds.; Society of Economic Paleontologists and Mineralogists: Tulsa, OK, USA, 1983; pp. 3-1–3-100. [Google Scholar]
- Marshall, J.D. Climatic and oceanographic isotopic signals from the carbonate rock record and their preservation. Geol. Mag. 1992, 129, 143–160. [Google Scholar] [CrossRef]
- Kolodny, Y.; Luz, B. Isotope signatures in phosphate deposits: Formation and diagenetic history. In Isotopic Signatures and Sedimentary Records, Lectures Notes in Earth Sciences 43; Clauer, N., Chaudhuri, S., Eds.; Springer: Berlin/Heidelberg, Germany, 1992; pp. 69–121. [Google Scholar]
- Veizer, J. Depositional and diagenetic history of limestones: Stable and radiogenic isotopes. In Isotopic Signatures and Sedimentary Records, Lectures Notes in Earth Sciences 43; Clauer, N., Chaudhuri, S., Eds.; Springer: Berlin/Heidelberg, Germany, 1992; pp. 13–48. [Google Scholar] [CrossRef]
- Kohn, M.J.; Cerling, T.E. Stable isotope composition of biological apatite. In Phosphates. Reviews in Mineralogy & Geochemistry; Kohn, M.J., Rakovan, J., Hughes, J.M., Eds.; Mineralogical Society of America, Geochemical Society: Chantilly, VA, USA, 2002; Volume 48, pp. 455–488. [Google Scholar]
- Maslin, M.A.; Swann, G.E.A. Isotopes in marine sediments. In Isotopes in Palaeoenvironmental Research, Developments in Paleoenvironmental Research; Leng, M.J., Ed.; Springer: Berlin/Heidelberg, Germany, 2006; Volume 10, pp. 227–290. [Google Scholar] [CrossRef]
- Sharp, Z. Principles of Stable Isotope Geochemistry; Pearson Prentice Hall: Hoboken, NJ, USA, 2007; pp. 1–344. [Google Scholar]
- Grossman, E.L. Applying oxygen isotope paleothermometry in deep time. In Reconstructing Earth’s Deep-Time Climate–The State of the Art in 2012, Paleontological Society Short Course, November 3, 2012; Ivany, L.T., Huber, B.T., Eds.; The Paleontological Society: McLean, VA, USA, 2012; Volume 18, pp. 39–68. [Google Scholar]
- Grossman, E.L. Oxygen isotope stratigraphy. In The Geologic Time Scale; Gradstein, F.M., Ogg, J.G., Schmitz, M., Ogg, G., Eds.; Elsevier: Amsterdam, The Netherlands, 2012; pp. 181–206. [Google Scholar]
- Pearson, P.N. Oxygen isotopes in foraminifera: Overview and historical review. In Reconstructing Earth’s Deep-Time Climate—The State of the Art in 2012. Paleontological Society Short Course November 3, 2012. The Paleontological Society Papers; Ivany, L.C., Huber, B.T., Eds.; The Paleontological Society: McLean, VA, USA, 2012; Volume 18, pp. 1–38. [Google Scholar]
- Lea, D.W. Elemental and isotopic proxies of past ocean temperatures. In Treatise on Geochemistry, 1st ed.; Holland, H.D., Turekian, K.K., Eds.; Elsevier: Oxford, UK, 2003; Volume 6, pp. 391–432. [Google Scholar]
- Lea, D.W. Elemental and isotopic proxies of past ocean temperatures. In Treatise on Geochemistry, 2nd ed.; Holland, H.D., Turekian, K.K., Eds.; Elsevier: Oxford, UK, 2014; Volume 8, pp. 373–397. [Google Scholar]
- Immenhauser, A.; Schöne, B.R.; Hoffmann, R.; Niedermayr, A. Mollusc and brachiopod skeletal hard parts: Intricate archives of their marine environment. Sedimentology 2015, 63, 1–59. [Google Scholar] [CrossRef] [Green Version]
- Banner, J.L.; Hanson, G.N. Calculation of simultaneous isotopic and trace element variations during water-rock interaction with applications to carbonate diagenesis. Geochim. Cosmochim. Acta 1990, 54, 3123–3137. [Google Scholar] [CrossRef]
- Blake, R.; O’Neil, J.; Garcia, G. Oxygen isotope systematics of biologically mediated reactions of phosphate: I. Microbial degradation of organophosphorus compounds. Geochim. Cosmochim. Acta 1997, 61, 4411–4422. [Google Scholar] [CrossRef]
- Sharp, Z.D. The effect of diagenesis on oxygen isotope ratios of biogenic phosphates. Am. J. Sci. 2000, 300, 222–237. [Google Scholar] [CrossRef]
- Zazzo, A.; Lecuyer, C.; Mariotti, A. Experimentally-controlled carbon and oxygen isotope exchange between bioapatites and water under inorganic and microbially-mediated conditions. Geochim. Cosmochim. Acta 2004, 68, 1–12. [Google Scholar] [CrossRef]
- Veizer, J.; Ala, D.; Azmy, K.; Bruckschen, P.; Buhl, D.; Bruhn, F.; Carden, G.A.; Diener, A.; Ebneth, S.; Godderis, Y.; et al. 87Sr/86Sr, δ13C and δ18O evolution of Phanerozoic seawater. Chem. Geol. 1999, 161, 59–88. [Google Scholar] [CrossRef] [Green Version]
- Jaffrés, J.B.; Shields, G.; Wallmann, K. The oxygen isotope evolution of seawater: A critical review of a long-standing controversy and an improved geological water cycle model for the past 3.4 billion years. Earth Sci. Rev. 2007, 83, 83–122. [Google Scholar] [CrossRef] [Green Version]
- Prokoph, A.; Shields, G.; Veizer, J. Compilation and time-series analysis of a marine carbonate δ18O, δ13C, 87Sr/86Sr and δ34S database through Earth history. Earth-Sci. Rev. 2008, 87, 113–133. [Google Scholar] [CrossRef]
- Goldberg, S.L.; Present, T.M.; Finnegan, S.; Bergmann, K.D. A high-resolution record of early Paleozoic climate. Proc. Natl. Acad. Sci. USA 2021, 118, 2013083118. [Google Scholar] [CrossRef]
- Veizer, J.; Prokoph, A. Temperatures and oxygen isotopic composition of Phanerozoic oceans. Earth-Sci. Rev. 2015, 146, 92–104. [Google Scholar] [CrossRef]
- Galili, N.; Shemesh, A.; Yam, R.; Brailovsky, I.; Sela-Adler, M.; Schuster, E.M.; Collom, C.; Bekker, A.; Planavsky, N.; Macdonald, F.A.; et al. The geologic history of seawater oxygen isotopes from marine iron oxides. Science 2019, 365, 469–473. [Google Scholar] [CrossRef]
- Henkes, G.A.; Passey, B.H.; Grossman, E.; Shenton, B.J.; Yancey, T.E.; Pérez-Huerta, A. Temperature evolution and the oxygen isotope composition of Phanerozoic oceans from carbonate clumped isotope thermometry. Earth Planet. Sci. Lett. 2018, 490, 40–50. [Google Scholar] [CrossRef]
- Hodel, F.; Macouin, M.; Trindade, R.; Triantafyllou, A.; Ganne, J.; Chavagnac, V.; Berger, J.; Rospabé, M.; Destrigneville, C.; Carlut, J.; et al. Fossil black smoker yields oxygen isotopic composition of Neoproterozoic seawater. Nat. Commun. 2018, 9, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Veizer, J. Chemical diagenesis of belemnite shells and possible consequences for paleotemperature determinations. Neues Jahrb. Geol. P-A. 1974, 147, 91–111. [Google Scholar]
- Brand, U.; Veizer, J. Chemical diagenesis of a multicomponent carbonate system--1: Trace Elements. J. Sediment. Res. 1980, 50, 1219–1236. [Google Scholar] [CrossRef]
- Ullmann, C.V.; Korte, C. Diagenetic alteration in low-Mg calcite from macrofossils: A review. Geol. Q. 2015, 58, 3–20. [Google Scholar] [CrossRef] [Green Version]
- Ullmann, C.V.; Hesselbo, S.P.; Korte, C. Tectonic forcing of Early to Middle Jurassic seawater Sr/Ca. Geology 2013, 41, 1211–1214. [Google Scholar] [CrossRef] [Green Version]
- Savard, M.M.; Veizer, J.; Hinton, R. Cathodoluminescene at low Fe and Mn concentrations; a SIMS study of zones in natural calcites. J. Sediment. Res. 1995, 65, 208–213. [Google Scholar] [CrossRef]
- Dickson, J.A.D. Carbonate identification and genesis as revealed by staining. J. Sediment. Res. 1966, 36, 491–505. [Google Scholar] [CrossRef]
- Nunn, E.V.; Price, G.D. Late Jurassic (Kimmeridgian–Tithonian) stable isotopes (δ18O, δ13C) and Mg/Ca ratios: New palaeoclimate data from Helmsdale, northeast Scotland. Palaeogeogr. Palaeoclim. Palaeoecol. 2010, 292, 325–335. [Google Scholar] [CrossRef]
- Wierzbowski, H.; Błażejowski, B.; Tyborowski, D. Oxygen isotope profiles of uppermost jurassic vertebrate teeth and oyster shells: A record of paleoenvironmental changes and animal habitats. Palaios 2019, 34, 585–599. [Google Scholar] [CrossRef]
- Barbin, V. Cathodoluminescence of carbonate shells: Biochemical vs diagenetic process. In Cathodoluminescence in Geosciences; Pagel, M., Barbin, V., Blanc, P., Ohnenstetter, D., Eds.; Springer: Berlin/Heidelberg, Germany, 2000; pp. 303–329. [Google Scholar] [CrossRef]
- Barbin, V. Application of cathodoluminescence microscopy to recent and past biological materials: A decade of progress. Miner. Pet. 2013, 107, 353–362. [Google Scholar] [CrossRef]
- Wendler, J.E.; Wendler, I.; Rose, T.; Huber, B.T. Using cathodoluminescence spectroscopy of Cretaceous calcareous microfossils to distinguish biogenic from early-diagenetic calcite. Microsc. Microanal. 2012, 18, 1313–1321. [Google Scholar] [CrossRef] [PubMed]
- Barbin, V. Application of cathodoluminescence to carbonate diagenesis. In Cathodoluminescence in Geosciences; Pagel, M., Barbin, V., Blanc, P., Ohnenstetter, D., Eds.; Springer: Berlin/Heidelberg, Germany, 2000; pp. 271–301. [Google Scholar] [CrossRef]
- Anderson, T.F.; Popp, B.; Williams, A.C.; Ho, L.-Z.; Hudson, J.D. The stable isotopic records of fossils from the Peterborough Member, Oxford Clay Formation (Jurassic), UK: Palaeoenvironmental implications. J. Geol. Soc. 1994, 151, 125–138. [Google Scholar] [CrossRef]
- Jones, C.E.; Jenkyns, H.; Coe, A.; Stephen, H.P. Strontium isotopic variations in Jurassic and Cretaceous seawater. Geochim. Cosmochim. Acta 1994, 58, 3061–3074. [Google Scholar] [CrossRef]
- Price, G.; Ruffell, A.H.; Jones, C.E.; Kalin, R.M.; Mutterlose, J. Isotopic evidence for temperature variation during the early Cretaceous (late Ryazanian–mid-Hauterivian). J. Geol. Soc. 2000, 157, 335–343. [Google Scholar] [CrossRef] [Green Version]
- Rosales, I.; Quesada, S.; Robles, S. Primary and diagenetic isotopic signals in fossils and hemipelagic carbonates: The Lower Jurassic of northern Spain. Sedimentology 2001, 48, 1149–1169. [Google Scholar] [CrossRef]
- Gröcke, D.R.; Price, G.D.; Ruffell, A.H.; Mutterlose, J.; Baraboshkin, E. Isotopic evidence for Late Jurassic–Early Cretaceous climate change. Palaeogeogr. Palaeoclim. Palaeoecol. 2003, 202, 97–118. [Google Scholar] [CrossRef]
- Rosales, I.; Quesada, S.; Robles, S. Paleotemperature variations of Early Jurassic seawater recorded in geochemical trends of belemnites from the Basque–Cantabrian basin, northern Spain. Palaeogeogr. Palaeoclim. Palaeoecol. 2004, 203, 253–275. [Google Scholar] [CrossRef]
- Voigt, S.; Wilmsen, M.; Mortimore, R.N.; Voigt, T. Cenomanian palaeotemperatures derived from the oxygen isotopic composition of brachiopods and belemnites: Evaluation of Cretaceous palaeotemperature proxies. Acta Diabetol. 2003, 92, 285–299. [Google Scholar] [CrossRef]
- Price, G.; Mutterlose, J. Isotopic signals from late Jurassic–early Cretaceous (Volgian–Valanginian) sub-Arctic belemnites, Yatria River, Western Siberia. J. Geol. Soc. 2004, 161, 959–968. [Google Scholar] [CrossRef]
- Price, G.D.; Rogov, M.A. An isotopic appraisal of the Late Jurassic greenhouse phase in the Russian Platform. Palaeogeogr. Palaeoclim. Palaeoecol. 2009, 273, 41–49. [Google Scholar] [CrossRef]
- Wierzbowski, H.; Rogov, M.A.; Matyja, B.A.; Kiselev, D.; Ippolitov, A. Middle–Upper Jurassic (Upper Callovian–Lower Kimmeridgian) stable isotope and elemental records of the Russian Platform: Indices of oceanographic and climatic changes. Glob. Planet. Chang. 2013, 107, 196–212. [Google Scholar] [CrossRef]
- Alberti, M.; Fürsich, F.T.; Pandey, D.K. The Oxfordian stable isotope record (δ18O, δ13C) of belemnites, brachiopods, and oysters from the Kachchh Basin (western India) and its potential for palaeoecologic, palaeoclimatic, and palaeogeographic reconstructions. Palaeogeogr. Palaeoclim. Palaeoecol. 2012, 344–345, 49–68. [Google Scholar] [CrossRef]
- Alberti, M.; Fürsich, F.T.; Pandey, D.K.; Ramkumar, M. Stable isotope analyses of belemnites from the Kachchh Basin, western India: Paleoclimatic implications for the Middle to Late Jurassic transition. Facies 2011, 58, 261–278. [Google Scholar] [CrossRef]
- Wierzbowski, H. Seawater temperatures and carbon isotope variations in central European basins at the Middle–Late Jurassic transition (Late Callovian–Early Kimmeridgian). Palaeogeogr. Palaeoclim. Palaeoecol. 2015, 440, 506–523. [Google Scholar] [CrossRef]
- Arabas, A. Middle–Upper Jurassic stable isotope records and seawater temperature variations: New palaeoclimate data from marine carbonate and belemnite rostra (Pieniny Klippen Belt, Carpathians). Palaeogeogr. Palaeoclim. Palaeoecol. 2016, 446, 284–294. [Google Scholar] [CrossRef]
- Korte, C.; Hesselbo, S.P. Shallow marine carbon and oxygen isotope and elemental records indicate icehouse-greenhouse cycles during the Early Jurassic. Paleoceanography 2011, 26, 4219. [Google Scholar] [CrossRef]
- Žák, K.; Košťák, M.; Man, O.; Zakharov, V.; Rogov, M.; Pruner, P.; Rohovec, J.; Dzyuba, O.; Mazuch, M. Comparison of carbonate C and O stable isotope records across the Jurassic/Cretaceous boundary in the Tethyan and Boreal Realms. Palaeogeogr. Palaeoclim. Palaeoecol. 2011, 299, 83–96. [Google Scholar] [CrossRef]
- Joachimski, M.M.; van Geldern, R.; Breisig, S.; Buggisch, W.; Day, J. Oxygen isotope evolution of biogenic calcite and apatite during the Middle and Late Devonian. Acta Diabetol. 2004, 93, 542–553. [Google Scholar] [CrossRef]
- Van Geldern, R.; Joachimski, M.; Day, J.; Jansen, U.; Alvarez, F.; Yolkin, E.; Ma, X.-P. Carbon, oxygen and strontium isotope records of Devonian brachiopod shell calcite. Palaeogeogr. Palaeoclim. Palaeoecol. 2006, 240, 47–67. [Google Scholar] [CrossRef]
- Korte, C.; Jasper, T.; Kozur, H.W.; Veizer, J. δ18O and δ13C of Permian brachiopods: A record of seawater evolution and continental glaciation. Palaeogeogr. Palaeoclim. Palaeoecol. 2005, 224, 333–351. [Google Scholar] [CrossRef]
- Korte, C.; Jones, P.J.; Brand, U.; Mertmann, D.; Veizer, J. Oxygen isotope values from high-latitudes: Clues for Permian sea-surface temperature gradients and Late Palaeozoic deglaciation. Palaeogeogr. Palaeoclim. Palaeoecol. 2008, 269, 1–16. [Google Scholar] [CrossRef]
- Armendáriz, M.; Rosales, I.; Quesada, C. Oxygen isotope and Mg/Ca composition of Late Viséan (Mississippian) brachiopod shells from SW Iberia: Palaeoclimatic and palaeogeographic implications in northern Gondwana. Palaeogeogr. Palaeoclim. Palaeoecol. 2008, 268, 65–79. [Google Scholar] [CrossRef]
- Bruckschen, P.; Veizer, J. Oxygen and carbon isotopic composition of Dinantian brachiopods: Paleoenvironmental implications for the Lower Carboniferous of western Europe. Palaeogeogr. Palaeoclim. Palaeoecol. 1997, 132, 243–264. [Google Scholar] [CrossRef]
- Bruckschen, P.; Oesmann, S.; Veizer, J. Isotope stratigraphy of the European Carboniferous: Proxy signals for ocean chemistry, climate and tectonics. Chem. Geol. 1999, 161, 127–163. [Google Scholar] [CrossRef]
- Zuo, F.; Heimhofer, U.; Huck, S.; Adatte, T.; Erbacher, J.; Bodin, S. Climatic fluctuations and seasonality during the Kimmeridgian (Late Jurassic): Stable isotope and clay mineralogical data from the Lower Saxony Basin, Northern Germany. Palaeogeogr. Palaeoclim. Palaeoecol. 2018, 517, 1–15. [Google Scholar] [CrossRef]
- Angiolini, L.; Jadoul, F.; Leng, M.J.; Stephenson, M.H.; Rushton, J.; Chenery, S.; Crippa, G. How cold were the Early Permian glacial tropics? Testing sea-surface temperature using the oxygen isotope composition of rigorously screened brachiopod shells. J. Geol. Soc. 2009, 166, 933–945. [Google Scholar] [CrossRef]
- Morrison, J.O.; Brand, U. Geochemistry of recent marine invertebrates. Geosci. Can. 1986, 13, 237–254. [Google Scholar]
- Brand, U.; Logan, A.; Hiller, N.; Richardson, J. Geochemistry of modern brachiopods: Applications and implications for oceanography and paleoceanography. Chem. Geol. 2003, 198, 305–334. [Google Scholar] [CrossRef]
- Morse, J.W.; Mackenzie, F.T. Geochemistry of Sedimentary Carbonates. Developments in Sedimentology; Elsevier: Amsterdam, The Netherlands, 1990; Volume 48, 418p. [Google Scholar]
- Grossman, E. Chemical variation in Pennsylvanian brachiopod shells—diagenetic, taxonomic, microstructural, and seasonal effects. J. Sediment. Res. 1996, 66, 1011–1022. [Google Scholar] [CrossRef]
- Mii, H.S.; Grossman, E.L.; Yancey, T.E. Carboniferous isotope stratigraphies of North America: Implications for Car-boniferous paleoceanography and Mississippian glaciation. Geol. Soc. Am. Bull. 1999, 111, 960–973. [Google Scholar] [CrossRef]
- Bomou, B.; Deconinck, J.-F.; Pucéat, E.; Amédro, F.; Joachimski, M.M.; Quillévéré, F. Isotopic seawater temperatures in the Albian Gault Clay of the Boulonnais (Paris Basin): Palaeoenvironmental implications. Proc. Geol. Assoc. 2016, 127, 699–711. [Google Scholar] [CrossRef]
- Dubicka, Z.; Wierzbowski, H.; Wierny, W. Oxygen and carbon isotope records of Upper Cretaceous foraminifera from Poland: Vital and microhabitat effects. Palaeogeogr. Palaeoclim. Palaeoecol. 2018, 500, 33–51. [Google Scholar] [CrossRef]
- Wierzbowski, H.; Joachimski, M. Reconstruction of late Bajocian–Bathonian marine palaeoenvironments using carbon and oxygen isotope ratios of calcareous fossils from the Polish Jura Chain (central Poland). Palaeogeogr. Palaeoclim. Palaeoecol. 2007, 254, 523–540. [Google Scholar] [CrossRef]
- Wierzbowski, H. Palaeoenvironmental changes recorded in the oxygen and carbon isotope composition of Kimmeridgian (Upper Jurassic) carbonates from central Poland. Geol. Q. 2019, 63, 359. [Google Scholar] [CrossRef] [Green Version]
- Danise, S.; Price, G.D.; Alberti, M.; Holland, S.M. Isotopic evidence for partial geochemical decoupling between a Jurassic epicontinental sea and the open ocean. Gondwana Res. 2020, 82, 97–107. [Google Scholar] [CrossRef]
- Sexton, P.F.; Wilson, P.A.; Pearson, P. Microstructural and geochemical perspectives on planktic foraminiferal preservation: “Glassy” versus “Frosty”. Geochem. Geophys. Geosyst. 2006, 7, 12–19. [Google Scholar] [CrossRef]
- Bice, K.L.; Huber, B.T.; Norris, R.D. Extreme polar warmth during the Cretaceous greenhouse? Paradox of the late Turonian δ18O record at Deep Sea Drilling Project Site 511. Paleoceanography 2003, 18, 1031. [Google Scholar] [CrossRef] [Green Version]
- Isaza-Londoño, C.; MacLeod, K.G.; Huber, B.T. Maastrichtian North Atlantic warming, increasing stratification, and foraminiferal paleobiology at three timescales. Paleoceanography 2006, 21, 1012. [Google Scholar] [CrossRef] [Green Version]
- D’Haenens, S.; Bornemann, A.; Roose, K.; Claeys, P.; Speijer, R.P. Stable isotope paleoecology (δ13C and δ18O) of Early Eocene Zeauvigerina aegyptiaca from the North Atlantic (DSDP site 401). Austrian J. Earth Sci. 2012, 105, 179–188. [Google Scholar]
- Wendler, I.; Huber, B.T.; MacLeod, K.G.; Wendler, J.E. Stable oxygen and carbon isotope systematics of exquisitely preserved Turonian foraminifera from Tanzania—Understanding isotopic signatures in fossils. Mar. Micropaleontol. 2013, 102, 1–33. [Google Scholar] [CrossRef]
- Reolid, M. Stable isotopes on foraminifera and ostracods for interpreting incidence of the Toarcian Oceanic Anoxic Event in Westernmost Tethys: Role of water stagnation and productivity. Palaeogeogr. Palaeoclim. Palaeoecol. 2014, 395, 77–91. [Google Scholar] [CrossRef]
- Falzoni, F.; Petrizzo, M.R.; Clarke, L.J.; MacLeod, K.G.; Jenkyns, H.C. Long-term Late Cretaceous oxygen- and carbon-isotope trends and planktonic foraminiferal turnover: A new record from the southern midlatitudes. GSA Bull. 2016, 128, 1725–1735. [Google Scholar] [CrossRef]
- Kozdon, R.; Kelly, C.; Kita, N.T.; Fournelle, J.; Valley, J. Planktonic foraminiferal oxygen isotope analysis by ion microprobe technique suggests warm tropical sea surface temperatures during the Early Paleogene. Paleoceanography 2011, 26, 3206. [Google Scholar] [CrossRef] [Green Version]
- Schrag, D.P. Effects of diagenesis on the isotopic record of late paleogene tropical sea surface temperatures. Chem. Geol. 1999, 161, 215–224. [Google Scholar] [CrossRef]
- Pearson, P.N.; Ditchfield, P.W.; Singano, J.; Harcourt-Brown, K.G.; Nicholas, C.J.; Olsson, R.K.; Shackleton, N.J.; Hall, M.A. Warm tropical sea surface temperatures in the Late Cretaceous and Eocene epochs. Nature 2001, 413, 481–487. [Google Scholar] [CrossRef] [PubMed]
- Pearson, P.; van Dongen, B.; Nicholas, C.J.; Pancost, R.; Schouten, S.; Singano, J.M.; Wade, B. Stable warm tropical climate through the Eocene Epoch. Geology 2007, 35, 211. [Google Scholar] [CrossRef]
- Ando, A.; Huber, B.T.; MacLeod, K.G.; Watkins, D.K. Early Cenomanian “hot greenhouse” revealed by oxygen isotope record of exceptionally well-preserved foraminifera from Tanzania. Paleoceanography 2015, 30, 1556–1572. [Google Scholar] [CrossRef]
- MacLeod, K.G.; Huber, B.T.; Berrocoso, Á.J.; Wendler, I. A stable and hot Turonian without glacial δ18O excursions is indicated by exquisitely preserved Tanzanian foraminifera. Geology 2013, 41, 1083–1086. [Google Scholar] [CrossRef]
- Bernard, S.; Daval, D.; Ackerer, P.; Pont, S.; Meibom, A. Burial-induced oxygen-isotope re-equilibration of fossil foraminifera explains ocean paleotemperature paradoxes. Nat. Commun. 2017, 8, 1134. [Google Scholar] [CrossRef] [Green Version]
- Bernard, S.; Daval, D.; Ackerer, P.; Pont, S.; Meibom, A. Reply to ’No substantial long-term bias in the Cenozoic benthic foraminifera oxygen-isotope record’. Nat. Commun. 2018, 9, 2874. [Google Scholar] [CrossRef]
- Evans, D.; Badger, M.P.S.; Foster, G.L.; Henehan, M.J.; Lear, C.H.; Zachos, J.C. No substantial long-term bias in the Cenozoic benthic foraminifera oxygen-isotope record. Nat. Commun. 2018, 9, 2875. [Google Scholar] [CrossRef] [PubMed]
- Stahl, W.; Jordan, R. General considerations on isotopic paleotemperature determinations and analyses on Jurassic ammonites. Earth Planet. Sci. Lett. 1969, 6, 173–178. [Google Scholar] [CrossRef]
- Forester, R.W.; Caldwell, W.G.E.; Oro, F.H. Oxygen and carbon isotopic study of ammonites from the Late Cretaceous Bearpaw Formation in southwestern Saskatchewan. Can. J. Earth Sci. 1977, 14, 2086–2100. [Google Scholar] [CrossRef]
- Buchardt, B.; Weiner, S. Diagenesis of aragonite from Upper Cretaceous ammonites: A geochemical case-study. Sedimentology 1981, 28, 423–438. [Google Scholar] [CrossRef]
- Dauphin, Y.; Denis, A. Analyse microstructurale des tests de mollusques du Callovien de Lukow (Pologne)—Com-paraison de l’etat de conservation de quelques types structuraux majeurs. Rev. Paléobiol. 1990, 9, 27–36. [Google Scholar]
- Dauphin, Y.; Denis, A. Diagenèse comparée des phases minérales et organiques solubles dans les tests aragonitiques de nautiles et d’ammonites. Bull. Soc. Géol. Fr. 1999, 170, 355–365. [Google Scholar]
- Cochran, J.K.; Kallenberg, K.; Landman, N.H.; Harries, P.; Weinreb, D.; Turekian, K.K.; Beck, A.J.; Cobban, W.A. Effect of diagenesis on the Sr, O, and C isotope composition of late Cretaceous mollusks from the Western Interior Seaway of North America. Am. J. Sci. 2010, 310, 69–88. [Google Scholar] [CrossRef]
- Knoll, K.; Landman, N.H.; Cochran, J.K.; MacLeod, K.; Sessa, J.A. Microstructural preservation and the effects of diagenesis on the carbon and oxygen isotope composition of Late Cretaceous aragonitic mollusks from the Gulf Coastal Plain and the Western Interior Seaway. Am. J. Sci. 2016, 316, 591–613. [Google Scholar] [CrossRef]
- Gothmann, A.M.; Stolarski, J.; Adkins, J.F.; Schoene, B.; Dennis, K.J.; Schrag, D.P.; Mazur, M.; Bender, M.L. Fossil corals as an archive of secular variations in seawater chemistry since the Mesozoic. Geochim. Cosmochim. Acta 2015, 160, 188–208. [Google Scholar] [CrossRef]
- Dubicka, Z.; Wierzbowski, H.; Pałczyńska, A. Can oxygen and carbon isotope ratios of Jurassic foraminifera be used in palaeoenvironmental reconstructions? Palaeogeogr. Palaeoclim. Palaeoecol. 2021, 577, 110554. [Google Scholar] [CrossRef]
- Samtleben, C.; Munnecke, A.; Bickert, T.; Pätzold, J. Shell succession, assemblage and species dependent effects on the C/O-isotopic composition of brachiopods—Examples from the Silurian of Gotland. Chem. Geol. 2001, 175, 61–107. [Google Scholar] [CrossRef]
- Fujioka, H.; Takayanagi, H.; Yamamoto, K.; Iryu, Y. The effects of meteoric diagenesis on the geochemical composition and microstructure of Pliocene fossil Terebratalia coreanica and Laqueus rubellus brachiopod shells from northeastern Japan. Prog. Earth Planet. Sci. 2019, 6, 45. [Google Scholar] [CrossRef]
- Wierzbowski, H. Detailed oxygen and carbon isotope stratigraphy of the Oxfordian in Central Poland. Acta Diabetol. 2002, 91, 304–314. [Google Scholar] [CrossRef]
- Krencker, F.-N.; Bodin, S.; Hoffmann, R.; Suan, G.; Mattioli, E.; Kabiri, L.; Föllmi, K.; Immenhauser, A. The middle Toarcian cold snap: Trigger of mass extinction and carbonate factory demise. Glob. Planet. Chang. 2014, 117, 64–78. [Google Scholar] [CrossRef]
- Colombié, C.; Carcel, D.; Lécuyer, C.; Ruffel, A.; Schnyder, J. Temperature and cyclone frequency in Kimmeridgian Greenhouse period (late Jurassic). Glob. Planet. Chang. 2018, 170, 126–145. [Google Scholar] [CrossRef]
- Dubicka, Z.; Peryt, D. Integrated biostratigraphy of Upper Maastrichtian chalk at Chełm (SE Poland). Ann. Soc. Geol. Pol. 2011, 81, 185–197. [Google Scholar]
- Lecuyer, C.; Grandjean, P.; O’Neil, J.; Cappetta, H.; Martineau, F. Thermal excursions in the ocean at the Cretaceous—Tertiary boundary (northern Morocco): δ18O record of phosphatic fish debris. Palaeogeogr. Palaeoclim. Palaeoecol. 1993, 105, 235–243. [Google Scholar] [CrossRef] [Green Version]
- Picard, S.; Garcia, J.-P.; Lécuyer, C.; Sheppard, S.M.F.; Cappetta, H.; Emig, C.C. δ18O values of coexisting brachio-pods and fish: Temperature differences and estimates of paleo–water depths. Geology 1998, 26, 975–978. [Google Scholar] [CrossRef]
- Billon-Bruyat, J.-P.; Lécuyer, C.; Martineau, F.; Mazin, J.-M. Oxygen isotope compositions of Late Jurassic vertebrate remains from lithographic limestones of western Europe: Implications for the ecology of fish, turtles, and crocodilians. Palaeogeogr. Palaeoclim. Palaeoecol. 2005, 216, 359–375. [Google Scholar] [CrossRef]
- Kocsis, L.; Ősi, A.; Vennemann, T.; Trueman, C.N.; Palmer, M.R. Geochemical study of vertebrate fossils from the Upper Cretaceous (Santonian) Csehbánya Formation (Hungary): Evidence for a freshwater habitat of mosasaurs and pycnodont fish. Palaeogeogr. Palaeoclim. Palaeoecol. 2009, 280, 532–542. [Google Scholar] [CrossRef]
- Bera, M.K.; Bhattacharya, K.; Sarkar, A.; Samanta, A.; Kumar, K.; Sahni, A. Oxygen isotope analysis of bone and tooth enamel phosphate from paleogene sediments: Experimental techniques and initial results. J. Geol. Soc. India 2010, 76, 275–282. [Google Scholar] [CrossRef]
- Elrick, M.; Rieboldt, S.; Saltzman, M.; McKay, R.M. Oxygen-isotope trends and seawater temperature changes across the Late Cambrian Steptoean positive carbon-isotope excursion (SPICE event). Geology 2011, 39, 987–990. [Google Scholar] [CrossRef] [Green Version]
- Bernard, A.; Lécuyer, C.; Vincent, P.; Amiot, R.; Bardet, N.; Buffetaut, E.; Cuny, G.; Fourel, F.; Martineau, F.; Mazin, J.-M.; et al. Regulation of Body Temperature by Some Mesozoic Marine Reptiles. Science 2010, 328, 1379–1382. [Google Scholar] [CrossRef]
- Pouech, J.; Amiot, R.; Lecuyer, C.; Mazin, J.; Martineau, F.; Fourel, F. Oxygen isotope composition of vertebrate phosphates from Cherves-de-Cognac (Berriasian, France): Environmental and ecological significance. Palaeogeogr. Palaeoclim. Palaeoecol. 2014, 410, 290–299. [Google Scholar] [CrossRef]
- Lécuyer, C.; Picard, S.; Garcia, J.-P.; Sheppard, S.M.F.; Grandjean, P.; Dromart, G. Thermal evolution of Tethyan surface waters during the Middle-Late Jurassic: Evidence from δ18O values of marine fish teeth. Paleoceanography 2003, 18, 1076. [Google Scholar] [CrossRef]
- Zazzo, A.; Lecuyer, C.; Sheppard, S.M.; Grandjean, P.; Mariotti, A. Diagenesis and the reconstruction of paleoenvironments: A method to restore original δ18O values of carbonate and phosphate from fossil tooth enamel. Geochim. Cosmochim. Acta 2004, 68, 2245–2258. [Google Scholar] [CrossRef]
- Shemesh, A. Crystallinity and diagenesis of sedimentary apatites. Geochim. Cosmochim. Acta 1990, 54, 2433–2438. [Google Scholar] [CrossRef]
- Pucéat, E.; Reynard, B.; Lécuyer, C. Can crystallinity be used to determine the degree of chemical alteration of biogenic apatites? Chem. Geol. 2004, 205, 83–97. [Google Scholar] [CrossRef]
- Thomas, D.B.; McGoverin, C.M.; Fordyce, R.E.; Frew, R.D.; Gordon, K.C. Raman spectroscopy of fossil bioapatite—A proxy for diagenetic alteration of the oxygen isotope composition. Palaeogeogr. Palaeoclim. Palaeoecol. 2011, 310, 62–70. [Google Scholar] [CrossRef]
- Epstein, A.G.; Epstein, J.B.; Harris, L.D. Conodont color alteration—An index to organic metamorphism. In Geological Survey Professional Paper 995; United States Government Printing Office: Washington, DC, USA, 1977; pp. 1–27. [Google Scholar]
- Rejebian, V.A.; Harris, A.G.; Huebner, J.S. Conodont color and textural alteration: An index to regional metamor-phism, contact metamorphism, and hydrothermal alteration. Geol. Soc. Am. Bull. 1987, 99, 471–479. [Google Scholar] [CrossRef]
- Dembicki, H. Practical Petroleum Geochemistry for Exploration and Production; Elsevier: Amsterdam, The Netherlands, 2017; p. 331. [Google Scholar] [CrossRef]
- Joachimski, M.; Breisig, S.; Buggisch, W.; Talent, J.; Mawson, R.; Gereke, M.; Morrow, J.; Day, J.; Weddige, K. Devonian climate and reef evolution: Insights from oxygen isotopes in apatite. Earth Planet. Sci. Lett. 2009, 284, 599–609. [Google Scholar] [CrossRef]
- Trotter, J.; Williams, I.S.; Barnes, C.R.; Männik, P.; Simpson, A. New conodont δ18O records of Silurian climate change: Implications for environmental and biological events. Palaeogeogr. Palaeoclim. Palaeoecol. 2016, 443, 34–48. [Google Scholar] [CrossRef]
- Wheeley, J.; Smith, M.P.; Boomer, I. Oxygen isotope variability in conodonts: Implications for reconstructing Palaeozoic palaeoclimates and palaeoceanography. J. Geol. Soc. 2012, 169, 239–250. [Google Scholar] [CrossRef]
- Nöth, S. Conodont color (CAI) versus microcrystalline and textural changes in Upper Triassic conodonts from Northwest Germany. Facies 1998, 38, 165–173. [Google Scholar] [CrossRef]
- Wierzbowski, H.; Szaniawski, H.; Błażejowski, B. Structural, chemical and isotope evidence for secondary phosphate mineralization of grasping spines of Early Palaeozoic chaetognaths. Lethaia 2021, 54, 245–259. [Google Scholar] [CrossRef]
- Bocherens, H.; Brinkman, D.B.; Dauphin, Y.; Mariotti, A. Microstructural and geochemical investigations on Late Cretaceous archosaur teeth from Alberta, Canada. Can. J. Earth Sci. 1994, 31, 783–792. [Google Scholar] [CrossRef]
- Kohn, M.; Schoeninger, M.J.; Barker, W.W. Altered states: Effects of diagenesis on fossil tooth chemistry. Geochim. Cosmochim. Acta 1999, 63, 2737–2747. [Google Scholar] [CrossRef]
- Reynard, B.; Lecuyer, C.; Grandjean, P. Crystal-chemical controls on rare-earth element concentrations in fossil biogenic apatites and implications for paleoenvironmental reconstructions. Chem. Geol. 1999, 155, 233–241. [Google Scholar] [CrossRef]
- Armstrong, H.; Pearson, D.; Griselin, M. Thermal effects on rare earth element and strontium isotope chemistry in single conodont elements. Geochim. Cosmochim. Acta 2001, 65, 435–441. [Google Scholar] [CrossRef] [Green Version]
- Lécuyer, C.; Bogey, C.; Garcia, J.-P.; Grandjean, P.; Barrat, J.-A.; Floquet, M.; Bardet, N.; Pereda-Superbiola, X. Stable isotope composition and rare earth element content of vertebrate remains from the Late Cretaceous of northern Spain (Laño): Did the environmental record survive? Palaeogeogr. Palaeoclim. Palaeoecol. 2003, 193, 457–471. [Google Scholar] [CrossRef]
- Trotter, J.A.; Eggins, S.M. Chemical systematics of conodont apatite determined by laser ablation ICPMS. Chem. Geol. 2006, 233, 196–216. [Google Scholar] [CrossRef]
- Kamenov, G.D.; Lofaro, E.M.; Goad, G.; Krigbaum, J. Trace elements in modern and archaeological human teeth: Implications for human metal exposure and enamel diagenetic changes. J. Archaeol. Sci. 2018, 99, 27–34. [Google Scholar] [CrossRef]
- Lübke, A.; Enax, J.; Loza, K.; Prymak, O.; Gaengler, P.; Fabritius, H.-O.; Raabe, D.; Epple, M. Dental lessons from past to present: Ultrastructure and composition of teeth from plesiosaurs, dinosaurs, extinct and recent sharks. RSC Adv. 2015, 5, 61612–61622. [Google Scholar] [CrossRef] [Green Version]
- De Renzi, M.; Manzanares, E.; Marin-Monfort, M.D.; Botella, H. Comments on “Dental lessons from past to present: Ultrastructure and composition of teeth from plesiosaurs, dinosaurs, extinct and recent sharks” by A. Lübke, J. Enax, K. Loza, O. Prymak, P. Gaengler, H.-O. Fabritius, D. Raabe and M. Epple, RSC Adv., 2015, 5, 61612. RSC Adv. 2016, 6, 74384–74388. [Google Scholar] [CrossRef] [Green Version]
- Luebke, A.; Loza, K.; Patnaik, R.; Enax, J.; Raabe, D.; Prymak, O.; Fabritius, H.-O.; Gaengler, P.; Epple, M. Reply to the ‘Comments on “Dental lessons from past to present: Ultrastructure and composition of teeth from plesiosaurs, dinosaurs, extinct and recent sharks”’ by H. Botella et al., RSC Adv., 2016, 6, 74384–74388. RSC Adv. 2017, 7, 6215–6222. [Google Scholar] [CrossRef] [Green Version]
- Lécuyer, C.; Reynard, B.; Grandjean, P. Rare earth element evolution of Phanerozoic seawater recorded in biogenic apatites. Chem. Geol. 2004, 204, 63–102. [Google Scholar] [CrossRef]
- Ségalen, L.; de Rafelis, M.; Lee-Thorp, J.A.; Maurer, A.-F.; Renard, M. Cathodoluminescence tools provide clues to depositional history in Miocene and Pliocene mammalian teeth. Palaeogeogr. Palaeoclim. Palaeoecol. 2008, 266, 246–253. [Google Scholar] [CrossRef]
- Fischer, J.; Voigt, S.; Franz, M.; Schneider, J.W.; Joachimski, M.M.; Tichomirowa, M.; Götze, J.; Furrer, H. Palaeoenvironments of the late Triassic Rhaetian Sea: Implications from oxygen and strontium isotopes of hybodont shark teeth. Palaeogeogr. Palaeocl. 2012, 353–355, 60–72. [Google Scholar] [CrossRef]
- Fischer, J.; Schneider, J.W.; Voigt, S.; Joachimski, M.M.; Tichomirowa, M.; Tütken, T.; Götze, J.; Berner, U. Oxygen and strontium isotopes from fossil shark teeth: Environmental and ecological implications for Late Palaeozoic European basins. Chem. Geol. 2013, 342, 44–62. [Google Scholar] [CrossRef]
- Wierzbowski, H. Effects of pre-treatments and organic matter on oxygen and carbon isotope analyses of skeletal and inorganic calcium carbonate. Int. J. Mass Spectrom. 2007, 268, 16–29. [Google Scholar] [CrossRef]
- Craig, H. The measurement of oxygen isotope paleotemperatures. In Proceedings of the Spoleto Conference on Stable Isotopes in Oceanographic Studies and Paleotemperatures; Tongiorgi, E., Ed.; Consiglio Nazionale delle Ricerche: Pisa, Italy, 1965; pp. 161–182. [Google Scholar]
- Coplen, T.B.; Kendall, C.; Hopple, J. Comparison of stable isotope reference samples. Nat. Cell Biol. 1983, 302, 236–238. [Google Scholar] [CrossRef]
- Friedman, I.; O’Neil, J.R. Compilation of Stable Isotope Fractionation Factors of Geochemical Interest, Data of Geochemistry, 6th ed.; United States Department of the Interior: Washington, DC, USA, 1977; pp. KK1–KK12.
- O’Neil, J.R.; Clayton, R.N.; Mayeda, T.K. Oxygen isotope fractionation in divalent metal carbonates. J. Chem. Phys. 1969, 51, 5547–5558. [Google Scholar] [CrossRef]
- Hays, P.; Grossman, E. Oxygen isotopes in meteoric calcite cements as indicators of continental paleoclimate. Geology 1991, 19, 441–444. [Google Scholar] [CrossRef]
- Kim, S.-T.; O’Neil, J.R. Equilibrium and nonequilibrium oxygen isotope effects in synthetic carbonates. Geochim. Cosmochim. Acta 1997, 61, 3461–3475. [Google Scholar] [CrossRef]
- Coplen, T.B. Calibration of the calcite–water oxygen-isotope geothermometer at Devils Hole, Nevada, a natural laboratory. Geochim. Cosmochim. Acta 2007, 71, 3948–3957. [Google Scholar] [CrossRef]
- Lynch-Stieglitz, J.; Curry, W.B.; Slowey, N.C. A geostrophic transport estimate for the Florida Current from the oxygen isotope composition of benthic foraminifera. Paleoceanography 1999, 14, 360–373. [Google Scholar] [CrossRef] [Green Version]
- Marchitto, T.; Curry, W.; Lynch-Stieglitz, J.; Bryan, S.; Cobb, K.; Lund, D. Improved oxygen isotope temperature calibrations for cosmopolitan benthic foraminifera. Geochim. Cosmochim. Acta 2014, 130, 1–11. [Google Scholar] [CrossRef]
- Grossman, E.; Ku, T.-L. Oxygen and carbon isotope fractionation in biogenic aragonite: Temperature effects. Chem. Geol. Isot. Geosci. Sect. 1986, 59, 59–74. [Google Scholar] [CrossRef]
- Böhm, F.; Joachimski, M.; Dullo, W.-C.; Eisenhauer, A.; Lehnert, H.; Reitner, J.; Wörheide, G. Oxygen isotope fractionation in marine aragonite of coralline sponges. Geochim. Cosmochim. Acta 2000, 64, 1695–1703. [Google Scholar] [CrossRef] [Green Version]
- Kim, S.-T.; O’Neil, J.R.; Hillaire-Marcel, C.; Mucci, A. Oxygen isotope fractionation between synthetic aragonite and water: Influence of temperature and Mg2+ concentration. Geochim. Cosmochim. Acta 2007, 71, 4704–4715. [Google Scholar] [CrossRef]
- Shackleton, N.; Kennett, J.; Houtz, R. Paleotemperature history of the Cenozoic and the initiation of Antarctic glaciation: Oxygen and carbon isotope analyses in DSDP Sites 277, 279 and 281. Initial Rep. Deep Sea Drill. Proj. 1975, 29, 743–755. [Google Scholar] [CrossRef]
- Zhou, G.-T.; Zheng, Y.-F. An experimental study of oxygen isotope fractionation between inorganically precipitated aragonite and water at low temperatures. Geochim. Cosmochim. Acta 2003, 67, 387–399. [Google Scholar] [CrossRef]
- Carpenter, S.J.; Lohmann, K.C. δ18O and δ13C values of modern brachiopod shells. Geochim. Cosmochim. Acta 1995, 59, 3749–3764. [Google Scholar] [CrossRef]
- James, N.P.; Bone, Y.; Kyser, T.K. Brachiopod δ18O values do reflect ambient oceanography: Lecepode Shelf, southern Australia. Geology 1997, 25, 551–554. [Google Scholar] [CrossRef]
- Parkinson, D.; Curry, G.B.; Cusack, M.; Fallick, A.E. Shell structure, patterns and trends of oxygen and carbon stable isotopes in modern brachiopod shells. Chem. Geol. 2005, 219, 193–235. [Google Scholar] [CrossRef]
- Hong, W.; Keppens, E.; Nielsen, P.; Van Riet, A. Oxygen and carbon isotope study of the Holocene oyster reefs and paleoenvironmental reconstruction on the northwest coast of Bohai Bay, China. Mar. Geol. 1995, 124, 289–302. [Google Scholar] [CrossRef]
- Kirby, M.X.; Soniat, T.M.; Spero, H. Stable isotope sclerochronology of Pleistocene and Recent oyster shells (Crassostrea virginica). Palaios 1998, 13, 560. [Google Scholar] [CrossRef]
- Surge, D.; Lohmann, K.; Dettman, D.L. Controls on isotopic chemistry of the American oyster, Crassostrea virginica: Implications for growth patterns. Palaeogeogr. Palaeoclim. Palaeoecol. 2001, 172, 283–296. [Google Scholar] [CrossRef]
- Surge, D.M.; Lohmann, K.C.; Goodfriend, G.A. Reconstructing estuarine conditions: Oyster shells as recorders of environmental change, Southwest Florida. Estuar. Coast. Shelf Sci. 2003, 57, 737–756. [Google Scholar] [CrossRef]
- Titschack, J.; Zuschin, M.; Spötl, C.; Baal, C. The giant oyster Hyotissa hyotis from the northern Red Sea as a decadal-scale archive for seasonal environmental fluctuations in coral reef habitats. Coral Reefs 2010, 29, 1061–1075. [Google Scholar] [CrossRef]
- Ullmann, C.V.; Wiechert, U.; Korte, C. Oxygen isotope fluctuations in a modern North Sea oyster (Crassostrea gigas) compared with annual variations in seawater temperature: Implications for palaeoclimate studies. Chem. Geol. 2010, 277, 160–166. [Google Scholar] [CrossRef]
- Shackleton, N.J. Attainment of isotopic equilibrium between ocean water and benthonic foraminifera genus Uvigerina: Isotopic changes in the ocean during the last glacial. Colloq. Int. C.N.R.S. 1974, 219, 203–209. [Google Scholar]
- Grossman, E. Stable isotopes in modern benthic foraminifera; a study of vital effect. J. Foraminifer. Res. 1987, 17, 48–61. [Google Scholar] [CrossRef]
- Keigwin, L.D.; Corliss, B.H. Stable isotopes in late middle Eocene to Oligocene foraminifera. GSA Bull. 1986, 97, 335–345. [Google Scholar] [CrossRef]
- McCorkle, D.C.; Corliss, B.H.; Emerson, S.R.; Keigwin, L.D. The influence of microhabitats on the carbon isotopic composition of deep-sea benthic foraminifera. Paleoceanography 1990, 5, 161–185. [Google Scholar] [CrossRef]
- McCorkle, D.C.; Corliss, B.H.; Farnham, C.A. Vertical distributions and stable isotopic compositions of live (stained) benthic foraminifera from the North Carolina and California continental margins. Deep. Sea Res. Part I Oceanogr. Res. Pap. 1997, 44, 983–1024. [Google Scholar] [CrossRef]
- Zachos, J.C.; Breza, J.R.; Wise, S.W. Early Oligocene ice-sheet expansion on Antarctica: Stable isotope and sedimentological evidence from Kerguelen Plateau, southern Indian Ocean. Geology 1992, 20, 569–573. [Google Scholar] [CrossRef]
- Rathburn, A.; Corliss, B.; Tappa, K.; Lohmann, K. Comparisons of the ecology and stable isotopic compositions of living (stained) benthic foraminifera from the Sulu and South China Seas. Deep. Sea Res. Part I Oceanogr. Res. Pap. 1996, 43, 1617–1646. [Google Scholar] [CrossRef]
- Duplessy, J.-C.; Labeyrie, L.; Waelbroeck, C. Constraints on the ocean oxygen isotopic enrichment between the Last Glacial Maximum and the Holocene: Paleoceanographic implications. Quat. Sci. Rev. 2002, 21, 315–330. [Google Scholar] [CrossRef]
- Schmiedl, G.; Pfeilsticker, M.; Hemleben, C.; Mackensen, A. Environmental and biological effects on the stable isotope composition of recent deep-sea benthic foraminifera from the western Mediterranean Sea. Mar. Micropaleontol. 2004, 51, 129–152. [Google Scholar] [CrossRef]
- Fontanier, C.; Mackensen, A.; Jorissen, F.; Anschutz, P.; Licari, L.; Griveaud, C. Stable oxygen and carbon isotopes of live benthic foraminifera from the Bay of Biscay: Microhabitat impact and seasonal variability. Mar. Micropaleontol. 2006, 58, 159–183. [Google Scholar] [CrossRef]
- Basak, C.; Rathburn, A.E.; Pérez, M.E.; Martin, J.B.; Kluesner, J.W.; Levin, L.; De Deckker, P.; Gieskes, J.M.; Abriani, M. Carbon and oxygen isotope geochemistry of live (stained) benthic foraminifera from the Aleutian Margin and the Southern Australian Margin. Mar. Micropaleontol. 2009, 70, 89–101. [Google Scholar] [CrossRef]
- Ishimura, T.; Tsunogai, U.; Hasegawa, S.; Nakagawa, F.; Oi, T.; Kitazato, H.; Suga, H.; Toyofuku, T. Variation in stable carbon and oxygen isotopes of individual benthic foraminifera: Tracers for quantifying the magnitude of isotopic disequilibrium. Biogeosciences 2012, 9, 4353–4367. [Google Scholar] [CrossRef] [Green Version]
- Franco-Fraguas, P.; Costa, K.B.; Toledo, F.A.D.L. Stable isotope/test size relationship in Cibicidoides wuellerstorfi. Braz. J. Oceanogr. 2011, 59, 287–291. [Google Scholar] [CrossRef]
- Erez, J.; Luz, B. Experimental paleotemperature equation for planktonic foraminifera. Geochim. Cosmochim. Acta 1983, 47, 1025–1031. [Google Scholar] [CrossRef]
- Bouvier-Soumagnac, Y.; Duplessy, J.-C. Carbon and oxygen isotopic composition of planktonic foraminifera from laboratory culture, plankton tows and Recent sediment; implications for the reconstruction of paleoclimatic conditions and of the global carbon cycle. J. Foraminifer. Res. 1985, 15, 302–320. [Google Scholar] [CrossRef]
- Bemis, B.E.; Spero, H.; Bijma, J.; Lea, D.W. Reevaluation of the oxygen isotopic composition of planktonic foraminifera: Experimental results and revised paleotemperature equations. Paleoceanography 1998, 13, 150–160. [Google Scholar] [CrossRef] [Green Version]
- Mulitza, S.; Boltovskoy, D.; Donner, B.; Meggers, H.; Paul, A.; Wefer, G. Temperature:δ18O relationships of planktonic foraminifera collected from surface waters. Palaeogeogr. Palaeoclim. Palaeoecol. 2003, 202, 143–152. [Google Scholar] [CrossRef]
- Jiménez-López, C.; Romanek, C.S.; Huertas, F.; Ohmoto, H.; Caballero, E. Oxygen isotope fractionation in synthetic magnesian calcite. Geochim. Cosmochim. Acta 2004, 68, 3367–3377. [Google Scholar] [CrossRef]
- Tarutani, T.; Clayton, R.N.; Mayeda, T.K. The effect of polymorphism and magnesium substitution on oxygen isotope fractionation between calcium carbonate and water. Geochim. Cosmochim. Acta 1969, 33, 987–996. [Google Scholar] [CrossRef]
- Brand, U.; Azmy, K.; Bitner, M.; Logan, A.; Zuschin, M.; Came, R.; Ruggiero, E. Oxygen isotopes and MgCO3 in brachiopod calcite and a new paleotemperature equation. Chem. Geol. 2013, 359, 23–31. [Google Scholar] [CrossRef]
- Day, C.; Henderson, G. Oxygen isotopes in calcite grown under cave-analogue conditions. Geochim. Cosmochim. Acta 2011, 75, 3956–3972. [Google Scholar] [CrossRef]
- Dietzel, M.; Tang, J.; Leis, A.; Köhler, S.J. Oxygen isotopic fractionation during inorganic calcite precipitation ― Effects of temperature, precipitation rate and pH. Chem. Geol. 2009, 268, 107–115. [Google Scholar] [CrossRef]
- Gabitov, R.I.; Watson, E.B.; Sadekov, A.Y. Oxygen isotope fractionation between calcite and fluid as a function of growth rate and temperature: An in situ study. Chem. Geol. 2012, 306-307, 92–102. [Google Scholar] [CrossRef]
- Watkins, J.M.; Nielsen, L.C.; Ryerson, F.J.; DePaolo, D.J. The influence of kinetics on the oxygen isotope composition of calcium carbonate. Earth Planet. Sci. Lett. 2013, 375, 349–360. [Google Scholar] [CrossRef]
- Watkins, J.M.; Hunt, J.D.; Ryerson, F.J.; DePaolo, D.J. The influence of temperature, pH, and growth rate on the δ18O composition of inorganically precipitated calcite. Earth Planet. Sci. Lett. 2014, 404, 332–343. [Google Scholar] [CrossRef] [Green Version]
- Lécuyer, C.; Hutzler, A.; Amiot, R.; Daux, V.; Grosheny, D.; Otero, O.; Martineau, F.; Fourel, F.; Balter, V.; Reynard, B. Carbon and oxygen isotope fractionations between aragonite and calcite of shells from modern molluscs. Chem. Geol. 2012, 332–333, 92–101. [Google Scholar] [CrossRef]
- Patterson, W.P.; Smith, G.R.; Lohmann, K.C. Continental paleothermometry and seasonality using the isotopic composition of aragonitic otoliths of freshwater fshes. Sea Ice 2013, 78, 191–202. [Google Scholar] [CrossRef]
- Thorrold, S.; Campana, S.E.; Jones, C.M.; Swart, P. Factors determining δ13C and δ18O fractionation in aragonitic otoliths of marine fish. Geochim. Cosmochim. Acta 1997, 61, 2909–2919. [Google Scholar] [CrossRef]
- Høie, H.; Otterlei, E.; Folkvord, A. Temperature-dependent fractionation of stable oxygen isotopes in otoliths of juvenile cod (Gadus morhua L.). ICES J. Mar. Sci. 2004, 61, 243–251. [Google Scholar] [CrossRef]
- White, R.M.P.; Dennis, P.F.; Atkinson, T.C. Experimental calibration and field investigation of the oxygen isotopic fractionation between biogenic aragonite and water. Rapid Commun. Mass. Spectrom 1999, 13, 1242–1247. [Google Scholar] [CrossRef]
- Pucéat, E.; Joachimski, M.; Bouilloux, A.; Monna, F.; Bonin, A.; Motreuil, S.; Morinière, P.; Hénard, S.; Mourin, J.; Dera, G. Revised phosphate–water fractionation equation reassessing paleotemperatures derived from biogenic apatite. Earth Planet. Sci. Lett. 2010, 298, 135–142. [Google Scholar] [CrossRef]
- Lécuyer, C.; Amiot, R.; Touzeau, A.; Trotter, J. Calibration of the phosphate δ18O thermometer with carbonate–water oxygen isotope fractionation equations. Chem. Geol. 2013, 347, 217–226. [Google Scholar] [CrossRef]
- Longinelli, A. Comment on work by Pucéat et al. (2010) on a revised phosphate–water fractionation equation. Earth Planet. Sci. Lett. 2013, 377–378, 378–379. [Google Scholar] [CrossRef]
- Pucéat, E.; Joachimski, M.; Bouilloux, A.; Monna, F.; Bonin, A.; Motreuil, S.; Morinière, P.; Henard, S.; Mourin, J.; Dera, G.; et al. Reply on Comment by Longinelli (2013) on a revised phosphate–water fractionation equation. Earth Planet. Sci. Lett. 2013, 377–378, 380–382. [Google Scholar] [CrossRef]
- Chenery, C.A.; Müldner, G.; Evans, J.; Eckardt, H.; Lewis, M. Strontium and stable isotope evidence for diet and mobility in Roman Gloucester, UK. J. Archaeol. Sci. 2010, 37, 150–163. [Google Scholar] [CrossRef] [Green Version]
- Wefer, G.; Berger, W.H. Isotope paleontology: Growth and composition of extant calcareous species. Mar. Geol. 1991, 100, 207–248. [Google Scholar] [CrossRef]
- McConnaughey, T. 13C and 18O isotopic disequilibrium in biological carbonates: I. Patterns. Geochim. Cosmochim. Acta 1989, 53, 151–162. [Google Scholar] [CrossRef]
- McConnaughey, T. 13C and 18O isotopic disequilibrium in biological carbonates: II. In vitro simulation of kinetic isotope effects. Geochim. Cosmochim. Acta 1989, 53, 163–171. [Google Scholar] [CrossRef]
- Adkins, J.F.; Boyle, E.A.; Curry, W.B.; Lutringer, A. Stable isotopes in deep-sea corals and a new mechanism for “vital effects”. Geochim. Cosmochim. Acta 2003, 67, 1129–1143. [Google Scholar] [CrossRef] [Green Version]
- Rollion-Bard, C.; Chaussidon, M.; France-Lanord, C. pH control on oxygen isotopic composition of symbiotic corals. Earth Planet. Sci. Lett. 2003, 215, 275–288. [Google Scholar] [CrossRef]
- Chen, S.; Gagnon, A.C.; Adkins, J.F. Carbonic anhydrase, coral calcification and a new model of stable isotope vital effects. Geochim. Cosmochim. Acta 2018, 236, 179–197. [Google Scholar] [CrossRef] [Green Version]
- McConnaughey, T.A.; Burdett, J.; Whelan, J.F.; Paull, C.K. Carbon isotopes in biological carbonates: Respiration and photosynthesis. Geochim. Cosmochim. Acta 1997, 61, 611–622. [Google Scholar] [CrossRef]
- Swart, P. Carbon and oxygen isotope fractionation in scleractinian corals: A review. Earth Sci. Rev. 1983, 19, 51–80. [Google Scholar] [CrossRef]
- Prada, F.; Yam, R.; Levy, O.; Caroselli, E.; Falini, G.; Dubinsky, Z.; Goffredo, S.; Shemesh, A. Kinetic and metabolic isotope effects in zooxanthellate and non-zooxanthellate Mediterranean corals along a wide latitudinal gradient. Front. Mar. Sci. 2019, 6. [Google Scholar] [CrossRef]
- Smith, J.E.; Schwarcz, H.P.; Risk, M.J.; McConnaughey, T.A.; Keller, N. Paleotemperatures from deep-sea corals: Overcoming ‘vital effects’. Palaios 2000, 15, 25–32. [Google Scholar] [CrossRef]
- Lutringer, A.; Blamart, D.; Frank, N.; Labeyrie, L. Paleotemperatures from deep-sea corals: Scale effects. In Cold-Water Corals and Ecosystems; Freiwald, A., Roberts, J.M., Eds.; Springer: Berlin, Germany, 2005; pp. 1081–1096. [Google Scholar] [CrossRef]
- Marali, S.; Wisshak, M.; Correa, M.L.; Freiwald, A. Skeletal microstructure and stable isotope signature of three bathyal solitary cold-water corals from the Azores. Palaeogeogr. Palaeoclim. Palaeoecol. 2013, 373, 25–38. [Google Scholar] [CrossRef]
- Chaabane, S.; Correa, M.L.; Montagna, P.; Kallel, N.; Taviani, M.; Linares, C.; Ziveri, P. Exploring the oxygen and carbon isotopic composition of the Mediterranean red coral (Corallium rubrum) for seawater temperature reconstruction. Mar. Chem. 2016, 186, 11–23. [Google Scholar] [CrossRef]
- Spero, H. Do planktic foraminifera accurately record shifts in the carbon isotopic composition of seawater ΣCO2? Mar. Micropaleontol. 1992, 19, 275–285. [Google Scholar] [CrossRef]
- Spero, H.; Lea, D.W. Intraspecific stable isotope variability in the planktic foraminifera Globigerinoides sacculifer: Results from laboratory experiments. Mar. Micropaleontol. 1993, 22, 221–234. [Google Scholar] [CrossRef]
- Spero, H.J.; Bijma, J.; Lea, D.W.; Bemis, B.E. Effect of seawater carbonate concentration on foraminiferal carbon and oxygen isotopes. Nat. Cell Biol. 1997, 390, 497–500. [Google Scholar] [CrossRef]
- Ziveri, P.; Thoms, S.; Probert, I.; Geisen, M.; Langer, G. A universal carbonate ion effect on stable oxygen isotope ratios in unicellular planktonic calcifying organisms. Biogeosciences 2012, 9, 1025–1032. [Google Scholar] [CrossRef] [Green Version]
- Uchikawa, J.; Zeebe, R.E. Examining possible effects of seawater pH decline on foraminiferal stable isotopes during the Paleocene-Eocene Thermal Maximum. Paleoceanography 2010, 25, 2216. [Google Scholar] [CrossRef] [Green Version]
- Lea, D.W.; Bijma, J.; Spero, H.J.; Archer, D. Implications of a carbonate ion effect on shell carbon and oxygen isotopes for glacial ocean conditions. In Use of Proxies in Paleoceanography: Examples from the South Atlantic; Fischer, G., Wefer, G., Eds.; Springer: Berlin/Heidelberg, Germany, 1999; pp. 513–522. [Google Scholar] [CrossRef]
- Zeebe, R.E. Seawater pH and isotopic paleotemperatures of Cretaceous oceans. Palaeogeogr. Palaeoclim. Palaeoecol. 2001, 170, 49–57. [Google Scholar] [CrossRef]
- Ye, F.; Jurikova, H.; Angiolini, L.; Brand, U.; Crippa, G.; Henkel, D.; Laudien, J.; Hiebenthal, C.; Šmajgl, D. Variation in brachiopod microstructure and isotope geochemistry under low-pH–ocean acidification conditions. Biogeosciences 2019, 16, 617–642. [Google Scholar] [CrossRef] [Green Version]
- Wilke, I.; Bickert, T.; Peeters, F.J. The influence of seawater carbonate ion concentration [CO32−] on the stable carbon isotope composition of the planktic foraminifera species Globorotalia inflata. Mar. Micropaleontol. 2006, 58, 243–258. [Google Scholar] [CrossRef]
- Barras, C.; Duplessy, J.-C.; Geslin, E.; Michel, E.; Jorissen, F. Calibration of δ18O of cultured benthic foraminiferal calcite as a function of temperature. Biogeosciences 2010, 7, 1349–1356. [Google Scholar] [CrossRef] [Green Version]
- Friedrich, O.; Schmiedl, G.; Erlenkeuser, H. Stable isotope composition of Late Cretaceous benthic foraminifera from the southern South Atlantic: Biological and environmental effects. Mar. Micropaleontol. 2006, 58, 135–157. [Google Scholar] [CrossRef]
- Bojanowski, M.J.; Dubicka, Z.; Minoletti, F.; Olszewska-Nejbert, D.; Surowski, M. Stable C and O isotopic study of the Campanian chalk from the Mielnik section (eastern Poland): Signals from bulk rock, belemnites, benthic foraminifera, nannofossils and microcrystalline cements. Palaeogeogr. Palaeoclim. Palaeoecol. 2017, 465, 193–211. [Google Scholar] [CrossRef]
- Yamamoto, K.; Asami, R.; Iryu, Y. Carbon and oxygen isotopic compositions of modern brachiopod shells from a warm-temperate shelf environment, Sagami Bay, central Japan. Palaeogeogr. Palaeoclim. Palaeoecol. 2010, 291, 348–359. [Google Scholar] [CrossRef]
- Yamamoto, K.; Asami, R.; Iryu, Y. Within-shell variations in carbon and oxygen isotope compositions of two modern brachiopods from a subtropical shelf environment off Amami-o-shima, southwestern Japan. Geochem. Geophys. Geosyst. 2010, 11, 10009. [Google Scholar] [CrossRef]
- Penman, D.E.; Hönisch, B.; Rasbury, E.T.; Hemming, N.G.; Spero, H.J. Boron, carbon, and oxygen isotopic composition of brachiopod shells: Intra-shell variability, controls, and potential as a paleo-pH recorder. Chem. Geology 2013, 340, 32–39. [Google Scholar] [CrossRef] [Green Version]
- Bajnai, D.; Fiebig, J.; Tomašových, A.; Garcia, S.M.; Rollion-Bard, C.; Raddatz, J.; Löffler, N.; Primo-Ramos, C.; Brand, U. Assessing kinetic fractionation in brachiopod calcite using clumped isotopes. Sci. Rep. 2018, 8, 533. [Google Scholar] [CrossRef] [PubMed]
- Auclair, A.-C.; Joachimski, M.; Lecuyer, C. Deciphering kinetic, metabolic and environmental controls on stable isotope fractionations between seawater and the shell of Terebratalia transversa (Brachiopoda). Chem. Geol. 2003, 202, 59–78. [Google Scholar] [CrossRef]
- Takayanagi, H.; Asami, R.; Otake, T.; Abe, O.; Miyajima, T.; Kitagawa, H.; Iryu, Y. Quantitative analysis of intraspecific variations in the carbon and oxygen isotope compositions of the modern cool-temperate brachiopod Terebratulina crossei. Geochim. Cosmochim. Acta 2015, 170, 301–320. [Google Scholar] [CrossRef]
- Owen, R.; Kennedy, H.; Richardson, C. Isotopic partitioning between scallop shell calcite and seawater: Effect of shell growth rate. Geochim. Cosmochim. Acta 2002, 66, 1727–1737. [Google Scholar] [CrossRef]
- Huyghe, D.; Emmanuel, L.; de Rafelis, M.; Renard, M.; Ropert, M.; Labourdette, N.; Lartaud, F. Oxygen isotope disequilibrium in the juvenile portion of oyster shells biases seawater temperature reconstructions. Estuar. Coast. Shelf Sci. 2020, 240, 106777. [Google Scholar] [CrossRef]
- Hahn, S.; Rodolfo-Metalpa, R.; Griesshaber, E.; Schmahl, W.W.; Buhl, D.; Hall-Spencer, J.M.; Baggini, C.; Fehr, K.T.; Immenhauser, A. Marine bivalve shell geochemistry and ultrastructure from modern low pH environments: Environmental effect versus experimental bias. Biogeosciences 2012, 9, 1897–1914. [Google Scholar] [CrossRef] [Green Version]
- Alberti, M.; Fürsich, F.T.; Abdelhady, A.A.; Andersen, N. Middle to Late Jurassic equatorial seawater temperatures and latitudinal temperature gradients based on stable isotopes of brachiopods and oysters from Gebel Maghara, Egypt. Palaeogeogr. Palaeoclim. Palaeoecol. 2017, 468, 301–313. [Google Scholar] [CrossRef]
- Price, G.; Harwood, E. Isotopic analysis of belemnites and brachiopods from the Cretaceous (Albian) Hunstanton Red Chalk Formation (Hunstanton, Norfolk, UK). Proc. Geol. Assoc. 2012, 123, 479–485. [Google Scholar] [CrossRef]
- Price, G.D.; Teece, C. Reconstruction of Jurassic (Bathonian) palaeosalinity using stable isotopes and faunal associations. J. Geol. Soc. 2010, 167, 1199–1208. [Google Scholar] [CrossRef]
- Mettam, C.; Johnson, A.; Nunn, E.; Schöne, B.R. Stable isotope (δ18O and δ13C) sclerochronology of Callovian (Middle Jurassic) bivalves (Gryphaea (Bilobissa) dilobotes) and belemnites (Cylindroteuthis puzosiana) from the Peterborough Member of the Oxford Clay Formation (Cambridgeshire, England): Evidence of palaeoclimate, water depth and belemnite behaviour. Palaeogeogr. Palaeoclim. Palaeoecol. 2014, 399, 187–201. [Google Scholar] [CrossRef] [Green Version]
- Wilmsen, M.; Niebuhr, B. High-resolution Campanian–Maastrichtian carbon and oxygen stable isotopes of bulk-rock and skeletal components: Palaeoceanographic and palaeoenvironmental implications for the Boreal shelf sea. Acta Geol. Pol. 2017, 67, 47–74. [Google Scholar] [CrossRef] [Green Version]
- Alberti, M.; Arabas, A.; Fürsich, F.T.; Andersen, N.; Ziółkowski, P. The Middle to Upper Jurassic stable isotope record of Madagascar: Linking temperature changes with plate tectonics during the break-up of Gondwana. Gondwana Res. 2019, 73, 1–15. [Google Scholar] [CrossRef]
- Fürsich, F.; Singh, I.; Joachimski, M.; Krumm, S.; Schlirf, M. Palaeoclimate reconstructions of the Middle Jurassic of Kachchh (western India): An integrated approach based on palaeoecological, oxygen isotopic, and clay mineralogical data. Palaeogeogr. Palaeoclim. Palaeoecol. 2005, 217, 289–309. [Google Scholar] [CrossRef]
- Geiger, M.; Schweigert, G. Toarcian–Kimmeridgian depositional cycles of the south-western Morondava Basin along the rifted continental margin of Madagascar. Facies 2006, 52, 85–112. [Google Scholar] [CrossRef]
- Ditchfield, P.W. High northern palaeolatitude Jurassic-Cretaceous palaeotemperature variation: New data from Kong Karls Land, Svalbard. Palaeogeogr. Palaeoclim. Palaeoecol. 1997, 130, 163–175. [Google Scholar] [CrossRef]
- Price, G.D.; Hart, M.B.; Wilby, P.R.; Page, K.N. Isotopic analysis of Jurassic (Callovian) mollusks from the Christian Malford Laggerstätte (UK): Implications for ocean water temperature estimates based on belemnoids. Palaios 2015, 30, 645–654. [Google Scholar] [CrossRef] [Green Version]
- Price, G.D.; Twitchett, R.J.; Wheeley, J.R.; Buono, G. Isotopic evidence for long term warmth in the Mesozoic. Sci. Rep. 2013, 3, 1438. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Benito, M.I.; Reolid, M.; Viedma, C. On the microstructure, growth pattern and original porosity of belemnite rostra: Insights from calcite Jurassic belemnites. J. Iber. Geol. 2016, 42, 201–226. [Google Scholar] [CrossRef]
- Hoffmann, R.; Richter, D.; Neuser, R.; Jöns, N.; Linzmeier, B.; Lemanis, R.; Fusseis, F.; Xiao, X.; Immenhauser, A. Evidence for a composite organic–inorganic fabric of belemnite rostra: Implications for palaeoceanography and palaeoecology. Sediment. Geol. 2016, 341, 203–215. [Google Scholar] [CrossRef] [Green Version]
- Wierzbowski, H.; Bajnai, D.; Wacker, U.; Rogov, M.A.; Fiebig, J.; Tesakova, E.M. Clumped isotope record of salinity variations in the Subboreal Province at the Middle–Late Jurassic transition. Glob. Planet. Chang. 2018, 167, 172–189. [Google Scholar] [CrossRef]
- Vickers, M.L.; Fernandez, A.; Hesselbo, S.P.; Price, G.D.; Bernasconi, S.M.; Lode, S.; Ullmann, C.V.; Thibault, N.; Hougaard, I.W.; Korte, C. Unravelling Middle to Late Jurassic palaeoceanographic and palaeoclimatic signals in the Hebrides Basin using belemnite clumped isotope thermometry. Earth Planet. Sci. Lett. 2020, 546, 116401. [Google Scholar] [CrossRef]
- Vickers, M.; Bajnai, D.; Price, G.D.; Linckens, J.; Fiebig, J. Southern high-latitude warmth during the Jurassic–Cretaceous: New evidence from clumped isotope thermometry. Geology 2019, 47, 724–728. [Google Scholar] [CrossRef]
- Wisshak, M.; Correa, M.L.; Gofas, S.S.; Salas, C.; Taviani, M.; Jakobsen, J.; Freiwald, A. Shell architecture, element composition, and stable isotope signature of the giant deep-sea oyster Neopycnodonte zibrowii sp. n. from the NE Atlantic. Deep. Sea Res. Part I Oceanogr. Res. Pap. 2009, 56, 374–407. [Google Scholar] [CrossRef]
- Fenger, T.; Surge, D.; Schöne, B.; Milner, N. Sclerochronology and geochemical variation in limpet shells (Patella vulgata): A new archive to reconstruct coastal sea surface temperature. Geochem. Geophys. Geosyst. 2007, 8, 07001. [Google Scholar] [CrossRef] [Green Version]
- Killingley, J.S.; Newman, W.A. 18O fractionation in barnacle calcite: A barnacle paleotemperature equation. J. Mar. Res. 1982, 40, 893–902. [Google Scholar]
- Kohn, M. Predicting animal δ18O: Accounting for diet and physiological adaptation. Geochim. Cosmochim. Acta 1996, 60, 4811–4829. [Google Scholar] [CrossRef]
- Clementz, M.T.; Koch, P.L. Differentiating aquatic mammal habitat and foraging ecology with stable isotopes in tooth enamel. Oecologia 2001, 129, 461–472. [Google Scholar] [CrossRef]
- Amiot, R.; Lecuyer, C.; Escarguel, G.; Billon-Bruyat, J.-P.; Buffetaut, E.; Langlois, C.; Martin, S.; Martineau, F.; Mazin, J.-M. Oxygen isotope fractionation between crocodilian phosphate and water. Palaeogeogr. Palaeoclim. Palaeoecol. 2007, 243, 412–420. [Google Scholar] [CrossRef]
- Amiot, R.; Wang, X.; Lécuyer, C.; Buffetaut, E.; Boudad, L.; Cavin, L.; Ding, Z.; Fluteau, F.; Kellner, A.W.; Tong, H.; et al. Oxygen and carbon isotope compositions of middle Cretaceous vertebrates from North Africa and Brazil: Ecological and environmental significance. Palaeogeogr. Palaeoclim. Palaeoecol. 2010, 297, 439–451. [Google Scholar] [CrossRef]
- Rohling, E.J. Oxygen Isotope Composition of Seawater. In The Encyclopedia of Quaternary Science; Elias, S.A., Ed.; Elsevier: Amsterdam, The Netherlands, 2013; Volume 2, pp. 915–922. [Google Scholar]
- Zachos, J.; Pagani, M.; Sloan, L.; Thomas, E.; Billups, K. Trends, Rhythms, and Aberrations in Global Climate 65 Ma to Present. Science 2001, 292, 686–693. [Google Scholar] [CrossRef] [PubMed]
- Cramer, B.S.; Miller, K.G.; Barrett, P.J.; Wright, J.D. Late Cretaceous–Neogene trends in deep ocean temperature and continental ice volume: Reconciling records of benthic foraminiferal geochemistry (δ18O and Mg/Ca) with sea level history. J. Geophys. Res. Space Phys. 2011, 116, 12023. [Google Scholar] [CrossRef] [Green Version]
- Abreu, V.S.; Anderson, J.B. Glacial Eustasy During the Cenozoic: Sequence Stratigraphic Implications. AAPG Bull. 1998, 82, 1385–1400. [Google Scholar] [CrossRef]
- Craig, H.; Gordon, L.I. Deuterium and oxygen 18 variation in the ocean and the marine atmosphere. In Proceedings of the Spoleto Conference on Stable Isotopes in Oceanographic Studies and Paleotemperatures; Tongiorgi, E., Ed.; Consiglio Nazionale delle Ricerche: Pisa, Italy, 1965; pp. 1–22. [Google Scholar]
- Bigg, G.R.; Rohling, E.J. An oxygen isotope data set for marine waters. J. Geophys. Res. Space Phys. 2000, 105, 8527–8535. [Google Scholar] [CrossRef]
- Schmidt, G.A.; Bigg, G.R.; Rohling, E.J. Global Seawater Oxygen-18 Database, NASA Goddard Inst. of Space Sci., New York, NY, USA. 1999. Available online: http://data.giss.nasa.gov/o18data/ (accessed on 6 April 2021).
- LeGrande, A.N.; Schmidt, G. Global gridded data set of the oxygen isotopic composition in seawater. Geophys. Res. Lett. 2006, 33, 12604. [Google Scholar] [CrossRef] [Green Version]
- Torniainen, J.; Lensu, A.; Vuorinen, P.J.; Sonninen, E.; Keinänen, M.; Jones, R.I.; Patterson, W.P.; Kiljunen, M. Oxygen and carbon isoscapes for the Baltic Sea: Testing their applicability in fish migration studies. Ecol. Evol. 2017, 7, 2255–2267. [Google Scholar] [CrossRef] [PubMed]
- Craig, H. Isotopic Variations in Meteoric Waters. Science 1961, 133, 1702–1703. [Google Scholar] [CrossRef]
- Dansgaard, W. Stable isotopes in precipitation. Tellus 1964, 16, 436–468. [Google Scholar] [CrossRef]
- Rozanski, K.; Araguás-Araguás, L.; Gonfiantini, R. Isotopic Patterns in Modern Global Precipitation. Magma Redox Geochem. 2013, 78, 1–36. [Google Scholar] [CrossRef]
- Bowen, G.J.; Wilkinson, B. Spatial distribution of δ18O in meteoric precipitation. Geology 2002, 30. [Google Scholar] [CrossRef]
- Zhou, J.; Poulsen, C.; Pollard, D.; White, T.S. Simulation of modern and middle Cretaceous marine δ18O with an ocean-atmosphere general circulation model. Paleoceanography 2008, 23, 3223. [Google Scholar] [CrossRef] [Green Version]
- Roberts, C.D.; LeGrande, A.N.; Tripati, A. Sensitivity of seawater oxygen isotopes to climatic and tectonic boundary conditions in an early Paleogene simulation with GISS ModelE-R. Paleoceanography 2011, 26, 4203. [Google Scholar] [CrossRef] [Green Version]
- Brunetti, M.; Vérard, C.; Baumgartner, P.O. Modeling the Middle Jurassic ocean circulation. J. Palaeogeogr. 2015, 4, 371–383. [Google Scholar] [CrossRef]
- Railsback, L.B.; Anderson, T.F.; Ackerly, S.C.; Cisne, J.L. Paleoceanographic modeling of temperature-salinity profiles from stable isotopic data. Paleoceanography 1989, 4, 585–591. [Google Scholar] [CrossRef]
- Railsback, L. Influence of changing deep ocean circulation on the Phanerozoic oxygen isotopic record. Geochim. Cosmochim. Acta 1990, 54, 1501–1509. [Google Scholar] [CrossRef]
- Woo, K.-S.; Anderson, T.F.; Railsback, L.B.; Sandberg, P.A. Oxygen isotope evidence for high-salinity surface seawater in the Mid-Cretaceous Gulf of Mexico: Implications for warm, saline deepwater formation. Paleoceanography 1992, 7, 673–685. [Google Scholar] [CrossRef]
- Wierzbowski, H.; Dubicka, Z.; Rychliński, T.; Durska, E.; Olempska, E.; Błażejowski, B. Depositional environment of the Owadów-Brzezinki conservation Lagerstätte (uppermost Jurassic, central Poland): Evidence from microfacies analysis, microfossils and geochemical proxies. Neues Jahrb. Geol. Paläontologie Abh. 2016, 282, 81–108. [Google Scholar] [CrossRef] [Green Version]
- Zachos, J.; Stott, L.D.; Lohmann, K. Evolution of Early Cenozoic marine temperatures. Paleoceanography 1994, 9, 353–387. [Google Scholar] [CrossRef]
- Müller, D.; Roest, W.R.; Royer, J.-Y.; Gahagan, L.M.; Sclater, J.G. Digital isochrons of the world’s ocean floor. J. Geophys. Res. Space Phys. 1997, 102, 3211–3214. [Google Scholar] [CrossRef]
- Grossman, E.; Mii, H.-S.; Yancey, T.E. Stable isotopes in Late Pennsylvanian brachiopods from the United States: Implications for Carboniferous paleoceanography. GSA Bull. 1993, 105, 1284–1296. [Google Scholar] [CrossRef]
- Mazzullo, S.J.; Boardman, D.R.; Grossman, E.; Dimmick-Wells, K. Oxygen-carbon isotope stratigraphy of Upper Carboniferous to Lower Permian marine deposits in Midcontinent U.S.A. (Kansas and ne Oklahoma): Implications for sea water chemistry and depositional cyclicity. Carbonates Evaporites 2007, 22, 55–72. [Google Scholar] [CrossRef] [Green Version]
- Dennis, K.; Cochran, J.; Landman, N.; Schrag, D. The climate of the Late Cretaceous: New insights from the application of the carbonate clumped isotope thermometer to Western Interior Seaway macrofossil. Earth Planet. Sci. Lett. 2013, 362, 51–65. [Google Scholar] [CrossRef]
- Alberti, M.; Parent, H.; Garrido, A.C.; Andersen, N.; Garbe-Schönberg, D.; Danise, S. Stable isotopes (δ13C, δ18O) and element ratios (Mg/Ca, Sr/Ca) of Jurassic belemnites, bivalves and brachiopods from the Neuquén Basin (Argentina): Challenges and opportunities for palaeoenvironmental reconstructions. J. Geol. Soc. 2021, 178, 2020–2163. [Google Scholar] [CrossRef]
- Montañez, I.P.; Osleger, D.J.; Chen, J.; Wortham, B.E.; Stamm, R.G.; Nemyrovska, T.I.; Griffin, J.M.; Poletaev, V.I.; Wardlaw, B.R. Carboniferous climate teleconnections archived in coupled bioapatite δ18OPO4 and 87Sr/86Sr records from the epicontinental Donets Basin, Ukraine. Earth Planet. Sci. Lett. 2018, 492, 89–101. [Google Scholar] [CrossRef]
- Yin, J.; Werner, W. Reconstruction of palaeosalinity using carbon isotopes and benthic associations: A comparison. Acta Diabetol. 1995, 84, 223–236. [Google Scholar] [CrossRef]
- Holmden, C.; Hudson, J.D. 87Sr/86Sr and Sr/Ca Investigation of Jurassic mollusks from Scotland: Implications for paleosalinities and the Sr/Ca ratio of seawater. GSA Bull. 2003, 115, 1249. [Google Scholar] [CrossRef]
- Wierzbowski, H. Strontium isotope composition of sedimentary rocks and its application to chemostratigraphy and palaeoenvironmental reconstructions. Ann. Univ. Mariae Curie-SklodowskaSect. AAA Phys. 2013, 68, 23–37. [Google Scholar] [CrossRef]
- Schouten, S.; Hopmans, E.C.; Schefuß, E.; Damste, J.S. Distributional variations in marine crenarchaeotal membrane lipids: A new tool for reconstructing ancient sea water temperatures? Earth Planet. Sci. Lett. 2002, 204, 265–274. [Google Scholar] [CrossRef]
- Herbert, T.D. Alkenone paleotemperature determinations. In Treatise on Geochemistry; Holland, H.D., Turekian, K.K., Eds.; Elsevier: Oxford, UK, 2003; Volume 6, pp. 391–432. [Google Scholar]
- Kim, J.-H.; Schouten, S.; Hopmans, E.C.; Donner, B.; Damste, J.S. Global sediment core-top calibration of the TEX86 paleothermometer in the ocean. Geochim. Cosmochim. Acta 2008, 72, 1154–1173. [Google Scholar] [CrossRef]
- Kim, J.-H.; van der Meer, J.; Schouten, S.; Helmke, P.; Willmott, V.; Sangiorgi, F.; Koç, N.; Hopmans, E.C.; Damste, J.S. New indices and calibrations derived from the distribution of crenarchaeal isoprenoid tetraether lipids: Implications for past sea surface temperature reconstructions. Geochim. Cosmochim. Acta 2010, 74, 4639–4654. [Google Scholar] [CrossRef]
- Sachs, J.P.; Pahnke, K.; Smittenberg, R.; Zhang, Z. Biomarker Indicators of Past Climate. In The Encyclopedia of Quaternary Science; Elias, S.A., Ed.; Elsevier: Amsterdam, The Netherlands, 2013; Volume 2, pp. 775–782. [Google Scholar]
- Holmden, C.; Creaser, R.A.; Muehlenbachs, K.; Leslie, S.A.; Bergström, S.M. Isotopic evidence for geochemical de-coupling between ancient eperic seas and bordering oceans: Implications for secular curves. Geology 1998, 26, 567–570. [Google Scholar] [CrossRef]
- Dera, G.; Pucéat, E.; Pellenard, P.; Neige, P.; Delsate, D.; Joachimski, M.; Reisberg, L.; Martinez, M. Water mass exchange and variations in seawater temperature in the NW Tethys during the Early Jurassic: Evidence from neodymium and oxygen isotopes of fish teeth and belemnites. Earth Planet. Sci. Lett. 2009, 286, 198–207. [Google Scholar] [CrossRef]
- Dera, G.; Prunier, J.; Smith, P.L.; Haggart, J.W.; Popov, E.; Guzhov, A.; Rogov, M.; Delsate, D.; Thies, D.; Cuny, G.; et al. Nd isotope constraints on ocean circulation, paleoclimate, and continental drainage during the Jurassic breakup of Pangea. Gondwana Res. 2015, 27, 1599–1615. [Google Scholar] [CrossRef] [Green Version]
- Moiroud, M.; Pucéat, E.; Donnadieu, Y.; Bayon, G.; Guiraud, M.; Voigt, S.; Deconinck, J.-F.; Monna, F. Evolution of neodymium isotopic signature of seawater during the Late Cretaceous: Implications for intermediate and deep circulation. Gondwana Res. 2016, 36, 503–522. [Google Scholar] [CrossRef] [Green Version]
- Manca, B.; Burca, M.; Giorgetti, A.; Coatanoan, C.; Garcia, M.-J.; Iona, A. (Sissy) Physical and biochemical averaged vertical profiles in the Mediterranean regions: An important tool to trace the climatology of water masses and to validate incoming data from operational oceanography. J. Mar. Syst. 2004, 48, 83–116. [Google Scholar] [CrossRef]
- Judd, E.J.; Bhattacharya, T.; Ivany, L.C. A Dynamical Framework for Interpreting Ancient Sea Surface Temperatures. Geophys. Res. Lett. 2020, 47, e2020GL089044. [Google Scholar] [CrossRef]
- Wierzbowski, H.; Dembicz, K.; Praszkier, T. Oxygen and carbon isotope composition of Callovian–Lower Oxfordian (Middle–Upper Jurassic) belemnite rostra from central Poland: A record of a Late Callovian global sea-level rise? Palaeogeogr. Palaeoclim. Palaeoecol. 2009, 283, 182–194. [Google Scholar] [CrossRef]
- Matyja, B.A.; Wierzbowski, A. Sea-bottom relief and bathymetry of Late Jurassic sponge megafacies in Central Poland. In Advances in Jurassic Research; Riccardi, A.C., Ed.; Trans Tech Publications: Baech, Switzerland, 1996; pp. 333–340. [Google Scholar]
- Pisera, A. Upper Jurassic siliceous sponges from Swabian Alb: Taxonomy and paleoecology. Paleontol. Pol. 1997, 57, 1–216. [Google Scholar]
- Wierzbowski, A.; Matyja, B.A.; Sobieraj, K. Stop 1.4. Julianka, coral colonization of the cyanobacteria-sponge bio-herms at the turn of the Oxfordian and Kimmeridgian. In Guide Book and Abstracts, Oxfordian and Kimmeridgian Joint Working Groups Meeting, Warszawa and Central Polish Uplands, September 7–12, 1992; Matyja, B.A., Wierzbowski, A., Radwański, A., Eds.; Verlag nicht ermittelbar: Warszawa, Poland, 1992; pp. 37–40. [Google Scholar]
- Matyja, B.A. Płytkowodna platforma węglanowa późnej jury na południowo-zachodnim obrzeżeniu Gór Świętokrzyskich (in Polish). In Jurassica IX, Małogoszcz, 6–8 września 2011. Materiały Konferencyjne; Polskie Towarzystwo Geologiczne—Polska Grupa Robocza Systemu Jurajskiego: Kraków, Poland, 2011; pp. 133–151. [Google Scholar]
- Wierzbowski, A. The Lower Kimmeridgian of the Wieluń Upland and adjoining regions in central Poland: Lithostratigraphy, ammonite stratigraphy (upper Planula/Platynota to Divisum zones), palaeogeography and climate-controlled cycles. Vol. Jurass. 2017, 15, 49–120. [Google Scholar] [CrossRef]
- Wierzbowski, A. The Kimmeridgian of the south-western margin of the Holy Cross Mts., central Poland: Stratigraphy and facies development. Part I. From deep-neritic sponge megafacies to shallow water carbonates. Vol. Jurass. 2020, 18, 161–234. [Google Scholar] [CrossRef]
- Gutowski, J. Field trip B2—Upper Jurassic shallow-water carbonate platform and open shelf facies. Introduction. In Jurassic of Poland and adjacent Slovakian Carpathians. Field Trip Guidebook 7th Interntational Congress on Jurassic System; Wierzbowski, A., Aubrecht, R., Golonka, J., Gutowski, J., Krobicki, M., Matyja, B.A., Pieńkowski, G., Uchman, A., Eds.; Polish Geological Institute: Kraków, Poland, 2006; pp. 169–173. [Google Scholar]
- Matyja, B.A.; Wierzbowski, A. Monografia Górnej Jury Pasma Krakowsko-Wieluńskiego. Projekt Badawczy KBN nr 600799101; 1994; pp. 1–39, (unpublished report). [Google Scholar]
- Norris, M.S.; Hallam, A. Facies variations across the Middle-Upper Jurassic boundary in Western Europe and the relationship to sea-level changes. Palaeogeogr. Palaeoclim. Palaeoecol. 1995, 116, 189–245. [Google Scholar] [CrossRef]
- Hallam, A. A review of the broad pattern of Jurassic sea-level changes and their possible causes in the light of current knowledge. Palaeogeogr. Palaeoclim. Palaeoecol. 2001, 167, 23–37. [Google Scholar] [CrossRef]
- Dromart, G.; Garcia, J.-P.; Gaumet, F.; Picard, S.; Rousseau, M.; Atrops, F.; Lécuyer, C.; Sheppard, S.M.F. Perturbation of the carbon cycle at the Middle/Late Jurassic transition: Geological and geochemical evidence. Am. J. Sci. 2003, 303, 667–707. [Google Scholar] [CrossRef]
- Dromart, G.; Garcia, J.-P.; Picard, S.; Atrops, F.; Lecuyer, C.; Sheppard, S. Ice age at the Middle–Late Jurassic transition? Earth Planet. Sci. Lett. 2003, 213, 205–220. [Google Scholar] [CrossRef]
- Nunn, E.V.; Price, G.; Hart, M.B.; Page, K.N.; Leng, M. Isotopic signals from Callovian–Kimmeridgian (Middle–Upper Jurassic) belemnites and bulk organic carbon, Staffin Bay, Isle of Skye, Scotland. J. Geol. Soc. 2009, 166, 633–641. [Google Scholar] [CrossRef]
- Dera, G.; Brigaud, B.; Monna, F.; Laffont, R.; Pucéat, E.; Deconinck, J.-F.; Pellenard, P.; Joachimski, M.; Durlet, C. Climatic ups and downs in a disturbed Jurassic world. Geology 2011, 39, 215–218. [Google Scholar] [CrossRef]
- Price, G.D.; Gröcke, D.R. Strontium-isotope stratigraphy and oxygen- and carbon-isotope variation during the Middle Jurassic–Early Cretaceous of the Falkland Plateau, South Atlantic. Palaeogeogr. Palaeoclim. Palaeoecol. 2002, 183, 209–222. [Google Scholar] [CrossRef]
- Jenkyns, H.C.; Schouten-Huibers, L.; Schouten, S.; Damsté, J.S.S. Warm Middle Jurassic–Early Cretaceous high-latitude sea-surface temperatures from the Southern Ocean. Clim. Past 2012, 8, 215–226. [Google Scholar] [CrossRef] [Green Version]
- Dutton, A.; Huber, B.T.; Lohmann, K.; Zinsmeister, W.J. High-resolution stable isotope profiles of a dimitobelid belemnite: Implications for paleodepth habitat and late maastrichtian climate seasonality. Palaios 2007, 22, 642–650. [Google Scholar] [CrossRef]
- Wierzbowski, H.; Joachimski, M. Stable isotopes, elemental distribution, and growth rings of belemnopsid belemnite rostra: Proxies for belemnite life habitat. Palaios 2009, 24, 377–386. [Google Scholar] [CrossRef]
- Nützel, A.; Joachimski, M.; Correa, M.L. Seasonal climatic fluctuations in the Late Triassic tropics—High-resolution oxygen isotope records from aragonitic bivalve shells (Cassian Formation, Northern Italy). Palaeogeogr. Palaeoclim. Palaeoecol. 2010, 285, 194–204. [Google Scholar] [CrossRef]
- Stevens, G.R.; Clayton, R.N. Oxygen isotope studies on Jurassic and Cretaceous belemnites from New Zealand and their biogeographic significance. N. Z. J. Geol. Geophys. 1971, 14, 829–897. [Google Scholar] [CrossRef] [Green Version]
- Ullmann, C.; Frei, R.; Korte, C.; Hesselbo, S. Chemical and isotopic architecture of the belemnite rostrum. Geochim. Cosmochim. Acta 2015, 159, 231–243. [Google Scholar] [CrossRef] [Green Version]
- Wierzbowski, H. Life span and growth rate of Middle Jurassic mesohibolitid belemnites deduced from rostrum microincrements. Vol. Jurass. 2013, 11, 1–18. [Google Scholar]
- Rexfort, A.; Mutterlose, J. Stable isotope records from Sepia officinalis—a key to understanding the ecology of belemnites? Earth Planet. Sci. Lett. 2006, 247, 212–221. [Google Scholar] [CrossRef]
- Bettencourt, V.; Guerra, A. Carbon- and oxygen-isotope composition of the cuttlebone of Sepia officinalis: A tool for predicting ecological information? Mar. Biol. 1999, 133, 651–657. [Google Scholar] [CrossRef]
- Rollion-Bard, C.; Erez, J.; Zilberman, T. Intra-shell oxygen isotope ratios in the benthic foraminifera genus Amphistegina and the influence of seawater carbonate chemistry and temperature on this ratio. Geochim. Cosmochim. Acta 2008, 72, 6006–6014. [Google Scholar] [CrossRef]
- Valley, J.W.; Kita, N.T. In situ oxygen isotope geochemistry by ion microprobe. In Secondary Ion Mass Spectrometry in the Earth Sciences: Gleaning the Big Picture from a Small Spot; Fayek, M., Ed.; Mineralogical Association of Canada Short Course 41: Toronto, ON, Canada, 2009; pp. 19–63. [Google Scholar]
- Žigaitė, Ž.; Whitehouse, M. Stable oxygen isotopes of dental biomineral: Differentiation at the intra- and inter-tissue level of modern shark teeth. GFF 2014, 136, 337–340. [Google Scholar] [CrossRef]
- Narkiewicz, M.; Narkiewicz, K.; Krzeminska, E.; Kruchek, S.A. Oxygen isotopic composition of conodont apatite in the equatorial epeiric Belarussian basin (Eifelian)—Relationship to fluctuating seawater salinity and temperature. Palaios 2017, 32, 439–447. [Google Scholar] [CrossRef]
- Kozdon, R.; Ushikubo, T.; Kita, N.; Spicuzza, M.; Valley, J. Intratest oxygen isotope variability in the planktonic foraminifer N. pachyderma: Real vs. apparent vital effects by ion microprobe. Chem. Geol. 2009, 258, 327–337. [Google Scholar] [CrossRef]
- Kozdon, R.; Kelly, D.C.; Kitajima, K.; Strickland, A.; Fournelle, J.H.; Valley, J.W. In situ δ18O and Mg/Ca analyses of diagenetic and planktic foraminiferal calcite preserved in a deep-sea record of the Paleocene-Eocene thermal maximum. Paleoceanography 2013, 28, 517–528. [Google Scholar] [CrossRef]
- Wycech, J.B.; Kelly, C.; Kitajima, K.; Kozdon, R.; Orland, I.J.; Valley, J.W. Combined effects of gametogenic calcification and dissolution on δ18O measurements of the planktic foraminifer Trilobatus sacculifer. Geochem. Geophys. Geosyst. 2018, 19, 4487–4501. [Google Scholar] [CrossRef]
- Wycech, J.B.; Kelly, D.C.; Kozdon, R.; Orland, I.J.; Spero, H.J.; Valley, J.W. Comparison of δ18O analyses on individual planktic foraminifer (Orbulina universa) shells by SIMS and gas-source mass spectrometry. Chem. Geol. 2018, 483, 119–130. [Google Scholar] [CrossRef] [Green Version]
- Kilburn, M.R.; Wacey, D. NanoSIMS as an analytical tool in the geosciences. In Principles and Practice of Analytical Techniques in Geosciences, Royal Society of Chemistry Special Volume; Grice, K., Ed.; Royal Society of Chemistry: London, UK, 2015; pp. 1–34. [Google Scholar] [CrossRef]
- Rollion-Bard, C.; Mangin, D.; Champenois, M. Development and application of oxygen and carbon isotopic measurements of biogenic carbonates by ion microprobe. Geostand. Geoanal. Res. 2007, 31, 39–50. [Google Scholar] [CrossRef]
- Helser, T.E.; Kastelle, C.R.; McKay, J.L.; Orland, I.J.; Kozdon, R.; Valley, J. Evaluation of micromilling/conventional isotope ratio mass spectrometry and secondary ion mass spectrometry of δ18O values in fish otoliths for sclerochronology. Rapid Commun. Mass Spectrom. 2018, 32, 1781–1790. [Google Scholar] [CrossRef] [PubMed]
- Balestra, B.; Orland, I.J.; Fessenden-Rahn, J.; Gorski, G.; Franks, R.; Rahn, T.; Paytan, A. Paired analyses of oxygen isotope and elemental ratios within individual shells of benthic foraminifera genus Uvigerina. Chem. Geol. 2020, 533, 119377. [Google Scholar] [CrossRef]
- Rollion-Bard, C.; Marin-Carbonne, J. Determination of SIMS matrix effects on oxygen isotopic compositions in carbonates. J. Anal. At. Spectrom. 2011, 26, 1285–1289. [Google Scholar] [CrossRef]
- Trotter, J.A.; Williams, I.S.; Barnes, C.R.; Lécuyer, C.; Nicoll, R.S. Did cooling oceans trigger Ordovician biodiversification? Evidence from Conodont Thermometry. Science 2008, 321, 550–554. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Tang, G.-Q.; Liu, Y.; He, S.; Chen, B.; Li, Q.-L.; Li, X.-H. Revisiting apatite SIMS oxygen isotope analysis and Qinghu-AP reference material. Chem. Geol. 2021, 582, 120445. [Google Scholar] [CrossRef]
- Trotter, J.; Williams, I.; Nicora, A.; Mazza, M.; Rigo, M. Long-term cycles of Triassic climate change: A new δ18O record from conodont apatite. Earth Planet. Sci. Lett. 2015, 415, 165–174. [Google Scholar] [CrossRef]
- Pietzner, H.; Vahl, J.; Werner, H.; Ziegler, W. Zur chemischen Zussamensetzung und Mikromorphologie der Conodonten. Palaeontogr. Abt. A 1968, 128, 115–152. [Google Scholar]
- Vennemann, T.; Hegner, E.; Cliff, G.; Benz, G. Isotopic composition of recent shark teeth as a proxy for environmental conditions. Geochim. Cosmochim. Acta 2001, 65, 1583–1599. [Google Scholar] [CrossRef]
- Aubert, M.; Williams, I.S.; Boljkovac, K.; Moffat, I.; Moncel, M.-H.; Dufour, E.; Grün, R. In situ oxygen isotope micro-analysis of faunal material and human teeth using a SHRIMP II: A new tool for palaeo-ecology and archaeology. J. Archaeol. Sci. 2012, 39, 3184–3194. [Google Scholar] [CrossRef]
- Wheeley, J.; Jardine, P.E.; Raine, R.; Boomer, I.; Smith, M.P. Paleoecologic and paleoceanographic interpretation of δ18O variability in Lower Ordovician conodont species. Geology 2018, 46, 467–470. [Google Scholar] [CrossRef] [Green Version]
- Ghosh, P.; Adkins, J.; Affek, H.; Balta, B.; Guo, W.; Schauble, E.A.; Schrag, D.; Eiler, J.M. 13C–18O bonds in carbonate minerals: A new kind of paleothermometer. Geochim. Cosmochim. Acta 2006, 70, 1439–1456. [Google Scholar] [CrossRef]
- Eiler, J.M. Paleoclimate reconstruction using carbonate clumped isotope thermometry. Quat. Sci. Rev. 2011, 30, 3575–3588. [Google Scholar] [CrossRef]
- Passey, B.H.; Henkes, G.A. Carbonate clumped isotope bond reordering and geospeedometry. Earth Planet. Sci. Lett. 2012, 351-352, 223–236. [Google Scholar] [CrossRef]
- Henkes, G.A.; Passey, B.; Grossman, E.; Shenton, B.J.; Pérez-Huerta, A.; Yancey, T.E. Temperature limits for preservation of primary calcite clumped isotope paleotemperatures. Geochim. Cosmochim. Acta 2014, 139, 362–382. [Google Scholar] [CrossRef]
- Stolper, D.A.; Eiler, J.M. The kinetics of solid-state isotope-exchange reactions for clumped isotopes: A study of inorganic calcites and apatites from natural and experimental samples. Am. J. Sci. 2015, 315, 363–411. [Google Scholar] [CrossRef]
- Spencer, C.; Kim, S.-T. Carbonate clumped isotope paleothermometry: A review of recent advances in CO2 gas evolution, purification, measurement and standardization techniques. Geosci. J. 2015, 19, 357–374. [Google Scholar] [CrossRef]
- Tripati, A.; Eagle, R.; Thiagarajan, N.; Gagnon, A.C.; Bauch, H.; Halloran, P.; Eiler, J.M. 13C–18O isotope signatures and ‘clumped isotope’ thermometry in foraminifera and coccoliths. Geochim. Cosmochim. Acta 2010, 74, 5697–5717. [Google Scholar] [CrossRef]
- Thiagarajan, N.; Adkins, J.; Eiler, J. Carbonate clumped isotope thermometry of deep-sea corals and implications for vital effects. Geochim. Cosmochim. Acta 2011, 75, 4416–4425. [Google Scholar] [CrossRef] [Green Version]
- Grauel, A.-L.; Schmid, T.W.; Hu, B.; Bergami, C.; Capotondi, L.; Zhou, L.; Bernasconi, S. Calibration and application of the ‘clumped isotope’ thermometer to foraminifera for high-resolution climate reconstructions. Geochim. Cosmochim. Acta 2013, 108, 125–140. [Google Scholar] [CrossRef]
- Henkes, G.A.; Passey, B.; Wanamaker, A.; Grossman, E.; Ambrose, W.G.; Carroll, M.L. Carbonate clumped isotope compositions of modern marine mollusk and brachiopod shells. Geochim. Cosmochim. Acta 2013, 106, 307–325. [Google Scholar] [CrossRef]
- Eagle, R.A.; Eiler, J.M.; Tripati, A.K.; Ries, J.B.; Freitas, P.S.; Hiebenthal, C.; Wanamaker, A.D.; Taviani, M.; Elliot, M.; Marenssi, S.; et al. The influence of temperature and seawater carbonate saturation state on 13C–18O bond ordering in bivalve mollusks. Biogeosciences 2013, 10, 4591–4606. [Google Scholar] [CrossRef] [Green Version]
- Zaarur, S.; Affek, H.P.; Brandon, M.T. A revised calibration of the clumped isotope thermometer. Earth Planet. Sci. Lett. 2013, 382, 47–57. [Google Scholar] [CrossRef]
- Wacker, U.; Fiebig, J.; Tödter, J.; Schöne, B.R.; Bahr, A.; Friedrich, O.; Tütken, T.; Gischler, E.; Joachimski, M. Empirical calibration of the clumped isotope paleothermometer using calcites of various origins. Geochim. Cosmochim. Acta 2014, 141, 127–144. [Google Scholar] [CrossRef]
- Kele, S.; Breitenbach, S.F.; Capezzuoli, E.; Meckler, A.N.; Ziegler, M.; Millan, I.M.; Kluge, T.; Deák, J.; Hanselmann, K.; John, C.; et al. Temperature dependence of oxygen- and clumped isotope fractionation in carbonates: A study of travertines and tufas in the 6–95°C temperature range. Geochim. Cosmochim. Acta 2015, 168, 172–192. [Google Scholar] [CrossRef]
- Bonifacie, M.; Calmels, D.; Eiler, J.M.; Horita, J.; Chaduteau, C.; Vasconcelos, C.; Agrinier, P.; Katz, A.; Passey, B.H.; Ferry, J.M.; et al. Calibration of the dolomite clumped isotope thermometer from 25 to 350 °C, and implications for a universal calibration for all (Ca, Mg, Fe)CO3 carbonates. Geochim. Cosmochim. Acta 2017, 200, 255–279. [Google Scholar] [CrossRef] [Green Version]
- Kelson, J.R.; Huntington, K.W.; Schauer, A.; Saenger, C.; Lechler, A.R. Toward a universal carbonate clumped isotope calibration: Diverse synthesis and preparatory methods suggest a single temperature relationship. Geochim. Cosmochim. Acta 2017, 197, 104–131. [Google Scholar] [CrossRef]
- Bernasconi, S.M.; Müller, I.A.; Bergmann, K.D.; Breitenbach, S.F.M.; Fernandez, A.; Hodell, D.A.; Jaggi, M.; Meckler, A.N.; Millan, I.; Ziegler, M. Reducing uncertainties in carbonate clumped isotope analysis through consistent carbonate-based standardization. Geochem. Geophys. Geosyst. 2018, 19, 2895–2914. [Google Scholar] [CrossRef] [Green Version]
- Petersen, S.V.; Defliese, W.F.; Saenger, C.; Daëron, M.; Huntington, K.W.; John, C.M.; Kelson, J.R.; Bernasconi, S.M.; Colman, A.S.; Kluge, T.; et al. Effects of Improved 17O Correction on Interlaboratory Agreement in Clumped Isotope Calibrations, Estimates of Mineral-Specific Offsets, and Temperature Dependence of Acid Digestion Fractionation. Geochem. Geophys. Geosyst. 2019, 20, 3495–3519. [Google Scholar] [CrossRef] [Green Version]
- Bajnai, D.; Guo, W.; Spötl, C.; Coplen, T.B.; Methner, K.; Löffler, N.; Krsnik, E.; Gischler, E.; Hansen, M.; Henkel, D.; et al. Dual clumped isotope thermometry resolves kinetic biases in carbonate formation temperatures. Nat. Commun. 2020, 11, 4005. [Google Scholar] [CrossRef]
- Meinicke, N.; Ho, S.; Hannisdal, B.; Nürnberg, D.; Tripati, A.; Schiebel, R.; Meckler, A.N. A robust calibration of the clumped isotopes to temperature relationship for foraminifers. Geochim. Cosmochim. Acta 2020, 270, 160–183. [Google Scholar] [CrossRef]
- Staudigel, P.; Swart, P. Isotopic behavior during the aragonite-calcite transition: Implications for sample preparation and proxy interpretation. Chem. Geol. 2016, 442, 130–138. [Google Scholar] [CrossRef]
- Ritter, A.; Mavromatis, V.; Dietzel, M.; Kwiecien, O.; Wiethoff, F.; Griesshaber, E.; Casella, L.A.; Schmahl, W.W.; Koelen, J.; Neuser, R.D.; et al. Exploring the impact of diagenesis on (isotope) geochemical and microstructural alteration features in biogenic aragonite. Sedimentology 2017, 64, 1354–1380. [Google Scholar] [CrossRef]
- Leutert, T.J.; Sexton, P.F.; Tripati, A.; Piasecki, A.; Ho, S.L.; Meckler, A.N. Sensitivity of clumped isotope temperatures in fossil benthic and planktic foraminifera to diagenetic alteration. Geochim. Cosmochim. Acta 2019, 257, 354–372. [Google Scholar] [CrossRef]
- Pepper, A.S.; Corvi, P.J. Simple kinetic models of petroleum formation. Part I: Oil and gas generation from kerogen. Mar. Pet. Geol. 1995, 12, 291–319. [Google Scholar] [CrossRef]
- Finnegan, S.; Bergmann, K.; Eiler, J.M.; Jones, D.S.; Fike, D.A.; Eisenman, I.; Hughes, N.C.; Tripati, A.K.; Fischer, W.W. The magnitude and duration of Late Ordovician-Early Silurian glaciation. Science 2011, 331, 903–906. [Google Scholar] [CrossRef] [Green Version]
- Petersen, S.; Tabor, C.R.; Lohmann, K.C.; Poulsen, C.; Meyer, K.W.; Carpenter, S.J.; Erickson, J.M.; Matsunaga, K.K.; Smith, S.; Sheldon, N. Temperature and salinity of the Late Cretaceous Western Interior Seaway. Geology 2016, 44, 903–906. [Google Scholar] [CrossRef] [Green Version]
- Saenger, C.; Affek, H.P.; Felis, T.; Thiagarajan, N.; Lough, J.M.; Holcomb, M. Carbonate clumped isotope variability in shallow water corals: Temperature dependence and growth-related vital effects. Geochim. Cosmochim. Acta 2012, 99, 224–242. [Google Scholar] [CrossRef]
- Tripati, A.K.; Hill, P.S.; Eagle, R.A.; Mosenfelder, J.L.; Tang, J.; Schauble, E.A.; Eiler, J.M.; Zeebe, R.E.; Uchikawa, J.; Coplen, T.B.; et al. Beyond temperature: Clumped isotope signatures in dissolved inorganic carbon species and the influence of solution chemistry on carbonate mineral composition. Geochim. Cosmochim. Acta 2015, 166, 344–371. [Google Scholar] [CrossRef] [Green Version]
- Kimball, J.; Eagle, R.; Dunbar, R. Carbonate “clumped” isotope signatures in aragonitic scleractinian and calcitic gorgonian deep-sea corals. Biogeosciences 2016, 13, 6487–6505. [Google Scholar] [CrossRef] [Green Version]
- Price, G.; Passey, B. Dynamic polar climates in a greenhouse world: Evidence from clumped isotope thermometry of Early Cretaceous belemnites. Geology 2013, 41, 923–926. [Google Scholar] [CrossRef]
- Tobin, T.S.; Wilson, G.P.; Eiler, J.M.; Hartman, J.H. Environmental change across a terrestrial Cretaceous-Paleogene boundary section in eastern Montana, USA, constrained by carbonate clumped isotope paleothermometry. Geology 2014, 42, 351–354. [Google Scholar] [CrossRef]
- Evans, D.; Sagoo, N.; Renema, W.; Cotton, L.J.; Müller, W.; Todd, J.A.; Saraswati, P.K.; Stassen, P.; Ziegler, M.; Pearson, P.N.; et al. Eocene greenhouse climate revealed by coupled clumped isotope-Mg/Ca thermometry. Proc. Natl. Acad. Sci. USA 2018, 115, 1174–1179. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Price, G.D.; Bajnai, D.; Fiebig, J. Carbonate clumped isotope evidence for latitudinal seawater temperature gradients and the oxygen isotope composition of Early Cretaceous seas. Palaeogeogr. Palaeoclim. Palaeoecol. 2020, 552, 109777. [Google Scholar] [CrossRef]
- Tesakova, E. Late Callovian and Early Oxfordian ostracods from the Dubki section (Saratov area, Russia): Implications for stratigraphy, paleoecology, eustatic cycles and palaeobiogeography. Neues Jahrb. Geol. Paläontologie Abh. 2008, 249, 25–45. [Google Scholar] [CrossRef]
- Głowniak, E.; Kiselev, D.N.; Rogov, M.; Wierzbowski, A.; Wright, J.K. The Middle Oxfordian to lowermost Kimmeridgian ammonite succession at Mikhalenino (Kostroma District) of the Russian Platform, and its stratigraphical and palaeobiogeographical importance. Vol. Jurass. 2010, 8, 5–48. [Google Scholar]
- Tesakova, E.M.; Demidov, S.M.; Guzhov, A.V.; Rogov, M.; Kiselev, D. Middle Oxfordian—Lower Kimmeridgian ostracod zones from the Mikhalenino section (Kostroma region) and their comparison with synchronous strata of the Eastern and Western Europe. Neues Jahrb. Geol. Paläontologie Abh. 2012, 266, 239–249. [Google Scholar] [CrossRef] [Green Version]
- Ustinova, M.A. Foraminifers and stratigraphy of Middle Oxfordian—Lower Kimmeridgian of Kostroma Region (Mikhalenino section). Bull. Moscow Soc. Nat. 2012, 87, 43–52. (In Russian) [Google Scholar]
- Colpaert, C.; Nikitenko, B.; Khafaeva, S.; Wall, A.F. The evolution of Late Callovian to Early Kimmeridgian foraminiferal associations from the central part of the Russian Sea (Makar’yev section, Volga River Basin, Russia). Palaeogeogr. Palaeoclim. Palaeoecol. 2016, 451, 97–109. [Google Scholar] [CrossRef]
- Donnadieu, Y.; Dromart, G.; Goddéris, Y.; Pucéat, E.; Brigaud, B.; Dera, G.; Dumas, C.; Olivier, N. A mechanism for brief glacial episodes in the Mesozoic greenhouse. Paleoceanography 2011, 26, 3212. [Google Scholar] [CrossRef] [Green Version]
- Wierzbowski, H.; Rogov, M. Reconstructing the palaeoenvironment of the Middle Russian Sea during the Middle–Late Jurassic transition using stable isotope ratios of cephalopod shells and variations in faunal assemblages. Palaeogeogr. Palaeoclim. Palaeoecol. 2011, 299, 250–264. [Google Scholar] [CrossRef]


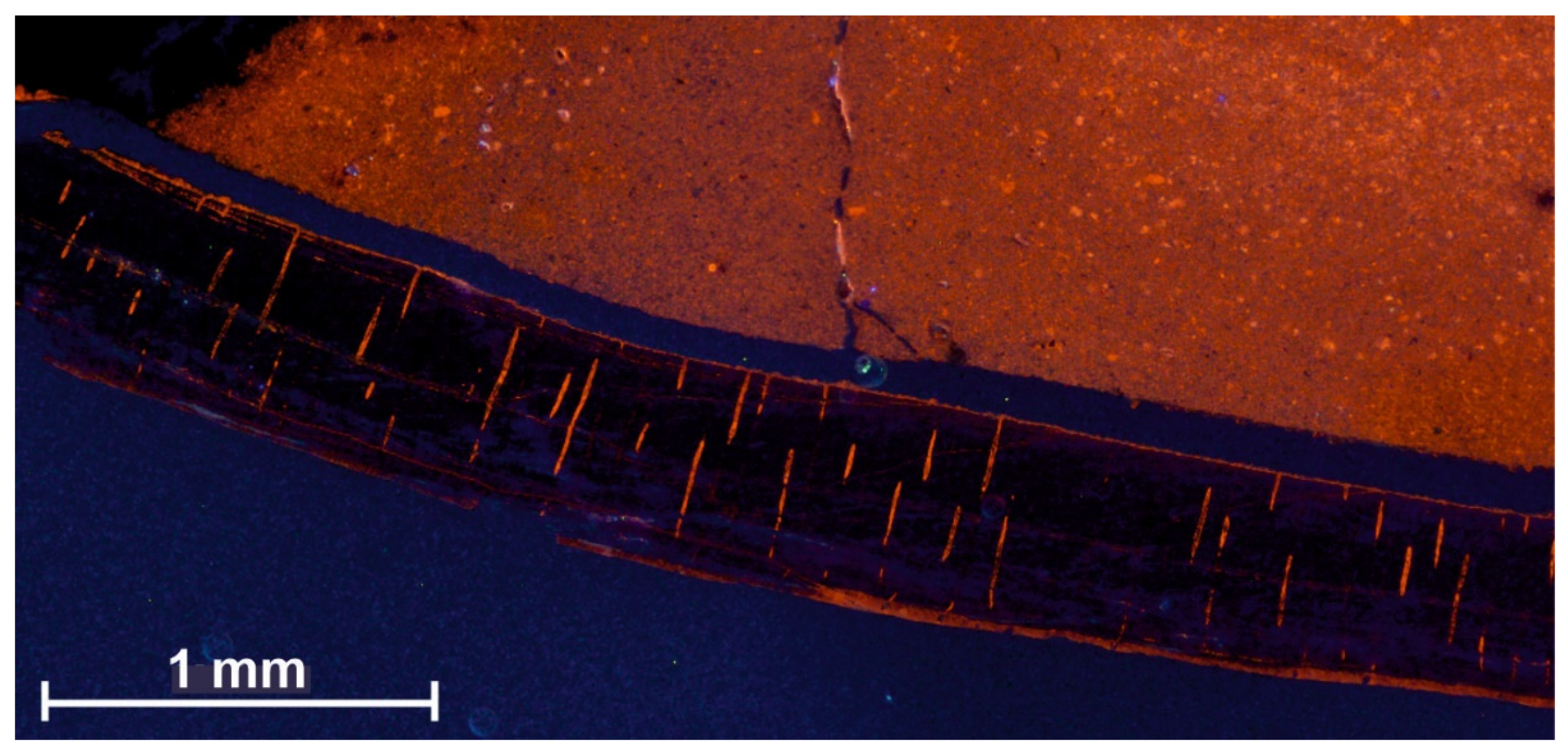



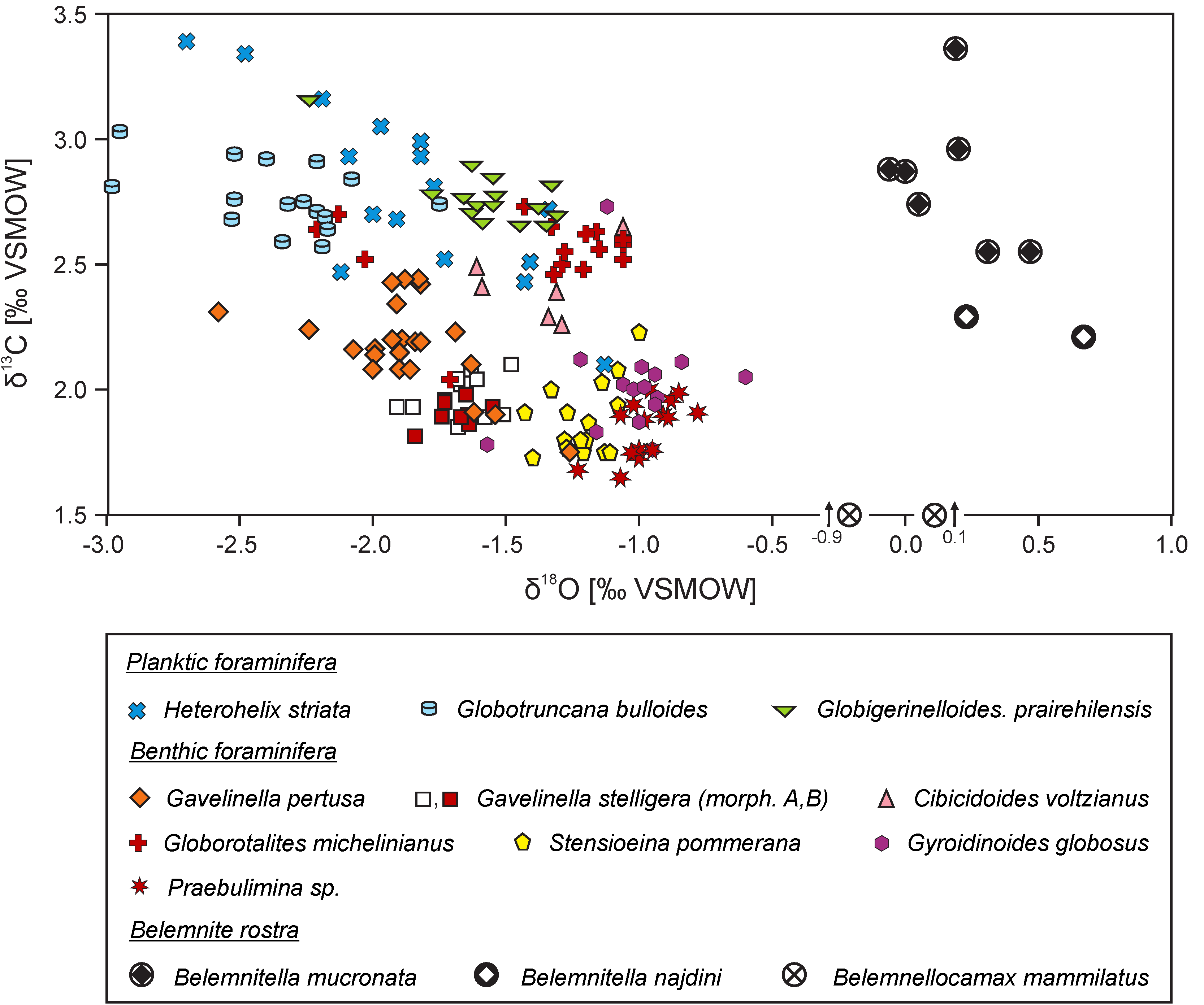

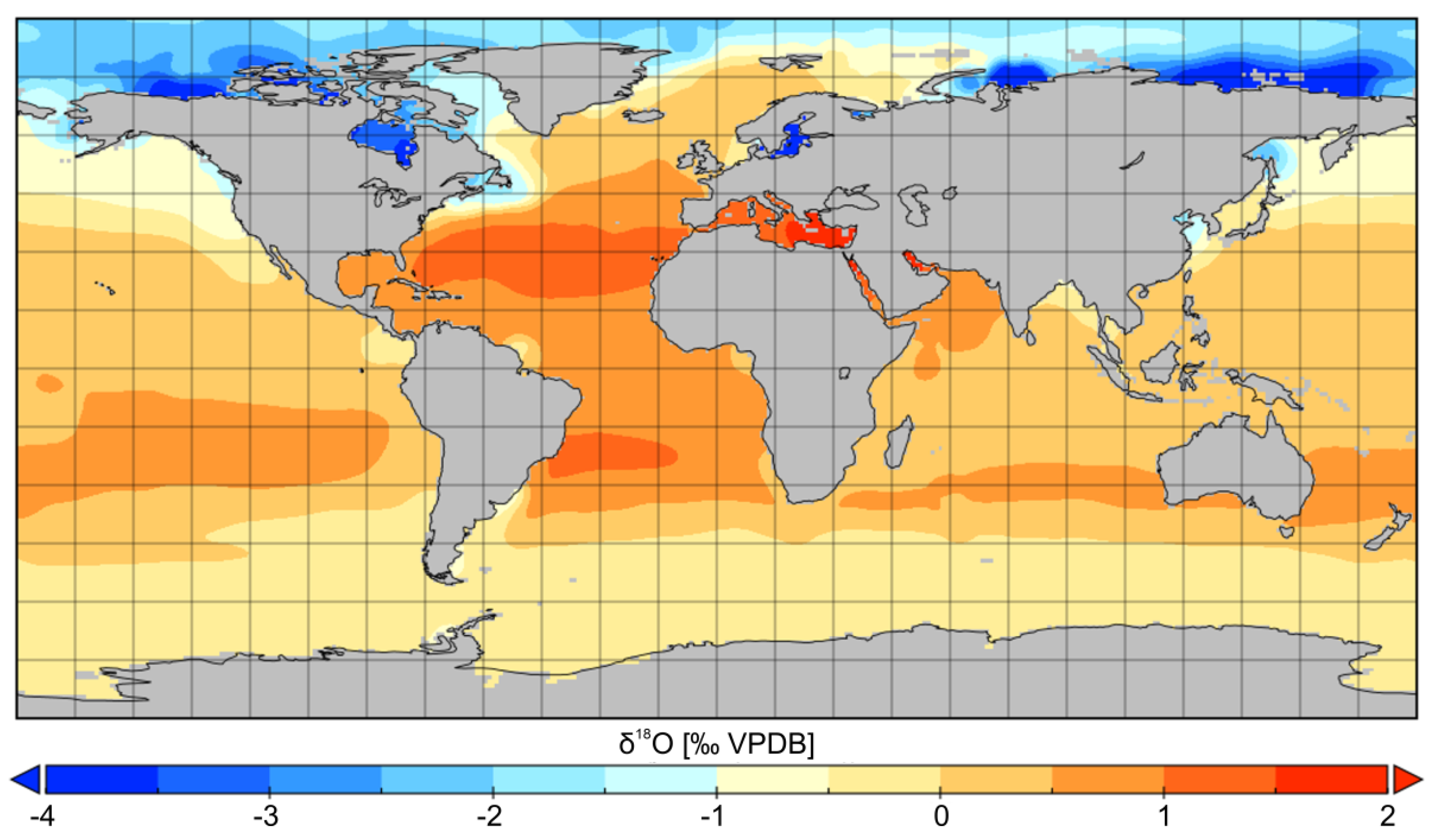
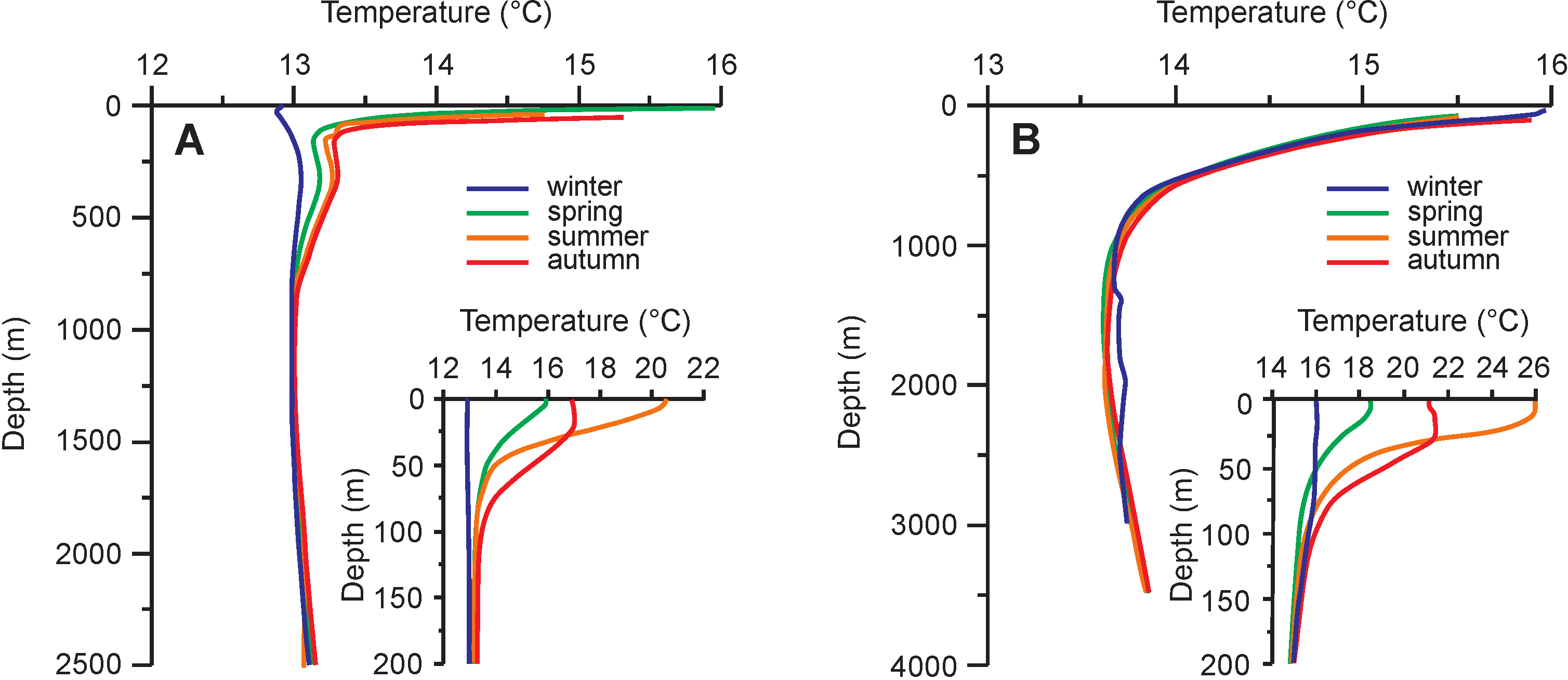

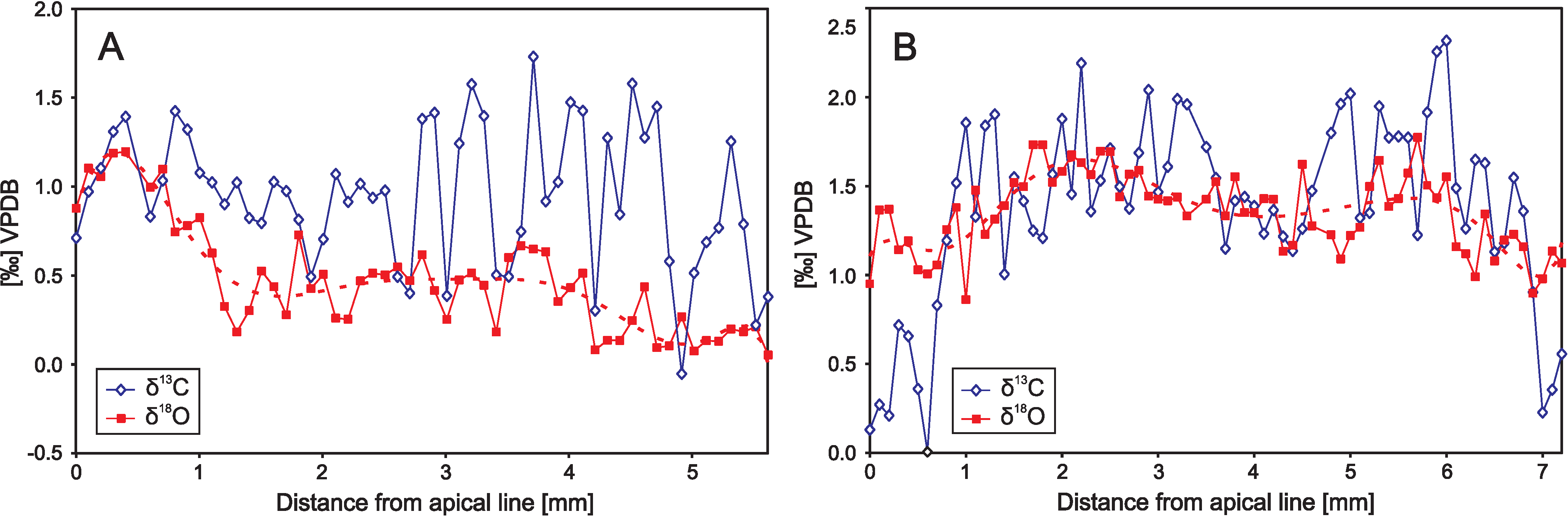
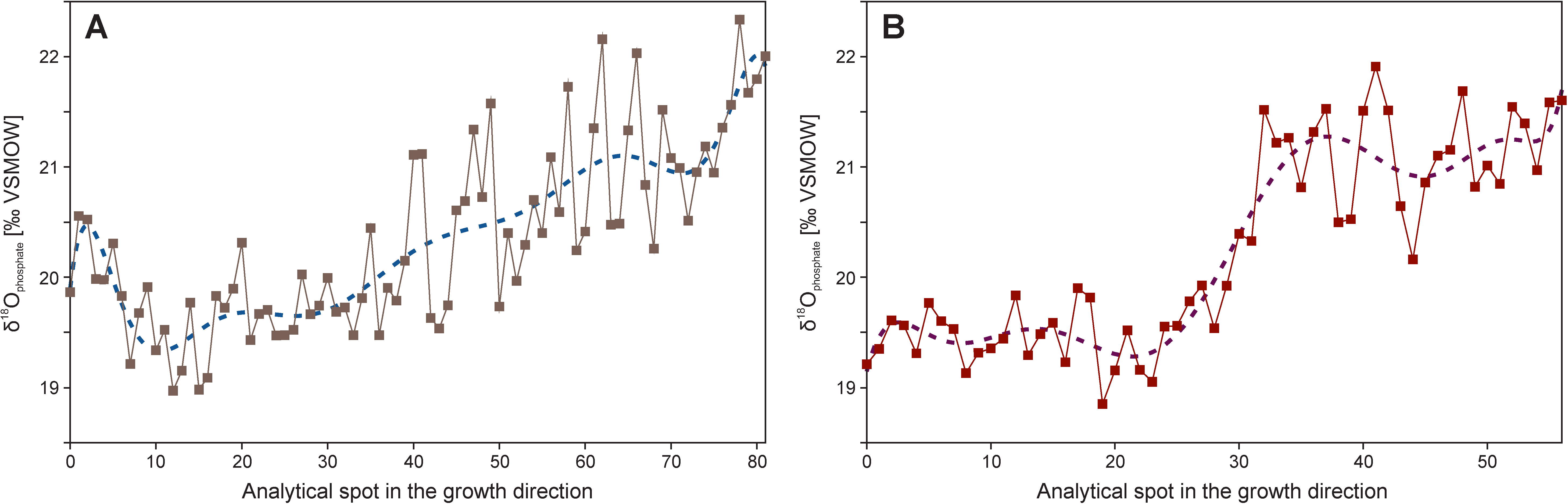

| Mn (ppm) | Fe (ppm) | Sr (ppm) | |
|---|---|---|---|
| Veizer et al. [26] | <500 | - | ≥800 |
| Anderson et al. [46] | <100 | <1000 | - |
| Jones et al. [47] | <50 | <150 | |
| Price et al. [48] | <100 | <250 | - |
| Rosales et al. [49] | <32 | <250 | >950 |
| Gröcke et al. [50] | <100 | <200 | - |
| Rosales et al. [51] | - | <250 | ≥900 |
| Voigt et al. [52] | <100 | <500 | - |
| Price and Mutterlose [53], Price and Rogov [54], Nunn and Price [40] | <100 | <150 | - |
| Wierzbowski et al. [55] | <100 | <200 | ≥800 |
| Alberti et al. [56] | <50 | <300 | ≥800 |
| Alberti et al. [57] | <100 | <300 | >800 |
| Wierzbowski [58], Arabas [59] | <100 | <200 | ≥900 |
| Korte and Hesselbo [60] | <250 | - | ≥400 |
| Žak et al. [61] | <150 | - | ≥900 |
| Mn (ppm) | Fe (ppm) | Sr (ppm) | |
|---|---|---|---|
| Joachimski et al. [62], van Geldern et al. [63] | <100 | <400 | >500 |
| Korte et al. [64,65], Korte and Hesselbo [60] | <250 | - | >400 |
| Armendáriz et al. [66] | <60 | <300 | - |
| Voigt et al. [52] | <100 | <500 | - |
| Bruckschen and Veizer [67] | <350 | - | >600 |
| Bruckschen et al. [68] | <200 | - | - |
| Zuo et al. [69] | <100 | <800 | >700 |
| Korte et al. [65] | <250 | - | >400 |
| Angiolini et al. [70] | <200 | - | - |
| Wierzbowski [58] | <100 | <300 | >340 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wierzbowski, H. Advances and Challenges in Palaeoenvironmental Studies Based on Oxygen Isotope Composition of Skeletal Carbonates and Phosphates. Geosciences 2021, 11, 419. https://doi.org/10.3390/geosciences11100419
Wierzbowski H. Advances and Challenges in Palaeoenvironmental Studies Based on Oxygen Isotope Composition of Skeletal Carbonates and Phosphates. Geosciences. 2021; 11(10):419. https://doi.org/10.3390/geosciences11100419
Chicago/Turabian StyleWierzbowski, Hubert. 2021. "Advances and Challenges in Palaeoenvironmental Studies Based on Oxygen Isotope Composition of Skeletal Carbonates and Phosphates" Geosciences 11, no. 10: 419. https://doi.org/10.3390/geosciences11100419
APA StyleWierzbowski, H. (2021). Advances and Challenges in Palaeoenvironmental Studies Based on Oxygen Isotope Composition of Skeletal Carbonates and Phosphates. Geosciences, 11(10), 419. https://doi.org/10.3390/geosciences11100419






