Evidence of Lithospheric Boudinage in the Grand Banks of Newfoundland from Geophysical Observations
Abstract
:1. Introduction
2. Boudinage
2.1. Mechanism
2.2. Classification
- Shearband
- Symmetric
- Domino
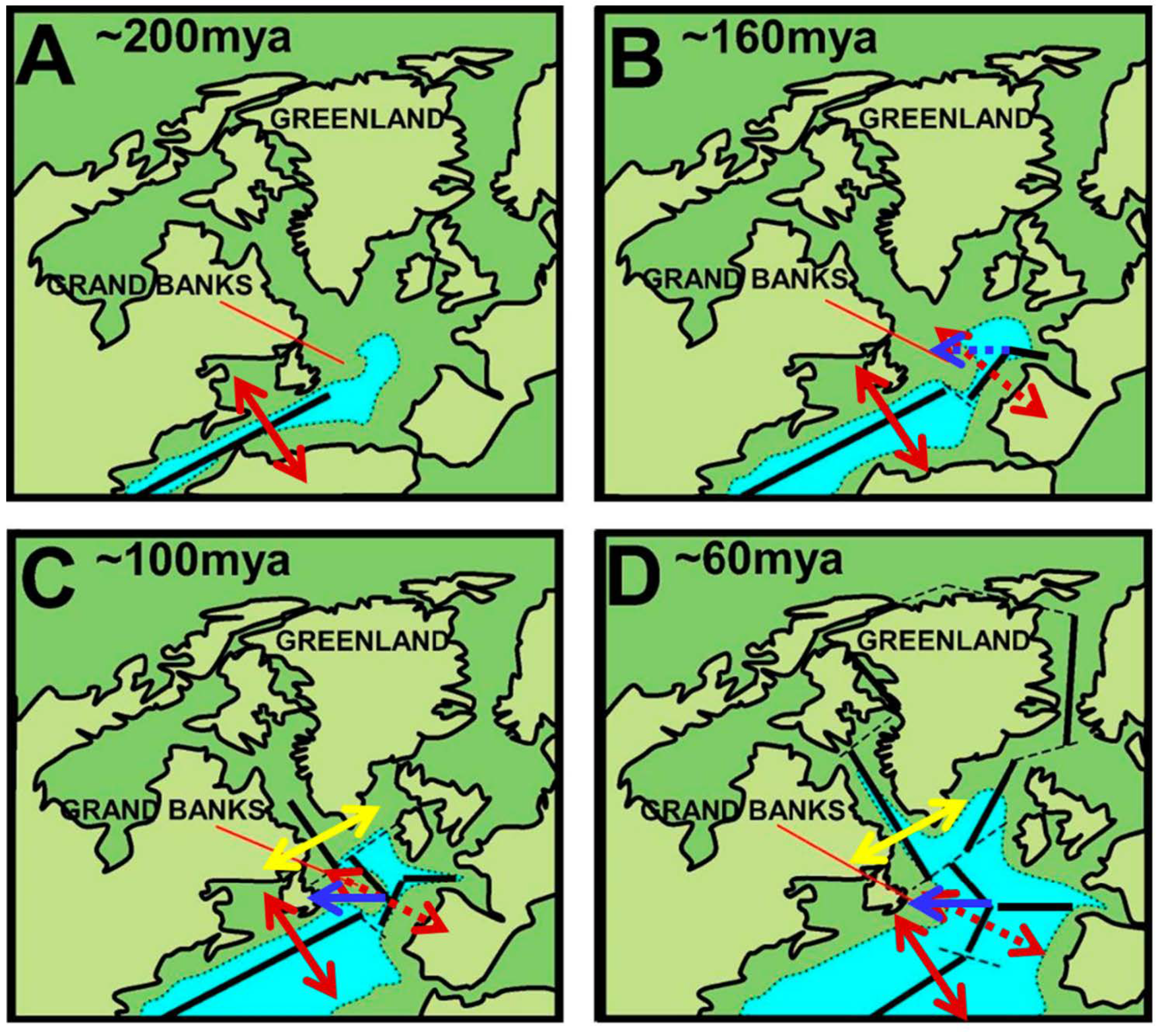
2.3. Analogue Model: Boudinage in the Norwegian Passive Margin
3. Data Analysis and Results
3.1. Fourier Analysis to Analyse Dominant Wavelengths
3.2. Out-of-Line Wavelength Corrections
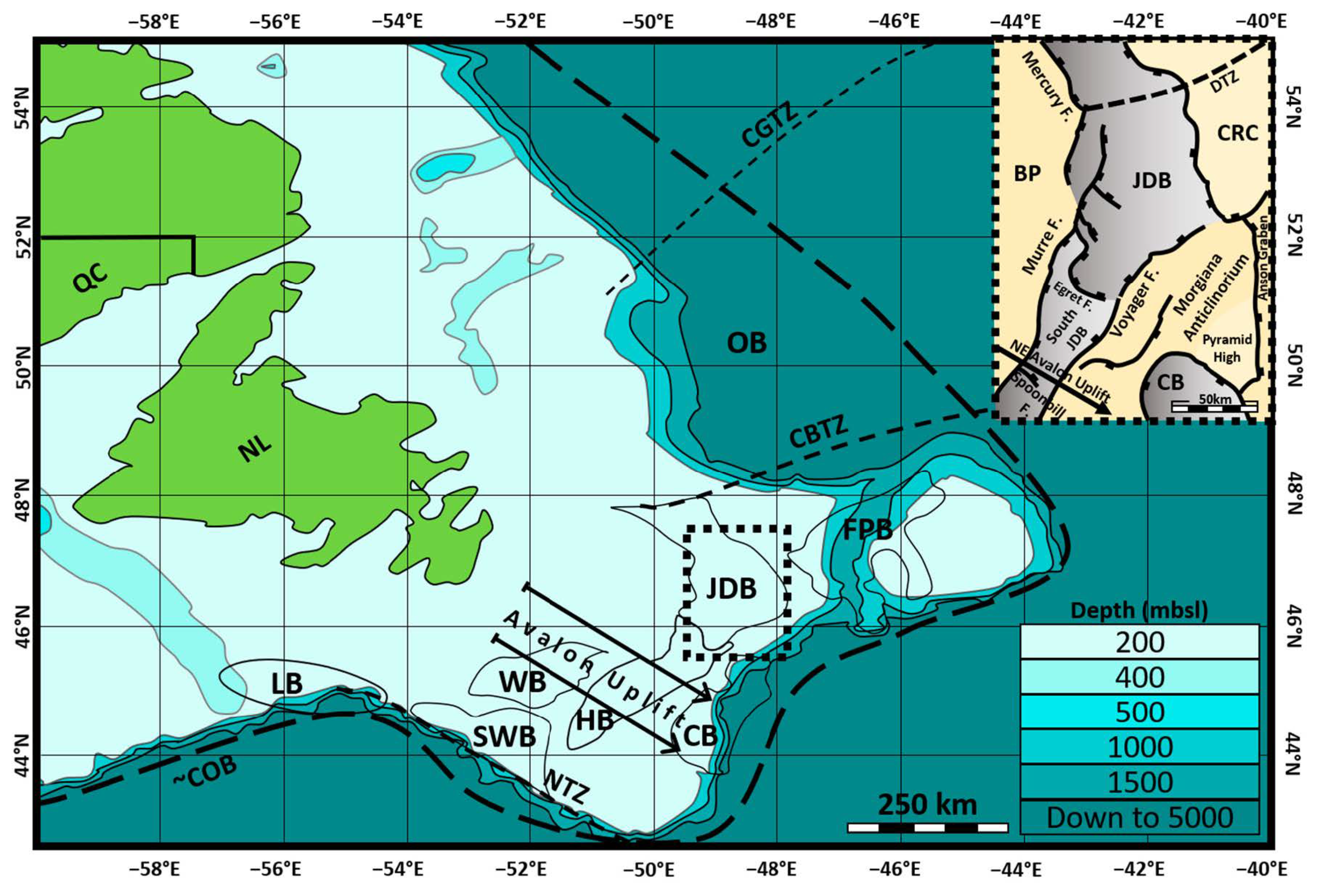
3.3. 2D Seismic Data
3.4. Free Air Gravity and Magnetic Anomaly Data
4. Discussion
- Longer wavelength features are likely a result of continued stretching through multiple successive rifting episodes, compounding the effects of deformation on the same package of rock.
- Due to the repeated oblique extension associated with successive rifting events, the upper competent layer (upper crust) is stretched such that the dominant wavelength observed is due to undulations of the Moho, and the inferred competent layer thickness is that of the lower competent layer (relatively brittle uppermost mantle).
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Enachescu, M.E. Tectonic and structural framework of the northeast Newfoundland continental margin. In Sedimentary Basins and Basin-Forming Mechanisms; Beaumont, C., Tankard, A.J., Eds.; Canadian Society of Petroleum Geologists: Calgary, AB, Canada, 1987; pp. 117–146. [Google Scholar]
- Sinclair, I.K. Tectonism: The dominant factor in mid-Cretaceous deposition in the Jeanne d’Arc Basin, Grand Banks. Mar. Pet. Geol. 1993, 10, 530–549. [Google Scholar] [CrossRef]
- Tankard, A.J.; Welsink, H.J.; Jenkins, W.A.M. Chapter 17: Structural Styles and Stratigraphy of the Jeanne d’Arc Basin, Grand Banks of Newfoundland. In Extensional Tectonics and Stratigraphy of the North Atlantic Margins. AAPG Memoir; Tankard, A.J., Balkwill, H.R., Eds.; American Association of Petroleum Geologists and Canadian Geological Foundation: Tulsa, OK, USA, 1989; Volume 46, pp. 265–282. [Google Scholar]
- Welford, J.K.; Shannon, P.M.; O’Reilly, B.M.; Hall, J. Comparison of lithosphere structure across the Orphan Basin–Flemish Cap and Irish Atlantic conjugate continental margins from constrained 3D gravity inversions. J. Geol. Soc. 2012, 169, 405–420. [Google Scholar] [CrossRef]
- Enachescu, M.E.; Fagan, P. Newfoundland and Labrador Call for Bids NF04-01, Government of Newfoundland and Labrador, Department of Natural Resources. 2004. Available online: http://www.gov.nl.ca/mines&en/oil/call_for_bids_nf04_01.stm (accessed on 1 June 2018).
- Burton-Ferguson, R.; Enachescu, M.; Hiscott, R. Preliminary Seismic Interpretation and Maps for the Paleogene-Neogene (Tertiary) Succession, Orphan Basin. Can. Soc. Explor. Geophys. Recorder 2006, 31, 28–32. [Google Scholar]
- Braun, A.; Marquart, G. Evolution of the Lofoten-Vesterålen margin inferred from gravity and crustal modeling. J. Geophys. Res. 2004, 109(B6), B06404. [Google Scholar] [CrossRef]
- Deng, H.; Ren, J.; Pang, X.; Rey, P.F.; McClay, K.R.; Watkinson, I.M.; Zheng, J.; Luo, P. South China Sea documents the transition from wide continental rift to continental break up. Nat. Commun. 2020, 11, 1–9. [Google Scholar] [CrossRef]
- Gernigon, L.; Ringenbach, J.-C.; Planke, S.; Le Gall, B. Deep structures and breakup along volcanic rifted margins: Insights from integrated studies along the outer Vøring Basin (Norway). Mar. Pet. Geol. 2004, 21, 363–372. [Google Scholar] [CrossRef]
- Keen, C.E.; Loncarevic, B.D.; Reid, I.; Woodside, J.; Haworth, R.T.; Williams, H. Chapter 2: Tectonic and Geophysical Overview; Natural Resources Canada: Hamilton, ON, Canada, 1990; pp. 31–85. [Google Scholar]
- Crosby, A.G.; White, N.; Edwards, G.; Shillington, D.J. Evolution of the Newfoundland–Iberia conjugate rifted margins. Earth Planet. Sci. Lett. 2008, 273, 214–226. [Google Scholar] [CrossRef]
- Parnell, J.; Middleton, D.; Honghan, C.; Hall, D. The use of integrated fluid inclusion studies in constraining oil charge history and reservoir compartmentation: Examples from the Jeanne d’Arc Basin, offshore Newfoundland. Mar. Pet. Geol. 2001, 18, 535–549. [Google Scholar] [CrossRef]
- Sinclair, I.K. Transpressional inversion due to episodic rotation of extensional stresses in Jeanne d’Arc Basin, offshore Newfoundland. Geol. Soc. Lond. Spéc. Publ. 1995, 88, 249–271. [Google Scholar] [CrossRef]
- Braun, A.; Marquart, G. La Naissance de l’Atlantic Norde. Pour Sci. 2001, 287, 2–11. [Google Scholar]
- Peace, A.L.; Phethean, J.; Franke, D.; Foulger, G.; Schiffer, C.; Welford, J.; McHone, G.; Rocchi, S.; Schnabel, M.; Doré, A. A review of Pangaea dispersal and Large Igneous Provinces—In search of a causative mechanism. Earth Sci. Rev. 2020, 206, 102902. [Google Scholar] [CrossRef]
- Sinclair, I.K.; McAlpine, K.D.; Sherwin, D.F.; McMillan, N.J.; Taylor, G.C.; Best, M.E.; Campbell, G.R.; Hea, J.P.; Henao, D.; Procter, R.M. Petroleum resources of the Jeanne d’Arc Basin and environs, Grand Banks, Newfoundland. Pet. Resour. Jeanne D’ARC Basin Environ. Grand Banks Nfld. 1992, 92, 48. [Google Scholar] [CrossRef]
- Braun, A.; Kim, H.; Csatho, B.; Vonfrese, R. Gravity-inferred crustal thickness of Greenland. Earth Planet. Sci. Lett. 2007, 262, 138–158. [Google Scholar] [CrossRef]
- Oakey, G.N.; Chalmers, J.A. A new model for the Paleogene motion of Greenland relative to North America: Plate reconstructions of the Davis Strait and Nares Strait regions between Canada and Greenland. J. Geophys. Res. Space Phys. 2012, 117, 10401. [Google Scholar] [CrossRef]
- Ramberg, H. Natural and Experimental Boudinage and Pinch-and-Swell Structures. J. Geol. 1955, 63, 512–526. [Google Scholar] [CrossRef]
- Fossen, H. Boudinage. Struct. Geol. 2010, 271–284. [Google Scholar] [CrossRef]
- Froidevaux, C. Basin and range large-scale tectonics: Constraints from gravity and reflection seismology. J. Geophys. Res. Space Phys. 1986, 91, 3625–3632. [Google Scholar] [CrossRef]
- Ricard, Y.; Froidevaux, C. Stretching instabilities and lithospheric boudinage. J. Geophys. Res. Space Phys. 1986, 91, 8314–8324. [Google Scholar] [CrossRef]
- Osmundsen, P.T.; Ebbing, J. Styles of extension offshore mid-Norway and implications for mechanisms of crustal thinning at passive margins. Tectonics 2008, 27, 6016. [Google Scholar] [CrossRef]
- Brune, S.; Heine, C.; Clift, P.D.; Pérez-Gussinyé, M. Rifted margin architecture and crustal rheology: Reviewing Iberia-Newfoundland, Central South Atlantic, and South China Sea. Mar. Pet. Geol. 2017, 79, 257–281. [Google Scholar] [CrossRef]
- Houseman, G.; England, P. A dynamical model of lithosphere extension and sedimentary basin formation. J. Geophys. Res. Space Phys. 1986, 91, 719–729. [Google Scholar] [CrossRef]
- Bassi, G. Factors controlling the style of continental rifting: Insights from numerical modelling. Earth Planet. Sci. Lett. 1991, 105, 430–452. [Google Scholar] [CrossRef]
- Sandoval, L.; Welford, J.K.; MacMahon, H.; Alexander, P. Determining continuous basins across conjugate margins: The East Orphan, Porcupine, and Galicia Interior basins of the southern North Atlantic Ocean. Mar. Pet. Geol. 2019, 110, 138–161. [Google Scholar] [CrossRef]
- England, P. Constraints on extension of continental lithosphere. J. Geophys. Res. Space Phys. 1983, 88, 1145–1152. [Google Scholar] [CrossRef]
- Bassi, G. Relative importance of strain rate and rheology for the mode of continental extension. Geophys. J. Int. 1995, 122, 195–210. [Google Scholar] [CrossRef] [Green Version]
- Spadini, G.; Podladchikov, Y.Y. Spacing of consecutive normal faulting in the lithosphere: A dynamic model for rift axis jumping (Tyrrhenian Sea). Earth Planet. Sci. Lett. 1996, 144, 21–34. [Google Scholar] [CrossRef]
- Geng, M.; Welford, J.K.; Farquharson, C.; Hu, X. Gravity modeling for crustal-scale models of rifted continental margins using a constrained 3D inversion method. Geophysics 2019, 84, G25–G39. [Google Scholar] [CrossRef]
- Buck, W.R.; Lavier, L.L.; Babeyko, A. A Numerical Model of Lithospheric Extension Producing Fault-Bounded Basins and Ranges. Int. Geol. Rev. 2003, 45, 712–723. [Google Scholar] [CrossRef]
- Fletcher, R.C.; Hallet, B. Unstable extension of the lithosphere: A mechanical model for basin-and-range structure. J. Geophys. Res. Space Phys. 1983, 88, 7457–7466. [Google Scholar] [CrossRef]
- Montési, L.G.J. Spacing of faults at the scale of the lithosphere and localization instability: 1. Theory. J. Geophys. Res. Space Phys. 2003, 108, 2110. [Google Scholar] [CrossRef] [Green Version]
- Montési, L.G.J.; Zuber, M.T. Spacing of faults at the scale of the lithosphere and localization instability: 2. Application to the Central Indian Basin. J. Geophys. Res. Space Phys. 2003, 108, 2111. [Google Scholar] [CrossRef] [Green Version]
- MacMahon, H.; Welford, J.K.; Sandoval, L.; Alexander, P. The Rockall and the Orphan Basins of the Southern North Atlantic Ocean: Determining Continuous Basins across Conjugate Margins. Geoscience 2020, 10, 178. [Google Scholar] [CrossRef]
- Welford, J.K.; Hall, J.; Sibuet, J.; Srivastava, S.P. Structure across the northeastern margin of Flemish Cap, offshore Newfoundland from Erable multichannel seismic reflection profiles: Evidence for a transtensional rifting environment. Geophys. J. Int. 2010, 183, 572–586. [Google Scholar] [CrossRef] [Green Version]
- Beneš, V.; Davy, P. Modes of continental lithospheric extension: Experimental verification of strain localization processes. Tectonophysics 1996, 254, 69–87. [Google Scholar] [CrossRef]
- Sokoutis, D.; Corti, G.; Bonini, M.; Brun, J.P.; Cloetingh, S.A.P.L.; Mauduit, T.; Manetti, P. Modelling the extension of heterogeneous hot lithosphere. Tectonophysics 2007, 444, 63–79. [Google Scholar] [CrossRef]
- Mora, C.A.S.; Huismans, R.S.; Fossen, H.; Egydio-Silva, M. The Wilson Cycle and Effects of Tectonic Structural Inheritance on Rifted Passive Margin Formation. Tectonics 2018, 37, 3085–3101. [Google Scholar] [CrossRef]
- Petersen, K.D.; Schiffer, C. Wilson cycle passive margins: Control of orogenic inheritance on continental breakup. Gondwana Res. 2016, 39, 131–144. [Google Scholar] [CrossRef]
- Schiffer, C.; Doré, A.G.; Foulger, G.R.; Franke, D.; Geoffroy, L.; Gernigon, L.; Holdsworth, B.; Kusznir, N.; Lundin, E.; McCaffrey, K.; et al. Structural inheritance in the North Atlantic. Earth Sci. Rev. 2020, 206, 102975. [Google Scholar] [CrossRef]
- Goscombe, B.D.; Passchier, C.W.; Hand, M. Boudinage classification: End-member boudin types and modified boudin structures. J. Struct. Geol. 2004, 26, 739–763. [Google Scholar] [CrossRef]
- Wernicke, B.; Burchfiel, B.C. Modes of extensional tectonics. J. Struct. Geol. 1982, 4, 105–115. [Google Scholar] [CrossRef] [Green Version]
- Smith, R.B. Unified theory of the onset of folding, boudinage, and mullion structure. Geol. Soc. Am. Bull. 1975, 86, 1601–1609. [Google Scholar] [CrossRef]
- Smith, R.B. Formation of folds, boudinage, and mullions in non-Newtonian materials. Geol. Soc. Am. Bull. 1977, 88, 312–320. [Google Scholar] [CrossRef]
- Ribeiro, A. Soft Plate and Impact Tectonics. In Soft Plate and Impact Tectonics; Springer Nature: London, UK, 2002; pp. 1–173. [Google Scholar]
- Zuber, M.T.; Parmentier, E.M.; Fletcher, R.C. Extension of continental lithosphere: A model for two scales of basin and range deformation. J. Geophys. Res. Space Phys. 1986, 91, 4826. [Google Scholar] [CrossRef]
- Kearey, P.; Klepeis, K.A.; Vine, F.J. Chapter 2.10.4 Lithospheric Strength Profiles. In Global Tectonics, 3rd ed.; Wiley-Blackwell: Oxford, UK, 2009; pp. 37–40. [Google Scholar]
- Van Wijk, J.; Cloetingh, S. Basin migration caused by slow lithospheric extension. Earth Planet. Sci. Lett. 2002, 198, 275–288. [Google Scholar] [CrossRef]
- Maystrenko, Y.P.; Gernigon, L.; Olesen, O.; Ottesen, D.; Rise, L. 3-D thermal effect of late Cenozoic erosion and deposition within the Lofoten–Vesterålen segment of the Mid-Norwegian continental margin. Geophys. J. Int. 2018, 213, 885–918. [Google Scholar] [CrossRef]
- Faleide, J.I.; Tsikalas, F.; Breivik, A.J.; Mjelde, R.; Ritzmann, O.; Engen, Ø. Structure and Evolution of the Continental Margin off Norway and the Barents Sea. Episodes 2008, 31, 82–91. [Google Scholar] [CrossRef] [Green Version]
- Clerc, C.; Ringenbach, J.-C.; Jolivet, L.; Ballard, J.-F. Rifted margins: Ductile deformation, boudinage, continentward-dipping normal faults and the role of the weak lower crust. Gondwana Res. 2018, 53, 20–40. [Google Scholar] [CrossRef] [Green Version]
- Lithoprobe, East Coast FGP Seismic Reflection Line 84-3; Natural Resources Canada: Vancouver, BC, Canada, 1990; Available online: https://open.canada.ca/data/en/dataset/cd626e58-5f33-5d9f-baba-3be7c28333f3 (accessed on 1 May 2018).
- Lithoprobe, East Coast FGP Seismic Reflection Line 85-3; Natural Resources Canada: Vancouver, BC, Canada, 1990; Available online: https://open.canada.ca/data/en/dataset/9643cd16-37e6-5fa7-8d21-753d85af838d (accessed on 1 May 2018).
- Lithoprobe, East Coast FGP Seismic Reflection Line 87-2; Natural Resources Canada: Vancouver, BC, Canada, 1990; Available online: https://open.canada.ca/data/en/dataset/d2958f0b-3ec1-59a2-8d0a-b9273ae58f50 (accessed on 1 May 2018).
- Sandwell, D.T.; Müller, R.D.; Smith, W.H.F.; Garcia, E.; Francis, R. New global marine gravity model from CryoSat-2 and Jason-1 reveals buried tectonic structure. Science 2014, 346, 65–67. [Google Scholar] [CrossRef]
- Schwarz, K.P.; Sideris, M.G.; Forsberg, R. The use of FFT techniques in physical geodesy. Geophys. J. Int. 1990, 100, 485–514. [Google Scholar] [CrossRef] [Green Version]
- Perwass, C. Projection. In Geometric Algebra with Applications in Engineering. Geometry and Computing; Springer: Berlin, Germany, 2008; Volume 4, pp. 82–83. [Google Scholar]
- Hammer, S. Terrain Corrections for Gravimeter Stations. Geophysics 1939, 4, 184–194. [Google Scholar] [CrossRef]
- Nowell, D. Gravity terrain corrections—An overview. J. Appl. Geophys. 1999, 42, 117–134. [Google Scholar] [CrossRef]
- Smith, W.H.F.; Sandwell, D.T. Global Sea Floor Topography from Satellite Altimetry and Ship Depth Soundings. Science 1997, 277, 1956–1962. [Google Scholar] [CrossRef] [Green Version]
- Dehler, S.A.; Roest, W.R. Gravity anomaly map, Atlantic region, Canada. Gravity Anom. Map Atl. Reg. Can. 1998, 1. [Google Scholar] [CrossRef]
- Oakey, G.N.; Dehler, S.A. Magnetic anomaly map, Atlantic region, Canada. Magn. Anom. Map Atl. Reg. Can. 1998, 1. [Google Scholar] [CrossRef]
- Wernicke, B.P.; Tilke, P.G. Chapter 2: Extensional Tectonic Framework of the U.S. Central Atlantic Passive Margin. In Extensional Tectonics and Stratigraphy of the North Atlantic Margins (AAPG Memoir); Tankard, A.J., Balkwill, H.R., Eds.; American Association of Petroleum Geologists and Canadian Geological Foundation: Tulsa, OK, USA, 1989; Volume 46, pp. 7–21. [Google Scholar]


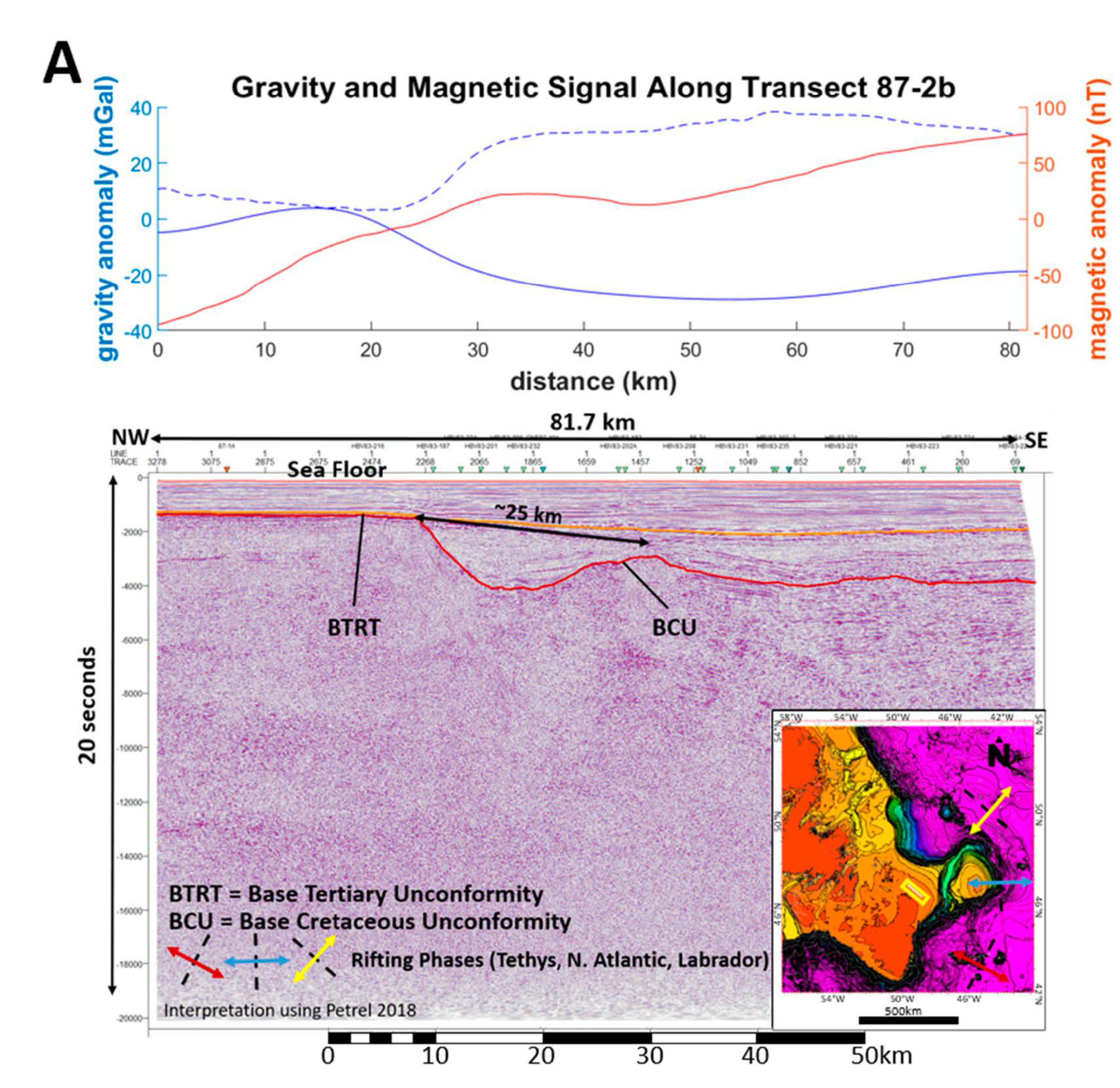
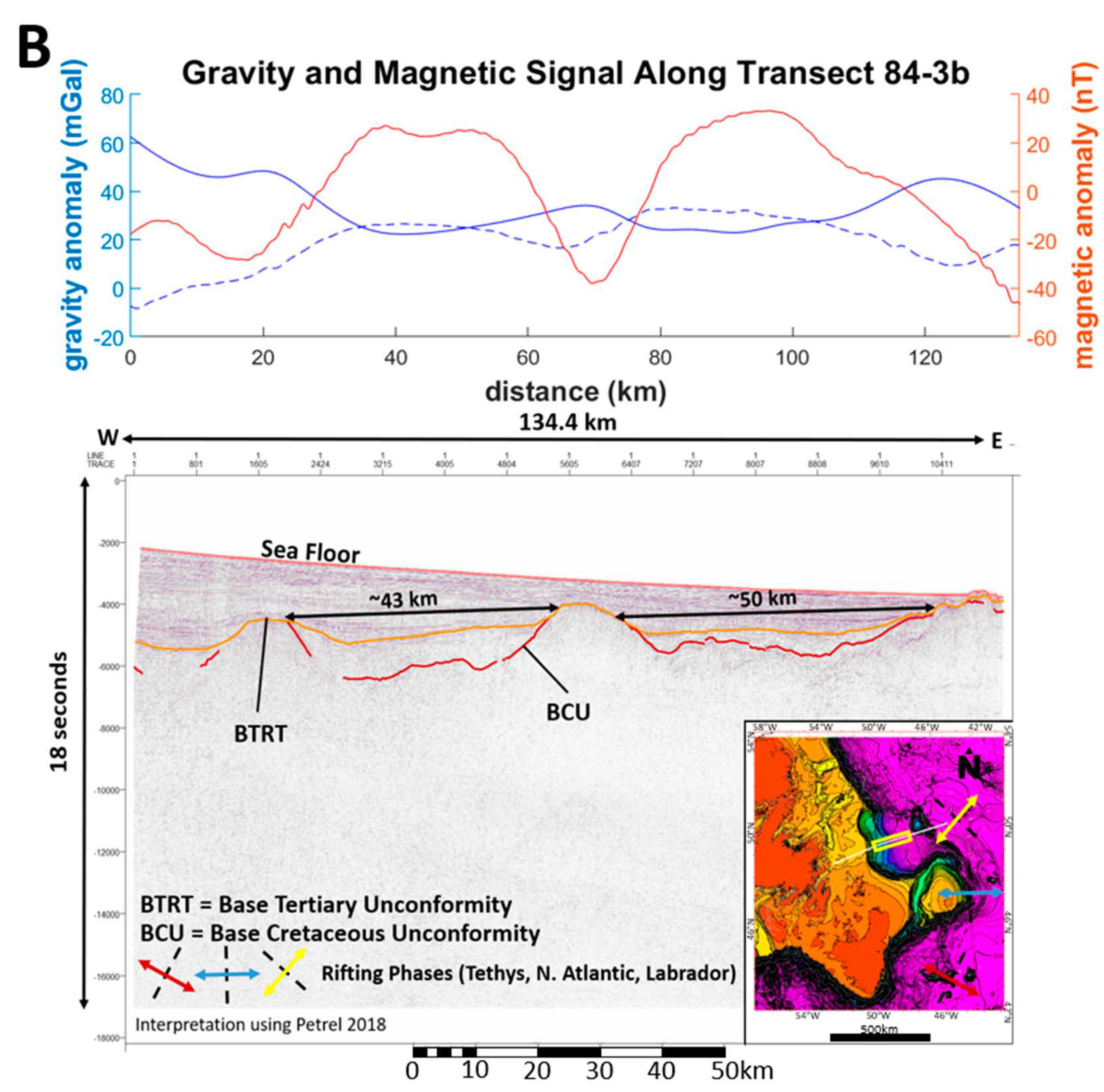
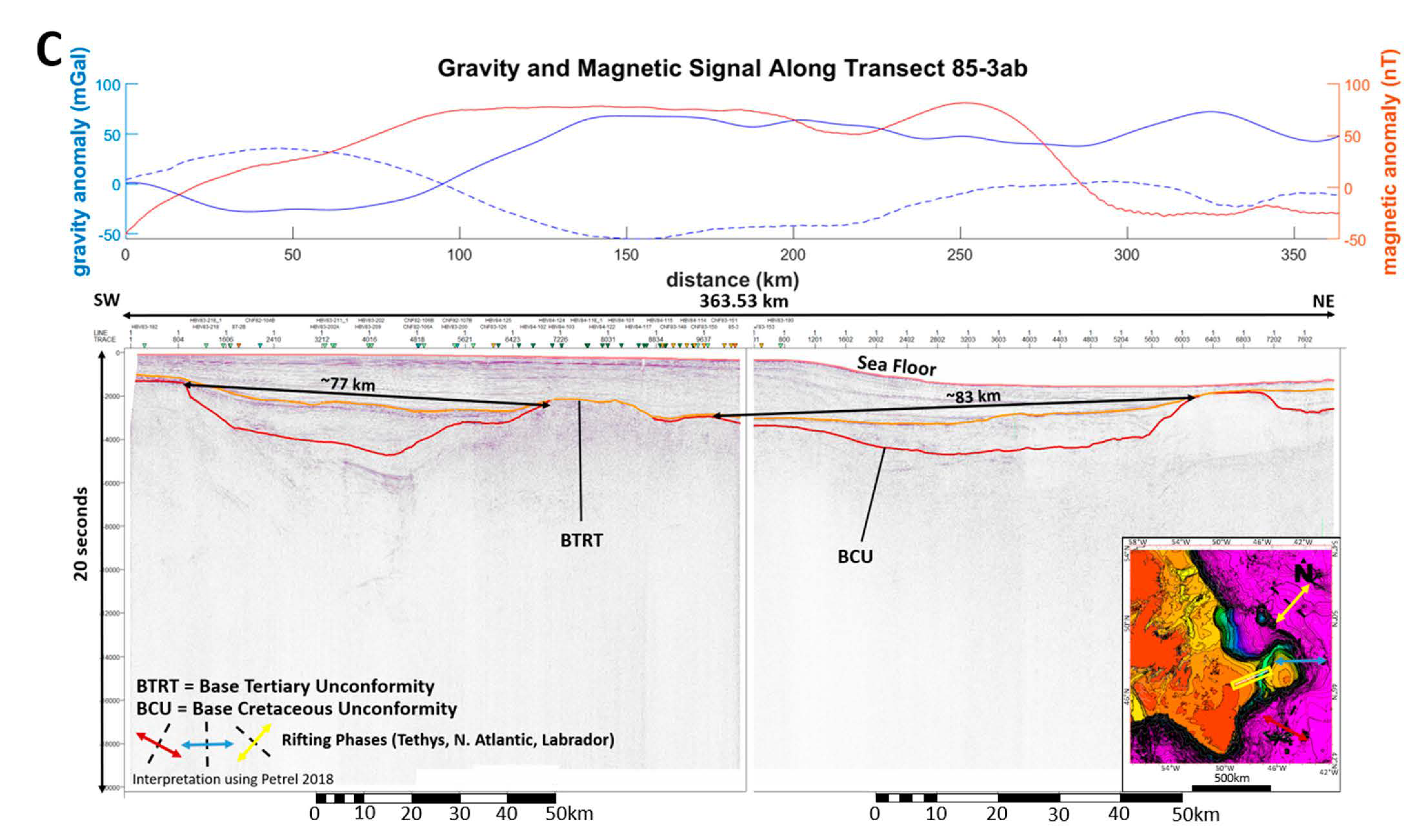
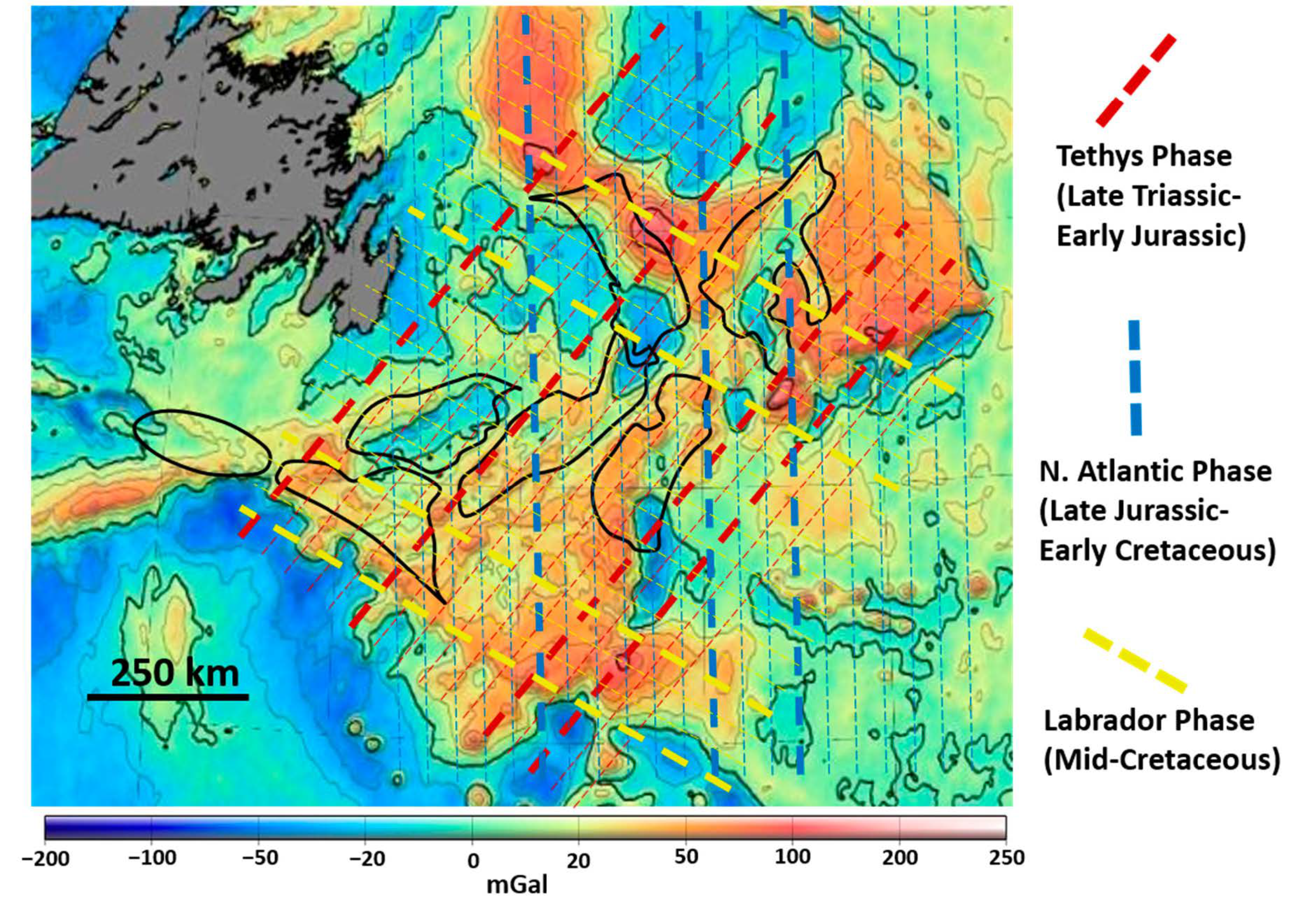
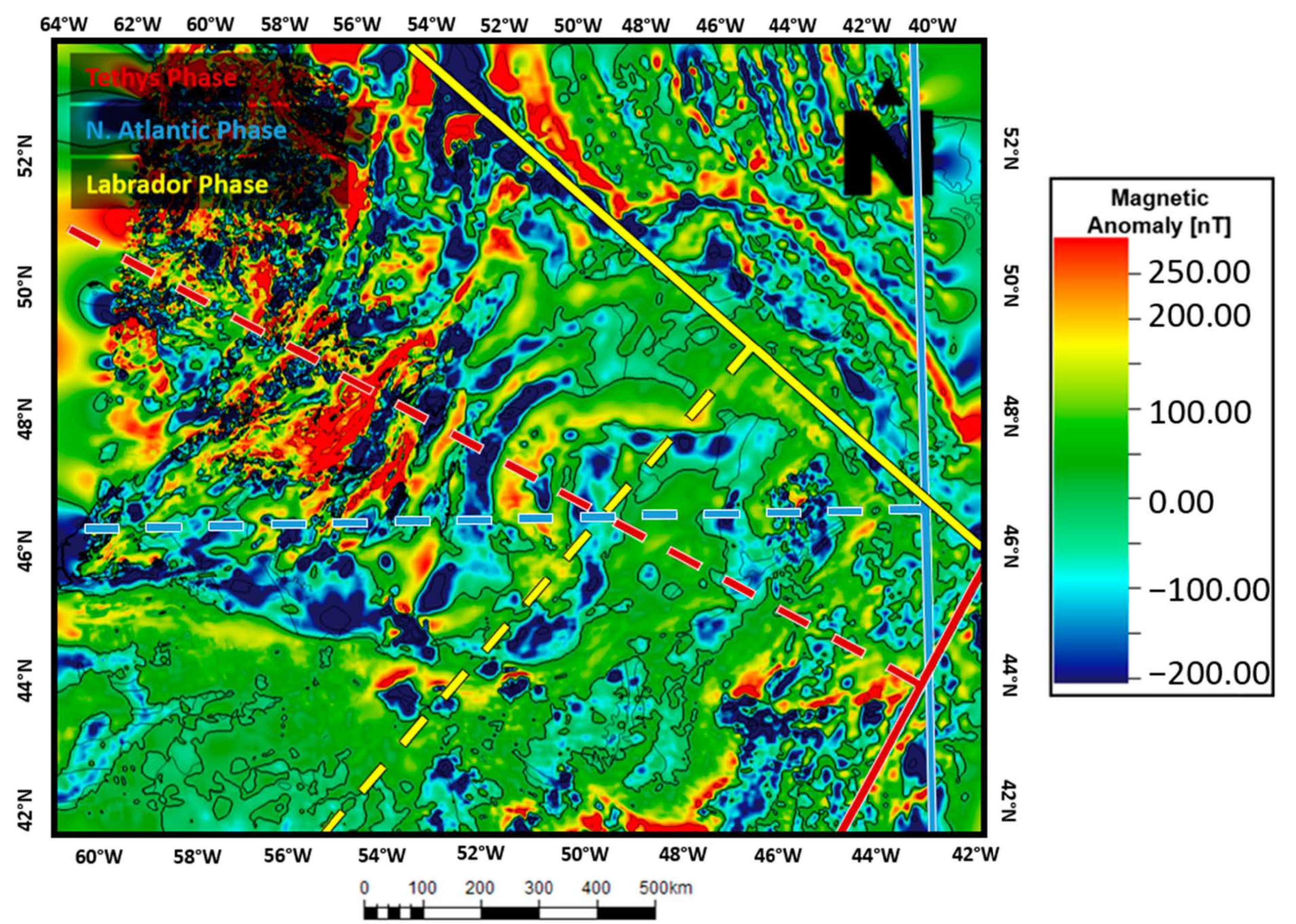
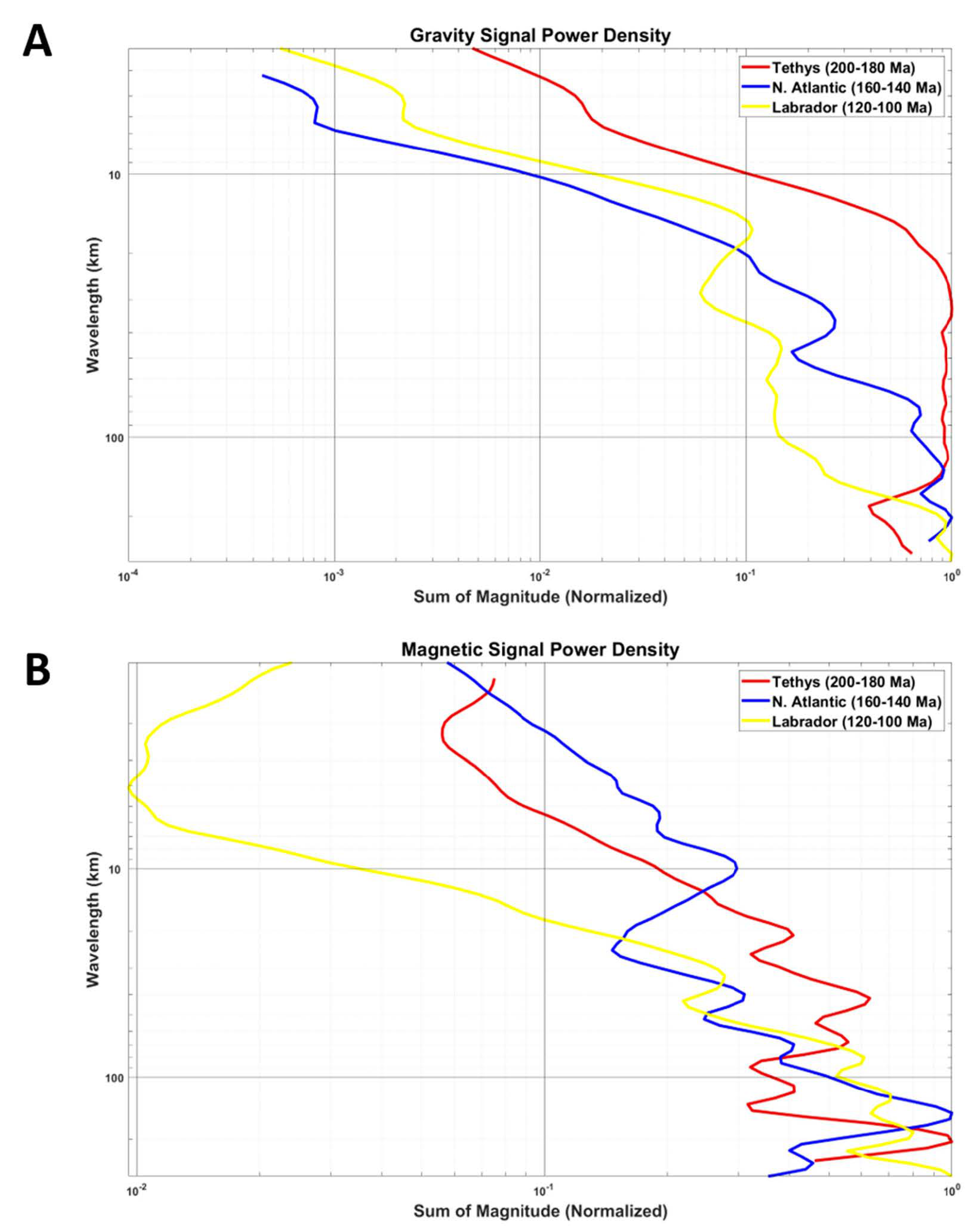
| Orientation | Rifting Phase | Dominant Wavelength Observed |
|---|---|---|
| NW-SE | Tethys | 25 km |
| W-E | North Atlantic | 50 km |
| SW-NE | Labrador | 80 km |
| Orientation | Rifting Phase | Dominant Wavelength Observed (Corrected Gravity Anomaly) | Dominant Wavelength Observed (Magnetic Anomaly) |
|---|---|---|---|
| NW-SE | Tethys | ~30–100 km | ~21–100, >200 km |
| W-E | North Atlantic | ~40–150 km | ~10, ~75, ~150 km |
| SW-NE | Labrador | ~16, ~45, >200 km | ~35, ~80–150, ~200 km |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
MacDougall, M.D.J.; Braun, A.; Fotopoulos, G. Evidence of Lithospheric Boudinage in the Grand Banks of Newfoundland from Geophysical Observations. Geosciences 2021, 11, 55. https://doi.org/10.3390/geosciences11020055
MacDougall MDJ, Braun A, Fotopoulos G. Evidence of Lithospheric Boudinage in the Grand Banks of Newfoundland from Geophysical Observations. Geosciences. 2021; 11(2):55. https://doi.org/10.3390/geosciences11020055
Chicago/Turabian StyleMacDougall, Malcolm D. J., Alexander Braun, and Georgia Fotopoulos. 2021. "Evidence of Lithospheric Boudinage in the Grand Banks of Newfoundland from Geophysical Observations" Geosciences 11, no. 2: 55. https://doi.org/10.3390/geosciences11020055
APA StyleMacDougall, M. D. J., Braun, A., & Fotopoulos, G. (2021). Evidence of Lithospheric Boudinage in the Grand Banks of Newfoundland from Geophysical Observations. Geosciences, 11(2), 55. https://doi.org/10.3390/geosciences11020055







