On the Annual and Semi-Annual Components of Variations in Extent of Arctic and Antarctic Sea-Ice
Abstract
:1. Introduction
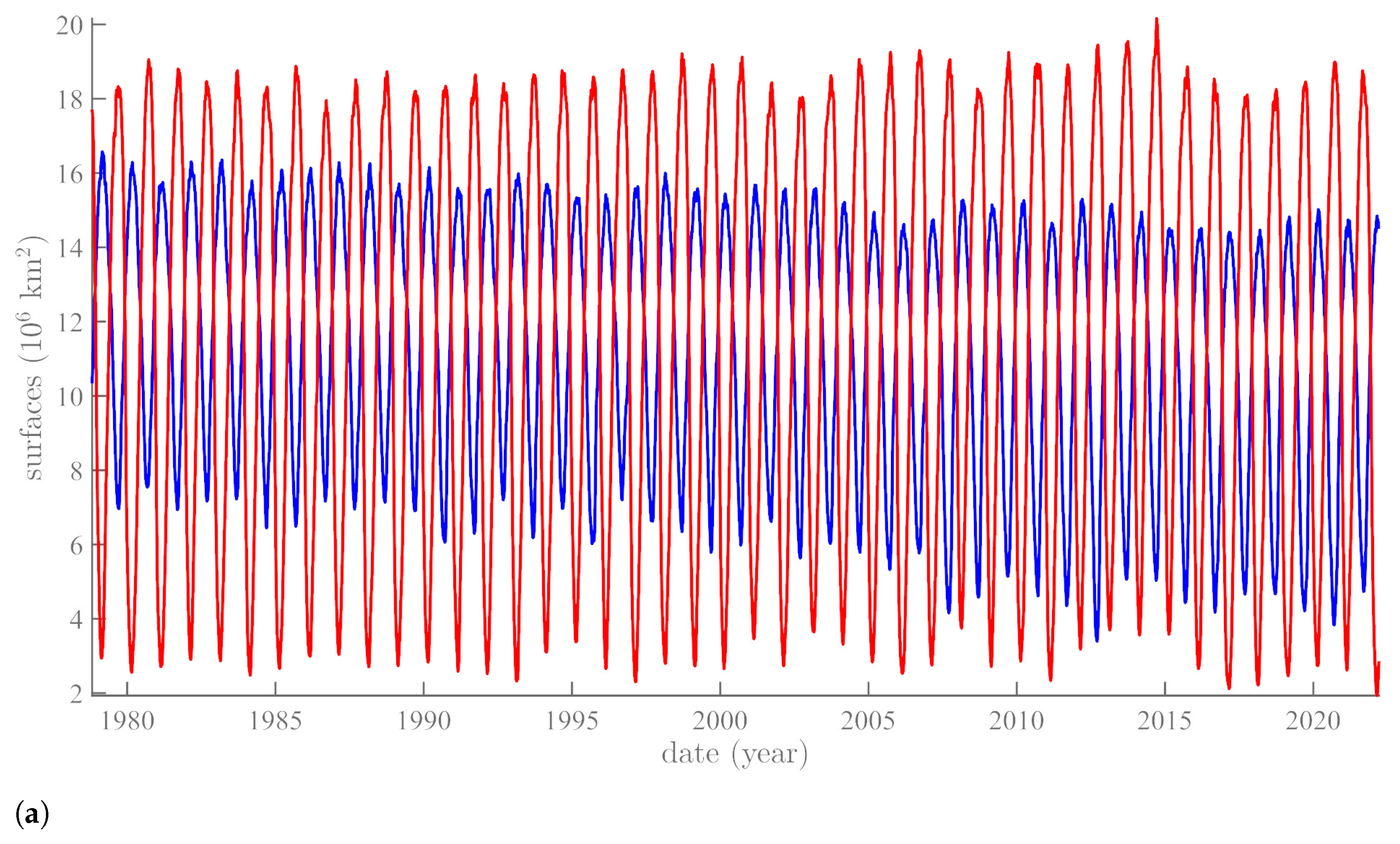
2. Available Data on the Extent of Sea Ice in Both Hemispheres
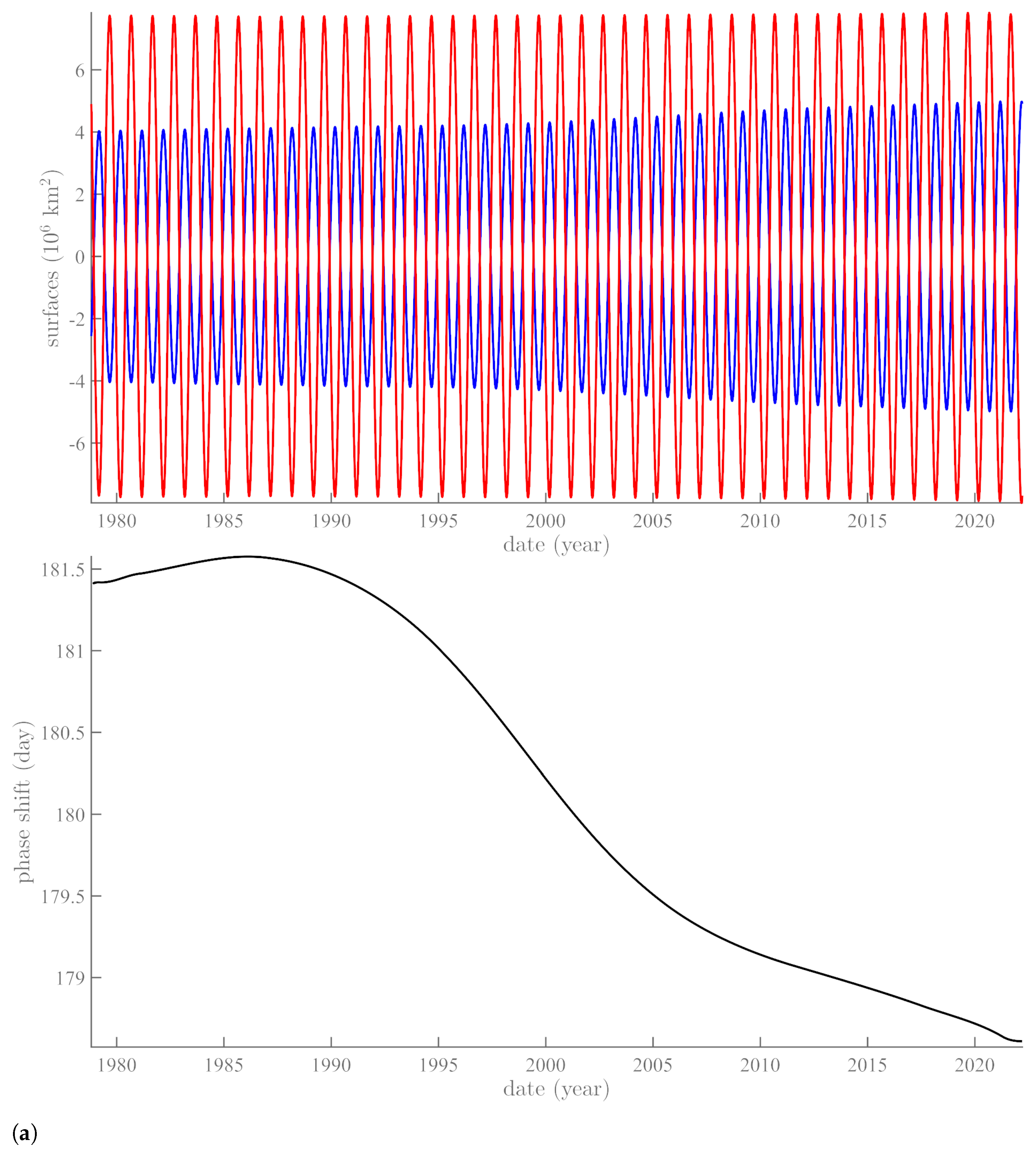
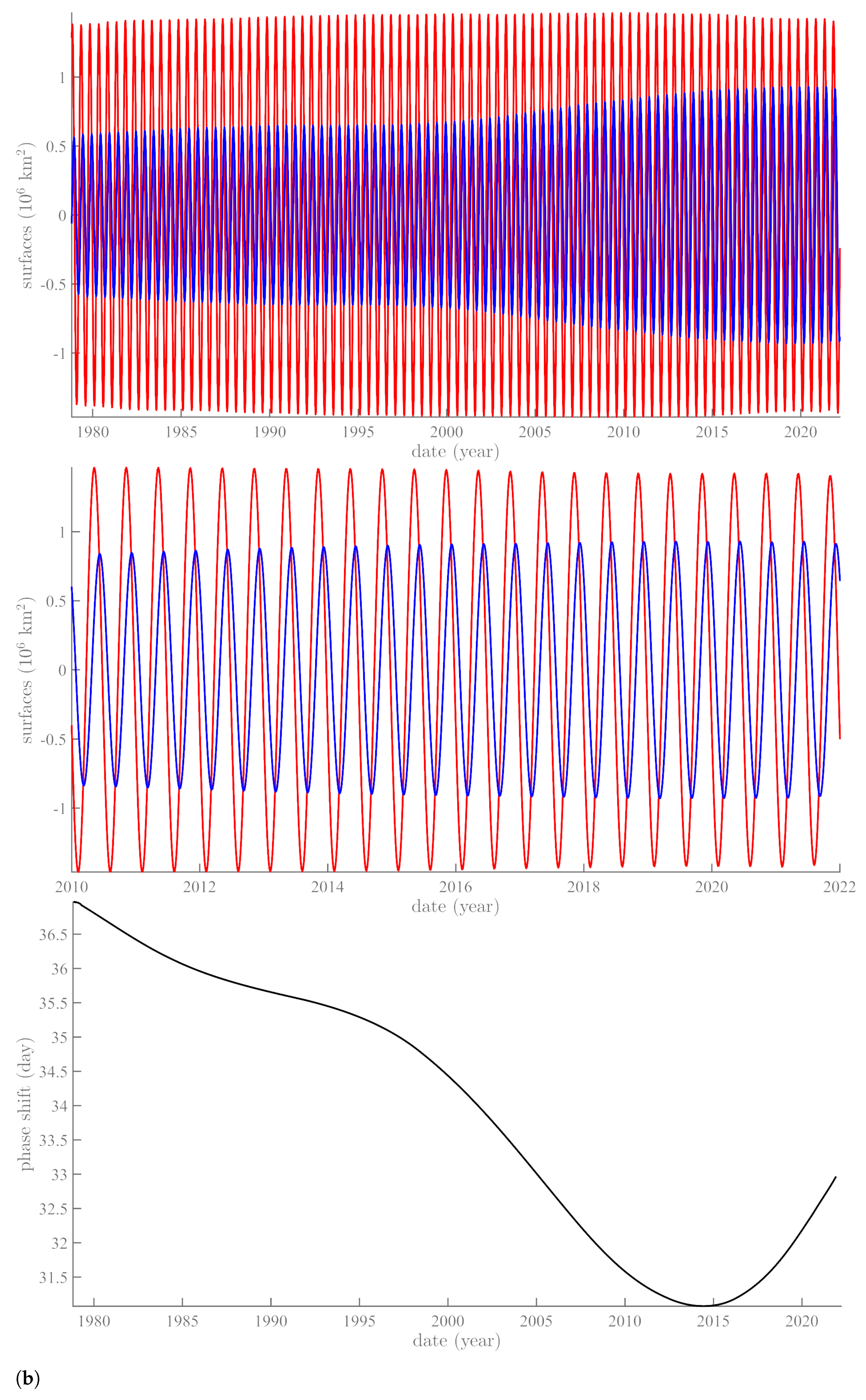
3. SSA Analysis of NHSI and SHSI Data on Sea Ice Extent
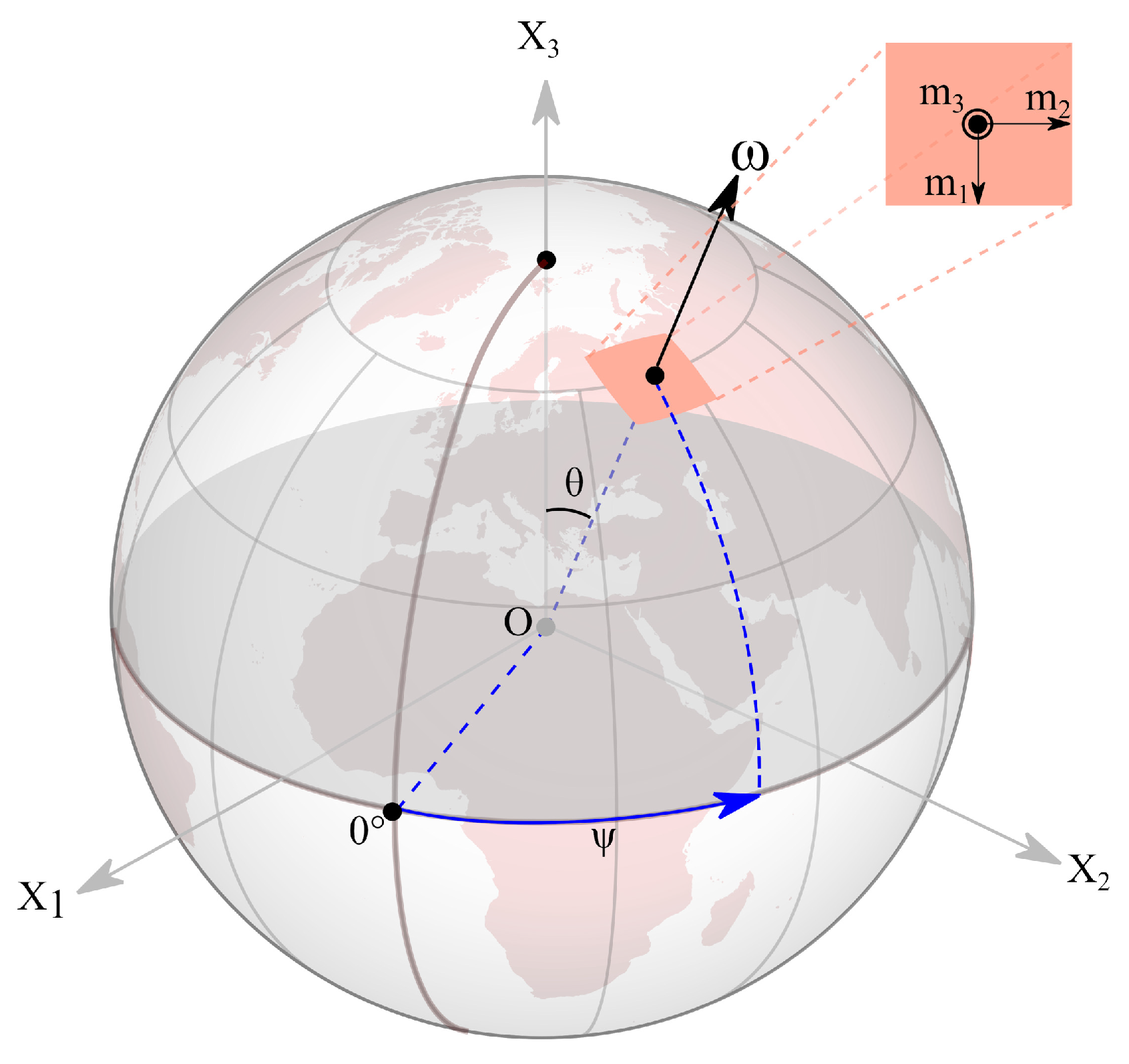
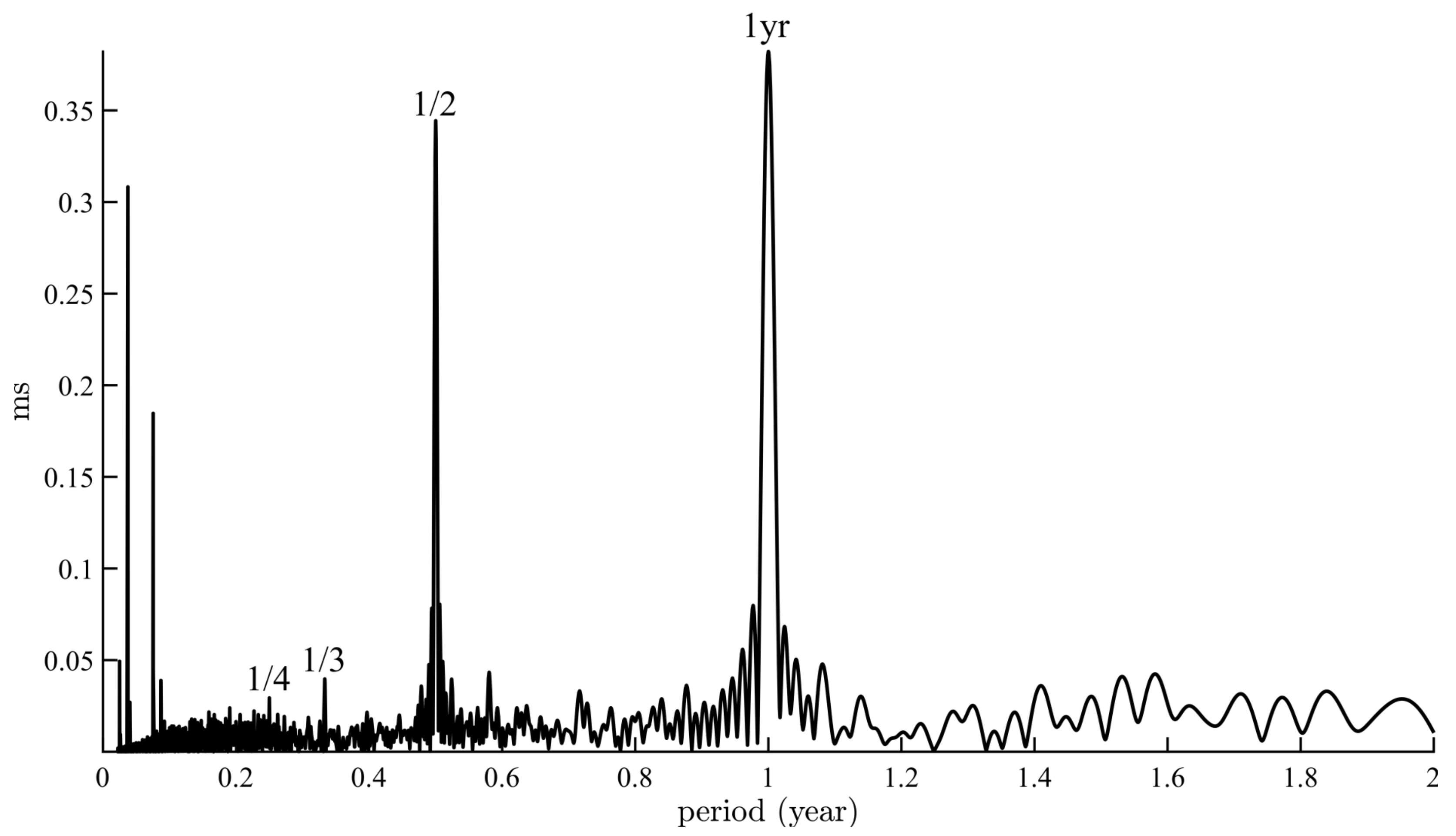
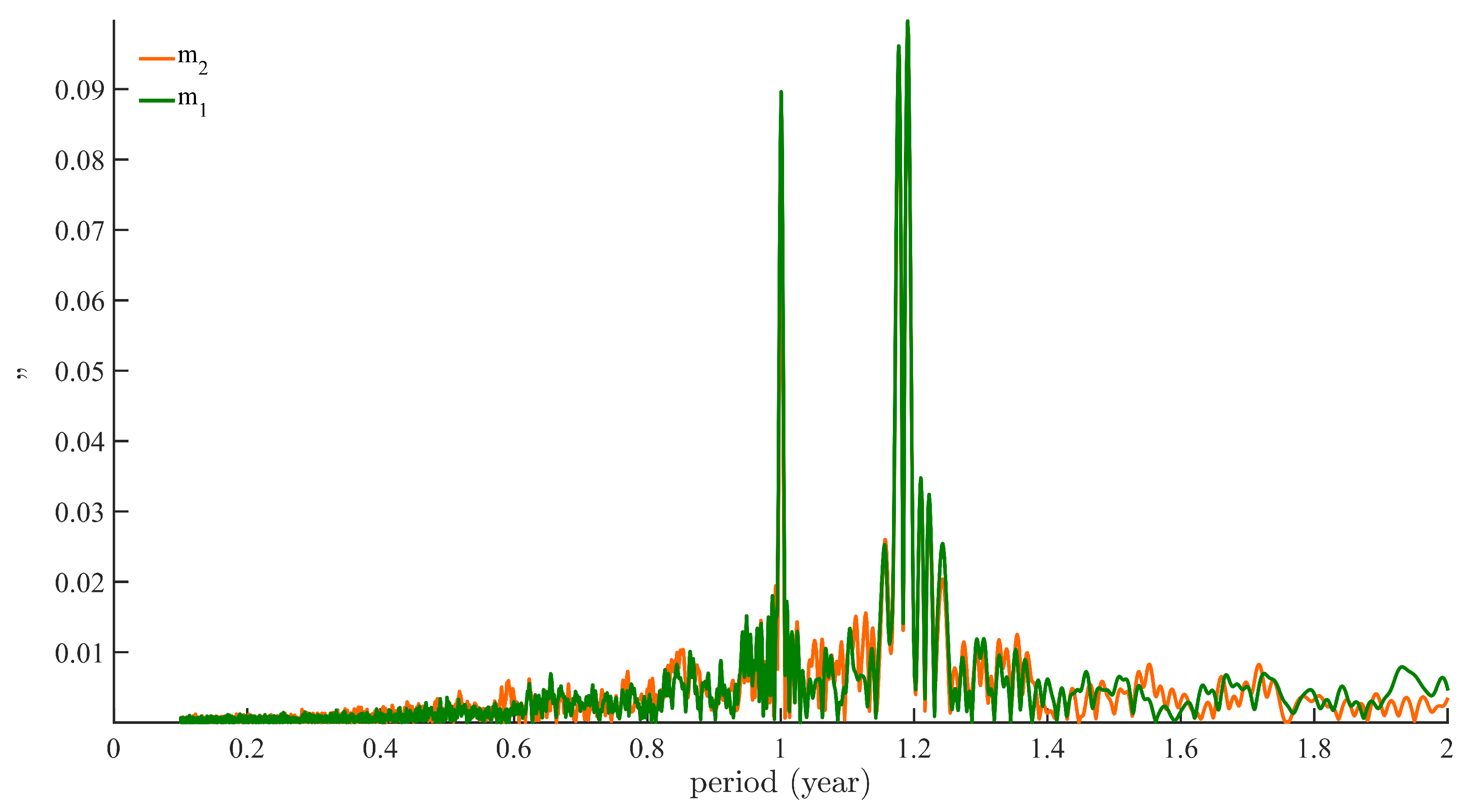
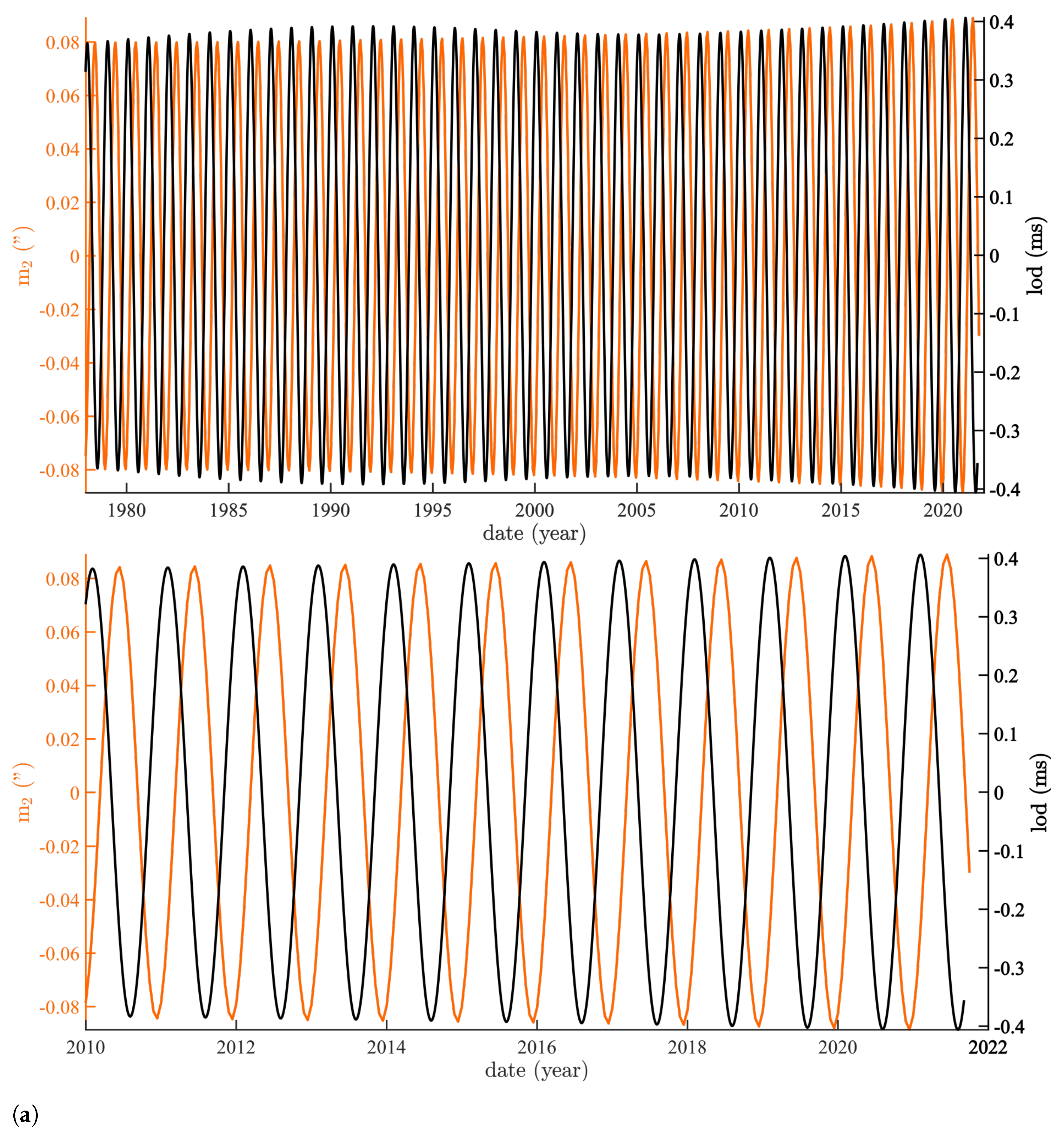
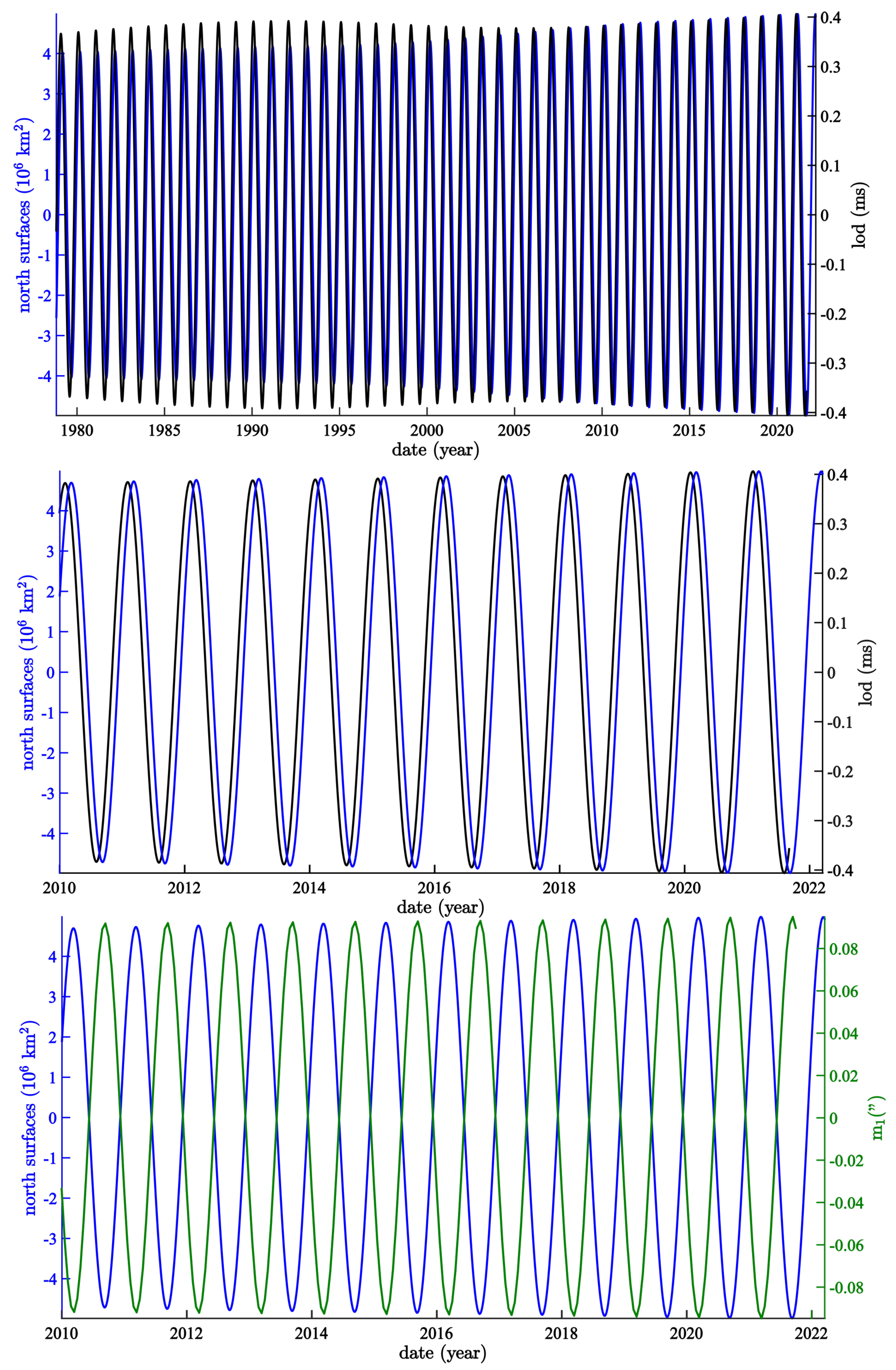
4. Complementary Results for Polar Motion and Length of Day
5. Comparison of Annual and Semi-Annual SSA Components of Polar Motion and Length of Day vs. Variations in Sea Ice Extent
6. Discussion and Conclusions
“The principal seasonal oscillation in the wobble is the annual term which has generally been attributed to a geographical redistribution of mass associated with meteorological causes. Jeffreys, in 1916, first attempted a detailed quantitative evaluation of this excitation function by considering the contributions from atmospheric and oceanic motion, of precipitation, of vegetation and of polar ice. Jeffreys concluded that these factors explain the observed annual polar motion, a conclusion still valid today, although the quantitative comparisons between the observed and computed annual components of the pole path are still not satisfactory. These discrepancies may be a consequence of (i) inadequate data for evaluating the known excitations functions, (ii) the neglect of additional excitation functions, (iii) systematic errors in the astronomical data, or (iv) year-to-year variability in the annual excitation functions”.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
“Nous avons fait voir (n°8), que le moyen mouvement de rotation de la Terre est uniforme, dans la supposition que cette planète est entièrement solide, et l’on vient de voir que la fluidité de la mer et de l’atmosphère ne doit point altérer ce résultat. Les mouvements que la chaleur du Soleil excite dans l’atmosphère, et d’où naissent les vents alizés semblent devoir diminuer la rotation de la Terre: ces vents soufflent entre les tropiques, d’occident en orient, et leur action continuelle sur la mer, sur les continents et les montagnes qu’ils rencontrent, paraît devoir affaiblir insensiblement ce mouvement de rotation. Mais le principe de conservation des aires, nous montre que l’effet total de l’atmosphère sur ce mouvement doit être insensible; car la chaleur solaire dilatant également l’air dans tous les sens, elle ne doit point altérer la somme des aires décrites par les rayons vecteurs de chaque molécule de la Terre et de l’atmosphère, et multipliées respectivement par leur molécules correspondantes; ce qui exige que le mouvement de rotation ne soit point diminué. Nous sommes donc assurés qu’en même temps que les vents analysés diminuent ce mouvement, les autres mouvements de l’atmosphère qui ont lieu au-delà des tropiques, l’accélèrent de la même quantité. On peut appliquer le même raisonnement aux tremblements de Terre, et en général, à tous ce qui peut agiter la Terre dans son intérieur et à sa surface. Le déplacement de ces parties peut seul altérer ce mouvement; si, par exemple un corps placé au pole, était transporté à l’équateur; la somme des aires devant toujours rester la même, le mouvement de la Terre en serait un peu diminué; mais pour que cela fut sensible, il faudrait supposer de grands changement dans la constitution de la Terre”.
Appendix B
References
- Le Mouël, J.L.; Lopes, F.; Courtillot, V.; Gibert, D. On forcings of length of day changes: From 9-day to 18.6-year oscillations. Phys. Earth Planet. Inter. 2019, 292, 1–11. [Google Scholar] [CrossRef]
- Lopes, F.; Le Mouël, J.L.; Courtillot, V.; Gibert, D. On the shoulders of Laplace. Phys. Earth Planet. Inter. 2021, 316, 106693. [Google Scholar] [CrossRef]
- Lopes, F.; Courtillot, V.; Gibert, D.; Mouël, J.-L.L. On Two Formulations of Polar Motion and Identification of Its Sources. Geosciences 2022, 12, 398. [Google Scholar] [CrossRef]
- Courtillot, V.; Le Mouël, J.L.; Kossobokov, V.; Gibert, D.; Lopes, F. Multi-Decadal Trends of Global Surface Temperature: A Broken Line with Alternating ∼30 yr Linear Segments ? NPJ Clim. Atmos. Sci. 2013, 3. Available online: http://www.scirp.org/journal/PaperInformation.aspx?PaperID=34080 (accessed on 12 January 2023). [CrossRef] [Green Version]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. A solar signature in many climate indices. J. Geophys. Res. Atmos. 2019, 124, 2600–2619. [Google Scholar] [CrossRef]
- Lopes, F.; Courtillot, V.; Le Mouël, J.-L. Triskeles and Symmetries of Mean Global Sea-Level Pressure. Atmosphere 2022, 13, 1354. [Google Scholar] [CrossRef]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. Characteristic time scales of decadal to centennial changes in global surface temperatures over the past 150 years. Earth Planets Space 2020, 7, e2019EA000671. [Google Scholar] [CrossRef] [Green Version]
- Lopes, F.; Courtillot, V.; Gibert, D.; Le Mouël, J.-L. Extending the Range of Milankovic Cycles and Resulting Global Temperature Variations to Shorter Periods (1–100 Year Range). Geosciences 2022, 12, 448. [Google Scholar] [CrossRef]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. Solar turbulence from sunspot records. Mon. Notices Royal Astron. Soc. 2021, 492, 1416–1420. [Google Scholar] [CrossRef]
- Courtillot, V.; Lopes, F.; Le Mouël, J.L. On the prediction of solar cycles. Sol. Phys. 2021, 296, 1–23. [Google Scholar] [CrossRef]
- Laplace, P.S. Traité de Mécanique Céleste; l’Imprimerie de Crapelet: Paris, France, 1799. [Google Scholar]
- Lopes, F.; Courtillot, C.; Gibert, D.; Le Mouël, J.L. On pseudo-periodic perturbations of planetary orbits, and oscillations of Earth’s rotation and revolution: Lagrange’s formulation. arXiv 2022, arXiv:2209.07213. [Google Scholar] [CrossRef]
- Milanković, M. Théorie Mathématique des phéNomènes Thermiques Produits par la Radiation Solaire; Faculté des Sciences de l’Université de Belgrade, Gauthier-Villard Edition: Paris, France, 1920. [Google Scholar]
- Mörth, H.T.; Schlamminger, L. Planetary Motion, Sunspots and Climate, Solar-Terrestrial Influences on Weather and Climate; Springer: Dordrecht, The Netherlands, 1979; Volume 193. [Google Scholar]
- Fairbridge, R.W. Planetary periodicities and terrestrial climate stress. In Climatic Changes on a Yearly to Millennial Basis; Springer: Dordrecht, The Netherlands, 1984; pp. 509–520. [Google Scholar]
- Mörner, N.A. Planetary, solar, atmospheric, hydrospheric and endogene processes as origin of climatic changes on the Earth. In Climatic Changes on a Yearly to Millennial Basis; Springer: Dordrecht, The Netherlands, 1984; pp. 483–507. [Google Scholar]
- Laskar, J.; Robutel, P.; Joutel, F.; Gastineau, M.; Correia, A.C.M.; Levrard, B. A long-term numerical solution for the insolation quantities of the Earth. Astron. Astrophys. 2004, 428, 261–285. [Google Scholar] [CrossRef] [Green Version]
- Scafetta, N. Empirical evidence for a celestial origin of the climate oscillations and its implications. J. Atmos. Sol. Terr. Phys. 2010, 72, 951–970. [Google Scholar] [CrossRef] [Green Version]
- Barnhart, B.L.; Eichinger, W.E. Empirical mode decomposition applied to solar irradiance, global temperature, sunspot number, and CO2 concentration data. J. Atmos. Sol. Terr. Phys. 2011, 73, 1771–1779. [Google Scholar] [CrossRef]
- Manzi, V.; Gennari, R.; Lugli, S.; Roveri, M.; Scafetta, N.; Schreiber, B.C. High-frequency cyclicity in the Mediterranean Messinian evaporites: Evidence for solar–lunar climate forcing. J. Sediment. Res. 2012, 82, 991–1005. [Google Scholar] [CrossRef]
- Scafetta, N. Multi-scale harmonic model for solar and climate cyclical variation throughout the Holocene based on Jupiter–Saturn tidal frequencies plus the 11-year solar dynamo cycle. J. Atmos. Sol. Terr. Phys. 2012, 80, 296–311. [Google Scholar] [CrossRef] [Green Version]
- Mörner, N.A. Planetary beat and solar–terrestrial responses. Pattern. Recognit. Phys. 2013, 1, 107–116. [Google Scholar] [CrossRef]
- Scafetta, N. Discussion on climate oscillations: CMIP5 general circulation models versus a semi-empirical harmonic model based on astronomical cycles. Earth-Sci. Rev. 2013, 126, 321–357. [Google Scholar] [CrossRef] [Green Version]
- Lopes, F.; Le Mouël, J.L.; Gibert, D. The mantle rotation pole position. A solar component. CR Geosci. 2017, 349, 159–164. [Google Scholar] [CrossRef]
- Boulila, S.; Laskar, J.; Haq, B.U.; Galbrun, B.; Hara, N. Long-term cyclicities in Phanerozoic sea-level sedimentary record and their potential drivers. Glob. Planet Chang. 2018, 165, 128–136. [Google Scholar] [CrossRef]
- Dumont, S.; Le Mouël, J.L.; Courtillot, V.; Lopes, F.; Sigmundsson, F.; Coppola, D.; Eibl, E.P.S.; Bean, C.J. The dynamics of a long-lasting effusive eruption modulated by Earth tides. Earth Planet. Sci. Lett. 2020, 536, 116145. [Google Scholar] [CrossRef]
- Dumont, S.; Silveira, G.; Custódio, S.; Lopes, F.; Le Mouël, J.L.; Courtillot, V.; Gouhier, M.; Guéhenneux, Y. Response of Fogo volcano (Cape Verde) to lunisolar gravitational forces during the 2014–2015 eruption. Phys. Earth Planet. Inter. 2021, 312, 106659. [Google Scholar] [CrossRef]
- Petrosino, S.; Dumont, S. Tidal modulation of hydrothermal tremor: Examples from Ischia and Campi Flegrei volcanoes, Italy. Front. Earth Sci. 2022, 9, 775269. [Google Scholar] [CrossRef]
- Bank, M.J.; Scafetta, N. Scaling, mirror symmetries and musical consonances among the distances of the planets of the solar system. Front. Astron. Space Sci. 2022, 8, 758184. [Google Scholar] [CrossRef]
- Dumont, S.; Custódio, S.; Petrosino, S.; Thomas, A.M.; Sottili, G. Tides, earthquakes, and volcanic eruptions. In A Journey through Tides; Elsevier: Amsterdam, The Netherlands, 2023; pp. 333–364. [Google Scholar] [CrossRef]
- Cavalieri, D.J.; Parkinson, C.L. Arctic sea ice variability and trends, 1979–2010. Cryosphere 2012, 6, 881. [Google Scholar] [CrossRef] [Green Version]
- Fetterer, F.; Knowles, K.; Meier, W.N.; Savoie, M.; Windnagel, A.K. Sea Ice Index; National Snow and Ice Data Center: Boulder, CO, USA, 2017. [Google Scholar]
- Cavalieri, D.J.; Parkinson, C.L.; Gloersen, P.; Zwally, H.J. Arctic and Antarctic Sea Ice Concentrations from Multichannel Passive-Microwave Satellite Data Sets: October 1978–September 1995 User’s Guide NASA Tech. Mem. 104647; Goddard Space Flight Center: Greenbelt, MD, USA, 1997; p. 21. [Google Scholar]
- Cavalieri, D.J.; Parkinson, C.L.; Gloersen, P.; Comiso, J.C.; Zwally, H.J. Deriving long-term time series of sea ice cover from satellite passive microwave multisensor data sets. J. Geophys. Res. Oceans 1999, 104, 15803–15814. [Google Scholar] [CrossRef]
- Cavalieri, D.J.; Parkinson, C.L.; Di Girolamo, N.; Ivanoff, A. Intersensor calibration between F13 SSMI and F17 SSMIS for global sea ice data records. IEEE Geosci. Remote. Sens. Lett. 2011, 9, 233–236. [Google Scholar] [CrossRef] [Green Version]
- Parkinson, C.L. A 40-y record reveals gradual Antarctic sea ice increases followed by decreases at rates far exceeding the rates seen in the Arctic. Proc. Natl. Acad. Sci. USA 2019, 116, 14414–14423. [Google Scholar] [CrossRef] [Green Version]
- Golyandina, N.; Zhigljavsky, A. Singular Spectrum Analysis; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Lemmerling, P.; Van Huffel, S. Analysis of the structured total least squares problem for Hankel/Toeplitz matrices. Numer. Algorithms 2001, 27, 89–114. [Google Scholar] [CrossRef]
- Golub, G.H.; Reinsch, C. Singular Value Decomposition and Least Squares Solutions; Linear Algebra; Springer: Berlin/Heidelberg, Germany, 1971; pp. 134–151. [Google Scholar]
- Markowitz, W. Concurrent astronomical observations for studying continental drift, polar motion, and the rotation of the Earth. In Symposium-International Astronomical Union; Cambridge University Press: Cambridge, UK, 1968; Volume 32, pp. 25–32. [Google Scholar]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. Identification of Gleissberg cycles and a rising trend in a 315-year-long series of sunspot numbers. Sol. Phys. 2017, 292, 1–9. [Google Scholar] [CrossRef]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. Sea-Level Change at the Brest (France) Tide Gauge and the Markowitz Component of Earth’s Rotation. J. Coast. Res. 2021, 37, 683–690. [Google Scholar] [CrossRef]
- Chandler, S.C. On the variation of latitude, I. Astron. J. 1891, 11, 59–61. [Google Scholar] [CrossRef]
- Chandler, S.C. On the variation of latitude, II. Astron. J. 1891, 11, 65–70. [Google Scholar] [CrossRef]
- Zwally, H.J.; Comiso, J.C.; Parkinson, C.L.; Cavalieri, D.J.; Gloersen, P. Variability of Antarctic sea ice 1979–1998. J. Geophys. Res. 2002, 107, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J. Increasing Antarctic sea ice under warming atmospheric and oceanic conditions. J. Clim. 2007, 20, 2515–2529. [Google Scholar] [CrossRef] [Green Version]
- Turner, J.; Hosking, J.S.; Bracegirdle, T.J.; Marshall, G.J.; Phillips, T. Recent changes in Antarctic sea ice. Philos. Trans. Royal Soc. A 2015, 373, 20140163. [Google Scholar] [CrossRef]
- Jeffreys, H. Causes contributory to the Annual Variation of Latitude. Mon. Notices Royal Astron. Soc. 1916, 76, 499–525. [Google Scholar] [CrossRef] [Green Version]
- Lambeck, K. The Earth’s Variable Rotation: Geophysical Causes and Consequences; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Mechanics; Mir: Moscow, Russia, 1989. [Google Scholar]
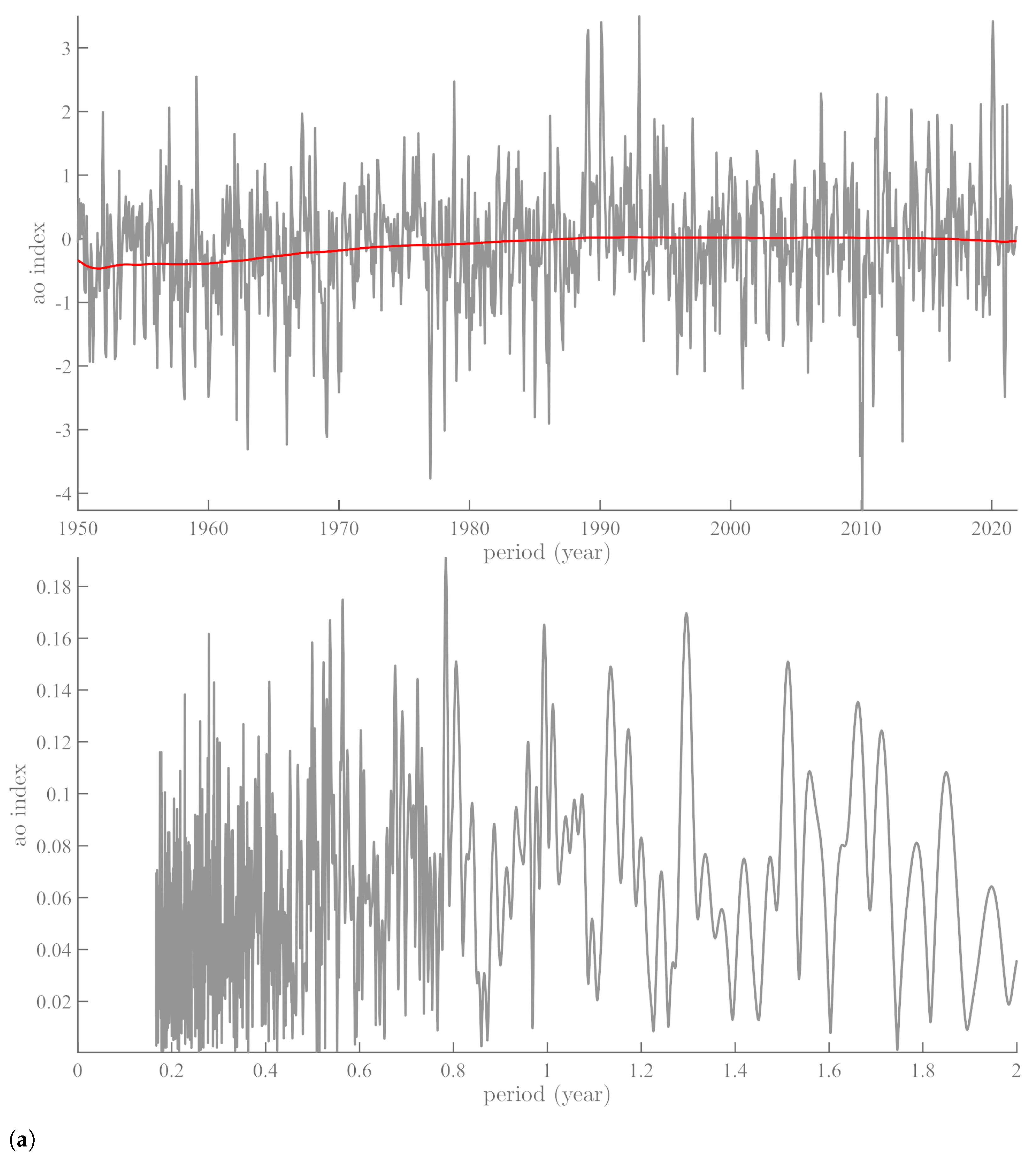
- Thompson, D.W.; Wallace, J.M. The Arctic Oscillation signature in the wintertime geopotential height and temperature fields. Geophys. Res. Lett. 1998, 25, 1297–1300. [Google Scholar] [CrossRef] [Green Version]
- Rigor, I.G.; Wallace, J.M.; Colony, R.L. Response of sea ice to the Arctic Oscillation. J. Clim. 2002, 15, 2648–2663. [Google Scholar] [CrossRef]
- Stroeve, J.; Holland, M.M.; Meier, W.; Scambos, T.; Serreze, M. Arctic sea ice decline: Faster than forecast. Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef]
- Serreze, M.C.; Meier, W.N. The Arctic’s sea ice cover: Trends, variability, predictability, and comparisons to the Antarctic. Ann. N. Y. Acad. Sci. 2019, 1436, 36–53. [Google Scholar] [CrossRef] [PubMed]
- Cohen, J.; Zhang, X.; Francis, J.; Jung, T.; Kwok, R.; Overland, J.; Yoon, J. Divergent consensuses on Arctic amplification influence on midlatitude severe winter weather. Nat. Clim. Chang. 2020, 10, 20–29. [Google Scholar] [CrossRef]
- White, W.B.; Peterson, R.G. An Antarctic circumpolar wave in surface pressure, wind, temperature and sea-ice extent. Nature 1996, 380, 699–702. [Google Scholar] [CrossRef]
- Courtillot, V.; Le Mouël, J.-L.; Lopes, F.; Gibert, D. On the Nature and Origin of Atmospheric Annual and Semi-Annual Oscillations. Atmosphere 2022, 13, 1907. [Google Scholar] [CrossRef]
- Schrauf, G. The first instability in spherical Taylor-Couette flow. J. Fluid Mech. 1986, 166, 287–303. [Google Scholar] [CrossRef] [Green Version]
- Mamun, C.K.; Tuckerman, L.S. Asymmetry and Hopf bifurcation in spherical Couette flow. Phys. Fluids 1995, 7, 80–91. [Google Scholar] [CrossRef] [Green Version]
- Nakabayashi, K.; Tsuchida, Y. Flow-history effect on higher modes in the spherical Couette system. J. Fluid Mech. 1995, 295, 43–60. [Google Scholar] [CrossRef]
- Hollerbach, R.; Junk, M.; Egbers, C. Non-axisymmetric instabilities in basic state spherical Couette flow. Fluid. Dyn. Res. 2006, 38, 257. [Google Scholar] [CrossRef]
- Mahloul, M.; Mahamdia, A.; Kristiawan, M. The spherical Taylor–Couette flow. Eur. J. Mech. B Fluids 2016, 59, 1–6. [Google Scholar] [CrossRef]
- Garcia, F.; Seilmayer, M.; Giesecke, A.; Stefani, F. Modulated rotating waves in the magnetised spherical Couette system. J. Nonlinear Sci. 2019, 29, 2735–2759. [Google Scholar] [CrossRef] [Green Version]
- Mannix, P.M.; Mestel, A.J. Bistability and hysteresis of axisymmetric thermal convection between differentially rotating spheres. J. Fluid Mech. 2021, 911. [Google Scholar] [CrossRef]
- Taylor, G.I. VIII. Stability of a viscous liquid contained between two rotating cylinders. Philos. Trans. R. Soc. Ser. A Contain. Pap. Math. Phys. Character 1923, 223, 289–343. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Fluid Mechanics; Pergamonl: New York, NY, USA, 1959; Volume 61. [Google Scholar]
- Chandrasekhar, S. Hydrodynamic and Hydromagnetic Stability; Oxford University Press: Oxford, UK, 1961. [Google Scholar]
- Frisch, U. Turbulence: The Legacy of AN Kolmogorov; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Holland, M.M.; Landrum, L.; Raphael, M.; Stammerjohn, S. Springtime winds drive Ross Sea ice variability and change in the following autumn. Nature Comm. 2017, 8, 1–8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bertler, N.A.; Conway, H.; Dahl-Jensen, D.; Emanuelsson, D.B.; Winstrup, M.; Vallelonga, P.T.; Zhang, X. The Ross Sea Dipole–temperature, snow accumulation and sea ice variability in the Ross Sea region, Antarctica, over the past 2700 years. Clim. Past 2018, 14, 193–214. [Google Scholar] [CrossRef] [Green Version]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. A strong link between variations in sea-ice extent and global atmospheric pressure? Cryosphere Dis. 2021, 1–28. [Google Scholar] [CrossRef]





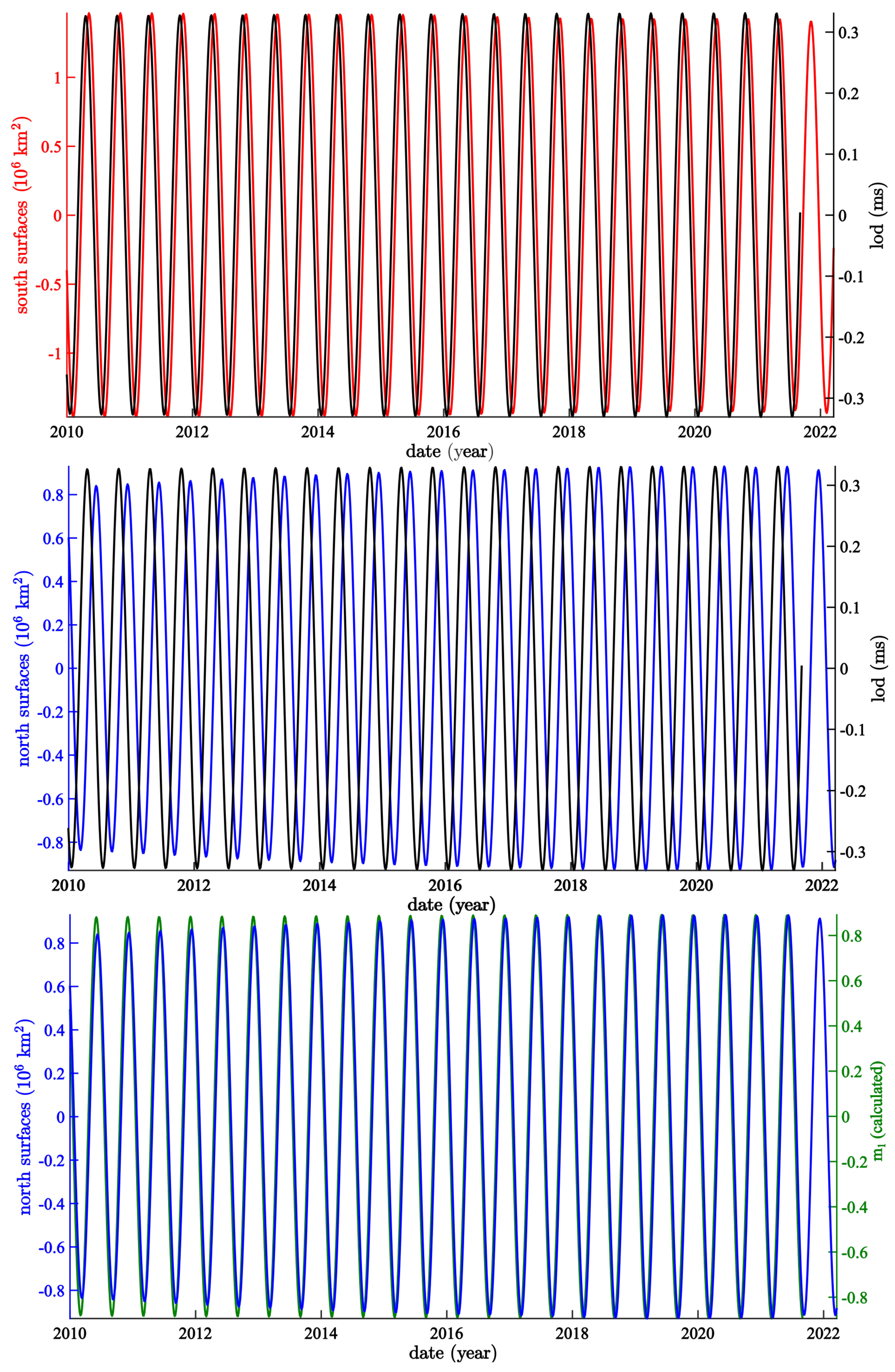

| D | NHSI | SHSI | |
|---|---|---|---|
| lod | 152.5 ± 4.1 | 33.0 ± 1.1 | 153.7 ± 7.1 |
| 65.6 ± 2.1 | 153.8 ± 4.2 | 11.5 ± 2.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lopes, F.; Courtillot, V.; Gibert, D.; Mouël, J.-L.L. On the Annual and Semi-Annual Components of Variations in Extent of Arctic and Antarctic Sea-Ice. Geosciences 2023, 13, 21. https://doi.org/10.3390/geosciences13010021
Lopes F, Courtillot V, Gibert D, Mouël J-LL. On the Annual and Semi-Annual Components of Variations in Extent of Arctic and Antarctic Sea-Ice. Geosciences. 2023; 13(1):21. https://doi.org/10.3390/geosciences13010021
Chicago/Turabian StyleLopes, Fernando, Vincent Courtillot, Dominique Gibert, and Jean-Louis Le Mouël. 2023. "On the Annual and Semi-Annual Components of Variations in Extent of Arctic and Antarctic Sea-Ice" Geosciences 13, no. 1: 21. https://doi.org/10.3390/geosciences13010021






