Evaluation of Strength Anisotropy in Foliated Metamorphic Rocks: A Review Focused on Microscopic Mechanisms
Abstract
:1. Introduction
2. Strength Anisotropy
3. Microstructural Characteristics
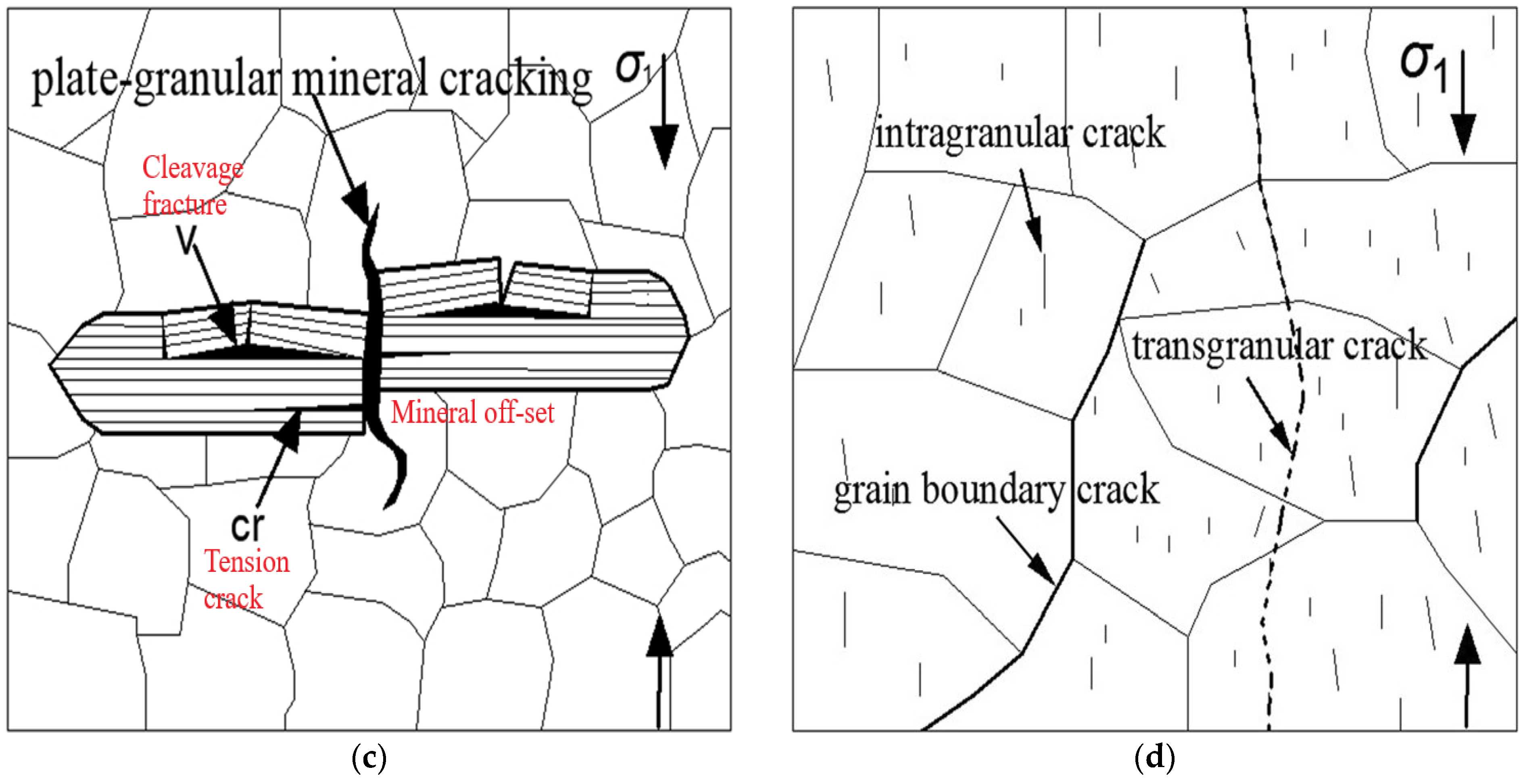
4. Damage Mechanism of Mica and Granular Minerals
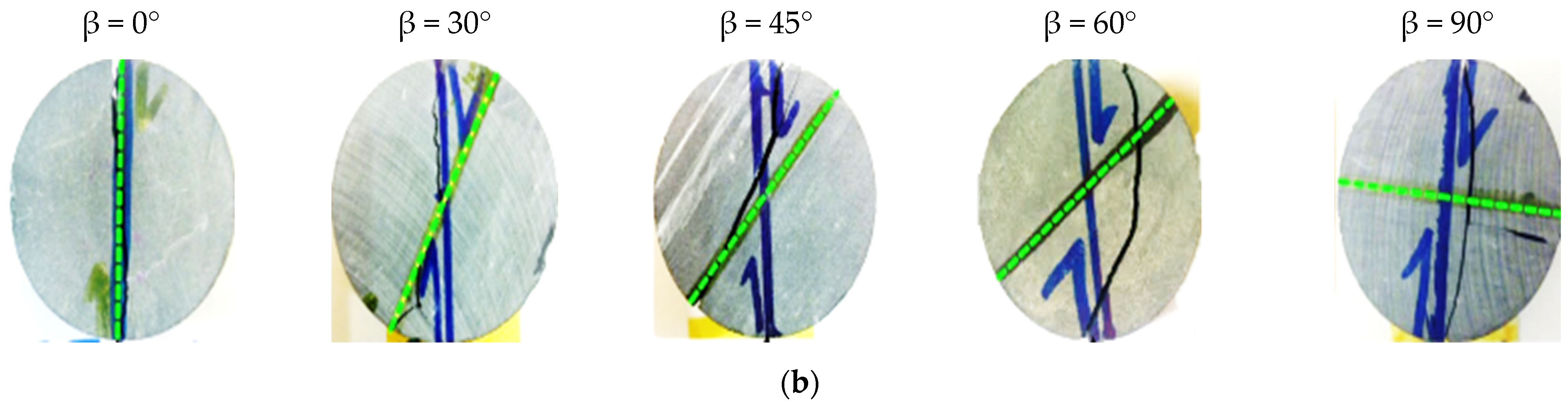
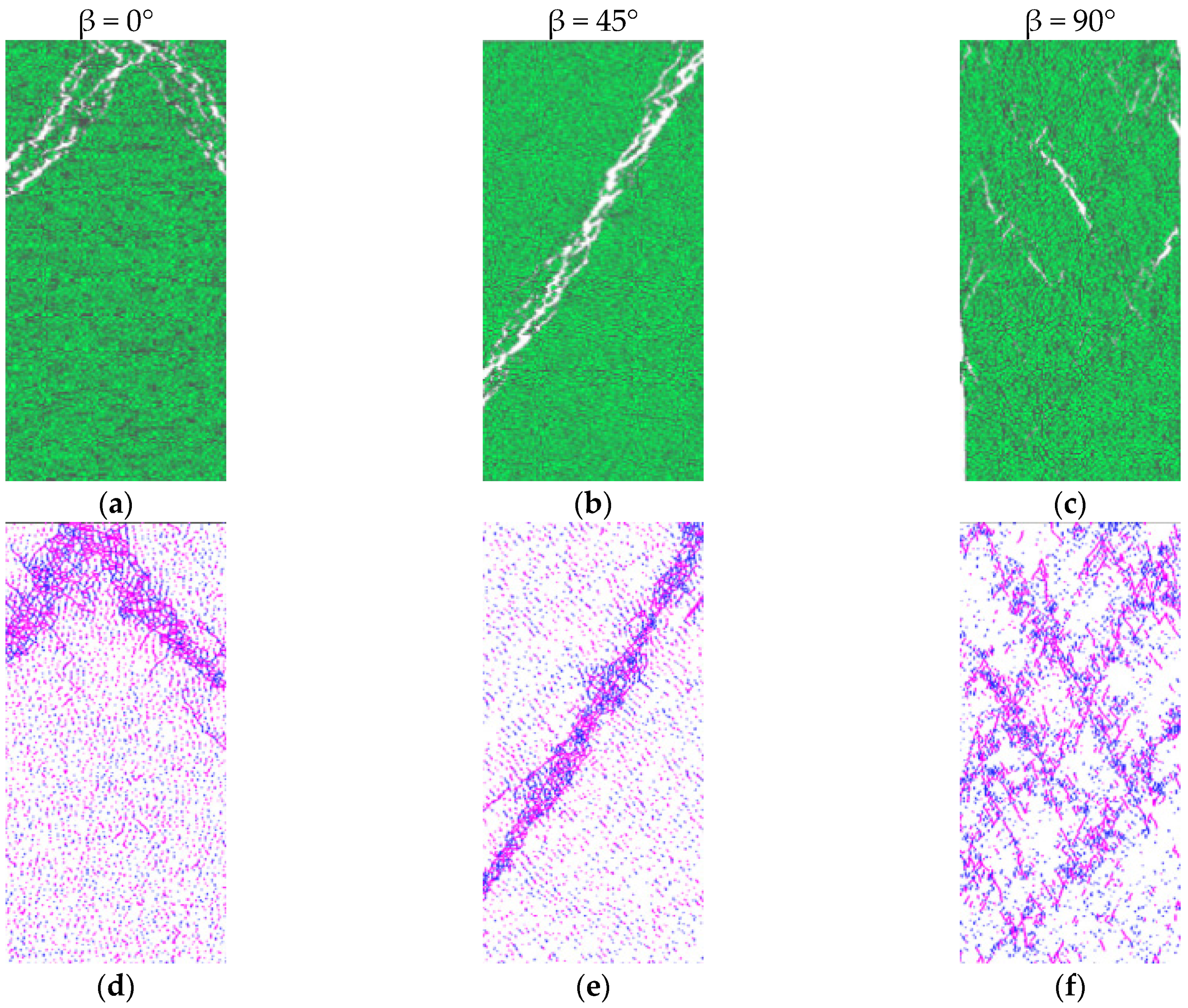
5. Damage Mechanism and Mode of Failures
6. Failure Criteria
7. Discontinuum Modeling and Simulation
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bagde, M.N. An investigation into strength and porous properties of metamorphic rocks in the Himalayas: A case study. Geotech. Geol. Eng. 2000, 18, 209–219. [Google Scholar] [CrossRef]
- Hoek, E. Fracture of anisotropic rock. J. S. Afr. Inst. Min. Metall. 1964, 64, 501–518. [Google Scholar]
- Nasseri, M.H.; Rao, K.S.; Ramamurthy, T. Failure mechanism in schistose rocks. Int. J. Rock Mech. Min. Sci. 1997, 34, 219-e1. [Google Scholar] [CrossRef]
- Özbek, A. Variation of Schmidt hammer values with imbrication direction in clastic sedimentary rocks. Int. J. Rock Mech. Min. Sci. 2009, 46, 548–554. [Google Scholar] [CrossRef]
- Heng, S.; Guo, Y.; Yang, C.; Daemen, J.J.; Li, Z. Experimental and theoretical study of the anisotropic properties of shale. Int. J. Rock Mech. Min. Sci. 2015, 74, 58–68. [Google Scholar] [CrossRef]
- Ahmed, M.F.; Waqas, U.; Arshad, M.; Rogers, J.D. Effect of heat treatment on dynamic properties of selected rock types taken from the Salt Range in Pakistan. Arab. J. Geosci. 2018, 11, 728. [Google Scholar] [CrossRef]
- Waqas, U.; Ahmed, M.F. Investigation of strength behavior of thermally deteriorated sedimentary rocks subjected to dynamic cyclic loading. Int. J. Rock Mech. Min. Sci. 2022, 158, 105201. [Google Scholar] [CrossRef]
- Ramamurthy, T.; Rao, G.V.; Singh, J. Engineering behavior of phyllites. Eng. Geol. 1993, 33, 209–225. [Google Scholar] [CrossRef]
- Bozkurt, E.; Oberhänsli, R. Menderes Massif (western Turkey): Structural, metamorphic and magmatic evolution—A synthesis. Int. J. Earth Sci. 2001, 89, 679–708. [Google Scholar] [CrossRef]
- Garzón, E.; Sánchez-Soto, P.J.; Romero, E. Physical and geotechnical properties of clay phyllites. Appl. Clay Sci. 2010, 48, 307–318. [Google Scholar] [CrossRef]
- Karaman, K.; Kesimal, A.; Ersoy, H. A comparative assessment of indirect methods for estimating the uniaxial compressive and tensile strength of rocks. Arab. J. Geosci. 2015, 8, 2393–2403. [Google Scholar] [CrossRef]
- Tursi, F. The key role of µ H2O gradients in deciphering microstructures and mineral assemblages of mylonites: Examples from the Calabria polymetamorphic terrane. Mineral. Petrol. 2022, 116, 1–14. [Google Scholar] [CrossRef]
- Yin, X.; Huang, Y.; Zhang, A.; Lei, Y. Effect of Fabric Factors on the Mechanical Behavior of Foliated Rocks: A Particle Flow Approach. Geotech. Geol. Eng. 2023, 41, 1031–1047. [Google Scholar] [CrossRef]
- Özbek, A.; Gül, M.; Karacan, E.; Alca, Ö. Anisotropy effect on strengths of metamorphic rocks. J. Rock Mech. Geotech. Eng. 2018, 10, 164–175. [Google Scholar] [CrossRef]
- Goshtasbi, K.; Ahmadi, M.; Seyedi, J. Anisotropic strength behaviour of slates in the Sirjan-Sanadaj zone. J. S. Afr. Inst. Min. Metall. 2006, 106, 71–75. [Google Scholar]
- Askaripour, M.; Saeidi, A.; Mercier-Langevin, P.; Rouleau, A. A review of relationship between texture characteristic and mechanical properties of rock. Geotechnics 2022, 2, 262–296. [Google Scholar] [CrossRef]
- Mclamore, R.; Gray, K.E. The mechanical behavior of anisotropic sedimentary rocks. J. Eng. Ind. 1967, 89, 62–73. [Google Scholar] [CrossRef]
- Singh, J.; Ramamurthy, T.; Venkatappa Rao, G. Strength anisotropies in rocks. Indian Geotech. J. 1989, 19, 147–166. [Google Scholar]
- Kwasniewski, M.A. Mechanical behavior of anisotropic rocks. Compr. Rock Eng. 1993, 1, 285–312. [Google Scholar]
- Nasseri, M.H.B.; Rao, K.S.; Ramamurthy, T. Anisotropic strength and deformational behavior of Himalayan schists. Int. J. Rock Mech. Min. Sci. 2003, 40, 3–23. [Google Scholar] [CrossRef]
- Cho, J.W.; Kim, H.; Jeon, S.; Min, K.B. Deformation and strength anisotropy of Asan gneiss, Boryeong shale, and Yeoncheon schist. Int. J. Rock Mech. Min. Sci. 2012, 50, 158–169. [Google Scholar] [CrossRef]
- Yin, X.; Zhang, Y.; Lei, Y.; Wang, L. Linear failure criterion for estimating the compressive strength of brittle foliated rocks in response to the loading direction. Bull. Eng. Geol. Environ. 2022, 81, 189. [Google Scholar] [CrossRef]
- Deklotz, E.J.; Brown, J.W.; Stemler, O.A. Anisotropy of a schistose gneiss. In Proceedings of the ISRM Congress, Lisbon, Portugal, 25 September–1 October 1966; p. ISRM-1CONGRESS. [Google Scholar]
- Brown, E.T. Shear strength characteristics of Delabole slates. In Proceedings of the Conference on Rock Engineering, New Castle upon Tyne, UK, 4–7 April 1977; pp. 31–51. [Google Scholar]
- Tien, Y.M.; Tsao, P.F. Preparation and mechanical properties of artificial transversely isotropic rock. Int. J. Rock Mech. Min. Sci. 2000, 37, 1001–1012. [Google Scholar] [CrossRef]
- Donath, F. Strength variation and deformational behavior in anisotropic rock. State Stress Earth’s Crust 1964, 281. [Google Scholar]
- Chenevert, M.E.; Gatlin, C. Mechanical anisotropies of laminated sedimentary rocks. Soc. Pet. Eng. J. 1965, 5, 67–77. [Google Scholar] [CrossRef]
- Stagg, K.G.; Zienkiewicz, O.C. Rock Mechanics in Engineering Practice; Wiley: London, UK, 1968. [Google Scholar]
- Attewell, P.B.; Sandford, M.R. Intrinsic shear strength of a brittle, anisotropic rock—I: Experimental and mechanical interpretation. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1974, 11, 423–430. [Google Scholar] [CrossRef]
- Ramamurthy, T.; Rao, G.V.; Singh, J. A strength criterion for anisotropic rocks. In Fifth Australia-New Zealand Conference on Geomechanics: Prediction Versus Performance; Preprints of Papers; Institution of Engineers: Barton, Australia, 1988; pp. 253–257. [Google Scholar]
- Behrestaghi, M.H.N.; Rao, K.S.; Ramamurthy, T. Engineering geological and geotechnical responses of schistose rocks from dam project areas in India. Eng. Geol. 1996, 44, 183–201. [Google Scholar] [CrossRef]
- Khanlari, G.R.; Heidari, M.; Sepahigero, A.A.; Fereidooni, D. Quantification of strength anisotropy of metamorphic rocks of the Hamedan province, Iran, as determined from cylindrical punch, point load and Brazilian tests. Eng. Geol. 2014, 169, 80–90. [Google Scholar] [CrossRef]
- Fereidooni, D.; Khanlari, G.R.; Heidari, M.; Sepahigero, A.A.; Kolahi-Azar, A.P. Assessment of inherent anisotropy and confining pressure influences on mechanical behavior of anisotropic foliated rocks under triaxial compression. Rock Mech. Rock Eng. 2016, 49, 2155–2163. [Google Scholar] [CrossRef]
- Jaeger, J.C. Shear failure of anistropic rocks. Geol. Mag. 1960, 97, 65–72. [Google Scholar] [CrossRef]
- Saroglou, H.; Tsiambaos, G. Classification of anisotropic rocks. In Proceedings of the 11th ISRM Congress, Lisbon, Portugal, 9–13 July 2007; pp. 9–13. [Google Scholar]
- Weng, M.C.; Li, J.H.; Lin, C.H.; Liao, C.T. Evaluating foliation tensile strength of metamorphic rock by using pull-off test. In Proceedings of the ISRM International Symposium-Asian Rock Mechanics Symposium, Singapore, 29 October–3 November 2018; p. ISRM-ARMS10. [Google Scholar]
- Barla, G.; Innaurato, N. Indirect tensile testing of anisotropic rocks. Rock Mech. 1973, 5, 215–230. [Google Scholar] [CrossRef]
- Nova, R.; Zaninetti, A. An investigation into the tensile behaviour of a schistose rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1990, 27, 231–242. [Google Scholar] [CrossRef]
- Amadei, B. Importance of anisotropy when estimating and measuring in situ stresses in rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1996, 33, 293–325. [Google Scholar] [CrossRef]
- Liao, J.J.; Yang, M.T.; Hsieh, H.Y. Direct tensile behavior of a transversely isotropic rock. Int. J. Rock Mech. Min. Sci. 1997, 34, 837–849. [Google Scholar] [CrossRef]
- Chen, C.S.; Hsu, S.C. Measurement of indirect tensile strength of anisotropic rocks by the ring test. Rock Mech. Rock Eng. 2001, 34, 293–321. [Google Scholar] [CrossRef]
- Alam, M.R.; Swamidas, A.S.J.; Gale, J.; Munaswamy, K. Mechanical and physical properties of slate from Britannia Cove, Newfoundland. Can. J. Civ. Eng. 2008, 35, 751–755. [Google Scholar] [CrossRef]
- Perras, M.A.; Diederichs, M.S. A review of the tensile strength of rock: Concepts and testing. Geotech. Geol. Eng. 2014, 32, 525–546. [Google Scholar] [CrossRef]
- Rastegar, F.; Nejati, H.R.; Ghazvinian, A.; Hadei, M.R.; Nazerigivi, A. On Applicability of Some Indirect Tests for Estimation of Tensile Strength of Anisotropic Rocks. J. Min. Environ. 2020, 11, 711–720. [Google Scholar]
- Weng, M.C.; Li, H.H.; Fu, Y.Y.; Fang, C.H.; Chen, H.R.; Chang, C.Y. A failure criterion for foliation and its application for strength estimation of foliated metamorphic rock. Int. J. Rock Mech. Min. Sci. 2022, 153, 105086. [Google Scholar] [CrossRef]
- Hobbs, D.W. The tensile strength of rocks. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1964, 1, 385–396. [Google Scholar] [CrossRef]
- McCabe, W.M.; Koerner, R.M. High pressure shear strength investigation of an anisotropic mica schist rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1975, 12, 219–228. [Google Scholar] [CrossRef]
- Shea Jr, W.T.; Kronenberg, A.K. Strength and anisotropy of foliated rocks with varied mica contents. J. Struct. Geol. 1993, 15, 1097–1121. [Google Scholar] [CrossRef]
- Button, E.A.; Blümel, M. Characterization of Phyllitic and Schistose rock masses: From System Behaviour to Key Parameters. In Proceedings of the ISRM Regional Symposion Eurock: Rock Engineering: From Theory to Practice, Salzburg, Austria, 7–9 October 2004; pp. 459–464. [Google Scholar]
- Cacciari, P.P.; Futai, M.M. Effects of mica content on rock foliation strength. Int. J. Rock Mech. Min. Sci. 2019, 124, 104143. [Google Scholar] [CrossRef]
- Dev, H. Shear strength of rock mass—Interpretation and analysis. In Proceedings of the International Conference On Engineering Geological Solutions for Sustainable Development (EGCON-2017), New Delhi, India, 7–8 October 2017. [Google Scholar]
- Hoek, E.; Brown, E.T. Underground Excavations in Rock; CRC Press: Boca Raton, FL, USA, 1980. [Google Scholar]
- Saeidi, O.; Vaneghi, R.G.; Rasouli, V.; Gholami, R. A modified empirical criterion for strength of transversely anisotropic rocks with metamorphic origin. Bull. Eng. Geol. Environ. 2013, 72, 257–269. [Google Scholar] [CrossRef]
- Donath, F.A. Effects of cohesion and granularity on deformational behavior of anisotropic rock. Stud. Mineral. Precambrian Geol. 1972, 135, 95–128. [Google Scholar]
- Gottschalk, R.R.; Kronenberg, A.K.; Russell, J.E.; Handin, J. Mechanical anisotropy of gneiss: Failure criterion and textural sources of directional behavior. J. Geophys. Res. Solid Earth 1990, 95, 21613–21634. [Google Scholar] [CrossRef]
- Powell, D.; Nicolas, A.; Poirier, J.P. Crystalline Plasticity and Solid State Flow in Metamorphic Rocks. Mineral. Mag. 1977, 41, 422. [Google Scholar] [CrossRef]
- Olsson, W.A.; Peng, S.S. Microcrack nucleation in marble. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1976, 13, 53–59. [Google Scholar] [CrossRef]
- Yin, X.; Zhang, A.; Zhang, X. A review of the influence of microscopic characteristics on the progressively brittle failure of foliated rocks subjected to compression loading. Geotech. Geol. Eng. 2021, 40, 1663–1673. [Google Scholar] [CrossRef]
- Fisher, G.W. The application of ionic equilibria to metamorphic differentiation: An example. Contrib. Mineral. Petrol. 1970, 29, 91–103. [Google Scholar] [CrossRef]
- Waqas, U.; Ahmed, M.F.; Arshad, M. Classification of the intact carbonate and silicate rocks based on their degree of thermal cracking using discriminant analysis. Bull. Eng. Geol. Environ. 2020, 79, 2607–2619. [Google Scholar] [CrossRef]
- Waqas, U.; Rashid, H.M.A.; Ahmed, M.F.; Rasool, A.M.; Al-Atroush, M.E. Damage characteristics of thermally deteriorated carbonate rocks: A review. Appl. Sci. 2022, 12, 2752. [Google Scholar] [CrossRef]
- Diederichs, M.S.; Kaiser, P.K.; Eberhardt, E. Damage initiation and propagation in hard rock during tunnelling and the influence of near-face stress rotation. Int. J. Rock Mech. Min. Sci. 2004, 41, 785–812. [Google Scholar] [CrossRef]
- Silk, E.C.H.; Barnes, R.S. The observation of dislocations in mica. Acta Metall. 1961, 9, 558–562. [Google Scholar] [CrossRef]
- Hörz, F. Static and dynamic origin of kink bands in micas. J. Geophys. Res. 1970, 75, 965–977. [Google Scholar] [CrossRef]
- Gay, N.C.; Weiss, L.E. The relationship between principal stress directions and the geometry of kinks in foliated rocks. Tectonophysics 1974, 21, 287–300. [Google Scholar] [CrossRef]
- Bell, I.A.; Wilson, C.J.L.; McLaren, A.C.; Etheridge, M.A. Kinks in mica: Role of dislocations and (001) cleavage. Tectonophysics 1986, 127, 49–65. [Google Scholar] [CrossRef]
- Kronenberg, A.K.; Kirby, S.H.; Pinkston, J. Basal slip and mechanical anisotropy of biotite. J. Geophys. Res. Solid Earth 1990, 95, 19257–19278. [Google Scholar] [CrossRef]
- Kanaori, Y.; Kawakami, S.I.; Yairi, K. Microstructure of deformed biotite defining foliation in cataclasite zones in granite, central Japan. J. Struct. Geol. 1991, 13, 777–785. [Google Scholar] [CrossRef]
- Mares, V.M.; Kronenberg, A.K. Experimental deformation of muscovite. J. Struct. Geol. 1993, 15, 1061–1075. [Google Scholar] [CrossRef]
- Dunham, R.E.; Crider, J.G.; Burmester, R.F.; Schermer, E.R.; Housen, B.A. Geometry, microstructures, and magnetic fabrics of kink bands in the Darrington Phyllite, northwestern Washington, USA: Processes within fixed-hinge kinking. J. Struct. Geol. 2011, 33, 1627–1638. [Google Scholar] [CrossRef]
- Wang, J.X. Research for the Anisotropy of Quartz Mica Schist and Its Effect on the Stability of the Surrounding Rock; Chengdu University of Technology: Chengdu, China, 2014. [Google Scholar]
- Zhang, X.P.; Wong, L.N.Y.; Wang, S.J.; Han, G.Y. Engineering properties of quartz mica schist. Eng. Geol. 2011, 121, 135–149. [Google Scholar] [CrossRef]
- Rawling, G.; Baud, P.; Wong, T. Dilatancy, brittle strength, and anisotropy of foliated rocks: Experimental deformation and micromechanical modeling. J. Geophys. Res. 2002, 107, ETG-8. [Google Scholar] [CrossRef]
- Christoffersen, R.; Kronenberg, A.K. Dislocation interactions in experimentally deformed biotite. J. Struct. Geol. 1993, 15, 1077–1095. [Google Scholar] [CrossRef]
- Ma, J.; Niu, X.; Xiong, C.; Lu, S.; Xia, D.; Zhang, B.; Tang, H. Experimental investigation of the physical properties and microstructure of slate under wetting and drying cycles using micro-CT and ultrasonic wave velocity tests. Sensors 2020, 20, 4853. [Google Scholar] [CrossRef]
- Sagong, M.; Bobet, A. Coalescence of multiple flaws in a rock-model material in uniaxial compression. Int. J. Rock. Mech. Min. Sci. 2002, 39, 229–241. [Google Scholar] [CrossRef]
- Yang, L.; Mei, J.; Li, S.C. Research on the initiation and propagation modes of 3-D crack under hydro-mechanical coupling. Adv. Eng. Sci. 2018, 50, 174–183. [Google Scholar]
- Berčáková, A.; Melichar, R.; Souček, K. Mechanical properties and failure patterns of migmatized gneiss with metamorphic foliation under UCS test. Rock Mech. Rock Eng. 2020, 53, 2007–2013. [Google Scholar] [CrossRef]
- Agliardi, F.; Zanchetta, S.; Crosta, G.B. Fabric controls on the brittle failure of folded gneiss and schist. Tectonophysics 2014, 637, 150–162. [Google Scholar] [CrossRef]
- Ohtsu, M.; Isoda, T.; Tomoda, Y. Acoustic emission techniques standardized for concrete structures. J. Acoust. Emiss. 2007, 25, 21–32. [Google Scholar]
- Basu, A.; Mishra, D.A.; Roychowdhury, K. Rock failure modes under uniaxial compression, Brazilian, and point load tests. Bull. Eng. Geol. Environ. 2013, 72, 457–475. [Google Scholar] [CrossRef]
- Condon, K.J.; Sone, H.; Wang, H.F. Anisotropic Strength and Elastic Properties of Poorman Schist at the EGS Collab Experiment 1 Site. In Proceedings of the ARMA US Rock Mechanics/Geomechanics Symposium, New York, NY, USA, 23–26 June 2019; p. ARMA-2019. [Google Scholar]
- Zhou, Y.; Su, S.R.; Li, P.; Ma, H.; Zhang, X. Microstructure and mechanical properties of broken phyllite. J. Jilin Univ. (Earth Sci. Ed.) 2019, 49, 504–513. [Google Scholar]
- Condon, K.J.; Sone, H.; Wang, H.F. Low static shear modulus along foliation and its influence on the elastic and strength anisotropy of Poorman Schist Rocks, Homestake Mine, South Dakota. Rock Mech. Rock Eng. 2020, 53, 5257–5281. [Google Scholar] [CrossRef]
- Li, Z.; Xu, G.; Dai, Y.; Zhao, X.; Fu, Y. Effects of foliation on deformation and failure mechanism of silty slates. Int. J. Rock Mech. Min. Sci. 2021, 141, 104703. [Google Scholar] [CrossRef]
- Alejano, L.; González-Fernández, M.; Estévez-Ventosa, X.; Song, F.; Delgado-Martín, J.; Muñoz-Ibáñez, A.; González-Molano, N.; Alvarellos, J. Anisotropic deformability and strength of slate from NW-Spain. Int. J. Rock Mech. Min. Sci. 2021, 148, 104923. [Google Scholar] [CrossRef]
- Wen, G.; Hu, J.; Wu, Y.; Zhang, Z.X.; Xu, X.; Xiang, R. Mechanical Properties and Failure Behavior of Dry and Water-Saturated Foliated Phyllite under Uniaxial Compression. Materials 2022, 15, 8962. [Google Scholar] [CrossRef] [PubMed]
- Cacciari, P.P.; Futai, M.M. Assessing the tensile strength of rocks and geological discontinuities via pull-off tests. Int. J. Rock Mech. Min. Sci. 2018, 105, 44–52. [Google Scholar] [CrossRef]
- Passchier, C.W.; Trouw, R.A. Microtectonics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Wu, C.; Chen, Q.; Basack, S.; Karekal, S. Laboratory investigation on rheological properties of greenschist considering anisotropy under multi-stage compressive creep condition. J. Struct. Geol. 2018, 114, 111–120. [Google Scholar] [CrossRef]
- Ambrose, J. Failure of Anisotropic Shales under Triaxial Stress Conditions. Ph.D. Thesis, Imperial College London, London, UK, 2014. [Google Scholar]
- Pariseau, W.G. Plasticity theory for anisotropic rocks and soil. In Proceedings of the ARMA US Rock Mechanics/Geomechanics Symposium, Austin, TX, USA, 20–22 May 1968; p. ARMA-68. [Google Scholar]
- Tsai, S.W.; Wu, E.M. A general theory of strength for anisotropic materials. J. Compos. Mater. 1971, 5, 58–80. [Google Scholar] [CrossRef]
- Boehler, J.P.; Sawczuk, A. On yielding of oriented solids. Acta Mech. 1977, 27, 185–204. [Google Scholar] [CrossRef]
- Ashour, H.A. A compressive strength criterion for anisotropic rock materials. Can. Geotech. J. 1988, 25, 233–237. [Google Scholar] [CrossRef]
- Cazacu, O.; Cristescu, N.D. Failure of Anisotropic Compressible Shale (No. CONF-950686-); University of California: Los Angeles, CA, USA, 1995. [Google Scholar]
- Kusabuka, M.; Takeda, H.; Kojo, H. Anisotropic yield function for rocks and evaluation of material parameters. In Congrès International de Mécanique des Roches; A.A. Balkema: Rotterdam, The Netherlands, 1999; pp. 921–924. [Google Scholar]
- Lee, Y.K.; Pietruszczak, S. Application of critical plane approach to the prediction of strength anisotropy in transversely isotropic rock masses. Int. J. Rock Mech. Min. Sci. 2008, 45, 513–523. [Google Scholar] [CrossRef]
- Mróz, Z.; Maciejewski, J. Critical plane approach to analysis of failure criteria for anisotropic geomaterials. In Bifurcations, Instabilities and Degradations in Geomaterials; Springer: Berlin/Heidelberg, Germany, 2011; pp. 69–89. [Google Scholar]
- Pei, J.; Einstein, H.H.; Whittle, A.J. The normal stress space and its application to constructing a new failure criterion for cross-anisotropic geomaterials. Int. J. Rock Mech. Min. Sci. 2018, 106, 364–373. [Google Scholar] [CrossRef]
- Jaeger, J.C.; Cook, N.G. Fundamentals of Rock Mechanics; Chapman and Hall: London, UK, 1979. [Google Scholar]
- Zienkiewicz, O.C.; Pande, G.N. Time-dependent multilaminate model of rocks—A numerical study of deformation and failure of rock masses. Int. J. Numer. Anal. Methods Geomech. 1977, 1, 219–247. [Google Scholar] [CrossRef]
- Pijaudier-Cabot, G.; Bažant, Z.P. Nonlocal damage theory. J. Eng. Mech. 1987, 113, 1512–1533. [Google Scholar] [CrossRef]
- Singh, B.; Goel, R.K.; Mehrotra, V.K.; Garg, S.K.; Allu, M.R. Effect of intermediate principal stress on strength of anisotropic rock mass. Tunn. Undergr. Space Technol. 1998, 13, 71–79. [Google Scholar]
- Tien, Y.M.; Kuo, M.C.; Juang, C.H. An experimental investigation of the failure mechanism of simulated transversely isotropic rocks. Int. J. Rock Mech. Min. Sci. 2006, 43, 1163–1181. [Google Scholar] [CrossRef]
- Saroglou, H.; Tsiambaos, G. A modified Hoek–Brown failure criterion for anisotropic intact rock. Int. J. Rock Mech. Min. Sci. 2008, 45, 223–234. [Google Scholar] [CrossRef]
- Rafiai, H. New empirical polyaxial criterion for rock strength. Int. J. Rock Mech. Min. Sci. 2011, 48, 922–931. [Google Scholar] [CrossRef]
- Hobbs, D.W. Rock tensile strength and its relationship to a number of alternative measures of rock strength. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1967, 4, 115–127. [Google Scholar] [CrossRef]
- Barron, K. Brittle fracture initiation in and ultimate failure of rocks: Part I—Anisotropic rocks: Theory. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1971, 8, 553–563. [Google Scholar] [CrossRef]
- Li, L.; Aubertin, M. Critère de rupture multiaxial pour les roches avec une anisotropie planaire. In Proceedings of the 53rd Canadian Geotechnical Conference, Montréal, QC, Canada, 15–18 October 2000. [Google Scholar]
- Claesson, J.; Bohloli, B. Brazilian test: Stress field and tensile strength of anisotropic rocks using an analytical solution. Int. J. Rock Mech. Min. Sci. 2002, 39, 991–1004. [Google Scholar] [CrossRef]
- Lee, Y.-K.; Pietruszczak, S. Tensile failure criterion for transversely isotropic rocks. Int. J. Rock Mech. Min. Sci. 2015, 79, 205–215. [Google Scholar] [CrossRef]
- Gao, F.; Stead, D.; Elmo, D. Numerical simulation of microstructure of brittle rock using a grain-breakable distinct element grain-based model. Comput. Geotech. 2016, 78, 203–217. [Google Scholar] [CrossRef]
- Wang, X.; Cai, M. A comprehensive parametric study of grain-based models for rock failure process simulation. Int. J. Rock Mech. Min. Sci. 2019, 115, 60–76. [Google Scholar] [CrossRef]
- Pant, S.R.; Adhikary, D.P.; Dyskin, A.V. Slope failure in a foliated rock mass with non-uniform joint spacing: A comparison between numerical and centrifuge model results. Rock Mech. Rock Eng. 2015, 48, 403–407. [Google Scholar] [CrossRef]
- Bouzeran, L.; Pierce, M.; Andrieux, P.; Williams, E. The role of rock mass heterogeneity and buckling mechanisms in excavation performance in foliated ground at Westwood Mine, Quebec. J. S. Afr. Inst. Min. Metall. 2020, 120, 41–48. [Google Scholar] [CrossRef]
- Wang, S.Y.; Sloan, S.W.; Tang, C.A.; Zhu, W.C. Numerical simulation of the failure mechanism of circular tunnels in transversely isotropic rock masses. Tunn. Undergr. Space Technol. 2012, 32, 231–244. [Google Scholar] [CrossRef]
- Lisjak, A.; Grasselli, G. A review of discrete modeling techniques for fracturing processes in discontinuous rock masses. J. Rock Mech. Geotech. Eng. 2014, 6, 301–314. [Google Scholar] [CrossRef]
- Cundall, P.A. A discontinuous future for numerical modelling in geomechanics. Proc. Inst. Civ. Eng.-Geotech. Eng. 2001, 149, 41–47. [Google Scholar] [CrossRef]
- Olsen-Kettle, L.; Dautriat, J.; Sarout, J. Impact of stress-induced rock damage on elastic symmetry: From transverse isotropy to orthotropy. Rock Mech. Rock Eng. 2022, 55, 3061–3081. [Google Scholar] [CrossRef]
- Yang, Q.; Leng, K. A microplane-based anisotropic damage effective stress. Int. J. Damage Mech. 2014, 23, 178–191. [Google Scholar] [CrossRef]
- Voyiadjis, G.Z.; Kattan, P.I. A comparative study of damage variables in continuum damage mechanics. Int. J. Damage Mech. 2009, 18, 315–340. [Google Scholar] [CrossRef]
- Potyondy, D.O.; Cundall, P.A. A bonded-particle model for rock. Int. J. Rock Mech. Min. Sci. 2004, 41, 1329–1364. [Google Scholar] [CrossRef]
- Tang, C.A.; Liu, H.; Lee, P.K.K.; Tsui, Y.; Tham, L. Numerical studies of the influence of microstructure on rock failure in uniaxial compression—Part I: Effect of heterogeneity. Int. J. Rock Mech. Min. Sci. 2000, 37, 555–569. [Google Scholar] [CrossRef]
- Cai, M.; Kaiser, P.K. Numerical simulation of the Brazilian test and the tensile strength of anisotropic rocks and rocks with pre-existing cracks. Int. J. Rock Mech. Min. Sci. 2004, 41, 478–483. [Google Scholar] [CrossRef]
- Lan, H.; Martin, C.D.; Hu, B. Effect of heterogeneity of brittle rock on micromechanical extensile behavior during compression loading. J. Geophys. Res. Solid Earth 2010, 115, B01202. [Google Scholar] [CrossRef]
- Ghazvinian, E.; Diederichs, M.S.; Quey, R. 3D random Voronoi grain-based models for simulation of brittle rock damage and fabric-guided micro-fracturing. J. Rock Mech. Geotech. Eng. 2014, 6, 506–521. [Google Scholar] [CrossRef]
- Azocar, K.D. Investigating the Mesh Dependency and Upscaling of 3D Grain-Based Models for the Simulation of Brittle Fracture Processes in Low-Porosity Crystalline Rock. Ph.D. Thesis, Queen’s University, Kingston, ON, Canada, 2016. [Google Scholar]
- Farahmand, K.; Diederichs, M.S. A calibrated Synthetic Rock Mass (SRM) model for simulating crack growth in granitic rock considering grain scale heterogeneity of polycrystalline rock. In Proceedings of the ARMA US Rock Mechanics/Geomechanics Symposium, San Francisco, CA, USA, 28 June–1 July 2015; p. ARMA-2015. [Google Scholar]
- Cho, N.A.; Martin, C.D.; Sego, D.C. A clumped particle model for rock. Int. J. Rock Mech. Min. Sci. 2007, 44, 997–1010. [Google Scholar] [CrossRef]
- Karampinos, E.; Hadjigeorgiou, J.; Turcotte, P.; Drolet, M.M.; Mercier-Langevin, F. Empirical and numerical investigation on the behaviour of foliated rock masses under high stress conditions. In Deep Mining 2014: Proceedings of the Seventh International Conference on Deep and High Stress Mining; Australian Centre for Geomechanics: Perth, Australia, 2014; pp. 345–361. [Google Scholar]
- Park, B.; Min, K.B. Bonded-particle discrete element modeling of mechanical behavior of transversely isotropic rock. Int. J. Rock Mech. Min. Sci. 2015, 76, 243–255. [Google Scholar] [CrossRef]
- Duan, K.; Kwok, C.Y. Discrete element modeling of anisotropic rock under Brazilian test conditions. Int. J. Rock Mech. Min. Sci. 2015, 78, 46–56. [Google Scholar] [CrossRef]
- Chong, Z.; Li, X.; Hou, P.; Chen, X.; Wu, Y. Moment tensor analysis of transversely isotropic shale based on the discrete element method. Int. J. Min. Sci. Technol. 2017, 27, 507–515. [Google Scholar] [CrossRef]
- Xia, L.; Zeng, Y. Parametric study of smooth joint parameters on the mechanical behavior of transversely isotropic rocks and research on calibration method. Comput. Geotech. 2018, 98, 1–7. [Google Scholar] [CrossRef]
- Park, B.; Min, K.B.; Thompson, N.; Horsrud, P. Three-dimensional bonded-particle discrete element modeling of mechanical behavior of transversely isotropic rock. Int. J. Rock Mech. Min. Sci. 2018, 110, 120–132. [Google Scholar] [CrossRef]
- Zhang, Y.; Shao, J.; de Saxcé, G.; Shi, C.; Liu, Z. Study of deformation and failure in an anisotropic rock with a three-dimensional discrete element model. Int. J. Rock Mech. Min. Sci. 2019, 120, 17–28. [Google Scholar] [CrossRef]
- Li, K.; Yin, Z.Y.; Cheng, Y.; Cao, P.; Meng, J. Three-dimensional discrete element simulation of indirect tensile behaviour of a transversely isotropic rock. Int. J. Numer. Anal. Methods Geomech. 2020, 44, 1812–1832. [Google Scholar] [CrossRef]
- He, F.; Liu, Q.; Deng, P. Investigation of the anisotropic characteristics of layered rocks under uniaxial compression based on the 3D printing technology and the combined finite-discrete element method. Adv. Mater. Sci. Eng. 2020, 2020, 8793214. [Google Scholar] [CrossRef]
- Shang, J.; Duan, K.; Gui, Y.; Handley, K.; Zhao, Z. Numerical investigation of the direct tensile behaviour of laminated and transversely isotropic rocks containing incipient bedding planes with different strengths. Comput. Geotech. 2018, 104, 373–388. [Google Scholar] [CrossRef]
- Yao, C.; Jiang, Q.H.; Shao, J.F.; Zhou, C.B. A discrete approach for modeling damage and failure in anisotropic cohesive brittle materials. Eng. Fract. Mech. 2016, 155, 102–118. [Google Scholar] [CrossRef]
- Debecker, B.; Vervoort, A. Two-dimensional discrete element simulations of the fracture behaviour of slate. Int. J. Rock Mech. Min. Sci. 2013, 61, 161–170. [Google Scholar] [CrossRef]
- Chiu, C.C.; Wang, T.T.; Weng, M.C.; Huang, T.H. Modeling the anisotropic behavior of jointed rock mass using a modified smooth-joint model. Int. J. Rock Mech. Min. Sci. 2013, 62, 14–22. [Google Scholar] [CrossRef]
- Chang, C.T.; Monteiro, P.; Nemati, K.; Shyu, K. Behavior of marble under compression. J. Mater. Civ. Eng. 1996, 8, 157–170. [Google Scholar] [CrossRef]
- Jing, L. Formulation of discontinuous deformation analysis (DDA)—An implicit discrete element model for block systems. Eng. Geol. 1998, 49, 371–381. [Google Scholar] [CrossRef]
- Shahami, M.H.; Bafghi, A.Y.; Marji, M.F. Investigating the effect of external forces on the displacement accuracy of discontinuous deformation analysis (DDA) method. Comput. Geotech. 2019, 111, 313–323. [Google Scholar] [CrossRef]
- Lin, C.T.; Amadei, B.; Jung, J.; Dwyer, J. Extensions of discontinuous deformation analysis for jointed rock masses. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1996, 33, 671–694. [Google Scholar] [CrossRef]
- Shi, G.H. Discontinuous deformation analysis: A new numerical model for the statics and dynamics of deformable block structures. Eng. Comput. 1992, 9, 157–168. [Google Scholar] [CrossRef]
- Sun, J.; Ning, Y.; Zhao, Z. Comparative study of Sarma’s method and the discontinuous deformation analysis for rock slope stability analysis. Geomech. Geoengin. 2011, 6, 293–302. [Google Scholar] [CrossRef]
- Zhang, X.L.; Jiao, Y.Y.; Liu, Q.S.; Chen, W.Z. Modeling of stability of a highway tunnel by using improved DDA method. Yantu Lixue (Rock Soil Mech.) 2007, 28, 1710–1714. [Google Scholar]
- Chen, G. Numerical modelling of rock fall using extended DDA. Yanshilixue Yu Gongcheng Xuebao/Chin. J. Rock Mech. Eng. 2003, 22, 926–931. [Google Scholar]
- Fu, X.; Kang, J.; Sheng, Q.; Zheng, L.; Du, W.; Ding, H. Investigation of 2D Seismic DDA Method for Numerical Simulation of Shaking Table Test of Rock Mass Engineering. Mathematics 2022, 10, 1330. [Google Scholar] [CrossRef]
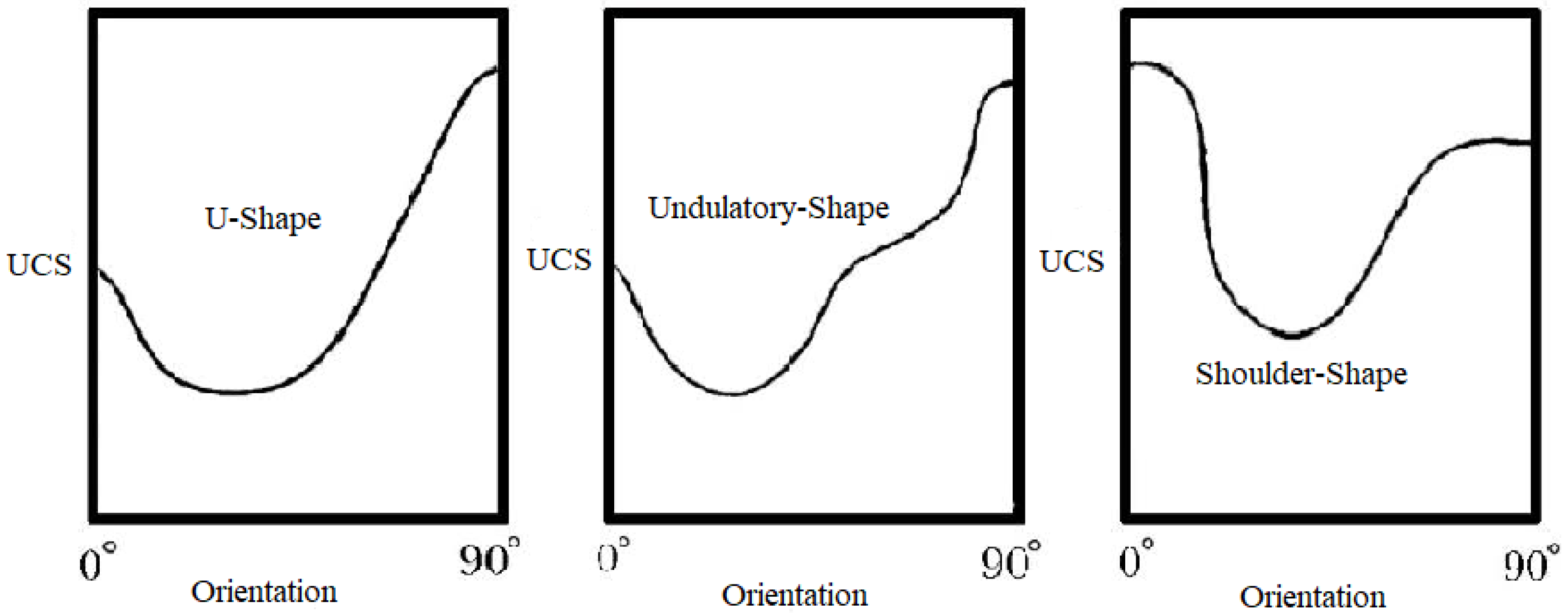
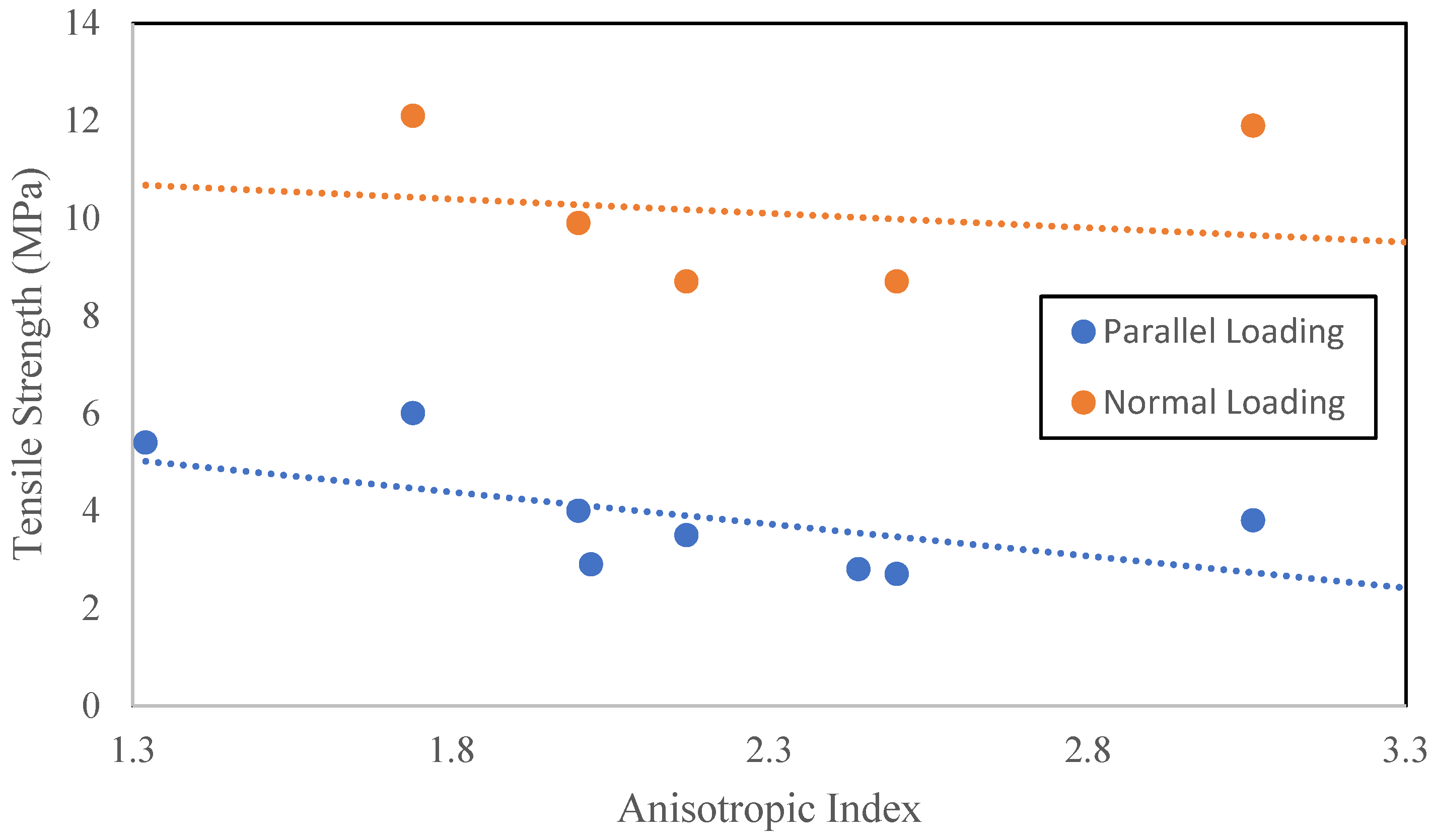
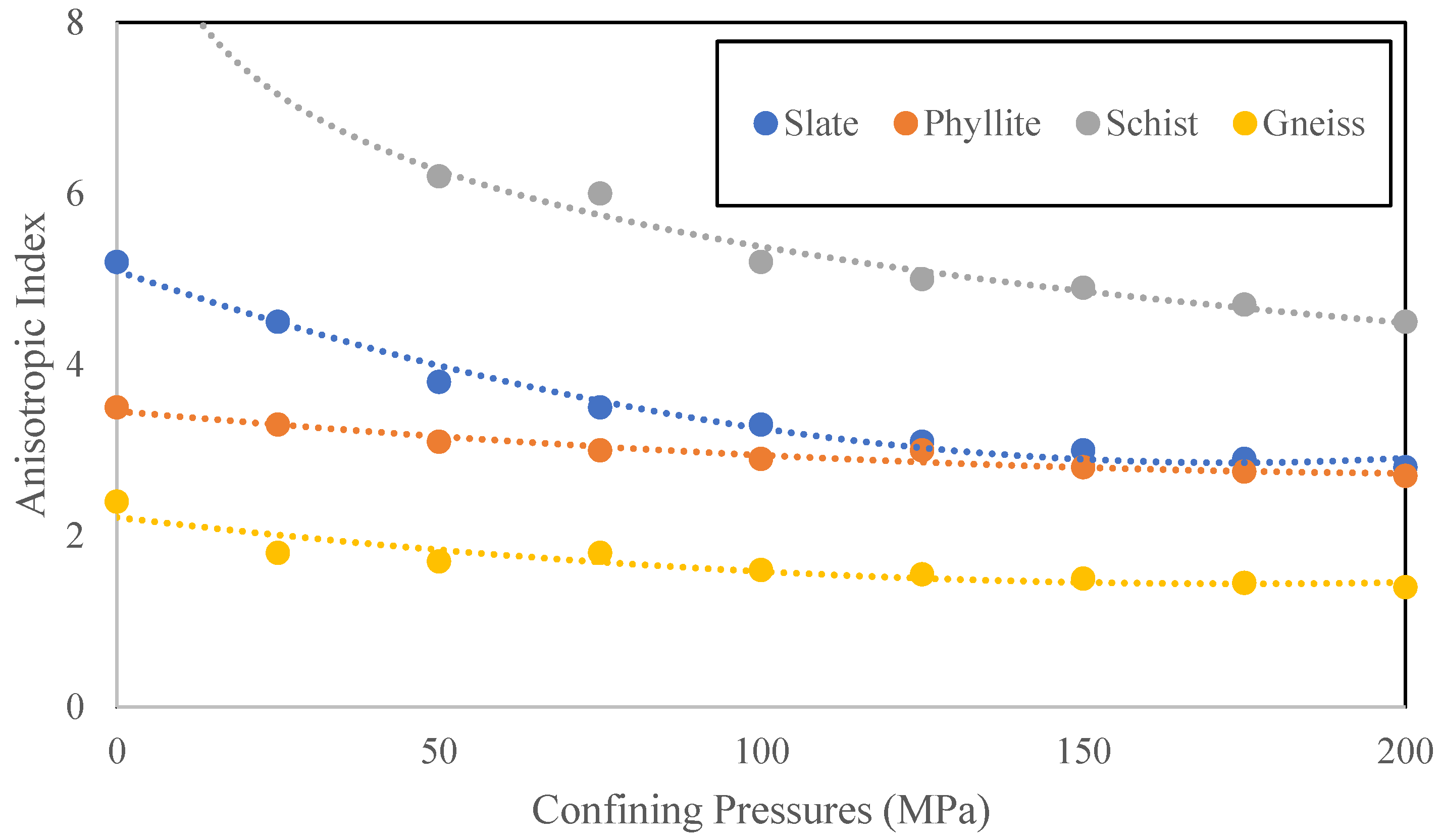



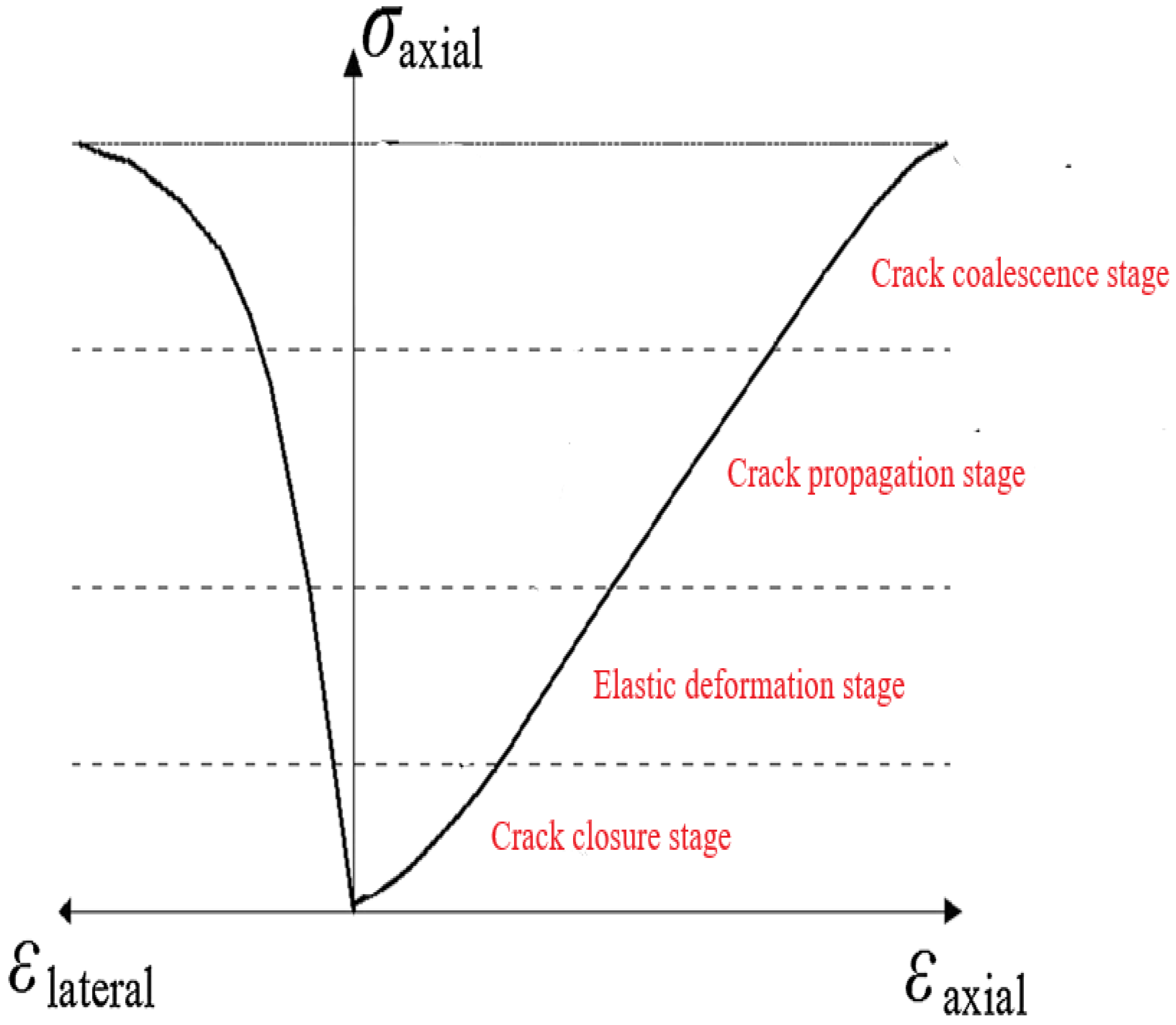
| Strength Ratio | Anisotropic Class | Reference |
|---|---|---|
| 1.1–2.0 | Weakly Anisotropic | [8] |
| 2.0–4.0 | Moderately Anisotropic | |
| 4.0–6.0 | Highly Anisotropic | |
| >6.0 | Very Highly Anisotropic | |
| 1.1–2.0 | Fairly Anisotropic | [35] |
| 2.0–3.0 | Moderately Anisotropic | |
| 3.0–5.0 | Highly Anisotropic | |
| >5.0 | Very Highly Anisotropic |
| Applied Load | Rock Type | Mode of Failure | Reference |
|---|---|---|---|
| Uniaxial Compression | Schist | β = 0° axial tensile splitting, 0° < β < 90° failure along foliation, and β = 90° shear failure | [81] |
| Triaxial Compression | Slate, Phyllite, Schist | β = 0°–60° slip failure along foliations and β = 60°–90° shear failure cross-cutting the foliations | [33] |
| Uniaxial Compression | Schist | β = 0° shear failure cross-cutting the foliations, β = 35° shear failure along foliations, and β = 45°–60° shear failure along and cross-cutting the foliations | [90] |
| Uniaxial Compression | Schist | β = 0° axial tensile splitting, β = 45°–60° slip failure along foliations, and β = 90° shear failure cross-cutting the foliations | [82] |
| Uniaxial Compression | Gneiss | β = 0°–30° splitting along foliations, β = ≥45° combined shear-tensile failure, and β = 60°–90° conjugate shear failure cross-cutting the foliations | [78] |
| Uniaxial Compression | Slate | β = 0° tensile splitting along foliations and β = 90° shear failure cross-cutting the foliations | [85] |
| Triaxial Compression | Slate | β = 0° double cone failure, 15° < β < 75° clean sliding along foliation, and β = 90° axial splitting | [86] |
| Uniaxial Compression | Phyllite | β = 0°–30° tensile-split and shear-slip along foliations, β = 45°–60° dominant shear-slip failure, and β = 60°–90° shear failure cross-cutting the foliations | [87] |
| Brazilian Tensile Test | Schist | β = 90° central tensile failure, non-central tensile failure, combined central tensile failure with shear crack, and multiple central tensile cracks | [81] |
| Brazilian Tensile Test | Phyllite | β = 0°–30° central tensile failure, β = 30°–60° slip in foliations or slip in foliations combined with central crack, and at β = 60°–90° non-central tensile failure | [44] |
| Major Developments in Failure Criteria | Reference |
|---|---|
| A criterion was proposed for anisotropic geological media based on the theory of plasticity. | [92] |
| A simple strength criterion was put forward based on the scalar function and strength tensors. | [93] |
| The yielding and failure criteria were developed for transversely isotropic-oriented materials using information from tensorial generators, independent, and mixed stresses. | [94] |
| A criterion was proposed for compressed anisotropic rocks subjected to a multiaxial state of stress considering the Von Mises failure criterion for ductile materials. | [95] |
| A failure criterion for compressed anisotropic rocks was formulated based on viscoplastic constitutive modeling. In this criterion, the failure function was dependent on the stress tensor invariants and mixed invariants. | [96] |
| A criterion was presented that generates an anisotropic yield envelope based on the 9 material parameters. The nonlinear yield function varies with hydrostatic pressure and orientation angle alteration. | [97] |
| A strength criterion was put forward to evaluate the anisotropy of transversely isotropic rocks under a multiaxial stress state. The direction of the failure plane was predicted using the Hoek-Brown failure criterion and critical plane method. | [98] |
| A failure criterion was derived for anisotropic geomaterials under a multiaxial stress state using the density distribution function of internal defects and damage evaluation rules. Furthermore, the failure plane’s orientation was also estimated using a critical plane approach. | [99] |
| A new criterion was proposed to predict the anisotropic strength of transversely isotropic rocks using the Matsuoka–Nakai criterion for isotropic materials and the Coulomb criterion. | [100] |
| Grain Characteristics | Contact Characteristics |
|---|---|
| Grain density (kg/m3) | Contact normal stiffness Jkn (GPa/m) |
| Elastic modulus E (GPa) | Contact shear stiffness Jks (GPa/m) |
| Poisson’s ratio | Contact stiffness ratio Jkn/Jks |
| Compressive strength UCS (MPa) | Contact compressive strength (MPa) |
| Tensile strength UTS (MPa) | Contact tensile strength (MPa) |
| Peak shear strength Cp (MPa) | Contact peak shear strength JCp (MPa) |
| Peak friction angle (deg) | Contact peak friction angle (deg) |
| Peak dilation angle (deg) | - |
| Residual shear strength Cr (MPa) | Contact residual shear strength JCr (MPa) |
| Residual friction angle (deg) | Contact residual friction angle (deg) |
| Residual dilation angle (deg) | - |
| Plastic strain (%) | - |
| DEM Simulation for Anisotropic Rocks | Reference |
|---|---|
| A 3D DEM was presented to investigate the squeezing ground condition in mines. The effect of buckling, weak planes’ orientation, and fractures on rock mass was modeled using the DEM approach. | [131] |
| Presented a systematically verified model for anisotropic rocks using bonded particle modeling (BPM) and smooth joint modeling (SJM). The model was extended from laboratory scale to field to solve upscaled engineering problems. | [132] |
| The effect of weak layer orientations on strength, stiffness, and fracture pattern was simulated using smooth joint modeling (SJM). | [133] |
| To evaluate the fracturing event in transversely isotropic rocks, the moment tensor was analyzed using acoustic emission (AE) based DEM. The AE agreed the deterioration of rock under deviatoric stress and DEM simulated the damage processes of rocks. | [134] |
| Simulated the relationship between microscopic characteristics of foliations with the macroscopic mechanical behavior of rocks using bonded particle modeling (BPM) and embedded smooth joint modeling (SJM). | [135] |
| A 3D bonded particle DEM with varying smooth joint contact ratios was used for the simulation of the elastic and strength behavior of anisotropic rocks. | [136] |
| The influence of confinement and weak planes on both microscopic and macroscopic failure processes was simulated using 3D DEM. The behavior of rock matrix and weak planes was modeled by nonlinear bond contact and smooth joint contact approaches. | [137] |
| A 3D DEM was proposed for anisotropic rocks using the flat joint model and smooth joint model to investigate the effect of weak planes’ orientation on tensile strength, fracture pattern, and stress distribution. | [138] |
| Investigated the anisotropic behavior of foliated rocks using 3D printing technology and hybrid finite discrete element modeling (FDEM) approach. Angular variation in weak plane orientation considerably affects the mechanical behavior of layered rocks. | He et al. 2020 [139] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Waqas, U.; Qureshi, M.U.; Saqib, S.; Rashid, H.M.A.; Rasool, A.M. Evaluation of Strength Anisotropy in Foliated Metamorphic Rocks: A Review Focused on Microscopic Mechanisms. Geosciences 2024, 14, 253. https://doi.org/10.3390/geosciences14100253
Waqas U, Qureshi MU, Saqib S, Rashid HMA, Rasool AM. Evaluation of Strength Anisotropy in Foliated Metamorphic Rocks: A Review Focused on Microscopic Mechanisms. Geosciences. 2024; 14(10):253. https://doi.org/10.3390/geosciences14100253
Chicago/Turabian StyleWaqas, Umer, Mohsin Usman Qureshi, Shahab Saqib, Hafiz Muhammad Awais Rashid, and Ali Murtaza Rasool. 2024. "Evaluation of Strength Anisotropy in Foliated Metamorphic Rocks: A Review Focused on Microscopic Mechanisms" Geosciences 14, no. 10: 253. https://doi.org/10.3390/geosciences14100253
APA StyleWaqas, U., Qureshi, M. U., Saqib, S., Rashid, H. M. A., & Rasool, A. M. (2024). Evaluation of Strength Anisotropy in Foliated Metamorphic Rocks: A Review Focused on Microscopic Mechanisms. Geosciences, 14(10), 253. https://doi.org/10.3390/geosciences14100253