The Application of Information Theory to Interpret Shore Platform Erosion Rates
Abstract
:1. Introduction
2. Study Area
3. Materials and Methods
3.1. Traversing Micro-Erosion Meter (TMEM)
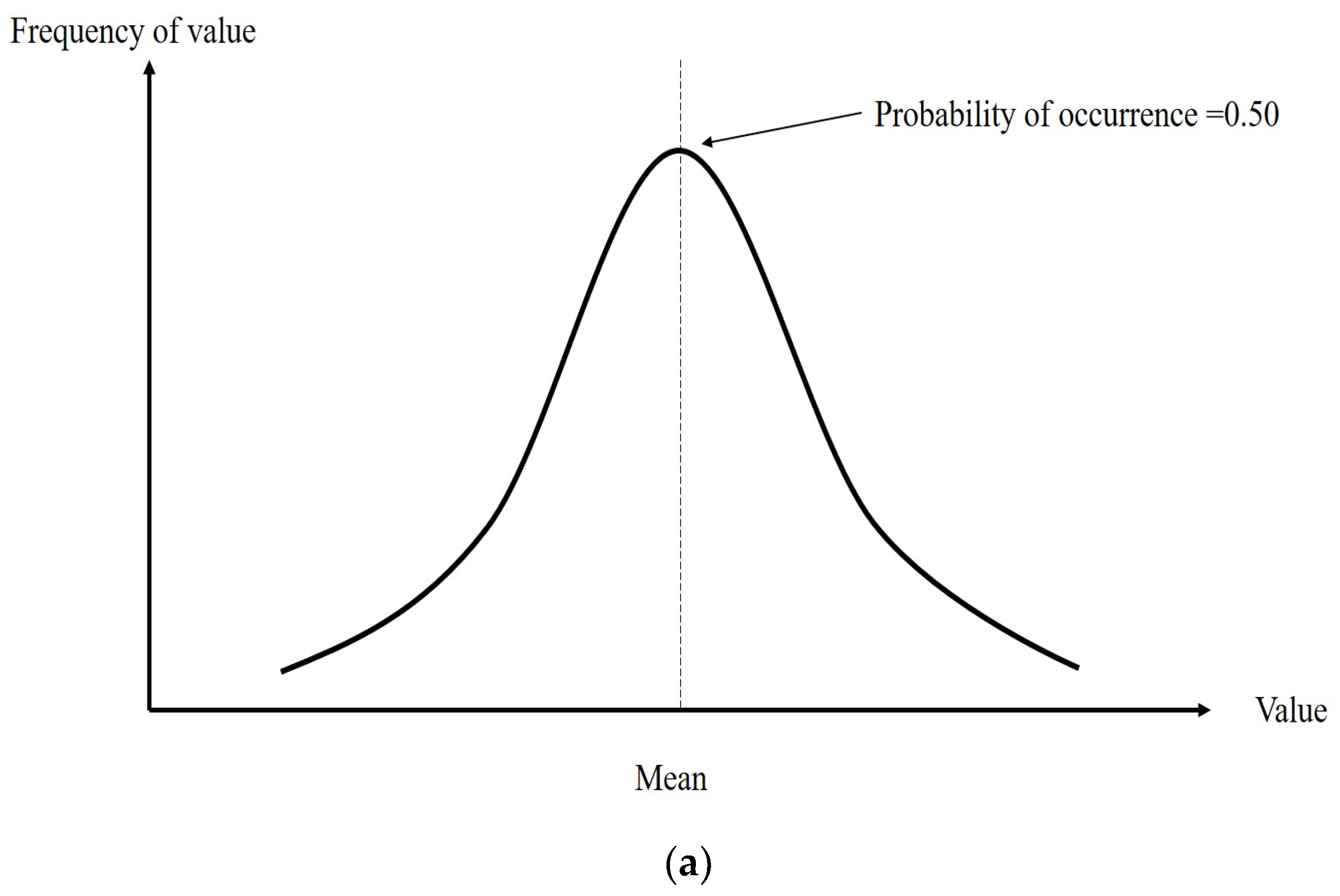
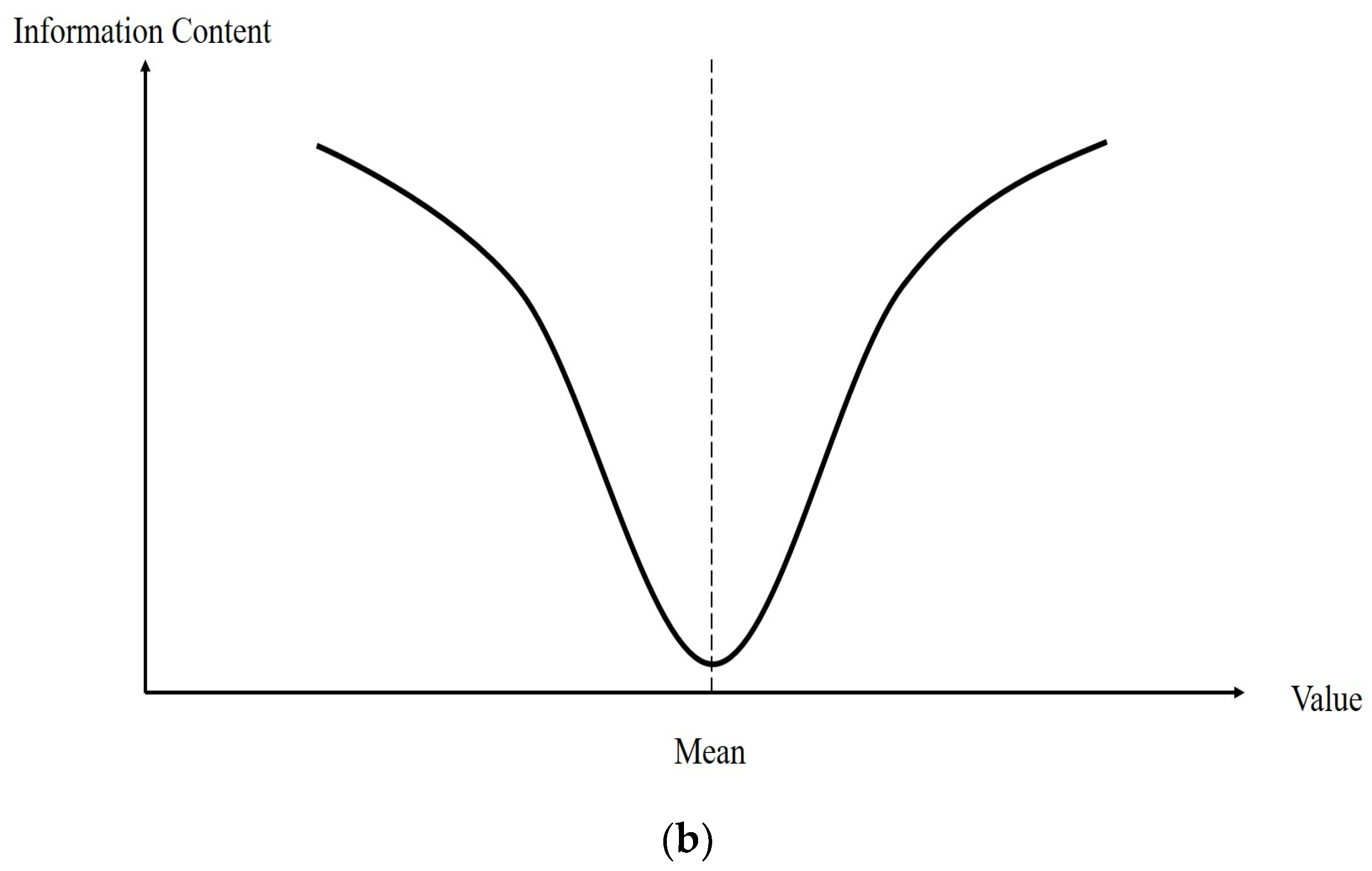
3.2. Constructing a Probability Distribution for Erosion Rates
4. Research Questions
- Is there variability in erosion rates and information content of different time periods at each bolt site?
- If so, are these patterns consistent for both erosion rates and information content?
- Is there variability in the erosion rates and information of the same time period across all bolt sites?
- If so, are these patterns consistent for both erosion rates and information content?
- Is there variability between the bolt sites on the same platform in information content?
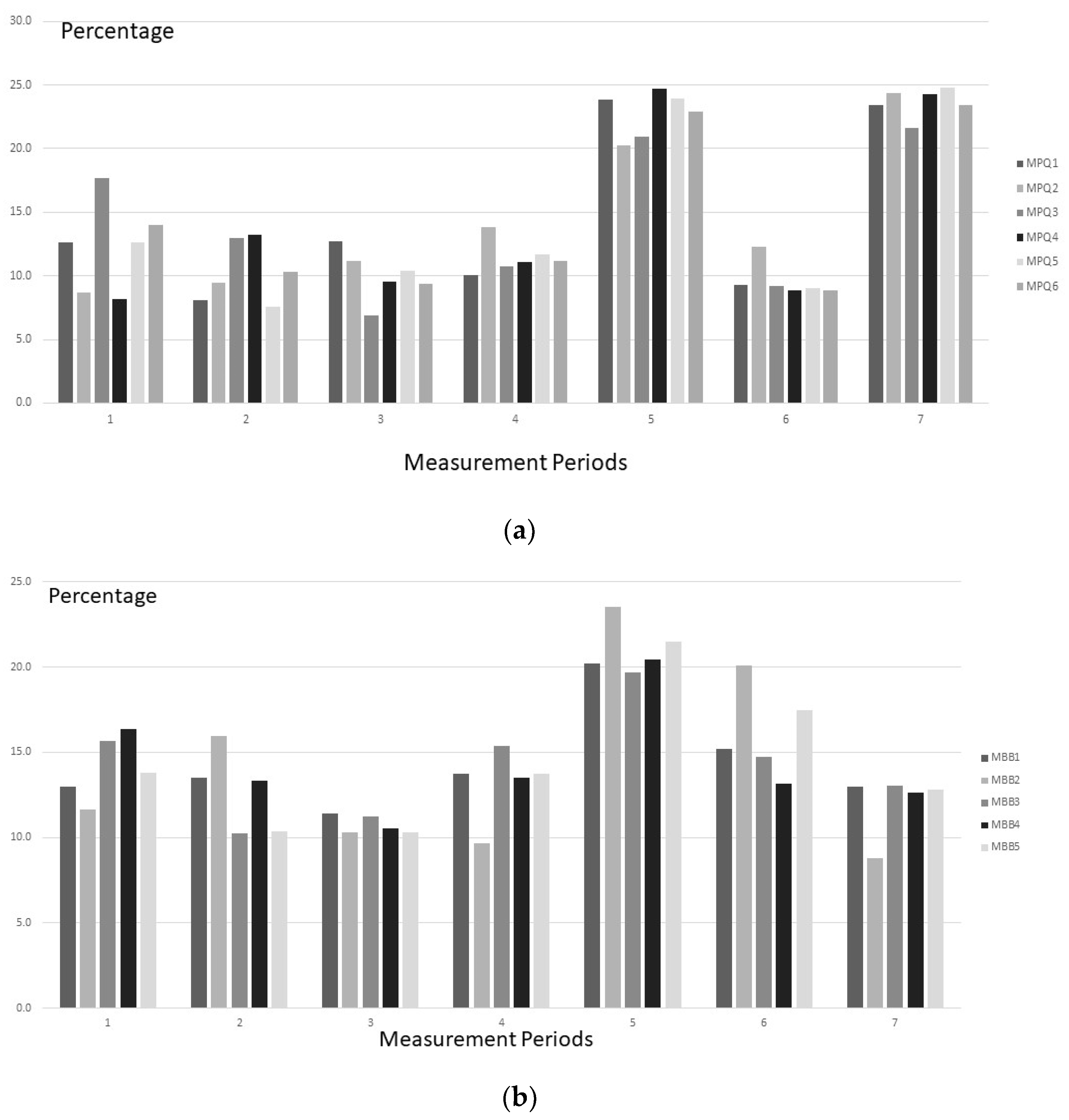
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fan, J.; Meng, J.; Ludescher, J.; Chen, X.; Ashkenazy, Y.; Kurths, J.; Havlin, S.; Schellnhuber, H.J. Statistical physics approaches to the complex Earth system. Phys. Rep. 2021, 896, 1–84. [Google Scholar] [PubMed]
- Krakauer, D.; Bertschinger, N.; Olbrich, E.; Flack, J.C.; Ay, N. The information theory of individuality. Theory Biosci. 2020, 139, 209–223. [Google Scholar] [CrossRef]
- Avery, J.S. Information Theory and Evolution; World Scientific: Singapore, 2021. [Google Scholar]
- Vopson, M.M.; Robson, S.C. A new method to study genome mutations using the information entropy. Phys. A 2021, 584, 12638. [Google Scholar] [CrossRef]
- Vopson, M. A possible information entropic law of genetic mutations. Appl. Sci. 2022, 12, 6912. [Google Scholar] [CrossRef]
- Vopson, M. The mass-energy-information equivalence principle. AIP Adv. 2019, 9, 095206. [Google Scholar] [CrossRef]
- Carbone, S.; Grasso, M.; Lentini, F.; Pedley, H.M. The distribution and palaeoenvironment of Early Miocene phosphorites of southeast Sicily and their relationships with the Maltese phosphorites. Palaeogeogr. Palaeoclimatol. Palaeoecol. 1987, 58, 35–53. [Google Scholar] [CrossRef]
- Gauci, R.; Scerri, S. A synthesis of different geomorphological landscapes on the Maltese islands. In Landscapes and Landforms of the Maltese Islands; Gauci, R., Schembri, J.A., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 49–65. [Google Scholar]
- Gauci, R.; Inkpen, R. The physical characteristics of limestone shore platforms on the Maltese islands and their neglected contribution to coastal land use development. In Landscapes and Landforms of the Maltese Islands; Gauci, R., Schembri, J.A., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 343–356. [Google Scholar]
- Pedley, H.M. Oligocene-Miocene Stratigraphy of the Maltese Islands. Ph.D. Dissertation, University of Hull, Hull, UK, 1975. [Google Scholar]
- Pedley, H.M.; Bennett, S.M. Phosphorites hardgrounds and syndepositional solution subsidence: A palaeoenvironmental model from the Miocene of the Maltese Islands. Sediment. Geol. 1985, 45, 1–34. [Google Scholar] [CrossRef]
- Baldassini, N.; Di Stefano, A. New insights on the Oligo-Miocene succession bearing phosphatic layers of the Maltese Archipelago. Ital. J. Geosci. 2015, 134, 355–366. [Google Scholar] [CrossRef]
- Pedley, H.M.; House, M.R.; Waugh, B. The geology of Malta and Gozo. Proc. Geol. Assoc. 1976, 87, 325–341. [Google Scholar] [CrossRef]
- Pedley, H.M.; House, M.R.; Waugh, B. The Geology of the Pelgian Block: The Maltese islands. In The Ocean Basins and Margins: Of the Western Mediterranean; Nairn, A.E.M., Greenough Stehli, F., Kanes, W.H., Eds.; Plenum Press: New York, NY, USA, 1978; Volume 4, pp. 417–433. [Google Scholar]
- Rose, E.P.F.; Pratt, S.K.; Bennett, S.M. Evidence for sea-level changes in the Globigerina Limestone Formation (Miocene) of the Maltese Islands. Paleontol. I Evol. 1992, 24, 265–278. [Google Scholar]
- Rehfeld, U.; Janssen, A.W. Development of phosphatized hardgrounds in the Miocene Globigerina Limestone of the Maltese Archipelago, including a description of Gamopleura melitensis sp. nov.(Gastropoda, Euthecosomata). Facies 1995, 33, 91–106. [Google Scholar] [CrossRef]
- Baldassini, N.; Di Stefano, A. Stratigraphic features of the Maltese Archipelago: A synthesis. Nat. Hazards 2017, 86, 203–231. [Google Scholar] [CrossRef]
- Open Topography. Available online: https://openotopgraphy.org (accessed on 22 June 2024).
- Yuan, R.; Kennedy, D.M.; Stephenson, W.J.; Finlayson, B.L. The precision and accuracy of measuring micro-scale erosion on shore platforms. Mar. Geol. 2022, 443, 106691. [Google Scholar] [CrossRef]
- Kirk, R.M. Rates and forms of erosion on intertidal platforms at Kaikoura Peninsula South Island New Zealand. N. Z. J. Geol. Geophys. 1977, 20, 571–613. [Google Scholar] [CrossRef]
- Robinson, D.A. Erosive processes on the shore platform of northeast Yorkshire England. Mar. Geol. 1977, 23, 339–361. [Google Scholar] [CrossRef]
- Gill, E.D.; Lang, J.G. Micro-erosion meter measurements of rock wear on the Otway coast of southeast Australia. Mar. Geol. 1983, 52, 141–156. [Google Scholar] [CrossRef]
- Porter, N.J.; Trenhaile, A.S.; Prestanski, K.J.; Kanyaya, J.I. Shore platform downwearing in eastern Canada: Micro-tidal Gaspé Québec. Geomorphology 2010, 116, 77–86. [Google Scholar] [CrossRef]
- Porter, N.J.; Trenhaile, A.S.; Prestanski, K.J.; Kanyaya, J.I. Patterns of surface downwearing on shore platforms in eastern Canada. Earth Surf. Process. Landf. 2010, 35, 1793–1810. [Google Scholar] [CrossRef]
- Gauci, R.; Inkpen, R.; Soar, P.J. Spatial analysis of eroding surface micro-topographies. Mar. Geol. 2022, 452, 106880. [Google Scholar] [CrossRef]
- Vopson, M.; Lepadatu, S. Second law of information dynamics. AIP Adv. 2022, 12, 075310. [Google Scholar] [CrossRef]
- Sakiam, R.M. The Box-Cox Transformation technique: A review. J. R. Stat. Soc. Ser. D Stat. 1992, 41, 169–178. [Google Scholar]
- Beasley, T.M.; Erickson, S.; Allison, D.B. Rank-based inverse normal transformation are increasingly used, but are they merited? Behav. Genet. 2009, 39, 580–595. [Google Scholar] [CrossRef]
- Velez, J.I.; Correa, J.C.; Marmolejo-Ramos, F. A new approach to Box-Cox transformation. Front. Appl. Math. Sci. 2015, 1, 12. [Google Scholar] [CrossRef]
- Malik, F.; Khan, A.W.; Shah, M.T.A. Box-Cox transformation for data normalization: A study of new product development in manufacturing sector of Pakistan. IBT J. Bus. Stud. 2018, 14, 110–119. [Google Scholar] [CrossRef]
- McCaw, Z.R.; Lane, J.M.; Saxena, R.; Redline, S.; Lin, X. Operating characteristics of the rank-based inverse normal transformation for quantitative trait analysis in genome-wide association studies. Biometrics 2020, 76, 1262–1272. [Google Scholar] [CrossRef]
- Yuan, R.; Kennedy, D.M.; Stephenson, W.J. Hourly to daily-scale microtopographic fluctuations of supratidal sandstone. Earth Surf. Process. Landf. 2018, 43, 3142–3151. [Google Scholar] [CrossRef]
- Yuan, R.; Kennedy, D.M.; Stephenson, W.J.; Gómez-Pujol, L. Experimental investigations into the influence of biofilms and environmental factors on short-term microtopographic fluctuations of supratidal sandstone. Earth Surf. Process. Landf. 2019, 44, 1377–1389. [Google Scholar] [CrossRef]
- Mayaud, J.R.; Viles, H.A.; Coombes, M.A. Exploring the influence of biofilm on short-term expansion and contraction of supratidal rock: An example from the Mediterranean. Earth Surf. Process. Landf. 2014, 39, 1404–1412. [Google Scholar] [CrossRef]
- Gauci, R. The Identification and Quantification of Surface Change on Limestone Shore Platforms of the Maltese Islands. Ph.D. Thesis, University of Portsmouth, Portsmouth, UK, 2018. [Google Scholar]
- Stephenson, W.J.; Finlayson, B.L. Measuring erosion with the micro-erosion meter-Contributions to understanding landform evolution. Earth Sci. Rev. 2009, 95, 53–62. [Google Scholar] [CrossRef]
- Stephenson, W.J.; Kirk, R.M. Surface swelling of coastal bedrock on inter-tidal shore platforms Kaikoura Peninsula South Island New Zealand. Geomorphology 2001, 41, 5–21. [Google Scholar] [CrossRef]
- Kanyaya, J.I.; Trenhaile, A.S. Tidal wetting and drying on shore platforms: An experimental assessment. Geomorphology 2005, 70, 129–146. [Google Scholar] [CrossRef]
- Yuan, R.; Kennedy, D.M.; Stephenson, W.J.; Finlayson, B.L. The influence of weathering processes on microtopographic changes of sandstone under simulated upper intertidal conditions from hourly to weekly scales. Geomorphology 2024, 446, 108999. [Google Scholar] [CrossRef]
- Yuan, R.; Kennedy, D.M.; Stephenson, W.J.; Finlayson, B.L. The influence of weathering processes on microtopographic changes of sandstone under simulated upper intertidal conditions from weekly to monthly scales. Geomorphology 2024, 455, 109209. [Google Scholar] [CrossRef]
- Robinson, D.A.; Jerwood, L.C. Frost and salt weathering of chalk shore platforms near Brighton Sussex UK. Trans. Inst. Br. Geogr. 1987, 12, 217–226. [Google Scholar] [CrossRef]
- Robinson, D.A.; Jerwood, L.C. Sub-aerial weathering of chalk shore platforms during harsh winters in Southeast England. Mar. Geol. 1987, 77, 1–14. [Google Scholar] [CrossRef]
- Gowell, M.R.; Coombes, M.A.; Viles, H.A. Rock-protecting seaweed? Experimental evidence of bioprotection in the intertidal zone. Earth Surf. Process. Landf. 2015, 40, 1364–1370. [Google Scholar] [CrossRef]
- Gomez-Pujol, L.; Fornós, J.J. Long-term vs. short-term subsite erosion rates on microtidal shore platforms (Southern Mallorca Balearic Islands Western Mediterranean). Environ. Earth Sci. 2023, 82, 570. [Google Scholar] [CrossRef]





| Date | Measurement Period |
|---|---|
| 13 August 2014–24 November 2014 | 1 |
| 24/11/14–19/3/15 | 2 |
| 19/3/15–12/6/15 | 3 |
| 12/6/15–4/9/15 | 4 |
| 4/9/15–26/12/15 | 5 |
| 26/12/15–29/3/16 | 6 |
| 29/3/16–29/6/16 | 7 |
| Measurement Period | Bolt Site | |||||
|---|---|---|---|---|---|---|
| (a) | ||||||
| 1 | 2 | 3 | 4 | 5 | 6 | |
| 1 | −0.36 | −0.12 | −0.19 | −0.25 | −0.40 | −0.37 |
| 2 | −0.18 | −0.04 | 0.06 | −0.76 | −0.13 | −0.17 |
| 3 | 0.05 | −0.16 | −0.07 | −0.03 | −0.09 | −0.07 |
| 4 | −0.04 | −0.14 | −0.01 | −0.01 | 0.07 | −0.01 |
| 5 | 0.74 | 0.18 | 0.95 | 0.83 | 0.92 | 0.90 |
| 6 | −0.11 | −0.25 | −0.13 | −0.03 | −0.12 | −0.13 |
| 7 | −1.02 | −1.42 | −1.11 | −1.14 | −1.18 | −1.11 |
| (b) | ||||||
| 1 | −0.17 | −0.07 | −0.21 | −0.25 | −0.12 | |
| 2 | 0.03 | −0.08 | −0.12 | −0.04 | −0.08 | |
| 3 | −0.07 | −0.03 | −0.13 | −0.09 | −0.13 | |
| 4 | −0.15 | −0.01 | −0.01 | −0.17 | −0.15 | |
| 5 | 0.11 | 0.20 | −0.07 | 0.07 | 0.12 | |
| 6 | −0.21 | −0.19 | −0.24 | −0.27 | −0.24 | |
| 7 | −0.12 | −0.02 | −0.11 | −0.14 | −0.01 | |
| Measurement Period | Bolt Sites | |||||
|---|---|---|---|---|---|---|
| (a) | ||||||
| 1 | 2 | 3 | 4 | 5 | 6 | |
| 1 | 2.15 | 1.49 | 3.01 | 1.39 | 2.14 | 2.38 |
| 2 | 1.44 | 1.61 | 2.31 | 2.26 | 1.28 | 1.77 |
| 3 | 2.00 | 1.90 | 1.17 | 1.63 | 1.76 | 1.60 |
| 4 | 1.65 | 2.36 | 1.83 | 1.89 | 1.99 | 1.91 |
| 5 | 4.18 | 3.45 | 3.56 | 4.22 | 4.26 | 3.90 |
| 6 | 1.51 | 2.10 | 1.56 | 1.52 | 1.54 | 1.50 |
| 7 | 3.62 | 4.15 | 3.67 | 4.15 | 4.21 | 3.99 |
| (b) | ||||||
| 1 | 2.22 | 1.98 | 2.68 | 2.80 | 2.36 | |
| 2 | 2.30 | 2.71 | 1.75 | 2.28 | 1.77 | |
| 3 | 1.94 | 1.84 | 1.92 | 1.80 | 1.76 | |
| 4 | 2.34 | 1.64 | 2.63 | 2.31 | 2.34 | |
| 5 | 3.62 | 4.00 | 3.36 | 3.49 | 3.67 | |
| 6 | 2.60 | 3.42 | 2.52 | 2.24 | 2.98 | |
| 7 | 2.14 | 1.49 | 2.23 | 2.16 | 2.19 | |
| Bolt Site | Data | F Value * | Significant Behaviour |
|---|---|---|---|
| MPQ1 | Erosion Rates | 150.76 | Measurement period 5 rises or low losses |
| Information Content | 34.34 | Measurement periods 5 and 7 high values | |
| MPQ2 | Erosion Rates | 54.23 | Measurement period 7 high losses |
| Information Content | 20.91 | Measurement periods 5 and 7 high values | |
| MPQ3 | Erosion Rates | 24.37 | Measurement period 5 rises or low losses, measurement period 7 high losses |
| Information Content | 20.40 | Measurement periods 5 and 7 high values | |
| MPQ4 | Erosion Rates | 159.05 | Measurement period 5 rises or low losses, measurement period 7 high losses |
| Information Content | 56.28 | Measurement periods 5 and 7 high values | |
| MPQ5 | Erosion Rates | 181.61 | Measurement period 5 rises, or low losses measurement period 7 high losses |
| Information Content | 62.42 | Measurement periods 5 and 7 high values | |
| MPQ6 | Erosion Rates | 112.63 | Measurement period 5 rises or low losses, measurement period 7 high losses |
| Information Content | 27.40 | Measurement periods 5 and 7 high values |
| Bolt Site | Data | F Value * | Significant Behaviour |
|---|---|---|---|
| MBB1 | Erosion Rates | 13.32 | Measurement periods 2 and 5 rises or low losses |
| Information Content | 3.83 | Measurement period 5 high values | |
| MBB2 | Erosion Rates | 10.08 | Measurement period 5 rises or low losses |
| Information Content | 18.55 | Measurement periods 5 and 6 high values | |
| MBB3 | Erosion Rates | 6.71 | Measurement period 4 rises or low losses, measurement period 6 high losses |
| Information Content | 3.81 | Measurement period 5 high values | |
| MBB4 | Erosion Rates | 5.44 | Measurement period 5 rises or low losses |
| Information Content | 3.97 | Measurement period 5 high values | |
| MBB5 | Erosion Rates | 18.39 | Measurement period 5 rises or low losses, measurement period 6 high losses |
| Information Content | 6.68 | Measurement period 5 high values |
| Measurement Period | Data | F Value * | Significant Behaviour |
|---|---|---|---|
| 1 | Erosion Rate | 1.24 | |
| Information Content | 12.85 | MPQ2 high values | |
| 2 | Erosion Rate | 8.13 | MPQ4 high losses |
| Information Content | 4.06 | MPQ3 and MPQ4 high values | |
| 3 | Erosion Rate | 0.85 | |
| Information Content | 3.97 | MPQ3 low values | |
| 4 | Erosion Rate | 4.64 | MPQ2 rises or low losses |
| Information Content | 1.78 | ||
| 5 | Erosion Rate | 34.26 | MPQ2 low losses |
| Information Content | 1.81 | ||
| 6 | Erosion Rate | 2.41 | MPQ2 high losses |
| Information Content | 4.09 | MPQ2 high values | |
| 7 | Erosion Rate | 2.41 | MPQ2 high losses |
| Information Content | 0.66 |
| Measurement Period | Data | F Value | Significant Behaviour |
|---|---|---|---|
| 1 | Erosion Rate | 2.97 | MPQ2 rises or low losses |
| Information Content | 1.48 | ||
| 2 | Erosion Rate | 1.83 | |
| Information Content | 3.09 | MPQ2 high values | |
| 3 | Erosion Rate | 1.70 | |
| Information Content | 0.12 | ||
| 4 | Erosion Rate | 5.20 | MPQ2 and MPQ3 rises or low losses |
| Information Content | 1.57 | ||
| 5 | Erosion Rate | 7.16 | MPQ2 rises or low losses, MPQ3 high losses |
| Information Content | 0.55 | ||
| 6 | Erosion Rate | 0.97 | |
| Information Content | 3.07 | MPQ2 high values | |
| 7 | Erosion Rate | 3.19 | MPQ2 and MPQ5 rises or low losses |
| Information Content | 1.96 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gauci, R.; Inkpen, R. The Application of Information Theory to Interpret Shore Platform Erosion Rates. Geosciences 2024, 14, 290. https://doi.org/10.3390/geosciences14110290
Gauci R, Inkpen R. The Application of Information Theory to Interpret Shore Platform Erosion Rates. Geosciences. 2024; 14(11):290. https://doi.org/10.3390/geosciences14110290
Chicago/Turabian StyleGauci, Ritienne, and Rob Inkpen. 2024. "The Application of Information Theory to Interpret Shore Platform Erosion Rates" Geosciences 14, no. 11: 290. https://doi.org/10.3390/geosciences14110290
APA StyleGauci, R., & Inkpen, R. (2024). The Application of Information Theory to Interpret Shore Platform Erosion Rates. Geosciences, 14(11), 290. https://doi.org/10.3390/geosciences14110290






