Ambient Vibrations Measurements and 1D Site Response Modelling as a Tool for Soil and Building Properties Investigation
Abstract
:1. Introduction
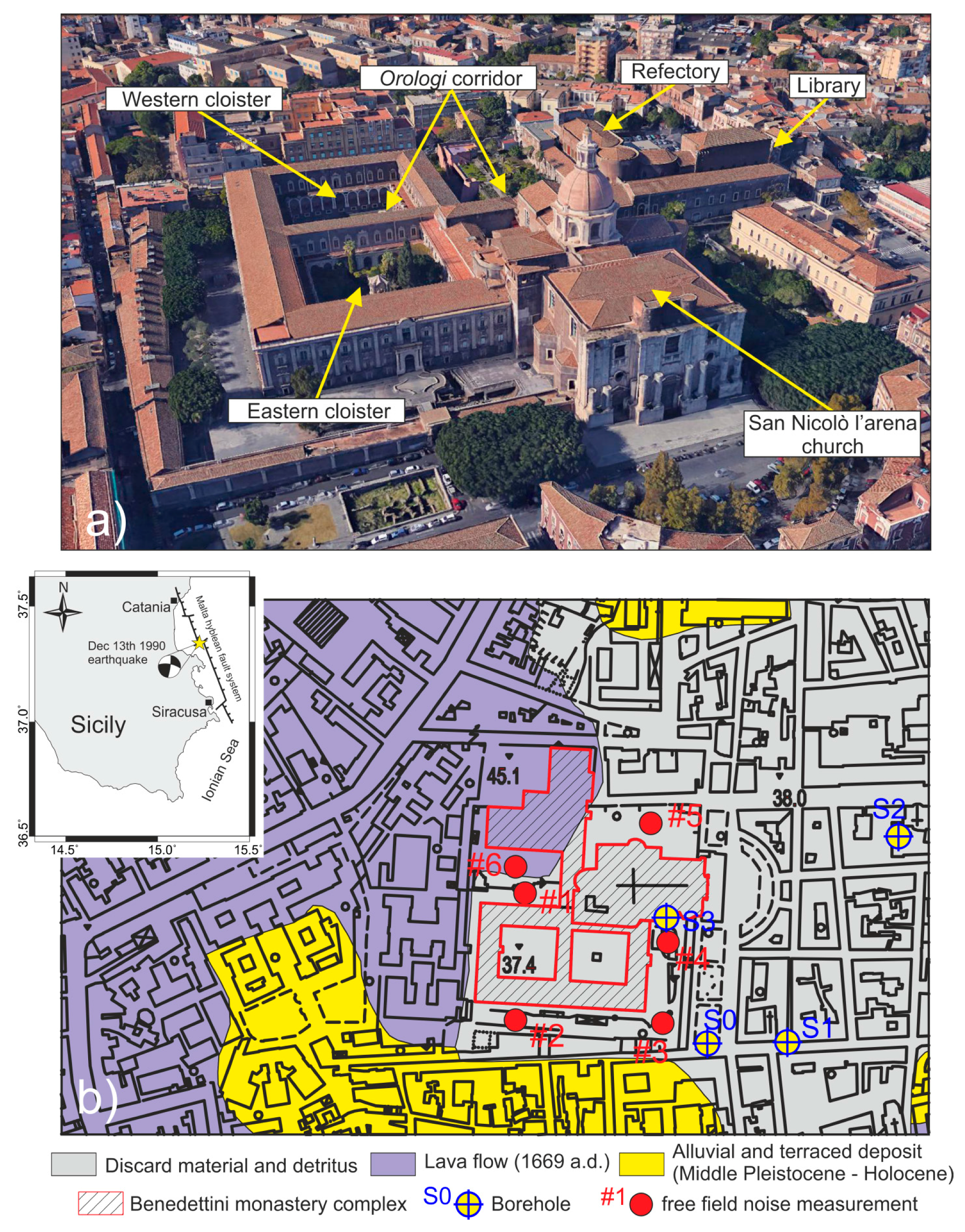
2. Benedettini Complex History
3. Geologic Setting
4. Experimental and Numerical Data Processing
4.1. Ambient Vibration Measurements
4.2. Modelling 1D Amplification Function
5. Concluding Remarks
- The HHSR results point out several oscillation modes (see Figure 4, Figure 5, Figure 6 and Figure 7) in the frequency range 2.0–10.0 Hz, that we correlate to the structural complexity of the Monastery. However, in some places, where the influence of the irregularities is lower, the presence of dominant fundamental frequencies was easily detected.
- The RDM was used to quantify the buildings damping. In particular, this method was applied only on sites showing a well detectable first mode of vibration. This means that for more specific damping estimates, operational mode analysis and/or finite element method could help in determining more accurate values for the investigated structure. However, although the performed analysis is quite simple, it allowed us to set into evidence an extreme variability of the building damping values.
- The soil fundamental frequencies, obtained from measurements performed outside the Monastery (Figure 8) range in the interval 3.0–6.0 Hz, highlighting an important role of the stratigraphic sequence on the site effects. Moreover, we observed that HHSRs and HVSRs share in many case the same frequency band, therefore pointing out possible site-to-building resonance effects.
- The spectral accelerations and amplification functions coming out from numerical modelling obtained by using the code STRATA, are consistent with the HVSR results and the estimated macroseismic intensity is tightly linked with the damage observed in the Monastery during the 1990 earthquake ground shaking.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Barilaro, D.; Majolino, D.; Gresta, S.; Imposa, S.; Schillaci, A.; Leone, A. Geophysics and thermographic investigations addressed to the study of the church of “S. Maria del Rosario” in Sicily (Italy). Contrib. Geophys. Geodesy 2006, 36, 239–253. [Google Scholar]
- Barilaro, D.; Branca, C.; Gresta, S.; Imposa, S.; Leone, A.; Majolino, D. Ground Penetrating radar (G.P.R.) surveys applied to the research of crypts in San Sebastiano’s church in Catania (Sicily-Italy). J. Cult. Herit. 2007, 8, 73–76. [Google Scholar] [CrossRef]
- Imposa, S. Infrared thermography and Georadar techniques applied to the “Sala delle Nicchie” (Niches Hall) of Palazzo Pitti, Florence (Italy). J. Cult. Herit. 2010, 11, 259–264. [Google Scholar] [CrossRef]
- Imposa, S.; Mele, G.; Coco, G.; Corrao, M.; Battaglia, G. Characterization of decay in the wooden roof of the S. Agata church of Ragusa Ibla (southern Sicily) by means of sonic tomography and resistograph penetration tests. Int. J. Archit. Herit. 2014, 8, 213–223. [Google Scholar] [CrossRef] [Green Version]
- Imposa, S.; Grassi, S. Georadar survey inside the Santa Maria Maggiore Church of Ispica (Sicily-Italy). Environ. Earth Sci. 2015, 73, 1939–1949. [Google Scholar] [CrossRef]
- Bard, P.-Y.; Bouchon, M. The two-dimensional resonance of sediment-filled valleys. Bull. Seismol. Soc. Am. 1985, 75, 519–541. [Google Scholar]
- Faccioli, E. Seismic amplification in the presence of geological and topographic irregularities. In Proceedings of the 2nd International Conference on Recent Advances in Geotechnical Earthquake Engineering and Soil Dynamics, St. Louis, MO, USA, 11–15 March 1991; pp. 1779–1797. [Google Scholar]
- Naganoh, M.; Kagami, H.; Muratami, H. Effects of surface and subsurface irregularities. In Earthquake Motions and Ground Conditions; The Architectural Institute: Tokyo, Japan, 1993; Section 3.3. [Google Scholar]
- Borcherdt, R.D. Effects of local geology on ground motion near San Francisco Bay. Bull. Seismol. Soc. Am. 1970, 60, 29–61. [Google Scholar]
- Nakamura, Y. A Method for Dynamic Characteristics Estimation of Sub Surface Using Microtremor on the Ground Surface; Railway Technical Research Institute: Tokyo, Japan, 1989; Volume 30, pp. 25–33. [Google Scholar]
- Lermo, J.; Chavez-Garcia, F.J. Site effect evaluation using spectral ratios with only one station. Bull. Seismol. Soc. Am. 1993, 83, 1574–1594. [Google Scholar]
- Seekins, L.C.; Wennerberg, L.; Marghereti, L.; Liu, H.P. Site amplification at five locations in San Fransisco, California: A comparison of S waves, codas, and microtremors. Bull. Seismol. Soc. Am. 1996, 86, 627–635. [Google Scholar]
- Haghshenas, E.; Bard, P.-Y.; Theodulidis, N.; SESAME WP04 Team. Empirical evaluation of microtremor H/V spectral ratio. Bull. Earthq. Eng. 2008, 6, 75–108. [Google Scholar] [CrossRef]
- Panzera, F.; Lombardo, G. Seismic property characterization of lithotypes cropping out in the Siracusa urban area, Italy. Eng. Geol. 2013, 153, 12–24. [Google Scholar] [CrossRef]
- Imposa, S.; Coco, G.; Corrao, M. Site effects close to structural lineaments in eastern Sicily (Italy). Eng. Geol. 2004, 72, 331–341. [Google Scholar] [CrossRef]
- Imposa, S.; Grassi, S.; De Guidi, G.; Battaglia, F.; Lanaia, G.; Scudero, S. 3D subsoil model of the San Biagio ‘Salinelle’ mud volcanoes (Belpasso, SICILY) derived from geophysical surveys. Surv. Geophys. 2016, 37, 1117–1138. [Google Scholar] [CrossRef]
- Imposa, S.; Grassi, S.; Fazio, F.; Rannisi, G.; Cino, P. Geophysical surveys to study a landslide body (north-eastern Sicily). Nat. Hazards 2017, 86, S327–S343. [Google Scholar] [CrossRef]
- Panzera, F.; Lombardo, G.; Rigano, R. Evidence of topographic effects through the analysis of ambient noise measurements. Seismol. Res. Lett. 2011, 82, 413–419. [Google Scholar] [CrossRef]
- Panzera, F.; Lombardo, G.; Monaco, C. New evidence of wavefield polarization on fault zone in the lower NE slope of Mt. Etna. Ital. J. Geosci. 2016, 135, 250–260. [Google Scholar] [CrossRef]
- Burjànek, J.; Moore, J.R.; Molina, F.X.Y.; Fäh, D. Instrumental evidence of normal mode rock slope vibration. Geophys. J. Int. 2012, 188, 559–569. [Google Scholar] [CrossRef]
- Imposa, S.; Barone, F.; Chiavetta, F.; Coco, G.; Corrao, M.; Chisari, D. A preliminary study of the subsoil of the Roman Amphitheatre of Catania (Sicily) through integrated geophysical and stratigraphic data. Il Nuovo Cimento C. 2007, 30, 577–586. [Google Scholar]
- Imposa, S.; Motta, E.; Capilleri, P.; Imposa, G. HVSR and MASW seismic survey for characterizing the local seismic response: A case study in Catania area (Italy). In Proceedings of the 1st IMEKO TC-4 International Workshop on Metrology for Geotechnics, Benevento, Italy, 17–18 March 2016; IMEKO-International Measurement Federation Secretariat: Budapest, Hungary, 2016; Volume 1, pp. 97–102. ISBN 978-929900750-1. [Google Scholar]
- Castellaro, S.; Imposa, S.; Barone, F.; Chiavetta, F.; Gresta, S.; Mulargia, F. Georadar and Passive Seismic Survey in the Roman Amphitheatre of Catania (Sicily). J. Cult. Herit. 2008, 9, 357–366. [Google Scholar] [CrossRef]
- Eurocode 8 Design of Structures for Earthquake Resistance—Part 1: General Rules, Seismic Actions and Rules for Buildings; European Committee for Standardization (CEN), EN 1998-1; CEN: Brussels, Belgium, 2004; Available online: www.eurocodes.jrc.eceuropa.eu/ (accessed on 6 November 2017).
- Trifunac, M.D. Comparison between ambient and forced vibration experiments. Earthq. Eng. Struct. Dyn. 1972, 1, 133–150. [Google Scholar] [CrossRef]
- Di Tommaso, R.; Parolai, S.; Mucciarelli, M.; Eggert, S.; Sobiesiak, M.; Zschau, J. Monitoring the response and the back-radiated energy of a building subjected to ambient vibration and impulsive action: The Falkenhof Tower (Potsdam, Germany). Bull. Earthq. Eng. 2010, 8, 705–722. [Google Scholar] [CrossRef]
- Michel, C.; Guéguen, P. Time–frequency analysis of small frequency variations in civil engineering structures under weak and strong motions using a reassignment method. Struct. Health Monit. 2010, 9, 159–171. [Google Scholar] [CrossRef] [Green Version]
- Todorovska, M.I. Soil-structure system identification of millikan library north–south response during four earthquakes (1970–2002): What caused the observed wandering of the system frequencies? Bull. Seismol. Soc. Am. 2009, 99, 626–635. [Google Scholar] [CrossRef]
- Gallipoli, M.R.; Mucciarelli, M.; Vona, M. Empirical estimate of fundamental frequencies and damping for Italian buildings. Earthq. Eng. Struct. Dyn. 2009, 38, 973–988. [Google Scholar] [CrossRef]
- Panzera, F.; D’amico, S.; Lombardo, G.; Longo, E. Evaluation of building fundamental periods and effects of local geology on ground motion parameters in the Siracusa area, Italy. J. Seismol. 2016, 20, 1001–1019. [Google Scholar] [CrossRef]
- Celebi, M. Comparison of damping in buildings under low-amplitude and strong motions. J. Wind Eng. Ind. Aerodyn. 1996, 59, 309–323. [Google Scholar] [CrossRef]
- Trifunac, M.D.; Todorovska, M.I.; Manić, M.I.; Bulajić, B.Đ. Variability of the fixed-base and soil-structure system frequencies of a building—The case of Borik-2 building. Struct. Control Health Monit. 2010, 17, 120–151. [Google Scholar] [CrossRef]
- Mucciarelli, M.; Gallipoli, M.R.; Ponzo, C.F.; Dolce, M. Seismic waves generated by oscillating building. Soil Dyn. Earthq. Eng. 2003, 23, 255–262. [Google Scholar] [CrossRef]
- Cornou, C.; Guéguen, P.; Bard, P.-Y.; Haghshenas, E. Ambient noise energy bursts observation and modeling: Trapping of harmonic structure-soil induced–waves in a topmost sedimentary layer. J. Seismol. 2004, 8, 507–524. [Google Scholar] [CrossRef]
- Gallipoli, M.R.; Mucciarelli, M.; Ponzo, F.; Dolce, M.; D’Alema, E.; Maistrello, M. Buildings as a Seismic Source: Analysis of a Release Test at Bagnoli, Italy. Bull. Seismol. Soc. Am. 2006, 96, 2457–2464. [Google Scholar] [CrossRef] [Green Version]
- Azzaro, R.; Barbano, M.S. Analysis of seismicity of Southeastern Sicily: Proposal of a tectonic interpretation. Ann. Geofis. 2000, 43, 1–18. [Google Scholar]
- Panzera, F.; Lombardo, G.; Rigano, R. Use of different approaches to estimate seismic hazard: The study cases of Catania and Siracusa, Italy. Bollettino di Geofisica Teorica e Applicata 2011, 52, 687–706. [Google Scholar]
- Locati, M.; Camassi, R.; Rovida, A.; Ercolani, E.; Bernardini, F.; Castelli, V.; Caracciolo, C.H.; Tertulliani, A.; Rossi, A.; Azzaro, R.; et al. DBMI15, the 2015 Version of the Italian Macroseismic Database; Istituto Nazionale di Geofisica e Vulcanologia: Rome, Italy, 2016. [Google Scholar] [CrossRef]
- Rovida, A.; Locati, M.; Camassi, R.; Lolli, B.; Gasperini, P. CPTI15, the 2015 Version of the Parametric Catalogue of Italian Earthquakes; Istituto Nazionale di Geofisica e Vulcanologia: Roma, Italy, 2016. [Google Scholar]
- Binda, L.; Saisi, A. Non Destructive Testing Applied to Historic Buildings: The Case of Some Sicilian Churches. Available online: http://www.csarmento.uminho.pt/docs/ncr/historical_constructions/page%2029-46%20_Binda_.pdf (accessed on 10 December 2017).
- Saisi, A.; Binda, L.; Zanzi, L. Diagnostic investigation of the stone pillars of S. Nicolò l’Arena, Catania. In Proceedings of the 9th Canadian Masonry Symposium “Spanning the Centuries with Masonry”, Fredericton, NB, Canada, 4–6 June 2001. [CD-ROM]. [Google Scholar]
- Monaco, C.; Catalano, S.; De Guidi, G.; Gresta, S.; Langer, H.; Tortorici, L. The geological map of the urban area of Catania (Eastern Sicily): Morphotectonic and seismotectonic implications. Mem. Soc. Geol. Ital. 2000, 55, 425–438. [Google Scholar]
- Lombardo, G.; Coco, G.; Corrao, M.; Imposa, S.; Azzara, R.; Cara, F.; Rovelli, A. Results of microtremor measurements in the urban area of Catania (Italy). Bollettino di Geofisica Teorica e Applicata 2001, 42, 317–334. [Google Scholar]
- Panzera, F.; Lombardo, G.; Monaco, C.; Di Stefano, A. Seismic site effects observed on sediments and basaltic lavas outcropping in a test site of Catania, Italy. Nat. Hazards 2015, 79, 1–27. [Google Scholar] [CrossRef]
- UniCT Seismic Microzonation Working Group. Microzonazione sismica (MS) di Livello 1. D.D.G. n. 219 del 08/07/2014. Available online: http://sit.protezionecivilesicilia.it/ (accessed on 6 November 2017).
- Grasso, S.; Massimino, M.R.; Maugeri, M. Evaluation of a design spectrum at Saint Nicola alla Rena Church in Catania (Italy). In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, BC, Canada, 1–6 August 2004; Paper No. 1432. 13WCEE: Vancouver, BC, Canada, 2004. [Google Scholar]
- Konno, K.; Ohmachi, T. Ground-motion characteristics estimated from spectral ratio between horizontal and vertical components of microtremor. Bull. Seismol. Soc. Am. 1998, 88, 228–241. [Google Scholar]
- Snieder, R.; Safak, E. Extracting the building response using seismic interferometry: Theory and application to the Millikan Library in Pasadena, California. Bull. Seismol. Soc. Am. 2006, 96, 586–598. [Google Scholar] [CrossRef]
- Castellaro, S.; Mulargia, F. How far from a building does the ground motion free field start? The cases of three famous towers and of a modern building. Bull. Seismol. Soc. Am. 2010, 100, 2080–2094. [Google Scholar] [CrossRef]
- SESAME Guidelines for the Implementation of the H/V Spectral Ratio Technique on Ambient Vibrations: Measurements, Processing and Interpretation. SESAME European Research Project WP12, Deliverable D23.12 2004. Available online: http://sesame.geopsy.org/SES_Reports.htm (accessed on 6 November 2017).
- Di Giacomo, D.; Gallipoli, M.R.; Mucciarelli, M.; Parolai, S.; Richwalski, S.M. Analysis and modeling of HVSR in the presence of a velocity inversion: The case of Venosa, Italy. Bull. Seismol. Soc. Am. 2005, 95, 2364–2372. [Google Scholar] [CrossRef]
- Castellaro, S.; Mulargia, F. The Effect of Velocity Inversions on H/V. Pure Appl. Geophys. 2009, 166, 567–592. [Google Scholar] [CrossRef]
- Panzera, F.; Lombardo, G.; Muzzetta, I. Evaluation of building dynamic properties through in-situ experimental techniques and 1D modelling: The example of Catania, Italy. Phys. Chem. Earth 2013, 63, 136–146. [Google Scholar] [CrossRef]
- Cole, H.A. On-Line Failure Detection and Damping Measurement of Aerospace Structures by Random Decrement Signatures; NASA CR-2205; NASA: Washington, DC, USA, 1973.
- Dunand, F.; Bard, P.-Y.; Chatelain, J.L.; Gueguen, P.; Vassail, T.; Farsi, M.N. Damping and frequency from randomec method applied to in-situ measurements of ambient vibrations: Evidence for effective soil structure interaction. In Proceedings of the 12th European Conference on Earthquake Engineering, London, UK, 9–13 September 2002; Paper No. 868; Elsevier Science Ltd.: Amsterdam, The Netherlands, 2002. [Google Scholar]
- Tamura, Y. Amplitude dependency of damping in buildings and critical tip drift ratio. Int. J. High-Rise Build. 2012, 1, 1–13. [Google Scholar]
- Kareem, A.; Gurley, K. Damping in structures: Its evaluation and treatment of uncertainty. J. Wind Eng. Ind. Aerodyn. 1996, 59, 131–157. [Google Scholar] [CrossRef]
- Salameh, C.; Guillier, B.; Harb, J.; Cornou, C.; Bard, P.-Y.; Voisin, C.; Mariscal, A. Seismic response of Beirut (Lebanon) buildings: Instrumental results from ambient vibrations. Bull. Earthq. Eng. 2016, 14, 2705–2730. [Google Scholar] [CrossRef]
- Mucciarelli, M. Reliability and applicability of Nakamura’s technique using microtremors: An experimental approach. J. Earthq. Eng. 1998, 2, 625–638. [Google Scholar] [CrossRef]
- Panzera, F.; Rigano, R.; Lombardo, G.; Cara, F.; Di Giulio, G.; Rovelli, A. The role of alternating outcrops of sediments and basaltic lavas on seismic urban scenario: The study case of Catania, Italy. Bull. Earthq. Eng. 2011, 9, 411–439. [Google Scholar] [CrossRef]
- Assimaki, D.; Kausel, E. An Equivalent Linear Algorithm with Frequency-and-Pressure-Dependent Moduli and Damping for Seismic Analysis of Deep Sites. Soil Dyn. Earthq. Eng. 2002, 22, 959–965. [Google Scholar] [CrossRef]
- Faccioli, E.; Pessina, V. The Catania Project: Earthquake Damage Scenarios for High Risk Area in the Mediterranean; CNR—Gruppo Nazionale per la Difesa Terremoti: Rome, Italy, 2000; p. 225. [Google Scholar]
- Carrubba, P.; Maugeri, M. Determinazione delle proprietà dinamiche di un’argilla mediante prove di colonna risonante. Rivista Italiana di Geotecnica 1988, 22, 101–113. [Google Scholar]
- Cavallaro, A.; Grasso, S.; Maugeri, M. A dynamic geothechnical characterization of soil at Saint Nicola alla Rena Church damaged by South Eastern Sicily Earthquake of 13 December 1990. In Proceedings of the 15th International Conference on Soil Mechanics and Geotechnical Engineering, Satellite Conference “Lessons Learned from Recent Strong Earthquake, Istanbul, Turkey, 25 August 2001; pp. 243–248. [Google Scholar]
- Seed, H.B.; Idriss, I.M. Soil Moduli and Damping Factors for Dynamic Response Analyses; Report No. EERC-70-10; University of California: Berkeley, CA, USA, 1970. [Google Scholar]
- Bessason, B.; Kaynia, A.M. Site amplification in lava rock on soft sediments. Soil Dyn. Earthq. Eng. 2002, 22, 525–540. [Google Scholar] [CrossRef]
- Cauzzi, C.; Faccioli, E.; Vanini, M.; Bianchini, A. Updated predictive equations for broadband (0.01–10 s) horizontal response spectra and peak ground motions, based on a global dataset of digital acceleration records. Bull. Earthq. Eng. 2015, 13, 1587–1612. [Google Scholar] [CrossRef]
- Luzi, L.; Puglia, R.; Russo, E.; ORFEUS WG5. Engineering Strong Motion Database, version 1.0; Istituto Nazionale di Geofisica e Vulcanologia, Observatories & Research Facilities for European Seismology: Rome, Italy, 2016. [Google Scholar] [CrossRef]
- Iervolino, I.; Galasso, C.; Cosenza, E. REXEL: Computer aided record selection for code-based seismic structural analysis. Bull. Earthq. Eng. 2010, 8, 339–362. [Google Scholar] [CrossRef]
- Locati, M.; Gomez Capera, A.; Puglia, R.; Santulin, M. Rosetta, a tool for linking accelerometric recordings and macroseismic observations: Description and applications. Bull. Earthq. Eng. 2017, 15, 2429–2443. [Google Scholar] [CrossRef]
- Valente, G.; Zigone, G. Methodology and techniques for seismic protection of the monumental patrimony. In Seismic Prevention of Damage a Case Study in Mediterranean City; Maugeri, M., Ed.; WIT Press: Southampton, UK, 2005; pp. 313–332. [Google Scholar]










© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Imposa, S.; Lombardo, G.; Panzera, F.; Grassi, S. Ambient Vibrations Measurements and 1D Site Response Modelling as a Tool for Soil and Building Properties Investigation. Geosciences 2018, 8, 87. https://doi.org/10.3390/geosciences8030087
Imposa S, Lombardo G, Panzera F, Grassi S. Ambient Vibrations Measurements and 1D Site Response Modelling as a Tool for Soil and Building Properties Investigation. Geosciences. 2018; 8(3):87. https://doi.org/10.3390/geosciences8030087
Chicago/Turabian StyleImposa, Sebastiano, Giuseppe Lombardo, Francesco Panzera, and Sabrina Grassi. 2018. "Ambient Vibrations Measurements and 1D Site Response Modelling as a Tool for Soil and Building Properties Investigation" Geosciences 8, no. 3: 87. https://doi.org/10.3390/geosciences8030087
APA StyleImposa, S., Lombardo, G., Panzera, F., & Grassi, S. (2018). Ambient Vibrations Measurements and 1D Site Response Modelling as a Tool for Soil and Building Properties Investigation. Geosciences, 8(3), 87. https://doi.org/10.3390/geosciences8030087






