The Influence of Magmatic Intrusions on Diagenetic Processes and Stress Accumulation
Abstract
1. Introduction
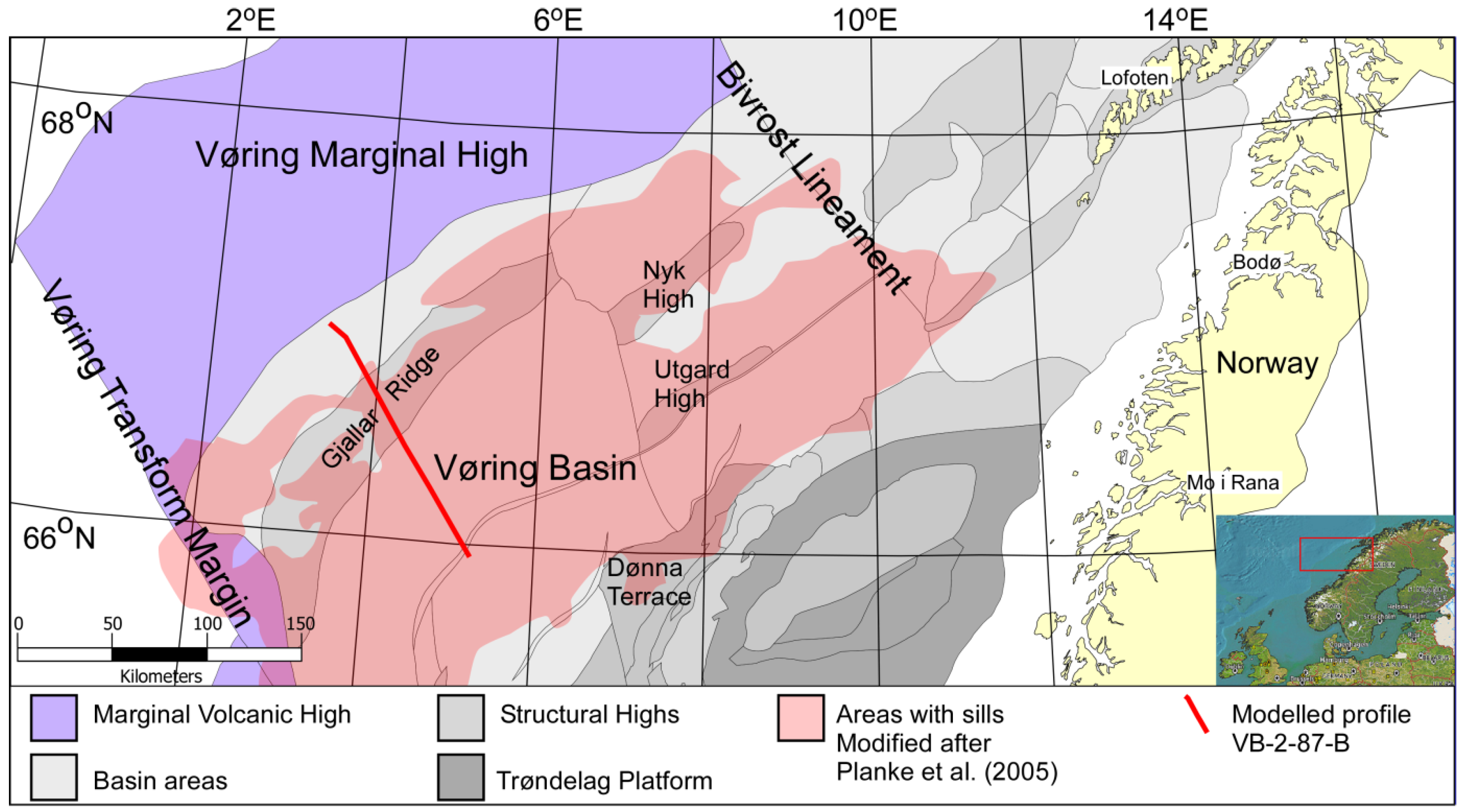
2. An Example of the Evolution of a Volcanic Basin—The Vøring Basin
3. Methods and Results for Magmatic Intrusion’s Influence on Diagenetic Processes
3.1. Geohistory, Temperature, Maturation, and Diagenesis
3.1.1. Silica Diagenesis—Quartz Diagenesis
3.1.2. Silica Diagenesis—Opal A to Quartz Via Opal CT
3.1.3. Clay Diagenesis—Smectite to Illite
3.2. Opal A to Opal CT—Testing of the Method
3.3. Example from the Vøring Basin, Section VB-2-87-B
3.3.1. Quartz Cement
3.3.2. Opal A to Opal CT to Quartz
3.3.3. Smectite to Illite
4. Methods and Results for Stress Accumulation Influenced by Diagenetic Processes and Sills Themselves
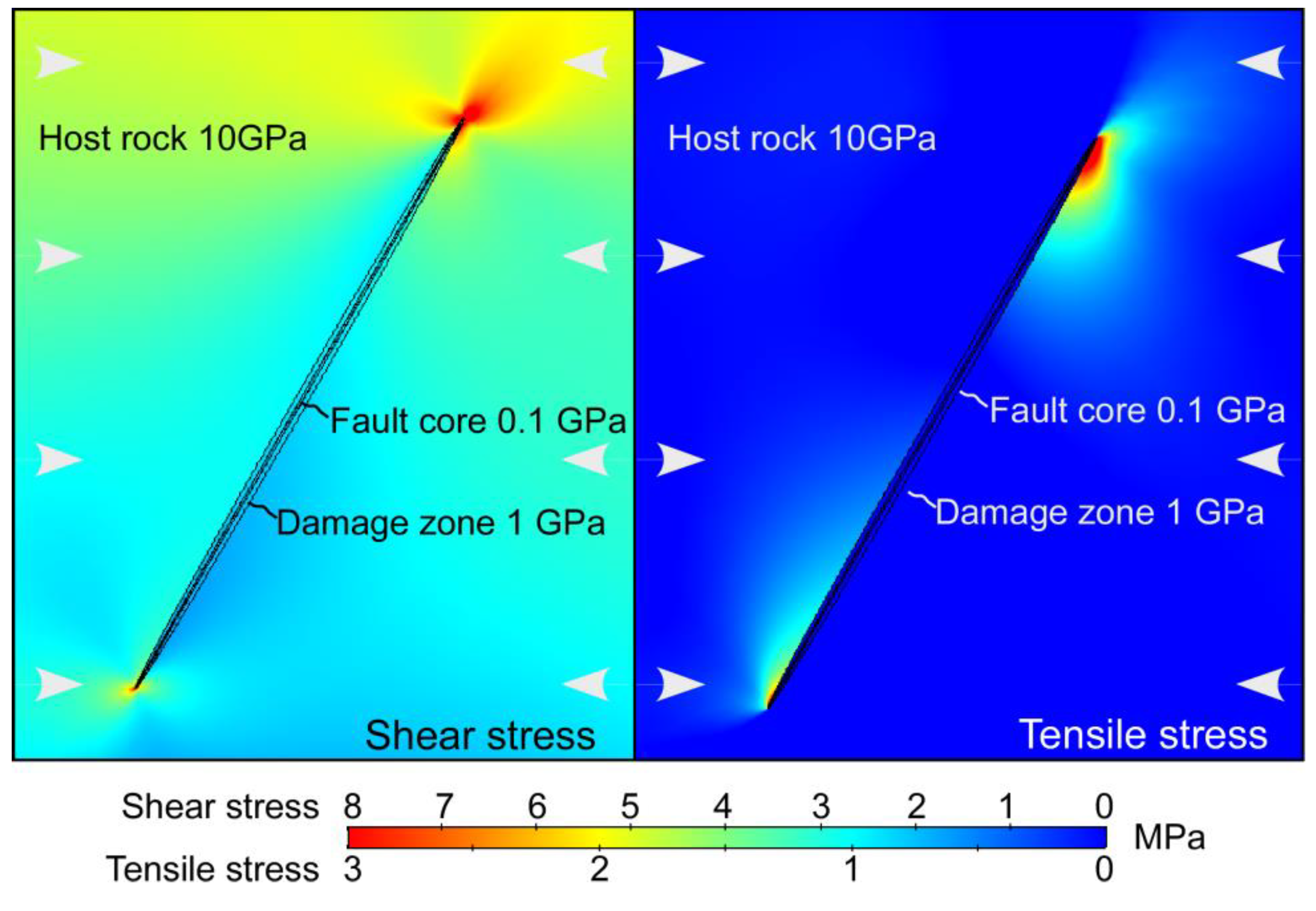
4.1. Stress Modeling
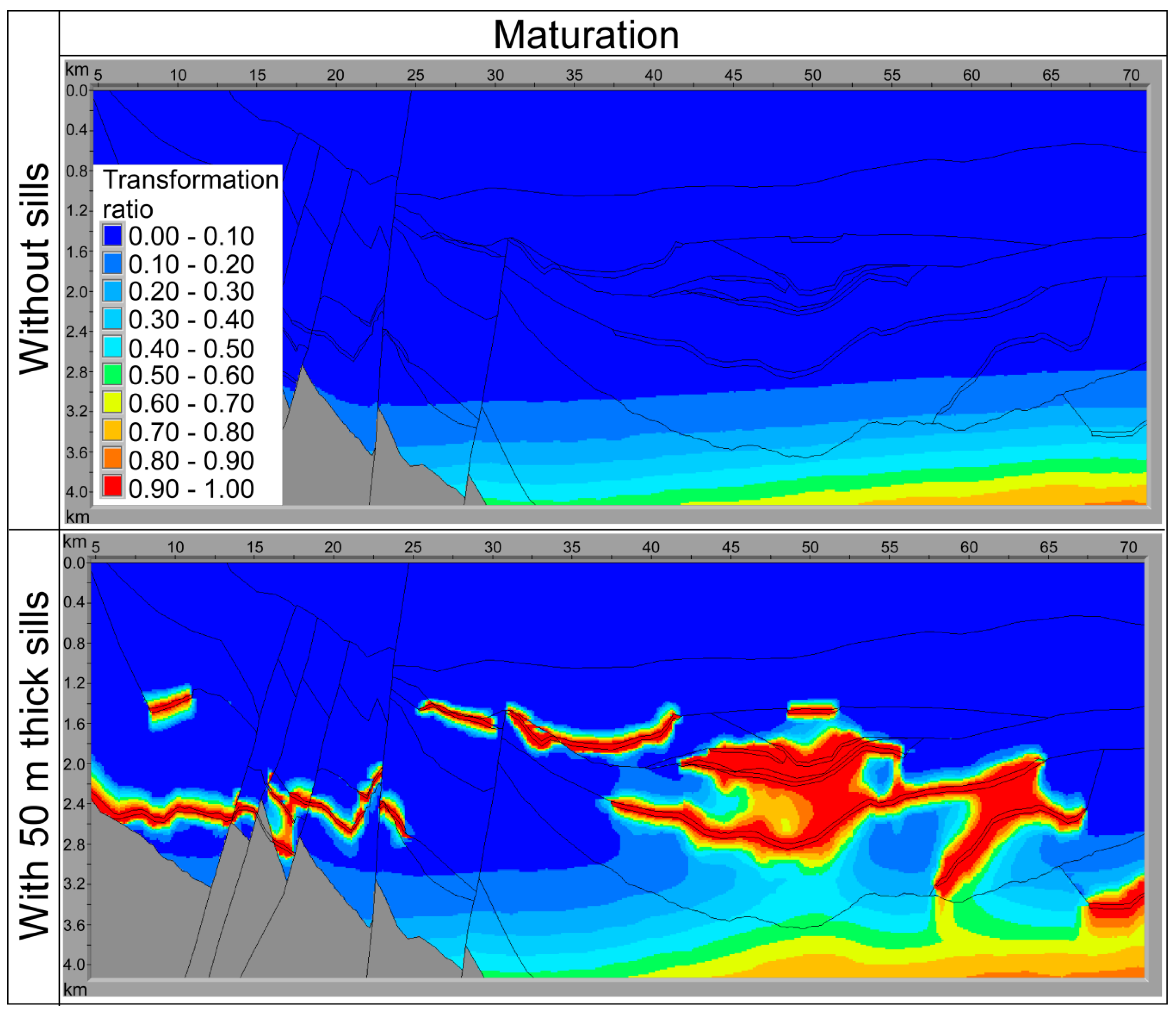
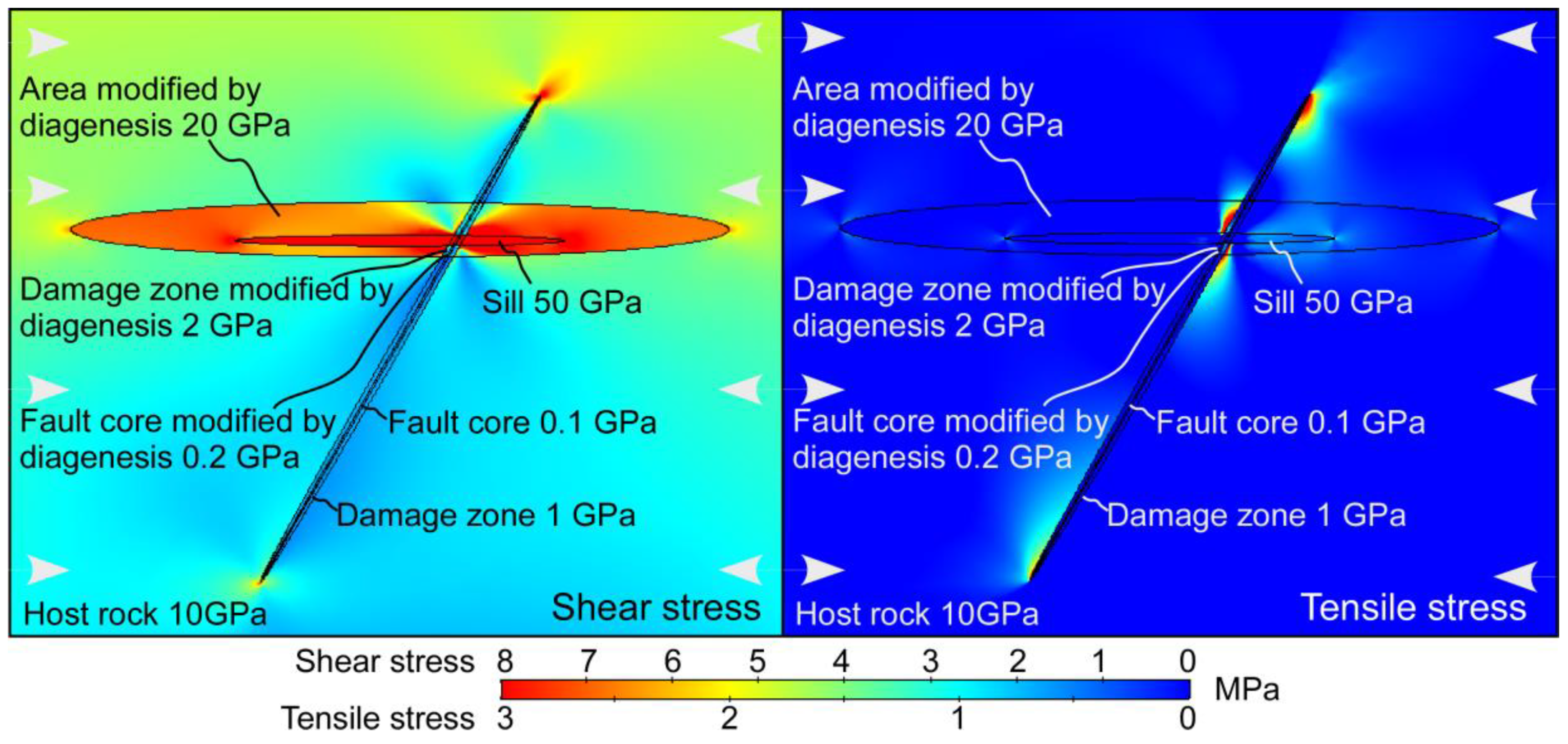
4.2. Stress Effects of Sills and Diagenesis
Synthetic Case
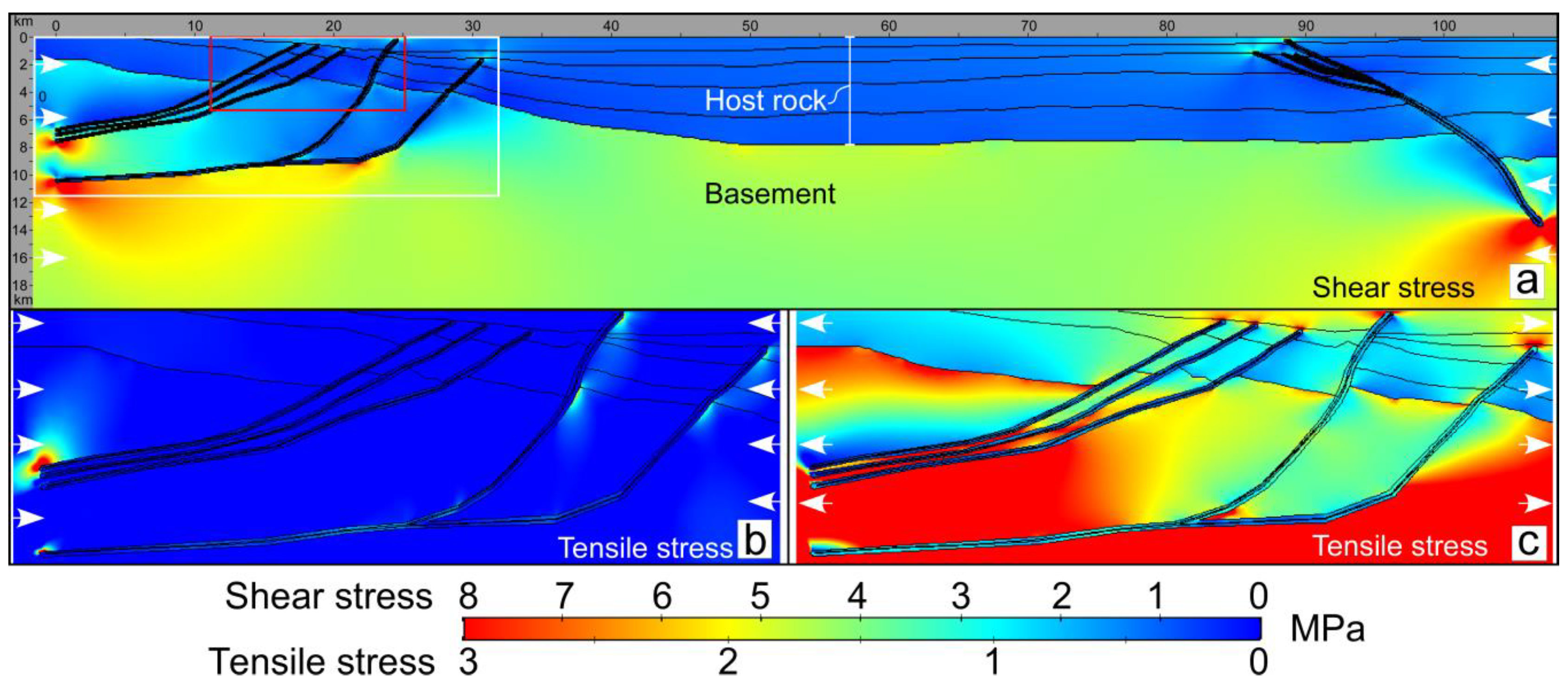
4.3. Example from the Vøring Area
5. Discussion
5.1. Effects of Diagenesis on Petroleum Systems
5.2. Effect of Magmatic Sills on the Diagenetic Process in Reservoir Rocks
5.3. Silica Diagenesis
Opal CT Boundary in the Vøring Basin
5.4. Smectite to Illite
5.5. Influence of Sills and Diagenesis on Stress Accumulations
5.6. Limitations of the Calculations
6. Concluding Remarks
- Conductive thermal effects of sills significantly influence the diagenetic processes in sedimentary basins.
- The effect of magmatic intrusions on the diagenetic processes depends on the depth at which the magmatic sills intrude. Maximum diagenetic changes occur at different temperatures for the different processes. For sills to influence the transition of opal A to opal CT they must intrude at depths >1500 m.
- Sill thickness influences the size of the diagenetically altered area, particularly where clusters of sills are closely spaced.
- Sills and diagenetically modified areas influence location of stress accumulations and may contribute to initiation of fault movement and opening of fractures. As fractures and faults can act as conduits for fluid flow, sills and areas modified by diagenesis may therefore contribute to increased permeability.
- A thorough case study is required to determine the sill’s specific effect on diagenetic processes and stress accumulations in the Vøring Basin and other volcanic basins. This is now made possible with the work done in this study.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Schutter, S.R. Hydrocarbon occurrence and exploration in and around igneous rocks. In Hydrocarbons in Crystalline Rocks, Special Publications; Petford, N., McCaffrey, K.J.W., Eds.; Geological Society: London, UK, 2003; Volume 214, pp. 7–33. [Google Scholar]
- Svensen, H.; Planke, S.; Malthe-Sørenssen, A.; Jamtveit, B.; Myklebust, R.; Eidem, T.R.; Rey, S.S. Release of methane from a volcanic basin as a mechanism for initial Eocene global warming. Nature 2004, 429, 542–545. [Google Scholar] [CrossRef] [PubMed]
- Fjeldskaar, W.; Helset, H.M.; Johansen, H.; Grunnaleite, I.; Horstad, I. Thermal modelling of magmatic intrusions in the Gjallar Ridge, Norwegian Sea: Implications for vitrinite reflectance and hydrocarbon maturation. Basin Res. 2008, 20, 143–159. [Google Scholar] [CrossRef]
- Galushkin, Y.I. Thermal effects of igneous intrusions on maturity of organic matter: A possible mechanism of intrusion. Org. Geochem. 1997, 26, 645–658. [Google Scholar] [CrossRef]
- Aarnes, I.; Svensen, H.; Connolly, J.A.D.; Podladchikov, Y.Y. How contact metamorphism can trigger global climate changes: Modeling gas generation around igneous sills in sedimentary basins. Geochim. Cosmochim. Acta 2010, 74, 7179–7195. [Google Scholar] [CrossRef]
- Aarnes, I.; Svensen, H.; Polteau, S.; Planke, S. Contact metamorphic devolatilization of shales in the Karoo Basin, South Africa, and the effects of multiple sill intrusions. Chem. Geol. 2011, 281, 181–194. [Google Scholar] [CrossRef]
- Peace, A.; McCaffrey, K.; Imber, J.; Hobbs, R.; van Hunen, J.; Gerdes, K. Quantifying the influence of sill intrusion on the thermal evolution of organic-rich sedimentary rocks in nonvolcanic passive margins: An example from ODP 210–1276, offshore Newfoundland, Canada. Basin Res. 2017, 29, 249–265. [Google Scholar] [CrossRef]
- Spacapan, J.B.; Palma, J.O.; Galland, O.; Manceda, R.; Rocha, E.; D’Odorico, A.; Leanza, H.A. Thermal impact of igneous sill-complexes on organic-rich formations and implications for petroleum systems: A case study in the northern Neuquén Basin, Argentina. Mar. Pet. Geol. 2018, 91, 519–531. [Google Scholar] [CrossRef]
- Spacapan, J.B.; D’Odorico, A.; Palma, O.; Galland, O.; Rojas Vera, E.; Leanza, H.A.; Medialdea, A.; Manceda, R. Igneous petroleum systems in the Malargüe fold and thrust belt, Río Grande Valley area, Neuquén Basin, Argentina. Mar. Pet. Geol. 2020, 111, 309–331. [Google Scholar] [CrossRef]
- Sydnes, M.; Fjeldskaar, W.; Løtveit, I.F.; Grunnaleite, I.; Cardozo, N. The importance of sill thickness and timing of sill emplacement on hydrocarbon maturation. Mar. Pet. Geol. 2018, 89, 500–514. [Google Scholar] [CrossRef]
- Sydnes, M.; Fjeldskaar, W.; Grunnaleite, I.; Løtveit, I.F.; Mjelde, R. Transient thermal effects in sedimentary basins with normal faults and magmatic sill intrusions—A sensitivity study. Geosciences 2019, 9, 160. [Google Scholar] [CrossRef]
- Gudmundsson, A.; Løtveit, I.F. Sills as hydrocarbon reservoirs: Examples and models. Geol. Soc. Lond. Spec. Publ. 2012, 374, 251–271. [Google Scholar] [CrossRef]
- Montanari, D.; Bonini, M.; Corti, G.; Agostini, A.; Del Ventisette, C. Forced folding above shallow magma intrusions: Insights on supercritical fluid flow from analogue modelling. J. Volcanol. Geotherm. Res. 2017, 345, 67–80. [Google Scholar] [CrossRef]
- Haile, B.G.; Klausen, T.G.; Jahren, J.; Braathen, A.; Hellevang, H. Thermal history of a Triassic sedimentary sequence verified by a multi-method approach: Edgeøya, Svalbard, Norway. Basin Res. 2018, 30, 1075–1097. [Google Scholar] [CrossRef]
- Haile, B.G.; Czarniecka, U.; Xi, K.; Smyrak-Sikora, A.; Jahren, J.; Braathen, A.; Hellevang, H. Hydrothermally induced diagenesis: Evidence from shallow marine-deltaic sediments, Wilhelmøya, Svalbard. Geosci. Front. 2019, 10, 629–649. [Google Scholar] [CrossRef]
- Therkelsen, J. Diagenesis and reservoir properties of Middle Jurassic sandstones, Traill Ø, East Greenland: The influence of magmatism and faulting. Mar. Pet. Geol. 2016, 78, 196–221. [Google Scholar] [CrossRef]
- Allen, P.A.; Allen, J.R. Basin Analysis: Principles and Application to Petroleum Play Assessment, 3rd ed.; Wiley-Blackwell: Chichester, UK, 2014. [Google Scholar]
- Gluyas, J.; Swarbrick, R. Petroleum Geosciences; Blackwell Publishing: Oxford, UK, 2015. [Google Scholar]
- Bjørlykke, K.; Ramm, M.; Saigal, G.C. Sandstone diagenesis and porosity modification during basin evolution. Geol. Rundsch. 1989, 78, 243–268. [Google Scholar] [CrossRef]
- Bjørlykke, K. Relationships between depositional environments, burial history and rock properties. Some principal aspects of diagenetic process in sedimentary basins. Sediment. Geol. 2014, 301, 1–14. [Google Scholar] [CrossRef]
- Nobes, D.C.; Murray, R.W.; Kuramoto, S.; Pisciotto, K.A.; Holler, P. Impact of silica diagenesis on physical property variations. In Proceedings of the Ocean Drilling Program, Scientific Results, College Station, TX, USA; 1992; Volume 127/128. [Google Scholar] [CrossRef]
- Roaldset, E.; Wei, H. Silica-phase transformation of opal-A to opal_CT to quartz, Part I: An experimental diagenetic approach to natural observations. Prepared for submittal to AAPG. 1997. [Google Scholar]
- Roaldset, E.; Wei, H. Silica-phase transformation of opal-A to opal-CT to quartz, Part II: Changes of physical properties. Prepared for submittal to AAPG. 1997. [Google Scholar]
- Peltonen, C.; Marcussen, Ø.; Bjørlykke, K.; Jahren, J. Clay mineral diagenesis and quartz cementation in mudstones: The effects of smectite to illite reaction on rock properties. Mar. Pet. Geol. 2009, 26, 887–898. [Google Scholar] [CrossRef]
- Neagu, R.C.; Cartwright, J.; Davies, R. Measurement of diagenetic compaction strain from quantitative analysis of fault plane. J. Struct. Geol. 2010, 32, 641–655. [Google Scholar] [CrossRef]
- Elliot, W.C.; Aronson, J.L.; Matisoff, G.; Gautier, D.L. Kinetics of the smectite to illite transformation in the Denver Basin; clay mineral, K-Ar data, and mathematical model results. AAPG Bull. 1991, 75, 436–462. [Google Scholar]
- Buryakovski, L.A.; Djevanshir, R.D.; Chilingar, G.V. Abnormally-high formation pressures in Azerbaijan and the South Caspian Basin (as related to smectite ↔ illite transformations during diagenesis and catagenesis). J. Pet. Sci. Eng. 1995, 13, 203–218. [Google Scholar] [CrossRef]
- Worden, R.H.; Charpentier, D.; Fisher, Q.J.; Aplin, A.C. Fabric development and the smectite to illite transition in Upper Cretaceous mudstones from the North Sea: An image Analysis Approach. In Understanding the Micro to Macro Behaviour of Rock-Fluid Systems; Shaw, R.P., Ed.; Geological Society, Special Publications: London, UK, 2005; Volume 249, pp. 103–114. [Google Scholar]
- Robin, V.; Hebert, B.; Beaufort, D.; Sardini, P.; Tertre, E.; Regnault, O.; Descostes, M. Occurrence of authigenic beidellite in the Eocene transitional sandy sediments of the Chu-Saryssu basin (South-Central Kazakhstan). Sediment. Geol. 2015, 321, 39–48. [Google Scholar] [CrossRef]
- Dou, W.; Liu, L.; Wu, K.; Xu, Z.; Feng, X. Diagenesis of tight oil sand reservoirs: Upper Triassic tight sandstones of Yanchang Formation in Ordos Basin, China. Geol. J. 2018, 53, 707–724. [Google Scholar] [CrossRef]
- Morley, C.K.; Maczak, A.; Rungprom, T.; Ghosh, J.; Cartwright, J.A.; Bertoni, C.; Panpichityota, N. New style of honeycomb structures revealed on 3D seismic data indicate widespread diagenesis offshore Great South Basin, New Zealand. Mar. Pet. Geol. 2017, 86, 140–154. [Google Scholar] [CrossRef]
- Higgins, J.A.; Blättler, C.L.; Lundstrom, E.A.; Santiago-Ramos, D.P.; Akhtar, A.A.; Crüger Ahm, A.-S.; Bialik, O.; Holmden, C.; Bradbury, H.; Murray, S.T.; et al. Mineralogy, early marine diagensis, and the chemistry of shallow-water carbonate sediments. Geochim. Cosmochim. Acta 2018, 220, 512–534. [Google Scholar] [CrossRef]
- Fawad, M.; Mondol, N.H.; Baig, I.; Jahren, J. Diagenetic related flat spots within the Paleogene Sotbakken Group in the vicinity of the Senja Ridge, Barents Sea. Pet. Geosci. 2019, 122. [Google Scholar] [CrossRef]
- He, J.; Wang, H.; Zhang, C.; Yang, X.; Shangguan, Y.; Zhao, R.; Gong, Y.; Wu, Z. A comprehensive analysis of the sedimentology, petrography, diagenesis and reservoir quality of sandstones from the Oligocene Xiaganchaigou (E3) Formation in the Lengdong area, Qaidam Basin, China. J. Pet. Explor. Prod. Technol. 2019, 9, 953–969. [Google Scholar] [CrossRef]
- Kruge, M.A. Biomarker geochemistry of the Miocene Monterey Formation, West San Joaquin Basin, California: Implications for petroleum generation. Org. Geochem. 1986, 10, 517–530. [Google Scholar] [CrossRef]
- Behl, R.J. Chert spheroids of the Monterey Formation, California (USA): Early-diagenetic structures of bedded siliceous deposits. Sedimentology 2011, 58, 325–351. [Google Scholar] [CrossRef]
- Weller, R.; Behl, R.J. Physical and Mechanical Characteristics of the Opal-A to Opal-CT Transition Zone: Enhanced Diatomite Permeabiltiy from Heterogeneous Diagenetic Embrittlement. Search Discov. Artic. 2015, #51112, adapted from poster presentation given at Pacific section AAPG, SEG and SEPM Joint Technical Conference, Oxnard, California, USA, 3–5 May, 2015. [Google Scholar]
- Dralus, D. Chemical Interactions between Silicates and Their Pore Fluids: How They Affect Rock Physics Properties from Atomic to Reservoir Scales. Ph.D. Thesis, Stanford University, Stanford, CA, USA, August 2013. [Google Scholar]
- Dralus, D.; Lewan, M.D.; Peters, K. Kinetics of the Opal-A to Opal-CT Phase transition in Low- and High-TOC Siliceous Shale Source Rocks. Search Discov. Artic. 2015, #41708, adapted form oral presentation given at AAPG Annual Convention & Exhibition, Denver, Colorado, USA, May 31–June 3, 2015. [Google Scholar]
- Blystad, P.; Brekke, H.; Færseth, R.B.; Larsen, B.T.; Skogseid, J.; Tørudbakken, B. Structural Elements of the Norwegian Continental Shelf, Part II: The Norwegian Sea Region; Technical Report for Norwegian petroleum Directorate; Norwegian Petroleum Directorate: Stavanger, Norway, 1995; Volume 8, ISBN 82-7257-452-7.
- Davies, R.J.; Cartwright, J.A. Kilometer-scale chemical reaction boundary patters and deformation in sedimentary rocks. Earth Planet. Sci. Lett. 2007, 262, 125–137. [Google Scholar] [CrossRef]
- Peltonen, C.; Marcussen, Ø.; Bjørlykke, K.; Jahren, J. Mineralogical control on mudstone compaction: A study of Late Cretaceous to Early Tertiary mudstones of the Vøring and Møre basins, Norwegian Sea. Pet. Geosci. 2008, 14, 127–138. [Google Scholar] [CrossRef]
- Davies, R.J.; Ireland, M.T.; Cartwright, J.A. Differential compaction due to the irregular topology of a diagenetic reaction boundary: A new mechanism for the formation of polygonal faults. Basin Res. 2009, 21, 354–359. [Google Scholar] [CrossRef]
- Neagu, R.C.; Cartwright, J.; Davies, R.; Jensen, L. Fossilisation of a silica diagenesis reaction front on the mid-Norwegian margin. Mar. Pet. Geol. 2010, 27, 2141–2155. [Google Scholar] [CrossRef]
- Davies, R.J.; Ireland, M.T. Initiation and propagation of polygonal fault arrays by thermally triggered volume reduction reactions in siliceous sediment. Mar. Geol. 2011, 289, 150–158. [Google Scholar] [CrossRef]
- Ireland, M.T.; Davies, R.J.; Goulty, N.R.; Carruthers, D. Structure of a silica diagenetic transformation zone: The Gjallar Ridge, offshore Norway. Sedimentology 2011, 58, 424–441. [Google Scholar] [CrossRef]
- Wrona, T.; Taylor, K.G.; Jackson, C.A.-L.; Huuse, M.; Najorka, J.; Pan, I. Impact of silica diagenesis on the porosity of fine-grained strata: An analysis of Cenozoic mudstones from the North Sea. Geochem. Geophys. Geosyst. 2017, 18, 1537–1549. [Google Scholar] [CrossRef]
- Fjeldskaar, W.; Andersen, Å.; Johansen, H.; Lander, R.; Blomvik, V.; Skurve, O.; Michelsen, J.K.; Grunnaleite, I.; Mykkeltveit, J. Bridging the gap between basin modelling and structural geology. Reg. Geol. Metallog. 2017, 72, 65–77. [Google Scholar]
- Brekke, H. The tectonic evolution of the Norwegian Sea Continental Margin with emphasis on the Vøring and Møre Basins. In Dynamics of the Norwegian Margin; Special Publications; Nøttvedt, A., Ed.; Geological Society: London, UK, 2000; Volume 167, pp. 327–378. [Google Scholar]
- Bukovics, C.; Cartier, E.G.; Shaw, N.D.; Ziegler, P.A. Structure and development of the mid-Norway Continental Margin. In Petroleum Geology of the North. European Margin; Spencer, A.M., Ed.; Norwegian Petroleum Society: Stavanger, Norway; Graham and Trotman: Bristol, UK, 1984; pp. 407–423. [Google Scholar]
- Gabrielsen, R.H.; Færseth, R.; Hamar, G.; Rønnevik, H. Nomenclature of the main structural features on the Norwegian Continental Shelf north of the 62nd parallel. In Petroleum Geology of the North European Margin; Spencer, A.M., Ed.; Norwegian Petroleum Society: Stavanger, Norway; Graham and Trotman: Bristol, UK, 1984; pp. 41–60. [Google Scholar]
- Price, I.; Rattey, R.P. Cretaceous tectonics off mid-Norway: Implications for the Rockall and Faeroe-Shetland troughs. J. Geol. Soc. 1984, 141, 985–992. [Google Scholar] [CrossRef]
- Surlyk, F.; Piasecki, S.; Rolle, F.; Stemmerik, L.; Thomsen, E.; Wrang, P. The Permian base of East Greenland. In Petroleum Geology of the North European Margin; Spencer, A.M., Ed.; Norwegian Petroleum Society: Stavanger, Norway; Graham and Trotman: Bristol, UK, 1984; pp. 303–315. [Google Scholar]
- Brekke, H.; Riis, F. Tectonics and basin evolution of the Norwegian shelf between 62°N and 72°N. Nor. Geol. Tidsskr. 1987, 67, 295–322. [Google Scholar]
- Doré, A.G.; Lundin, E.R. Cenozoic compressional structures on the NE Atlantic margin: Nature, origin and potential significance for hydrocarbon exploration. Pet. Geosci. 1996, 2, 299–311. [Google Scholar] [CrossRef]
- Swiecicki, T.; Gibbs, P.B.; Farrow, G.E.; Coward, M.P. A tectonostratigraphic framework for the Mid-Norway region. Mar. Pet. Geol. 1998, 15, 245–276. [Google Scholar] [CrossRef]
- Scheck-Wenderoth, M.; Raum, T.; Faleide, J.I.; Mjelde, R.; Horsfield, B. The transition from the continent to the ocean: A deeper view on the Norwegian margin. J. Geol. Soc. 2007, 164, 855–868. [Google Scholar] [CrossRef]
- Mjelde, R.; Kodaira, S.; Shimamura, H.; Kanazawa, T.; Shiobara, H.; Berg, E.W.; Riise, O. Crustal structure of the central part of the Vøring Basin, mid- Norway margin, from ocean bottom seismographs. Tectonophysics 1997, 277, 235–257. [Google Scholar] [CrossRef]
- Talwani, M.; Eldholm, O. Evolution of the Norwegian-Greenland Sea. GSA Bull. 1977, 88, 969–999. [Google Scholar] [CrossRef]
- Eldholm, O.; Thiede, J.; Taylor, E. The Norwegian continental margin: Tectonic, volcanic, and paleoenvironmental framework. In Proceedings of the Ocean Drilling Program, Scientific Results, College Station, TX, USA; 1989; Volume 104. [Google Scholar] [CrossRef]
- Stuevold, L.M.; Eldholm, O. Cenozoic uplift of Fennoscandia inferred from a study of the mid-Norwegian margin. Glob. Planet. Chang. 1996, 12, 359–386. [Google Scholar] [CrossRef]
- Grunnaleite, I.; Fjeldskaar, W.; Wilson, J.; Faleide, J.I.; Zweigel, J. Effect of local variations of vertical and horizontal stresses on the Cenozoic structuring of the mid-Norwegian shelf. Tectonophysics 2009, 470, 267–283. [Google Scholar] [CrossRef]
- Dalland, A.; Worsley, D.; Ofstad, K. A Lithostrategraphic Scheme for the Mesozoic and Cenozoic Succession Offshore Mid- and Northern Norway; Technical Report for Norwegian petroleum Directorate; Norwegian Petroleum Directorate: Stavanger, Norway, 1988; Volume 4, ISBN 82-7275-241-9.
- Hjelstuen, B.O.; Eldholm, O.; Skogseid, J. Ceonzoic evolution of the northern Vøring margin. GSA Bull. 1999, 111, 1792–1807. [Google Scholar] [CrossRef]
- Svensen, H.; Planke, S.; Corfu, F. Zircon dating ties NE Atlantic sill emplacement to initial Eocene global warming. J. Geol. Soc. 2010, 167, 433–436. [Google Scholar] [CrossRef]
- Bungum, H.; Alsaker, A.; Kvamme, L.B.; Hansen, R.A. Seismicity and Seismotectonics of Norway and Nearby Continental Shelf Areas. J. Geophys. Res. 1991, 96, 2249–2265. [Google Scholar] [CrossRef]
- Byrkjeland, U.; Bungum, H.; Eldholm, O. Seismotectonics of the Norwegian continental margin. J. Geophys. Res. 2000, 105, 6221–6236. [Google Scholar] [CrossRef]
- Fjeldskaar, W.; Lindholm, C.; Dejls, J.F.; Fjeldskaar, I. Post-glacial uplift, neotectonics and seismicity in Fennoscandia. Quat. Sci. Rev. 2000, 19, 1413–1422. [Google Scholar] [CrossRef]
- Hicks, E.C.; Bungum, H.; Lidholm, C.D. Stress inversion of earthquake focal mechanism solutions from onshore and offshore Norway. Nor. Geol. Tidsskr. 2000, 80, 235–250. [Google Scholar] [CrossRef]
- Bungum, H.; Olesen, O.; Pascal, C.; Gibbons, S.; Lindholm, C.; Vestøl, O. To what extent is the present seismicity of Norway driven by post-glacial rebound? J. Geol. Soc. 2010, 167, 373–384. [Google Scholar] [CrossRef]
- Lander, R.H.; Langfeldt, M.; Bonnell, L.; Fjeldskaar, W. BMT User’s Guide; Tectonor AS Proprietary Publication: Stavanger, Norway, 1994. [Google Scholar]
- Fjeldskaar, W. BMTTM—Exploration tool combining tectonic and temperature modeling: Business Briefing: Exploration & Production. Oil Gas Rev. 2003, 1–4. [Google Scholar]
- Walderhaug, O.; Lander, R.H.; Bjørkum, P.A.; Oelkers, E.H.; Bjørlykke, K.; Nadeau, P.H. Modelling Quartz Cementation and Porosity in Reservoir Sandstones: Examples from the Norwegian Continental Shelf. In Quartz Cementation in Sandstones, Special Publication; Worden, R.H., Morad, S., Eds.; International Association of Sedimentologists: Algiers, Algeria, 2000; Volume 29, pp. 39–49. [Google Scholar]
- Walderhaug, O. Precipitation rates for quartz cement in sandstones determined by fluid-inclusion microthermometry and temperature-history modeling. J. Sediment. Res. 1994, A64, 324–333. [Google Scholar] [CrossRef]
- Walderhaug, O. Kinetic Modeling of Quartz Cementation and Porosity Loss in Deeply Buried Sandstone Reservoirs. AAPG Bull. 1996, 80, 731–745. [Google Scholar]
- Lander, R.H.; Walderhaug, O. Predicting Porosity through Simulating Sandstone Compaction and Quartz Cementation. AAPG Bull. 1999, 83, 433–449. [Google Scholar]
- Angevine, C.L.; Turcotte, D.L. Porosity reduction by pressure solution: A theoretical model for quartz arenites. Geol. Soc. Am. Bull. 1983, 94, 1129–1134. [Google Scholar] [CrossRef]
- Dewers, T.; Ortoleva, P. A coupled reaction/transport/mechanical model for intergranular pressure solution, stylolites, and differential compaction and cementation in clean sandstones. Geochim. Cosmochim. Acta 1990, 54, 1609–1625. [Google Scholar] [CrossRef]
- Mullis, A.M. The Role of Silica Precipitation Kinetics in Determining the Rate of Quartz Pressure Solution. J. Geophys. Res. 1991, 96, 10007–10013. [Google Scholar] [CrossRef]
- Ramm, M. Porosity-depth trends in reservoir sandstones: Theoretical models related to Jurassic sandstones offshore Norway. Mar. Pet. Geol. 1992, 9, 553–567. [Google Scholar] [CrossRef]
- Stephenson, L.P.; Plumley, W.J.; Palciauskas, V.V. A model for sandstone compaction by grain interpenetration. J. Sediment. Res. 1992, 62, 11–22. [Google Scholar]
- DeMaster, D.J. The supply and accumulation of silica in the marine environment. Geochim. Cosmochim. Acta 1981, 45, 1715–1732. [Google Scholar] [CrossRef]
- Kastner, M.; Keene, J.B.; Gieskes, J.M. Diagenesis of siliceous oozes—I. chemical controls on the rate of opal-A to opal-CT transformation—An experimental study. Geochim. Cosmochim. Acta 1977, 41, 1041–1059. [Google Scholar] [CrossRef]
- Grau, A.; Sterling, R.; Kidney, R. Success! Using Seismic Attributes and Horizontal Drilling to Delineate and Exploit a Diagenetic Trap, Monterey Shale, San Joaquin Valley, California. In Proceedings of the AAPG Annual Convention, Salt Lake City, Utah, USA, 11–14 May 2003. [Google Scholar]
- Kidney, R.; Sterling, R.; Grau, A. Delineation of a diagenetic trap using P-wave and converted-wave seismic data in Miocene McLure Shale, San Joaquin Basin, CA. In SEG Technical Program Expanded Abstracts 2005; Society of Exploration Geophysicists: Tulsa, OK, USA, 2005; pp. 2329–2332. [Google Scholar]
- Isaacs, C.M. Influence of rock composition on kinetics of silica phase changes in the Monterey Formation, Santa Barbara area, California. Geology 1982, 10, 304–308. [Google Scholar] [CrossRef]
- Keller, M.A.; Isaacs, C.M. An Evaluation of Temperature Scales for Silica Diagenesis in Diatomaceous Sequences Including a New Approach Based on the Miocene Formation, California. Geo-Mar. Lett. 1985, 5, 31–35. [Google Scholar] [CrossRef]
- Ernst, W.G.; Calvert, S.E. An Experimental Study of the Recrystallization of Porcelanite and its bearing on the Origin of some bedded Cherts. Am. J. Sci. 1969, 267, 114–133. [Google Scholar]
- Castellan, G.W. Physical Chemistry, 3rd ed.; Addison-Wesley Publishing Company: Reading, MA, USA, 1969. [Google Scholar]
- Jiang, S. Clay Minerals from the Perspective of Oil and Gas Exploration. In Clay Minerals in Nature—Their Characterization, Modification and Application; Valaškova, M., Martynkova, G.S., Eds.; IntechOpen: London, UK, 2012. [Google Scholar] [CrossRef]
- Bjørkum, P.A.; Nadeau, P.H. Temperature Controlled Porosity/Permeability Reduction, Fluid Migration, and Petroleum Exploration in Sedimentary Basins. APPEA J. 1998, 38, 453–465. [Google Scholar] [CrossRef]
- Pevear, D.R. Illite and hydrocarbon exploration. Proc. Natl. Acad. Sci. USA 1999, 96, 3440–3446. [Google Scholar] [CrossRef] [PubMed]
- Boles, J.R.; Franks, S.G. Clay Diagenesis in Wilcox sandstones of southwest Texas: Implications of smectite diagenesis on sandstone cementation. J. Sediment. Res. 1979, 49, 55–70. [Google Scholar]
- Huang., W.-L.; Longo, J.M.; Pevear, D.R. An experimentally derived kinetic model for smectite-to-illite conversion and its use as a geothermometer. Clays Clay Miner. 1993, 41, 162–177. [Google Scholar] [CrossRef]
- Roaldset, E.; Wei, H.; Grimstad, S. Smectite to illite conversion by hydrous pyrolysis. Clay Miner. 1998, 33, 147–158. [Google Scholar] [CrossRef]
- Nadeau, P.H.; Wilson, M.J.; McHardy, W.J.; Tait, J.M. Interstratified Clays as Fundamental Particles. Science 1984, 225, 923–925. [Google Scholar] [CrossRef]
- Nadeau, P.H.; Wilson, M.J.; McHardy, W.J.; Tait, J.M. The conversion of smectite to illite during diagenesis: Evidence from some illitic clays from bentonites and sandstones. Mineral. Mag. 1985, 49, 393–400. [Google Scholar] [CrossRef]
- Nadeau, P.H.; Bain, D.C. Composition of some smectites and diagenetic illitic clays and implications for their origin. Clays Clay Miner. 1986, 34, 455–464. [Google Scholar] [CrossRef]
- Bjørlykke, K.; Aagaard, P.; Egeberg, P.K.; Simmons, S.P. Geochemical constraints from formation water analyses from the North Sea and the Gulf Coast Basins on quartz, feldspar and illilte precipitation in reservoir rocks. In The Geochemistry of Reservoirs; Special Publication; Cubitt, J.M., England, W.A., Eds.; Geological Society: London, UK, 1995; Volume 86, pp. 33–50. [Google Scholar]
- Eberl, D.; Hower, J. Kinetics of illite formation. Geol. Soc. Am. Bull. 1976, 87, 1326–1330. [Google Scholar] [CrossRef]
- Wei, H.; Roaldset, E.; Bjorøy, M. Parallel Reaction kinetics of smectite to illite conversion. Clay Miner. 1996, 31, 365–376. [Google Scholar] [CrossRef]
- Hillier, S.; Matyas, J.; Matter, A.; Vasseur, G. Illite/Smectite diagenesis and its variable correlation with vitrinite reflectance in the Pannonian Basin. Clays Clay Miner. 1995, 43, 174–183. [Google Scholar] [CrossRef]
- Sachsenhofer, R.F.; Rantitcsh, G.; Hasenhüttl, C.; Russegger, B.; Jelen, B. Smectite to illite diagenesis in early Miocene sediments from the hyperthermal western Pannonian Basin. Clay Miner. 1998, 33, 523–537. [Google Scholar]
- Anell, I.; Thybo, H.; Artemieva, I.M. Cenozoic uplift and subsidence in the North Atlantic region: Geological evidence revisited. Tectonophysics 2009, 474, 78–105. [Google Scholar] [CrossRef]
- Anell, I.; Thybo, H.; Stratford, W. Relating Cenozoic North Sea sediments to topography in southern Norway: The interplay between tectonics and climate. Earth Planet. Sci. Lett. 2010, 300, 19–32. [Google Scholar] [CrossRef]
- Nielsen, S.B.; Gallagher, K.; Leighton, C.; Balling, N.; Svenningsen, L.; Jacobsen, B.H.; Thomsen, E.; Nielsen, O.B.; Heilmann-Clausen, C.; Egholm, D.L.; et al. The evolution of western Scandinavian topography: A review of Neogene uplift versus the ICE (isostasy–climate–erosion) hypothesis. J. Geodyn. 2009, 47, 72–95. [Google Scholar] [CrossRef]
- Nielsen, S.B.; Clausen, O.R.; Jacobsen, B.H.; Thomsen, E.; Huuse, M.; Gallagher, K.; Balling, N.; Egholm, D. The ICE hypothesis stands: How the dogma of late Ceonozoic tectonic uplift can no longer be sustained in the light of data and physical laws. J. Geodyn. 2010, 50, 102–111. [Google Scholar] [CrossRef]
- Sclater, J.G.; Christie, P.A.F. Continental stretching: An explanation of the post-mid-cretaceous subsidence of the central North Sea basin. J. Geophys. Res. 1980, 85, 3711–3739. [Google Scholar] [CrossRef]
- Čermác, V.; Rybach, L. Thermal properties: Thermal conductivity and specific heat of minerals and rocks. In Landolt-Börnstein Zahlenwerte und Functionen aus Naturwissenschaften und Technik, Neue Serie, Physikalische Eigenschaften der Gesteine; Angeneister, G., Ed.; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1982; pp. 305–343. [Google Scholar]
- Pollastro, R.M. Considerations and Applications of the Illite/Smectite Geothermometer in Hydrocarbon-Bearing Rocks of Miocene to Mississippian Age. Clays Clay Miner. 1993, 41, 119–133. [Google Scholar] [CrossRef]
- Gudmundsson, A. Rock Fractures in Geological Processes, 1st ed.; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Bell, F.G. Engineering Properties of Soils and Rocks, 4th ed.; Blackwell: Oxford, UK, 2000. [Google Scholar]
- Farmer, I. Engineering Behaviour of Rocks, 2nd ed.; Chapman and Hall: London, UK, 1983. [Google Scholar]
- Paterson, M.S.; Wong, T.F. Experimental Rock Deformation: The Brittle Field, 2nd ed.; Springer: Berlin, Germany, 2005. [Google Scholar]
- Haimson, B.C.; Rummel, F. Hydrofracturing stress measurements in the Iceland research drilling project drill hole at Reydarfjördur, Iceland. J. Geophys. Res. 1982, 87, 6631–6649. [Google Scholar] [CrossRef]
- Schultz, R.A. Limits on strength and deformation properties of jointed basaltic rock masses. Rock Mech. Rock Eng. 1995, 28, 1–15. [Google Scholar] [CrossRef]
- Amadei, B.; Stephansson, O. Rock Stress and Its Measurement; Chapman and Hall: London, UK, 1997. [Google Scholar]
- Gudmundsson, A.; Brenner, S.L. How hydrofractures become arrested. Terra Nova 2001, 13, 456–462. [Google Scholar] [CrossRef]
- Gudmundsson, A.; Fjeldskaar, I.; Brenner, S.L. Propagation pathways and fluid transport of hydrofractures in jointed and layered rocks in geothermal fields. J. Volcanol. Geotherm. Res. 2002, 116, 257–278. [Google Scholar] [CrossRef]
- Fjeldskaar, W.; Prestholm, E.; Guargena, C.; Stephenson, M. Mineralogical and diagenetic control on the thermal conductivity of the sedimentary sequences in the Bjørnøya Basin, Barents Sea. In Basin Modelling: Advances and Applications, Special Publication; Doré, A.G., Ed.; NPF: Stavanger, Norway, 1993; Volume 3, pp. 445–453. [Google Scholar]
- Ireland, M.; Goulty, N.R.; Davies, R.J. Influence of pore water chemistry on silica diagenesis: Evidence from the interaction of diagenetic readtion zones with polygonal fault systems. J. Geol. Soc. 2010, 167, 273–279. [Google Scholar] [CrossRef]
- McKinley, J.M.; Worden, R.H.; Ruffell, A.H. Contact Diagenesis: The effect of an intrusion on reservoir quality in the Triassic Sherwood Sandstone group, Northern Ireland. J. Sediment. Res. 2001, 71, 484–495. [Google Scholar] [CrossRef]
- Bernet, M.; Gaupp, R. Diagenetic history of Triassic sandstone from the Beacon Supergroup in central Victoria Land, Antarctica. N. Z. J. Geol. Geophys. 2005, 48, 447–458. [Google Scholar] [CrossRef]
- Grove, C. Direct and Indirect Effects of Flood Basalt Volcanism on Reservoir Quality Sandstone. Ph.D. Thesis, Durham University, Durham and Stockton-on-Tees, UK, 2014. [Google Scholar]
- Nejbert, K.; Krajewski, K.P.; Dubinska, E.; Pécskay, Z. Dolerites of Svalbard, north-west Barents Sea Shelf: Age, tectonic setting and significance for geotectonic interpretation of the High-Arctic Large Igneous Province. Polar Res. 2011, 30, 7306. [Google Scholar] [CrossRef]
- Ehrenberg, S.N. Relationship between Diagenesis and Reservoir Quality in Sandstones of the Garn Formation, Haltenbanken, Mid-Norwegian Continental Shelf. AAPG Bull. 1990, 74, 1538–1558. [Google Scholar] [CrossRef]
- Bjørlykke, K.; Nedkvitne, T.; Ramm, M.; Saigal, G.C. Diagenetic processes in the Brent Group (Middle Jurassic) reservoirs of the North Sea: An overview. In Geology of the Brent Group; Special Publication; Morton, A.C., Haszeldine, R.S., Giles, M.R., Brown, S., Eds.; Geological Society: London, UK, 1992; Volume 61, pp. 263–287. [Google Scholar]
- Ehrenberg, S.N. Preservation of Anomalously High Porosity in Deeply Buried Sandstones by Grain-Coating Chlorite: Examples from the Norwegian Continental Shelf. AAPG Bull. 1993, 77, 1260–1286. [Google Scholar]
- Freiburg, J.T.; Ritzi, R.W.; Kehoe, K.S. Depositional and diagenetic controls on anomalously high porosity within a deeply buried CO2 storage reservoir—The Cambrian Mt. Simon Sandstone, Illinois Basin, USA. Int. J. Greenh. Gas Control 2016, 55, 42–54. [Google Scholar] [CrossRef]
- Lin, W.; Chen, L.; Lu, Y.; Hu, H.; Liu, L.; Liu, X.; Wei, W. Diagenesis and its impact on reservoir quality for the Chang 8 oil group tight sandstone of the Yanchang Formation (upper Triassic) in southwestern Ordos basin, China. J. Pet. Explor. Prod. Technol. 2017, 7, 947–959. [Google Scholar] [CrossRef]
- PetroWiki.org. Available online: https://petrowiki.org/Diatomite (accessed on 27 September 2019).
- Tsuji, T.; Masui, Y.; Yokoi, S. New hydrocarbon trap models for the diagenetic transformation of opal-CT to quartz in Neogene siliceaous rocks. AAPG Bull. 2011, 95, 449–477. [Google Scholar] [CrossRef]
- Davies, R.J.; Cartwright, J. A fossilized Opal A to Opal C/T transformation on the northeast Atlantic margin: Support for a significantly elevated Palaeogeothermal gradient during the Neogene? Basin Res. 2002, 14, 467–486. [Google Scholar] [CrossRef]
- Hall, P.L.; Astill, D.M.; McConnell, J.D.C. Thermodynamic and structural aspects of the dehydration of smectites in sedimentary rocks. Clay Miner. 1986, 21, 633–648. [Google Scholar] [CrossRef]
- Singer, A.; Müller, G. Diagenesis in argillaceous sediments. In Diagenesis in Sediments and Sedimentary Rocks; Larsen, G., Chilingar, G.V., Eds.; Elsevier: Amsterdam, The Netherlands, 1983. [Google Scholar]
- Essene, E.J.; Peacor, D.R. Clay mineral thermometry—A critical perspective. Clays Clay Miner. 1995, 43, 540–553. [Google Scholar] [CrossRef]
- Podladchikov, Y.Y.; Wickham, S.M. Crystallization of Hydrous Magmas: Calculation of Associated Thermal Effects, Volatile Fluxes, and Isotopic Alteration. J. Geol. 1994, 102, 25–45. [Google Scholar] [CrossRef]
- Iyer, K.; Rüpke, L.; Galerne, C.Y. Modeling fluid flow in sedimentary basins with sill intrusions: Implications for hydrothermal venting and climate change. Geochem. Geophys. Geosyst. 2013, 14, 5244–5262. [Google Scholar] [CrossRef]
- Iyer, K.; Schmid, D.W.; Planke, S.; Millett, J. Modelling hydrothermal venting in volcanic sedimentary basins: Impact on hydrocarbon maturation and paleoclimate. Earth Planet. Sci. Lett. 2017, 467, 30–42. [Google Scholar] [CrossRef]
- Wang, D.; Manga, M. Organic matter maturation in the contact aureole of an igneous sill as a tracer of hydrothermal convection. J. Geophys. Res. Solid Earth 2015, 120, 4102–4112. [Google Scholar] [CrossRef]
- Annen, C. Factors affecting the thickness of thermal aureoles. Front. Earth Sci. 2017, 5, 1–13. [Google Scholar] [CrossRef]
- Planke, S.; Rasmussen, T.; Rey, S.; Myklebust, R. Seismic characteristics and distribution of volcanic intrusions and hydrothermal vent complexes in the Vøring and Møre basins. In Petroleum Geology: North-West Europe and Global Perspectives, Proceedings of the 6th Petroleum Geology Conference; Dorè, A.G., Vining, B.A., Eds.; Geological Society: London, UK, 1 January 2005; pp. 833–844. [Google Scholar]









| Diagenetic Process | Ea (kJ mol−1) | A0 (mol/cm2s) | |
|---|---|---|---|
| Opal A—opal CT—quartz | Dralus et al. [39] | 36.99 (low TOC) | 1.04 × 1014 |
| 33.44 (high TOC) | 3.01 × 1010 | ||
| 32.52 (Quartz) | 1.96 × 109 | ||
| Smectite to illite | Roaldset et al. [95] | 33 | 1.02 × 108 |
| 2.72 × 109 | |||
| Huang et al. [94] | 28 | 8.08 × 104 | |
| Hillier et al. [102]* | 31 | 1.81 × 103 |
| Lithology | Porosity—Depth Trend | Conductivity (kv) (Wm−1 K−1) | Heat Capacity | ||
|---|---|---|---|---|---|
| Surface Porosity | Exponential Constant (km−1) | Low Porosity | High Porosity | (Jkg−1K−1) | |
| Sandstone | 0.45 | 0.27 | 3.30 (6%) | 1.50 (45%) | 1080 |
| Claystone | 0.63 | 0.51 | 1.70 (6%) | 1.00 (60%) | 940 |
| Shale | 0.63 | 0.51 | 2.00 (6%) | 1.40 (60%) | 1190 |
| Basement, metamorphic | 3.10 | 3.10 | 1100 | ||
| Magmatic intrusions | 3.10 | 3.10 | 1100 | ||
| Asthenosphere | 3.50 | 3.50 | 1100 | ||
| Young’s Modules | |||||
|---|---|---|---|---|---|
| Host Rock | Area Modified by Diagenesis | Basement | Fault Core/Damage Zone | Fault Core/Damage Zone Modified by Diagenesis | Sills |
| 10 GPa | 20 GPa | 50 GPa | 0.1 GPa/1 GPa | 0.2 GPa/2 GPa | 50 GPa |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sydnes, M.; Fjeldskaar, W.; Grunnaleite, I.; Løtveit, I.F.; Mjelde, R. The Influence of Magmatic Intrusions on Diagenetic Processes and Stress Accumulation. Geosciences 2019, 9, 477. https://doi.org/10.3390/geosciences9110477
Sydnes M, Fjeldskaar W, Grunnaleite I, Løtveit IF, Mjelde R. The Influence of Magmatic Intrusions on Diagenetic Processes and Stress Accumulation. Geosciences. 2019; 9(11):477. https://doi.org/10.3390/geosciences9110477
Chicago/Turabian StyleSydnes, Magnhild, Willy Fjeldskaar, Ivar Grunnaleite, Ingrid Fjeldskaar Løtveit, and Rolf Mjelde. 2019. "The Influence of Magmatic Intrusions on Diagenetic Processes and Stress Accumulation" Geosciences 9, no. 11: 477. https://doi.org/10.3390/geosciences9110477
APA StyleSydnes, M., Fjeldskaar, W., Grunnaleite, I., Løtveit, I. F., & Mjelde, R. (2019). The Influence of Magmatic Intrusions on Diagenetic Processes and Stress Accumulation. Geosciences, 9(11), 477. https://doi.org/10.3390/geosciences9110477





