Modelling the Repair of Carbon-Centered Protein Radicals by Phenolic Antioxidants
Abstract
:1. Introduction
2. Computational Methodology
3. Results and Discussion
3.1. Investigating the Formal-Hydrogen Atom Transfer (f-HAT) Repair Reactions
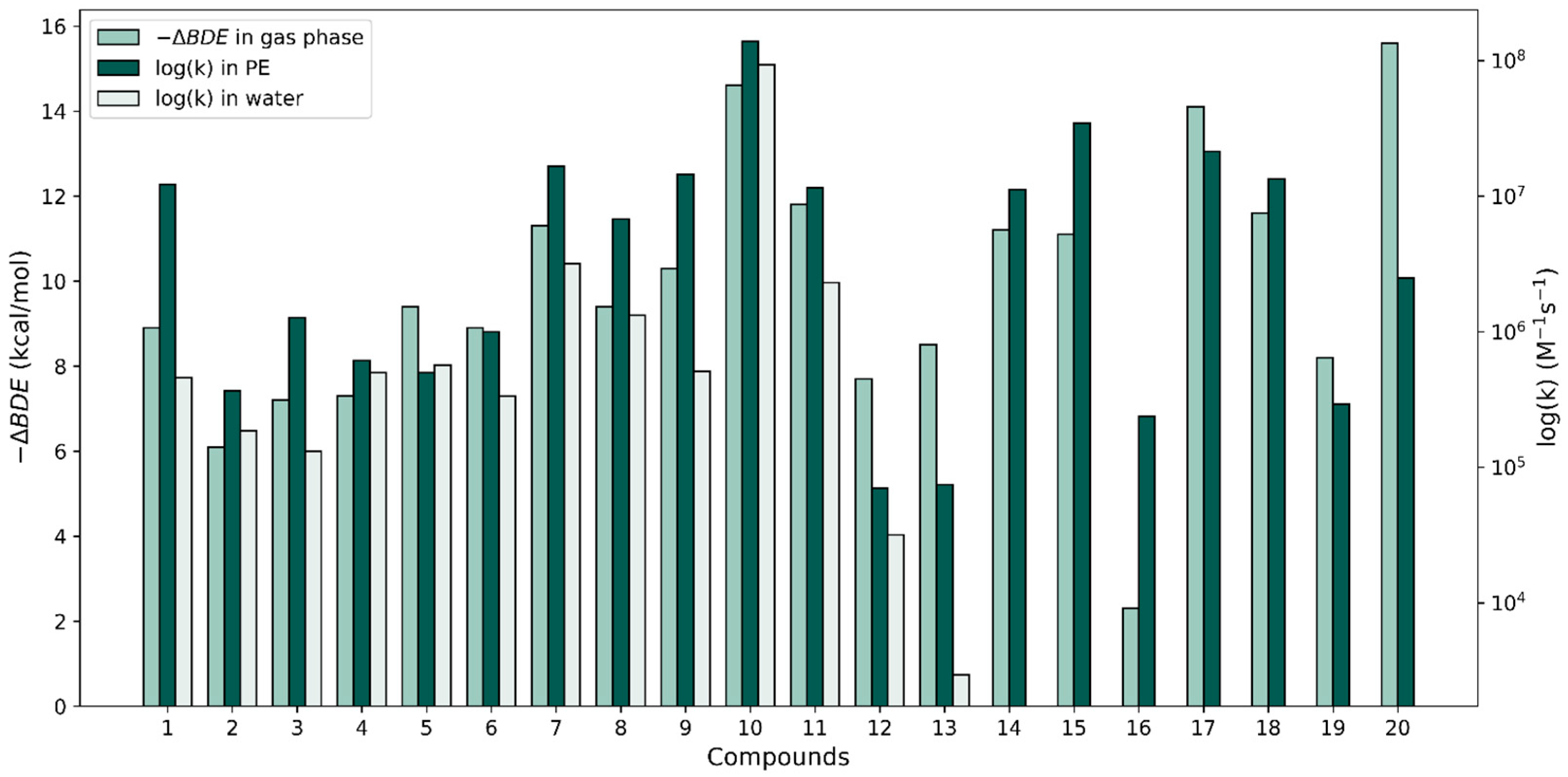
3.1.1. Thermodynamic Study of f-HAT Reactions
3.1.2. Kinetic Study of Hydrogen Atom Transfer Reactions
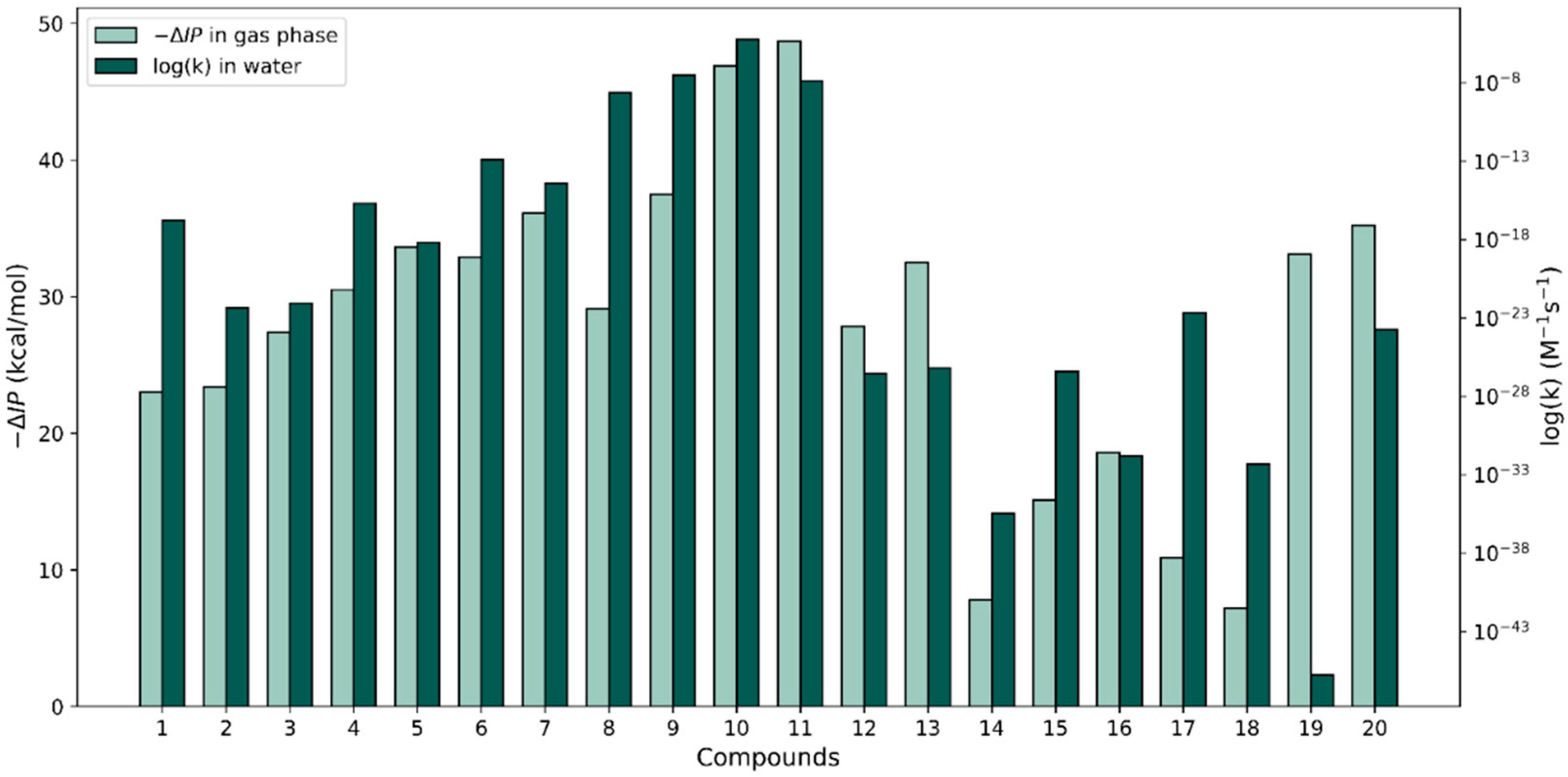
3.2. Investigating the Single-Electron Transfer Repair Reaction
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Davies, M.J. The oxidative environment and protein damage. Biochim. Biophys. Acta Proteins Proteom. 2005, 1703, 93–109. [Google Scholar] [CrossRef] [PubMed]
- Liguori, I.; Russo, G.; Curcio, F.; Bulli, G.; Aran, L.; Della-Morte, D.; Gargiulo, G.; Testa, G.; Cacciatore, F.; Bonaduce, D.; et al. Oxidative stress, aging, and diseases. Clin. Interv. Aging 2018, 13, 757–772. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Wang, W.; Li, L.; Perry, G.; Lee, H.G.; Zhu, X. Oxidative stress and mitochondrial dysfunction in Alzheimer’s disease. Biochim. Biophys. Acta Mol. Basis Dis. 2014, 1842, 1240–1247. [Google Scholar] [CrossRef] [PubMed]
- Valko, M.; Leibfritz, D.; Moncol, J.; Cronin, M.; Mazur, M.; Telser, J. Free radicals and antioxidants in normal physiological functions and human disease. Int. J. Biochem. Cell Biol. 2007, 39, 44–84. [Google Scholar] [CrossRef]
- Anderson, E.J.; Kypson, A.P.; Rodriguez, E.; Anderson, C.A.; Lehr, E.J.; Neufer, P.D. Substrate-Specific Derangements in Mitochondrial Metabolism and Redox Balance in the Atrium of the Type 2 Diabetic Human Heart. J. Am. Coll. Cardiol. 2009, 54, 1891–1898. [Google Scholar] [CrossRef]
- Serpillon, S.; Floyd, B.C.; Gupte, R.S.; George, S.; Kozicky, M.; Neito, V.; Recchia, F.; Stanley, W.; Wolin, M.S.; Gupte, S.A. Superoxide production by NAD(P)H oxidase and mitochondria is increased in genetically obese and hyperglycemic rat heart and aorta before the development of cardiac dysfunction. The role ofglucose-6-phosphate dehydrogenase-derived NADPH. Am. J. Physiol. Heart Circ. Physiol. 2009, 297, H153–H162. [Google Scholar] [CrossRef]
- Pisoschi, A.M.; Pop, A.; Iordache, F.; Stanca, L.; Predoi, G.; Serban, A.I. Oxidative stress mitigation by antioxidants—An overview on their chemistry and influences on health status. Eur. J. Med. Chem. 2021, 209, 112891. [Google Scholar] [CrossRef]
- Wu, D.; Cederbaum, A.I. Alcohol, oxidative stress, and free radical damage. Alcohol Res. Health 2003, 27, 277–284. [Google Scholar]
- Lü, J.M.; Lin, P.H.; Yao, Q.; Chen, C. Chemical and molecular mechanisms of antioxidants: Experimental approaches and model systems. J. Cell. Mol. Med. 2010, 14, 840–860. [Google Scholar] [CrossRef]
- Khalil, I.; Yehye, W.A.; Etxeberria, A.E.; Alhadi, A.A.; Dezfooli, S.M.; Julkapli, N.B.M.; Basirun, W.J.; Seyfoddin, A. Nanoantioxidants: Recent Trends in Antioxidant Delivery Applications. Antioxidants 2019, 9, 24. [Google Scholar] [CrossRef]
- Pisoschi, A.M.; Pop, A. The role of antioxidants in the chemistry of oxidative stress: A review. Eur. J. Med. Chem. 2015, 97, 55–74. [Google Scholar] [CrossRef] [PubMed]
- Kehrer, J.P. The Haber–Weiss reaction and mechanisms of toxicity. Toxicology 2000, 149, 43–50. [Google Scholar] [CrossRef] [PubMed]
- Lipinski, B. Hydroxyl Radical and Its Scavengers in Health and Disease. Oxid. Med. Cell. Longev. 2011, 2011, 809696. [Google Scholar] [CrossRef] [PubMed]
- Sies, H. Strategies of antioxidant defense. Eur. J. Biochem. 1993, 215, 213–219. [Google Scholar] [CrossRef]
- Poljsak, B.; Šuput, D.; Milisav, I. Achieving the Balance between ROS and Antioxidants: When to Use the Synthetic Antioxidants. Oxid. Med. Cell. Longev. 2013, 2013, 956792. [Google Scholar] [CrossRef]
- Dündar, Y.; Aslan, R. Antioxidative Stress. East. J. Med. 2000, 5, 45–47. [Google Scholar]
- Podmore, I.D.; Griffiths, H.R.; Herbert, K.E.; Mistry, N.; Mistry, P.; Lunec, J. Vitamin C exhibits pro-oxidant properties. Nature 1998, 392, 559. [Google Scholar] [CrossRef]
- Procházková, D.; Boušová, I.; Wilhelmová, N. Antioxidant and prooxidant properties of flavonoids. Fitoterapia 2011, 82, 513–523. [Google Scholar] [CrossRef]
- Chong, J.; Poutaraud, A.; Hugueney, P. Metabolism and roles of stilbenes in plants. Plant Sci. 2009, 177, 143–155. [Google Scholar] [CrossRef]
- Davies, M.J. Oxidative Damage to Proteins. In Encyclopedia of Radicals in Chemistry, Biology and Materials; American Cancer Society: Atlanta, GA, USA, 2012. [Google Scholar]
- Buxton, G.V.; Greenstock, C.L.; Helman, W.P.; Ross, A.B. Critical review of rate constants for reactions of hydrated electrons, hydrogen atoms and hydroxyl radicals (∙OH/∙O-) in aqueous solution. J. Phys. Chem. Ref. Data 1988, 17, 513. [Google Scholar] [CrossRef]
- Ramis, R.; Casasnovas, R.; Ortega-Castro, J.; Frau, J.; Álvarez-Idaboy, J.R.; Mora-Diez, N. Modelling the repair of carbon-centred protein radicals by the antioxidants glutathione and Trolox. New J. Chem. 2019, 43, 2085–2097. [Google Scholar] [CrossRef]
- Castañeda-Arriaga, R.; Domínguez-Castro, A.; Lee, J.; Alvarez-Idaboy, J.R.; Mora-Diez, N. Chemical repair of protein carbon-centred radicals: Long-distance dynamic factors. Can. J. Chem. 2016, 94, 1119–1126. [Google Scholar] [CrossRef]
- Castañeda-Arriaga, R.; Mora-Diez, N.; Alvarez-Idaboy, J.R. Modelling the chemical repair of protein carbon-centered radicals formed via oxidative damage with dihydrolipoic acid. RSC Adv. 2015, 5, 96714–96719. [Google Scholar] [CrossRef]
- Watts, Z.I.; Easton, C.J. Peculiar Stability of Amino Acids and Peptides from a Radical Perspective. J. Am. Chem. Soc. 2009, 131, 11323–11325. [Google Scholar] [CrossRef] [PubMed]
- Muñoz-Rugeles, L.; Galano, A.; Alvarez-Idaboy, J.R. The role of acid-base equilibria in formal hydrogen transfer reactions: Tryptophan radical repair by uric acid as paradigmatic case. Phys. Chem. Chem. Phys. 2017, 19, 15296. [Google Scholar] [CrossRef]
- Domazou, A.S.; Koppenol, W.H.; Gebicki, J.M. Efficient repair of protein radicals by ascorbate. Free Radic. Biol. Med. 2009, 46, 1049. [Google Scholar] [CrossRef]
- Felter, S.P.; Zhang, X.; Thompson, C. Butylated hydroxyanisole: Carcinogenic food additive to be avoided or harmless antioxidant important to protect food supply? Regul. Toxicol. Pharmacol. 2021, 121, 104887. [Google Scholar] [CrossRef]
- Guitard, R.; Nardello-Rataj, V.; Aubry, J.M. Theoretical and Kinetic Tools for Selecting Effective Antioxidants: Application to the Protection of Omega-3 Oils with Natural and Synthetic Phenols. Int. J. Mol. Sci. 2016, 17, 1220. [Google Scholar] [CrossRef]
- Tappel, A.L.; Zalkin, H. Inhibition of Lipid Peroxidation in Microsomes by Vitamin E. Nature 1960, 185, 35. [Google Scholar] [CrossRef]
- Tappel, A.L. Vitamin E and selenium protection from in vivo lipid peroxidation*. Ann. N. Y. Acad. Sci. 1980, 355, 18–31. [Google Scholar] [CrossRef]
- Penner, N.A.; Nesterenko, P.N. Simultaneous determination of dihydroxybenzenes, aminophenols and phenylenediamines in hair dyes by high-performance liquid chromatography on hypercross-linked polystyrene. Analyst 2000, 125, 1249–1254. [Google Scholar] [CrossRef] [PubMed]
- Mitchell, S.C.; Waring, R.H. Aminophenols. In Kirk-Othmer Encyclopedia of Chemical Technology, 5th ed.; Kirk-Othmer, Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Tsopmo, A.; Awah, F.M.; Kuete, V. 12—Ligands and stilbenes from African medicinal plants. In Medicinal Plant Research in Africa; Elsevier: Amsterdam, The Netherlands, 2013; pp. 435–478. [Google Scholar]
- Giacomini, E.; Rupiani, S.; Guidotti, L.; Recanatini, M.; Roberti, M. The use of stilbene scaffold in medicinal chemistry and multi-target drug design. Curr. Med. Chem. 2016, 23, 2439–2489. [Google Scholar] [CrossRef] [PubMed]
- Özduran, G.; Becer, E.; Vatansever, H.S. The Role and Mechanisms of Action of Catechins in Neurodegenerative Diseases. JANA 2023, 42, 67–74. [Google Scholar]
- Wright, J.S.; Johnson, E.R.; DiLabio, G.A. Predicting the activity of phenolic antioxidants: Theoretical method, analysis of substituent effects, and application to major families of antioxidants. J. Am. Chem. Soc. 2001, 123, 1173–1183. [Google Scholar] [CrossRef]
- Bell, R.P. The theory of reactions involving proton transfers. Proc. R. Soc. Lond. Ser. A 1936, 154, 414–429. [Google Scholar]
- Evans, M.G.; Polanyi, M. Further considerations on the thermodynamics of chemical equilibria and reaction rates. Trans. Faraday Soc. 1936, 32, 1333–1360. [Google Scholar] [CrossRef]
- Carreon-Gonzalez, M.; Muñoz-Rugeles, L.; Vivier-Bunge, A.; Alvarez-Idaboy, J.R. Chemical repair of damaged leucine and tryptophane by thiophenols at close to diffusion-controlled rates: Mechanisms and kinetics. J. Comput. Chem. 2022, 43, 556. [Google Scholar] [CrossRef]
- Galano, A.; Raúl Alvarez-Idaboy, J.R. Computational strategies for predicting free radical scavengers’ protection against oxidative stress: Where are we and what might follow? Int. J. Quantum Chem. 2019, 119, e25665. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2016.
- Zhao, Y.; Truhlar, D.G. The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: Two new functionals and systematic testing of four M06-class functionals and 12 other function. Theor. Chem. Acc. 2008, 120, 215–241. [Google Scholar] [CrossRef]
- Allodi, M.A.; Kirschner, K.N.; Shields, G.C. Thermodynamics of the Hydroxyl Radical Addition to Isoprene. J. Phys. Chem. A 2008, 112, 7064–7071. [Google Scholar] [CrossRef]
- Marenich, A.V.; Cramer, C.J.; Truhlar, D.G. Universal solvation model based on solute electron density and on a continuum model of the solvent defined by the bulk dielectric constant and atomic surface tensions. J. Phys. Chem. B 2009, 113, 6378–6396. [Google Scholar] [CrossRef] [PubMed]
- Castañeda-Arriaga, R.; Pérez-González, A.; Reina, M.; Alvarez-Idaboy, J.R.; Galano, A. Comprehensive Investigation of the Antioxidant and Pro-oxidant Effects of Phenolic Compounds: A Double-Edged Sword in the Context of Oxidative Stress? J. Phys. Chem. B 2018, 122, 6198–6214. [Google Scholar] [CrossRef] [PubMed]
- Vo, Q.V.; Van Bay, M.; Nam, P.C.; Mechler, A. Hydroxyl Radical Scavenging of Indole-3-Carbinol: A Mechanistic and Kinetic Study. ACS Omega 2019, 4, 19375–19381. [Google Scholar] [CrossRef]
- Ramis, R.; Casasnovas, R.; Mariño, L.; Frau, J.; Adrover, M.; Vilanova, B.; Mora-Diez, N.; Ortega-Castro, J. A Density Functional Theory Study of the Free-Radical Scavenging Activity of Aminoguanidine. Int. J. Quantum Chem. 2019, 119, e25911. [Google Scholar] [CrossRef]
- Galano, A.; Alvarez-Idaboy, J.R. Kinetics of radical-molecule reactions in aqueous solution: A benchmark study of the performance of density functional methods. J. Comp. Chem. 2014, 35, 2019–2026. [Google Scholar] [CrossRef]
- Brown, R.L. A Method of Calculating Tunneling Corrections for Eckart Potential Barriers. J. Res. Natl. Bur. Stand. 1981, 86, 357–359. [Google Scholar] [CrossRef]
- Okuno, Y. Theoretical Investigation of the Mechanism of the Baeyer-Villiger Reaction in Nonpolar Solvents. Chem. Eur. J. 1997, 3, 212–218. [Google Scholar] [CrossRef]
- Ramesh, N.; Davis, P.K.; Zielinski, J.M.; Danner, R.P.; Duda, J.L. Application of free-volume theory to self diffusion of solvents in polymers below the glass transition temperature: A review. J. Polym. Sci. B Polym. Phys. 2011, 49, 1629–1644. [Google Scholar] [CrossRef]
- Collins, F.C.; Kimball, G.E. Diffusion-controlled reaction rates. J. Colloid Sci. 1949, 4, 425–437. [Google Scholar] [CrossRef]
- Smoluchowski, M.Z. Versucheiner Mathematischen Theorie der Koagulations Kinetic Kolloider Lousungen. J. Phys. Chem. 1917, 92, 129–168. [Google Scholar]
- Einstein, A. On the motion of small particles suspended in liquids at rest required by the molecular-kinetic theory of heat. Ann. Phys. 1905, 17, 549–560. [Google Scholar] [CrossRef]
- Stokes, G.G. Mathematical and Physical Papers; Cambridge University Press: Cambridge, UK, 1903; Volume 3. [Google Scholar]
- Walton-Raaby, M.; Floen, T.; García-Díez, G.; Mora-Diez, N. Calculating the Aqueous pKa of Phenols: Predictions for Antioxidants and Cannabinoids. Antioxidants 2023, 12, 1420. [Google Scholar] [CrossRef] [PubMed]
- Marcus, R.A. Chemical and Electrochemical Electron-Transfer Theory. Annu. Rev. Phys. Chem. 1964, 15, 155–196. [Google Scholar] [CrossRef]
- Marcus, R.A. Electron transfer reactions in chemistry theory and experiment. Rev. Mod. Phys. 1993, 65, 599–610. [Google Scholar] [CrossRef]
- Galano, A.; Alvarez-Idaboy, J.R. A computational methodology for accurate predictions of rate constants in solution: Application to the assessment of primary antioxidant activity. J. Comput. Chem. 2013, 34, 2430–2445. [Google Scholar] [CrossRef]
- Sun, Z.D.; Yang, X.X.; Liu, Q.S.; Li, C.H.; Zhou, Q.F.; Fiedler, H.; Liao, C.; Zhang, J.; Jiang, G. Butylated hydroxyanisole isomers induce distinct adipogenesis in 3T3-L1 cells. J. Hazard. Mater. 2019, 379, 120794. [Google Scholar] [CrossRef]
- Shahidi, F.; Ambigaipalan, P. Phenolics and polyphenolics in foods, beverages and spices: Antioxidant activity and health effects—A review. J. Funct. Foods 2015, 18, 820–897. [Google Scholar]
- Amorati, R.; Cavalli, A.; Fumo, M.G.; Masetti, M.; Menichetti, S.; Pagliuca, C.; Pedulli, G.F.; Viglianisi, C. Kinetic and Thermochemical Study of the Antioxidant Activity of Sulfur-Containing Analogues of Vitamin E. Chem. Eur. J. 2007, 13, 8223–8230. [Google Scholar] [CrossRef]
- Wright, J.S.; Carpenter, D.J.; Mckay, D.J.; Ingold, K.U. Theoretical Calculation of Substituent Effects on the O−H Bond Strength of Phenolic Antioxidants Related to Vitamin E. J. Am. Chem. Soc. 1997, 119, 4245–4252. [Google Scholar] [CrossRef]
- Mikulski, D.; Molski, M. A quantum chemical study on the antioxidant activity of bioactive polyphenols from peanut (Arachis hypogaea) and the major metabolites of trans-resveratrol. Comput. Theor. Chem. 2012, 981, 38–46. [Google Scholar] [CrossRef]
- Iuga, C.; Álvarez-Idaboy, J.R.; Russo, N. Antioxidant Activity of trans-Resveratrol toward Hydroxyl and Hydroperoxyl Radicals: A Quantum Chemical and Computational Kinetics Study. J. Org. Chem. 2012, 77, 3868–3877. [Google Scholar] [CrossRef] [PubMed]
- Sies, H. Oxidative stress: A concept in redox biology and medicine. Redox Biol. 2015, 4, 180–183. [Google Scholar] [CrossRef] [PubMed]
- Hoey, B.M.; Butler, J. The repair of oxidized amino acids by antioxidants. Biochim. Biophys. Acta 1984, 791, 212–218. [Google Scholar] [PubMed]
- Domazou, A.S.; Gebicki, J.M.; Nauser, T.; Koppenol, W.H. Repair of Protein Radicals by Antioxidants. Isr. J. Chem. 2014, 54, 254–264. [Google Scholar] [CrossRef]
- Brigati, G.; Lucarini, M.; Mugnaini, V.; Pedulli, G.F. Determination of the Substituent Effect on the O−H Bond Dissociation Enthalpies of Phenolic Antioxidants by the EPR Radical Equilibration Technique. J. Org. Chem. 2002, 67, 4828–4832. [Google Scholar] [CrossRef]
- Boulmokh, Y.; Belguidoum, K.; Meddour, F.; Amira-Guebailia, H. Investigation of antioxidant activity of epigallocatechin gallate and epicatechin as compared to resveratrol and ascorbic acid: Experimental and theoretical insights. Struct. Chem. 2021, 32, 1907–1923. [Google Scholar] [CrossRef]
- Cordova-Gomez, M.; Galano, A.; Alvarez-Idaboy, J.R. Piceatannol, a better peroxyl radical scavenger than resveratrol. RSC Adv. 2013, 3, 20209–20218. [Google Scholar] [CrossRef]
- Castro-Gonzalez, L.M.; Galano, A.; Alvarez-Idaboy, J.R. Free radical scavenging activity of newly designed sesamol derivatives. New J. Chem. 2021, 45, 11960–11967. [Google Scholar] [CrossRef]
- Pérez-González, A.; García-Hernández, E.; Chigo-Anota, E. The antioxidant capacity of an imidazole alkaloids family through single-electron transfer reactions. J. Mol. Mod. 2020, 26, 321. [Google Scholar] [CrossRef]
- Richards, J.T.; Adams, F.A. Study of reaction rates of the antioxidants gallic acid, BHT and BHA using the technique of pulse radiolysis. Int. J. Food Sci. 1987, 22, 501–508. [Google Scholar] [CrossRef]

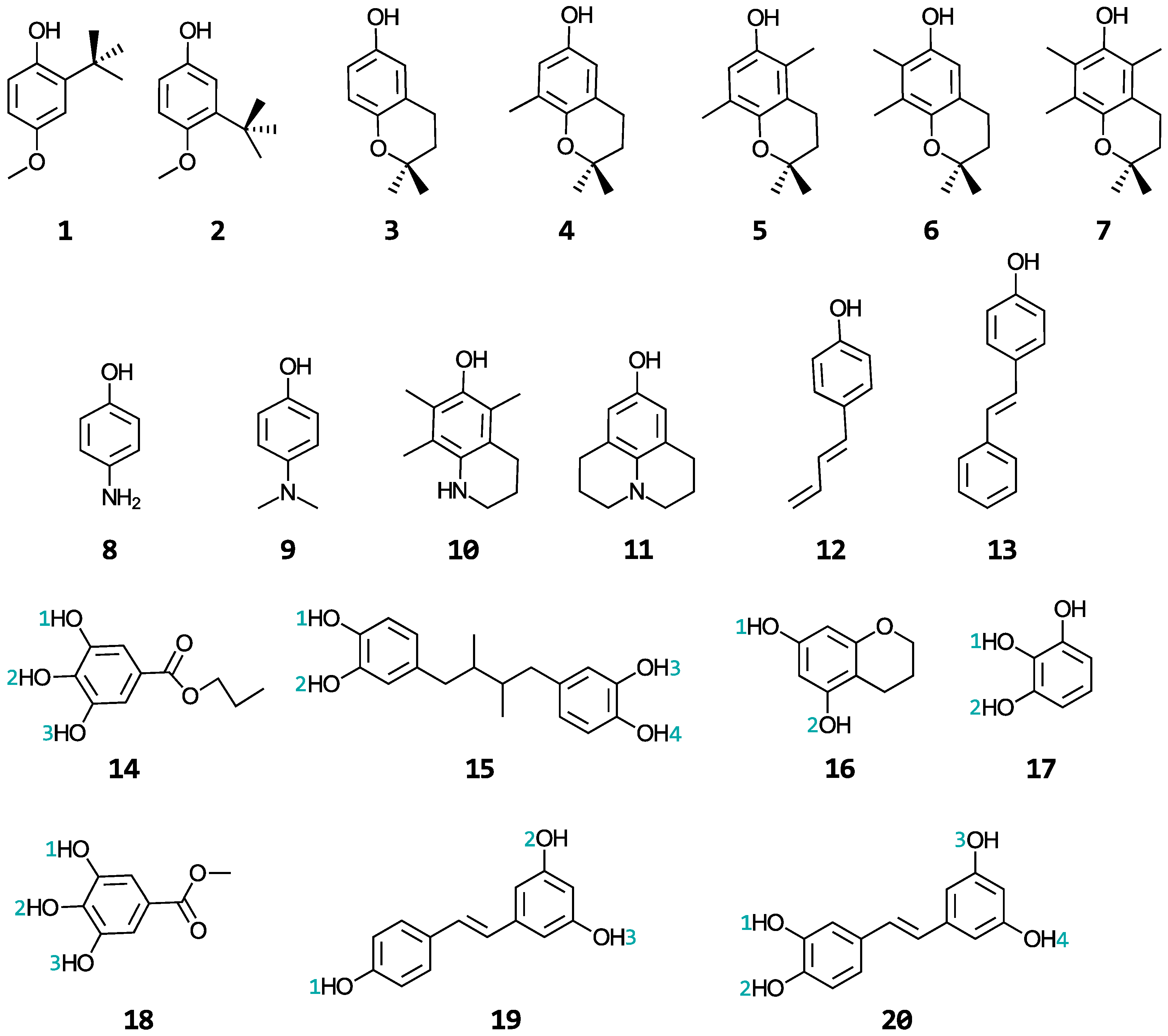

| ∆G° (kcal/mol) | ∆G≠ (kcal/mol) | k (M−1s−1) | ∆BDE c | |||||
|---|---|---|---|---|---|---|---|---|
| Reactants | Water | PE | Water | PE | Water | PE | Overall (PE) | Gas |
| Leucine-β-dmg + 1 | −19.6 | −20.7 | 10.9 | 10.4 | 4.57 × 105 | 3.04 × 105 | −8.9 | |
| Leucine-γ-dmg + 1 | −14.6 | −15.9 | 14.2 | 13.7 | 9.32 × 103 | 1.59 × 104 | ||
| Leucine-δ-dmg + 1 | −20.4 | −21.9 | 11.3 | 8.2 | 2.29 × 105 | 1.22 × 107 | ||
| Leucine-β-dmg + 2 | −17.4 | −18.0 | 15.5 | 13.0 | 5.74 × 103 | 3.00 × 104 | −6.1 | |
| Leucine-γ-dmg + 2 | −12.4 | −13.2 | 14.5 | 14.5 | 1.19 × 104 | 1.01 × 104 | ||
| Leucine-δ-dmg + 2 | −18.3 | −19.2 | 12.7 | 11.8 | 1.86 × 105 | 3.69 × 105 | ||
| Leucine-β-dmg + 3 | −17.6 | −18.3 | 14.8 | 10.5 | 9.86 × 103 | 1.27 × 106 | −7.2 | |
| Leucine-γ-dmg + 3 | −12.6 | −13.5 | 15.1 | 14.1 | 4.80 × 103 | 1.64 × 104 | ||
| Leucine-δ-dmg + 3 | −18.5 | −19.5 | 12.9 | 11.8 | 1.31 × 105 | 4.40 × 105 | ||
| Leucine-β-dmg + 4 | −18.4 | −18.7 | 11.4 | 11.6 | 4.98 × 105 | 3.25 × 105 | −7.3 | |
| Leucine-γ-dmg + 4 | −13.4 | −13.9 | 15.2 | 13.6 | 4.46 × 103 | 3.80 × 104 | ||
| Leucine-δ-dmg + 4 | −19.3 | −19.9 | 12.9 | 11.6 | 1.28 × 105 | 6.14 × 105 | ||
| Leucine-β-dmg + 5 | −20.0 | −20.3 | 10.7 | 10.6 | 5.66 × 105 | 3.53 × 105 | −9.4 | |
| Leucine-γ-dmg + 5 | −15.0 | −15.5 | 13.5 | 13.2 | 4.67 × 104 | 5.63 × 104 | ||
| Leucine-δ-dmg + 5 | −20.9 | −21.4 | 12.3 | 11.1 | 7.85 × 104 | 4.98 × 105 | ||
| Leucine-β-dmg + 6 | −19.9 | −20.1 | 11.2 | 11.0 | 3.34 × 105 | 2.75 × 105 | −8.9 | |
| Leucine-γ-dmg + 6 | −14.9 | −15.3 | 13.9 | 12.8 | 2.37 × 104 | 1.21 × 105 | ||
| Leucine-δ-dmg + 6 | −20.8 | −21.3 | 12.7 | 11.1 | 1.36 × 105 | 1.00 × 106 | ||
| Leucine-β-dmg + 7 | −22.3 | −22.4 | 9.0 | 8.5 | 3.18 × 106 | 6.86 × 106 | −11.3 | |
| Leucine-γ-dmg + 7 | −17.3 | −17.5 | 13.0 | 12.3 | 5.43 × 104 | 1.57 × 105 | ||
| Leucine-δ-dmg + 7 | −23.2 | −23.5 | 11.3 | 8.0 | 4.60 × 105 | 1.66 × 107 | ||
| Leucine-β-dmg + 8 | −23.7 | −22.6 | 11.4 | 11.1 | 1.32 × 106 | 7.63 × 105 | −9.4 | |
| Leucine-γ-dmg + 8 | −18.7 | −17.7 | 13.8 | 12.9 | 3.86 × 104 | 9.97 × 104 | ||
| Leucine-δ-dmg + 8 | −24.5 | −23.7 | 12.5 | 9.9 | 1.84 × 105 | 6.78 × 106 | ||
| Leucine-β-dmg + 9 | −25.0 | −23.6 | 12.1 | 11.5 | 5.07 × 105 | 3.08 × 105 | −10.3 | |
| Leucine-γ-dmg + 9 | −20.0 | −18.7 | 13.0 | 11.9 | 1.40 × 105 | 5.16 × 105 | ||
| Leucine-δ-dmg + 9 | −25.9 | −24.7 | 12.2 | 9.2 | 3.69 × 105 | 1.45 × 107 | ||
| Leucine-β-dmg + 10 | −29.9 | −27.7 | 6.9 | 6.6 | 9.34 × 107 a | 1.38 × 108 a | −14.6 | |
| Leucine-γ-dmg + 10 | −24.9 | −22.9 | 9.8 | 11.3 | 1.93 × 106 | 2.34 × 105 | ||
| Leucine-δ-dmg + 10 | −30.7 | −28.9 | 9.3 | 6.7 | 1.92 × 106 | 1.23 × 108 a | ||
| Leucine-β-dmg + 11 | −27.5 | −26.6 | 10.0 | 9.6 | 2.30 × 106 | 1.21 × 106 | −11.8 | |
| Leucine-γ-dmg + 11 | −22.5 | −21.8 | 12.8 | 11.8 | 2.34 × 105 | 4.14 × 105 | ||
| Leucine-δ-dmg + 11 | −28.3 | −27.8 | 12.2 | 8.9 | 1.85 × 105 | 1.15 × 107 | ||
| Leucine-β-dmg + 12 | −15.7 | −16.1 | 14.3 | 13.0 | 3.18 × 104 | 7.01 × 104 | −7.7 | |
| Leucine-γ-dmg + 12 | −10.7 | −11.2 | 16.1 | 15.3 | 1.01 × 103 | 3.04 × 103 | ||
| Leucine-δ-dmg + 12 | −16.5 | −17.2 | 14.6 | 13.5 | 1.34 × 104 | 4.73 × 104 | ||
| Leucine-β-dmg + 13 | −14.5 | −15.6 | 15.8 | 13.0 | 2.96 × 103 | 6.87 × 104 | −8.5 | |
| Leucine-γ-dmg + 13 | −9.5 | −10.8 | 16.9 | 15.0 | 2.49 × 102 | 4.85 × 103 | ||
| Leucine-δ-dmg + 13 | −15.4 | −16.8 | 15.7 | 13.3 | 2.26 × 103 | 7.46 × 104 | ||
| Leucine-δ-dmg + 14 (1) | −15.9 | 8.9 | 3.94 × 106 | 1.12 × 107 | −11.2 | |||
| Leucine-δ-dmg + 14 (2) | −21.9 | 8.9 | 4.03 × 106 | |||||
| Leucine-δ-dmg + 14 (3) | −16.3 | 9.0 | 3.23 × 106 | |||||
| Leucine-δ-dmg + 15 (1) | −20.9 | 8.3 | 9.47 × 106 | 3.44 × 107 | −11.1 | |||
| Leucine-δ-dmg + 15 (2) | −22.4 | 8.1 | 1.37 ×107 | |||||
| Leucine-δ-dmg + 15 (3) | −23.6 | 8.6 | 6.48 × 106 | |||||
| Leucine-δ-dmg + 15 (4) | −20.9 | 8.8 | 4.74 × 106 | |||||
| Leucine-δ-dmg + 16 (1) | −14.0 | 10.9 | 1.38 × 105 | 2.39 × 105 | −2.3 −1.3 | |||
| Leucine-δ-dmg + 16 (2) | −13.6 | 11.3 | 1.01 × 105 | |||||
| Leucine-δ-dmg + 17 (1) | −23.9 | 7.9 | 2.10 × 107 | 2.14 × 107 | −14.1 | |||
| Leucine-δ-dmg + 17 (2) | −18.0 | 11.0 | 1.98 × 105 b | |||||
| Leucine-δ-dmg + 18 (1) | −16.4 | 8.8 | 4.69 × 106 | 1.34 × 107 | −11.6 | |||
| Leucine-δ-dmg + 18 (2) | −21.9 | 8.7 | 4.95 × 106 | |||||
| Leucine-δ-dmg + 18 (3) | −15.9 | 8.9 | 3.80 × 106 | |||||
| Leucine-δ-dmg + 19 (1) | −16.1 | 13.1 | 9.36 × 104 | 2.91 × 105 | −8.2 | |||
| Leucine-δ-dmg + 19 (2) | −11.5 | 11.8 | 6.52 × 104 | |||||
| Leucine-δ-dmg + 19 (3) | −11.7 | 11.3 | 1.33 × 105 | |||||
| Leucine-δ-dmg + 20 (1) | −19.8 | 9.7 | 1.05 × 106 | 2.49 × 106 | −15.6 | |||
| Leucine-δ-dmg + 20 (2) | −23.0 | 10.2 | 1.26 × 106 | |||||
| Leucine-δ-dmg + 20 (3) | −11.6 | 11.7 | 3.20 × 104 | |||||
| Leucine-δ-dmg + 20 (4) | −12.3 | 11.3 | 1.52 × 105 | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Walton-Raaby, M.; Floen, T.; Mora-Diez, N. Modelling the Repair of Carbon-Centered Protein Radicals by Phenolic Antioxidants. Antioxidants 2024, 13, 1368. https://doi.org/10.3390/antiox13111368
Walton-Raaby M, Floen T, Mora-Diez N. Modelling the Repair of Carbon-Centered Protein Radicals by Phenolic Antioxidants. Antioxidants. 2024; 13(11):1368. https://doi.org/10.3390/antiox13111368
Chicago/Turabian StyleWalton-Raaby, Max, Tyler Floen, and Nelaine Mora-Diez. 2024. "Modelling the Repair of Carbon-Centered Protein Radicals by Phenolic Antioxidants" Antioxidants 13, no. 11: 1368. https://doi.org/10.3390/antiox13111368
APA StyleWalton-Raaby, M., Floen, T., & Mora-Diez, N. (2024). Modelling the Repair of Carbon-Centered Protein Radicals by Phenolic Antioxidants. Antioxidants, 13(11), 1368. https://doi.org/10.3390/antiox13111368









