Performance of Hypersaline Brine Desalination Using Spiral Wound Membrane: A Parametric Study
Abstract
1. Introduction
2. Methodology
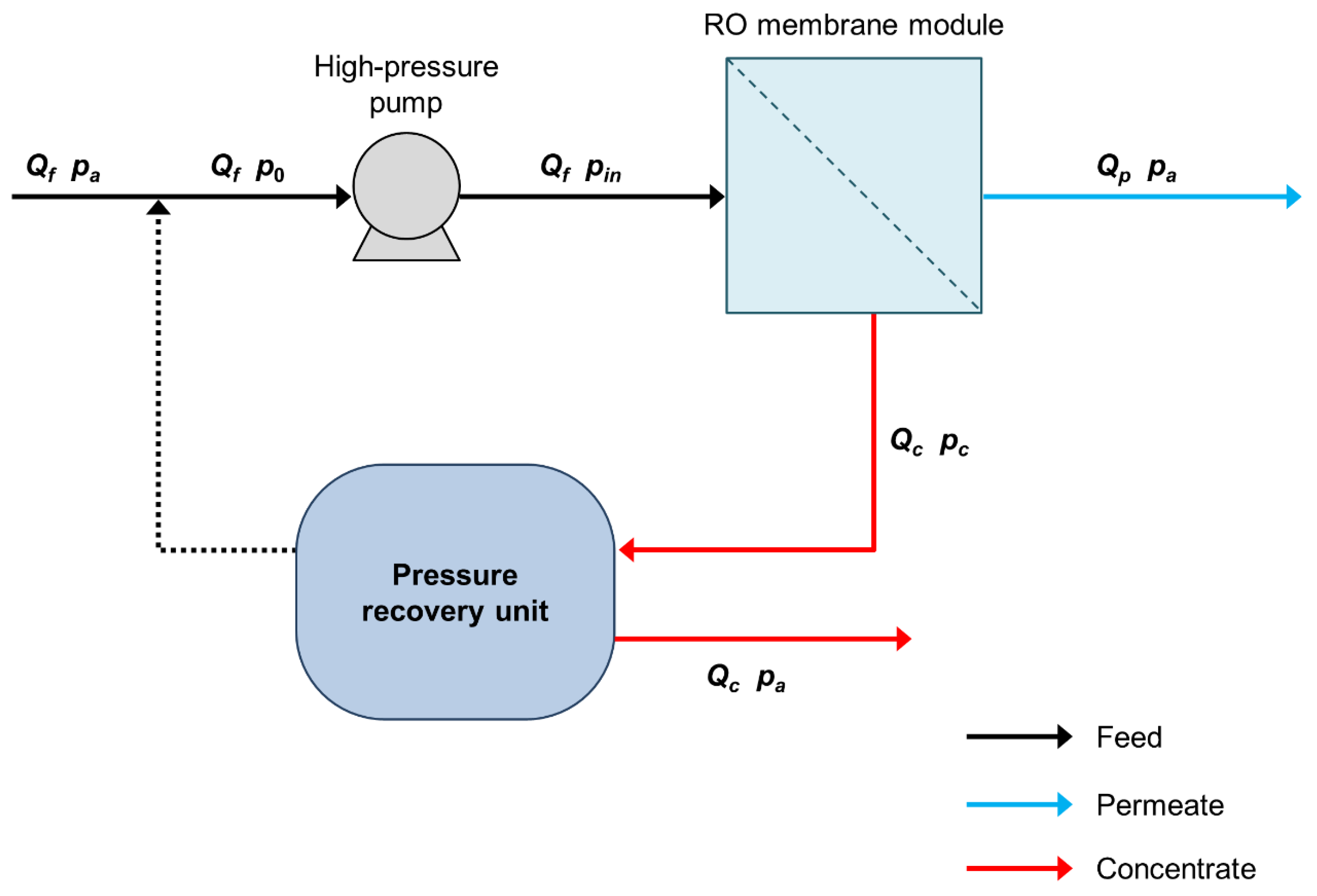
2.1. Module-Scale Analysis
2.2. Analysis of Results
2.3. Comparison between HBRO and SWRO
3. Results and Discussion
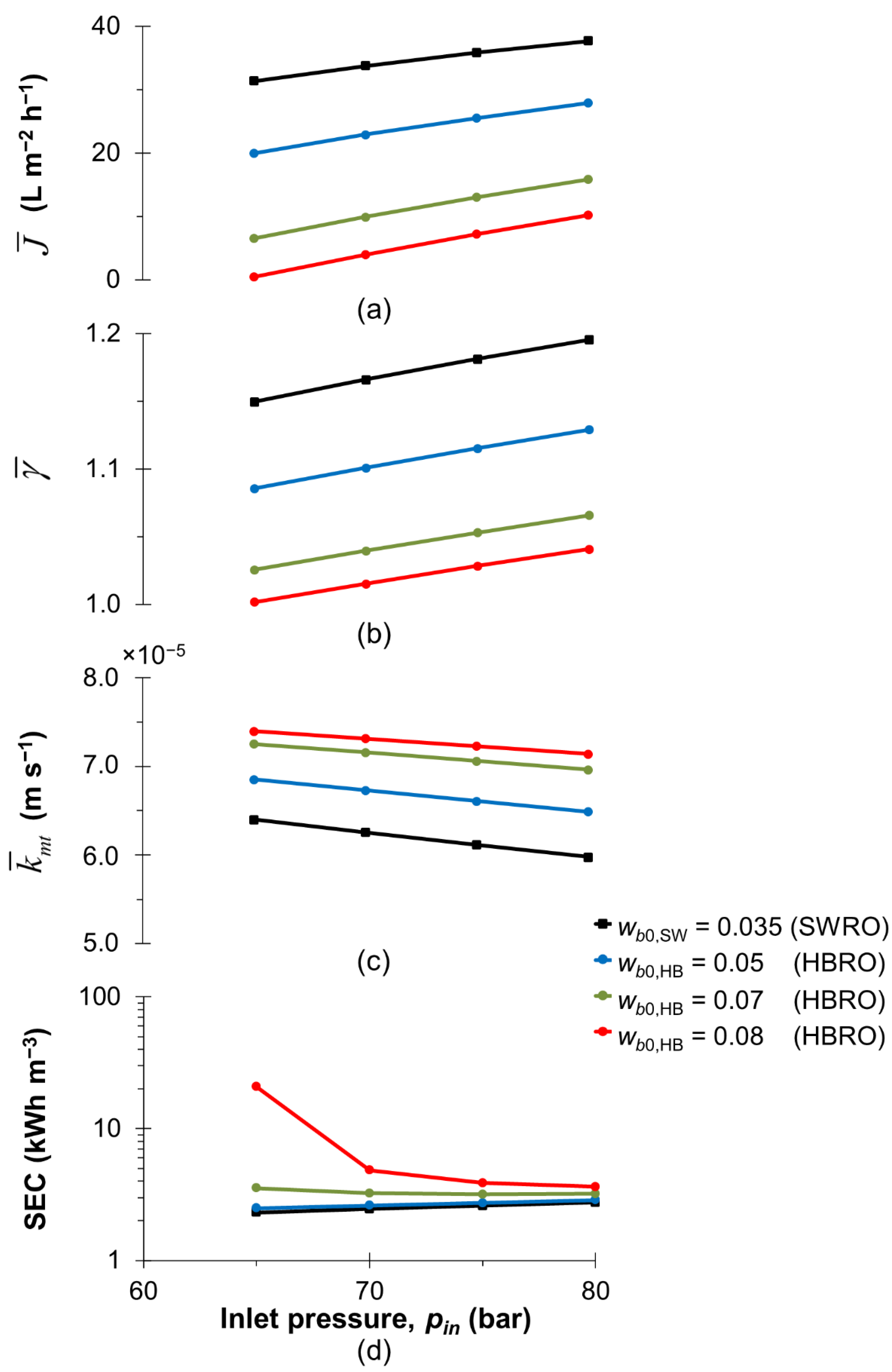
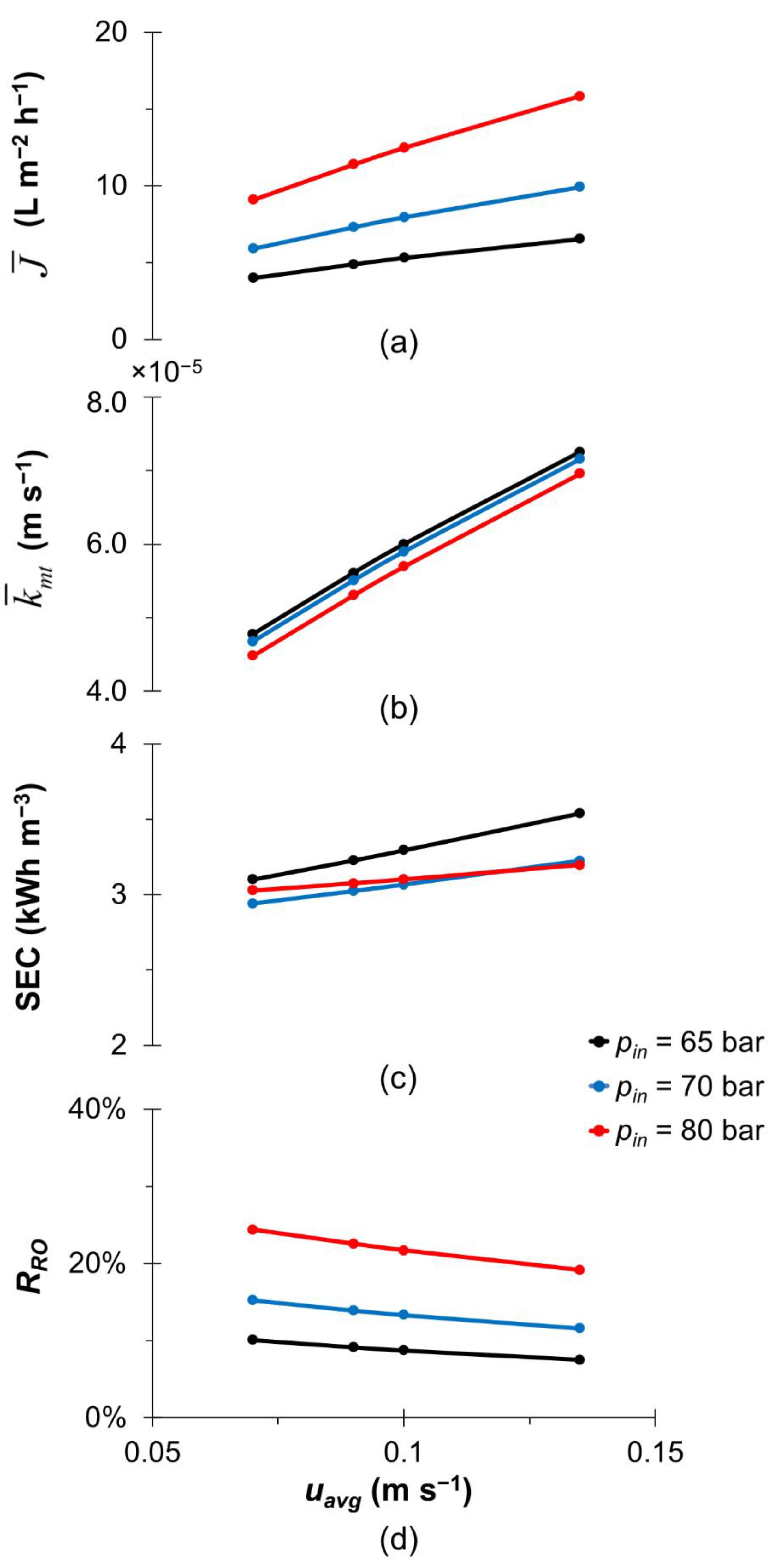
3.1. Effect of the Feed Conditions on HBRO Performance
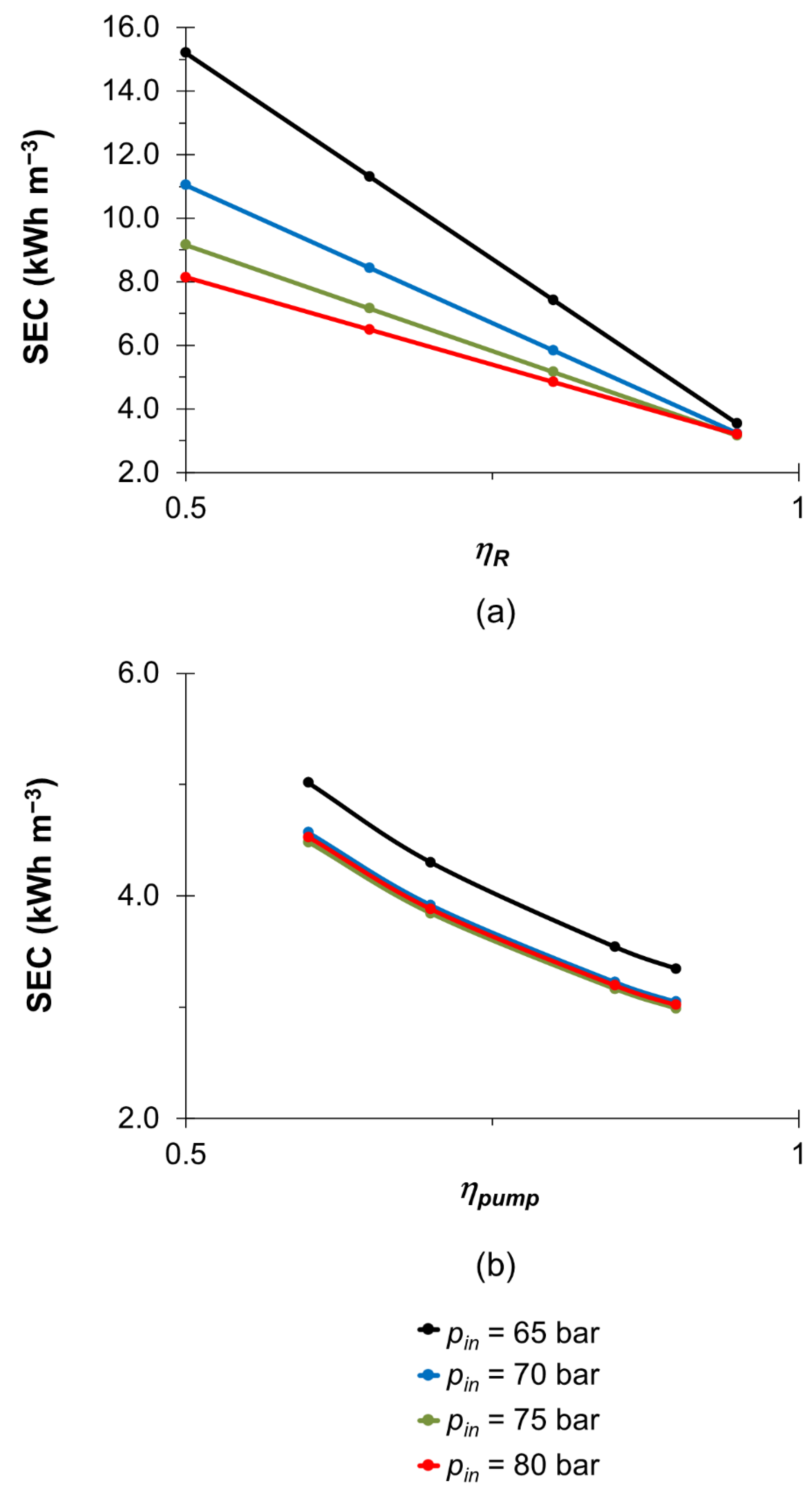
3.2. Effect of Retentate Pressure Recovery and Pump Efficiency
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbols | |
| Am | Membrane area (m2) |
| D | Diffusion coefficient (m2 s−1) |
| dh | Hydraulic diameter (m) |
| Fanning friction factor | |
| hch | Membrane channel height (m) |
| J | Mass flux (kg m−2 s−1, L m−2 h−1) |
| kmt | Mass transfer coefficient (m s−1) |
| Lm | Membrane length (m) |
| Lp | Membrane permeance (m Pa−1 s−1) |
| N | Number of envelopes |
| p | Pressure (Pa, bar) |
| Transmembrane pressure (bar) | |
| Q | Volumetric flow rate (m3 s−1) |
| Membrane intrinsic rejection | |
| Hydraulic Reynolds number | |
| RRO | Recovery ratio |
| Schmidt number | |
| Sherwood number | |
| t | Time (s) |
| uavg | Inlet velocity (m s−1) |
| Effective velocity (m s−1) | |
| Velocity vector (m s−1) | |
| w | Solute mass fraction |
| x | Distance in the bulk flow direction, parallel to the membrane surface (m) |
| y | Distance from the bottom membrane surface, in the direction normal to the surface (m) |
| Greek letters | |
| δch | Channel width (m) |
| ε | Porosity |
| Concentration polarisation modulus | |
| μ | Dynamic viscosity (kg m−1 s−1) |
| ηpump | Pump efficiency |
| Retentate pressure recovery efficiency | |
| π | Osmotic pressure (Pa) |
| Inlet osmotic pressure (Pa) | |
| φ | Osmotic pressure coefficient (Pa) |
| ψ | Ratio of volumetric flux to the impermeable mass transfer coefficient |
| ρ | Density (kg m−3) |
| σ | Reflection coefficient |
| Subscripts | |
| b | Value at the inlet bulk conditions |
| f | Value for the feed |
| HB | Value for hypersaline brine |
| imp | Value for the impermeable wall condition |
| in | Value for the inlet |
| out | Value at the domain outlet |
| p | Value for the permeate |
| per | Value for the permeable wall condition |
| r | Value for the retentate/concentrate |
| SW | Value for seawater |
| w | Value on the feed side membrane surface (wall) |
References
- Mavukkandy, M.O.; Chabib, C.M.; Mustafa, I.; Al Ghaferi, A.; AlMarzooqi, F. Brine management in desalination industry: From waste to resources generation. Desalination 2019, 472, 114187. [Google Scholar] [CrossRef]
- Brady, P.V.; Kottenstette, R.J.; Mayer, T.M.; Hightower, M.M. Inland desalination: Challenges and research needs. J. Contemp. Water Res. Educ. 2005, 132, 46–51. [Google Scholar] [CrossRef]
- Elimelech, M.; Phillip, W.A. The Future of Seawater Desalination: Energy, Technology, and the Environment. Science 2011, 333, 712–717. [Google Scholar] [CrossRef] [PubMed]
- Park, K.; Kim, J.; Hong, S. Brine management systems using membrane concentrators: Future directions for membrane development in desalination. Desalination 2022, 535, 115839. [Google Scholar] [CrossRef]
- Muhammad, Y.; Lee, W. Zero-liquid discharge (ZLD) technology for resource recovery from wastewater: A review. Sci. Total Environ. 2019, 681, 551–563. [Google Scholar] [CrossRef]
- Shah, K.M.; Billinge, I.H.; Chen, X.; Fan, H.; Huang, Y.; Winton, R.K.; Yip, N.Y. Drivers, challenges, and emerging technologies for desalination of high-salinity brines: A critical review. Desalination 2022, 538, 115827. [Google Scholar] [CrossRef]
- Pérez-González, A.; Urtiaga, A.M.; Ibáñez, R.; Ortiz, I. State of the art and review on the treatment technologies of water reverse osmosis concentrates. Water Res. 2012, 46, 267–283. [Google Scholar] [CrossRef]
- Mauter, M.S.; Fiske, P.S. Desalination for a circular water economy. Energy Environ. Sci. 2020, 13, 3180–3184. [Google Scholar] [CrossRef]
- Bello, A.S.; Zouari, N.; Da’ana, D.A.; Hahladakis, J.N.; Al-Ghouti, M.A. An overview of brine management: Emerging desalination technologies, life cycle assessment, and metal recovery methodologies. J. Environ. Manag. 2021, 288, 112358. [Google Scholar] [CrossRef]
- Panagopoulos, A.; Haralambous, K.-J.; Loizidou, M. Desalination brine disposal methods and treatment technologies—A review. Sci. Total Environ. 2019, 693, 133545. [Google Scholar] [CrossRef]
- Shatat, M.; Riffat, S.B. Water desalination technologies utilizing conventional and renewable energy sources. Int. J. Low-Carbon Technol. 2014, 9, 1–19. [Google Scholar] [CrossRef]
- Davenport, D.M.; Deshmukh, A.; Werber, J.R.; Elimelech, M. High-Pressure Reverse Osmosis for Energy-Efficient Hypersaline Brine Desalination: Current Status, Design Considerations, and Research Needs. Environ. Sci. Technol. Lett. 2018, 5, 467–475. [Google Scholar] [CrossRef]
- Al-Obaidi, M.A.; Rasn, K.H.; Aladwani, S.H.; Kadhom, M.; Mujtaba, I.M. Flexible design and operation of multi-stage reverse osmosis desalination process for producing different grades of water with maintenance and cleaning opportunity. Chem. Eng. Res. Des. 2022, 182, 525–543. [Google Scholar] [CrossRef]
- Nakagawa, K.; Togo, N.; Takagi, R.; Shintani, T.; Yoshioka, T.; Kamio, E.; Matsuyama, H. Multistage osmotically assisted reverse osmosis process for concentrating solutions using hollow fiber membrane modules. Chem. Eng. Res. Des. 2020, 162, 117–124. [Google Scholar] [CrossRef]
- Goosen, M.F.A.; Sablani, S.S.; Al-Maskari, S.S.; Al-Belushi, R.H.; Wilf, M. Effect of feed temperature on permeate flux and mass transfer coefficient in spiral-wound reverse osmosis systems. Desalination 2002, 144, 367–372. [Google Scholar] [CrossRef]
- Koutsou, C.P.; Kritikos, E.; Karabelas, A.J.; Kostoglou, M. Analysis of temperature effects on the specific energy consumption in reverse osmosis desalination processes. Desalination 2020, 476, 114213. [Google Scholar] [CrossRef]
- Toh, K.Y.; Liang, Y.Y.; Lau, W.J.; Fletcher, D.F. CFD study of the effect of perforated spacer on pressure loss and mass transfer in spacer-filled membrane channels. Chem. Eng. Sci. 2020, 222, 115704. [Google Scholar] [CrossRef]
- Kamal, A.M.; El-Sayed, T.A.; El-Butch, A.M.A.; Farghaly, S.H. Analytical and finite element modeling of pressure vessels for seawater reverse osmosis desalination plants. Desalination 2016, 397, 126–139. [Google Scholar] [CrossRef]
- Fritzmann, C.; Löwenberg, J.; Wintgens, T.; Melin, T. State-of-the-art of reverse osmosis desalination. Desalination 2007, 216, 1–76. [Google Scholar] [CrossRef]
- Xu, Y.; Peng, X.; Tang, C.Y.; Fu, Q.S.; Nie, S. Effect of draw solution concentration and operating conditions on forward osmosis and pressure retarded osmosis performance in a spiral wound module. J. Membr. Sci. 2010, 348, 298–309. [Google Scholar] [CrossRef]
- Salim, W.; Vakharia, V.; Chen, Y.; Wu, D.; Han, Y.; Ho, W.S.W. Fabrication and field testing of spiral-wound membrane modules for CO2 capture from flue gas. J. Membr. Sci. 2018, 556, 126–137. [Google Scholar] [CrossRef]
- Chen, K.K.; Salim, W.; Han, Y.; Wu, D.; Ho, W.S.W. Fabrication and scale-up of multi-leaf spiral-wound membrane modules for CO2 capture from flue gas. J. Membr. Sci. 2020, 595, 117504. [Google Scholar] [CrossRef]
- Scholes, C.A.; Motuzas, J.; Smart, S.; Kentish, S.E. Membrane Adhesives. Ind. Eng. Chem. Res. 2014, 53, 9523–9533. [Google Scholar] [CrossRef]
- Liu, M.; Yu, S.; Qi, M.; Pan, Q.; Gao, C. Impact of manufacture technique on seawater desalination performance of thin-film composite polyamide-urethane reverse osmosis membranes and their spiral wound elements. J. Membr. Sci. 2010, 348, 268–276. [Google Scholar] [CrossRef]
- Li, D.; Yan, Y.; Wang, H. Recent advances in polymer and polymer composite membranes for reverse and forward osmosis processes. Prog. Polym. Sci. 2016, 61, 104–155. [Google Scholar] [CrossRef]
- Lin, W.; Lei, J.; Wang, Q.; Wang, X.-M.; Huang, X. Performance enhancement of spiral-wound reverse osmosis membrane elements with novel diagonal-flow feed channels. Desalination 2022, 523, 115447. [Google Scholar] [CrossRef]
- Lin, W.; Zhang, Y.; Li, D.; Wang, X.-M.; Huang, X. Roles and performance enhancement of feed spacer in spiral wound membrane modules for water treatment: A 20-year review on research evolvement. Water Res. 2021, 198, 117146. [Google Scholar] [CrossRef]
- Zhang, Z.; Wu, Y.; Luo, L.; Li, G.; Li, Y.; Hu, H. Application of disk tube reverse osmosis in wastewater treatment: A review. Sci. Total Environ. 2021, 792, 148291. [Google Scholar] [CrossRef]
- Panagopoulos, A. Process simulation and analysis of high-pressure reverse osmosis (HPRO) in the treatment and utilization of desalination brine (saline wastewater). Int. J. Energy Res. 2022, 46, 23083–23094. [Google Scholar] [CrossRef]
- Davenport, D.M.; Wang, L.; Shalusky, E.; Elimelech, M. Design principles and challenges of bench-scale high-pressure reverse osmosis up to 150 bar. Desalination 2021, 517, 115237. [Google Scholar] [CrossRef]
- Qiu, T.Y.; Davies, P.A. Concentration polarization model of spiral-wound membrane modules with application to batch-mode RO desalination of brackish water. Desalination 2015, 368, 36–47. [Google Scholar] [CrossRef]
- Toh, K.Y.; Liang, Y.Y.; Lau, W.J.; Weihs, G.A.F. The techno-economic case for coupling advanced spacers to high-permeance RO membranes for desalination. Desalination 2020, 491, 114534. [Google Scholar] [CrossRef]
- Ali, Z.; Al Sunbul, Y.; Pacheco, F.; Ogieglo, W.; Wang, Y.; Genduso, G.; Pinnau, I. Defect-free highly selective polyamide thin-film composite membranes for desalination and boron removal. J. Membr. Sci. 2019, 578, 85–94. [Google Scholar] [CrossRef]
- Li, Y.; Qi, S.; Tian, M.; Widjajanti, W.; Wang, R. Fabrication of aquaporin-based biomimetic membrane for seawater desalination. Desalination 2019, 467, 103–112. [Google Scholar] [CrossRef]
- Liu, L.; Xie, X.; Qi, S.; Li, R.; Zhang, X.; Song, X.; Gao, C. Thin film nanocomposite reverse osmosis membrane incorporated with UiO-66 nanoparticles for enhanced boron removal. J. Membr. Sci. 2019, 580, 101–109. [Google Scholar] [CrossRef]
- Goh, P.S.; Liang, Y.Y.; Ismail, A.F. Energy Efficient Seawater Desalination: Strategies and Opportunities. Energy Technol. 2021, 9, 2100008. [Google Scholar] [CrossRef]
- Liang, Y.Y.; Toh, K.Y.; Fimbres-Weihs, G.A. 3D CFD study of the effect of multi-layer spacers on membrane performance under steady flow. J. Membr. Sci. 2019, 580, 256–267. [Google Scholar] [CrossRef]
- Guillen, G.; Hoek, E.M.V. Modeling the impacts of feed spacer geometry on reverse osmosis and nanofiltration processes. Chem. Eng. J. 2009, 149, 221–231. [Google Scholar] [CrossRef]
- Kedem, O.; Katchalsky, A. Thermodynamic analysis of the permeability of biological membranes to non-electrolytes. Biochim. Biophys. Acta 1958, 27, 229–246. [Google Scholar] [CrossRef]
- Fimbres-Weihs, G.A.; Wiley, D.E. Review of 3D CFD modeling of flow and mass transfer in narrow spacer-filled channels in membrane modules. Chem. Eng. Process. Process Intensif. 2010, 49, 759–781. [Google Scholar] [CrossRef]
- Geraldes, V.; Afonso, M.D. Generalized mass-transfer correction factor for nanofiltration and reverse osmosis. AIChE J. 2006, 52, 3353–3362. [Google Scholar] [CrossRef]
- Werber, J.R.; Deshmukh, A.; Elimelech, M. The Critical Need for Increased Selectivity, Not Increased Water Permeability, for Desalination Membranes. Environ. Sci. Technol. Lett. 2016, 3, 112–120. [Google Scholar] [CrossRef]
- Fimbres-Weihs, G.A.; Wiley, D.E. Numerical study of two-dimensional multi-layer spacer designs for minimum drag and maximum mass transfer. J. Membr. Sci. 2008, 325, 809–822. [Google Scholar] [CrossRef]
- Cohen-Tanugi, D.; McGovern, R.K.; Dave, S.H.; Lienhard, J.H.; Grossman, J.C. Quantifying the potential of ultra-permeable membranes for water desalination. Energy Environ. Sci. 2014, 7, 1134–1141. [Google Scholar] [CrossRef]
- Shi, B.; Marchetti, P.; Peshev, D.; Zhang, S.; Livingston, A.G. Will ultra-high permeance membranes lead to ultra-efficient processes? Challenges for molecular separations in liquid systems. J. Membr. Sci. 2017, 525, 35–47. [Google Scholar] [CrossRef]
- McGovern, R.K.; Lienhard, V.J.H. On the asymptotic flux of ultrapermeable seawater reverse osmosis membranes due to concentration polarisation. J. Membr. Sci. 2016, 520, 560–565. [Google Scholar] [CrossRef]
- Baker, R.W. Membrane Technology and Applications; John Wiley & Sons Ltd.: Oxford, UK, 2004. [Google Scholar]
- Liang, Y.Y.; Fimbres-Weihs, G.A.; Fletcher, D.F. CFD study of the effect of unsteady slip velocity waveform on shear stress in membrane systems. Chem. Eng. Sci. 2018, 192, 16–24. [Google Scholar] [CrossRef]
- Goi, Y.K.; Liang, Y.Y.; Lau, W.J.; Fimbres Weihs, G.A. Analysis of the effect of advanced FO spacer on the specific energy consumption of hybrid RO desalination system. J. Membr. Sci. 2023, 668, 121247. [Google Scholar] [CrossRef]
- Lim, Y.J.; Ma, Y.; Chew, J.W.; Wang, R. Assessing the potential of highly permeable reverse osmosis membranes for desalination: Specific energy and footprint analysis. Desalination 2022, 533, 115771. [Google Scholar] [CrossRef]
- Ruiz-García, A.; de la Nuez Pestana, I. Feed Spacer Geometries and Permeability Coefficients. Effect on the Performance in BWRO Spriral-Wound Membrane Modules. Water 2019, 11, 152. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, Y.; Wang, T.; Zhang, B.; Ma, H. Design and Energy Consumption Analysis of Small Reverse Osmosis Seawater Desalination Equipment. Energies 2021, 14, 2275. [Google Scholar] [CrossRef]
- Shrivastava, A.; Rosenberg, S.; Peery, M. Energy efficiency breakdown of reverse osmosis and its implications on future innovation roadmap for desalination. Desalination 2015, 368, 181–192. [Google Scholar] [CrossRef]
- Reima, I.; Jae Min, H.; Kunio, K. Analyses of three-dimensional flow calculations in a driven cavity. Fluid Dyn. Res. 1990, 6, 91–102. [Google Scholar] [CrossRef]
- Goh, P.S.; Lau, W.J.; Othman, M.H.D.; Ismail, A.F. Membrane fouling in desalination and its mitigation strategies. Desalination 2018, 425, 130–155. [Google Scholar] [CrossRef]
- DOW, FILMTECTM Membranes: FILMTEC SW30-8040 Seawater Reverse Osmosis Element Product Information; The Dow Chemical Company: Midland, MI, USA, 2018.
- Aladwani, S.H.; Al-Obaidi, M.A.; Mujtaba, I.M. Performance of reverse osmosis based desalination process using spiral wound membrane: Sensitivity study of operating parameters under variable seawater conditions. Clean. Eng. Technol. 2021, 5, 100284. [Google Scholar] [CrossRef]
- Voutchkov, N. Energy use for membrane seawater desalination—Current status and trends. Desalination 2018, 431, 2–14. [Google Scholar] [CrossRef]
- Kim, J.; Park, K.; Yang, D.R.; Hong, S. A comprehensive review of energy consumption of seawater reverse osmosis desalination plants. Appl. Energy 2019, 254, 113652. [Google Scholar] [CrossRef]
- Park, K.; Kim, J.; Yang, D.R.; Hong, S. Towards a low-energy seawater reverse osmosis desalination plant: A review and theoretical analysis for future directions. J. Membr. Sci. 2020, 595, 117607. [Google Scholar] [CrossRef]
- Wu, J.; Jin, Q.; Wang, Y.; Tandon, P. Theoretical analysis and auxiliary experiment of the optimization of energy recovery efficiency of a rotary energy recovery device. Desalination 2017, 415, 1–7. [Google Scholar] [CrossRef]
| Case Parameters | SWRO | HBRO |
|---|---|---|
| Feed mass fraction, wb0 | 0.035 | 0.05–0.08 |
| Inlet pressure, pin (bar) | 65–80 | |
| Inlet velocity, uavg (m s−1) | 0.07–0.135 | |
| Parameters | Value |
|---|---|
| Membrane area of module, Am (m2) | 28 |
| Number of envelopes, N | 14 |
| Module length, Lm (m) | 1 |
| Number of module units per pressure vessel | 4 |
| Channel width of module, δch (m) | 1 |
| Channel height, hch (m) | 0.001 |
| Schmidt number, Sc | 600 |
| Membrane permeance, Lp (m Pa−1 s−1) | 6 × 10−12 |
| Reflection coefficient, σ | 1 |
| Osmotic pressure coefficient, φ (Pa) | 8.051 × 107 |
| Intrinsic rejection, R | 0.996 |
| Inlet velocity, uavg (m s−1) | 0.07–0.135 |
| Retentate pressure recovery efficiency, ηR | 0.95 |
| Pump efficiency, ηpump | 0.85 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Foo, K.; Liang, Y.Y.; Lau, W.J.; Khan, M.M.R.; Ahmad, A.L. Performance of Hypersaline Brine Desalination Using Spiral Wound Membrane: A Parametric Study. Membranes 2023, 13, 248. https://doi.org/10.3390/membranes13020248
Foo K, Liang YY, Lau WJ, Khan MMR, Ahmad AL. Performance of Hypersaline Brine Desalination Using Spiral Wound Membrane: A Parametric Study. Membranes. 2023; 13(2):248. https://doi.org/10.3390/membranes13020248
Chicago/Turabian StyleFoo, Kathleen, Yong Yeow Liang, Woei Jye Lau, Md Maksudur Rahman Khan, and Abdul Latif Ahmad. 2023. "Performance of Hypersaline Brine Desalination Using Spiral Wound Membrane: A Parametric Study" Membranes 13, no. 2: 248. https://doi.org/10.3390/membranes13020248
APA StyleFoo, K., Liang, Y. Y., Lau, W. J., Khan, M. M. R., & Ahmad, A. L. (2023). Performance of Hypersaline Brine Desalination Using Spiral Wound Membrane: A Parametric Study. Membranes, 13(2), 248. https://doi.org/10.3390/membranes13020248









