1. Introduction
Nanofiltration has the potential to be a highly effective separation method for the utilization of viscose-fiber production side streams. Furthermore, it can be used as a downstream process for the purification of process chemicals. A possible application is the removal of hemi celluloses and other wood degradation products from the highly caustic steeping lye. Hence, the lye could be recycled to the process and the demand of the make-up caustic solution can be significantly reduced. The total organic carbon (TOC) content can basically be divided into two fractions. The xylose oligo- and polymers with a molecular weight from ~600 to ~50,000 g mol−1 (beta fraction) and the hydroxyl acids, ranging from C2 up to C6 acids (gamma fraction).
The application of ceramic ultrafiltration membranes for the hemi cellulose removal (feed concentration: 30 g L
−1) from steeping lye containing 18% sodium hydroxide was tested by Saurabh [
1]. The used membranes had a molecular weight cut of (MWCO) of 3, 5, and 15 kDa. The membranes showed maximum retention of 0.68 (MWCO = 3 kDa), 0.67 (MWCO = 5 kDa) and less than 0.1 (MWCO = 15 kDa). Moreover, Saurabh [
2] published results for polymeric ultrafiltration membranes for a similar feed stream. The retention was about 0.7 for the membrane with 3000 Da MWCO and decreased to about 0.15 (MWCO = 20,000).
Schlesinger [
3] tested different polymeric nanofiltration membranes for an analog application. The membranes NTR-7470 (Nitto-Denko), MPF-34 (Koch), NP030 (Microdyn Nadir) and Osmonics GE (Desal) showed retention higher than 0.90 for molecules with a mass higher than 1000 g mol
−1. This is significantly higher compared to the ceramic ultrafiltration membranes however there is still a low retention for the gamma fraction. A more detailed study on the retention of different components has not been performed yet. Furthermore intense fouling was observed by Schlesinger [
4,
5].
In addition to the viscose-fiber production, membrane processes in strong alkaline solutions are under investigation for streams of the cotton industry as studied by Son [
6]. An additional application, which is under investigation, is the treatment of caustic cleaning agents used in the dairy industry [
7,
8,
9]. It has to be mentioned that the sodium hydroxide concentrations in these applications are much smaller than in the viscose-fiber industry.
There is much research work that describes the transport of small organic and inorganic molecules through nanofiltration membranes. Different research groups [
10,
11,
12,
13] published the influence of the pH value, the zeta potential and the hydrophobicity of the membrane as well as the charge of the molecule on the transport mechanism. Furthermore, Simon [
14] studied the impact of caustic cleaning on the membrane performance. All this research was done in pH ranges from 2 to 12, hence there is a lack of knowledge for higher pH values.
The influence of sodium hydroxide concentrations in the range of 1 up to 5 mol L−1 on the retention performance of nanofiltration membranes has not been studied so far. For several decades, highly alkaline stable nanofiltration membranes are available, although there are only few polymers available that can be used for such pH-robust membranes. One example is the membrane NP030 from Microdyn Nadir that is based on sulfonated polyethersulfone. Further, Advanced Membrane System Technologies supplies a highly alkaline stable nanofiltration membrane based on melamine polyamine.
Current research work is done to develop new membrane materials. Dalwani [
15] developed a sulfonated polyether ether ketone (SPEEK)-based membrane with very high pH stability. Daems [
16] developed a new technique to enhance the alkaline stability of PVDF membranes by grafting with polystyrene sulfonic acid.
Van Gestel [
17] produced multilayer ceramic membranes with a MWCO lower than 200 Da. This was achieved with α-Al
2O
3/γ-Al
2O
3/anatase membranes that are stable in the pH range from 3 to 11. At higher and lower pH values, the combination anatase on an α-Al
2O
3 performs better.
In the present work, succinic acid was chosen as the model compound to investigate the retention behavior of gamma components due to several reasons. It has a molecular weight of 118 g mol−1, which is in the middle between acetic acid (60 g mol−1), and glucoisosaccharinic acid (180 g mol−1), two representative gamma components. Furthermore, its anion can act as mono- or divalent ion depending on the pH value.
A better understanding of the NaOH concentration impact on the retention behavior is important for the design of an efficient nanofiltration process, as partial dilution can improve the caustic recovery rate. The comparison of disodium succinate and sodium sulfate should help to improve the knowledge of membrane retention mechanisms and could indicate the possibility of separation between organic and inorganic divalent ions.
2. Material and Methods
2.1. Material
Succinic acid with a purity of 99.9% and disodium succinate with a purity of 99% were obtained from Sigma Aldrich. Hydroxide solution was prepared from deionized water and dry pellets with a purity of 99% (Sigma Aldrich, Taufkirchen, Germany).
NP030 membrane samples from Microdyn Nadir (Wiesbaden, Germany) in DIN A4 format were purchased directly from the manufacturer and were used without any pretreatment. Membrane properties are shown in
Table 1.
2.2. Nanofiltration
Experiments were performed on two different lab-scale nanofiltration rigs. One was a modified OSMO-MC-01 flat-sheet lab-scale device from OSMOTA (Rutesheim, Germany), the other one was a MEMCELL 3 from OSMO (Korntal-Münchingen, Germany). A heat exchanger was added in the feed stream of both systems for temperature regulation. The membrane area was 80 cm2; for the OSMO-MC-01 and three times 80 cm2 in the MEMCELL 3 device. The cells were arranged in parallel. Permeate and retentate were returned to the feed tank to keep the feed concentration constant. The samples amount was kept low (~1 mL) compared to the feed volume (5 L). The feed concentration was measured throughout the experiment to ensure stable concentrations.
The refractive index of permeate was observed to identify if a quasi-stationary state was achieved. Samples of at least six different pressures were collected with each combination of parameters.
All experiments at a certain NaOH concentration were performed with the same membrane. Before the experiment, the membrane was rinsed with a NaOH solution until constant permeate flux (approx. 50–80 h). The NaOH concentration during this pretreatment was equal to the concentration in the following experiment.
2.3. Refractometer
Refractive index measurements were performed with the refractometer Abbemat 500 from Anton Paar (Graz, Austria). The refractometer was used on the one hand to check constant permeate composition and on the other hand to monitor constant feed composition.
Furthermore, the refractive index measurements were used to measure the NaOH concentration.
Calibration data were measured for different solutions of succinic acid (0–0.85 mol L
−1) in different NaOH concentrations (0–5 mol L
−1). The correlation of the refractive index (
n) based on NaOH concentration (
cNaOH in mol L
−1) and succinic acid concentration (
cSA in mol L
−1) is given by Equation (1).
where
A = 1.333350;
B = 0.010348;
C = 0.008236;
D = −0.001227;
E = −0.000337;
F = −0.000284.
Based on this correlation, the NaOH concentration can be calculated for a given succinic acid concentration.
2.4. Titration
To verify the calculated NaOH concentration (based on the refractive index), a batch of samples was additionally analyzed by titration with 0.25 mol L−1 sulfuric acid to pH 3.7.
To eliminate the influence of the titrant, preliminary tests were done with sulfuric acid and hydrochloric acid. No significant influence of the titrant was observed.
Furthermore, preliminary tests showed that succinic acid is completely undissociated at pH 3.7. This needs to be considered for the calculation of the sodium retention based on titration.
2.5. Chromatography
Succinic acid and disodium succinate were quantified by high performance liquid chromatography (HPLC) analysis. A Thermo Hypersil GOLD aQ 250 mm column with the photodiode array detector Dionex UVD 340U was used. A wavelength of 210 nm was applied for detection. Sulfate concentration was analyzed using ion chromatography. A CarboPac PA10 was used as a column. The detector for quantification was a Dionex ED 50. All the HPLC instruments used were purchased from Thermo Fisher Scientific (Vienna, Austria). The data processing was done with the software Chromeleon (version 7.2).
2.6. Scanning Electron Microscopy
SEM measurements were done for different membranes before and after the experiments. The used membrane samples were washed with deionized water and subsequently dried. The unused membrane sample was used as received from the manufacturer.
The samples were gold-plated prior to the analysis. Images were taken using a FEI Quanta 450 with 10 Kv Thermo Fisher Scientific (Vienna, Austria). For the image processing the software Phenom ProSuite (version 2.9.0.0) was used.
2.7. Attenuated Total Reflectance Infrared Spectroscopy
All analyzed membrane samples except the unused one were washed with deionized water for one minute after exposure to NaOH solution.
Measurements were done with a Bruker Tensor 27 Spectrometer, which was equipped with a Specac Single Reflection Diamond ATR “Golden Gate” (Bruker Austria GmbH, Vienna, Austria). The software OPUS (version 7.5). was used for data processing.
4. Results and Discussion
4.1. Saturation Solubility of Succinic Acid
The saturation solubility of succinic acid increases with increasing concentration of NaOH as shown in
Figure 1. The solubility in 5 M NaOH is four times higher than in water. Succinic acid in protonated form is less soluble than the salt form. An increasing NaOH concentration enhances the dissociation and therefore the solubility. Solubility at 40 °C is on average 0.24 mol L
−1 higher than at 30 °C. The solubility of disodium succinate in water is approximately twice as high as that of succinic acid. Thus, the succinic acid and disodium succinate concentrations used in the nanofiltration experiments were far below the solubility limits.
4.2. Flux
Figure 2 shows the permeate flux of water and NaOH solutions with different concentrations. As can be seen, the flux is linearly dependent on the applied pressure in the range of 5 to 30 bar.
Figure 3 shows the influence of the NaOH concentration on the permeability coefficient (
LP) at 40 °C. By increasing the NaOH concentration from 0 to 5 mol, L
−1 LP decreases from 2.05 to 0.15 kg m
−2 h
−1 bar
−1. The flux decrease by increasing NaOH concentration occurs for two different reasons. Firstly, the viscosity increases from 0.65 mPa·s (water) to 2.5 mPa·s (5 M NaOH). The second reason is the difference in osmotic pressure. The NP030 shows a small retention for NaOH. Consequently, an osmotic pressure difference occurs leading to a reduction of permeate flux. The higher the feed concentration, the higher is the osmotic pressure difference.
4.3. Retention of Sodium Sulfate
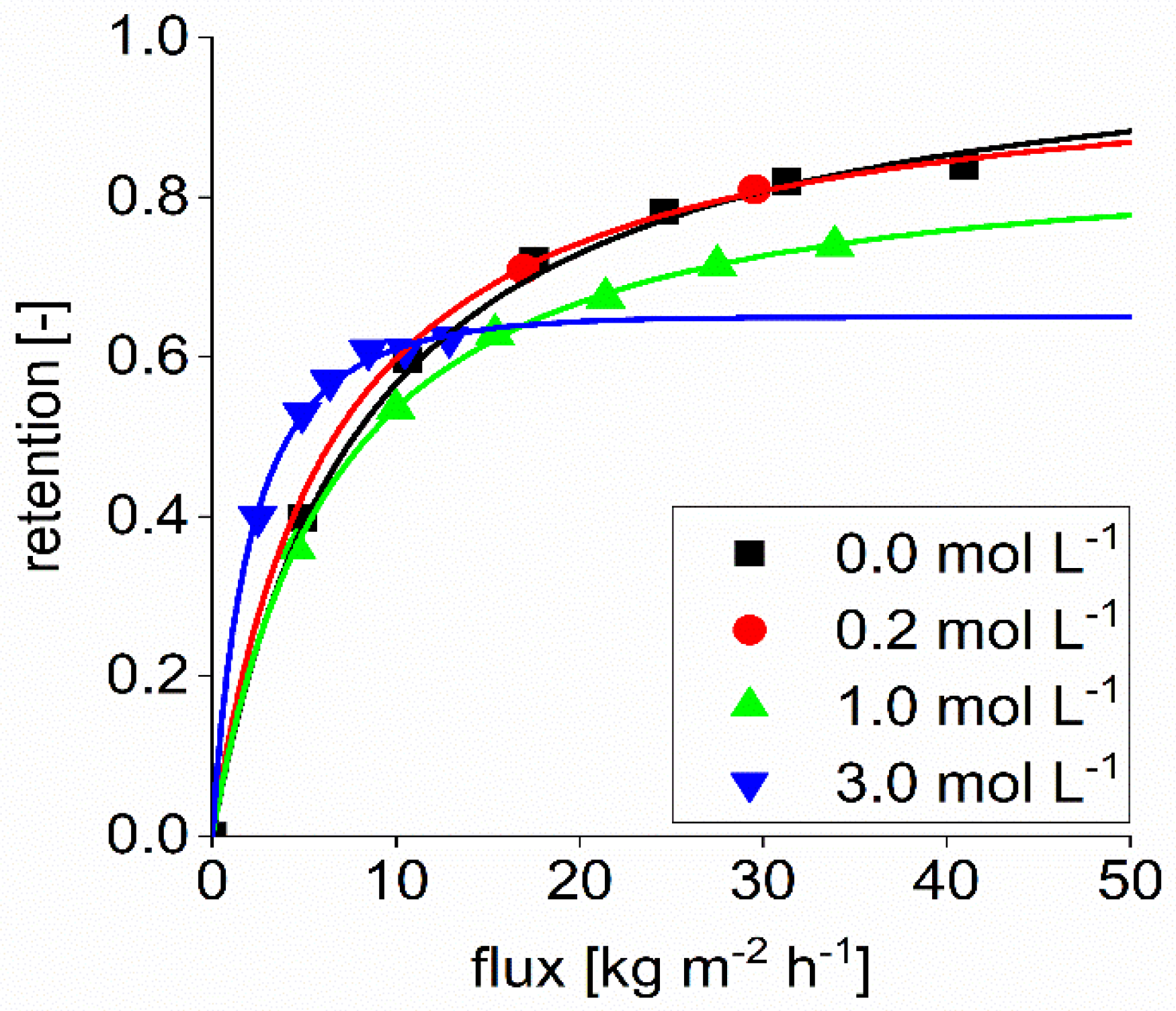
The retention value of sulfate, according to manufacturer data, is between 0.80 and 0.95. The experiments were performed with 40 bar at 20 °C, details on the feed concentration were not given. Sulfate retention was studied to compare the influence of the structure of two different divalent ions. The organic succinate ion has an oval structure with the negatively charged groups on both ends. In contrast, the sulfate ion is circular.
Figure 4 shows the retention of sulfate with a feed concentration of 0.1 mol L
−1 sodium sulfate. As can be seen in the performed experiments, the retention of sulfate in aqueous solution was lower than 0.8 for permeate fluxes lower than 30 kg m
−2 h
−1. At higher fluxes, the sulfate retention was in the range specified by the supplier. The reflection coefficient found in the Spiegler and Kedem model was calculated to be 0.9.
Furthermore, it can be seen that the sulfate retention is reduced by adding NaOH to the solution. The higher the NaOH concentration is, the lower the sulfate retention.
4.4. Retention of Succinic Acid
Figure 5 shows the retention at infinite permeate flux calculated with the Pusch model (
R∞) in dependency of the temperature, the feed concentration of succinic acid and the NaOH concentration.
Figure 6 shows the reflection coefficient (
σSA) of the Spiegler and Kedem model with the same parameters. The standard deviation for the Spiegler and Kedem fit is significantly smaller than for the Pusch Model. Consequently, the
R∞ values show a higher variance than the
σSA values. Hence, further calculations were performed only with the Spiegler and Kedem model.
As it is shown in
Figure 6, the reflection coefficient of succinic acid is independent from the NaOH concentration as long as the succinate ion is completely dissociated. The incompletely dissociated form shows significantly lower
σSA values. The
σSA values at 0.43 mol L
−1 succinic acid in 1 molar NaOH solution are lower compared to the data points at complete dissociation.
The experiments with 0.2 mol L−1 succinic acid were performed twice, once as a first experiment of a series and a second time at the end of a series. As shown, the results have a good reproducibility.
The succinate ion shows a similar retention in 0.1 molar aqueous disodium succinate solution (chapter 4.6.) as sulfate in a 0.1 molar sodium sulfate solution. The situation changes for the sulfate ion in a caustic solution. Its retention decreases with increasing NaOH concentration. In comparison, the succinate retention is unaffected from NaOH in a wide concentration range. A difference in the capability of hydration shell formation can explain this. It seems that the succinate ion is able to pull the water molecules stronger than the sulfate ion. Bouchoux [
23] pointed out, that the sodium lactate retention is almost unaffected by adding sodium chloride to the solution, in contrast glucose retention decreased significantly. This means organic anions like lactate and succinate are able to form hydration shells, which are less affected from competitive hydration of other ions in the solution. Hence, the type and concentration of co-ions in the solution can control the separation performance of ions. To prove this assumption, further investigations with molecules with different structures are necessary.
In the case of incomplete dissociation of the succinic acid, the retention decreases by about half. This is within the same range as the chloride retention specified by the manufacturer (0.25–0.35).
4.5. Retention of Sodium Ions
Figure 7 shows the reflection coefficient of sodium ions (
σNa). As mentioned in Equation (8) the retention of sodium ions is related to the retention of succinate ions by the law of electroneutrality, assuming that no further cations are present. In solutions containing excess NaOH with respect to the amount of carboxylic acid groups, this requirement is met. According to Equation (8) the slope of the data points for 1 M NaOH is five times steeper than for 5 M NaOH. The calculated slopes are 1.09 (1 M NaOH) and 0.22 (5 M NaOH). The decrease of the pH value leads to the presence of hydronium ions, which decouples the retention between the sodium and succinate ions. Thus, this point was not taken into account for the slope calculation.
Additionally, the retention of sodium ions was calculated with Equation (8). The results are given as crosses in
Figure 7. As can be seen, the experimental data and the calculated values are in good agreement.
4.6. Retention of Disodium Succinate
To investigate the retention of equimolar solutions of sodium ions and carboxylic groups, experiments with disodium succinate were performed.
Figure 8 shows the reflection coefficient in relation to feed concentrations. As can be seen, the retention for disodium succinate in water is higher than the retention of succinic acid in NaOH solutions. Furthermore, it can be seen, that the reflection coefficient decreases with increasing feed concentration.
4.7. SEM Measurements
Rinsing the membrane with water leads to a tripling of the active membrane layer thickness due to a swelling effect. Swelling in caustic solution leads to the same result (
Figure 9).
No changes of the active layer thickness can be seen by samples of the used membranes stressed up to 25 bar at 40 °C. The shape of the pores in the first support layer changes from an oval shape to an elongated shape. This does not influence the overall membrane performance.
By increasing the pressure up to 55 bar (40 °C), a significant change can be observed, see
Figure 10. The small pores of the first support layer disappear and a compact layer is formed. The compression of this support layer is irreversible and leads to a significantly lower permeate flux. The bigger pores in the bottom part of the first support layer are still present.
4.8. ATR–IR Measurement
Figure 11 shows ATR-IR measurements of different conditioned membranes.
Essentially, the spectra of all tested membranes resemble the spectrum of PES [Poly(p-phenylene ether sulfone)] which is declared as the main membrane polymer by the supplier.
The band at a wavelength from 3200–3500 cm−1 represents the O–H stretching band. In contact with NaOH solution, a change in the ATR-IR diagram can be observed but is not related to any changes in the membrane polymer composition. These changes appear within the first hour and do not alter significantly over time.
The bands at 2934 and 2879 cm
−1 represent aliphatic C-H stretching bonds which decrease in contact with NaOH solution. The bands at 1040 and 925 cm
−1 refer to glycerol, which was used for membrane preparation or storage and is washed out immediately by the solutions. The band at 1400 cm
−1 indicates decompositions of carbonates on the membrane. Carbonates were formed from carbon dioxide, which is dissolved in the solution. By longer exposure to the NaOH solution, the band increases slightly. None of the characteristic polymer bands changes significantly with the treatment time. Consequently, NP030 can be considered as chemically stable in alkaline solutions with concentrations up to 5 mol L
−1 NaOH. This is in good agreement with the data published by Schlesinger [
3], which indicates high alkali resistance of the NP030.
5. Conclusions
Based on the retention results of aqueous solutions, it can be concluded that the organic salt (disodium succinate) behaves similarly to the inorganic sodium sulfate. A different retention behavior is observed in NaOH solutions. Sulfate ions are more affected by the presence of co-ions than succinate ions. Degree of dissociation is the factor with the highest impact on the retention of succinic acid. Consequently, the charge selectivity of the NP030 nanofiltration membrane could be verified with an organic salt by changing its degree of dissociation. A higher NaOH concentration in the feed solution leads to a significant decrease of permeate flux.
The retention of completely dissociated succinate, is unaffected by the NaOH concentration. Hence, a dilution during the nanofiltration process may improve the process efficiency, as the flux increases. Furthermore, the selectivity between sulfate and succinate can be controlled by the NaOH concentration.
The results of the ATR–IR measurements and the thermomechanical stress tests indicate an applicability of the NP030 membrane in highly alkaline conditions. Further performance tests with model solutions are necessary to prove this.
Furthermore, it is shown, that the Spiegler and Kedem model appropriately describes the retention behavior of all investigated species, even in five molar NaOH.