Membrane Fouling Due to Protein—Polysaccharide Mixtures in Dead-End Ultrafiltration; the Effect of Permeation Flux on Fouling Resistance
Abstract
:1. Introduction
2. Theoretical Background
3. Materials and Methods
3.1. Organic Foulants-Feed Solutions
3.2. Analytical Methods
3.3. Membrane Type
3.4. Experimental Set-Up and Procedures
4. Results and Discussion
4.1. Temporal Variation of Pressure Drop Due to Fouling ΔPc
- (a)
- In all cases of different foulants composition, at low fluxes (J < 40 L/m2h), there is almost linear temporal variation of ΔPc which is indicative of nearly constant specific fouling resistance α. At greater fluxes, the concave shape is typical of fouling layer compressibility effects, already observed in previous studies [29].
- (b)
- The resistance to liquid permeation due to fouling Rc is high even at relatively low fluxes; this is also shown in Table S2, where values of intrinsic membrane resistance Rm are included for comparison. At the higher fluxes (J > ~40 L/m2h), fouling resistance Rc reaches high values, i.e., an order of magnitude greater than Rm within the time period of present tests.
- (c)
- Pure BSA, and rich in BSA, foulants (Figure 1b–d) particularly at low fluxes, exhibit initially (for a few minutes) a non-linear (convex) ΔPc increase. Figure 2, at greater resolution, better shows this trend, which is due to the unclear mechanism of incipient membrane fouling. One might hypothesize that there is a partial pore blocking and gradual membrane-surface coverage by the relatively compact BSA molecules and agglomerates. Beyond this initial period, a linear ΔPc variation (at the smaller fluxes) is a likely manifestation of a coherent fouling layer formation and further growth due to organic mass deposition. As the proportion of SA in the foulant mixture is increased, this trend disappears, the alginate gel matrix apparently dominates, and the ΔPc profiles exhibit linearity throughout the test period.
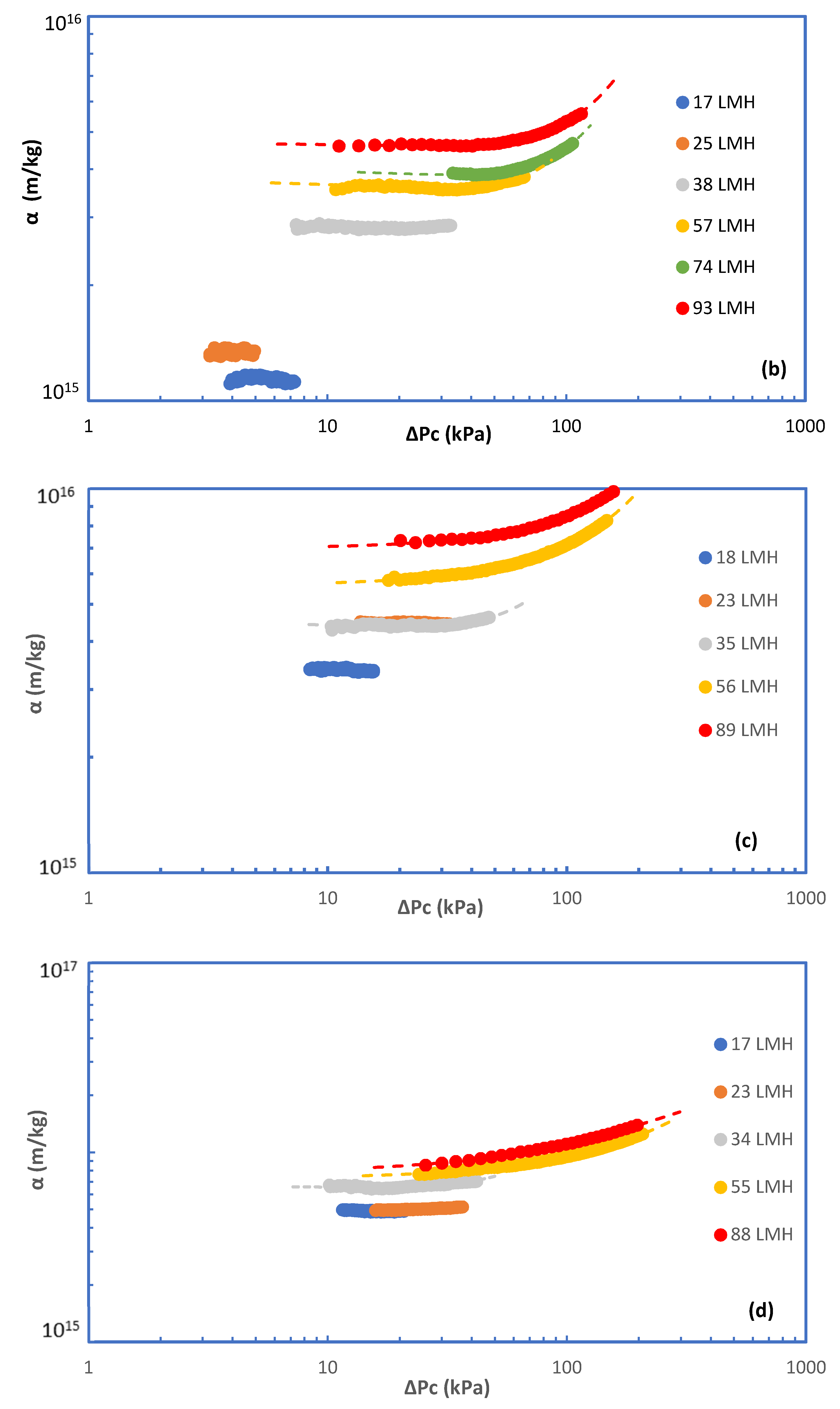
4.2. Characteristics of Specific Fouling Resistance α
4.3. Correlation of Fouling Resistance α Data-Comparison with Previous Studies
4.4. Organic Matter Rejection
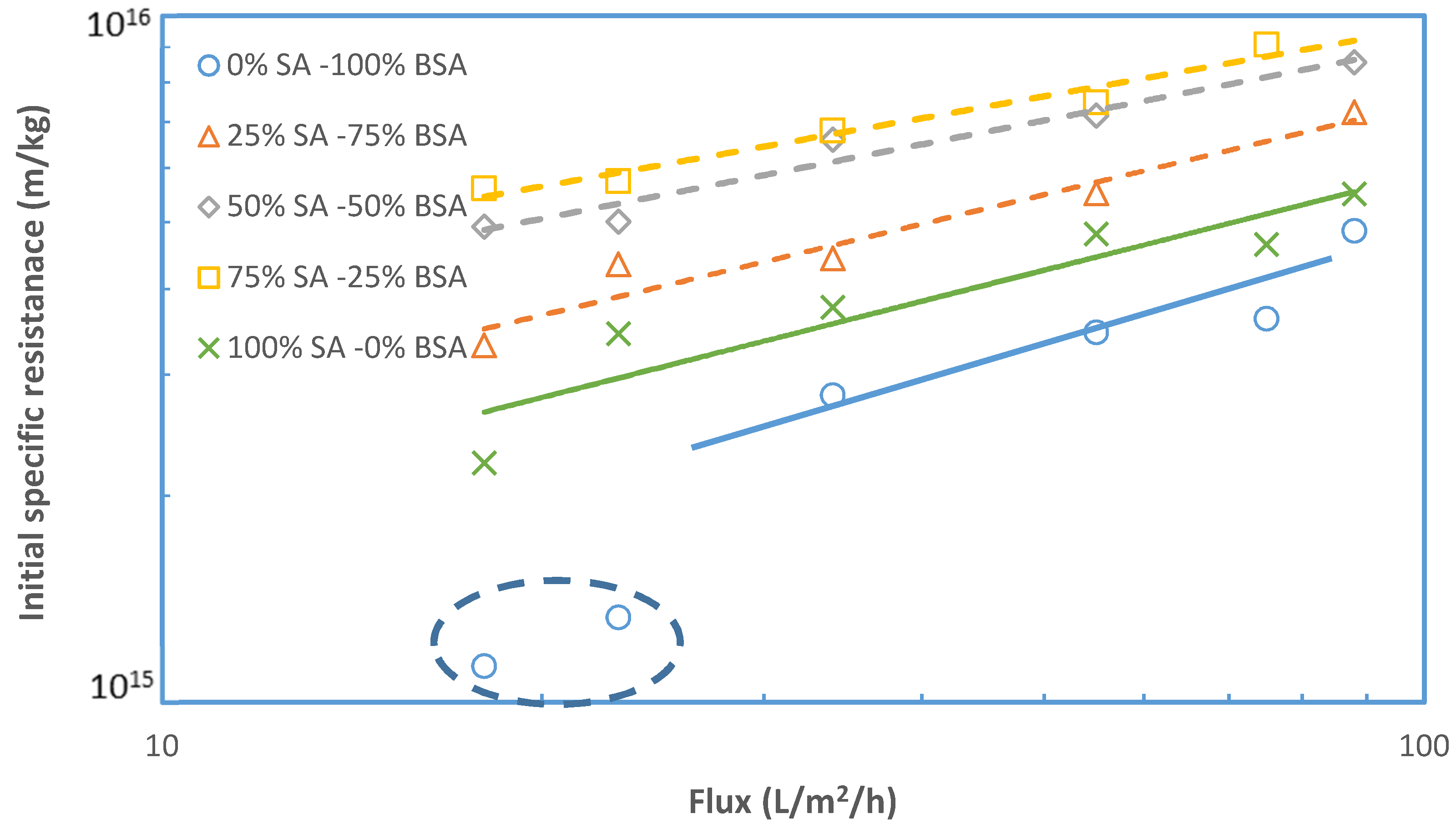
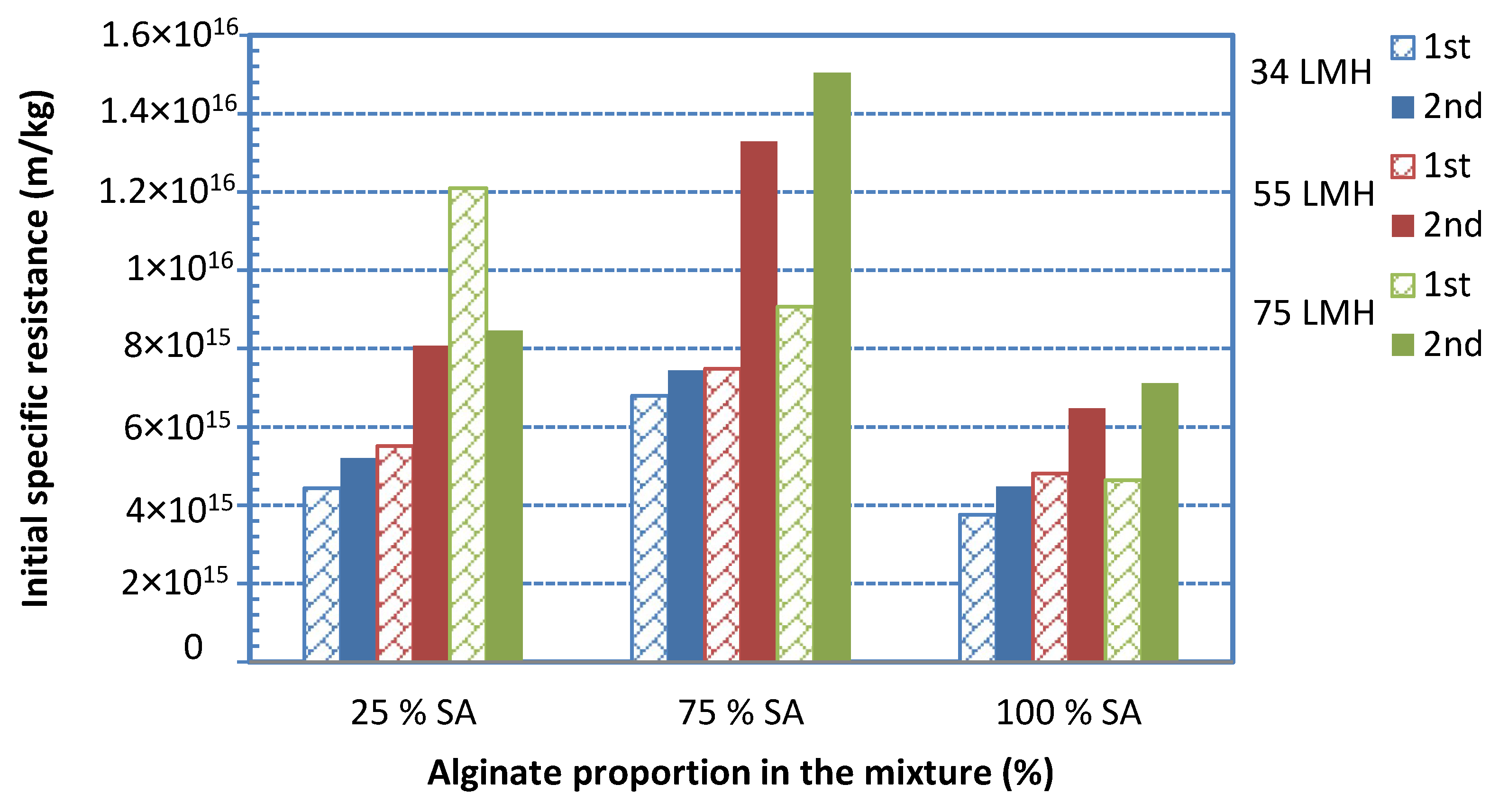
4.5. Effect of Feed-Solution Origin/Preparation on Fouling Resistance a
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Fouling Species | Flux (L/m2h) | αi (m/kg) | Po (kPa) | n (-) | Correlations |
|---|---|---|---|---|---|
| SA 100% | 18 | 2.23 × 1015 | 1.9 | 0.30 | αi = γ·Jβ where |
| 22 | 3.44 × 1015 | 5.4 | 0.14 | γ = 6.94 × 1014, β = 0.46 | |
| 36 | 3.76 × 1015 | 8.6 | 0.19 | Po = γ·Jβ where | |
| 55 | 4.81 × 1015 | 24.4 | 0.37 | γ = 1.98 × 10−3, β = 1.26 | |
| 73 | 4.64 × 1015 | 75.3 | 0.61 | n = γ·Jβ where | |
| 87 | 5.50 × 1015 | 110.2 | 0.70 | γ = 2.48 × 10−3, β = 1.26 | |
| SA 75%-BSA 25% | 18 | 5.60 × 1015 | 10.0 | 0.23 | αi = γ·Jβ |
| 23 | 5.74 × 1015 | 25.7 | 0.29 | γ = 2.13 × 1015, β = 0.33 | |
| 34 | 6.80 × 1015 | 51.6 | 0.34 | Po = γ·Jβ, | |
| 55 | 7.48 × 1015 | 110.0 | 0.63 | γ = 3.95 × 10−2, β = 2.00 | |
| 71 | 9.07 × 1015 | 188.9 | 0.87 | n = γ·Jβ | |
| 87 | 2.56 × 1016 | 327.2 | 1.26 | γ = 8.37 × 10−3, β = 1.09 | |
| SA 50%-BSA 50% | 17 | 4.93 × 1015 | 0 | 0 | αi = γ·Jβ |
| 24 | 5.01 × 1015 | 0 | 0 | γ = 1.75 × 1015, β = 0.36 | |
| 37 | 6.60 × 1015 | 34.5 | 0.08 | Po = γ·Jβ, | |
| 54 | 7.15 × 1015 | 235.6 | 0.92 | γ = 1.04 × 10−2, β = 2.37 | |
| 88 | 8.55 × 1015 | 327.3 | 1.19 | n = γ·Jβ | |
| γ = 84.96E-06, β = 2.85 | |||||
| SA 25%-BSA 75% | 18 | 3.31 × 1015 | 0 | 0 | αi = γ·Jβ |
| 23 | 4.34 × 1015 | 0 | 0 | γ = 9.89 × 1014, β = 0.44 | |
| 35 | 4.43 × 1015 | 0 | 0 | Po = γ·Jβ, | |
| 56 | 5.51 × 1015 | 117.3 | 0.56 | γ = 1.04 E-4, β = 3.47 | |
| 73 | 1.21 × 1016 | 287.0 | 1.11 | n = γ·Jβ | |
| 89 | 7.23 × 1015 | 624.7 | 1.84 | γ = 2.57 × 10−5, β = 2.47 | |
| BSA 100% | 17 | 1.13 × 1015 | 0 | 0 | αi = γ·Jβ |
| 25 | 1.33 × 1015 | 0 | 0 | γ = 4.87 × 1014, β = 0.48 | |
| 38 | 2.80 × 1015 | 0 | 0 | Po = γ·Jβ, | |
| 57 | 3.46 × 1015 | 53.9 | 0.23 | γ = 6.32 × 10−5, β = 3.37 | |
| 74 | 3.62 × 1015 | 84.5 | 0.48 | n = γ·Jβ | |
| 93 | 4.86 × 1015 | 302.8 | 1.11 | γ = 5.44 × 10−7, β = 3.21 |
References
- Amy, G. Fundamental understanding of organic matter fouling of membranes. Desalination 2008, 231, 44–51. [Google Scholar] [CrossRef]
- Susanto, H.; Arafat, H.; Janssen, E.M.L.; Ulbricht, M. Ultrafiltration of polysaccharide-protein mixtures: Elucidation of fouling mechanisms and fouling control by membrane surface modification. Sep. Purif. Technol. 2008, 63, 558–565. [Google Scholar] [CrossRef]
- Nagaoka, H.; Yamanishi, S.; Miya, A. Modeling of biofouling by extracellular polymers in a membrane separation activated sludge system. Water Sci. Technol. 1998, 38, 497–504. [Google Scholar] [CrossRef]
- Rosenberger, S.; Evenblij, H.; Poele, S.T.; Wintgens, T.; Laabs, C. The importance of liquid phase analyses to understand fouling in membrane assisted activated sludge processes—Six case studies of different European research groups. J. Membr. Sci. 2005, 263, 113–126. [Google Scholar] [CrossRef]
- Le-Clech, P.; Chen, V.; Fane, T.A.G. Fouling in membrane bioreactors used in wastewater treatment. J. Membr. Sci. 2006, 284, 17–53. [Google Scholar] [CrossRef]
- Nataraj, S.; Schomäcker, R.; Kraume, M.; Mishra, I.M.; Drews, A. Analyses of polysaccharide fouling mechanisms during crossflow membrane filtration. J. Membr. Sci. 2008, 308, 152–161. [Google Scholar] [CrossRef]
- Zheng, X.; Zietzschmann, F.; Plume, S.; Paar, H.; Ernst, M.; Wang, Z.; Jekel, M. Understanding and Control of Biopolymer Fouling in Ultrafiltration of Different Water Types. Water 2017, 9, 298. [Google Scholar] [CrossRef]
- Jarusutthirak, C.; Amy, G.; Croué, J.-P. Fouling characteristics of wastewater effluent organic matter (EfOM) isolates on NF and UF membranes. Desalination 2002, 145, 247–255. [Google Scholar] [CrossRef]
- Sutherland, I.W. Biofilm exopolysaccharides: A strong and sticky framework. Microbiology 2001, 147, 3–9. [Google Scholar] [CrossRef]
- Zheng, X.; Khan, M.T.; Croué, J.-P. Contribution of effluent organic matter (EfOM) to ultrafiltration (UF) membrane fouling: Isolation, characterization, and fouling effect of EfOM fractions. Water Res. 2014, 65, 414–424. [Google Scholar] [CrossRef]
- Shi, X.; Tal, G.; Hankins, N.P.; Gitis, V. Fouling and cleaning of ultrafiltration membranes: A review. J. Water Process. Eng. 2014, 1, 121–138. [Google Scholar] [CrossRef]
- Grant, G.T.; Morris, E.R.; Rees, D.A.; Smith, P.J.C.; Thom, D. Biological interactions between polysaccharides and divalent cations: The egg-box model. FEBS Lett. 1973, 32, 195–198. [Google Scholar] [CrossRef] [Green Version]
- Braccini, I.; Pérez, S. Molecular Basis of Ca2+-Induced Gelation in Alginates and Pectins: The Egg-Box Model Revisited. Biomacromolecules 2001, 2, 1089–1096. [Google Scholar] [CrossRef]
- Wloka, M.; Rehage, H.; Flemming, H.C.; Wingender, J. Rheological properties of viscoelastic biofilm extracellular polymeric substances and comparison to the behavior of calcium alginate gels. Colloid Polym. Sci. 2004, 282, 1067–1076. [Google Scholar] [CrossRef]
- McClements, D.J. Non-covalent interactions between proteins and polysaccharides. Biotechnol. Adv. 2006, 24, 621–625. [Google Scholar] [CrossRef]
- Neemann, F.; Rosenberger, S.; Jefferson, B.; McAdam, E.J. Non-covalent protein-polysaccharide interactions and their influence on membrane fouling. J. Membr. Sci. 2013, 446, 310–317. [Google Scholar] [CrossRef]
- Neiser, S.; Draget, K.I.; Smidsrød, O. Interactions in bovine serum albumin–calcium alginate gel systems. Food Hydrocoll. 1999, 13, 445–458. [Google Scholar] [CrossRef]
- Jiang, S.; Li, Y.; Ladewig, B.P. A review of reverse osmosis membrane fouling and control strategies. Sci. Total Environ. 2017, 595, 567–583. [Google Scholar] [CrossRef] [Green Version]
- Wu, J.; Contreras, A.E.; Li, Q. Studying the impact of RO membrane surface functional groups on alginate fouling in seawater desalination. J. Membr. Sci. 2014, 458, 120–127. [Google Scholar] [CrossRef]
- Ang, W.S.; Elimelech, M. Protein (BSA) fouling of reverse osmosis membranes: Implications for wastewater reclamation. J. Membr. Sci. 2007, 296, 83–92. [Google Scholar] [CrossRef]
- Mo, H.; Tay, K.G.; Ng, H.Y. Fouling of reverse osmosis membrane by protein (BSA): Effects of pH, calcium, magnesium, ionic strength and temperature. J. Membr. Sci. 2008, 315, 28–35. [Google Scholar] [CrossRef]
- Li, Q.; Xu, Z.; Pinnau, I. Fouling of reverse osmosis membranes by biopolymers in wastewater secondary effluent: Role of membrane surface properties and initial permeate flux. J. Membr. Sci. 2007, 290, 173–181. [Google Scholar] [CrossRef]
- Ang, W.S.; Tiraferri, A.; Chen, K.L.; Elimelech, M. Fouling and cleaning of RO membranes fouled by mixtures of organic foulants simulating wastewater effluent. J. Membr. Sci. 2011, 376, 196–206. [Google Scholar] [CrossRef]
- Katsoufidou, K.S.; Sioutopoulos, D.C.; Yiantsios, S.G.; Karabelas, A.J. UF membrane fouling by mixtures of humic acids and sodium alginate: Fouling mechanisms and reversibility. Desalination 2010, 264, 220–227. [Google Scholar] [CrossRef]
- Sioutopoulos, D.C.; Karabelas, A.J.; Yiantsios, S.G. Organic fouling of RO membranes: Investigating the correlation of RO and UF fouling resistances for predictive purposes. Desalination 2010, 261, 272–283. [Google Scholar] [CrossRef]
- Sioutopoulos, D.C.; Yiantsios, S.G.; Karabelas, A.J. Relation between fouling characteristics of RO and UF membranes in experiments with colloidal organic and inorganic species. J. Membr. Sci. 2010, 350, 62–82. [Google Scholar] [CrossRef]
- Sioutopoulos, D.C.; Karabelas, A.J. Correlation of organic fouling resistances in RO and UF membrane filtration under constant flux and constant pressure. J. Membr. Sci. 2012, 407, 34–46. [Google Scholar] [CrossRef]
- Karabelas, A.J.; Sioutopoulos, D.C. New insights into organic gel fouling of reverse osmosis desalination membranes. Desalination 2015, 368, 114–126. [Google Scholar] [CrossRef]
- Sioutopoulos, D.C.; Karabelas, A.J. Evolution of organic gel fouling resistance in constant pressure and constant flux dead-end ultrafiltration: Differences and similarities. J. Membr. Sci. 2016, 511, 265–277. [Google Scholar] [CrossRef]
- Karabelas, A.J.; Sioutopoulos, D.C. Toward improvement of methods for predicting fouling of desalination membranes—The effect of permeate flux on specific fouling resistance. Desalination 2014, 343, 97–105. [Google Scholar] [CrossRef]
- Sioutopoulos, D.C.; Karabelas, A.J. The effect of permeation flux on the specific resistance of polysaccharide fouling layers developing during dead-end ultrafiltration. J. Membr. Sci. 2015, 473, 292–301. [Google Scholar] [CrossRef]
- Sioutopoulos, D.C.; Goudoulas, T.Β.; Kastrinakis, E.G.; Nychas, S.G.; Karabelas, A.J. Rheological and permeability characteristics of alginate fouling layers developing on reverse osmosis membranes during desalination. J. Membr. Sci. 2013, 434, 74–84. [Google Scholar] [CrossRef]
- Albumin from Bovine Serum, CAS Number: 9048-46-8, Sigma-Aldrich. Available online: https://www.sigmaaldrich.com/content/dam/sigma-aldrich/docs/Sigma/Product_Information_Sheet/a2153pis.pdf (accessed on 26 September 2018).
- Dubois, M.; Hamilton, J.K.; Rebers, P.A.; Smith, F. Colorimetric method for determination of sugars and related substances. Anal. Chem. 1956, 28, 350–356. [Google Scholar] [CrossRef]
- American Public Health Association. Standard Methods for the Examination of Water and Wastewater, 17th ed.; APHA: Washington, NY, USA, 1998. [Google Scholar]
- Boerlage, S.F.E.; Kennedy, M.D.; Dickson, M.R.; El-Hodali, D.E.Y.; Schippers, J.C. The modified fouling index using ultrafiltration membranes (MFI-UF): Characterization, filtration mechanisms and proposed reference membrane. J. Membr. Sci. 2002, 197, 1–21. [Google Scholar] [CrossRef]
- Patsios, S.I.; Goudoulas, T.B.; Kastrinakis, E.G.; Nychas, S.G.; Karabelas, A.J. A novel method for rheological characterization of biofouling layers developing in Membrane Bioreactors (MBR). J. Membr. Sci. 2015, 482, 13–24. [Google Scholar] [CrossRef]












© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sioutopoulos, D.; Karabelas, A.; Mappas, V. Membrane Fouling Due to Protein—Polysaccharide Mixtures in Dead-End Ultrafiltration; the Effect of Permeation Flux on Fouling Resistance. Membranes 2019, 9, 21. https://doi.org/10.3390/membranes9020021
Sioutopoulos D, Karabelas A, Mappas V. Membrane Fouling Due to Protein—Polysaccharide Mixtures in Dead-End Ultrafiltration; the Effect of Permeation Flux on Fouling Resistance. Membranes. 2019; 9(2):21. https://doi.org/10.3390/membranes9020021
Chicago/Turabian StyleSioutopoulos, Dimitrios, Anastasios Karabelas, and Vasileios Mappas. 2019. "Membrane Fouling Due to Protein—Polysaccharide Mixtures in Dead-End Ultrafiltration; the Effect of Permeation Flux on Fouling Resistance" Membranes 9, no. 2: 21. https://doi.org/10.3390/membranes9020021
APA StyleSioutopoulos, D., Karabelas, A., & Mappas, V. (2019). Membrane Fouling Due to Protein—Polysaccharide Mixtures in Dead-End Ultrafiltration; the Effect of Permeation Flux on Fouling Resistance. Membranes, 9(2), 21. https://doi.org/10.3390/membranes9020021






