Assessment of the Influence of Added Resistance on Ship Pollutant Emissions and Freight Throughput Using High-Fidelity Numerical Tools
Abstract
1. Introduction
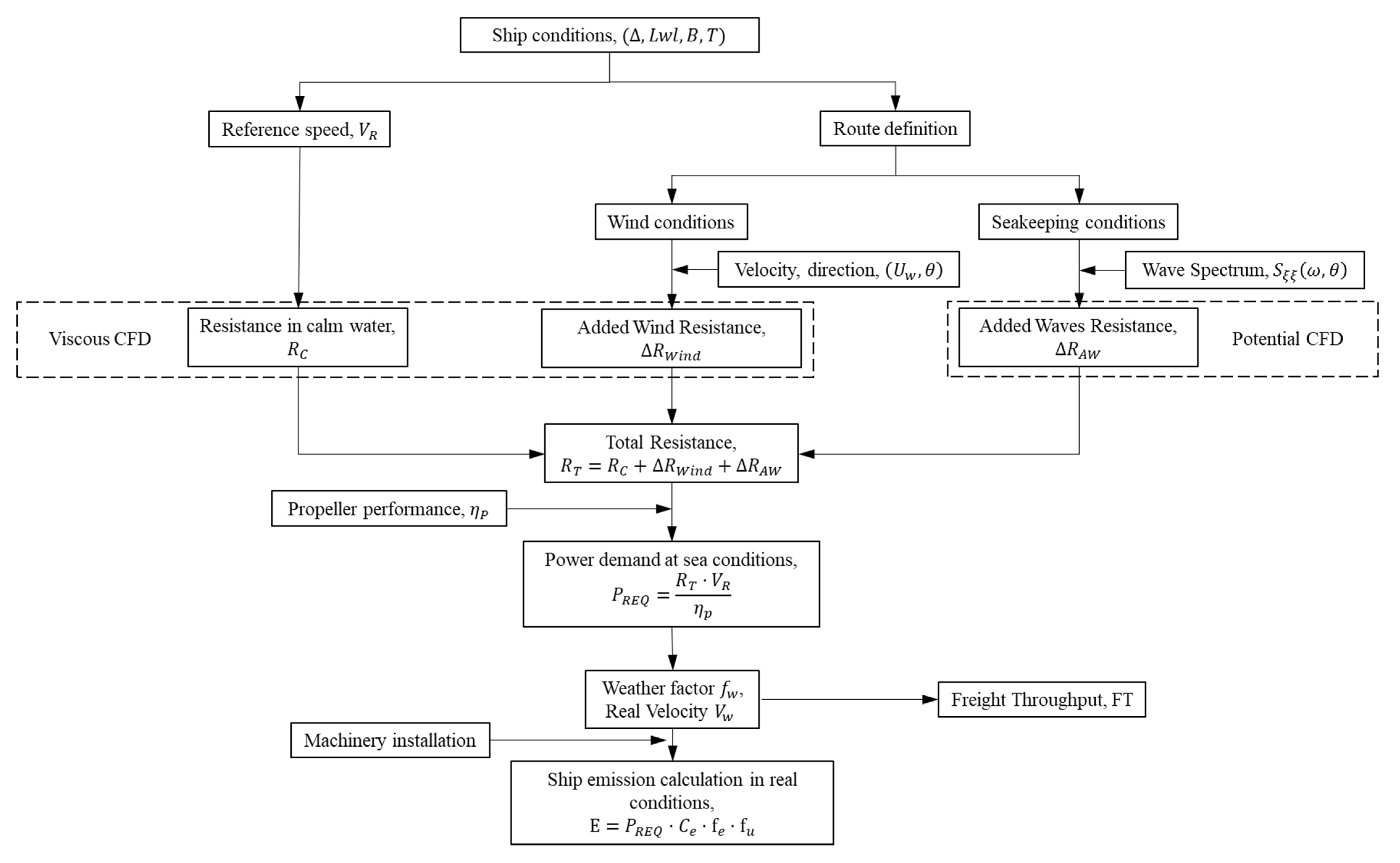
2. Aim of This Work and Methodology
3. Calculation of Weather Factor
3.1. Main Particulars and Ship Conditions
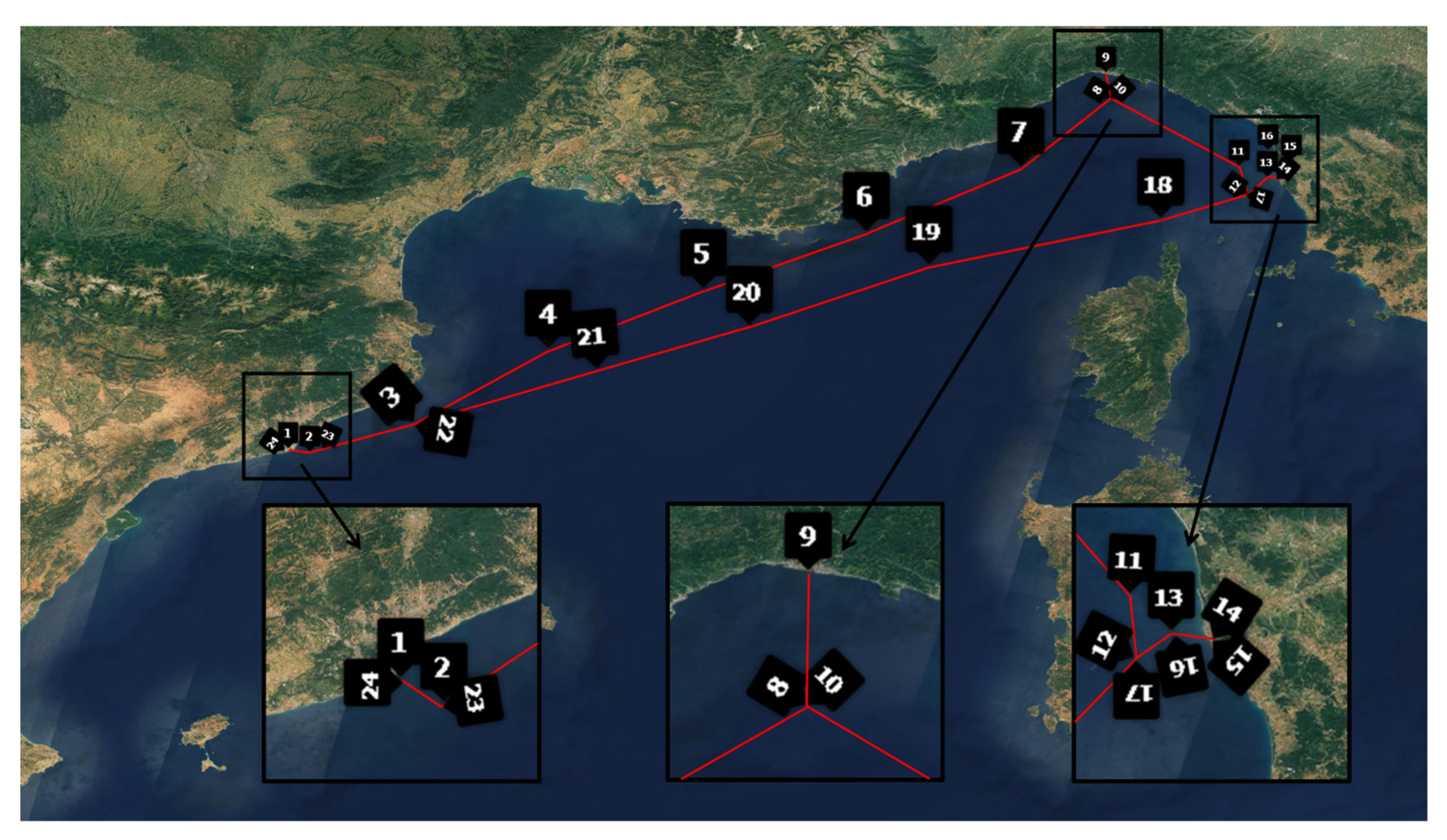
3.2. Route and Environmental Conditions
3.3. Calm Water Resistance
3.4. Added Resistance in Waves
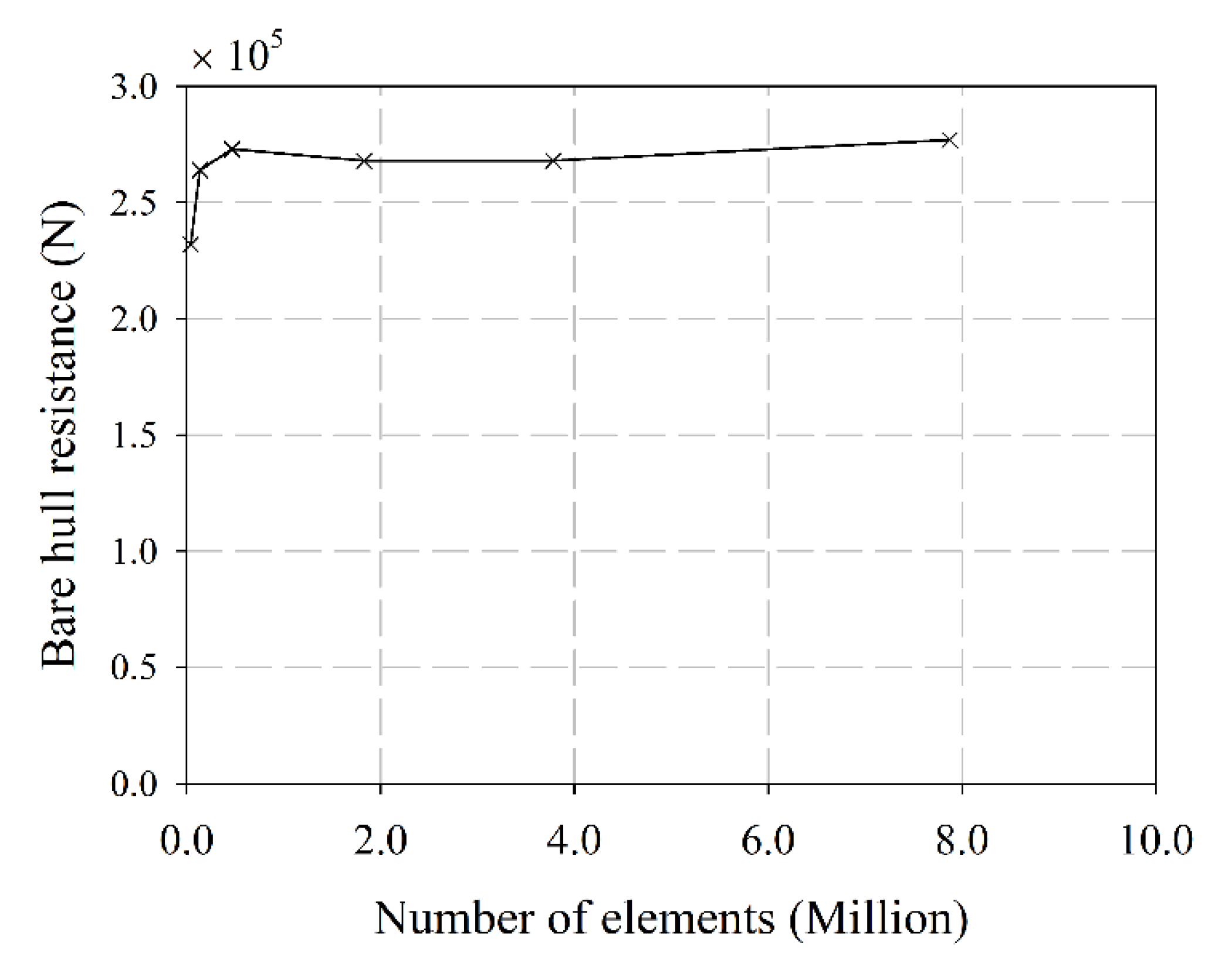
3.4.1. Convergence Analysis
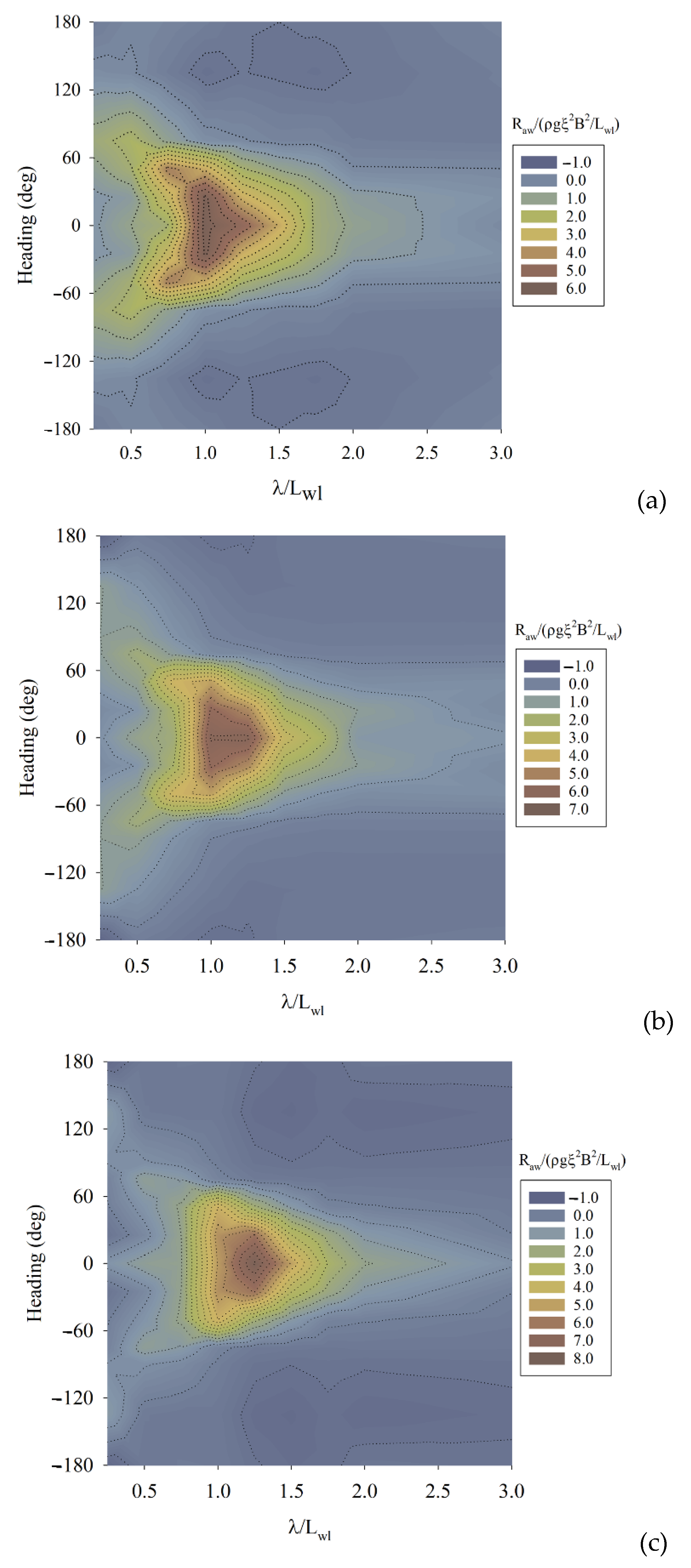
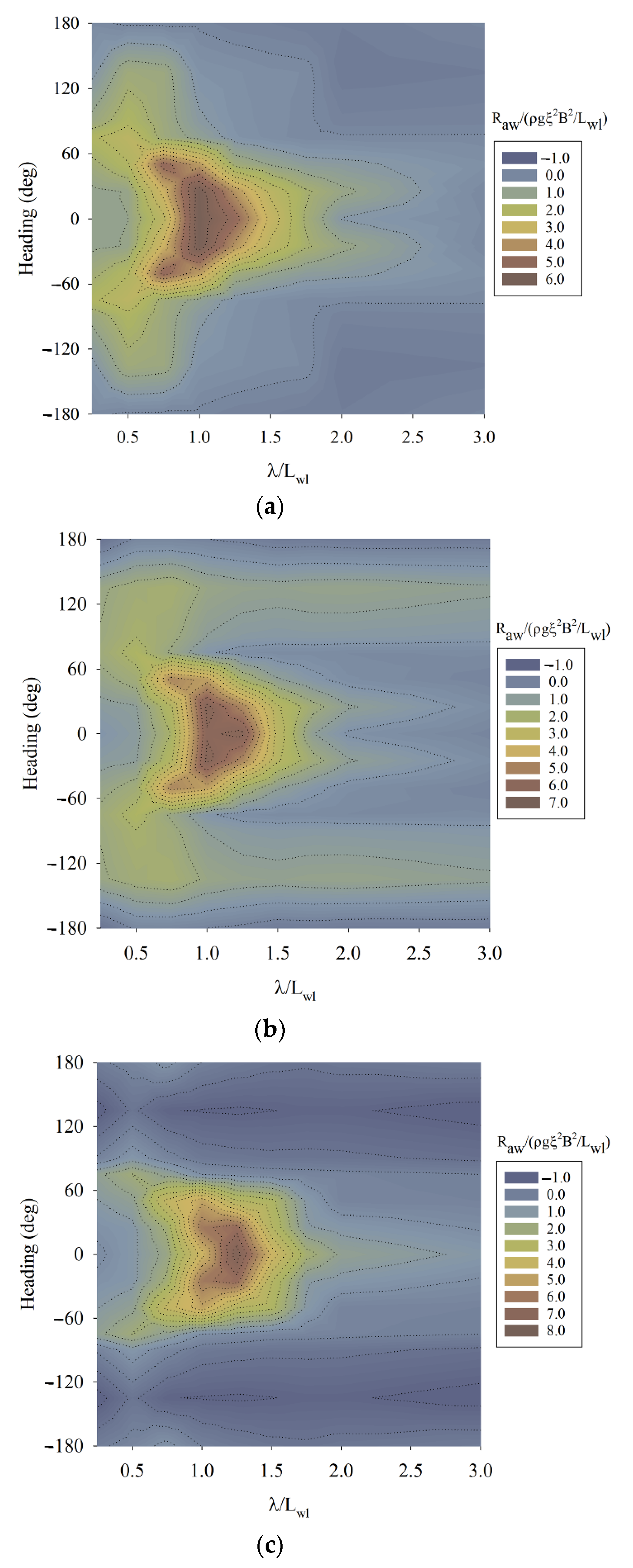
3.4.2. Results of Added Resistance in Regular Waves
3.4.3. Results of Added Resistance in Irregular Waves
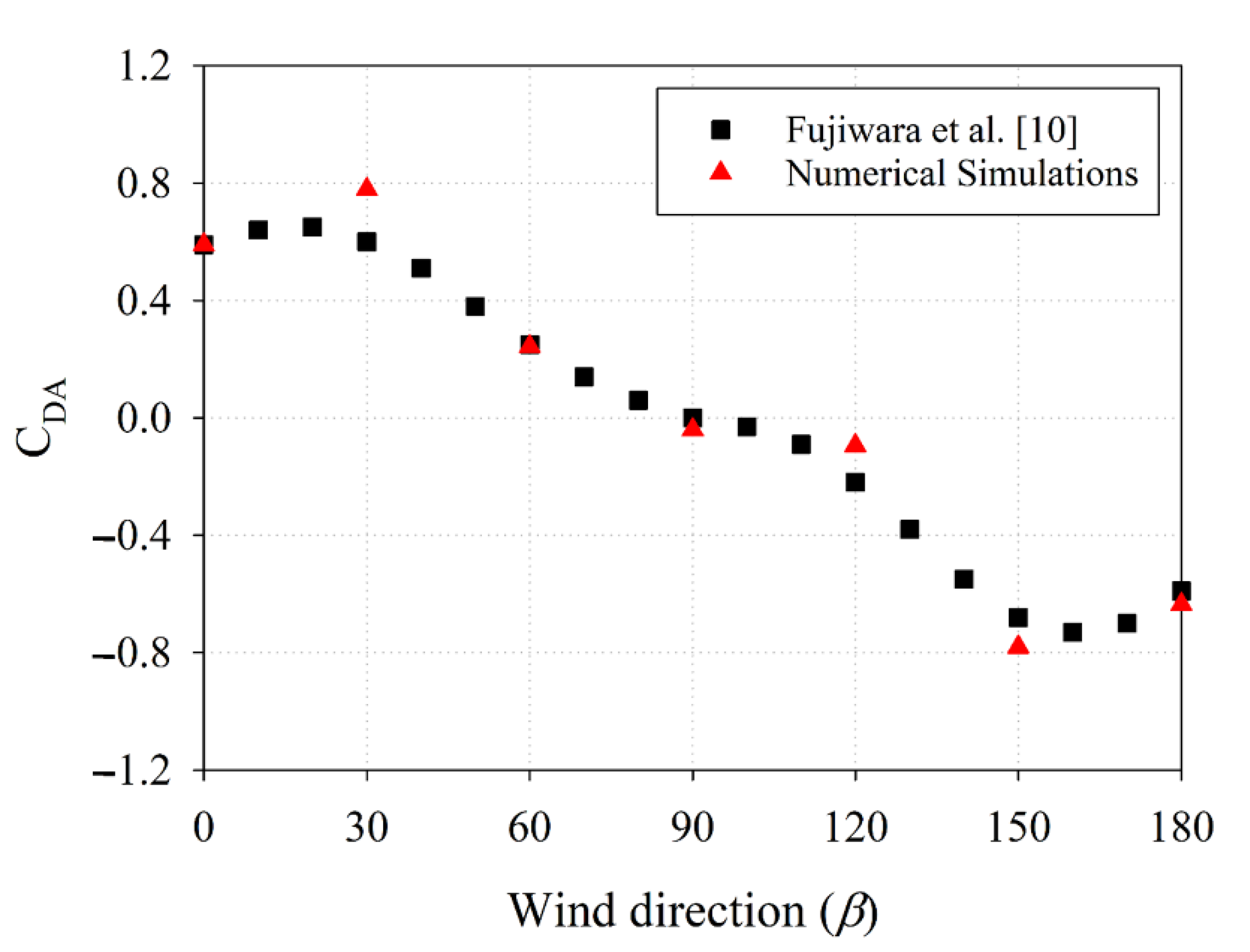
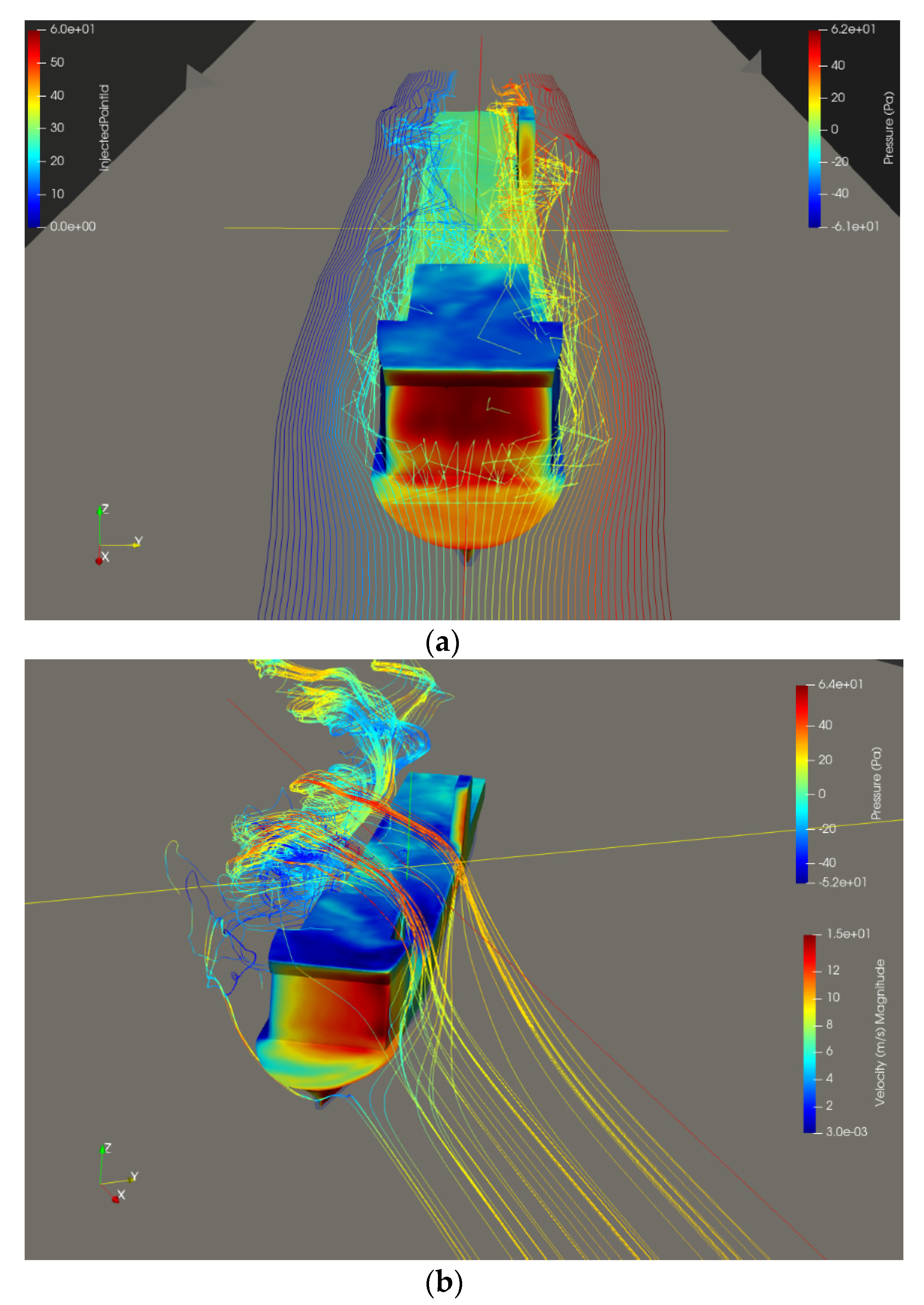
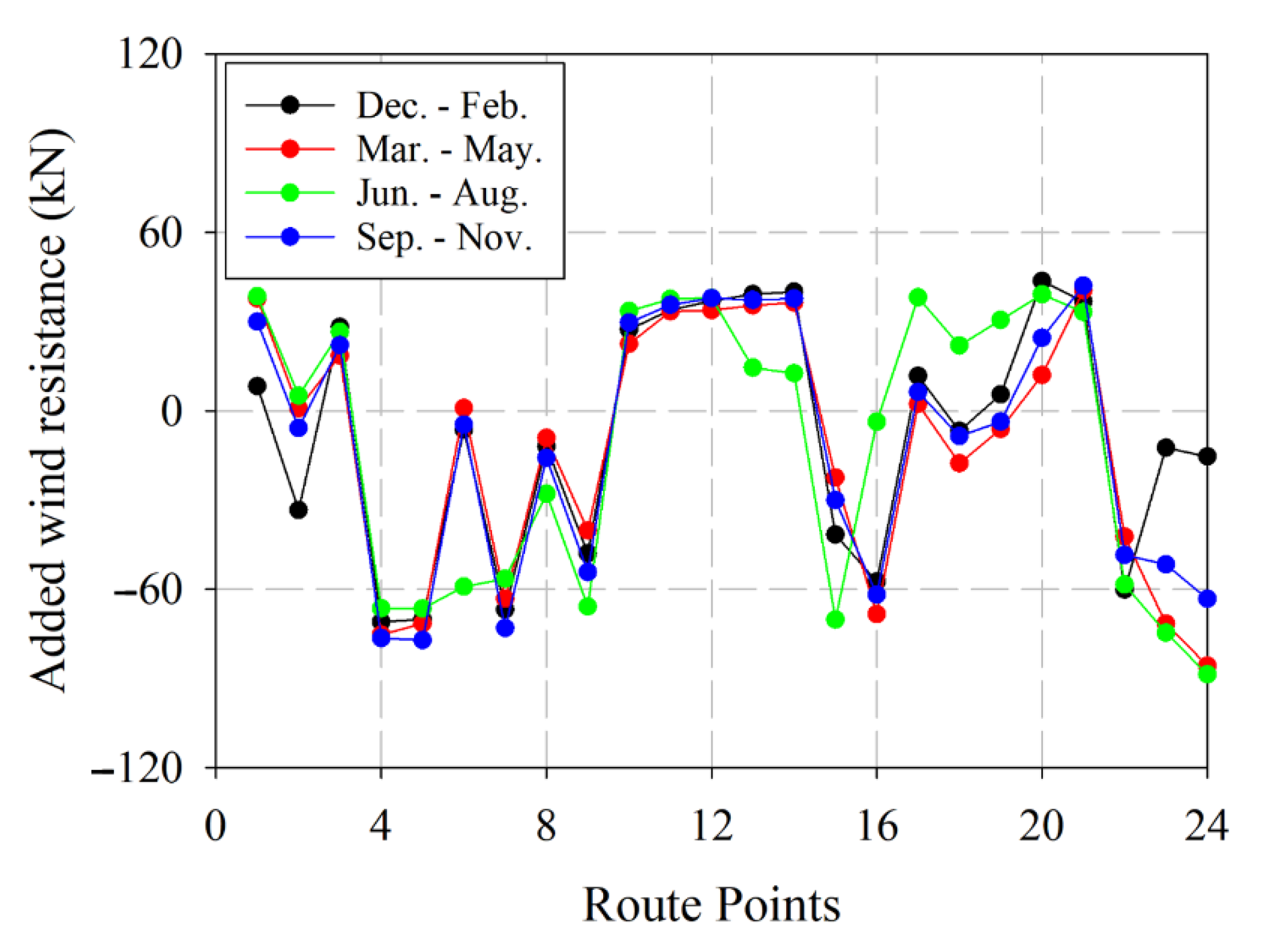
3.5. Wind-Added Resistance
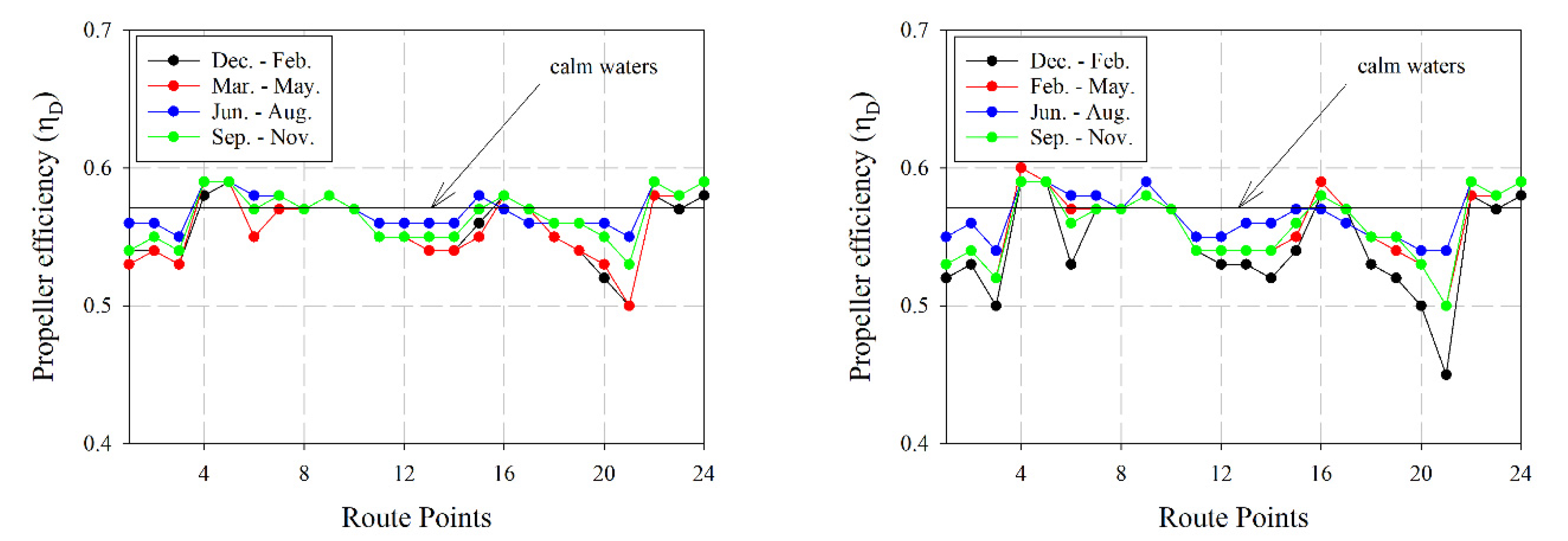
3.6. Propeller Efficiency
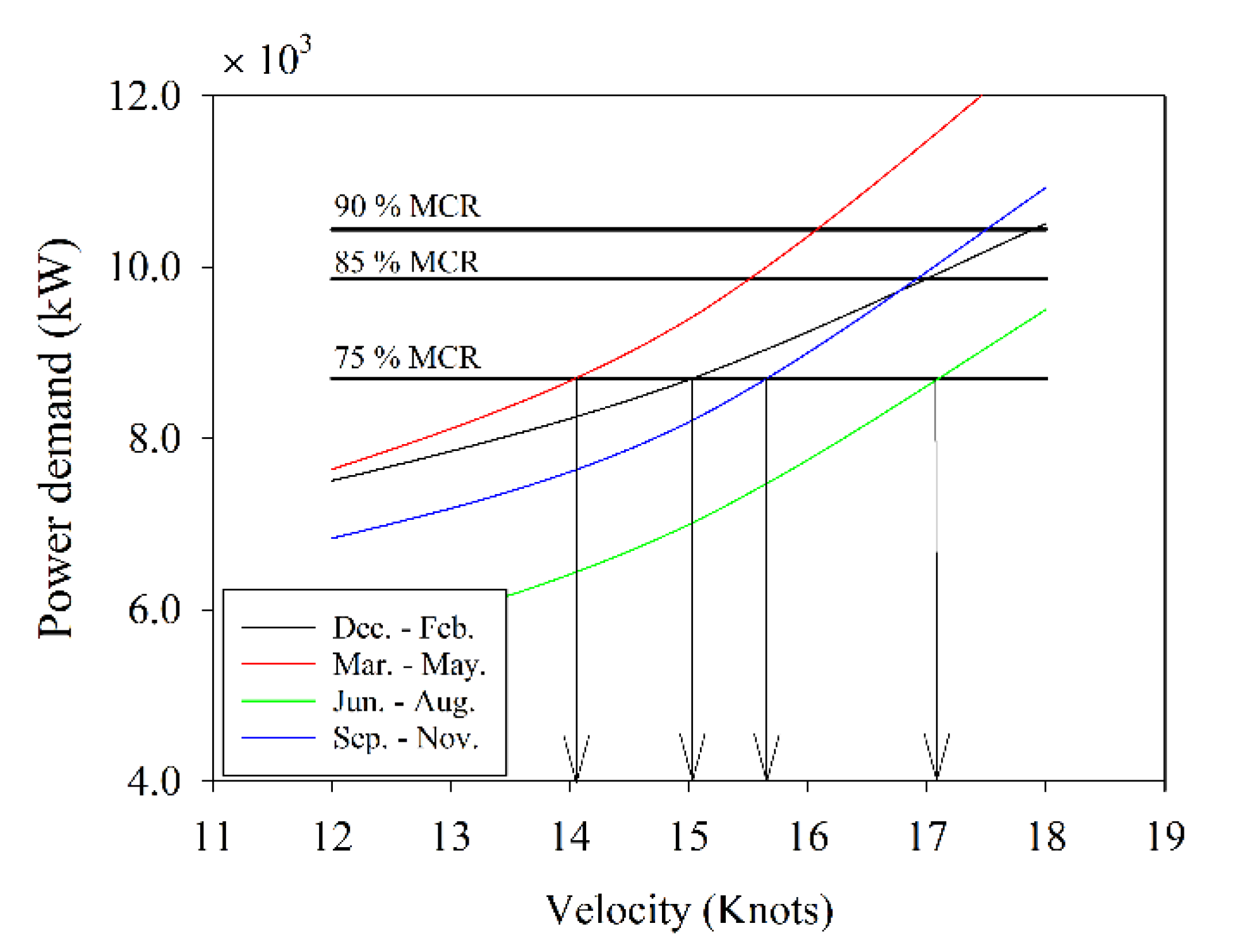
3.7. Power Demand
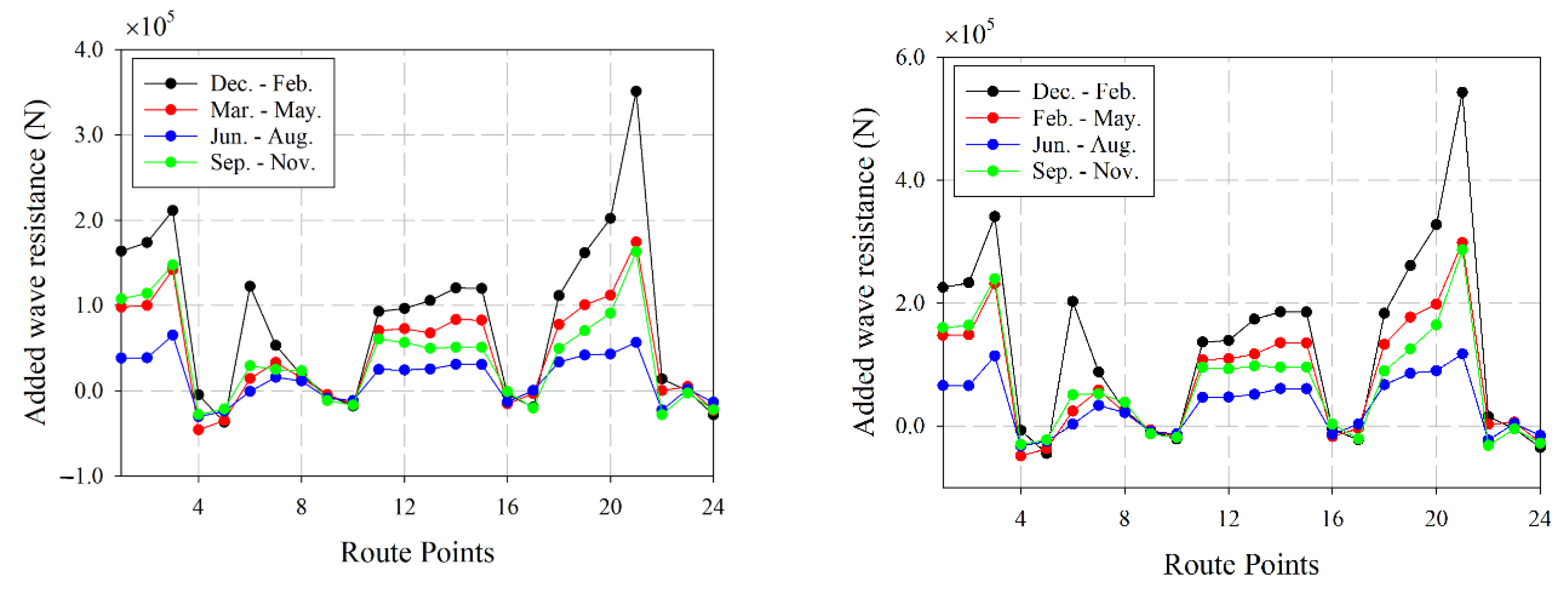
4. Speed and Time Loss Calculation
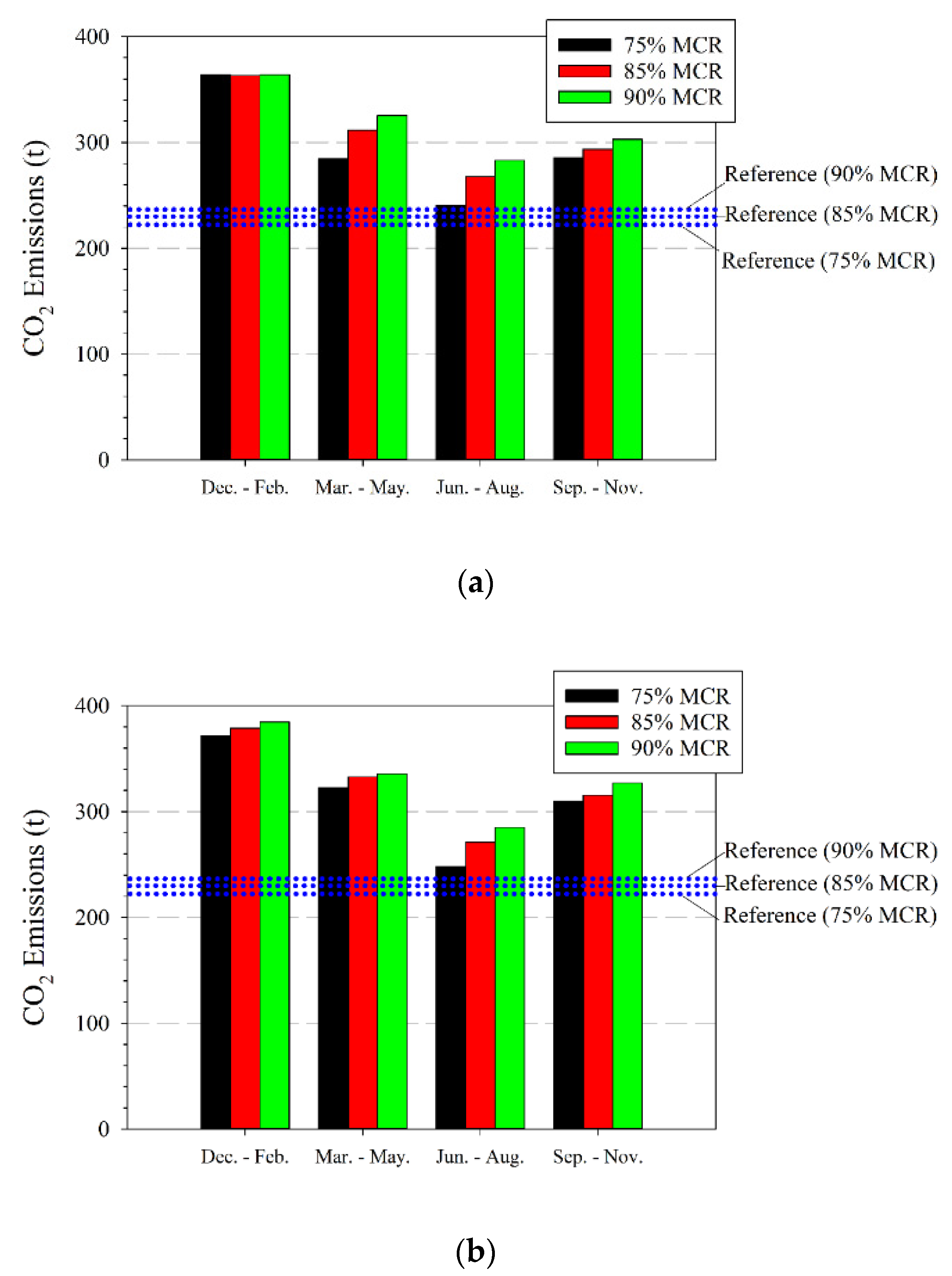
5. Emission and Fuel Consumption Calculations
6. Assessment of Freight Loss Due to the Effect of Added Resistance
7. Discussion of Results
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Points | Latitude | Longitude |
|---|---|---|
| 1 | 41°20.9′ | 002°11.1′ |
| 2 | 41°19.6′ | 002°13.6′ |
| 3 | 41°41.2′ | 003°28.1′ |
| 4 | 42°10.7′ | 004°38.3′ |
| 5 | 42°39.5′ | 005°49.8′ |
| 6 | 43°06.2′ | 007°02.8′ |
| 7 | 43°37.2′ | 008°13.2′ |
| 8 | 44°23.4′ | 009°02.5′ |
| 9 | 44°23.4′ | 008°56.4′ |
| 10 | 44°24.0′ | 008°54.6′ |
| 11 | 44°23.4′ | 008°56.7′ |
| 12 | 44°19.9′ | 008°58.0′ |
| 13 | 43°43.3′ | 010°04.0′ |
| 14 | 43°33.1′ | 010°10.2′ |
| 15 | 43°29.3′ | 010°12.8′ |
| 16 | 43°32.5′ | 010°17.4′ |
| 17 | 43°32.7′ | 010°17.1′ |
| 18 | 43°14.3′ | 009°27.8′ |
| 19 | 42°48.5′ | 007°29.4′ |
| 20 | 42°25.7′ | 006°02.8′ |
| 21 | 42°01.9′ | 004°38.5′ |
| 22 | 41°41.2′ | 003°28.1′ |
| 23 | 41°19.6′ | 002°13.6′ |
| 24 | 41°20.9′ | 002°11.1′ |
| Points | Significant Height (m) | Peak Period (s) | Wave Heading (deg) | Wind Velocity (m/s) | Wind Heading (deg) |
|---|---|---|---|---|---|
| 1 | 1.055 | 6.50 | 126.6 | 3.68 | 193.9 |
| 2 | 1.055 | 6.50 | 126.6 | 3.68 | 193.9 |
| 3 | 1.524 | 5.96 | 112.0 | 4.57 | 108.2 |
| 4 | 1.773 | 5.97 | 183.7 | 9.24 | 234.1 |
| 5 | 1.583 | 5.97 | 198.8 | 9.33 | 238.1 |
| 6 | 1.447 | 6.01 | 139.9 | 7.74 | 155.7 |
| 7 | 0.911 | 6.02 | 131.3 | 5.51 | 182.4 |
| 8 | 0.879 | 6.24 | 141.8 | 5.14 | 162.3 |
| 9 | 0.879 | 6.24 | 141.8 | 5.14 | 162.3 |
| 10 | 0.879 | 6.24 | 141.8 | 5.14 | 162.3 |
| 11 | 0.879 | 6.24 | 141.8 | 5.14 | 162.3 |
| 12 | 0.879 | 6.24 | 141.8 | 5.14 | 162.3 |
| 13 | 1.110 | 5.87 | 171.0 | 6.44 | 173.2 |
| 14 | 1.110 | 5.87 | 171.0 | 6.44 | 173.2 |
| 15 | 1.110 | 5.87 | 171.0 | 6.44 | 173.2 |
| 16 | 1.110 | 5.87 | 171.0 | 6.44 | 173.2 |
| 17 | 1.110 | 5.87 | 171.0 | 6.44 | 173.2 |
| 18 | 1.187 | 6.03 | 182.8 | 7.74 | 155.7 |
| 19 | 1.624 | 6.15 | 176.5 | 7.77 | 171.9 |
| 20 | 1.589 | 6.11 | 188.1 | 8.43 | 210.2 |
| 21 | 1.846 | 6.05 | 214.0 | 9.53 | 240.9 |
| 22 | 1.763 | 6.11 | 130.3 | 4.57 | 108.2 |
| 23 | 1.055 | 6.50 | 126.6 | 3.68 | 193.9 |
| 24 | 1.055 | 6.50 | 126.6 | 3.68 | 193.9 |
| Points | Significant Height (m) | Peak Period (s) | Wave Heading (deg) | Wind Velocity (m/s) | Wind Heading (deg) |
|---|---|---|---|---|---|
| 1 | 0.960 | 5.99 | 131.7 | 3.98 | 150.5 |
| 2 | 0.960 | 5.99 | 131.7 | 3.98 | 150.5 |
| 3 | 1.273 | 5.86 | 107.3 | 4.29 | 118.9 |
| 4 | 1.468 | 5.58 | 207.4 | 7.31 | 210.4 |
| 5 | 1.323 | 5.54 | 205.4 | 6.72 | 210.1 |
| 6 | 1.176 | 5.60 | 174.2 | 6.60 | 142.1 |
| 7 | 0.827 | 5.69 | 136.2 | 4.60 | 180.8 |
| 8 | 0.820 | 6.03 | 141.5 | 4.07 | 165.7 |
| 9 | 0.820 | 6.03 | 141.5 | 4.07 | 165.7 |
| 10 | 0.820 | 6.03 | 141.5 | 4.07 | 165.7 |
| 11 | 0.820 | 6.03 | 141.5 | 4.07 | 165.7 |
| 12 | 0.820 | 6.03 | 141.5 | 4.07 | 165.7 |
| 13 | 0.981 | 5.77 | 184.7 | 5.29 | 162.7 |
| 14 | 0.981 | 5.77 | 184.7 | 5.29 | 162.7 |
| 15 | 0.981 | 5.77 | 184.7 | 5.29 | 162.7 |
| 16 | 0.981 | 5.77 | 184.7 | 5.29 | 162.7 |
| 17 | 0.981 | 5.77 | 184.7 | 5.29 | 162.7 |
| 18 | 1.022 | 5.80 | 189.0 | 6.60 | 142.1 |
| 19 | 1.316 | 5.74 | 184.5 | 6.49 | 153.1 |
| 20 | 1.306 | 5.68 | 192.8 | 6.33 | 180.1 |
| 21 | 1.519 | 5.63 | 215.7 | 7.22 | 210.8 |
| 22 | 1.438 | 5.76 | 127.6 | 4.29 | 118.9 |
| 23 | 0.960 | 5.99 | 131.7 | 3.98 | 150.5 |
| 24 | 0.960 | 5.99 | 131.7 | 3.98 | 150.5 |
| Points | Significant Height (m) | Peak Period (s) | Wave Heading (deg) | Wind Velocity (m/s) | Wind Heading (deg) |
|---|---|---|---|---|---|
| 1 | 0.740 | 5.49 | 132.6 | 3.86 | 143.8 |
| 2 | 0.740 | 5.49 | 132.6 | 3.86 | 143.8 |
| 3 | 0.973 | 5.49 | 95.2 | 3.58 | 108.2 |
| 4 | 1.110 | 4.92 | 215.2 | 6.66 | 247.8 |
| 5 | 1.014 | 4.92 | 210.9 | 6.35 | 249.4 |
| 6 | 0.900 | 5.02 | 180.3 | 4.57 | 198.6 |
| 7 | 0.671 | 5.04 | 128.1 | 2.98 | 177.3 |
| 8 | 0.674 | 5.23 | 128.7 | 2.73 | 147.3 |
| 9 | 0.674 | 5.23 | 128.7 | 2.73 | 147.3 |
| 10 | 0.674 | 5.23 | 128.7 | 2.73 | 147.3 |
| 11 | 0.674 | 5.23 | 128.7 | 2.73 | 147.3 |
| 12 | 0.674 | 5.23 | 128.7 | 2.73 | 147.3 |
| 13 | 0.775 | 5.19 | 192.6 | 4.05 | 219.5 |
| 14 | 0.775 | 5.19 | 192.6 | 4.05 | 219.5 |
| 15 | 0.775 | 5.19 | 192.6 | 4.05 | 219.5 |
| 16 | 0.775 | 5.19 | 192.6 | 4.05 | 219.5 |
| 17 | 0.775 | 5.19 | 192.6 | 4.05 | 219.5 |
| 18 | 0.826 | 5.22 | 191.1 | 4.57 | 198.6 |
| 19 | 1.000 | 5.14 | 190.6 | 4.84 | 205.3 |
| 20 | 0.999 | 5.07 | 198.0 | 5.61 | 231.2 |
| 21 | 1.143 | 5.00 | 224.7 | 6.50 | 248.5 |
| 22 | 1.076 | 5.10 | 107.0 | 3.58 | 108.2 |
| 23 | 0.740 | 5.49 | 132.6 | 3.86 | 143.8 |
| 24 | 0.740 | 5.49 | 132.6 | 3.86 | 143.8 |
| Points | Significant Height (m) | Peak Period (s) | Wave Heading (deg) | Wind Velocity (m/s) | Wind Heading (deg) |
|---|---|---|---|---|---|
| 1 | 0.970 | 6.13 | 124.3 | 3.58 | 167.0 |
| 2 | 0.970 | 6.13 | 124.3 | 3.58 | 167.0 |
| 3 | 1.293 | 5.82 | 101.4 | 4.57 | 115.5 |
| 4 | 1.471 | 5.56 | 194.4 | 7.32 | 223.0 |
| 5 | 1.323 | 5.53 | 194.2 | 7.23 | 220.7 |
| 6 | 1.196 | 5.50 | 163.9 | 5.86 | 151.7 |
| 7 | 0.817 | 5.04 | 123.9 | 4.51 | 192.5 |
| 8 | 0.812 | 5.80 | 128.9 | 4.58 | 157.8 |
| 9 | 0.812 | 5.80 | 128.9 | 4.58 | 157.8 |
| 10 | 0.812 | 5.80 | 128.9 | 4.58 | 157.8 |
| 11 | 0.812 | 5.80 | 128.9 | 4.58 | 157.8 |
| 12 | 0.812 | 5.80 | 128.9 | 4.58 | 157.8 |
| 13 | 0.981 | 5.19 | 164.5 | 5.12 | 168.1 |
| 14 | 0.981 | 5.19 | 164.5 | 5.12 | 168.1 |
| 15 | 0.981 | 5.19 | 164.5 | 5.12 | 168.1 |
| 16 | 0.981 | 5.19 | 164.5 | 5.12 | 168.1 |
| 17 | 0.981 | 5.19 | 164.5 | 5.12 | 168.1 |
| 18 | 0.996 | 5.59 | 169.9 | 5.86 | 151.7 |
| 19 | 1.304 | 5.61 | 171.4 | 5.86 | 160.9 |
| 20 | 1.302 | 5.61 | 181.1 | 6.47 | 192.6 |
| 21 | 1.517 | 5.62 | 205.0 | 7.31 | 222.1 |
| 22 | 1.452 | 5.71 | 113.5 | 4.57 | 115.5 |
| 23 | 0.970 | 6.13 | 124.3 | 3.58 | 167.0 |
| 24 | 0.970 | 6.13 | 124.3 | 3.58 | 167.0 |
References
- Lloyd, A.R.J.M. Ship performance in rough weather. J. Navig. 1978, 31, 93–103. [Google Scholar] [CrossRef]
- Szlapczynska, J. Multi-objective weather routing with customised criteria and constraints. J. Navig. 2015, 68, 338–354. [Google Scholar] [CrossRef]
- Journée, J.M.J.; Meijers, J.H.C. Ship Routering for Optimum Performance. Trans. Inst. Mar. Eng. 1980, 92, C56. Available online: http://resolver.tudelft.nl/uuid:805c45f7-bbc0-4baa-802d-637871416490 (accessed on 5 May 2021).
- Wilson, P.A. A review of the methods of calculation of added resistance for ships in a seaway. J. Wind Eng. Ind. Aerodyn. 1985, 20, 187–199. [Google Scholar] [CrossRef]
- Lu, R.; Turan, O.; Boulougouris, E. Voyage optimisation: Prediction of ship specific fuel consumption for energy efficient shipping. In Proceedings of the 3rd International Conference on Technologies, Operations, Logistics and Modelling for Low Carbon Shipping, London, UK, 9–11 September 2013. [Google Scholar]
- Prpić-Oršić, J.; Faltinsen, O. Estimation of ship speed loss and associated CO2 emissions in a seaway. Ocean Eng. 2012, 44, 1–10. [Google Scholar] [CrossRef]
- Prpić-Oršić, J.; Roberto, V.; Guedes Soares, C.; Faltinsen, O. Influence of ship routes on fuel consumption and CO2 emission. In Proceedings of the 2nd Maritime Technology and Engineering Conference— MARTECH 2014, Lisbon, Portugal, 15–17 October 2014; pp. 857–864. [Google Scholar]
- Vettor, R.; Prpić-Oršić, J.; Guedes Soares, C. Impact of wind loads on long -terms fuel consumption and emission in trans-oceanic shipping. Brodogradnja 2018, 69, 15–28. [Google Scholar] [CrossRef]
- Isherwood, R.M. Wind Resistance of Merchant Ships. R. Inst. Nav. Archit. 1972, 115, 327–338. [Google Scholar]
- Fujiwara, T.; Ueno, M.; Ikeda, Y. A new estimation method of wind forces and moment acting on ships on the basis of physical component models. J. Jpn. Soc. Nav. Archit. Ocean Eng. 2005, 2, 243–255. [Google Scholar] [CrossRef][Green Version]
- Degiuli, N.; Ćatipović, I.; Martić, I.; Werner, A.; Čorić, V. Increase of ship fuel consumption due to the added resistance in waves. J. Sustain. Dev. Energy Water Environ. Syst. 2017, 5, 1–14. [Google Scholar] [CrossRef]
- Kobayashi, H.; Kume, K.; Orihara, H.; Ikebuchi, T.; Aoki, I.; Yoshida, R.; Yoshida, H.; Ryu, T.; Arai, Y.; Katagiri, K.; et al. Parametric study of added resistance and ship motion in head waves through RANS: Calculation guideline. Appl. Ocean Res. 2021, 110, 102573. [Google Scholar] [CrossRef]
- Shivachev, E.; Khorasanchi, M.; Day, S.; Turan, O. Impact of trim on added resistance of KRISO container ship (KCS) in head waves: An experimental and numerical study. Ocean Eng. 2020, 211, 107594. [Google Scholar] [CrossRef]
- Lang, X.; Mao, W. A semi-empirical model for ship speed loss prediction at head sea and it validation by full-scale measurements. Ocean Eng. 2020, 209, 107494. [Google Scholar] [CrossRef]
- Li, X.; Sun, B.; Zhao, Q.; Li, Y.; Shen, Z.; Du, W.; Xu, N. Model of speed optimization of oil tanker with irregular winds and waves for given route. Ocean Eng. 2018, 164, 628–639. [Google Scholar] [CrossRef]
- Taskar, B.; Andersen, P. Comparison of added resistance methods using digital twin and full-scale data. Ocean Eng. 2021, 229, 108710. [Google Scholar] [CrossRef]
- Saettone, S.; Taskar, B.; Steen, S.; Andersen, P. Experimental measurements of propulsive factors in following and head waves. Appl. Ocean Res. 2021, 111, 102639. [Google Scholar] [CrossRef]
- Zis, T.; Psaraftis, H.N.; Ding, L. Ship weather routing: A taxonomy and survey. Ocean Eng. 2020, 213, 107697. [Google Scholar] [CrossRef]
- Stopford, M. Maritime Economics, 3rd ed.; Routledge: Oxfordshire, UK, 2009. [Google Scholar]
- Recommended procedures and guidelines. Calculation of the weather factor fw for decrease of ship speed in wind and waves. 7.5-02-07-02.8. In Proceedings of the ITTC International Towing Tank Conference, Zürich, Switzerland, 5–9 November 2018.
- Compass Ingeniería y Sistemas. Tdyn Theory Manual. Available online: http://www.compassis.com/downloads/Manuals/Tdyn_Theory_Manual.pdf (accessed on 20 January 2020).
- García-Espinosa, J.; Serván-Camas, B. A non-linear finite element method on unstructured meshes for added resistance in waves. Ships Offshore Struct. 2019, 14, 153–164. [Google Scholar] [CrossRef]
- Nadukandi, P.; Serván-Camas, B.; Becker, P.A.; García-Espinosa, J. Seakeeping with the semi-lagrangian particle finite element method. Comput. Part. Mech. 2017, 4, 321–329. [Google Scholar] [CrossRef]
- Colom-Cobb, J.; Garcia-Espinosa, J.; Servan-Camas, B.; Nadukandi, P. A second-order semi-Lagrangian particle finite element method for fluid flows. Comput. Part. Mech. 2020, 7, 3–18. [Google Scholar] [CrossRef]
- Eurostat. Maritime Transport Statistics—Short Sea Shipping of Goods. Available online: https://ec.europa.eu/eurostat/statistics-explained/index.php?title=Maritime_transport_statistics_-_short_sea_shipping_of_goods (accessed on 29 June 2020).
- De Osés, F.X.M.; Castells, M. Heavy weather in european short sea shipping: Its influence on selected routes. J. Nav. 2008, 61, 165–176. [Google Scholar] [CrossRef]
- ECSA (European Community Shipowners’ Associations). Short Sea Shipping. The Full Potential Yet to Be Unleashed; ECSA: Brussels, Belgium, 2016. [Google Scholar]
- ESPO (European Sea Ports Organisation). Annual Report European Sea Ports Organisation 2018–2019; ESPO: Brussels, Belgium, 2019. [Google Scholar]
- Puertos del Estado. Oceanography. Available online: http://www.puertos.es/en-us/oceanografia/Pages/portus.aspx (accessed on 15 January 2020).
- AEMET (Agencia Estatal de Meteorología). Available online: http://www.aemet.es/es/idi/prediccion/meteorologia_maritima (accessed on 25 March 2021).
- The Wamdi Group. The WAM model—A third generation ocean wave prediction model. J. Phys. Ocean. 1988, 18, 1775–1810. [Google Scholar] [CrossRef]
- Tolman, H.L. User Manual and System Documentation of WAVEWATCH III Version 4.18; NOAA/NWS/NCEP/MMAB Technical Note, 316; NOAA: College Park, MD, USA; NWS: College Park, MD, USA; NCEP: College Park, MD, USA; MMAB: College Park, MD, USA, 2014; p. 194. [Google Scholar]
- Bengtsson, L.; Andrae, U.; Asplien, T.; Batrak, Y.; Calvo, J.; de Roy, W.; Gleeson, E.; Hansen-Sass, B.; Mariken, H.; Hortal, M.; et al. The Harmonie–Arome model configuration in the Aladin–Hirlam NWP system. Mon. Weather Rev. 2017, 145, 1919–1935. [Google Scholar] [CrossRef]
- García-Espinosa, J.; Oñate, E. An unstructured finite element solver for ship hydrodynamics problems. J. Appl. Mech. 2003, 70, 18–26. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. Am. Inst. Aeronaut. Astronaut. J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Wilcox, D.C. Turbulence Modelling for CFD; DCW Industries: La Cañada, CA, USA, 2002. [Google Scholar]
- Holtrop, J.A.; Mennen, G.G.J. An approximate power prediction method. Int. Shipbuild. Prog. 1982, 29, 166–170. [Google Scholar] [CrossRef]
- Holtrop, J.A. Statistical reanalysis of resistance and propulsion data. Int. Shipbuild. Prog. 1984, 31, 272–276. [Google Scholar]
- Fung, S.C.; Leibman, L. Revised speed-dependent powering predictions for high-speed transom stern hull forms. In Proceedings of the FAST ’95: Third International Conference on Fast Sea Transportation, Lübeck-Travemünde, Germany, 25–27 September 1995. [Google Scholar]
- IMO (International Maritime Organisation). Interim Guidelines for the Calculation of the Coefficient fw for Decrease in Ship Speed in a Representative Sea Condition for Trial Use; IMO Resolution MEPC.1/Circ.796; IMO: London, UK, 2012. [Google Scholar]
- Compass Ingeniería y Sistemas. SeaFEM Theory Manual. Available online: http://www.compassis.com/downloads/Manuals/SeaFEM_Theory_Manual.pdf (accessed on 10 February 2020).
- Janssen, W.D.; Blocken, B.; Wan Wijhe, H.J. CFD simulations of wind loads on a container ship: Validation and impact of geometrical simplifications. J. Wind Eng. Ind. Aerodyn. 2017, 166, 106–116. [Google Scholar] [CrossRef]
- Faltinsen, O.M.; Minsaas, K.J.; Liapis, N.; Skjørdal, S.O. Prediction of resistance and propulsion of a ship in a seaway. In Proceedings of the 13th Symposium on Naval Hydrodynamics, Tokyo, Japan, 6–10 October 1980. [Google Scholar]
- Barnitsas, M.M.; Ray, D.; Kinley, P. Kt, Kq and Efficiency Curves for the Wageningen B-Series Propellers; Report 237; University of Michigan-Department of Naval Architecture and Marine Engineering: An Arbor, MI, USA, 1981. [Google Scholar]
- Recommended Procedures and Guidelines; Preparation, Conduct and Analisys of Speed/Power Trials. 7.5-04-01-01.1; ITTC: Zürich, Switzerland, 2017.
- Taskar, B.; Koosup, K.; Sverre, Y.; Steen, S.; Pedersen, E. The effect of waves on engine-propeller dynamics and propulsion performance of ships. Ocean Eng. 2016, 122, 262–277. [Google Scholar] [CrossRef]
- IMO (International Maritime Organisation). Guidelines on the Method of Calculation of the Attained Energy Efficiency Design Index for New Ships; IMO Resolution MEPC. 254 (67); IMO: London, UK, 2014. [Google Scholar]
- Gutiérrez-Romero, J.E.; Esteve-Pérez, J.; Zamora-Parra, B. Implementing onshore power supply from renewable energy sources for requirements of ships at berth. Appl. Energy 2019, 255, 113883. [Google Scholar] [CrossRef]
- Nunes, R.A.O.; Alvim-Ferraz, M.C.M.; Martins, F.G.; Sousa, S.I.V. The activity-based methodology to assess ship emissions—A review. Environ. Pollut. 2017, 231, 87–103. [Google Scholar] [CrossRef] [PubMed]
- Trozzi, C. Emission estimate methodology for maritime navigation. In Proceedings of the 19th Annual International Emission Inventory Conference. Informing Emerging Issues, San Antonio, TX, USA, 27–30 September 2010. [Google Scholar]
- Lee, C.Y.; Lee, H.L.; Zhang, J. The impact of slow ocean steaming on delivery reliability and fuel consumption. Transp. Res. Part E 2015, 76, 176–190. [Google Scholar] [CrossRef]
- Parthibaraj, C.S.; Subramanian, N.; Palaniappan, P.L.K.; Lai, K. Sustainable decision model for liner shipping industry. Comput. Oper. Res. 2016, 89, 213–229. [Google Scholar] [CrossRef]
- Lee, H.; Aydin, N.; Choi, Y.; Lekhavat, S.; Irani, Z. A decision support system for vessel speed decision in maritime logistics using weather archive big data. Comput. Oper. Res. 2015, 98, 330–342. [Google Scholar] [CrossRef]
- Kontovas, C.A. The green ship routing and scheduling problem (GSRSP): A conceptual approach. Transp. Res. Part D 2014, 31, 61–69. [Google Scholar] [CrossRef]





| Particular | Value | Unit |
|---|---|---|
| Length overall | 180.6 | m |
| Beam | 22.9 | m |
| Depth | 14.1 | m |
| Displacement | 14,918 | Metric Tons |
| Deadweight | 7500 | Metric Tons |
| Block coefficient (Cb) | 0.6 | |
| Power installed | 11,600 | kW |
| Velocity at 85% of MCR | 18.0 | Knots |
| Autonomy | 8000 | Milles |
| Particular | Load Condition 1 | Load Condition 2 | Unit |
|---|---|---|---|
| Displacement | 14,312 | 15,526 | Metric tons |
| Draught at Forward Perpendicular | 5.69 | 6.04 | m |
| Draught at After Perpendicular | 5.98 | 6.32 | m |
| Draught Amidships | 5.83 | 6.18 | m |
| Wetted area | 4569 | 4784 | m2 |
| Vertical center of gravity | 10.68 | 10.03 | m |
| Transversal metacentric height a | 1.92 | 2.42 | m |
| Longitudinal metacentric height | 478.73 | 454.39 | m |
| Parameter | Value | Unit |
|---|---|---|
| Number of Blades (Z) | 4 | |
| Diameter (m) | 4.65 | m |
| Extended–disc area ratio (Ae/Ao) | 0.5 | |
| Pitch–diameter ratio (P/D) | 1.4 |
| Element | Size (mm) |
|---|---|
| Hull surface | 1.0 |
| Inner free surface | 8.0 |
| Outer free surface | 20.0 |
| General size | 30.0 |
| Fr | LC 1 (CFD) | LC 1 (HM) | LC 1 (FL) | LC 2 (CFD) | LC 2 (HM) | LC 2 (FL) |
|---|---|---|---|---|---|---|
| 0.16 | 253.9 | 221 | 175 | 240.4 | 211 | 168 |
| 0.20 | 325.9 | 401 | 325 | 335.2 | 384 | 313 |
| 0.24 | 517.0 | 610 | 512 | 505.6 | 584 | 495 |
| Mesh | Wetted Surface Mesh Size (M) | Inner Surface Mesh Size (M) | Outer Surface Mesh Size (M) | Relative Difference (%) | Number of Elements (×105) | |
|---|---|---|---|---|---|---|
| 1 | 2.0 | 2.0 | 6.0 | 13.74 | 1.05 | |
| 2 | 1.5 | 2.0 | 6.0 | 5.20 | 62 | 1.46 |
| 3 | 1.5 | 1.5 | 4.0 | 6.09 | 17 | 1.48 |
| 4 | 1.0 | 1.5 | 4.0 | 6.07 | 0.3 | 1.55 |
| 5 | 1.0 | 1.0 | 2.0 | 5.67 | 1.8 | 1.91 |
| 6 | 0.5 | 0.5 | 2.0 | 6.22 | 0.7 | 10.75 |
| Parameter | Value | Unit |
|---|---|---|
| Lateral projected area of superstructures, deckhouses, etc. on deck (AOD) | 450.6 | m2 |
| Area of maximum transverse section exposed to the winds (AXV) | 315.8 | m2 |
| Projected lateral area above the waterline (AYV) | 1337.0 | m2 |
| Horizontal distance from midship section to center of lateral projected area AYV (CMC) | 0.9 | m |
| Height of top of superstructure (navigation bridge, etc.) (hBR) | 17.5 | m |
| Height from waterline to center of lateral projected area AYV (hC) | 6.7 | m |
| Smoothing range; normally 10 (deg.) (μ) | 0 | deg |
| Relative wind direction; 0 means heading winds (ψWR) | 180 | deg |
| MCR (%) | 75 | 80 | 90 | |||
|---|---|---|---|---|---|---|
| Load Condition | 1 | 2 | 1 | 1 | 2 | 1 |
| December–February (h) | 72.5 | 74.1 | 63.5 | 72.5 | 74.1 | 63.5 |
| March–May (h) | 56.6 | 64.2 | 54.4 | 56.6 | 64.2 | 54.4 |
| June–August (h) | 48.0 | 49.4 | 46.8 | 48.0 | 49.4 | 46.8 |
| September–November (h) | 56.8 | 61.7 | 51.3 | 56.8 | 61.7 | 51.3 |
| Reference Time (h) | 48.5 | 46.4 | 45.8 | |||
| LC | MCR | 75% | 85% | 90% | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Season | 1 | 2 | 3 | 4 | 1 | 2 | 3 | 4 | 1 | 2 | 3 | 4 | |
| 1 | CO2 (t) | 363.9 | 284.4 | 240.9 | 285.2 | 363.3 | 311.5 | 267.9 | 293.6 | 364.0 | 325.4 | 283.1 | 302.8 |
| SO2 (t) | 2.4 | 1.9 | 1.6 | 1.9 | 2.4 | 2.0 | 1.8 | 1.9 | 2.4 | 2.1 | 1.9 | 2.0 | |
| NOx (t) | 8.5 | 6.7 | 5.6 | 6.7 | 8.5 | 7.3 | 6.3 | 6.9 | 8.5 | 7.6 | 6.6 | 7.1 | |
| PMx (t) | 2.8 | 2.2 | 1.9 | 2.2 | 2.8 | 2.4 | 2.1 | 2.3 | 2.8 | 2.5 | 2.2 | 2.3 | |
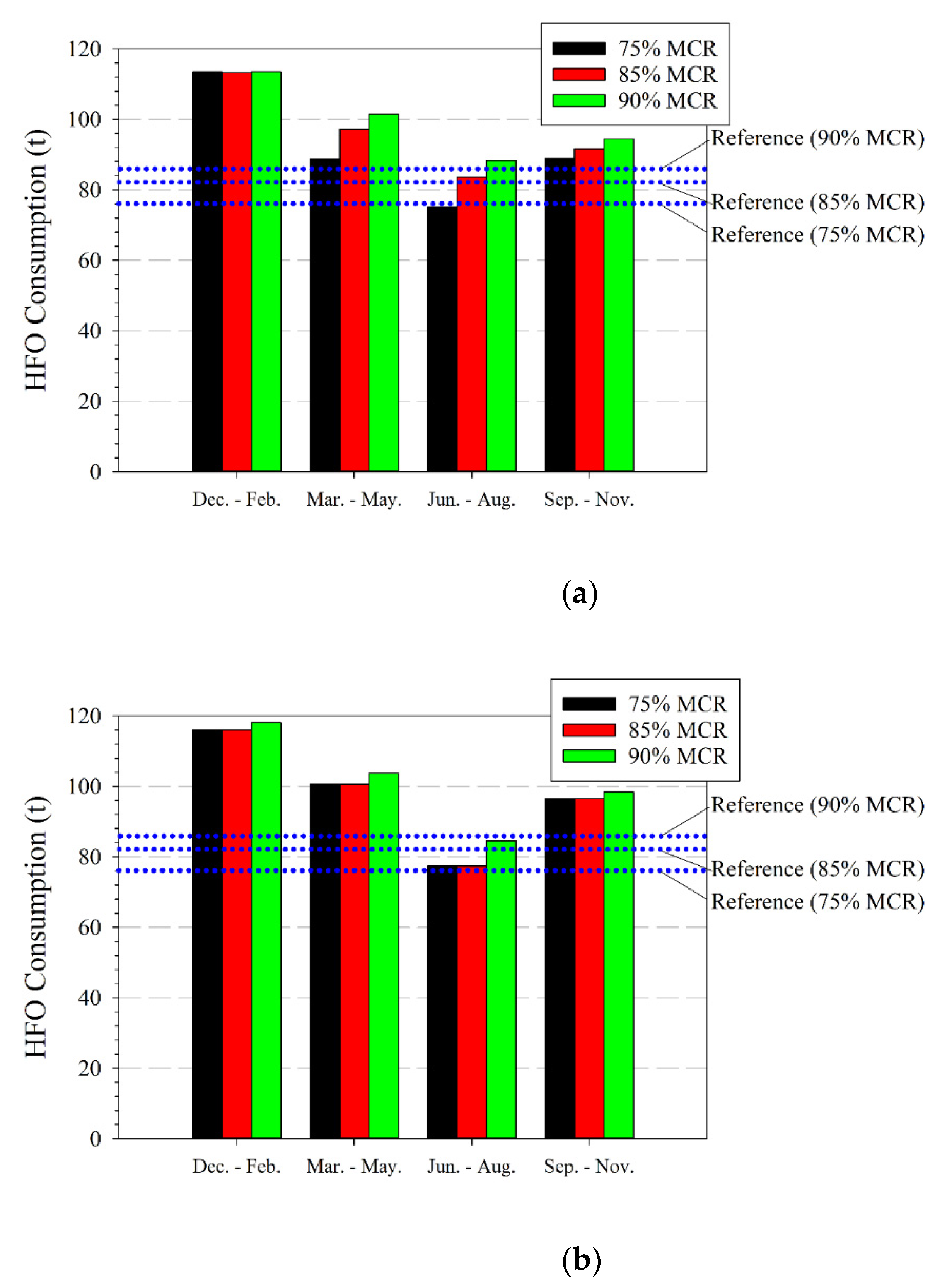
| HFO (t) | 113.5 | 88.7 | 75.1 | 89.0 | 113.3 | 97.2 | 83.6 | 91.6 | 113.5 | 101.5 | 88.3 | 94.4 | |
| 2 | CO2 (t) | 371.9 | 322.5 | 248.2 | 309.7 | 378.8 | 332.6 | 271.2 | 315.4 | 384.6 | 335.7 | 285.0 | 327.1 |
| SO2 (t) | 2.4 | 2.1 | 1.6 | 2.0 | 2.5 | 2.2 | 1.8 | 2.1 | 2.5 | 2.2 | 1.9 | 2.1 | |
| NOx (t) | 8.7 | 7.5 | 5.8 | 7.2 | 8.9 | 7.8 | 6.3 | 7.4 | 9.0 | 7.9 | 6.7 | 7.7 | |
| PMx (t) | 2.9 | 2.5 | 1.9 | 2.4 | 2.9 | 2.6 | 2.1 | 2.4 | 3.0 | 2.6 | 2.2 | 2.5 | |
| HFO (t) | 116.0 | 100.6 | 77.4 | 96.6 | 118.1 | 103.7 | 84.6 | 98.4 | 120.0 | 104.7 | 88.9 | 102.0 | |
| B | CO2 (t) | 243.4 | 264.1 | 275.9 | |||||||||
| SO2 (t) | 1.6 | 1.7 | 1.8 | ||||||||||
| NOx (t) | 5.7 | 6.2 | 6.5 | ||||||||||
| PMx (t) | 1.9 | 2.0 | 2.1 | ||||||||||
| HFO (t) | 75.9 | 82.4 | 86.0 | ||||||||||
| Ports | Linear Meters Capacity (%) | Linear Meters Capacity (m) |
|---|---|---|
| Cargo from Barcelona to Genoa | 40 | 710 |
| Cargo from Barcelona to Livorno | 60 | 1065 |
| Cargo from Genoa to Livorno | 20 | 355 |
| Cargo from Genoa to Barcelona | 20 | 355 |
| Cargo from Livorno to Barcelona | 80 | 1420 |
| Total freight (linear meters) | 3905 |
| MCR (%) | 75 | 85 | 90 | ||||
|---|---|---|---|---|---|---|---|
| Item | CW | SC | CW | SC | CW | SC | |
| Total time per round-trip voyage (h) | December–February | 62.49 | 86.48 | 60.41 | 77.50 | 59.79 | 73.75 |
| March–May | 70.64 | 68.45 | 67.42 | ||||
| June–August | 61.98 | 60.82 | 60.48 | ||||
| September–November | 70.81 | 65.32 | 63.70 | ||||
| Number of round-trip voyages per season | December–February | 34 | 24 | 35 | 27 | 35 | 28 |
| March–May | 30 | 31 | 31 | ||||
| June–August | 34 | 35 | 35 | ||||
| September–November | 30 | 32 | 33 | ||||
| Number of round-trip voyages per year | 136 | 118 | 140 | 125 | 140 | 127 | |
| Maximum freight per season (thousand linear meters) | December–February | 132.8 | 93.7 | 136.7 | 105.4 | 136.7 | 109.3 |
| March–May | 117.2 | 121.1 | 121.1 | ||||
| June–August | 132.8 | 136.7 | 136.7 | ||||
| September–November | 117.2 | 125.0 | 128.9 | ||||
| Maximum freight per year (thousand linear meters) | 531.2 | 460.9 | 546.8 | 488.2 | 546.8 | 496.0 | |
| Freight loss (% linear meters) | −13.24 | −10.71 | −9.29 | ||||
| MCR (%) | 75 | 85 | 90 | ||||
|---|---|---|---|---|---|---|---|
| Item | CW | SC | CW | SC | CW | SC | |
| Total time per round-trip voyage (h) | December–February | 62.49 | 88.07 | 60.41 | 80.20 | 59.79 | 77.13 |
| March–May | 78.23 | 72.13 | 69.10 | ||||
| June–August | 63.43 | 61.40 | 60.78 | ||||
| September–November | 75.68 | 69.12 | 67.70 | ||||
| Number of round-trip voyages per season | December–February | 34 | 24 | 35 | 26 | 35 | 27 |
| Mach–May | 27 | 29 | 30 | ||||
| June–August | 33 | 34 | 35 | ||||
| September–November | 28 | 30 | 31 | ||||
| Number of round-trip voyages per year | 136 | 112 | 140 | 119 | 140 | 123 | |
| Maximum freight per season(thousand linear meters) | December–February | 132.8 | 93.7 | 136.7 | 101.5 | 136.7 | 105.4 |
| March–May | 105.4 | 113.2 | 117.2 | ||||
| June–August | 128.9 | 132.8 | 136.7 | ||||
| September–November | 109.3 | 117.2 | 121.1 | ||||
| Maximum freight per year (thousand linear meters) | 531.2 | 437.3 | 546.8 | 464.7 | 546.8 | 480.4 | |
| Freight loss (% linear meters) | −17.65 | −15.00 | −12.14 | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gutiérrez-Romero, J.E.; Esteve-Pérez, J. Assessment of the Influence of Added Resistance on Ship Pollutant Emissions and Freight Throughput Using High-Fidelity Numerical Tools. J. Mar. Sci. Eng. 2022, 10, 88. https://doi.org/10.3390/jmse10010088
Gutiérrez-Romero JE, Esteve-Pérez J. Assessment of the Influence of Added Resistance on Ship Pollutant Emissions and Freight Throughput Using High-Fidelity Numerical Tools. Journal of Marine Science and Engineering. 2022; 10(1):88. https://doi.org/10.3390/jmse10010088
Chicago/Turabian StyleGutiérrez-Romero, José Enrique, and Jerónimo Esteve-Pérez. 2022. "Assessment of the Influence of Added Resistance on Ship Pollutant Emissions and Freight Throughput Using High-Fidelity Numerical Tools" Journal of Marine Science and Engineering 10, no. 1: 88. https://doi.org/10.3390/jmse10010088
APA StyleGutiérrez-Romero, J. E., & Esteve-Pérez, J. (2022). Assessment of the Influence of Added Resistance on Ship Pollutant Emissions and Freight Throughput Using High-Fidelity Numerical Tools. Journal of Marine Science and Engineering, 10(1), 88. https://doi.org/10.3390/jmse10010088







