Stability and Tracking Control of Nonlinear Rigid-Body Ship Motions
Abstract
1. Introduction
2. Fundamental Equations of Constrained Motion
3. Unconstrained Ship’s Motions
4. Ship’s Constraint Equations
5. Constrained Ship’s Motions
5.1. Control of Ship’s Constrained Motion
5.2. Control of Ship System’s Uncertainties
6. Numerical Results and Simulations
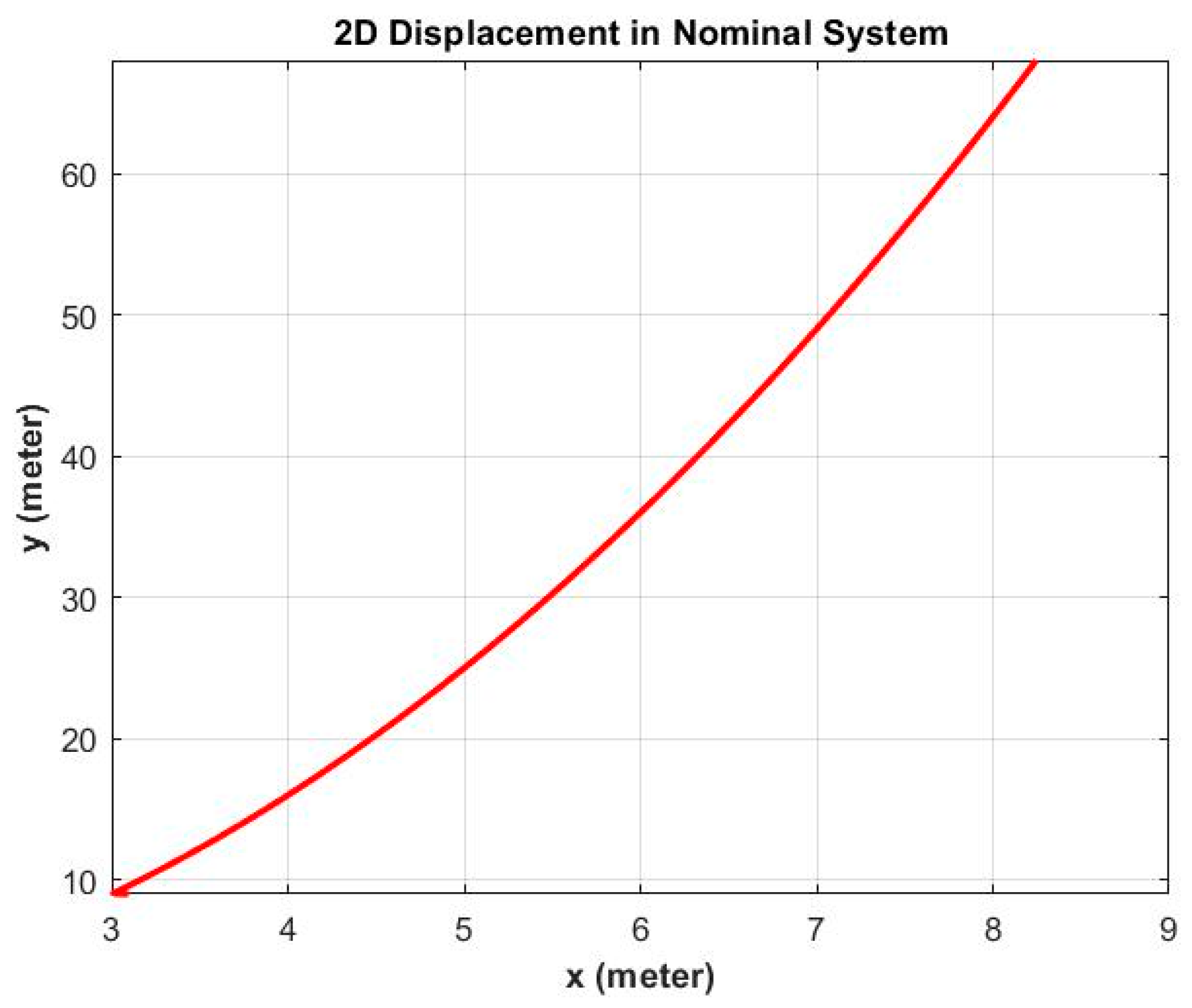
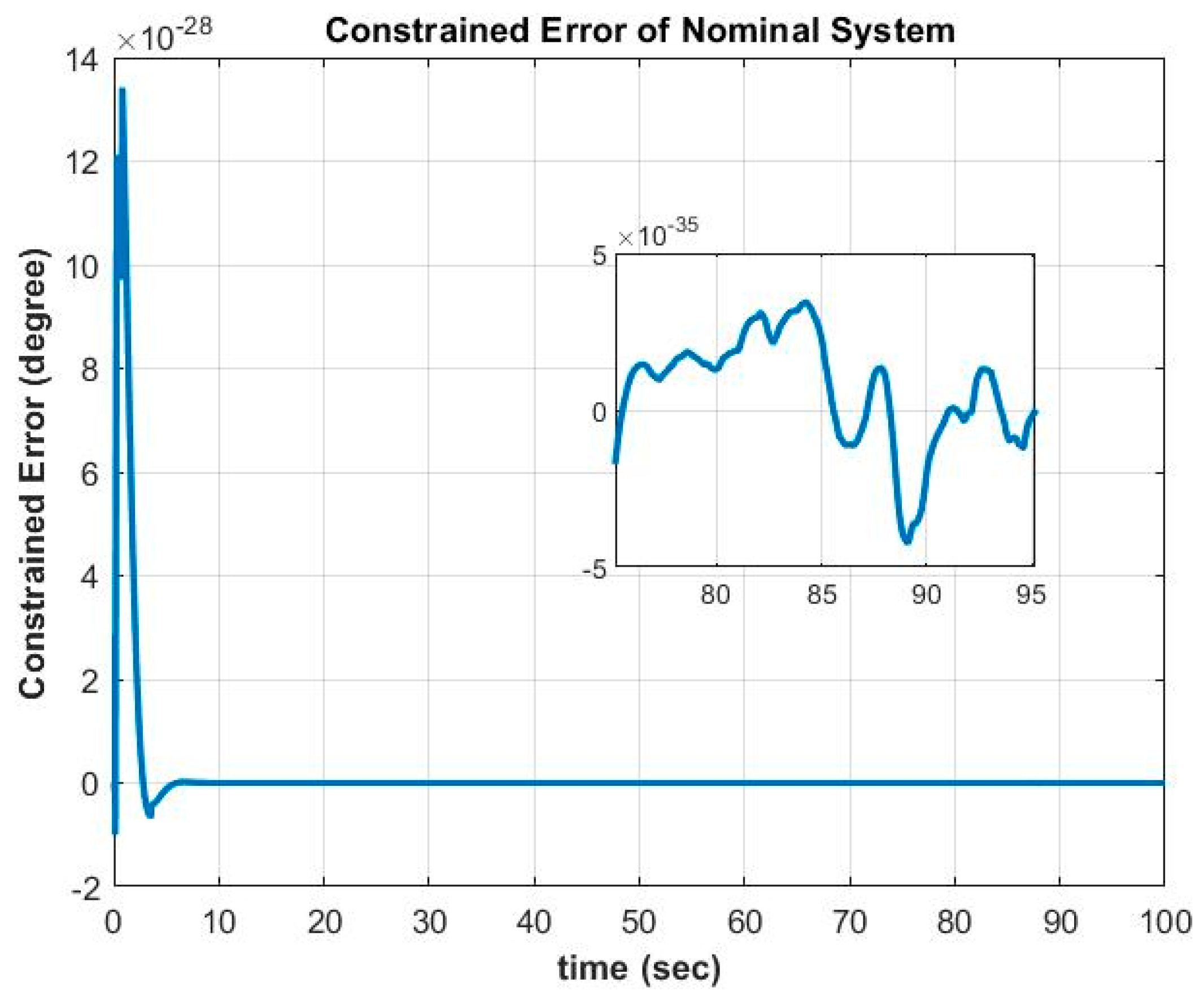
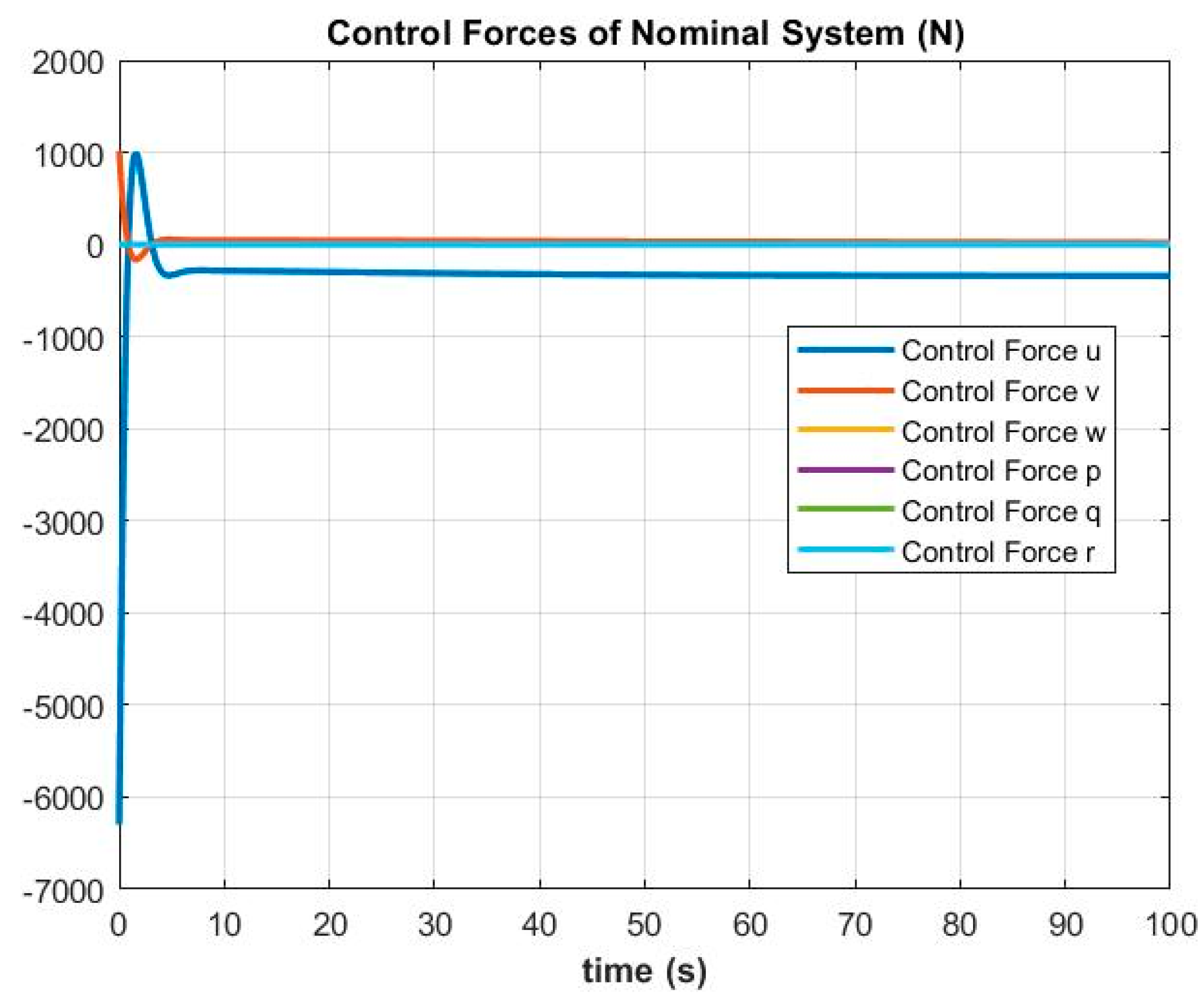
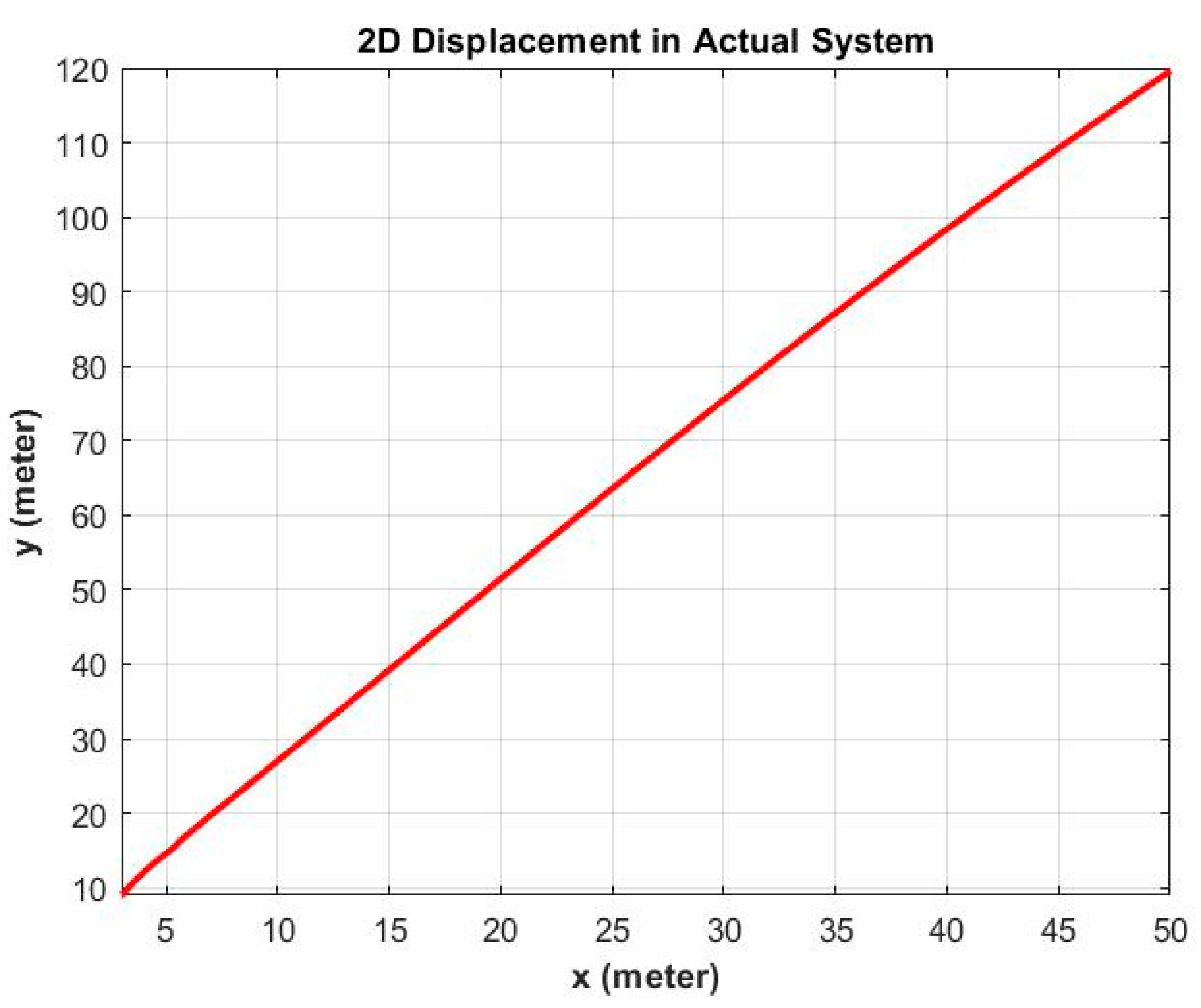
6.1. The Simulation Results for the Constrained Motion in the Nominal System
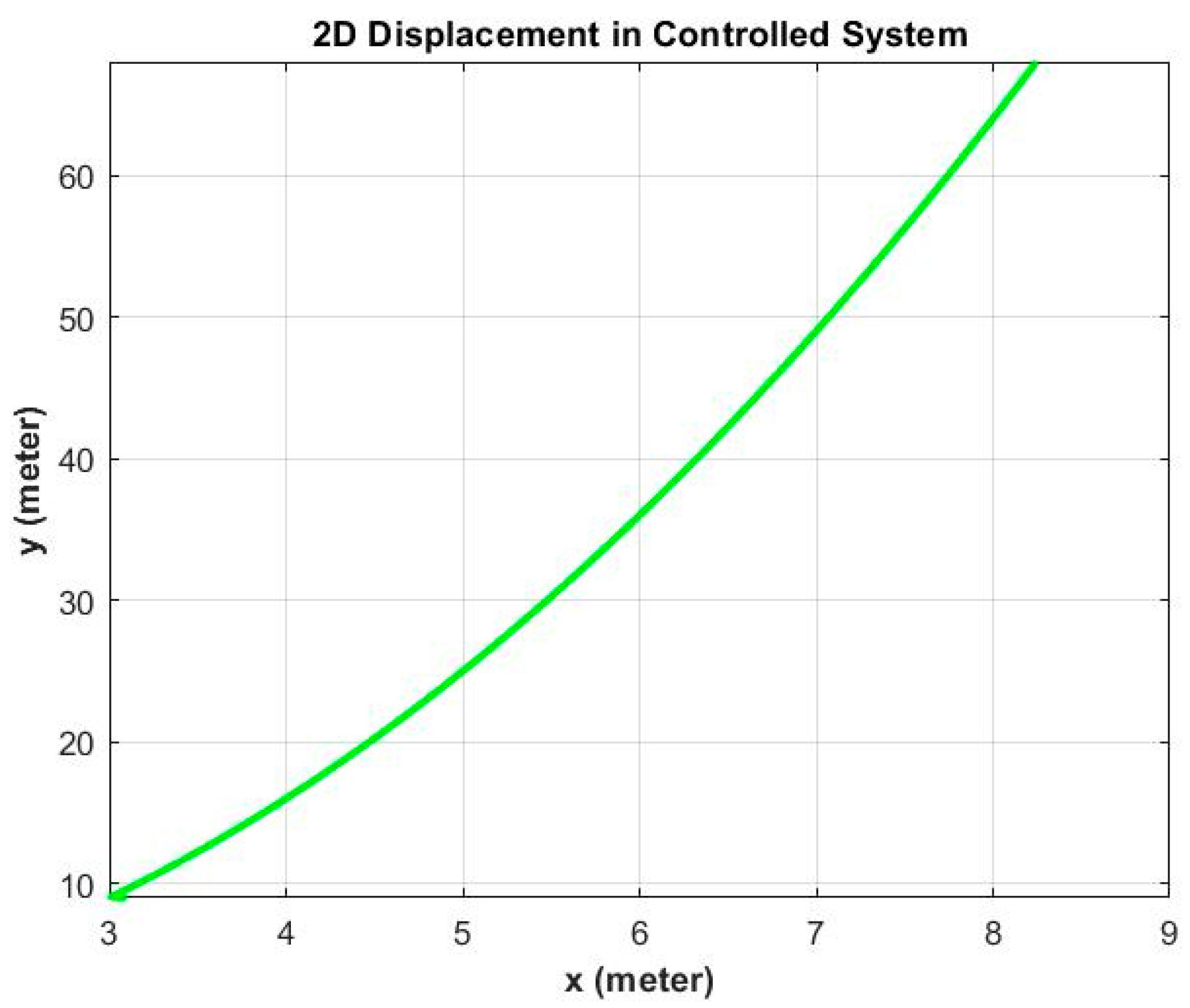
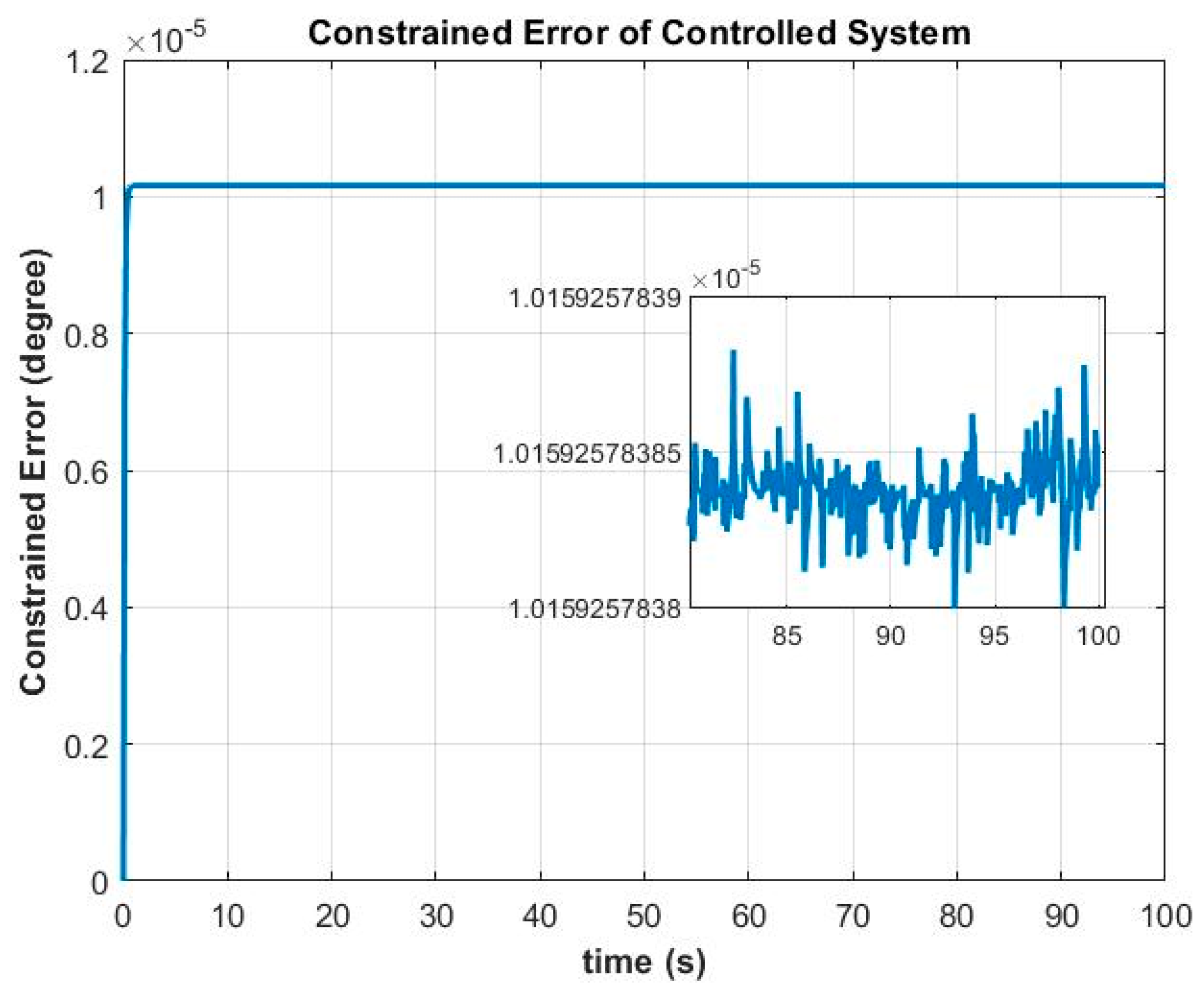
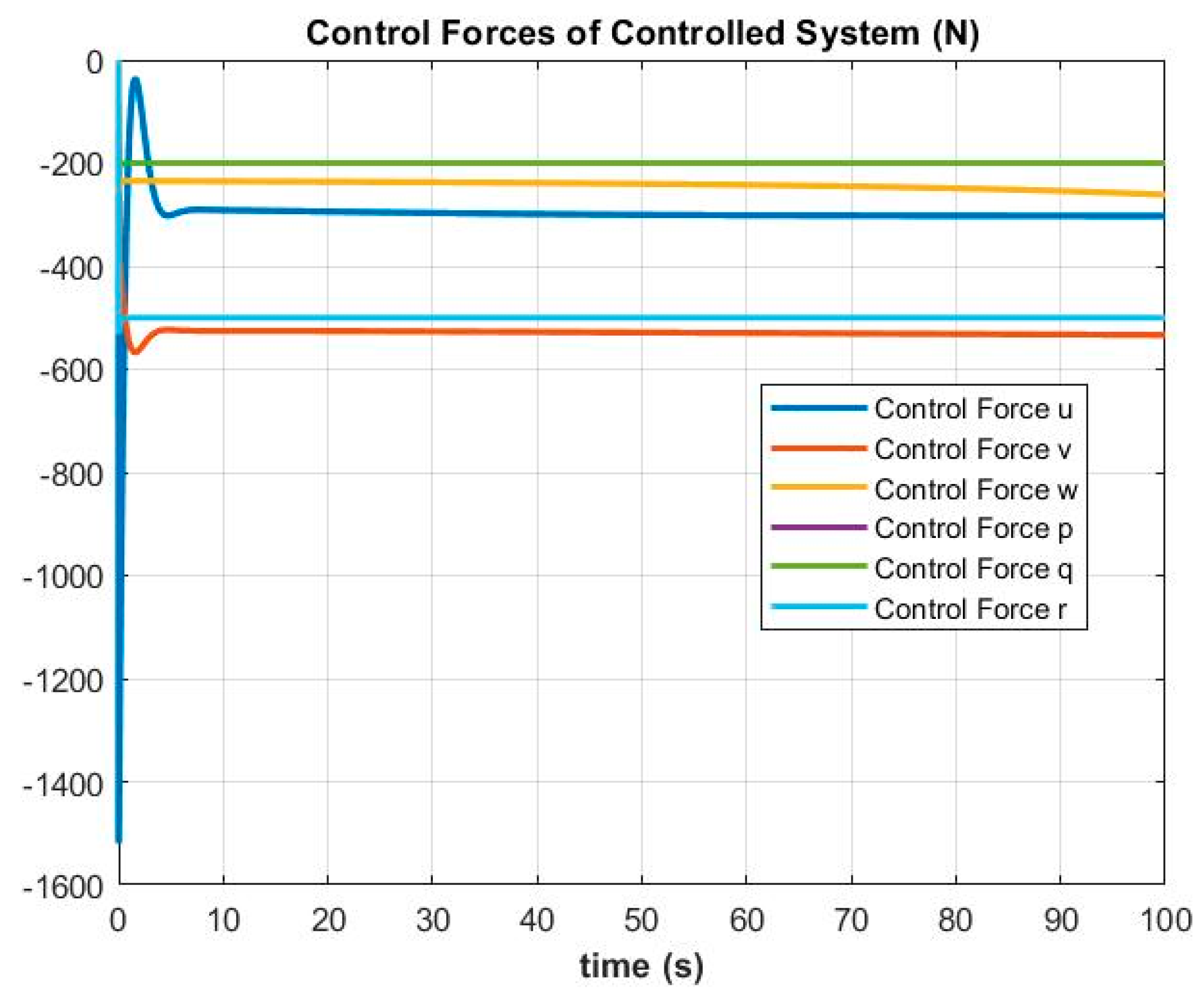
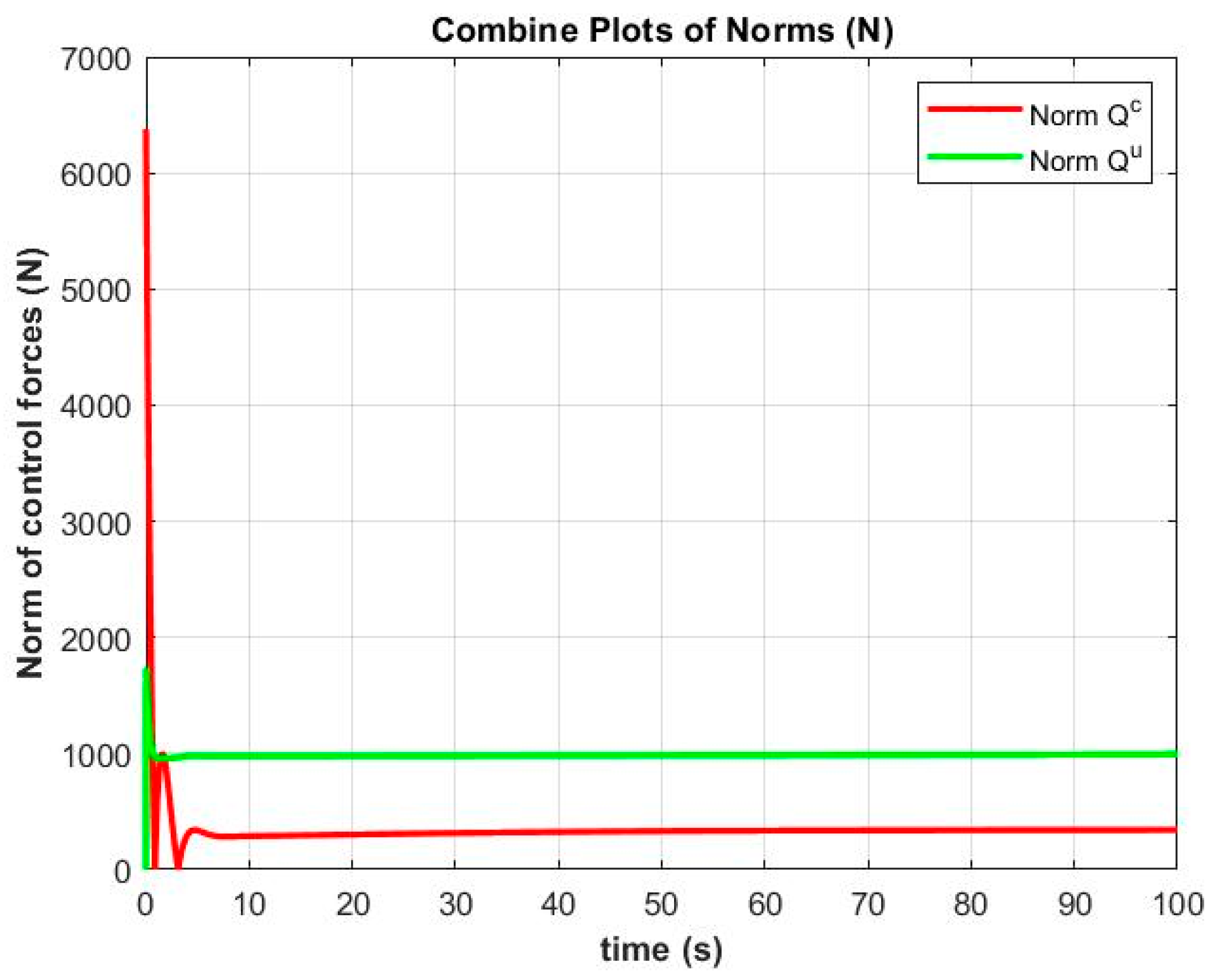
6.2. The Simulation Results for the Constrained Motion in the Controlled System
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pedone, P.; Zizzari, A.A.; Indiveri, G. Path-Following for the Dynamic Model of a Marine Surface Vessel without Closed-Loop Control of the Surge Speed. IFAC Proc. Vol. 2010, 43, 243–248. [Google Scholar] [CrossRef]
- Ashrafiuon, H.; Muske, K.R.; McNinch, L.C.; Soltan, R.A. Sliding-mode tracking control of surface vessels. IEEE Trans. Ind. Electron. 2008, 55, 4004–4012. [Google Scholar] [CrossRef]
- Soltan, R.A.; Ashrafiuon, H.; Muske, K.R. State-dependent trajectory planning and tracking control of unmanned surface vessels. In Proceedings of the 2009 American Control Conference, St. Louis, MO, USA, 10–12 June 2009; pp. 3597–3602. [Google Scholar]
- Du, J.; Guo, C. Nonlinear adaptive ship course tracking control based on backstepping and Nussbaum gain. In Proceedings of the 2004 American Control Conference, Boston, MA, USA, 30 June–2 July 2004; Volume 4, pp. 3845–3850. [Google Scholar]
- Sørensen, A.J. A survey of dynamic positioning control systems. Annu. Rev. Control 2011, 35, 123–136. [Google Scholar] [CrossRef]
- Li, Z.; Sun, J. Disturbance compensating model predictive control with application to ship heading control. IEEE Trans. Control Syst. Technol. 2011, 20, 257–265. [Google Scholar] [CrossRef]
- Chwa, D. Global tracking control of underactuated ships with input and velocity constraints using dynamic surface control method. IEEE Trans. Control Syst. Technol. 2010, 19, 1357–1370. [Google Scholar] [CrossRef]
- Wang, Y.L.; Han, Q.L.; Fei, M.R.; Peng, C. Network-based T–S fuzzy dynamic positioning controller design for unmanned marine vehicles. IEEE Trans. Cybern. 2018, 48, 2750–2763. [Google Scholar] [CrossRef]
- Ye, L.; Zong, Q. Tracking control of an underactuated ship by modified dynamic inversion. ISA Trans. 2018, 83, 100–106. [Google Scholar] [CrossRef]
- Li, D.; Du, L. AUV Trajectory Tracking Models and Control Strategies: A Review. Journal of Marine Science and Engineering. J. Mar. Sci. Eng. 2021, 9, 1020. [Google Scholar] [CrossRef]
- Fossen, T.I. Guidance and Control of Ocean Vehicles. Ph.D. Thesis, University of Trondheim, Trondheim, Norway, 1999. [Google Scholar]
- Chu, P.C.; Fan, C. Prediction of falling cylinder through air-water-sediment columns. ASME J. Appl. Mech. Rev. 2006, 73, 300–314. [Google Scholar] [CrossRef][Green Version]
- Alsos, H.S.; Faltinsen, O.M. 3D motion dynamics of axisymmetric bodies falling through water. Ocean Eng. 2018, 169, 442–456. [Google Scholar] [CrossRef]
- Xiang, G.; Xiang, X. 3D trajectory optimization of the slender body freely falling through water using cuckoo search algorithm. Ocean Eng. 2021, 235, 109354. [Google Scholar] [CrossRef]
- Liu, Z. Ship adaptive course keeping control with nonlinear disturbance observer. IEEE Access. 2017, 5, 17567–17575. [Google Scholar] [CrossRef]
- Le Sourne, H.; Donner, R.; Besnier, F.; Ferry, M. External dynamics of ship-submarine collision. In Proceedings of the Preprints of 2nd International Conference on Collision and Grounding of Ships, Copenhagen, Denmark, 1–3 July 2001; pp. 137–144. [Google Scholar]
- Zhang, N.; Zong, Z. The effect of rigid-body motions on the whipping response of a ship hull subjected to an underwater bubble. J. Fluids Struct. 2011, 27, 1326–1336. [Google Scholar] [CrossRef]
- Chen, X.; Tan, W.W. Tracking control of surface vessels via fault-tolerant adaptive backstepping interval type-2 fuzzy control. Ocean Eng. 2013, 70, 97–109. [Google Scholar] [CrossRef]
- Bui, V.P.; Kim, Y.B. Design of sliding mode controller for ship position control. J. Inst. Control Robot. Syst. 2011, 17, 869–874. [Google Scholar] [CrossRef]
- Fotakis, J.O.H.N.; Grimble, M.; Kouvaritakis, B. A comparison of characteristic locus and optimal designs for dynamic ship positioning systems. IEEE Trans. Automat. Contr. 1982, 27, 1143–1157. [Google Scholar] [CrossRef]
- Borkowski, P. Adaptive system for steering a ship along the desired route. Mathematics. J. Mar. Sci. Eng. 2018, 6, 196. [Google Scholar]
- Udwadia, F.E.; Wanichanon, T. Hamel’s paradox and the foundations of analytical dynamics. Appl. Math. Comput. 2010, 217, 1253–1265. [Google Scholar] [CrossRef]
- Udwadia, F.E.; Wanichanon, T. A New Approach to the Tracking Control of Uncertain Nonlinear Multi-Body Mechanical Systems. In Nonlinear Approaches in Engineering Applications 2; Springer: New York, NY, USA, 2014; pp. 101–136. [Google Scholar]
- Cho, H.; Wanichanon, T.; Udwadia, F.E. New continuous control methodology for nonlinear dynamical systems with uncertain parameters. In Proceedings of the 1st ECCOMAS Thematic Conference on International Conference on Uncertainty Quantification in Computational Sciences and Engineering, Crete, Greece, 25–27 May 2015. [Google Scholar]
- Wanichanon, T.; Udwadia, F.E. Nonlinear damping control for uncertain nonlinear multi-body mechanical systems. J. Res. Appl. 2014, 2, 7–19. [Google Scholar]
- Udwadia, F.E.; Wanichanon, T. Comparison of Methods for Tracking Control of Nonlinear Uncertain Multi-Body Mechanical Systems. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Houston, TX, USA, 9–15 November 2012; American Society of Mechanical Engineers: New York, NY, USA; pp. 921–940. [Google Scholar]
- Wanichanon, T.; Cho, H.; Udwadia, F.E. An effective approach for the control of uncertain systems. In Proceedings of the 23rd ABCM International Congress of Mechanical Engineering, Rio de Janeiro, Brazil, 6–11 December 2015. [Google Scholar]
- Cho, H.; Wanichanon, T.; Udwadia, F.E. Continuous sliding mode controllers for multi-input multi-output systems. Nonlinear Dyn. 2018, 94, 2727–2747. [Google Scholar] [CrossRef]
- Udwadia, F.E.; Wanichanon, T. A closed-form approach to tracking control of nonlinear uncertain systems using the fundamental equation. In Proceedings of the Earth and Space 2012: Engineering, Science, Construction, and Operations in Challenging Environments, Pasadena, CA, USA, 15–18 April 2012; pp. 1339–1348. [Google Scholar]
- Wanichanon, T.; Cho, H.; Udwadia, F.E. An approach to the dynamics and control of uncertain multi-body systems. Procedia IUTAM 2015, 13, 43–52. [Google Scholar] [CrossRef][Green Version]
- Wanichanon, T.; Udwadia, F.E.; Cho, H. Formation-keeping of uncertain satellites using nonlinear damping control. J. Res. Appl. 2014, 2, 20–33. [Google Scholar]
- Udwadia, F.E.; Kalaba, R.E. Equations of motion for mechanical systems. J. Aerosp. Eng. 1996, 9, 64–69. [Google Scholar] [CrossRef]
- Udwadia, F.E.; Wanichanon, T. Explicit equation of motion of constrained systems. In Nonlinear Approaches in Engineering Applications; Springer: New York, NY, USA, 2012; pp. 315–347. [Google Scholar]
- Udwadia, F.E.; Wanichanon, T. Control of uncertain nonlinear multibody mechanical systems. J. Appl. Mech. 2014, 81, 1–39. [Google Scholar] [CrossRef]
- Fossen, T.I. Marine Control Systems–Guidance. Navigation, and Control of Ships, Rigs and Underwater Vehicles; Marine Cybernetics: Trondheim, Norway, 2002. [Google Scholar]
- Hammad, M.M.; Elshenawy, A.K.; El Singaby, M.I. Trajectory following and stabilization control of fully actuated AUV using inverse kinematics and self-tuning fuzzy PID. PLoS ONE 2017, 12, e0179611. [Google Scholar] [CrossRef]
- Pettersen, K.Y.; Nijmeijer, H. Semi-global practical stabilization and disturbance adaptation for an underactuated ship. In Proceedings of the 39th IEEE Conference on Decision and Control, Sydney, Australia, 12–15 December 2000; pp. 2144–2149. [Google Scholar]



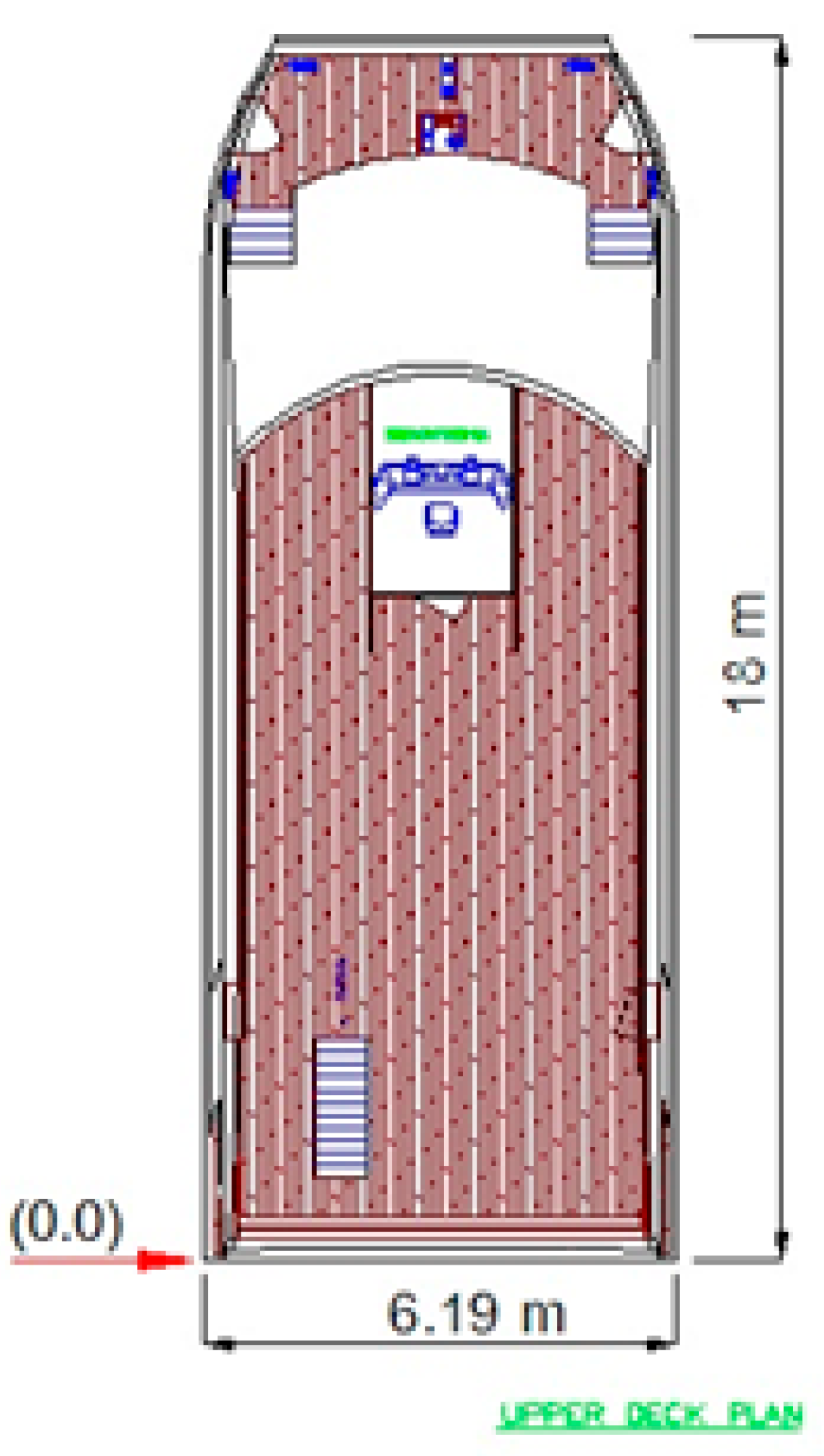


| Parameter | Units | Value |
|---|---|---|
| Length of Hull | m | 18.00 |
| Beam of Hull | m | 6.19 |
| Maximum Depth | m | 3.24 |
| Maximum Draught | m | 1.33 |
| Volume Displacement | m3 | 35.054 |
| Weight | kg | 33,000.00 |
| Block Coefficient | - | 0.640 |
| Water Plane Area | m2 | 49.24 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aumtab, C.; Wanichanon, T. Stability and Tracking Control of Nonlinear Rigid-Body Ship Motions. J. Mar. Sci. Eng. 2022, 10, 153. https://doi.org/10.3390/jmse10020153
Aumtab C, Wanichanon T. Stability and Tracking Control of Nonlinear Rigid-Body Ship Motions. Journal of Marine Science and Engineering. 2022; 10(2):153. https://doi.org/10.3390/jmse10020153
Chicago/Turabian StyleAumtab, Chatchawan, and Thanapat Wanichanon. 2022. "Stability and Tracking Control of Nonlinear Rigid-Body Ship Motions" Journal of Marine Science and Engineering 10, no. 2: 153. https://doi.org/10.3390/jmse10020153
APA StyleAumtab, C., & Wanichanon, T. (2022). Stability and Tracking Control of Nonlinear Rigid-Body Ship Motions. Journal of Marine Science and Engineering, 10(2), 153. https://doi.org/10.3390/jmse10020153





