Experimental Investigation on the Characteristic of Hydrodynamic-Acoustic Cavitation (HAC)
Abstract
:1. Introduction
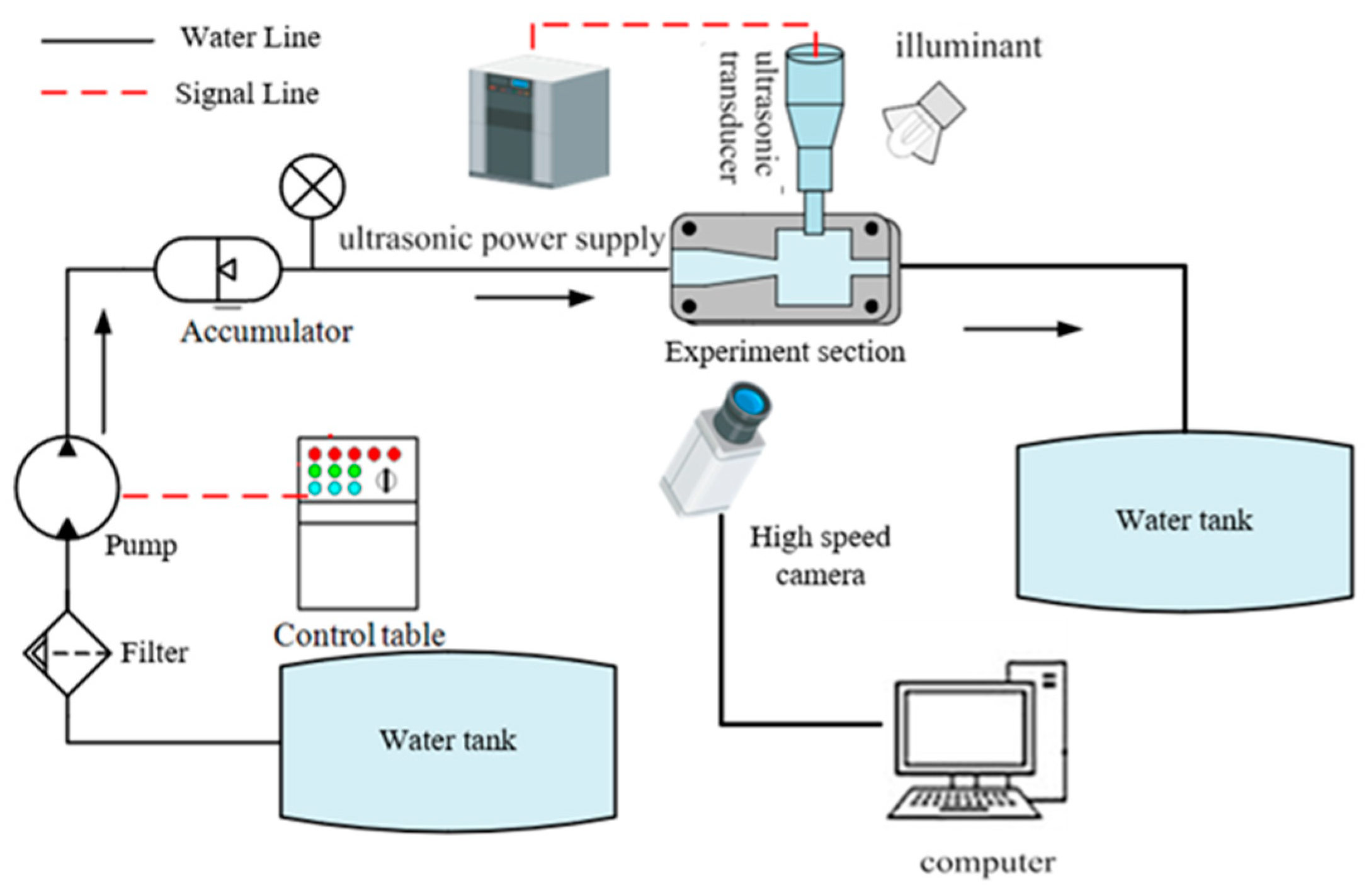
2. Experiment and Methods
2.1. Experimental Setup
2.2. Experimental Setup
3. Result and Discussion
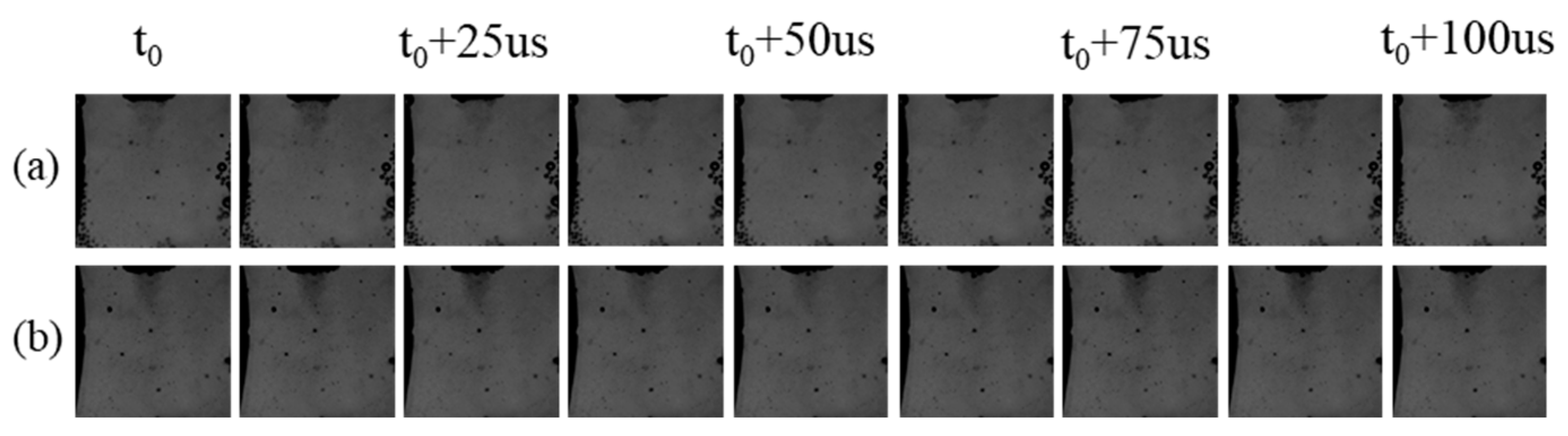
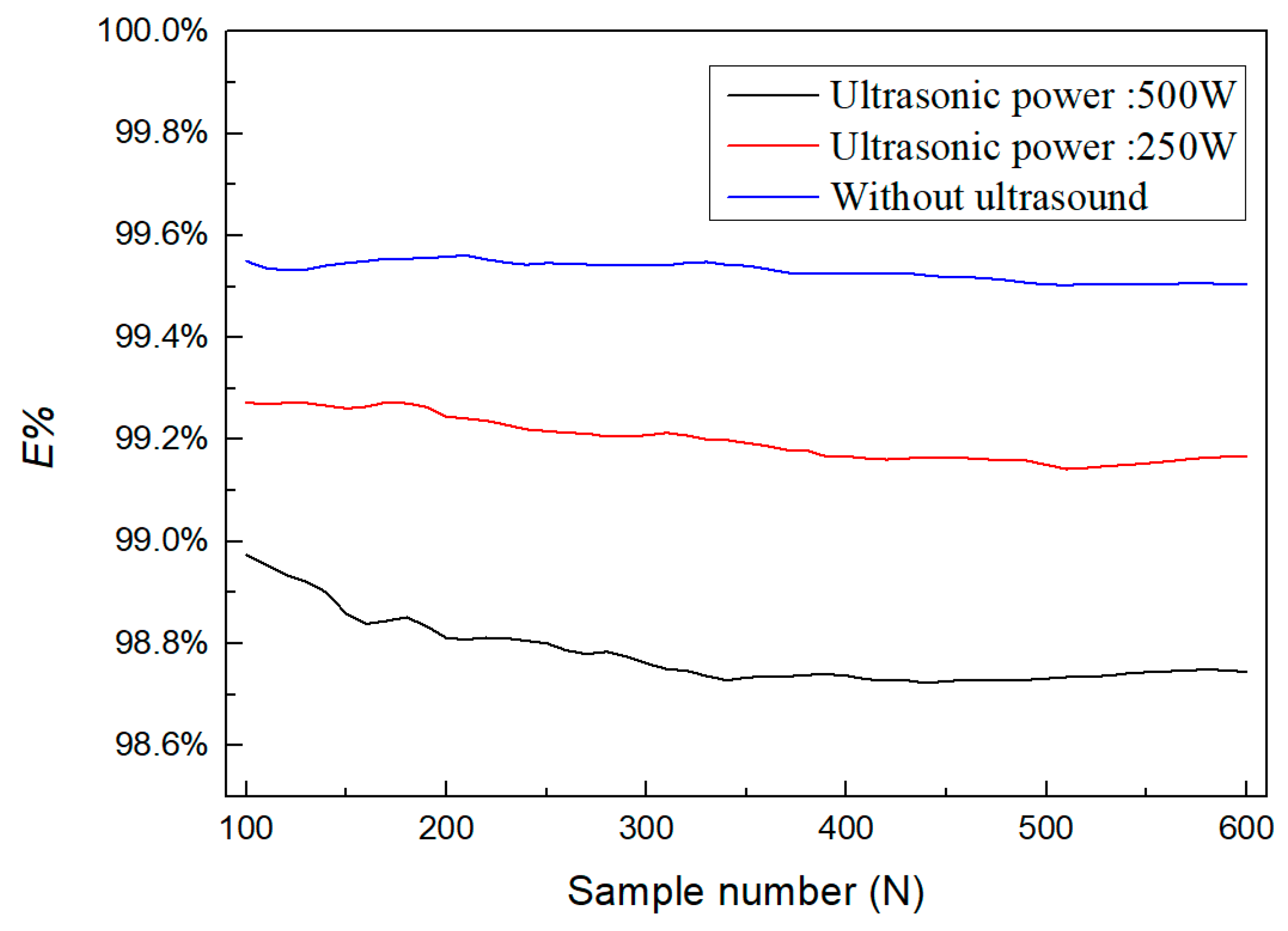
3.1. The Cavitation Performance Governed by Different Methods
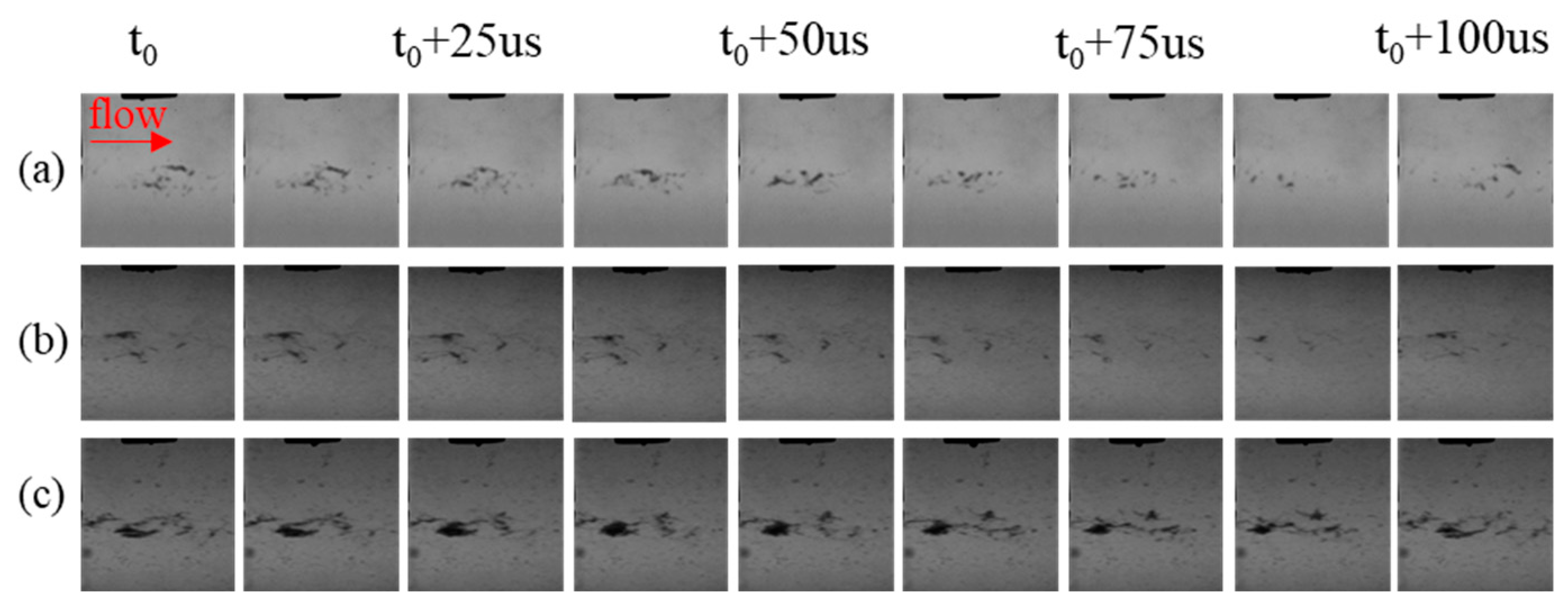
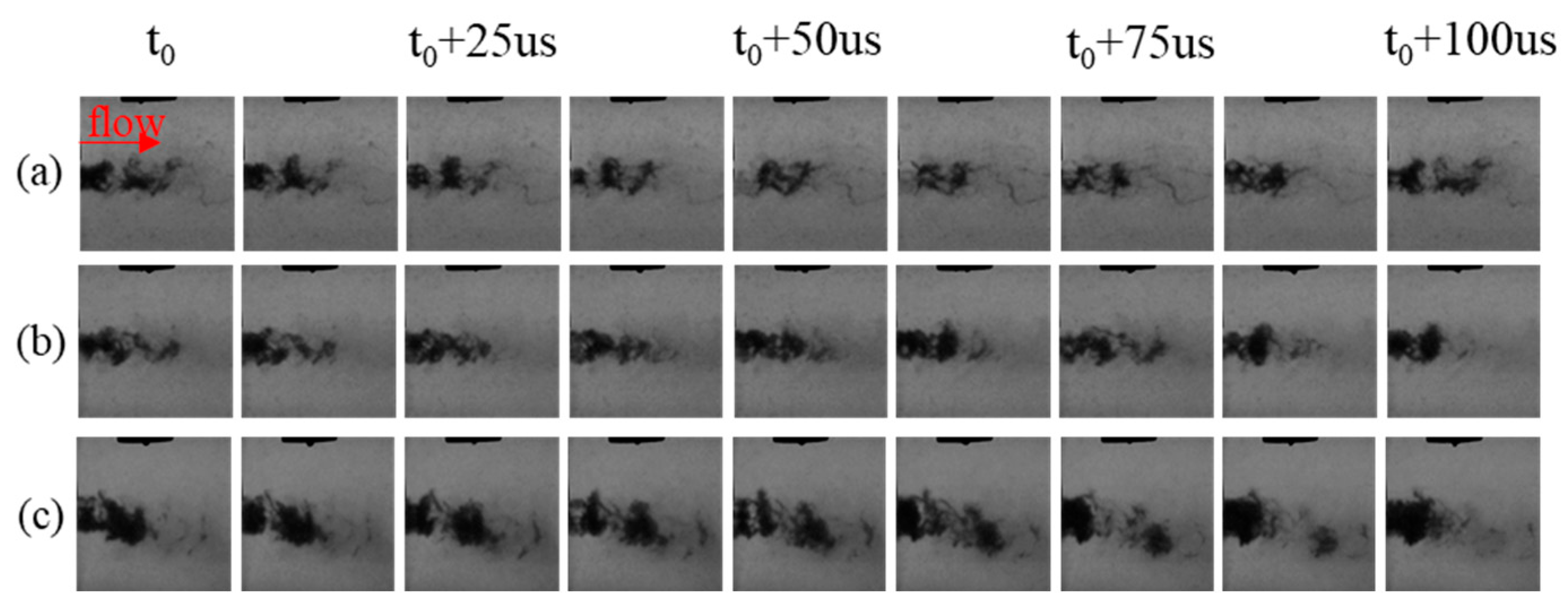
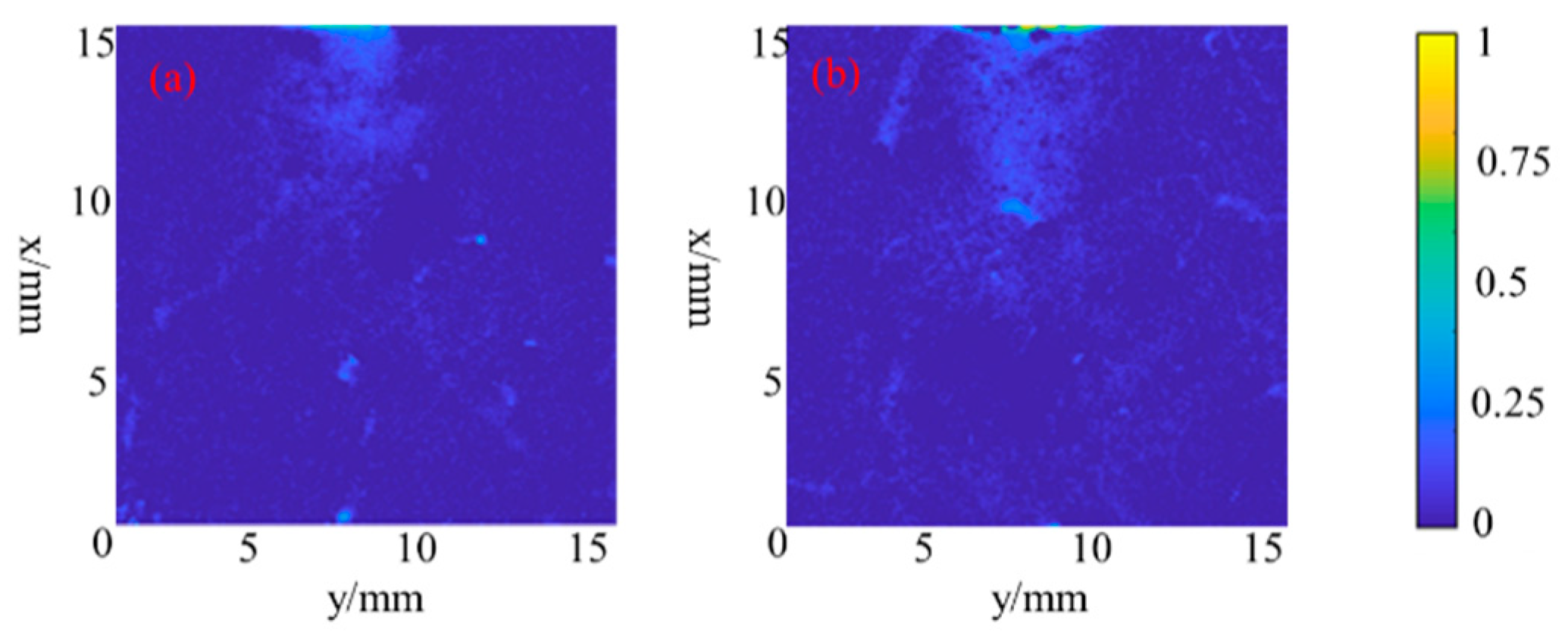
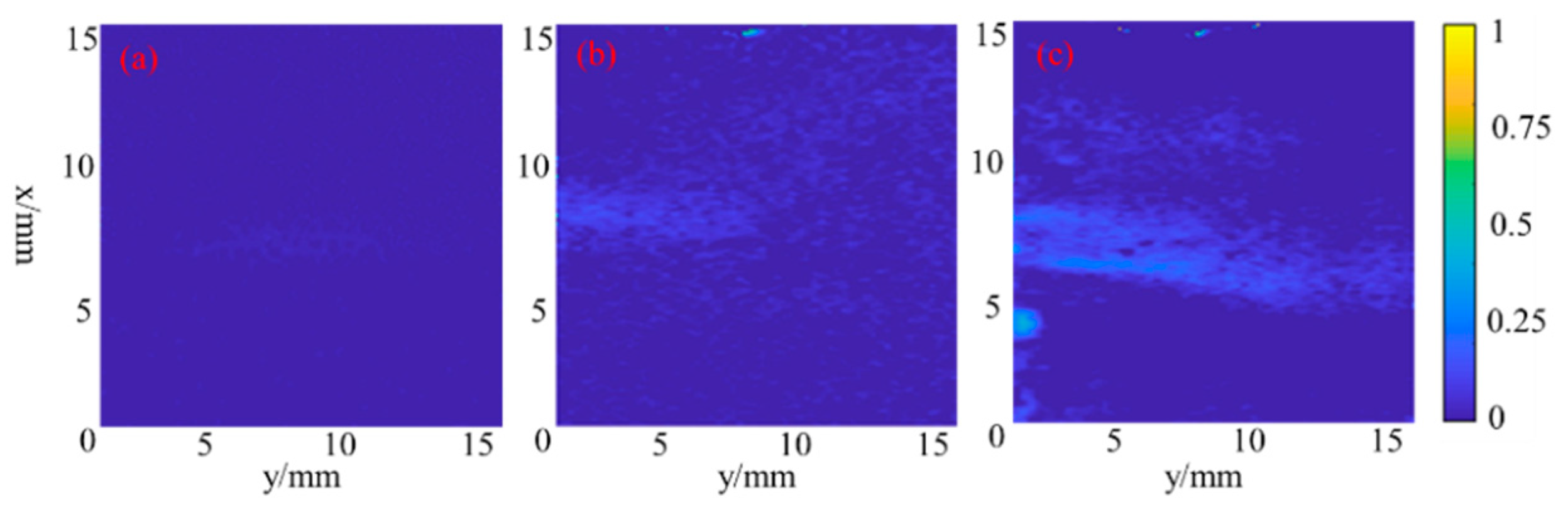
3.2. The Spatial Distribution of Cavitation Cloud
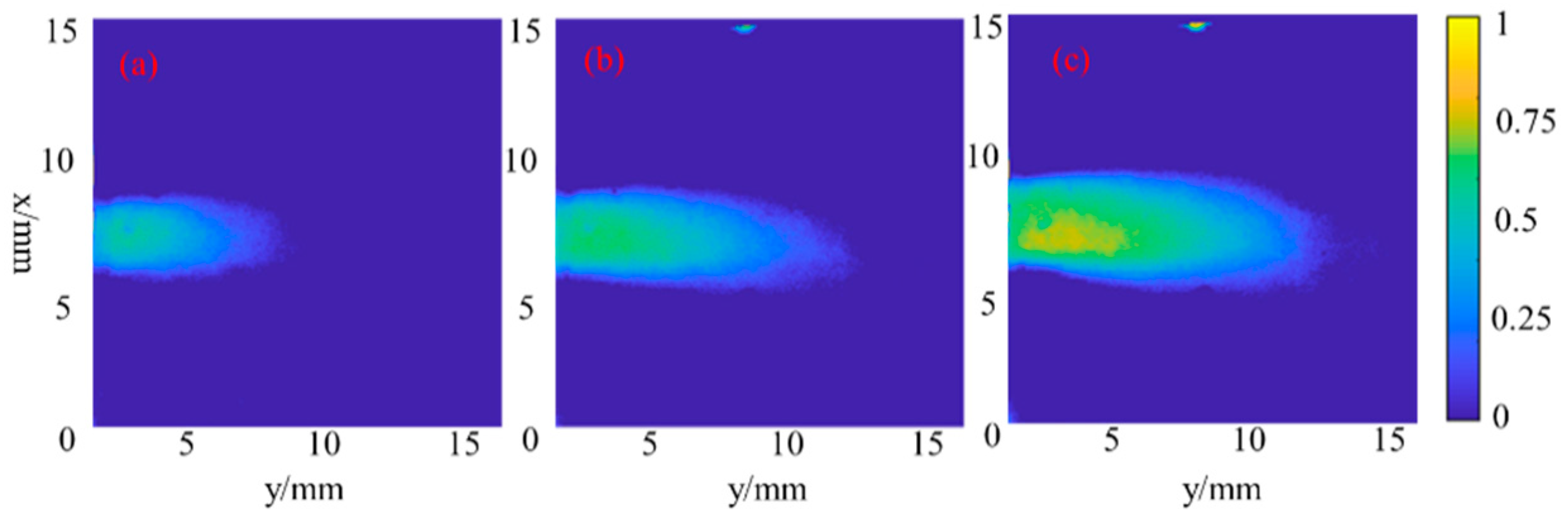
3.3. The Spatial-Temporal Profiles of Cavitation Cloud
3.4. POD Analysis
4. Conclusions
- The vapor phase volume fraction in the rectangular cavity of hydrodynamic-acoustic cavitation is significantly higher than those of ultrasonic cavitation independently and hydraulic cavitation independently, thereby indicating the synergy of hydraulic cavitation and ultrasonic cavitation. When the inlet pressure is 2 MPa, the average value of the volume-averaging cavitation intensity variable is 0.029, 0.058, and 0.092, respectively, and the corresponding growth rate is 95% and 58.5%.
- The average collapse position of Hydraulic cavitation and hydrodynamic-acoustic cavitation and the increasing gap between the maximum value and the minimum value employed in this paper shows that due to the existence of ultrasound, the periodic shedding and collapse of cavitation clouds is more violent.
- Based on the POD analysis, the ultrasound was not altered the large-scale vortex structure in the flow field. The high-frequency pressure pulsation of ultrasound strengthens the instability exhibited by the shear layer and induces small-scale vortex structures at the shear layer, which is suggested as the more violently shed and collapse.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dular, M.; Griessler-Bulc, T.; Gutierrez-Aguirre, I.; Heath, E.; Kosjek, T.; Klemenčič, A.K.; Oder, M.; Petkovšek, M.; Rački, N.; Ravnikar, M.; et al. Use of hydrodynamic cavitation in (waste)water treatment. Ultrason. Sonochem. 2016, 29, 577–588. [Google Scholar] [CrossRef] [PubMed]
- Eskin, D.; Tzanakis, I.; Wang, F.; Lebon, G.; Subroto, T.; Pericleous, K.; Mi, J. Fundamental studies of ultrasonic melt processing. Ultrason. Sonochem. 2018, 52, 455–467. [Google Scholar] [CrossRef] [PubMed]
- Bjorndalen, N.; Islam, M. The effect of microwave and ultrasonic irradiation on crude oil during production with a horizontal well. J. Pet. Sci. Eng. 2004, 43, 139–150. [Google Scholar] [CrossRef]
- Antes, F.G.; Diehl, L.O.; Pereira, J.S.; Guimarães, R.C.; Guarnieri, R.A.; Ferreira, B.M.; Flores, E.M. Effect of ultrasonic frequency on separation of water from heavy crude oil emulsion using ultrasonic baths. Ultrason. Sonochem. 2017, 35, 541–546. [Google Scholar] [CrossRef]
- Fedotkin, I.; Oleg, J. Some Problems of Development of Cavitation Technologies for Industry Application. Ned Tijdschr Geneeskd 2001, 145, 1632–1637. [Google Scholar]
- Long, X.; Cheng, H.; Ji, B.; Arndt, R.E.; Peng, X. Large eddy simulation and Euler–Lagrangian coupling investigation of the transient cavitating turbulent flow around a twisted hydrofoil. Int. J. Multiph. Flow 2018, 100, 41–56. [Google Scholar] [CrossRef]
- Wang, Y.; Zhuang, S.; Liu, H.; Zhao, Z.; Dular, M. Image post-processed approaches for cavitating flow in orifice plate. J. Mech. Sci. Technol. 2017, 31, 3305–3315. [Google Scholar] [CrossRef]
- Wittekind, D.; Schuster, M. Propeller cavitation noise and background noise in the sea. Ocean Eng. 2016, 120, 116–121. [Google Scholar] [CrossRef]
- Brennen, C.E. Cavitation and Bubble Dynamics; Oxford University Press: Oxford, UK, 1995. [Google Scholar]
- Guild, F.J.; Kinloch, A.J.; Taylor, A.C. Particle cavitation in rubber toughened epoxies: The role of particle size. J. Mater. Sci. 2010, 45, 3882–3894. [Google Scholar] [CrossRef] [Green Version]
- Schiffers, W.; Shaw, S.; Emmony, D. Acoustical and optical tracking of the collapse of a laser-generated cavitation bubble near a solid boundary. Ultrasonics 1998, 36, 559–563. [Google Scholar] [CrossRef]
- Arndt, R.E.A. Cavitation in Fluid Machinery and Hydraulic Structures. Annu. Rev. Fluid Mech. 1981, 13, 273–326. [Google Scholar] [CrossRef]
- Chen, H.; Jiang, L.; Chen, D.; Jiadao, W. Damages on steel surface at the incubation stage of the vibration cavitation erosion in water. Wear 2008, 266, 69–75. [Google Scholar]
- Hutli, E.; Nedeljkovic, M.S.; Radovic, N.A.; Bonyár, A. The relation between the high speed submerged cavitating jet behaviour and the cavitation erosion process. Int. J. Multiph. Flow 2016, 83, 27–38. [Google Scholar] [CrossRef] [Green Version]
- Tzanakis, I.; Bolzoni, L.; Eskin, D.G.; Hadfield, M. Evaluation of cavitation erosion behavior of commercial steel grades used in the design of fluid machinery. Metall. Mater. Trans. A 2017, 48, 2193–2206. [Google Scholar] [CrossRef] [Green Version]
- Mettin, R.; Cairós, C. Handbook of Ultrasonics and Sonochemistry; Springer: Singapore, 2016. [Google Scholar]
- Flannigan, D.; Suslick, K. Plasma formation and temperature measurement during single-bubble cavitation. Nature 2005, 434, 52–55. [Google Scholar] [CrossRef]
- Ma, X.; Huang, B.; Zhao, X.; Wang, Y.; Chang, Q.; Qiu, S.; Fu, X.; Wang, G. Comparisons of spark-charge bubble dynamics near the elastic and rigid boundaries. Ultrason. Sonochem. 2018, 43, 80–90. [Google Scholar] [CrossRef]
- Peng, C.; Tian, S.; Li, G.; Wei, M. Enhancement of cavitation intensity and erosion ability of submerged cavitation jet by adding micro-particles. Ocean Eng. 2020, 209, 107516. [Google Scholar] [CrossRef]
- Yasuda, K.; Nguyen, T.T.; Asakura, Y. Measurement of distribution of broadband noise and sound pressures in sonochemical reactor. Ultrason. Sonochem. 2018, 43, 23–28. [Google Scholar] [CrossRef]
- Henglein, A.; Kormann, C. Scavenging of OH Radicals Produced in the Sonolysis of Water. Int. J. Radiat. Biol. Relat. Stud. Phys. Chem. Med. 1985, 48, 251–258. [Google Scholar] [CrossRef]
- Olson, T.M.; Barbier, P.F. Oxidation kinetics of natural organic matter by sonolysis and ozone. Water Res. 1994, 28, 1383–1391. [Google Scholar] [CrossRef]
- Stock, N.L.; Peller, J.; Vinodgopal, K.; Kamat, P.V. Combinative Sonolysis and Photocatalysis for Textile Dye Degradation. Environ. Sci. Technol. 2000, 34, 1747–1750. [Google Scholar] [CrossRef]
- Davydov, L.; Reddy, E.P.; France, P.; Smirniotis, P.G. Sonophotocatalytic destruction of organic contaminants in aqueous systems on TiO2 powders. Appl. Catal. B Environ. 2001, 32, 95–105. [Google Scholar] [CrossRef]
- Chahine, G.L.; Kapahi, A.; Choi, J.-K.; Hsiao, C.-T. Modeling of surface cleaning by cavitation bubble dynamics and collapse. Ultrason. Sonochem. 2016, 29, 528–549. [Google Scholar] [CrossRef]
- Morton, J.A.; Khavari, M.; Qin, L.; Maciejewska, B.M.; Tyurnina, A.V.; Grobert, N.; Eskin, D.G.; Mi, J.; Porfyrakis, K.; Prentice, P.; et al. New insights into sono-exfoliation mechanisms of graphite: In situ high-speed imaging studies and acoustic measurements. Mater. Today 2021, 49, 10–22. [Google Scholar] [CrossRef]
- Pandit, A.B.; Sivakumar, P.S. Improve Reactions with Hydrodynamic Cavitation. Chem. Eng. Prog. 1999, 95, 43–50. [Google Scholar]
- Asgharzadehahmadi, S.; Raman, A.A.A.; Parthasarathy, R.; Sajjadi, B. Sonochemical reactors: Review on features, advantages and limitations. Renew. Sustain. Energy Rev. 2016, 63, 302–314. [Google Scholar] [CrossRef]
- Jyoti, K.K.; Pandit, A.B. Water disinfection by acoustic and hydrodynamic cavitation. Biochem. Eng. J. 2001, 7, 201–212. [Google Scholar] [CrossRef]
- Kumar, P.S.; Pandit, A.B. Modeling Hydrodynamic Cavitation. Chem. Eng. Technol. 1999, 22, 1017–1027. [Google Scholar] [CrossRef]
- Pandit, A.B.; Joshi, J.B. hydrolysis of fatty oils: Effect of cavitation. Chem. Eng. Sci. 1993, 48, 3440–3442. [Google Scholar] [CrossRef]
- Kalumuck, K.M.; Chahine, G.L. The Use of Cavitating Jets to Oxidize Organic Compounds in Water. J. Fluids Eng. 2000, 122, 465–470. [Google Scholar] [CrossRef] [Green Version]
- Gogate, P.R.; Sutkar, V.S.; Pandit, A.B. Sonochemical reactors: Important design and scale up considerations with a special emphasis on heterogeneous systems. Chem. Eng. J. 2011, 166, 1066–1082. [Google Scholar] [CrossRef]
- Chatterjee, D.; Arakeri, V.H. Towards the concept of hydrodynamic cavitation control. J. Fluid Mech. 1997, 332, 377–394. [Google Scholar] [CrossRef]
- Jyoti, K.K.; Pandit, A.B. Hybrid cavitation methods for water disinfection. Biochem. Eng. J. 2003, 14, 9–17. [Google Scholar] [CrossRef]
- Amin, L.P.; Gogate, P.R.; Burgess, A.E.; Bremner, D.H. Optimization of a hydrodynamic cavitation reactor using salicylic acid dosimetry. Chem. Eng. J. 2010, 156, 165–169. [Google Scholar] [CrossRef] [Green Version]
- Franke, M.; Braeutigam, P.; Wu, Z.-L.; Ren, Y.; Ondruschka, B. Enhancement of chloroform degradation by the combination of hydrodynamic and acoustic cavitation. Ultrason. Sonochem. 2011, 18, 888–894. [Google Scholar] [CrossRef]
- Braeutigam, P.; Franke, M.; Schneider, R.J.; Lehmann, A.; Stolle, A.; Ondruschka, B. Degradation of carbamazepine in environmentally relevant concentrations in water by Hydrodynamic-Acoustic-Cavitation (HAC). Water Res. 2012, 46, 2469–2477. [Google Scholar] [CrossRef]
- Foldyna, J.; Sitek, L.; Švehla, B.; Švehla, Š. Utilization of ultrasound to enhance high-speed water jet effects. Ultrason. Sonochem. 2004, 11, 131–137. [Google Scholar] [CrossRef]
- Johansson, Ö.; Pamidi, T.; Shankar, V. Extraction of tungsten from scheelite using hydrodynamic and acoustic cavitation. Ultrasonics Sonochem. 2020, 71, 105408. [Google Scholar] [CrossRef]
- Wu, P.; Bai, L.; Lin, W.; Wang, X. Mechanism and dynamics of hydrodynamic-acoustic cavitation (HAC). Ultrason. Sonochem. 2018, 49, 89–96. [Google Scholar] [CrossRef]
- Aeschlimann, V.; Prothin, S.; Barre, S.; Djeridi, H. High speed visualizations of the cavitating vortices of 2D mixing layer. Eur. J. Mech.—B/Fluids 2012, 31, 171–180. [Google Scholar] [CrossRef]
- Wu, Q.; Wei, W.; Deng, B.; Jiang, P.; Li, D.; Zhang, M.; Fang, Z. Dynamic characteristics of the cavitation clouds of submerged Helmholtz self-sustained oscillation jets from high-speed photography. J. Mech. Sci. Technol. 2019, 33, 621–630. [Google Scholar] [CrossRef]
- Liu, W.; Kang, Y.; Wang, X.; Liu, Q.; Fang, Z. Integrated CFD-aided theoretical demonstration of cavitation modulation in self-sustained oscillating jets. Appl. Math. Model. 2020, 79, 521–543. [Google Scholar] [CrossRef]
- Holt, R.G.; Crum, L.A. Mie scattering used to determine spherical bubble oscillations. Appl. Opt. 1990, 29, 4182–4191. [Google Scholar] [CrossRef]
- Tuziuti, T.; Yasui, K.; Iida, Y. Spatial study on a multibubble system for sonochemistry by laser-light scattering. Ultrason. Sonochem. 2005, 12, 73–77. [Google Scholar] [CrossRef]
- Ganesh, H.; Mäkiharju, S.A.; Ceccio, S.L. Bubbly shock propagation as a mechanism for sheet-to-cloud transition of partial cavities. J. Fluid Mech. 2016, 802, 37–78. [Google Scholar] [CrossRef] [Green Version]
- Lumley, J.L. The structure of inhomogeneous turbulence. In Atmospheric Turbulence & Radio Wave Propagation; Nauka: Moscow, Russia, 1967. [Google Scholar]
- Sirovich, L. Turbulence and the dynamics of coherent structures. I—Coherent structures. II—Symmetries and transformations. III—Dynamics and scaling. Q. Appl. Math. 1987, 45, 561–571. [Google Scholar] [CrossRef] [Green Version]
- Wang, P.; Liu, Y. Intensified flow dynamics by second-order acoustic standing-wave mode: Vortex-excited acoustic resonances in channel branches. Phys. Fluids 2019, 31, 035105. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, M.; Kang, Y.; Shi, H.; Li, D.; Li, H. Experimental Investigation on the Characteristic of Hydrodynamic-Acoustic Cavitation (HAC). J. Mar. Sci. Eng. 2022, 10, 309. https://doi.org/10.3390/jmse10030309
Yuan M, Kang Y, Shi H, Li D, Li H. Experimental Investigation on the Characteristic of Hydrodynamic-Acoustic Cavitation (HAC). Journal of Marine Science and Engineering. 2022; 10(3):309. https://doi.org/10.3390/jmse10030309
Chicago/Turabian StyleYuan, Miao, Yong Kang, Hanqing Shi, Dezheng Li, and Hongchao Li. 2022. "Experimental Investigation on the Characteristic of Hydrodynamic-Acoustic Cavitation (HAC)" Journal of Marine Science and Engineering 10, no. 3: 309. https://doi.org/10.3390/jmse10030309
APA StyleYuan, M., Kang, Y., Shi, H., Li, D., & Li, H. (2022). Experimental Investigation on the Characteristic of Hydrodynamic-Acoustic Cavitation (HAC). Journal of Marine Science and Engineering, 10(3), 309. https://doi.org/10.3390/jmse10030309




