Underwater Absorber for a Remotely Operated Vehicle
Abstract
:1. Introduction
2. Design of Underwater Absorber
2.1. Design Composition
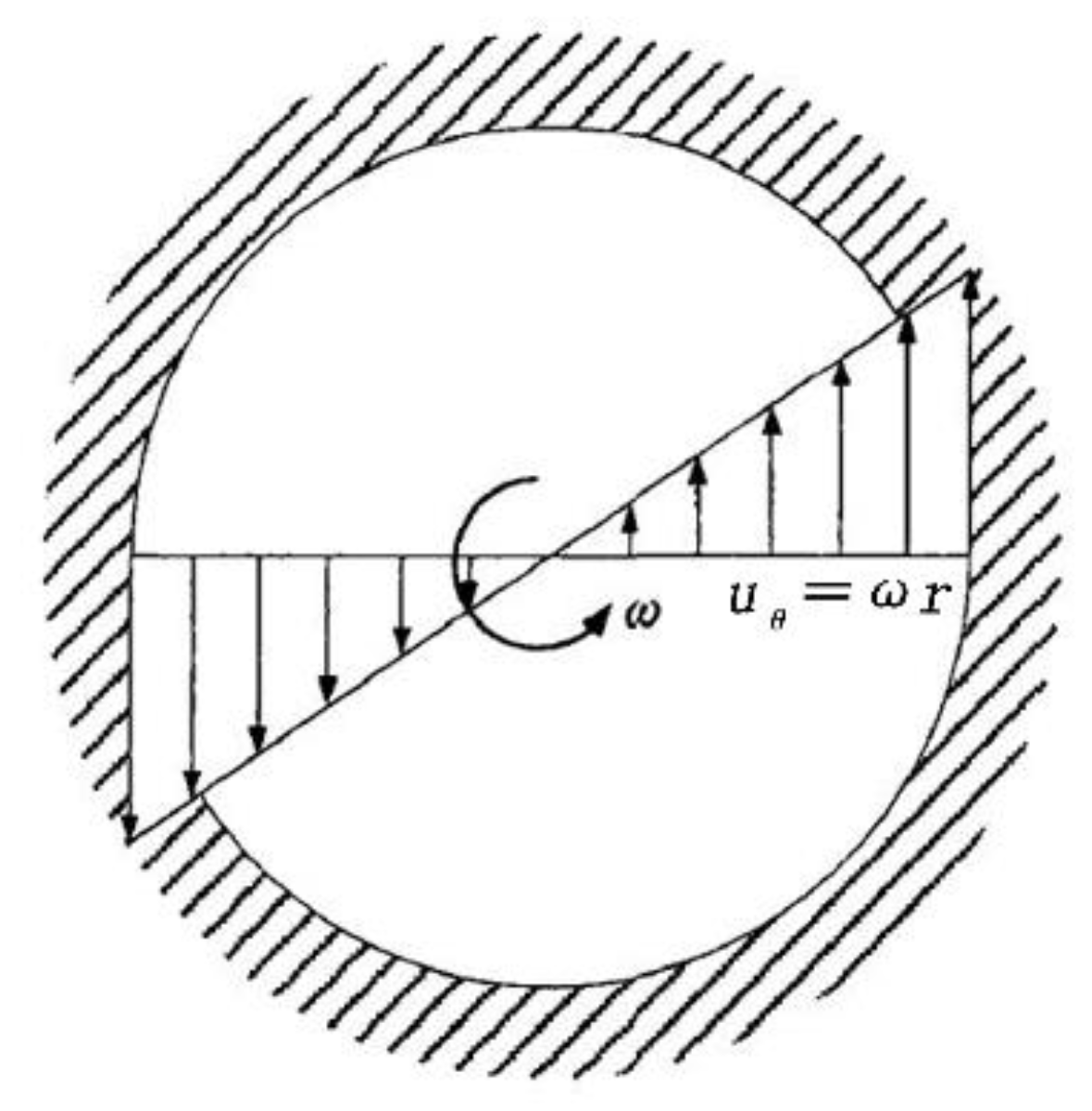
2.2. Operating Principle
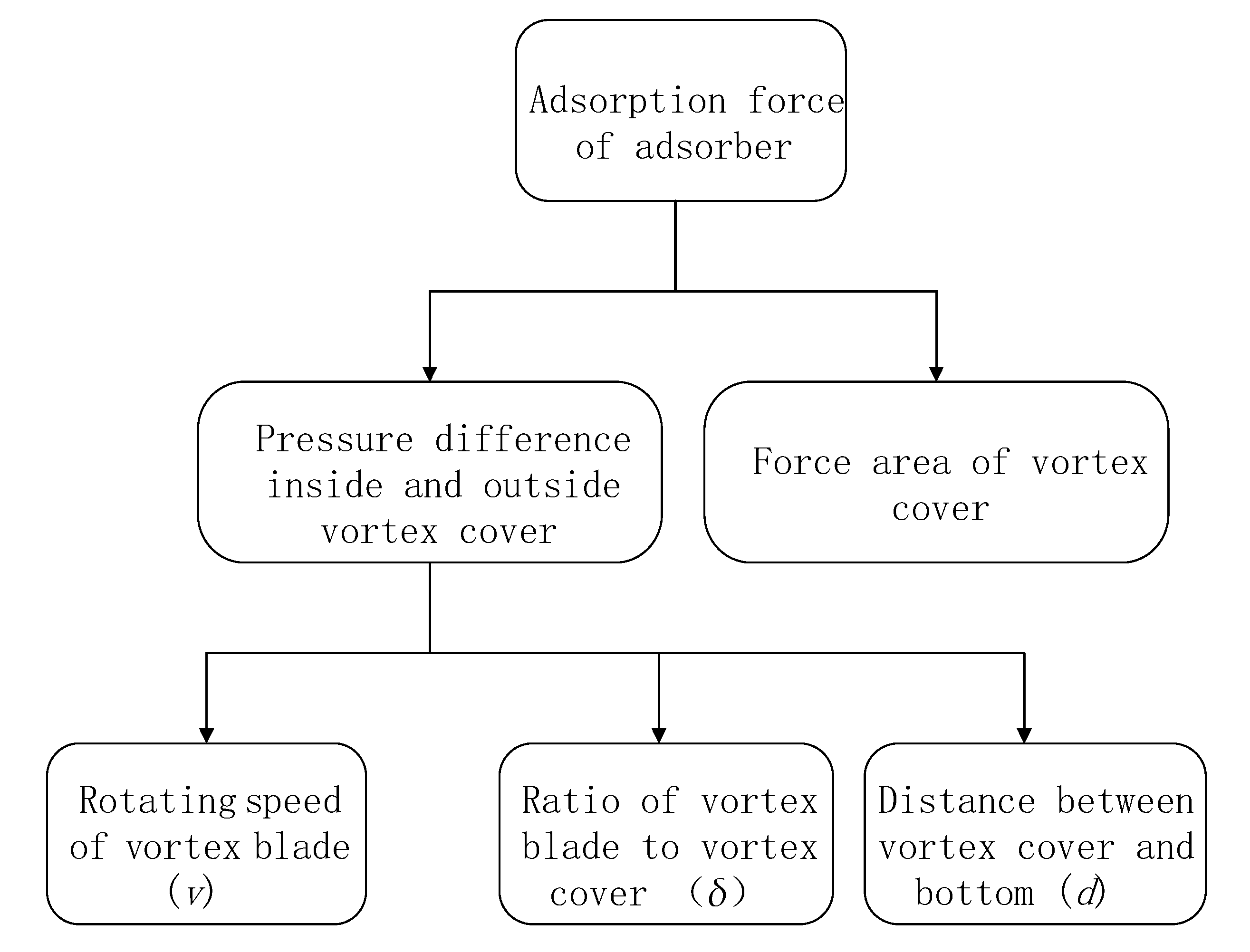
- The adsorption force F is proportional to the quadratic speed.
- F is proportional to the fourth power of the inner diameter of the vortex cover.
3. Operating Performance of Underwater Absorber
3.1. Main Factors Influencing Adsorption Performance
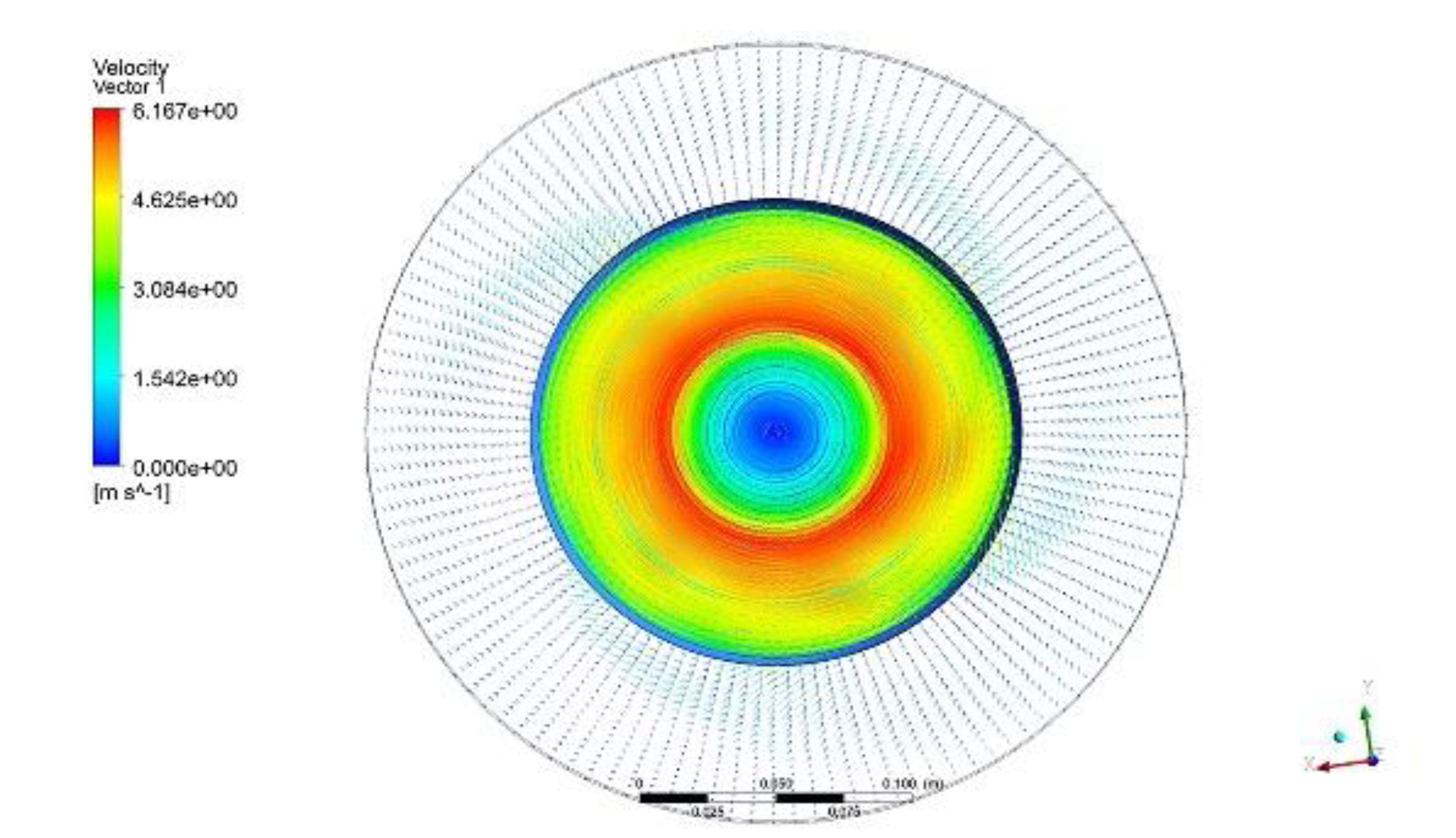
3.2. Numerical Simulation of Operating Performance
- (1)
- Water was regarded as an incompressible fluid.
- (2)
- Flow consisted mainly of circular motion. Gravity was ignored, as was disturbance in the Z direction of the leaf disk.
- (3)
- The inner surface of the vortex cover and the adsorption wall were assumed to be rigid surfaces that could not be penetrated.
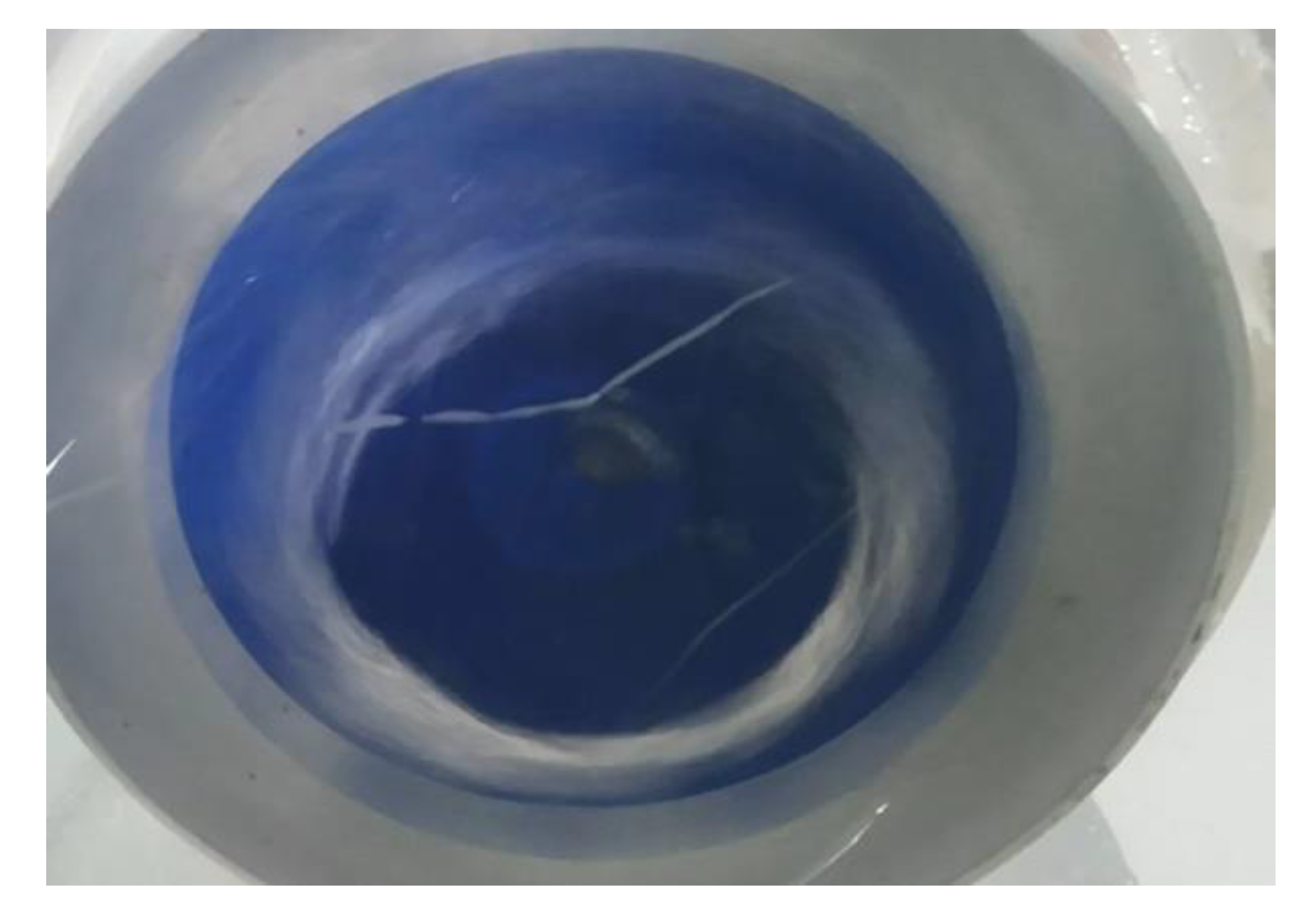
3.3. Test of Physical Prototype
3.4. Analysis
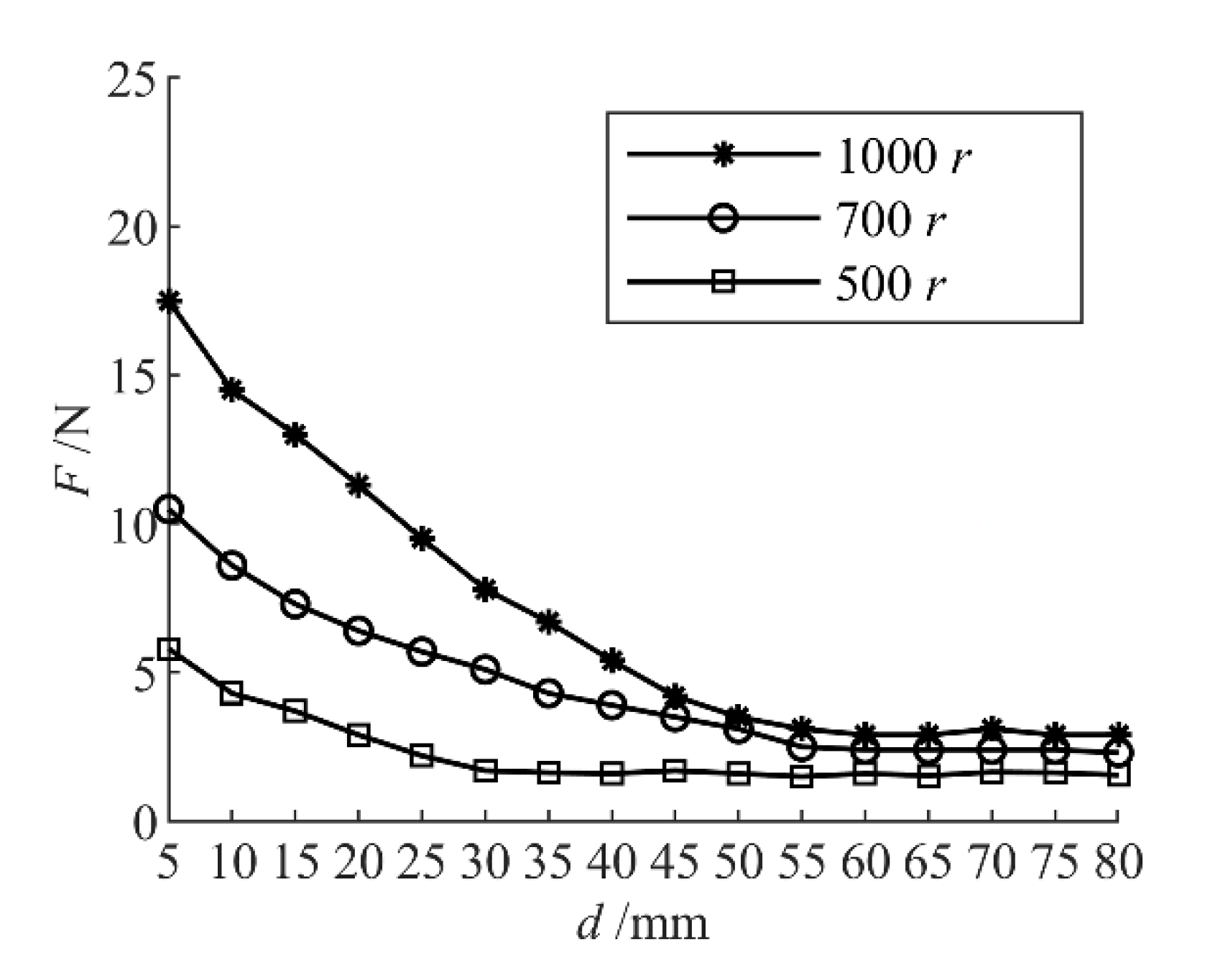
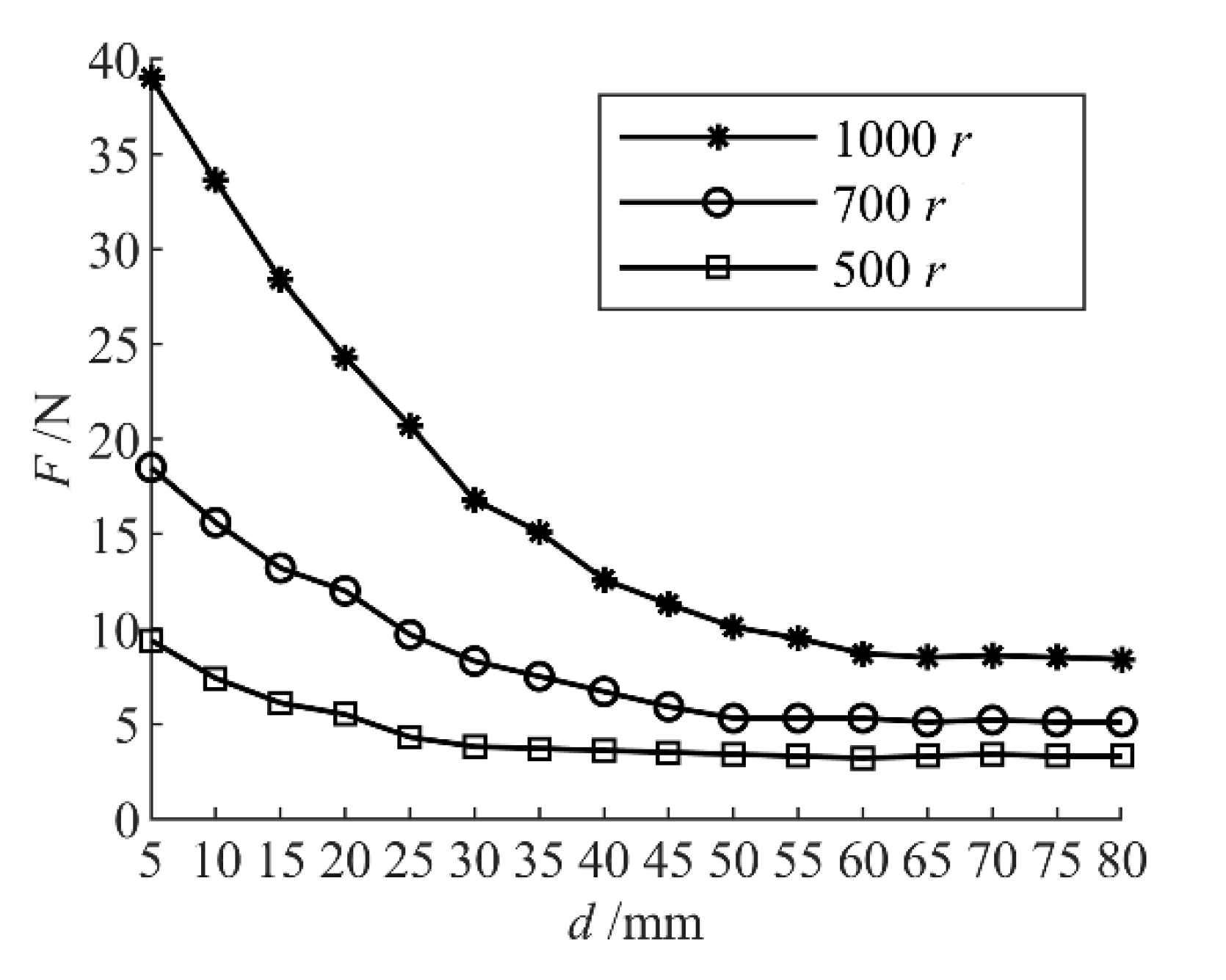
- (1)
- df had no relation to δ.
- (2)
- When δ was constant, df increased with v.
- (1)
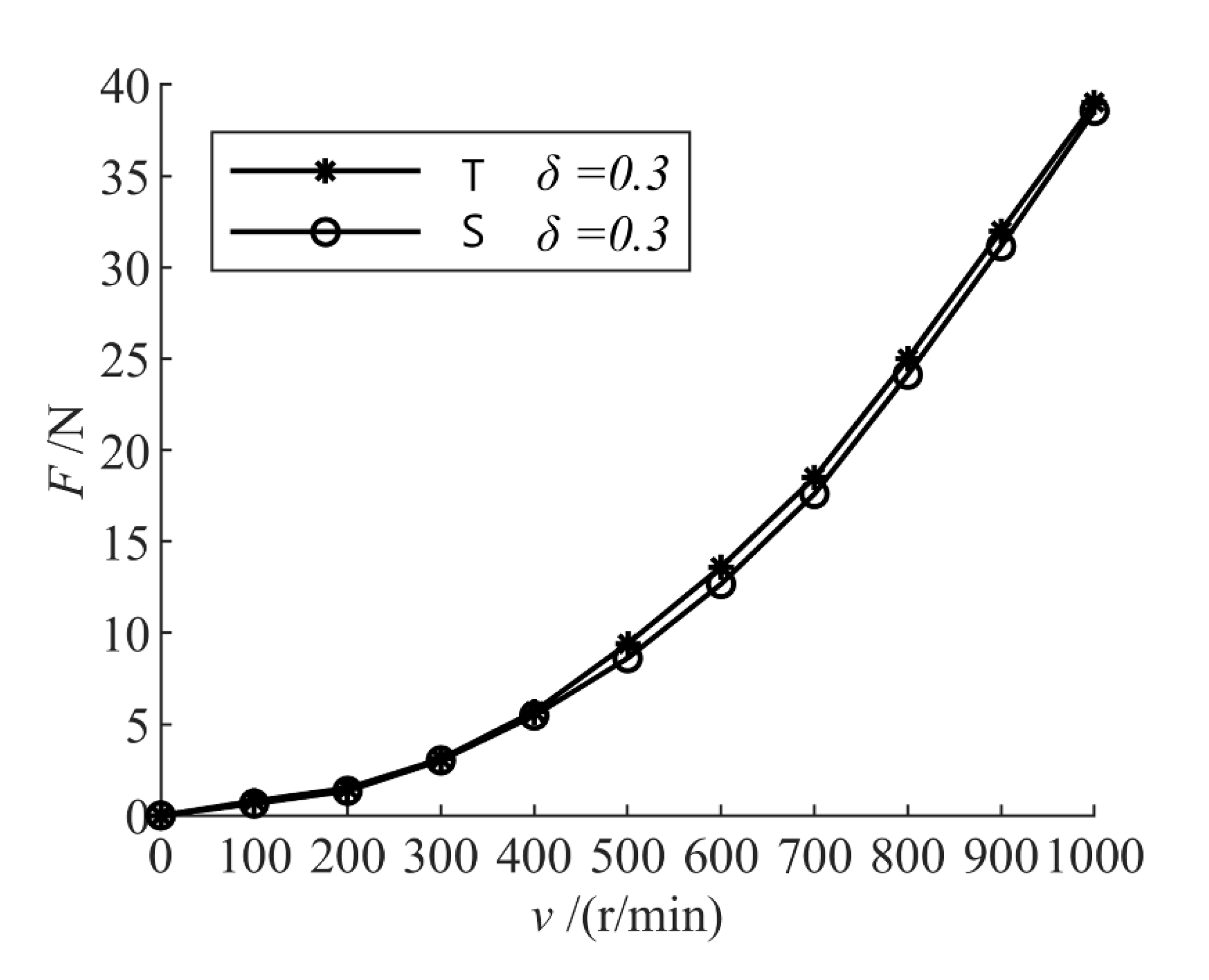
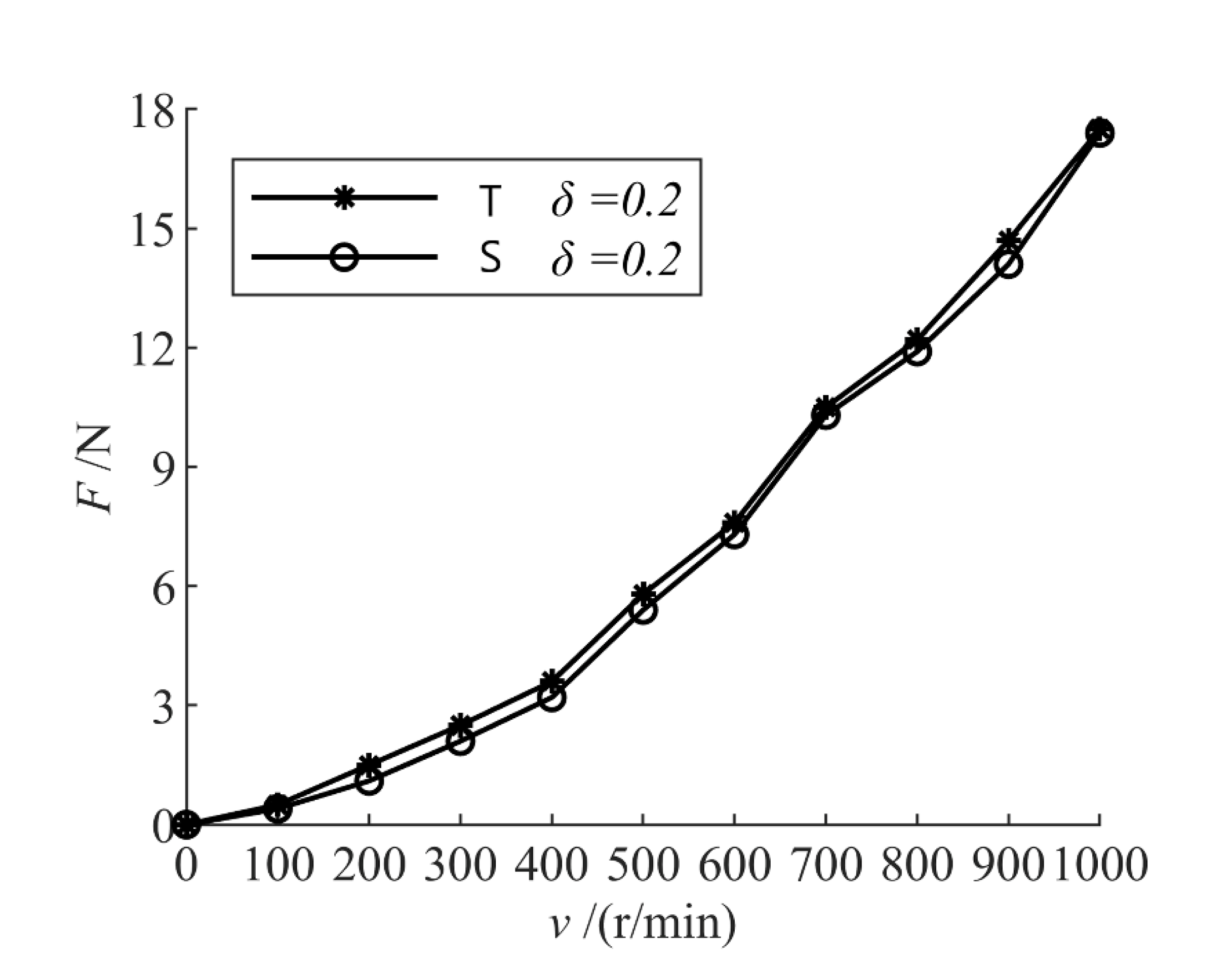
- Under the same value of v, Fd increased with δ.
- (2)
- Under the same value of δ, Fd increased with v.
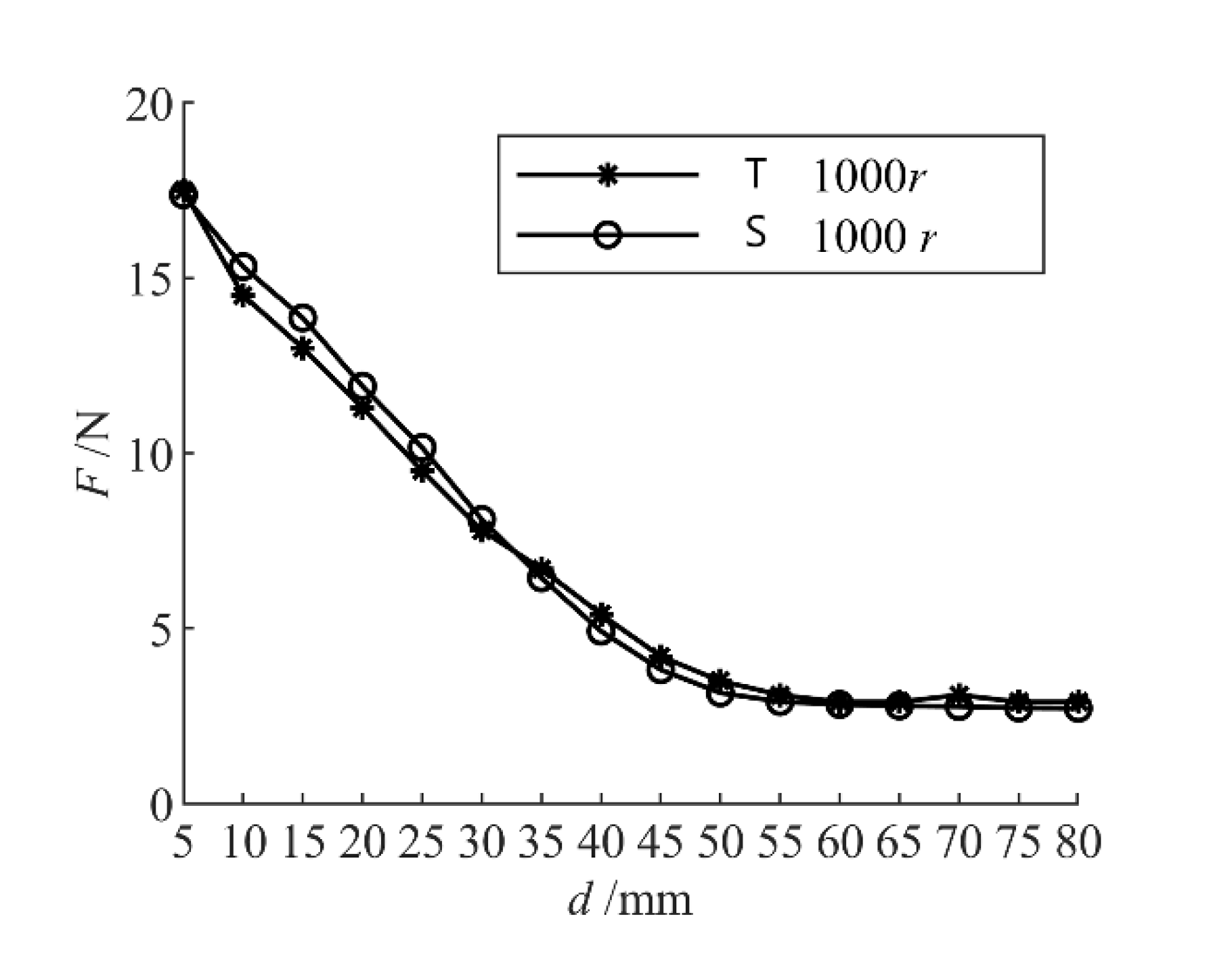
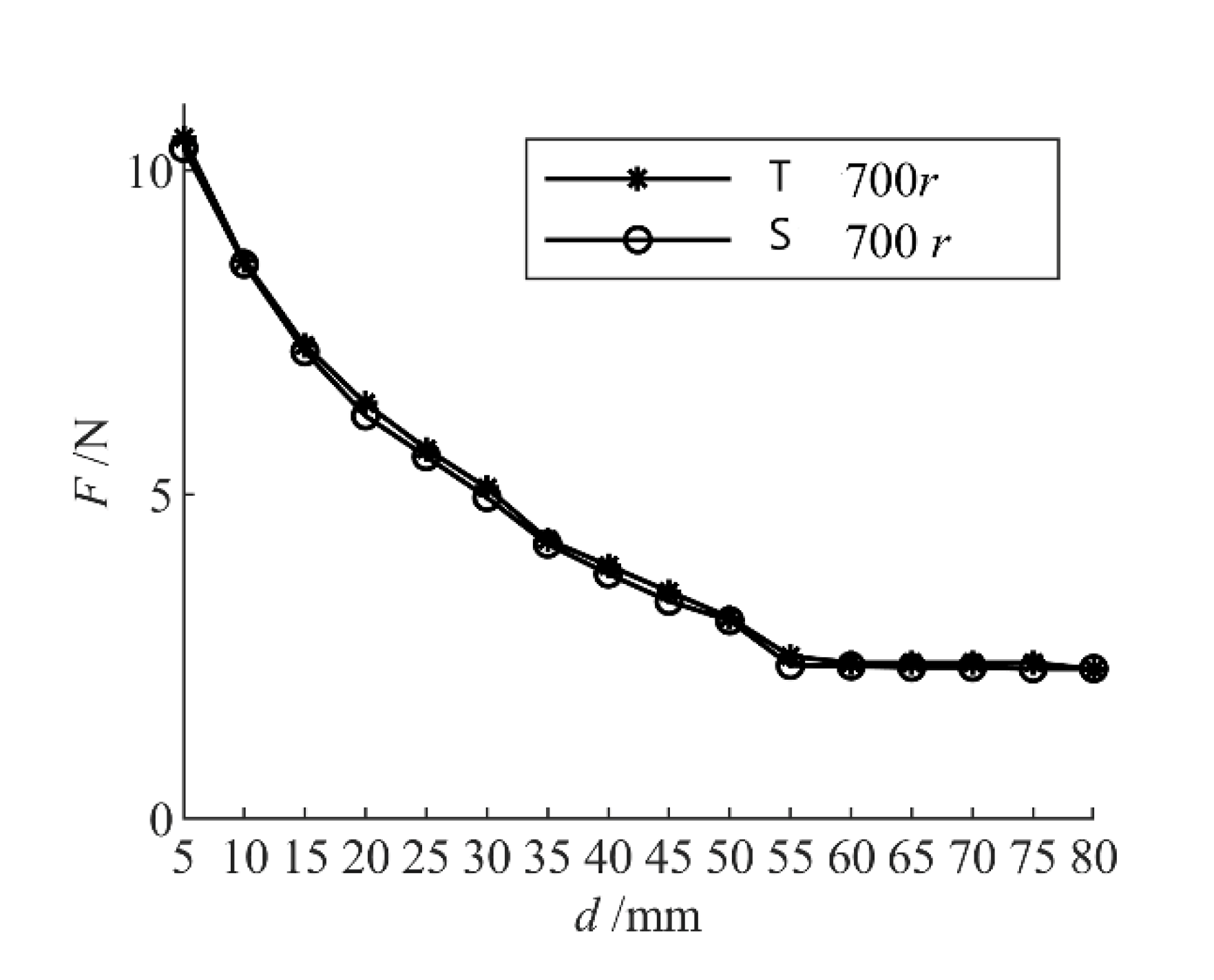
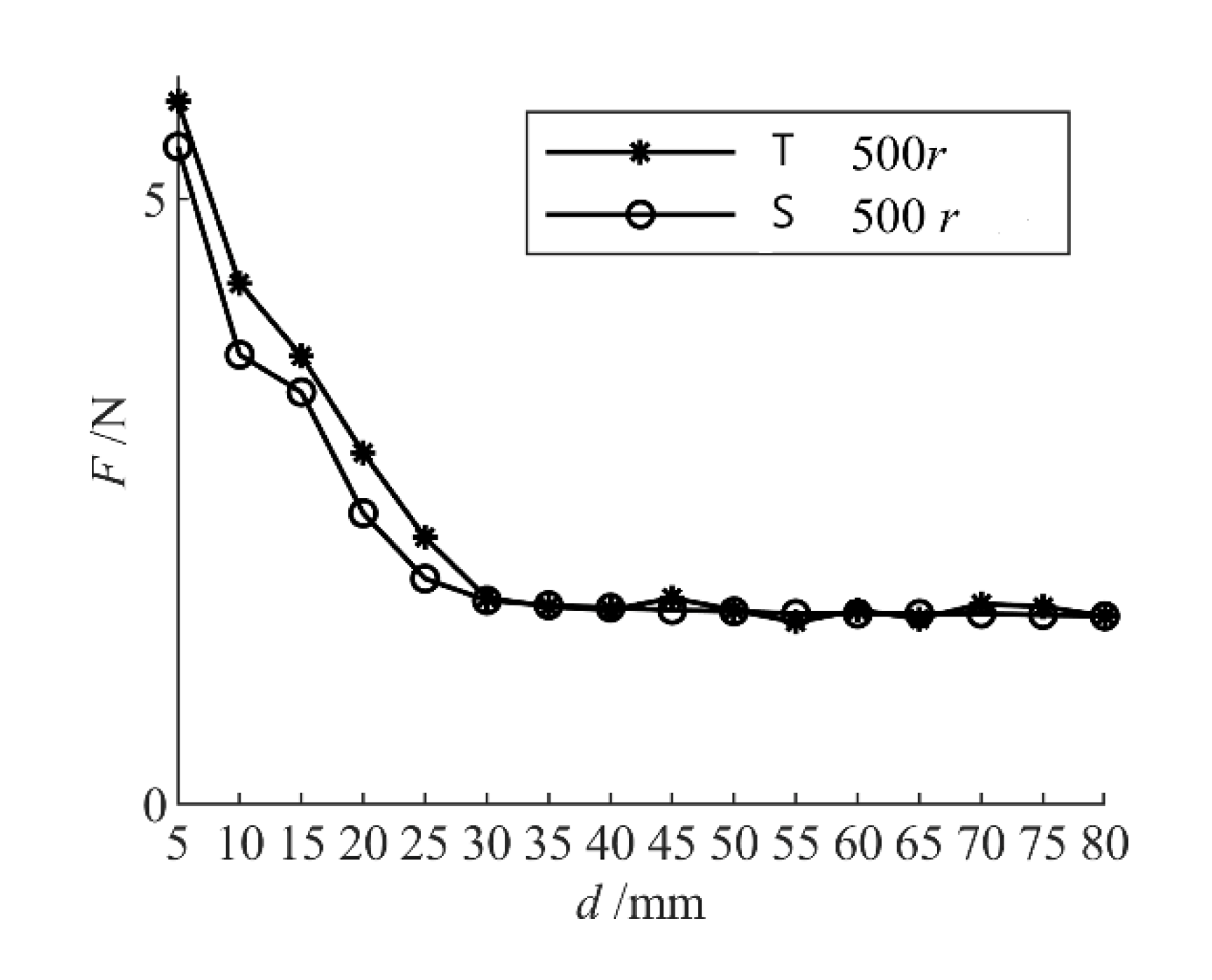
- (1)
- The results of the numerical simulation in terms of F-d were consistent with the experimental results.
- (2)
- When δ = 0.2 and v = 500 r/min, error in the numerical simulation reached its maximum value of 10.8%; when δ = 0.2 and v = 700 r/min, the minimum error of 2.7% was obtained.
- (1)
- There is a quadratic relationship between the absorber F and v.
- (2)
- F decreases with the increase in d, and the law of distribution of fluid velocity in the dome of the vortex is independent of d. When d exceeds a certain fixed value, the value of F becomes stable.
- (3)
- F increases with the increase in δ, and the distribution of fluid velocity in the vortex cover is independent of δ.
4. Conclusions
- (1)
- When v was identical to d, F increased with δ. A larger δ meant that the larger the range of direct influence of the blade on fluid in the vortex cover was, the greater the peak velocity of the fluid in the vortex cover was at the same speed and that the greater the velocity of fluid in the vortex cover was, the greater the adsorption force was. In the practical application of the absorber, when we cannot make the size of the absorber larger, we can consider increasing the speed of the blade. Moreover, the adsorption force of the absorber is quadratically related to the rotational speed of the fan blade and the increase in the rotational speed still greatly improves the adsorption force of the absorber.
- (2)
- When v and δ were identical, F decreased with d. When d increased to a certain fixed value, namely df, F did not change. This was a stable value, namely, Fd. df was independent of δ and increased only with v. Under the same value of v, Fd increased with δ; under the same value of δ, Fd increased with v. That is, when the absorber needs to be far away from the wall, the absorber with larger δ can be selected; when the absorber needs to be close to the wall, the larger v absorber can be selected.
- (3)
- When d and δ were identical, F increased with v, and there was a quadratic relationship between them. The law of distribution of fluid velocity in the vortex cover was independent of δ.
- (4)
- The numerical simulations used here represented only some phenomena, and the results were inaccurate. Error with data from the physical test was large in many cases. In the physical experiment, prominent bubbles were observed inside and at the bottom of the vortex cover. The gas–liquid two-phase flow phenomenon was not realized in the numerical simulation, and only the rotation of single-phase water was implemented.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, Y.; Li, P. Development trend of underwater vehicles. Nat. J. 2011, 33, 125–132. [Google Scholar]
- Tan, J.; Tian, J.; Wang, M. The present situation of underwater robot technology and its application prospect in water conservancy industry. China Water Conserv. 2018, 6, 33–36. [Google Scholar]
- Xu, J.; Wang, Y.; Hou, B.; Yang, L. R&D status and development trend of ROV. J. Sichuan Ordnance Ind. 2011, 32, 71–74. [Google Scholar]
- Bessa, W.M.; Dutra, M.S.; Kreuzer, E. Dynamic Positioning of Underwater Robotic Vehicles with Thruster Dynamics Compensation. Int. J. Adv. Robot. Syst. 2013, 10, 325. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, M.; Ceng, Q.; Qi, X.; Song, Z. Research on dynamic positioning of underwater robot against wave disturbance. Comput. Meas. Control 2016, 24, 199–203. [Google Scholar]
- Jiang, H.; Yang, X.; Jiang, H.H. Design and implementation of a climbing robot with adsorption and peristalsis for ships. Ship Sci. Technol. 2010, 32, 31–34, 38. [Google Scholar]
- Peng, J.; Li, J.; Shao, J.; Li, J. Research on suction system of negative pressure wall-climbing robot. China Mech. Eng. 2012, 23, 2160–2164, 2168. [Google Scholar]
- Zhang, B.; Zhu, R.; Yang, J.; Zhang, G. Study on adsorption capacity of climbing robot with compound adsorption. Mech. Des. Manuf. 2016, 250–252, 255. [Google Scholar]
- Zhang, Y.; Fu, G.; Guang, L. Design of controllable underwater adsorption structure model based on electric screw. J. Ocean Technol. 2018, 37, 40–43. [Google Scholar]
- Li, X.; Kagawa, T. Non-Contact Conveyance Equipment. U.S. Patent 20130272803, 27 December 2011. [Google Scholar]
- Cai, Z.; Luo, S. Calculating 3D viscous flow in turbomachinery by finite volume method. J. Huazhong Univ. Sci. Technol. 2000, 9, 72–75. [Google Scholar]
- Wang, F. Computational Fluid Dynamics Analysis: Principle and Application of CFD Software; Tsinghua University Press: Beijing, China, 2004; pp. 39–45. [Google Scholar]















| Parameter | Range | Remarks |
|---|---|---|
| Rotor speed v (r/min) | 100–1000 | Increment by 100 |
| Area ratio δ of disk to vortex cover | 0.2–0.9 | 0.2, 0.3, 0.6, 0.9 |
| Distance d (mm) between vortex cover and working surface | 5–80 | Increment by 5 |
| Grid Size (mm) | Leaf Grid | Outflow Field Grid | Total Grid | |||
|---|---|---|---|---|---|---|
| Number of Nodes | Unit Number | Number of Nodes | Unit Number | Number of Nodes | Unit Number | |
| 0.5 | 564,231 | 3,099,892 | 1,982,550 | 1,905,640 | 2,546,781 | 5,005,532 |
| 1 | 94,467 | 473,112 | 538,208 | 498,438 | 632,675 | 971,550 |
| 1.5 | 39,477 | 177,353 | 261,987 | 234,696 | 301,464 | 412,049 |
| 2 | 27,482 | 115,762 | 164,311 | 143,256 | 191,793 | 259,018 |
| Grid Size (mm) | F Stable Calculation Result (N) | Error (%) |
|---|---|---|
| 0.5 | 24.15 | 2.15 |
| 1 | 22.95 | 2.9 |
| 1.5 | 23.64 | 0 |
| 2 | 24.35 | 3.0 |
| Scheme | Gradient | Pressure | Momentum | Turbulent Kinetic Energy | Turbulent Dissipation Rate |
|---|---|---|---|---|---|
| Simple | least squares cell based | second order | second order upwind | first order upwind | first order upwind |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, P.; Han, C.; Lv, T.; Cheng, H. Underwater Absorber for a Remotely Operated Vehicle. J. Mar. Sci. Eng. 2022, 10, 485. https://doi.org/10.3390/jmse10040485
Xu P, Han C, Lv T, Cheng H. Underwater Absorber for a Remotely Operated Vehicle. Journal of Marine Science and Engineering. 2022; 10(4):485. https://doi.org/10.3390/jmse10040485
Chicago/Turabian StyleXu, Pengfei, Chenbo Han, Tao Lv, and Hongxia Cheng. 2022. "Underwater Absorber for a Remotely Operated Vehicle" Journal of Marine Science and Engineering 10, no. 4: 485. https://doi.org/10.3390/jmse10040485
APA StyleXu, P., Han, C., Lv, T., & Cheng, H. (2022). Underwater Absorber for a Remotely Operated Vehicle. Journal of Marine Science and Engineering, 10(4), 485. https://doi.org/10.3390/jmse10040485







