Active Flooding Mitigation for Stability Enhancement in a Damaged RoPax Ship
Abstract
:1. Introduction
2. General Description of the RoPax Vessel
3. Collision Damage Opening Times
4. Numerical Simulations and Model Tests on Flooding Mitigation
4.1. The Code HSVA Rolls
4.2. Introduction to Numerical Simulations and Model Tests on Flooding Mitigation
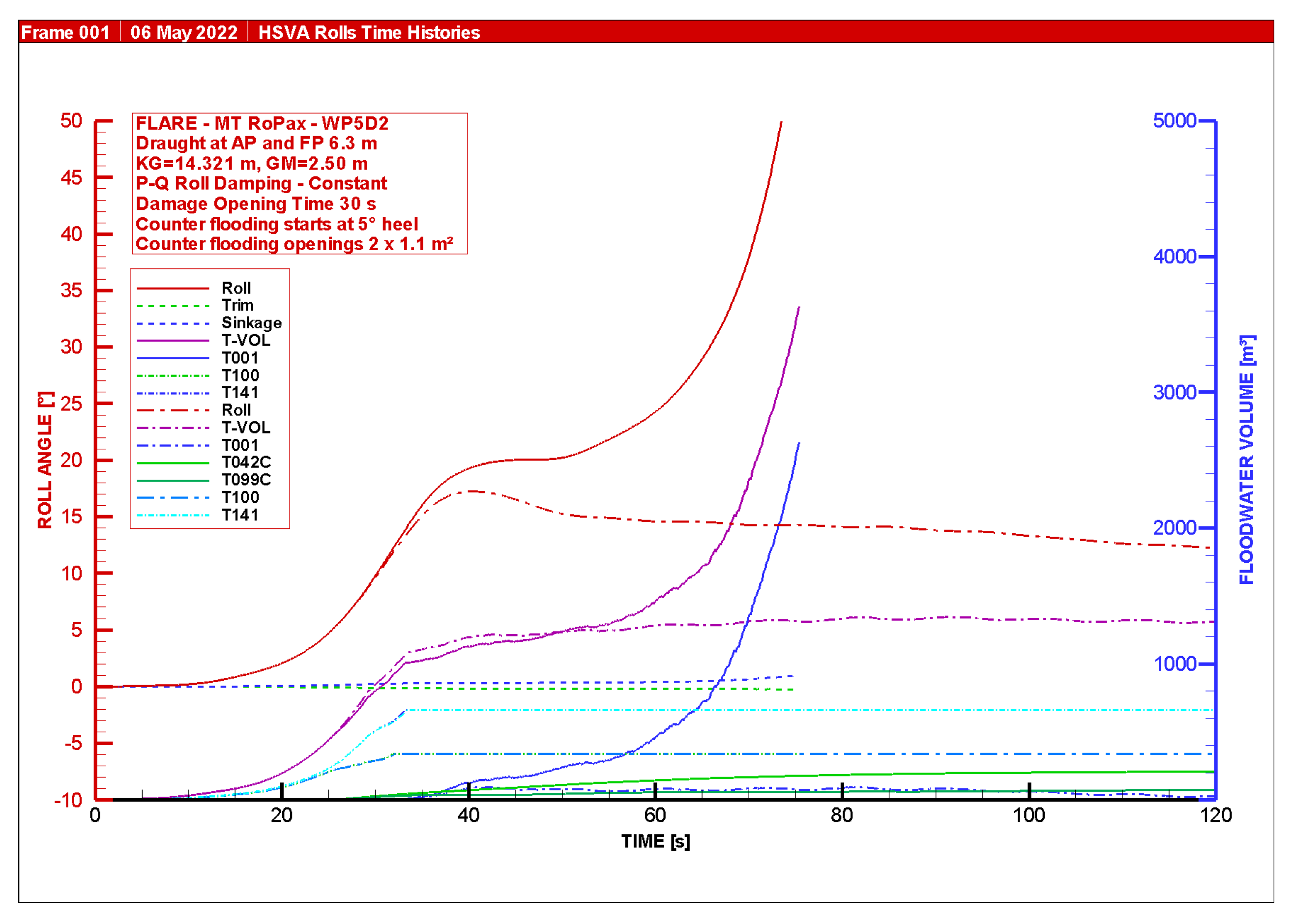
4.3. Numerical Simulation of Damage Case 2 with and without Counter Flooding
- At the low significant wave height HS 2.0 m, there is no acute need for counter flooding as the ship survives also without. However, if counter flooding is used, this results in a lower heeling angle, which would be beneficial for all rescue and disembarkation operations on the ship;
- At significant wave heights HS 2.5, 3.0, and 3.5 m, the counter flooding results in a significant improvement in the ship survivability. The corresponding survival rates increase from 50% (%) to 100%, 10% to 80%, and 0% to 60%, respectively;
- At significant wave height HS 4.0 m, the counter flooding slightly improves the time to capsize, but in this higher sea state the ship is able to survive due to gradual flooding only in 10% of the cases. Thus, the effect of flooding mitigation is small. The capsize mechanism in higher sea states is, as usual in RoPax ships, the further accumulation of water on the trailer deck, even if in this case the extent of the trailer deck is already limited with transverse bulkheads;
- It is noteworthy that the mitigation in Damage Case 2 considerably improves the ship survivability in a very large portion of the most common sea states, being very effective at the wave heights HS 2.5–3.5 m, the range of which covers a large portion of those sea states at which ship-to-ship collisions statistically tend to take place. Thus, the mitigation through counter flooding is effective in the relevant, most common sea state range. A duct opening size for each counter flooding compartment of about 1.1 m2 to 1.5 m2 is required.
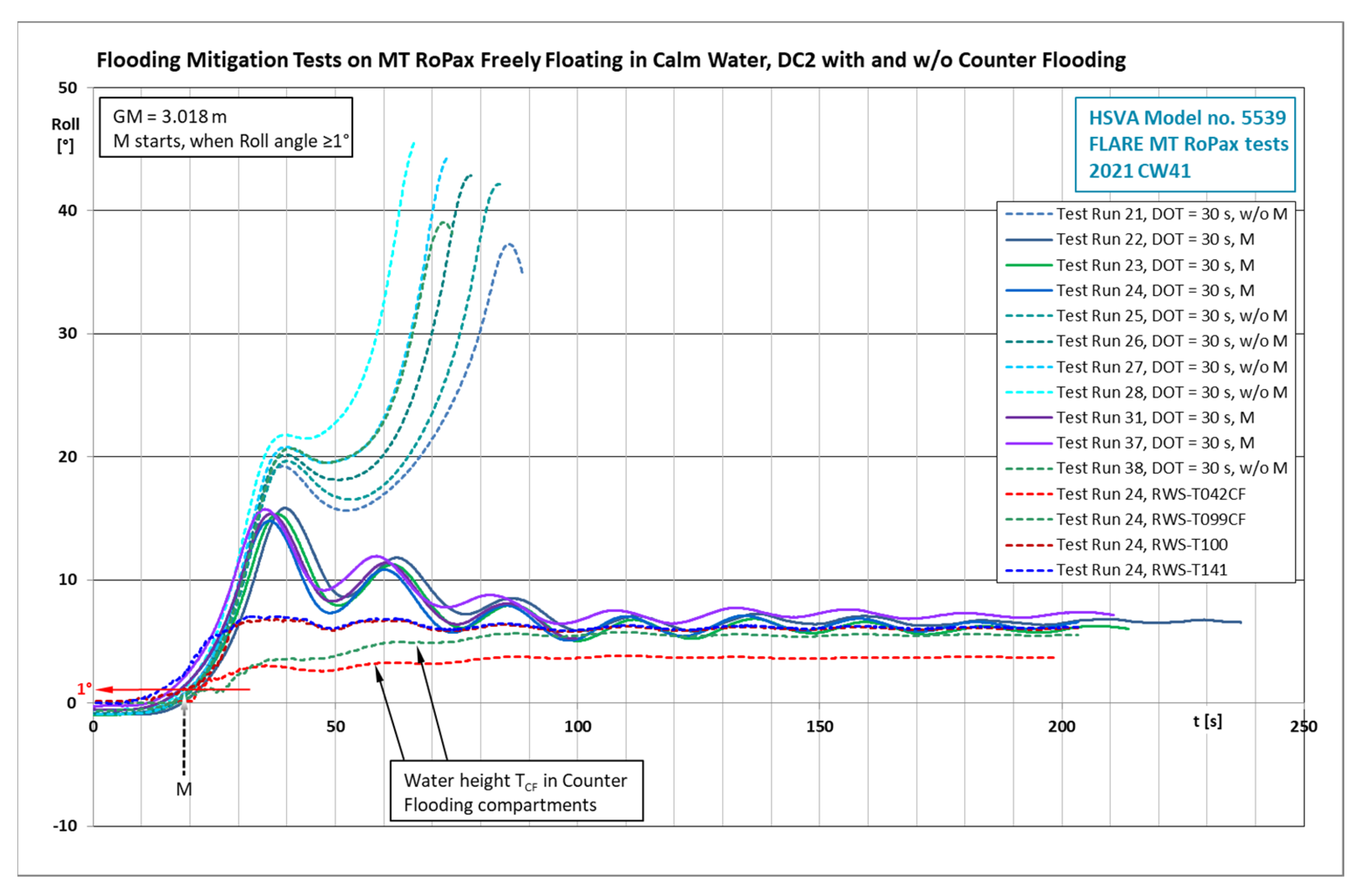
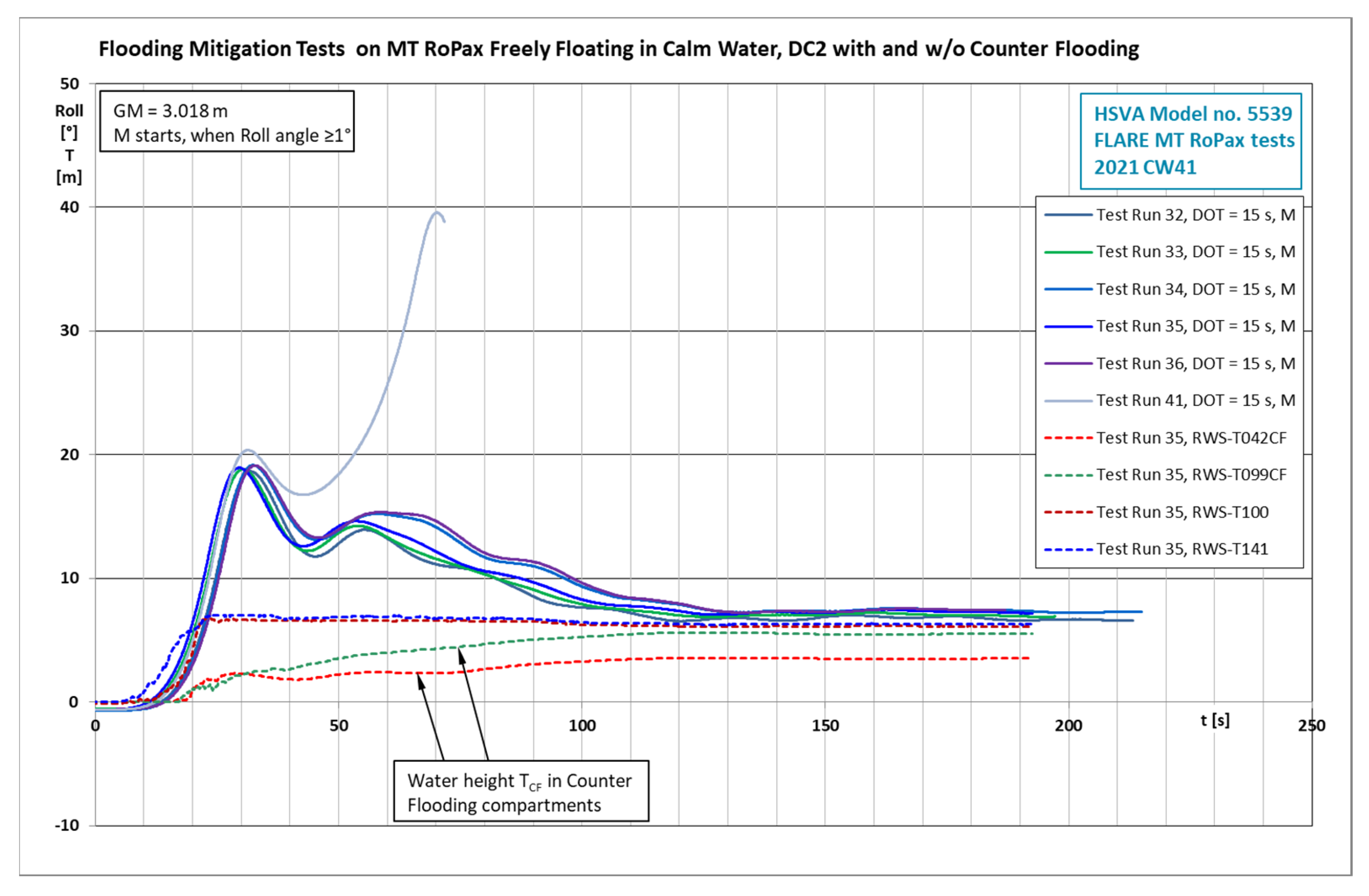
4.4. Model Test Results on Damage Case 2 with and without Counter Flooding
4.5. Numerical Hindcast of the Flooding Mitigation with Counter Flooding
4.6. Conclusions on Damage Case 2
- The mitigation computations on Damage Case 2 show that the counter flooding in calm water and in lower-to-middle sea states can prevent the ship from capsizing, and can thus have a significant effect on the potential loss of life (PLL);
- In higher sea states in beam seas, the counter flooding prevents the ship from rapid capsize, but the ship with the damaged and counter flooding compartments being all flooded has a reduced survivability. Thus, when the significant wave height increases to HS 4.0 m, the ship with GM 3.4 m starts, according to simulations, to capsize. However, even in these cases, capsize is delayed, allowing more passengers and crew to disembark the ship, reducing the potential loss of life (PLL). According to the model tests, the damaged ship with intact GM 3.48 m still survives HS 5.0 m in 100% of the cases with mitigation;
- The counter flooding has the significant advantage that few best suitable compartments on each side of the ship can be prepared for this. Their ship stabilizing effect can be applied to a large variety of damage cases on the opposite side of the ship. In the particular case of the MT RoPax, it is not difficult to find two to four suitable compartments, for example void spaces, on each ship side for this purpose;
- The present numerical modeling techniques available are sufficient for quite-accurate numerical modeling of ship behavior with counter flooding when attention is paid to the dynamic character of the flooding phenomena.
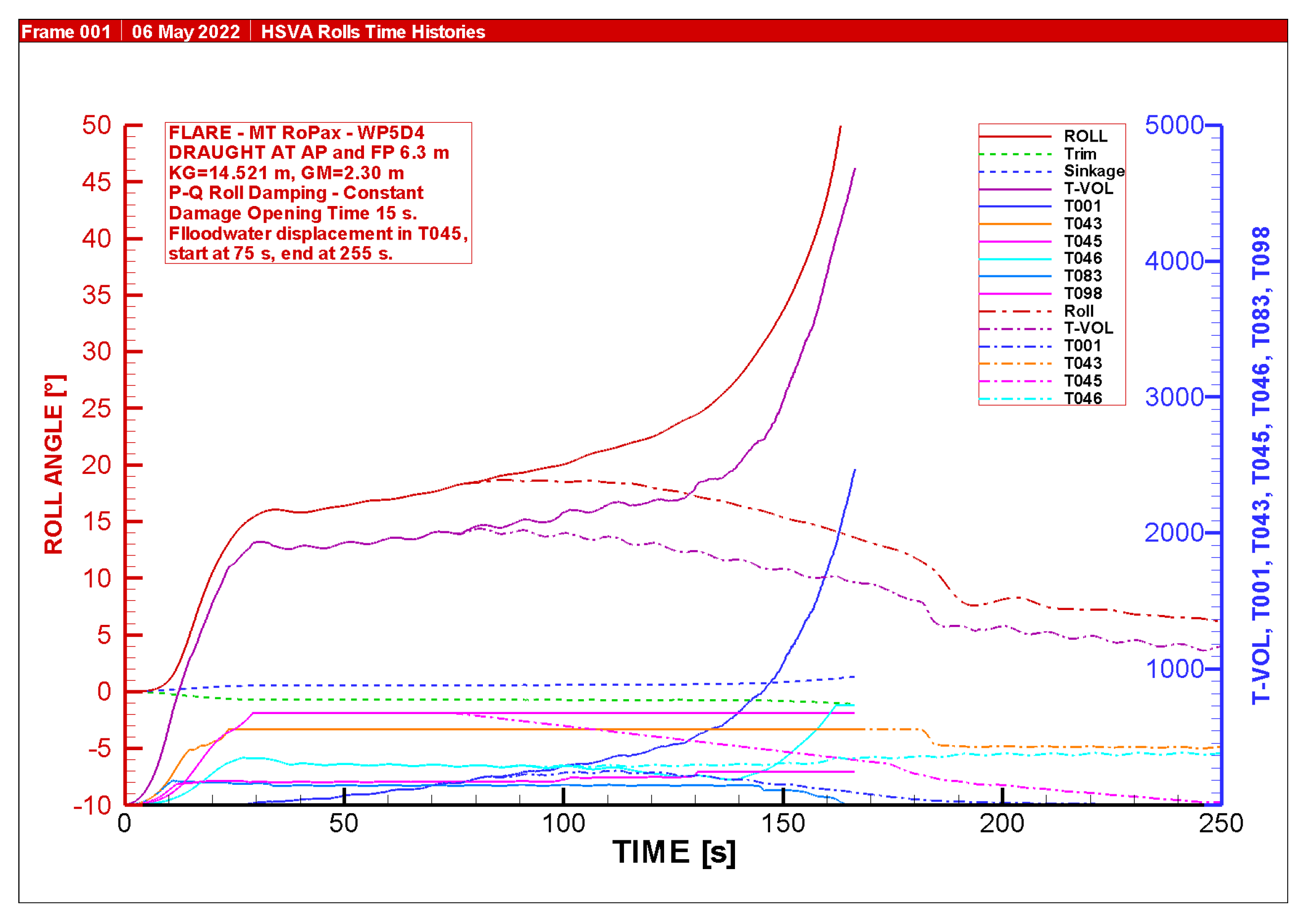
4.7. Numerical Simulation of Damage Case 4 with and without Recovery of Lost Buoyancy
- At low significant wave heights HS 2.0–4.5 m there is no acute need for recovering the lost buoyancy in compartment T045, as the ship survives also without. However, if the mitigation method is used, this results in a lower heeling angle, which would be beneficial for all rescue and disembarkation operations on the ship;
- At significant wave heights HS 5.0, 5.5 m, and 6.0 m, recovering the lost buoyancy results in a clear and significant improvement of the ship survivability. The corresponding survival rates increase from 80% to 100%, 40% to 80%, and 10% to 70%, respectively;
- At significant wave height HS 7.0 m, recovering the lost buoyancy in compartment T045 does not improve the time to capsize, as in this higher sea state the ship is not able to survive the gradual flooding. Thus, the effect of mitigation is practically non-existent. The capsize mechanism in higher sea states is, as usual in RoPax ships, the further accumulation of water on the trailer deck, even if the extent of the trailer deck is already limited, as shown in Figure 11;
- The mitigation in Damage Case 4 with the recovery of the lost buoyancy in compartment T045 considerably improves the ship survivability in a large portion of the prevailing sea states, being very effective at the wave heights HS 5.0–6.0 m, the range of which covers a portion of the prevailing sea states. In Damage Case 4, the ship survives well in lower beam sea states without mitigation. If the damage were larger, the effective range of mitigation would be located at lower, more frequent sea states. The mitigation through the recovery of lost buoyancy in a damaged compartment is a suitable method for this;
- In its simplest form, the recovery of lost buoyancy in a damaged compartment reduces the heeling angle of the ship and thus in most cases also the water ingress on the trailer deck, which is crucial for RoPax ship survival. The reducing effect of the mitigation on ship draught is likely to be less important.
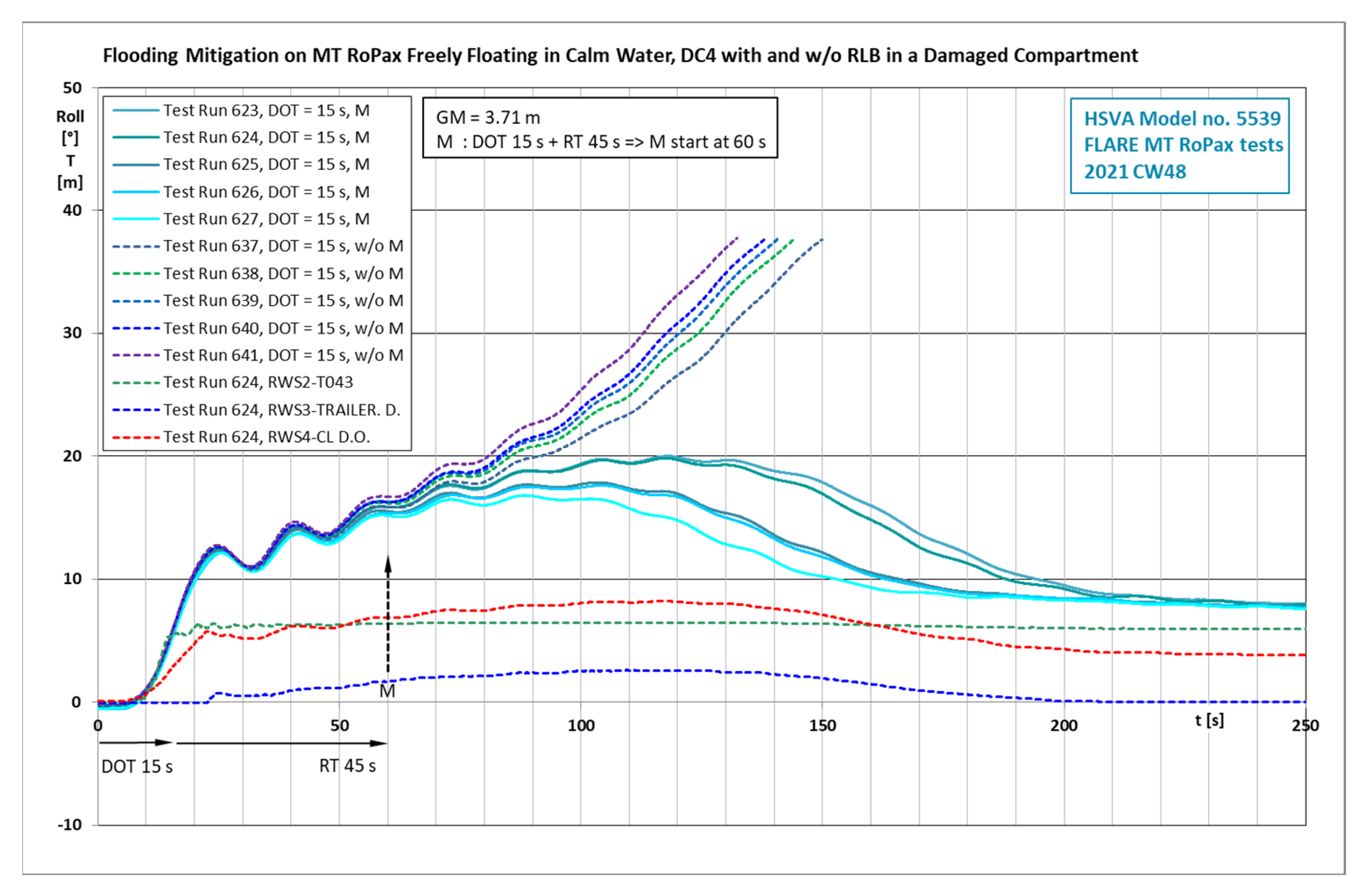
4.8. Model Test Results on Damage Case 4 with and without Recovery of Lost Buoyancy
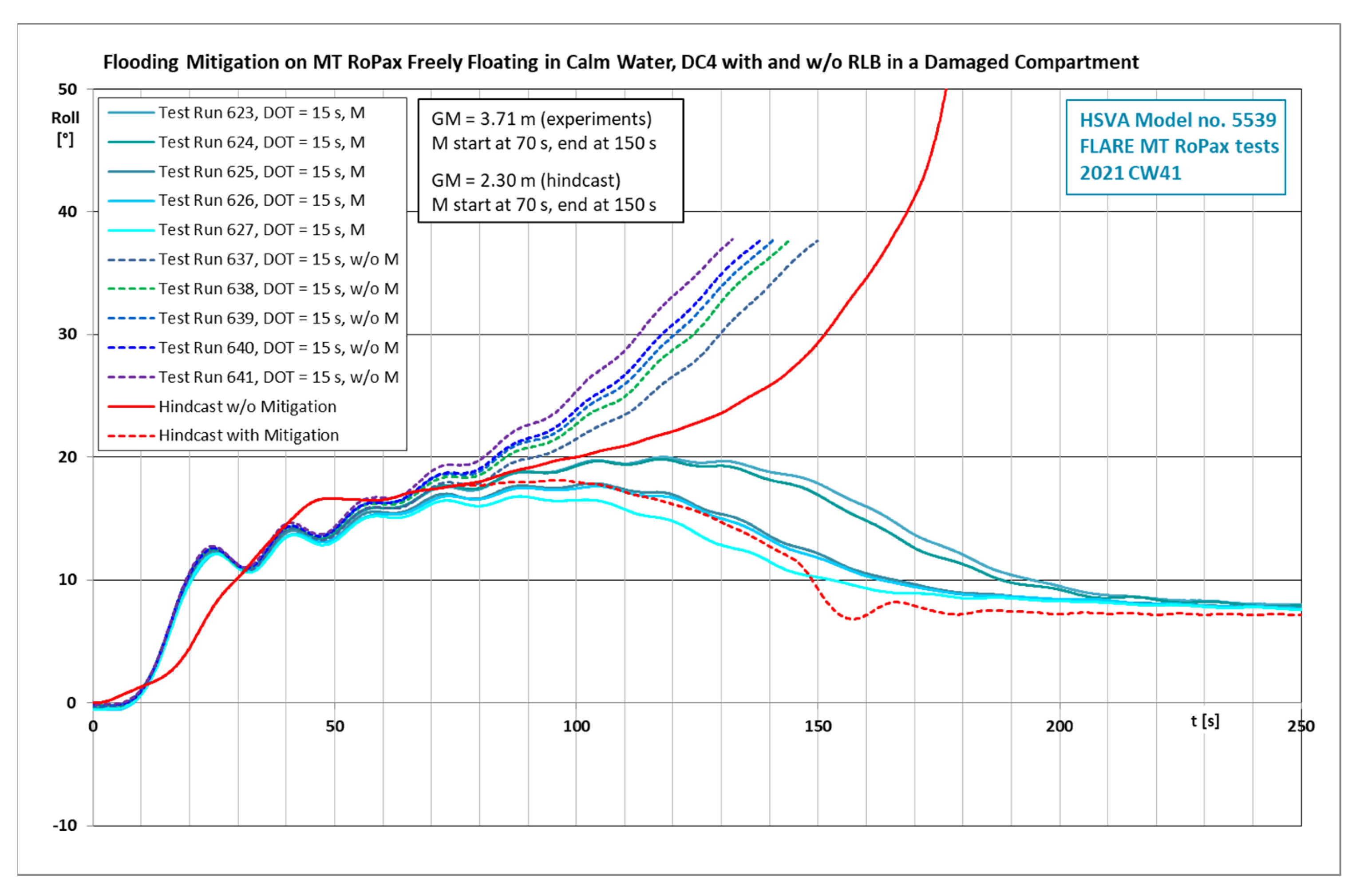
4.9. Numerical Hindcast of the Flooding Mitigation with Recovery of Lost Buoyancy
4.10. Conclusions on Damage Case 4 with and without Recovery of Lost Buoyancy
- The flooding mitigation with the recovery of lost buoyancy in a damaged compartment is sufficient to prevent capsize and the ship survives 1800 s easily in the studied cases;
- In its simplest form, recovering lost buoyancy in a damaged compartment reduces the heeling angle of the ship and thus in most cases also water ingress on the trailer deck, which is crucial for RoPax ship survival. The reducing effect of the mitigation on the draught is likely to be less important;
- The righting lever provided by the recovery of the lost buoyancy in a damaged compartment is an important factor for the effectiveness of the mitigation. This depends on the compartment volume and the distance of its center of volume to the centerline of the ship;
- Recovering lost buoyancy in the damaged compartments in principle implies the preparation of each compartment that can get damaged for this. At least preparation of the largest compartments far away from the centerline is necessary;
- The idea to displace flood water in a damaged compartment with a lighter substance, e.g., expandable foam, will always leave some questions open with respect to the functionality of such a mitigation system in a compartment possibly already heavily damaged by a collision or grounding;
- On one hand, the flooding mitigation with the recovery of lost buoyancy is more difficult to arrange than counter flooding. On the other hand, some of the buoyancy lost in the damaged compartment is recovered, which improves the ship survivability;
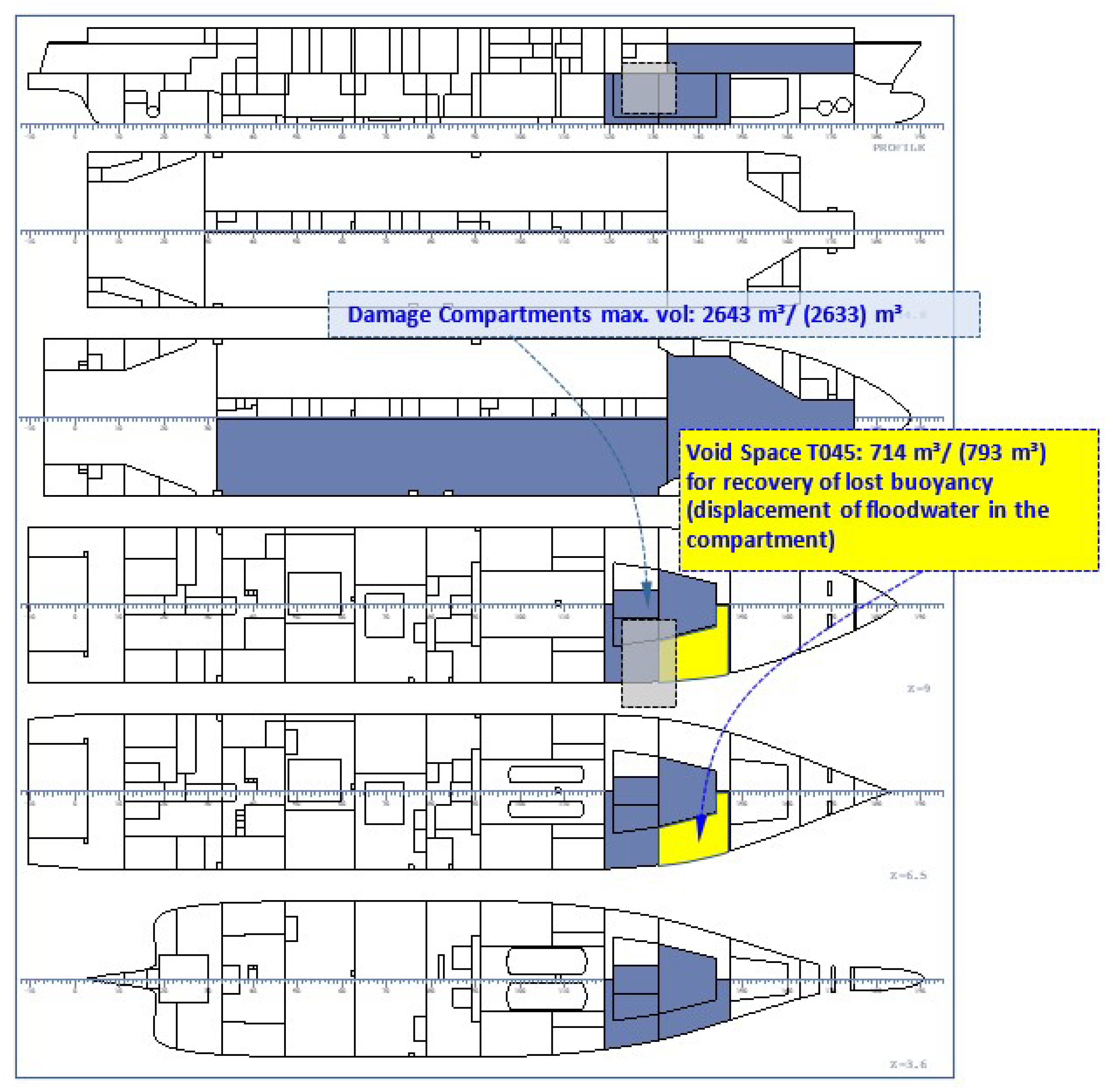
- As the recovery system of the lost buoyancy in a damaged compartment system should be available at any arbitrary compartment that can get damaged, it is difficult to consider that the system could be deployed very rapidly. In this study, the reaction times needed to be shortened from those originally planned to yield positive results. During the chosen mitigation time of 180 s between ca. 500 m3 and 793 m3, floodwater needed to displaced out of the damaged compartment. This is a formidable task.
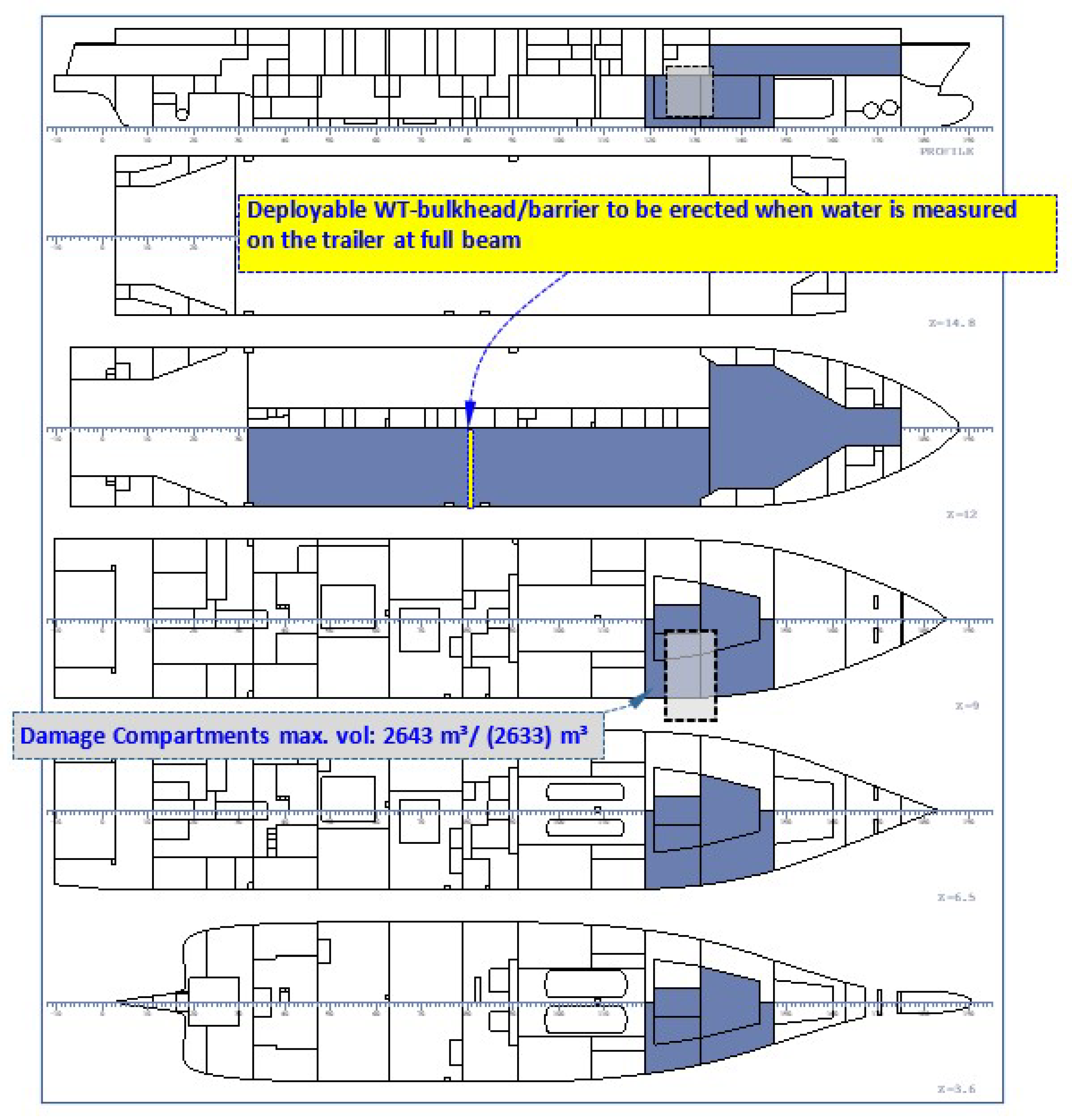
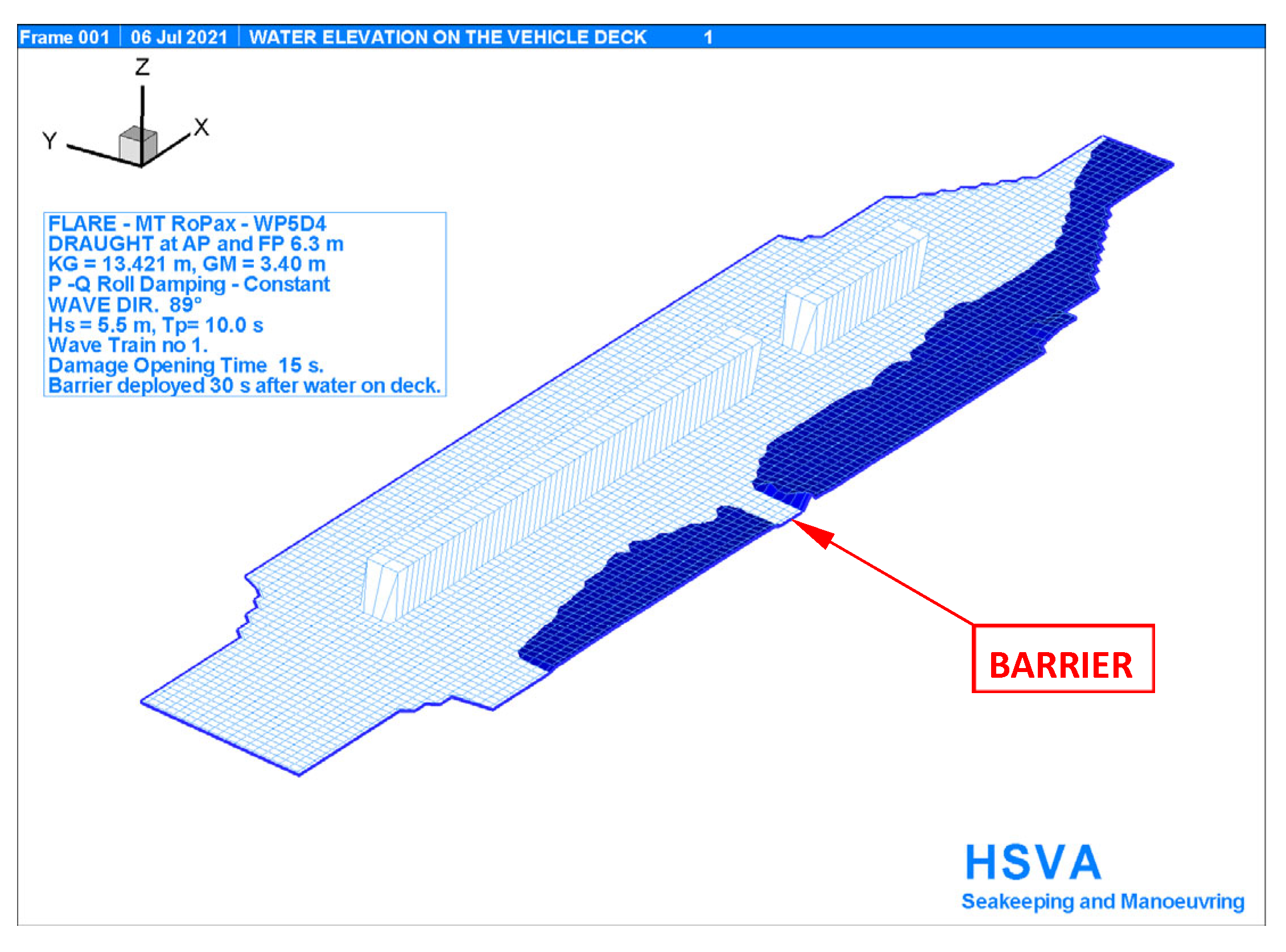
4.11. Numerical Simulation of Damage Case 4 with and without Deployment of a Watertight Barrier on the Trailer Deck
- The deployment time of such a barrier should be as short as possible;
- If any floodwater goes past the barrier, there should a possibility to let this water to flow out.
- At significant wave heights up to HS 4.0 m, there is no acute need for the deployment of the WT barrier in the given damage case, as the ship survives with the lowest GM value 3.4 m on the limit curve also without;
- At significant wave heights HS 5.0, 5.5, 6.0, and 7.0 m, the deployment of the WT barrier results in a clear and significant improvement of the ship survivability. With the ship GM value 3.4 m, the corresponding survival rates increase from 80% to 100%, from 40% to 100%, from 10% to 100%, and from 0% to 100%, respectively;
- The mitigation in Damage Case 4 with the deployment of a WT barrier considerably improves the ship survivability in a large portion of the prevailing sea states, being very effective at the wave heights HS 5.0–7.0 m. If the damage were larger, the effective range of mitigation would be located at lower, more frequent wave heights. The mitigation through the deployment of the WT barrier is a suitable method for this.
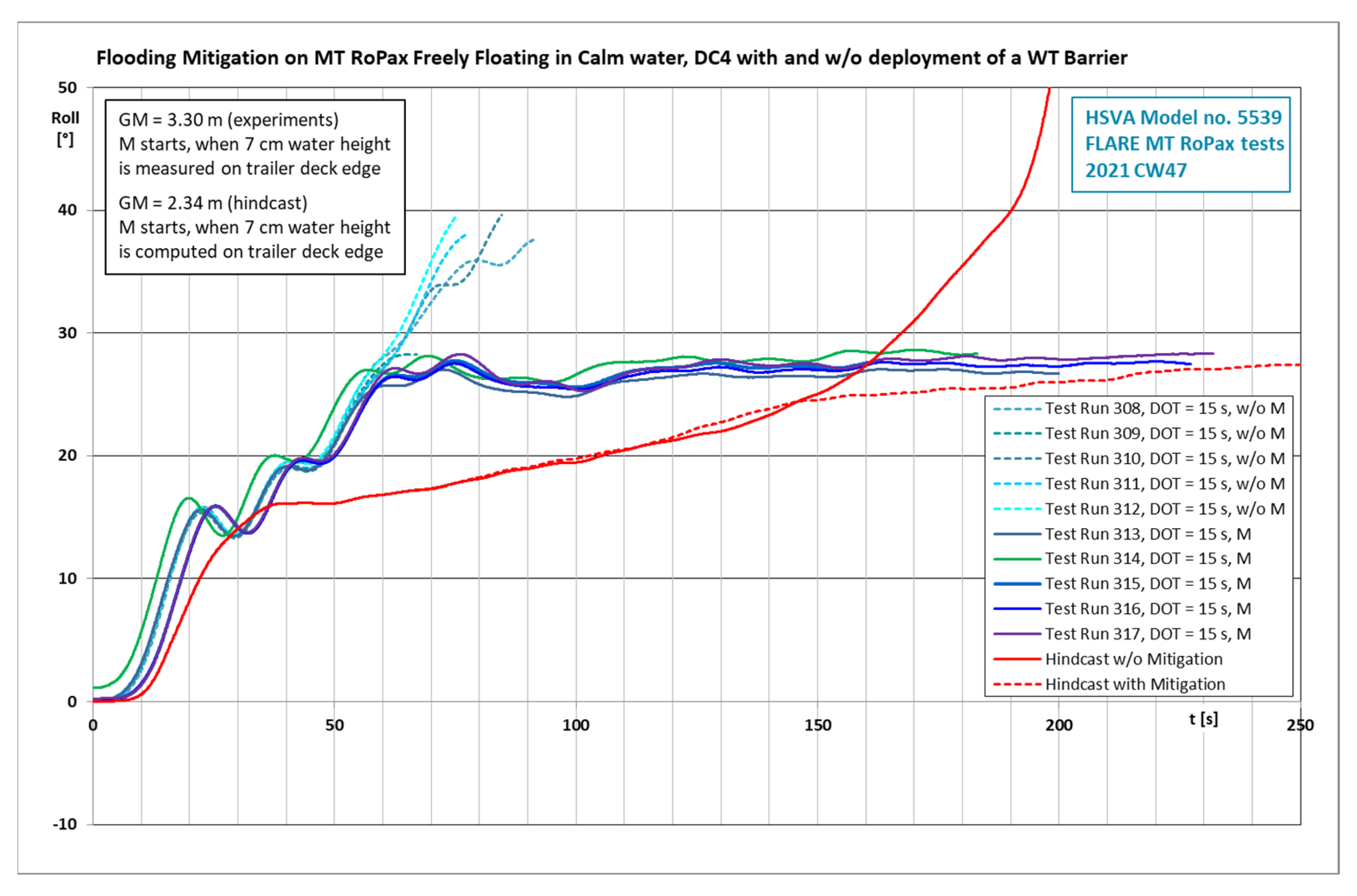
4.12. Model Test Results on Damage Case 4 with and without Deployment of a Watertight Barrier on the Trailer Deck
4.13. Numerical Hindcast of the Flooding Mitigation with Deployment of a Watertight Barrier
4.14. Conclusions on Damage Case 4 with and without Deployment of a Watertight Barrier
- The deployment of the watertight barrier on the trailer deck is a very straightforward flooding mitigation measure and can easily be installed on new or existing RoPax ships. The deployment time 30 s is based on built roll gates in use at HSVA. Before the barrier descended in the mitigation tests, some floodwater had already passed its position and had spread further onto the trailer deck. Regardless of this, the mitigation gives good results;
- If the capsize mechanism is gradual flooding, a short deployment time is certainly beneficial, but it does not appear to be crucial: in a few test computations with 30 s and 180 s deployment times, no significant differences in the results were found. Such a difference can be expected, when one or more high waves hit the breach on the ship hull just when it has opened. In a ship-to-ship collision, however, the damaged bow of the other ship would be just outside of the breach on the struck ship, between incoming waves and the breach. Thus, a sheltering effect against incoming waves can be expected;
- An earlier start of the deployment is of course beneficial. There is no practical reason that would prevent such roll gates to be lowered before the damage opens, letting a massive amount of floodwater in. The mitigation tests carried out demonstrate that also a fully automatic start of the mitigation based on floodwater detected on the trailer deck functions very well;
- The weakness of the method is that it does not recover anything, reduce heeling angle, or increase freeboard at damage location. It just stops the flooding progressing further on the important trailer deck. The heeling angle, as in this damage case, can remain high, and any disembarkation, if needed, would remain difficult in these conditions;
- Altogether, the curtain barrier remains an interesting, possibly cost-efficient solution, which can be added also to existing ships with relative ease. Instead of using a sensor to detect water on the vehicle deck, also other trigger/release mechanisms can be considered: (1) a general closure of WT doors, including the deployment of curtain barriers as a precautionary measure in case of a potential flooding hazard, (2) or at the latest, at the very onset of an accident, such as a collision.
5. An Overview of the Mitigation Methods
6. Conclusions
- Flooding mitigation measures on a modern RoPax design were investigated with numerical simulation and model tests in calm water and in irregular beam seas in two damage cases with and without the following mitigation efforts: (1) counter flooding; (2) recovery of lost buoyancy by displacing floodwater in a damaged compartment; and (3) deployment of a watertight barrier to prevent floodwater spreading on the large open trailer deck of the RoPax ship;
- The choice of the compartments for active flooding mitigation measures is based on the principles: (1) to provide righting moment to reduce ship list due to damage, (2) to maintain a slight trim (slope down) on the trailer deck towards the damage opening, (3) not to flood any compartments essential for the ship functions, and (4) to displace water only in compartments in which a foam system (or equiv.) can be applied, e.g., a potable water tank may not be suitable;
- The investigation throws light on the applicability and effectiveness of the investigated mitigation methods in calm water and in different beam sea conditions. Information on the ship stability, damage, and wave parameters suitable for model testing was generated;
- Damage Case 2 was investigated with and without counter flooding (CF). First, some computations with different duct opening sizes to the counter flooding compartments were carried out to show how the mitigation works in ideal calm water conditions with suitable ship GM values;
- Damage Case 4 was accordingly investigated with numerical simulations with and without the recovery of the lost buoyancy (RLB) by displacing floodwater in a damaged compartment, and also with and without the deployment of a watertight barrier (WTB) on the trailer deck to prevent further flooding;
- Although the numerical computations and model test results show some differences, they all very clearly demonstrate the benefits of the three flooding mitigation methods in the damage cases investigated;
- All the three mitigation methods investigated were found to be effective in either preventing or postponing ship capsize, thus providing good potential for the clear improvement of ship survivability in foreseeable damage cases. The mitigation methods studied are suitable for new and existing ships. This applies both to sudden flooding cases with transient floodwater and ship behavior as well as to gradual flooding cases;
- The GM values used in the tests deviate from the lowest GM value on the limit curve 3.4 m because GM values suitable for demonstrating the mitigation efforts were used in the computations and model tests. Using the GM value 3.4 m in all tests would have meant that a less clear demonstration of the effects would have been obtained, while in some cases the mitigation would not have been necessary, and in some cases, it would not have been sufficient;
- Planning the flooding mitigation for a ship design with a given GM would involve defining the extent of the mitigation methods that are sufficient to reach a desired survivability level, e.g., at given sea state. This is certainly possible in view of the information gained through the computations and model tests of the present study;
- Numerical simulations are required to design a flooding mitigation system. The simulation code should be able to handle different damage opening times and various release mechanisms for mitigation, e.g., the heeling angle or water depth in a compartment, and adjustable time delays in mitigation set-up. The programming effort for such amendments to an existing simulation code is small;
- The tested mitigation methods improve the ship survivability in these two damage cases on a certain range of wave heights. At low sea states, mitigation is often not needed. At the rarer high sea states, the effects of mitigation are not always sufficient to secure survival. In between, there is a range of sea states in which all the tested mitigation efforts considerably increase the ship survivability;
- In the simulations, the damage opening time of 15 s was mostly used. This is based on the assumption of the colliding ship pulling itself back at full power astern with its damaged bow withdrawing out of the collision damage penetration in the other ship. Presently, this is the best estimate of the shortest possible damage opening time. In a real case, the chances that the opening time would be longer should be rather high. This means that the calculated results should be conservative. That is, the mitigation systems are likely to be more effective and more largely applicable to a larger variety of cases than what the simulations and model tests here show;
- Counter flooding was found to be the easiest and fastest way to stabilize the damaged RoPax ship under study. Counter flooding has the advantage that few best suitable compartments on each side of the ship can be prepared for this. Their ship stabilizing effect can be applied to a large variety of damage cases on the opposite side of the ship.
- The recovery of lost buoyancy in the damaged compartment in principle implies the preparation of each compartment that can get damaged. At least preparation of the largest compartments far away from the centerline is necessary;
- The typical capsize mechanism of a RoPax ship involves the flooding of the trailer deck. Once this has progressed far enough, a capsize in waves is only a matter of limited time. The deployment of a watertight barrier on the trailer deck effectively prevents this, and the ship can survive in a damaged condition also in very high sea states. As there is no recovery in the damage extend, but just limitation, and the heeling angle can remain high, which has an adverse effect on all actions onboard. However, once the vessel is not in acute danger, the crew can concentrate on further stabilizing the ship.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Model Testing Techniques Applied in the Flooding Mitigation Tests
Appendix A.1. Damage Opening Mechanisms

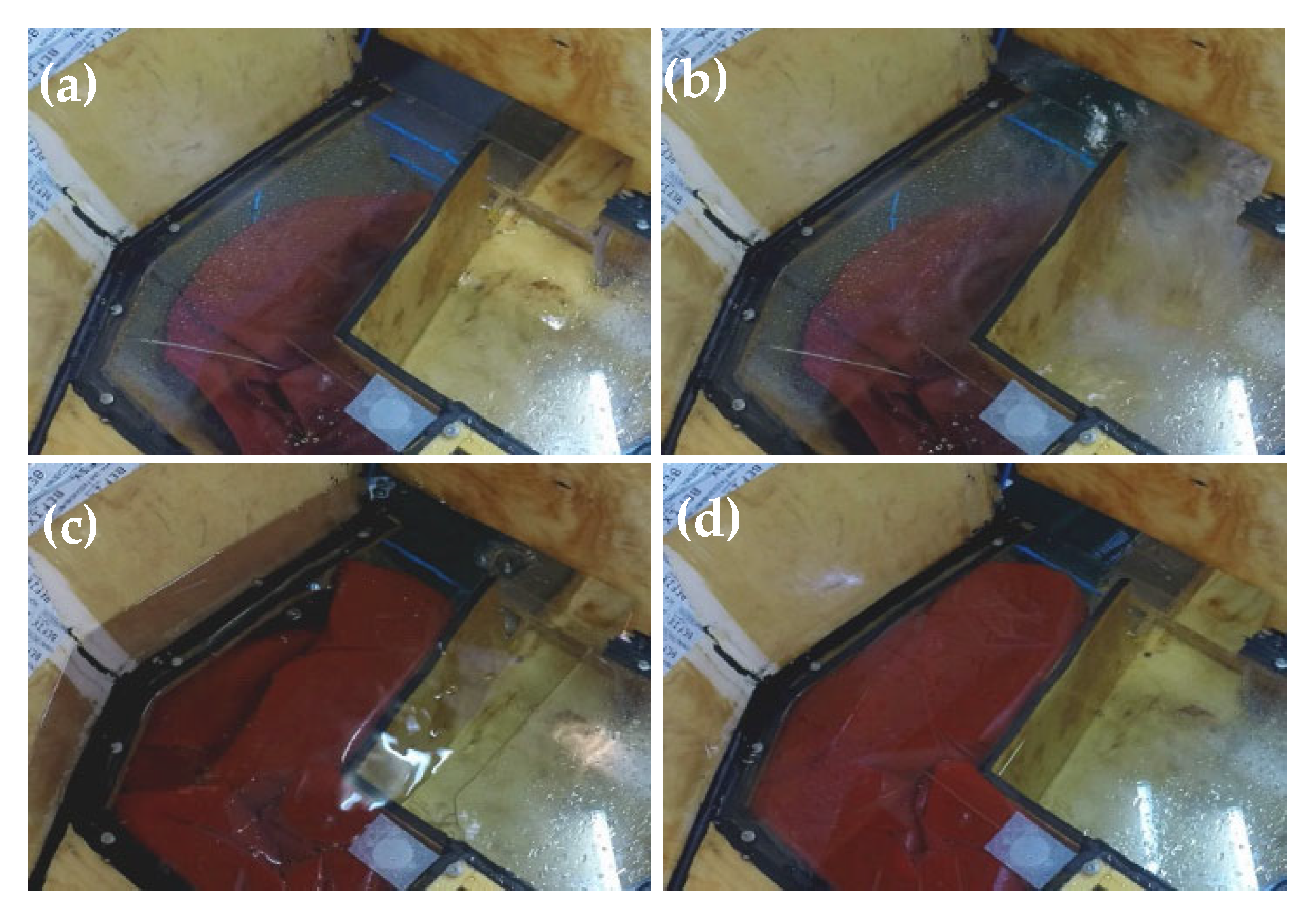
Appendix A.2. Dynamic Inflow into Counter flooding Compartments

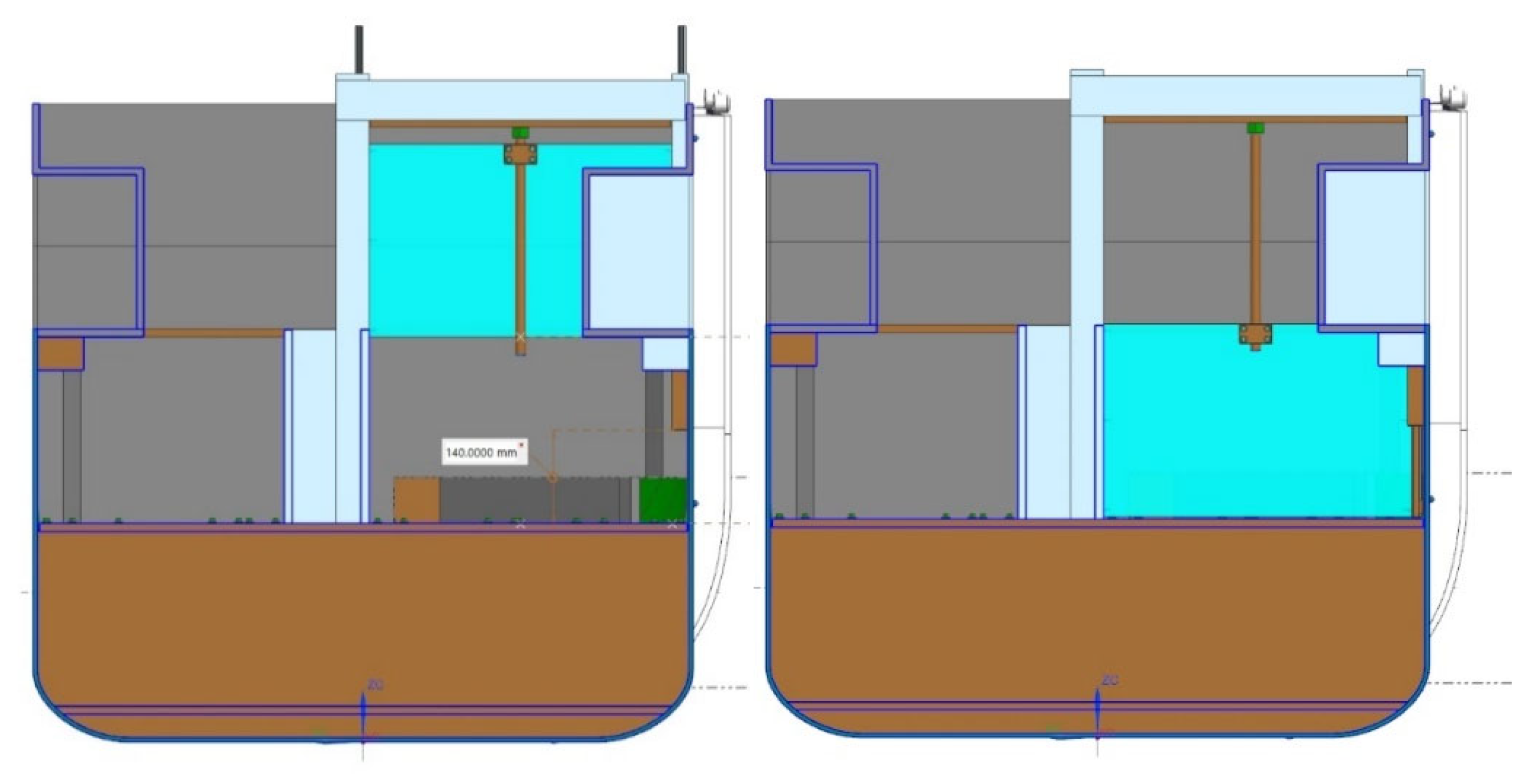
Appendix A.3. Recovery of Lost Buoyancy in a Damaged Compartment by Displacing Flood Water

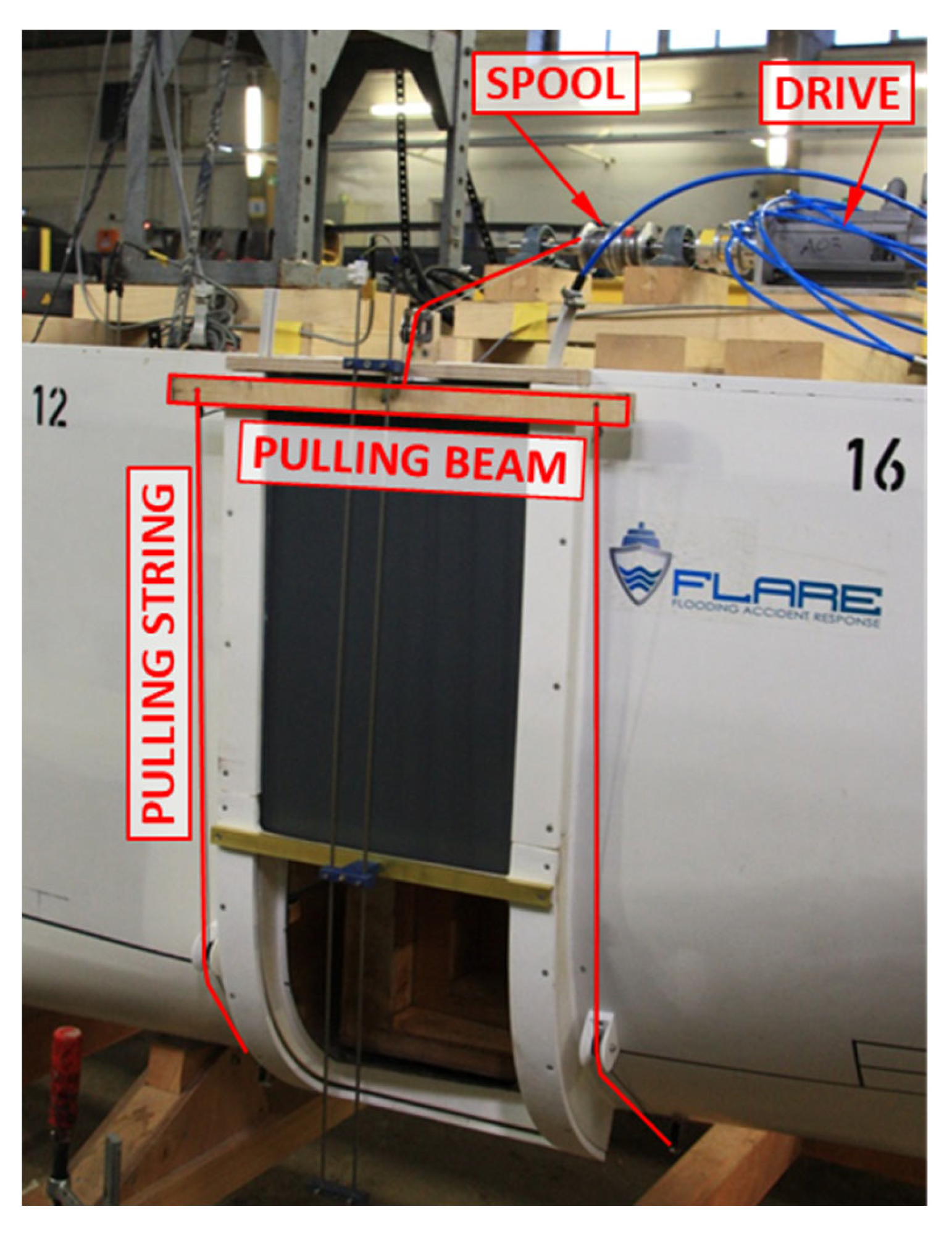
Appendix A.4. Deployment of a Watertight Barrier on the Trailer Deck

Appendix A.5. Damage Opening Time and Start of the Flooding Mitigation
References
- Vassalos, D.; Paterson, D. Reconfiguring Passenger Ship Internal Environment for Damage Stability Enhancement. J. Mar. Sci. Eng. 2020, 8, 693. [Google Scholar] [CrossRef]
- Söding, H. Leckstabilität im Seegang; Institut für Schiffbau der Universität Hamburg, Bericht Nr. 429: Hamburg, Germany, 1982. [Google Scholar]
- Söding, H. Simulation of the Motions of Damaged Ships in Waves; DCAMM Ph.D.-Course Stability of Ships: Lyngby, Denmark, 2002. [Google Scholar]
- Söding, H.; Schigunov, V.; Zorn, T.; Soukup, P. Method Rolls for Simulating Roll Motions of Ships, Ship Technology Research 60(2); University of Duisburg-Essen: Duisburg, Germany, 2013; pp. 70–84. [Google Scholar]
- Valanto, P. Numerical Models—HSVA Rolls; EU-Project Flooding Accident Response (FLARE) Deliverable D4.1: Bremen, Germany, 2020; Volume 2. [Google Scholar]
- Kujanpää, J. Sample Ship No. 6; Deliverable D2.1.6; FLARE: Bremen, Germany, 2019. [Google Scholar]
- Ruponen, P.; Valanto, P.; Acanfora, M.; Dankowski, H.; Lee, G.J.; Mauro, F.; Murphy, A.; Rosano, G.; van’t Veer, R. Results of an international benchmark study on numerical simulation of flooding and motions of a damaged ropax ship. Appl. Ocean. Res. 2022, 123, 103153. [Google Scholar] [CrossRef]
- Valanto, P. Simulation Input for Mitigation of Flooding in WP6, FLARE Deliverable D4.5, Appendix 10 by H. Weede; FLARE: Bremen, Germany, 2021. [Google Scholar]
- Lee, G.J. Dynamic orifice flow model and compartment models for flooding simulation of a damaged ship. Ocean. Eng. 2015, 109, 635–653. [Google Scholar] [CrossRef] [Green Version]



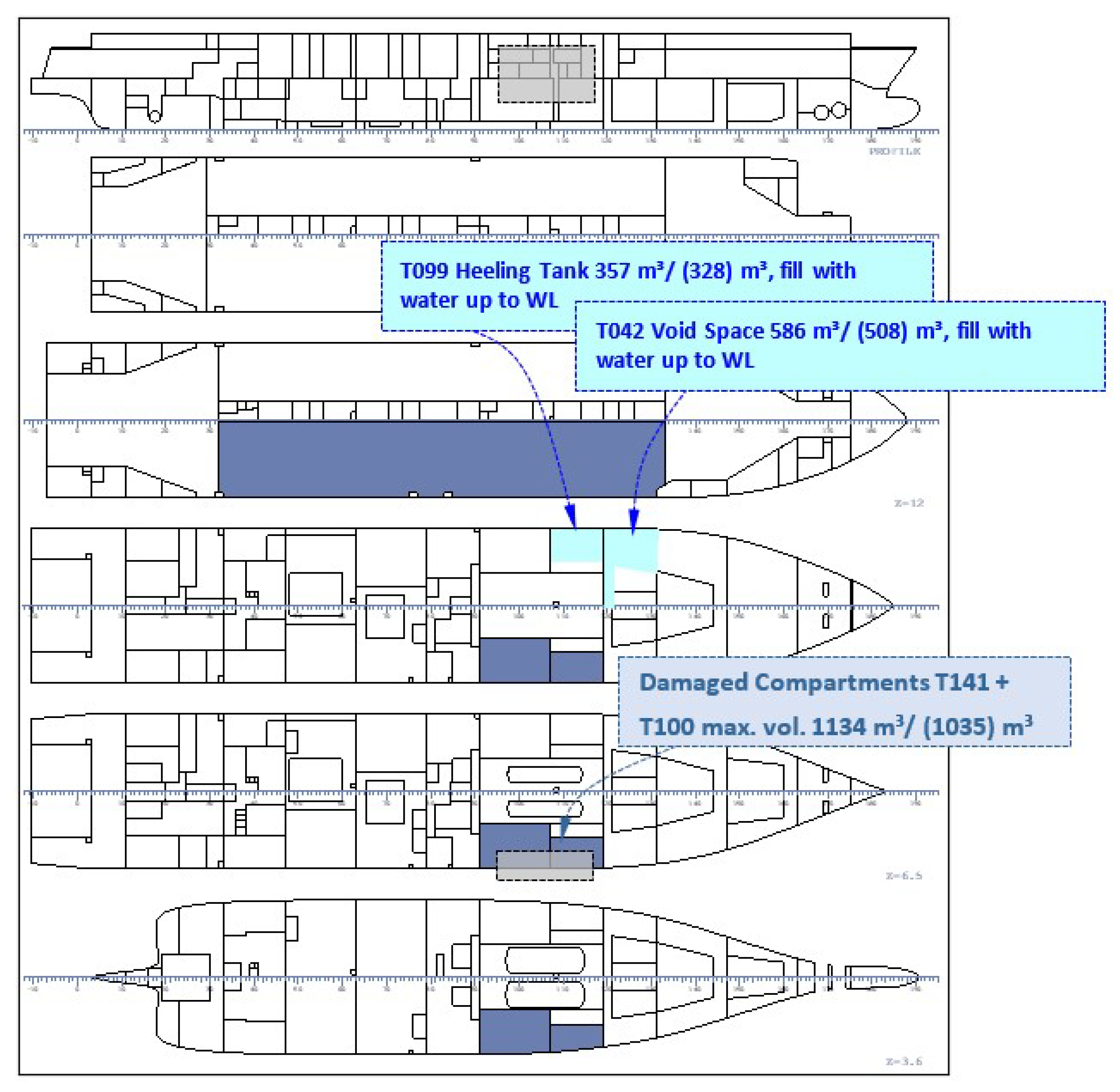







| MT RoPax—HSVA Model No: 5539 | Symbol | Unit | Ship |
|---|---|---|---|
| Length overall | LOA | m | 162.00 |
| Length between perpendiculars | LPP | m | 146.72 |
| Breadth at waterline | BWL | m | 28.00 |
| Draught at aft perpendicular | TA | m | 6.30 |
| Draught at forward perpendicular | TF | m | 6.30 |
| Depth to trailer deck | D | m | 9.20 |
| Displaced volume (bare hull) | ∇BH | m3 | 16,799.4 |
| Block coefficient | CB | - | 0.6522 |
| Intact transverse GM | GM | m | 2.50/3.40 |
| DC2 with GM 3.4 m, DOT 15 s, Counter Flooding Start at 5°, Heel—TTC Shown in [s], 1800 = Survival. Duct Openings from Sea to CF Compartments = 2 × 1.5 m2, Tp = 10.0 s | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| HS [m] | 2.0 | 2.0 | 2.5 | 2.5 | 3.0 | 3.0 | 3.5 | 3.5 | 4.0 | 4.0 |
| Mitigation | No | Yes | No | Yes | No | Yes | No | Yes | No | Yes |
| 1 | 1800 | (1800) | 1800 | 1800 | 496.3 | 1800 | 412.2 | 1800 | 145.9 | 528.7 |
| 2 | 1800 | (1800) | 1800 | 1800 | 230.3 | 1800 | 122.1 | 1800 | 92.1 | 249.9 |
| 3 | 1800 | (1800) | 1150 | 1800 | 410.1 | 1800 | 328.6 | 1800 | 203.9 | 431 |
| 4 | 1800 | (1800) | 459.8 | 1800 | 299.1 | 1800 | 116.6 | 1800 | 80 | 132.8 |
| 5 | 1800 | (1800) | 252.4 | 1800 | 67.5 | 192 | 58.2 | 70.2 | 50.3 | 54.2 |
| 6 | 1800 | (1800) | 466.5 | 1800 | 216.7 | 514.6 | 159.3 | 467.7 | 139.5 | 227.2 |
| 7 | 1800 | (1800) | 356.7 | 1800 | 312 | 1800 | 247.8 | 369 | 222.9 | 279.6 |
| 8 | 1800 | (1800) | 1800 | 1800 | 1800 | 1800 | 380.7 | 1800 | 245.4 | 1800 |
| 9 | 1800 | (1800) | 1800 | 1800 | 202.5 | 1800 | 120.2 | 964.3 | 114.7 | 155.2 |
| 10 | 1800 | (1800) | 1800 | 1800 | 228.4 | 1800 | 89.5 | 1800 | 71.5 | 109.6 |
| Survival | 10/10 | (10/10) | 5/10 | 10/10 | 1/10 | 8/10 | 0/10 | 6/10 | 0/10 | 1/10 |
| DC4 with GM 3.4 m, DOT 15 s, RLB: Displacement of Floodwater in T045 Starts at 75 s, Ends at 255 s, —TTC Shown in [s], 1800 = Survival, Tp = 10.0 s | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| HS [m] | 2.0 | 2.0 | 5.0 | 5.0 | 5.5 | 5.5 | 6.0 | 6.0 | 7.0 | 7.0 |
| Mitigation | No | Yes | No | Yes | No | Yes | No | Yes | No | Yes |
| 1 | 1800 | (1800) | 1800 | 1800 | 1132.6 | 1800 | 843.0 | 1800 | 430.8 | 492.9 |
| 2 | 1800 | (1800) | 1800 | 1800 | 1800 | 1800 | 636.8 | 1800 | 468.6 | 190.0 |
| 3 | 1800 | (1800) | 1800 | 1800 | 706.3 | 1800 | 707.2 | 1800 | 418.8 | 387.8 |
| 4 | 1800 | (1800) | 1800 | 1800 | 1800 | 1800 | 495.6 | 1800 | 218.1 | 249.9 |
| 5 | 1800 | (1800) | 1800 | 1800 | 1800 | 1800 | 1800 | 1800 | 349.7 | 155.9 |
| 6 | 1800 | (1800) | 611.2 | 1800 | 426.4 | 548.5 | 303.6 | 493.1 | 178.1 | 179.4 |
| 7 | 1800 | (1800) | 1187.7 | 1800 | 348.7 | 349.1 | 347.9 | 332.1 | 336.6 | 280.1 |
| 8 | 1800 | (1800) | 1800 | 1800 | 1800 | 1800 | 814.2 | 1800 | 641.3 | 687.5 |
| 9 | 1800 | (1800) | 1800 | 1800 | 890.9 | 1800 | 300.0 | 223.3 | 184.9 | 170.1 |
| 10 | 1800 | (1800) | 1800 | 1800 | 841.5 | 1800 | 842.0 | 1800 | 215.2 | 100.7 |
| Survival | 10/10 | (10/10) | 8/10 | 10/10 | 4/10 | 8/10 | 1/10 | 7/10 | 0/10 | 0/10 |
| DC4 with GM 3.4 m, DOT 15 s, WTB Deployment Time 30 s, TTC Shown in [s], 1800 = Survival, Tp = 10.0 s | ||||||||
|---|---|---|---|---|---|---|---|---|
| HS [m] | 5.0 | 5.0 | 5.5 | 5.5 | 6.0 | 6.0 | 7.0 | 7.0 |
| Mitigation | No | Yes | No | Yes | No | Yes | No | Yes |
| 1 | 1800 | 1800 | 1132.6 | 1800 | 843.1 | 1800 | 430.9 | 1800 |
| 2 | 1800 | 1800 | 1800 | 1800 | 636.9 | 1800 | 468.7 | 1800 |
| 3 | 1800 | 1800 | 706.3 | 1800 | 707.3 | 1800 | 418.9 | 1800 |
| 4 | 1800 | 1800 | 1800 | 1800 | 495.7 | 1800 | 218.2 | 1800 |
| 5 | 1800 | 1800 | 1800 | 1800 | 1800 | 1800 | 349.8 | 1800 |
| 6 | 611.3 | 1800 | 426.4 | 1800 | 303.7 | 1800 | 178.2 | 1800 |
| 7 | 1187.8 | 1800 | 348.7 | 1800 | 348.0 | 1800 | 336.7 | 1800 |
| 8 | 1800 | 1800 | 1800 | 1800 | 814.3 | 1800 | 641.4 | 1800 |
| 9 | 1800 | 1800 | 890.9 | 1800 | 300.1 | 1800 | 185.0 | 1800 |
| 10 | 1800 | 1800 | 841.5 | 1800 | 842.1 | 1800 | 215.3 | 1800 |
| Survival | 8/10 | 10/10 | 4/10 | 10/10 | 1/10 | 10/10 | 0/10 | 10/10 |
| DC2 | Calm | HS 2.5 m | HS 3.0 m | HS 3.5 m | HS 3.5 m | HS 4.0 m | HS 5.0 m | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| GM [m] | 3.018 | 3.4 | 3.4 | 3.4 | 3.48 | 3.4 | 3.48 | |||||||||
| DOT [s] | 15 | 30 | 15 | 15 | 15 | 15 | 15 | 15 | ||||||||
| CF | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes |
| Survival rate | 0/5 | 5/5 | 0/6 | 5/6 | 5/10 | 10/10 | 1/10 | 8/10 | 0/10 | 6/10 | 2/10 | 10/10 | 0/10 | 1/10 | 0/10 | 10/10 |
 |  |  |  |  |  |  |  | |||||||||
| DC4 | Calm | HS 2.5 m | HS 4.0 m | HS 5.0 m | HS 5.5 m | HS 6.0 m | HS 7.0 m | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| GM [m] | 3.71 | 3.81 | 3.81 | 3.4 | 3.4 | 3.4 | 3.4 | |||||||||
| DOT [s] | 15 | 30 | 15 | 15 | 15 | 15 | 15 | 15 | ||||||||
| RLB | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes |
| Survival rate | 0/5 | 5/5 | 0/5 | 5/5 | 0/10 | 10/10 | 0/10 | 8/10 | 8/10 | 10/10 | 4/10 | 6/10 | 1/10 | 4/10 | 0/10 | 0/10 |
 |  |  |  |  |  |  |  | |||||||||
| DC4 | Calm | HS 2.5 m | HS 4.0 m | HS 5.0 m | HS 5.5 m | HS 6.0 m | HS 7.0 m | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| GM [m] | 3.3 | 3.71 | 3.71 | 3.4 | 3.4 | 3.4 | 3.4 | |||||||||
| DOT [s] | 15 | 30 | 15 | 15 | 15 | 15 | 15 | 15 | ||||||||
| WTB | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes | no | yes |
| Survival rate | 0/5 | 5/5 | 0/5 | 5/5 | 0/10 | 10/10 | 0/10 | 10/10 | 8/10 | 10/10 | 4/10 | 10/10 | 1/10 | 10/10 | 0/10 | 10/10 |
 |  |  |  |  |  |  |  | |||||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Valanto, P. Active Flooding Mitigation for Stability Enhancement in a Damaged RoPax Ship. J. Mar. Sci. Eng. 2022, 10, 797. https://doi.org/10.3390/jmse10060797
Valanto P. Active Flooding Mitigation for Stability Enhancement in a Damaged RoPax Ship. Journal of Marine Science and Engineering. 2022; 10(6):797. https://doi.org/10.3390/jmse10060797
Chicago/Turabian StyleValanto, Petri. 2022. "Active Flooding Mitigation for Stability Enhancement in a Damaged RoPax Ship" Journal of Marine Science and Engineering 10, no. 6: 797. https://doi.org/10.3390/jmse10060797
APA StyleValanto, P. (2022). Active Flooding Mitigation for Stability Enhancement in a Damaged RoPax Ship. Journal of Marine Science and Engineering, 10(6), 797. https://doi.org/10.3390/jmse10060797





