Modeling and Dynamic Analysis of a Triple-Tagline Anti-Swing System for Marine Cranes in an Offshore Environment
Abstract
:1. Introduction
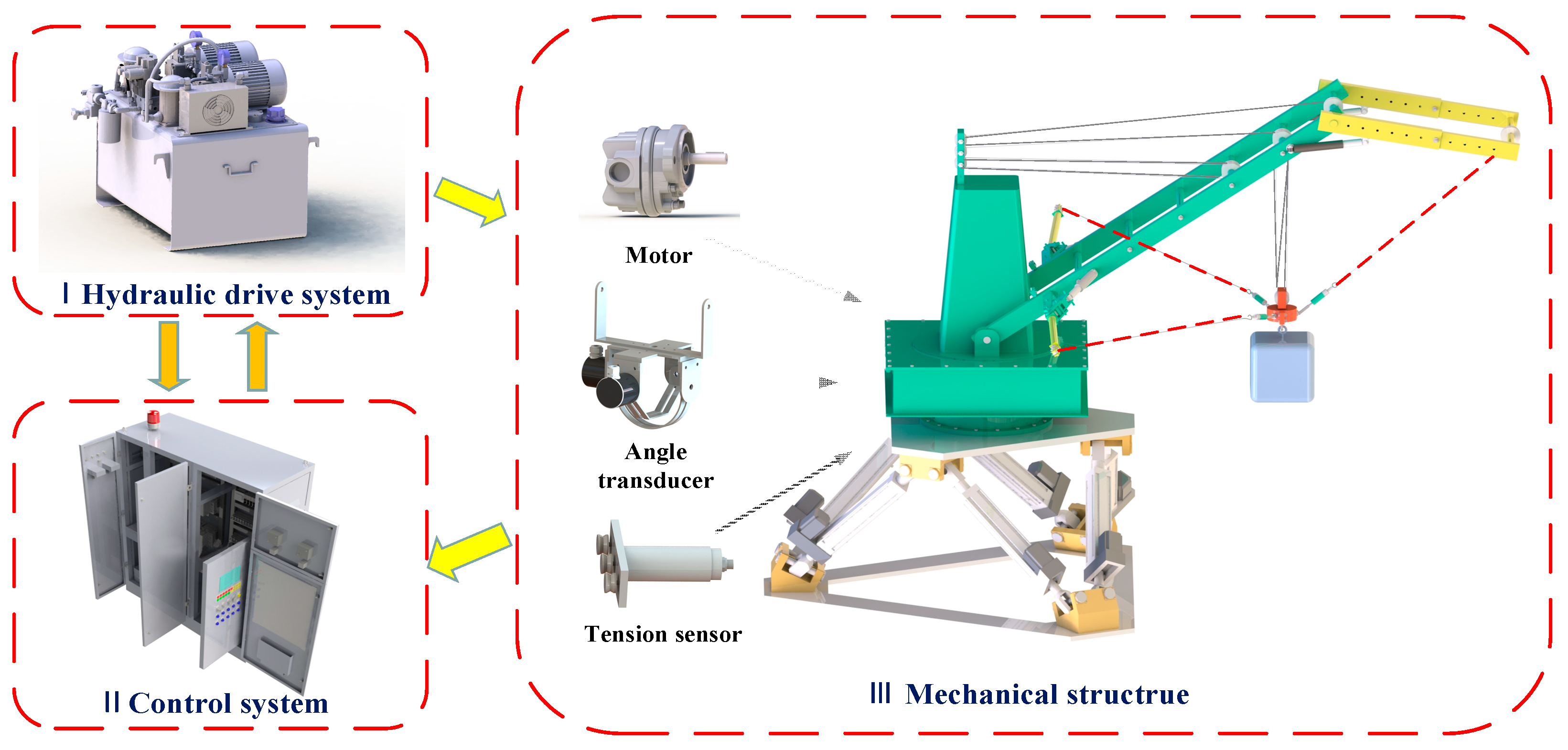
2. TTAS Overall Architecture
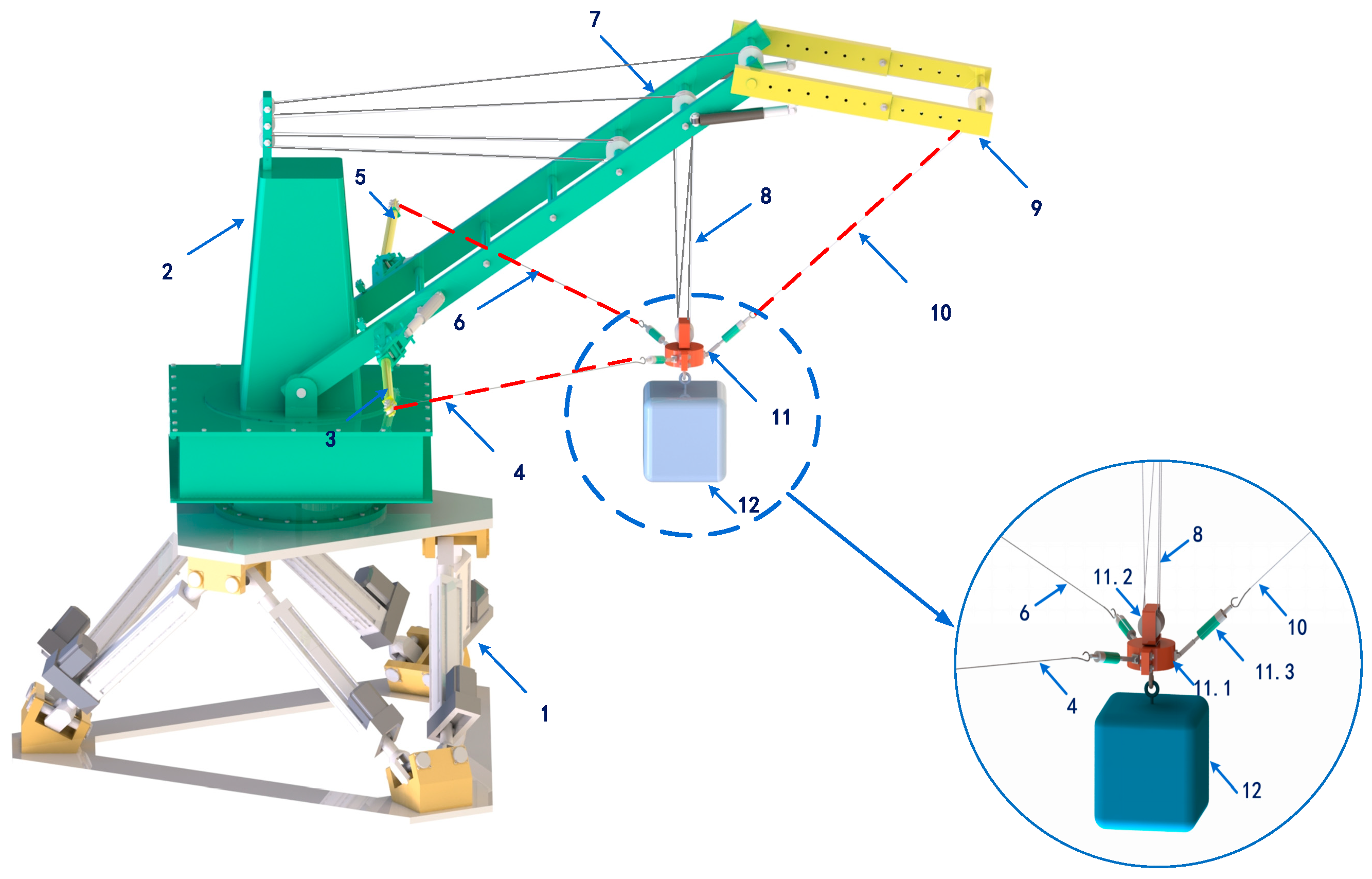
3. Structure of TTAS
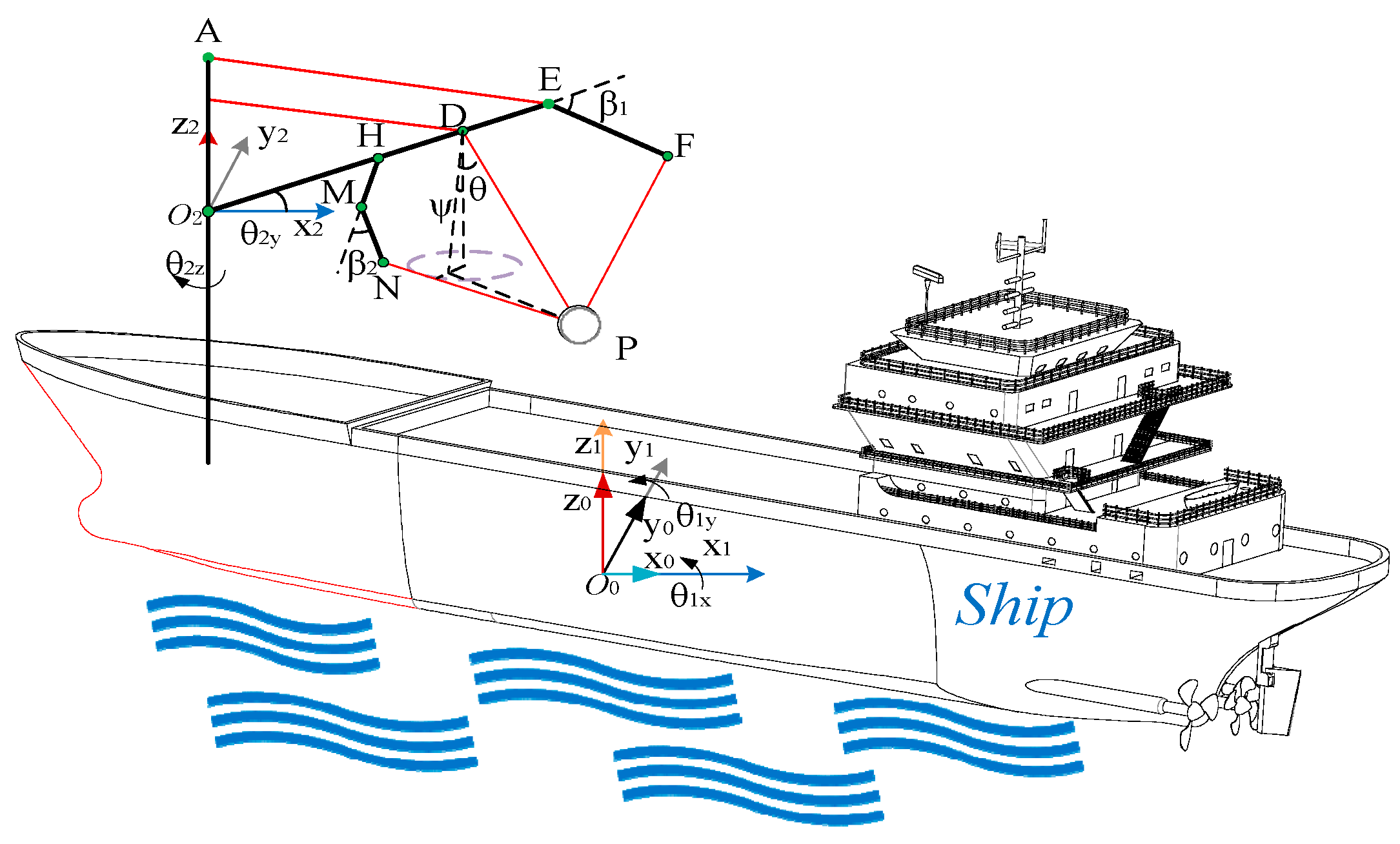
4. Dynamic Modeling of TTAS
- (1)
- The jibs of marine cranes equipped with TTAS are rigid bodies.
- (2)
- The elastic deformation of the three taglines and the hoist cable is ignored.
- (3)
- The hook and payload can be considered a mass point.
- (4)
- The dynamic model of TTAS is established when the crane is stationary. It does not consider the crane’s own rotation and luffing.
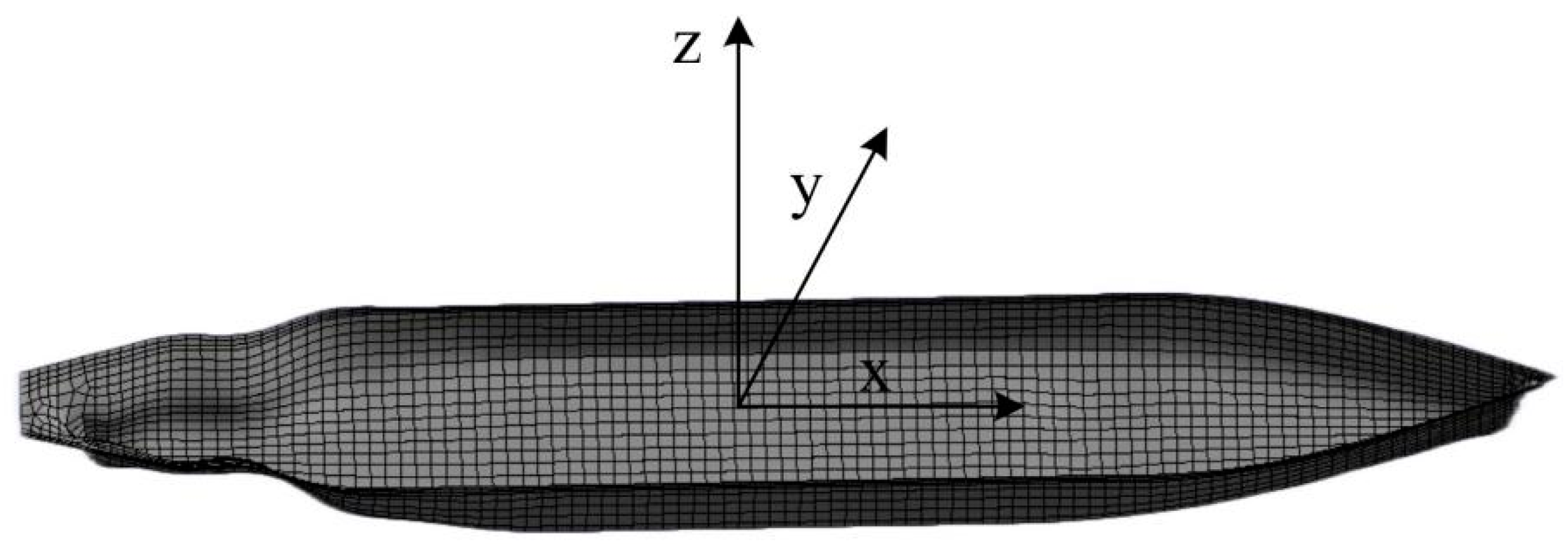
4.1. Wave-Load Model
4.2. Kinematic Model
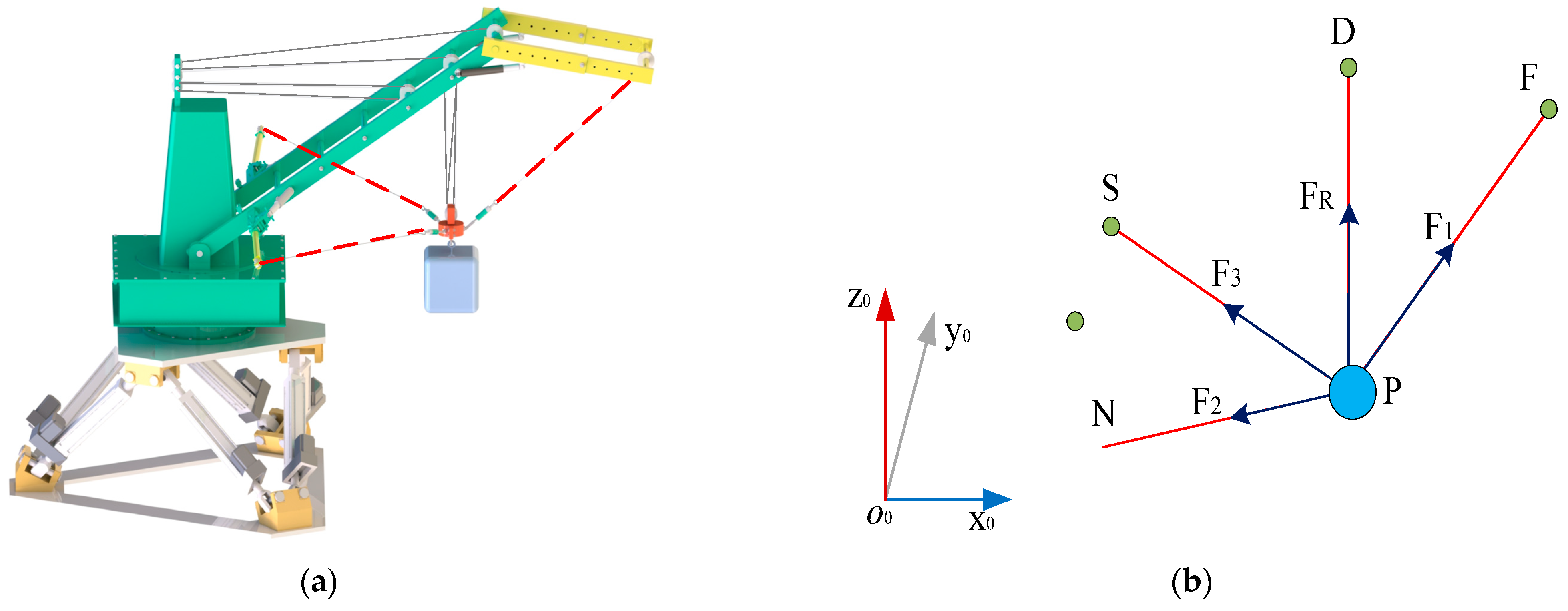
4.3. Statics Model
4.4. Dynamics Model
5. Dynamic Analysis
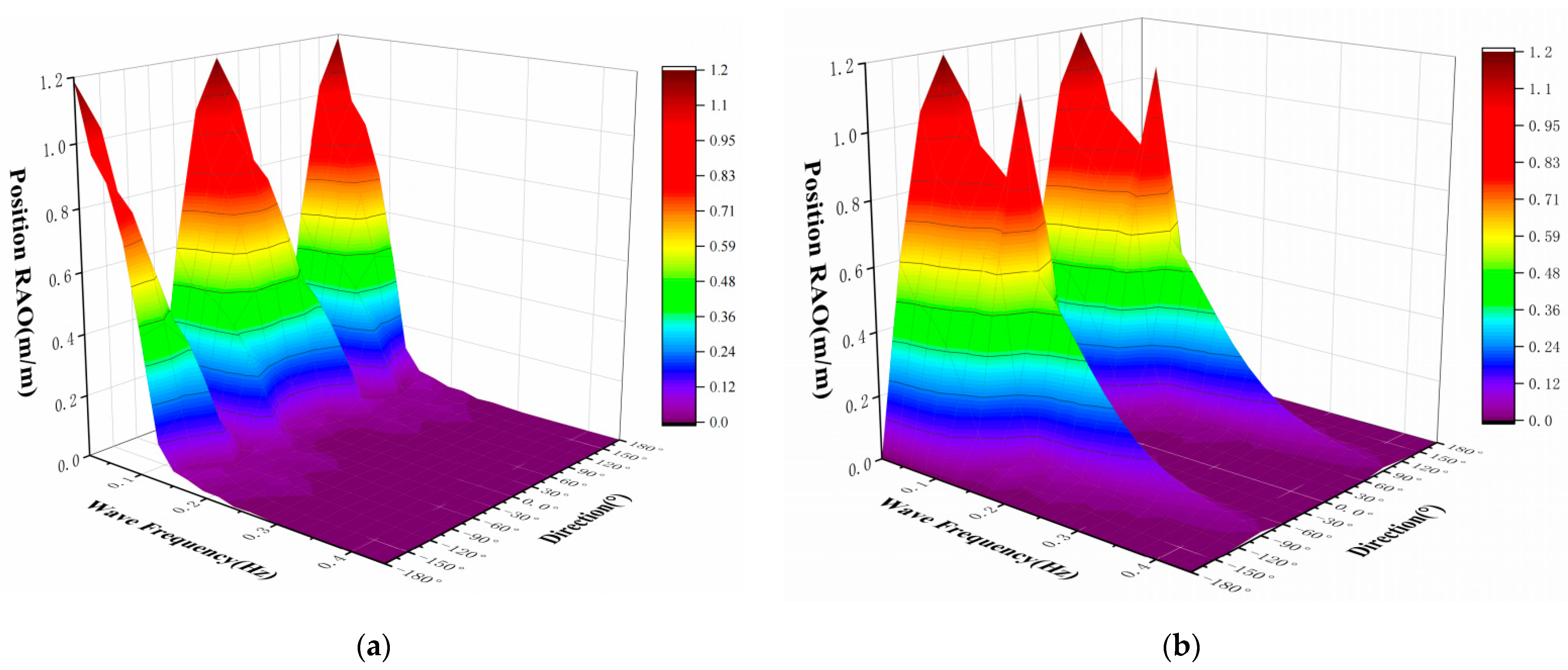
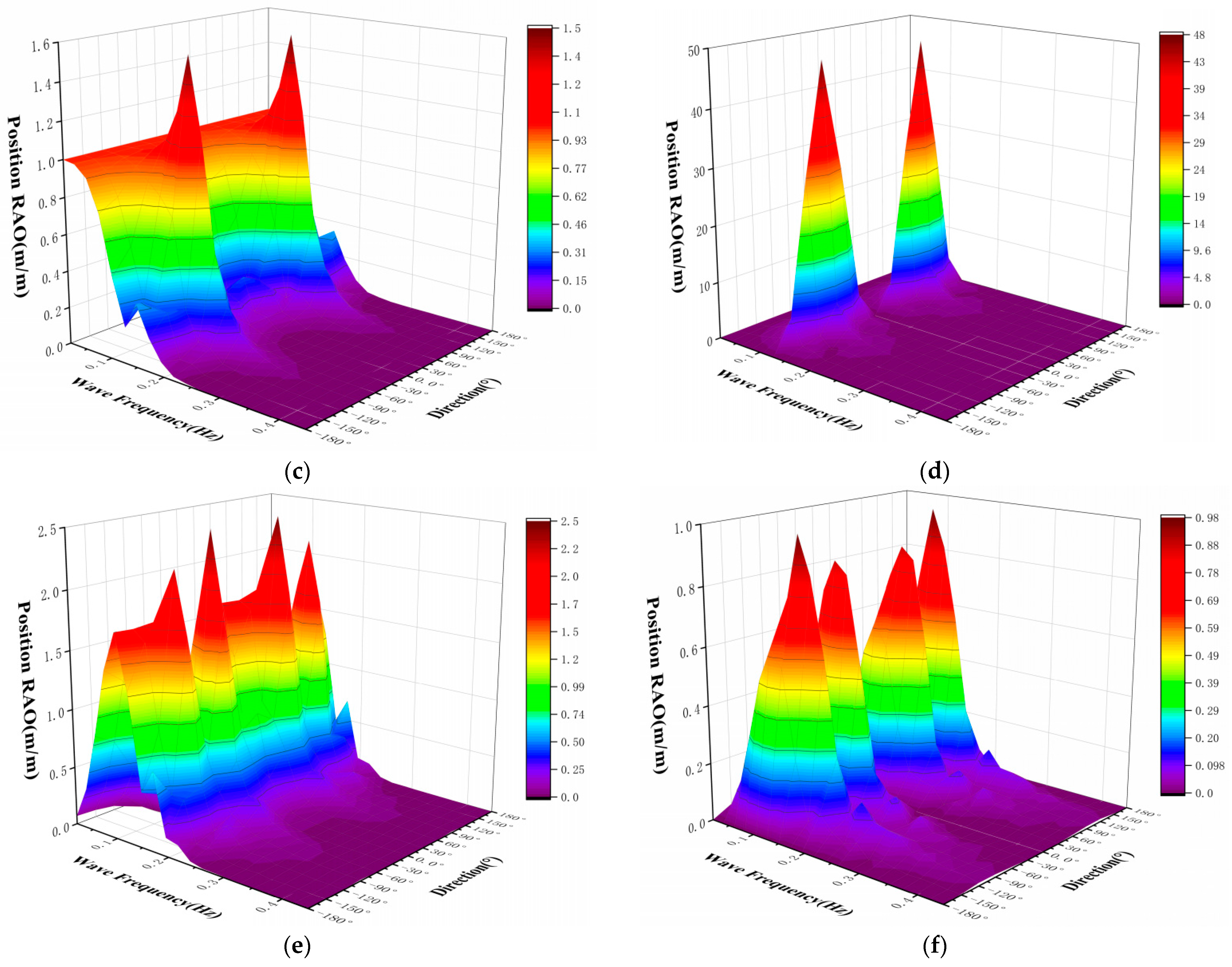
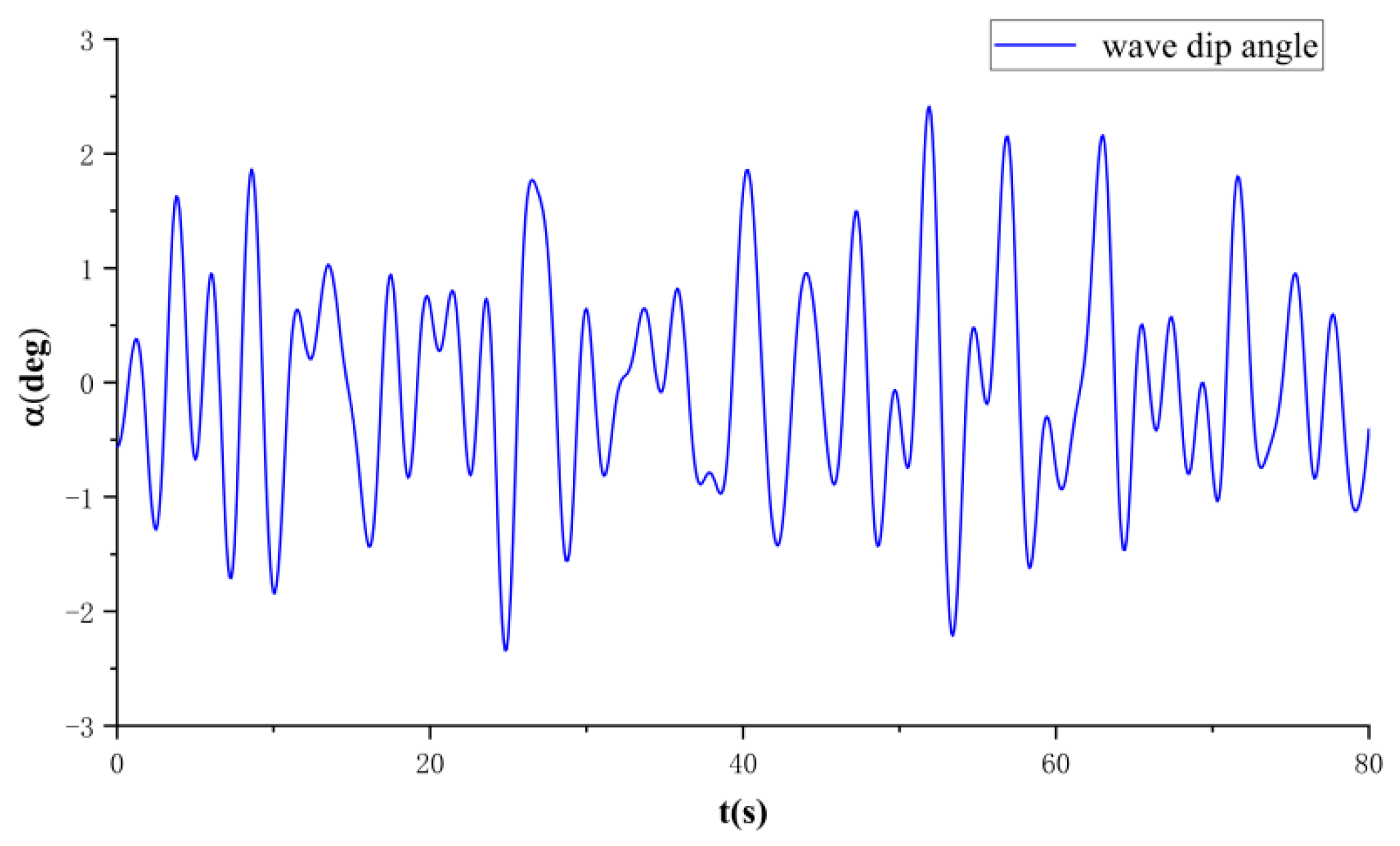
5.1. Wave-Load Model Simulation
5.2. Regular Environment Excitation Simulation
6. Conclusions
- (1)
- The irregular wave-load model was integrated into the TTAS dynamic system model, and the TTAS dynamic system model was simplified as a constrained-pendulum system with moving base excitations. Furthermore, the dynamic system model was established by applying the methods in robotics.
- (2)
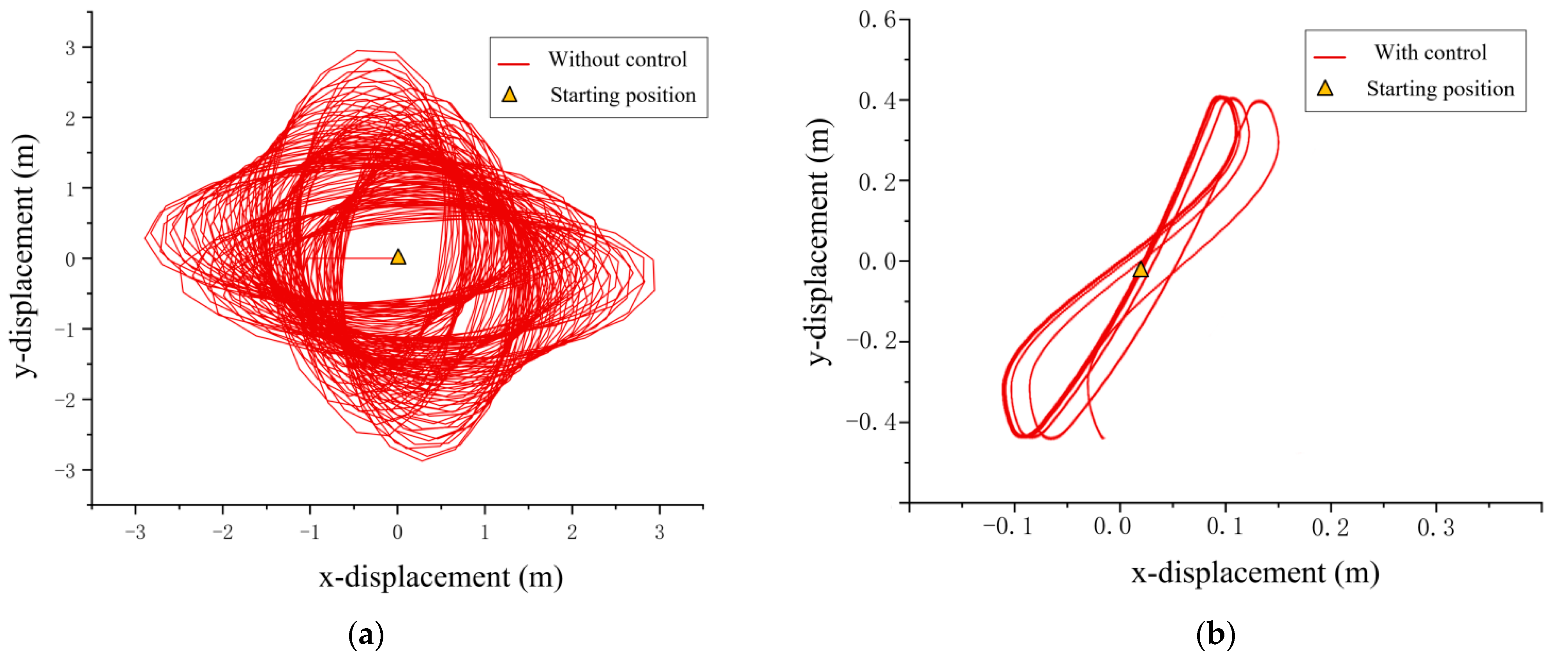
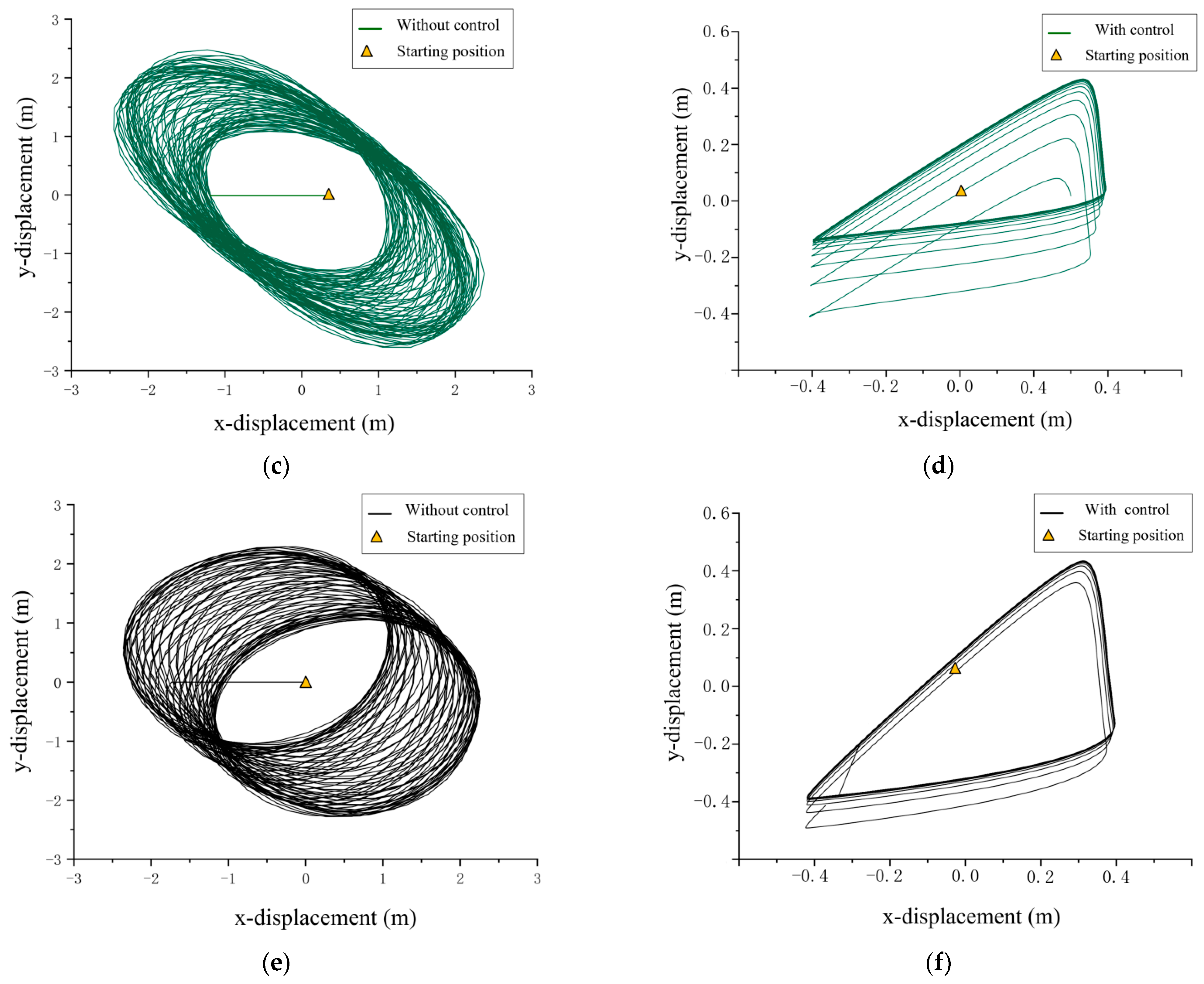
- Under irregular environment excitation, the average amplitude of the in-plane angle is reduced by 63%, and that of the out-of-plane angle is reduced by 82% using TTAS. Moreover, the two-dimensional trajectory of the payload is reduced by 92%.
- (3)
- Under regular environment excitation, it was found that the shape of the two-dimensional trajectory of the payload is elliptic without anti-swing control, and it is generally 8-shaped or triangular with anti-swing control. By applying anti-swing control, the two-dimensional trajectory of the payload is reduced by more than 90%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Smoczek, J.; Szpytko, J.; Hyla, P. The Application of an Intelligent Crane Control System. IFAC Proc. Vol. 2012, 45, 280–285. [Google Scholar] [CrossRef]
- Chang, C.Y. The switching algorithm for the control of overhead crane. Neural Comput. Appl. 2006, 15, 350–358. [Google Scholar] [CrossRef]
- Mc, A.; Lta, B. A family of anti-swing motion controllers for 2D-cranes with load hoisting/lowering—ScienceDirect. Mech. Syst. Signal Processing 2019, 133, 106253. [Google Scholar]
- Belunce, A.; Pandolfo, V.; Roozbahani, H.; Handroos, H. Novel Control Method for Overhead Crane’s Load Stability. Procedia Eng. 2015, 106, 108–125. [Google Scholar] [CrossRef]
- Tran, Q.; Huh, J.; Nguyen, V.; Kang, C.; Ahn, J.H.; Park, I.J. Sensitivity Analysis for Ship-to-Shore Container Crane Design. Appl. Sci. 2018, 8, 1667. [Google Scholar] [CrossRef]
- Maghsoudi, M.J.; Ramli, L.; Sudin, S.; Mohamed, Z.; Wahid, H. Improved unity magnitude input shaping scheme for sway control of an underactuated 3D overhead crane with hoisting. Mech. Syst. Signal Process. 2019, 123, 466–482. [Google Scholar] [CrossRef]
- Yuan, G.H.; Hunt, B.R.; Grebogi, C.; Ott, E.; Kostelich, E.J. Design and Control of Shipboard Cranes. In Proceedings of the ASME 1997 Design Engineering Technical Conferences, Sacramento, CA, USA, 14–17 September 1997. [Google Scholar]
- Bing, W.; Homaifar, A.; Bikdash, M.; Kimiaghalam, B. Modeling and optimal control design of shipboard crane. In Proceedings of the American Control Conference, San Diego, CA, USA, 2–4 June 1999. [Google Scholar]
- Kimiaghalam, B.; Homaifar, A.; Bikdash, M. Feedback and feedforward control law for a ship crane with Maryland rigging system. In Proceedings of the American Control Conference, Chicago, IL, USA, 28–30 June 2000. [Google Scholar]
- Albada, S.B.V.; Albada, G.D.V.; Hildre, H.P.; Zhang, H. A Novel Approach to Anti-Sway Control for Marine Shipboard Cranes. In Proceedings of the 27th Conference on Modelling and Simulation, Ålesund, Norway, 27–30 May 2013. [Google Scholar]
- Yan, M.; Ma, X.; Bai, W.; Lin, Z.; Li, Y. Numerical Simulation of Wave Interaction with Payloads of Different Postures Using OpenFOAM. J. Mar. Sci. Eng. 2020, 8, 433. [Google Scholar] [CrossRef]
- Fragopoulos, D.; Spathopoulos, M.P.; Zheng, Y. A pendulation control system for offshore lifting operations-ScienceDirect. IFAC Proc. Vol. 1999, 32, 1475–1480. [Google Scholar] [CrossRef]
- Ren, Z.; Verma, A.S.; Ataei, B.; Halse, K.H.; Hildre, H.P. Model-free anti-swing control of complex-shaped payload with offshore floating cranes and a large number of lift wires. Ocean Eng. 2021, 228, 108868. [Google Scholar] [CrossRef]
- Küchler, S.; Mahl, T.; Neupert, J.; Schneider, K.; Sawodny, O. Active Control for an Offshore Crane Using Prediction of the Vessel’s Motion. IEEE ASME Trans. Mechatron. 2011, 16, 297–309. [Google Scholar] [CrossRef]
- Küchler, S.; Sawodny, O. Nonlinear control of an active heave compensation system with time-delay. In Proceedings of the IEEE International Conference on Control Applications, Yokohama, Japan, 8–10 September 2010. [Google Scholar]
- Ngo, Q.H. Adaptive sliding mode control of container cranes. Control Theory Appl. IET 2012, 6, 662–668. [Google Scholar] [CrossRef]
- Kharola, A.; Patil, P.P. Automated Control and Optimisation of Overhead Cranes. Int. J. Manuf. 2017, 7, 41–68. [Google Scholar] [CrossRef]
- Ismail, R.; That, N.D.; Ha, Q.P. Modelling and robust trajectory following for offshore container crane systems. Autom. Constr. 2015, 59, 179–187. [Google Scholar] [CrossRef]
- Ku, N.K.; Cha, J.H.; Roh, M.I.; Lee, K.Y. A tagline proportional-derivative control method for the anti-swing motion of a heavy load suspended by a floating crane in waves. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2013, 227, 357–366. [Google Scholar] [CrossRef]
- Hu, Y.; Tao, L.; Wei, L. Anti-pendulation analysis of parallel wave compensation systems. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2014, 230, 268–271. [Google Scholar] [CrossRef]
- Martin, I.A.; Irani, R.A. A generalized approach to anti-sway control for shipboard cranes. Mech. Syst. Signal Processing 2021, 148, 107168. [Google Scholar] [CrossRef]
- Martin, I.A.; Irani, R.A. Dynamic modeling and self-tuning anti-sway control of a seven degree of freedom shipboard knuckle boom crane. Mech. Syst. Signal Process. 2021, 153, 107441. [Google Scholar]
- Jin, Y.P.; Wan, B.Y.; Liu, D.S.; Peng, Y.D.; Guo, Y. Dynamic analysis of launch & recovery system of seafloor drill under irregular waves. Ocean Eng. 2016, 117, 321–331. [Google Scholar] [CrossRef]
- Lu, B.; Fang, Y.; Sun, N.; Wang, X. Antiswing Control of Offshore Boom Cranes with Ship Roll Disturbances. IEEE Trans. Control. Syst. Technol. 2017, 26, 740–747. [Google Scholar] [CrossRef]



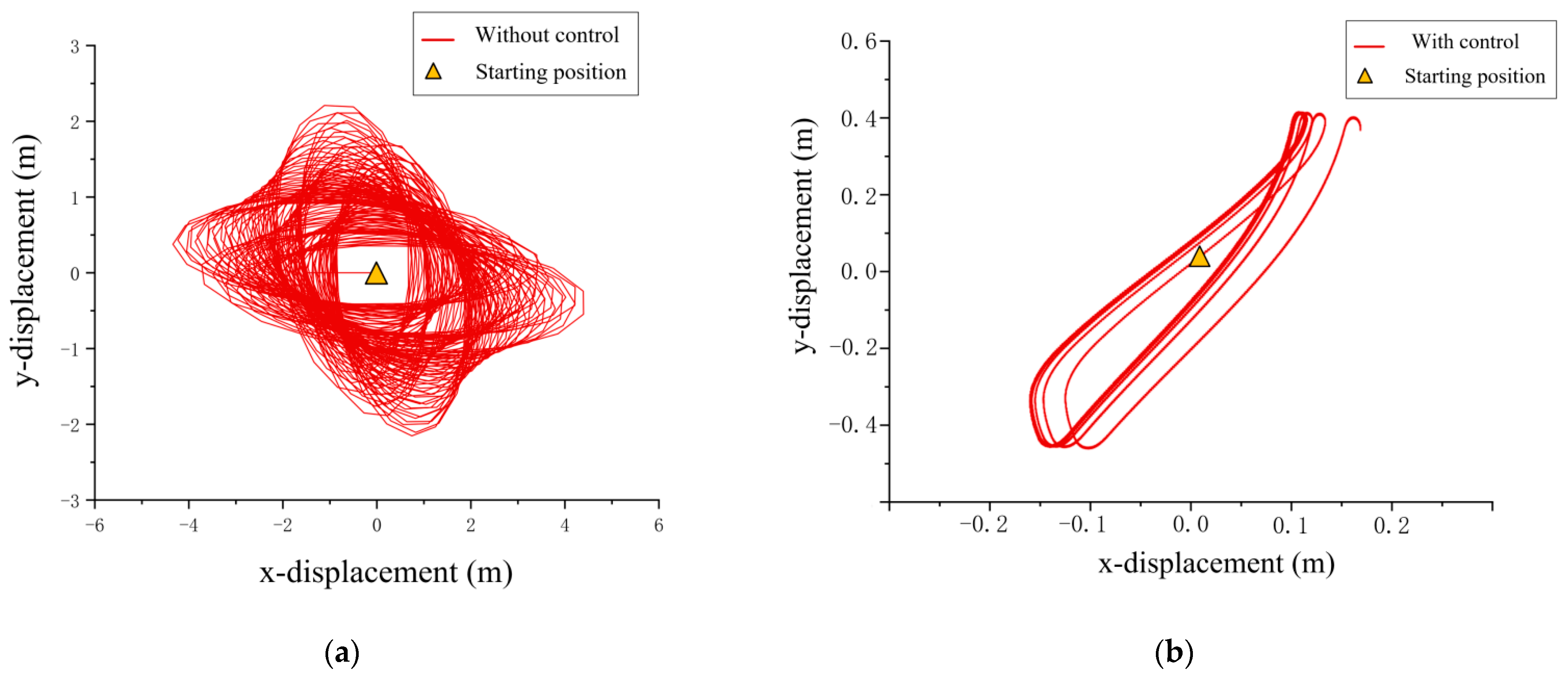
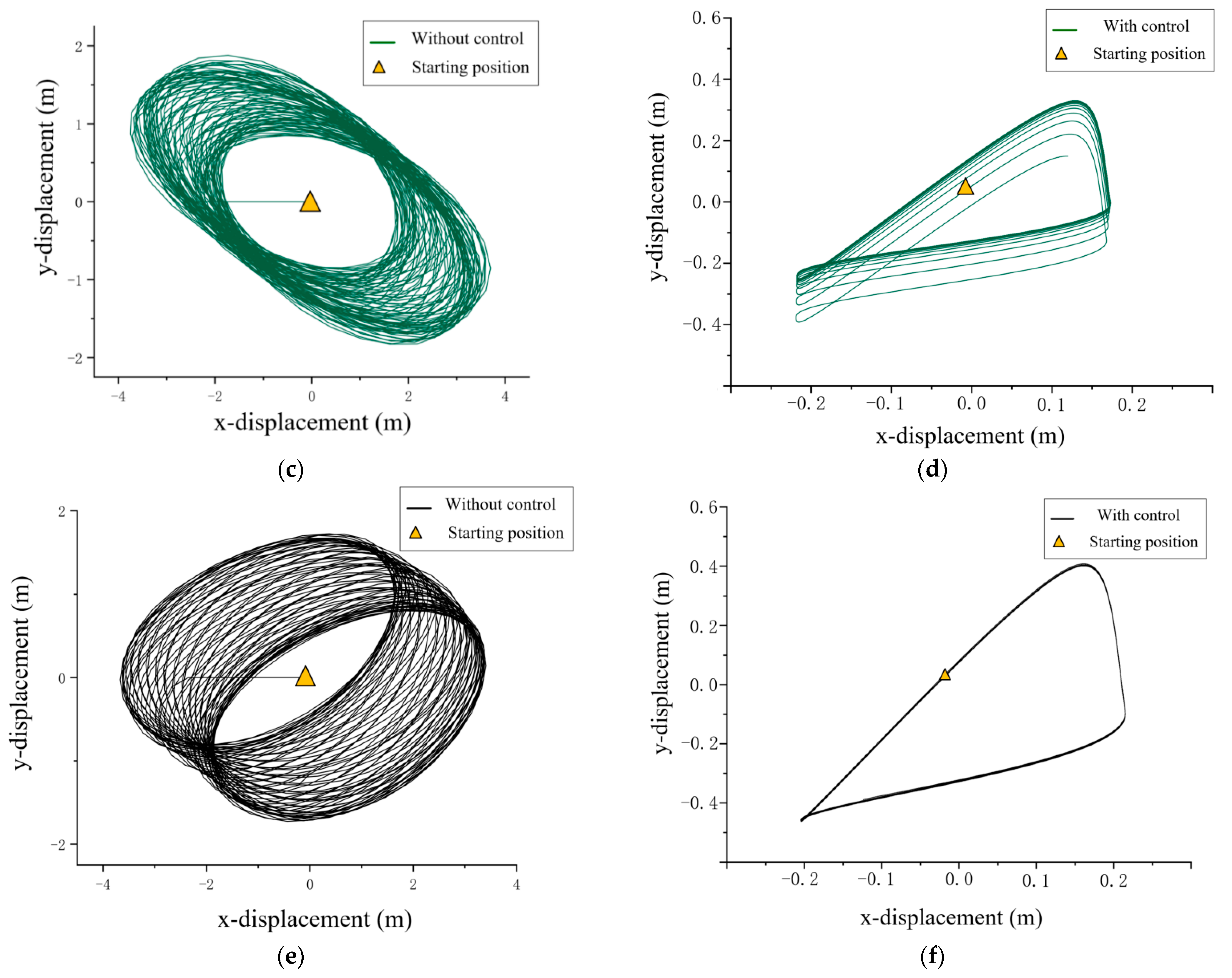
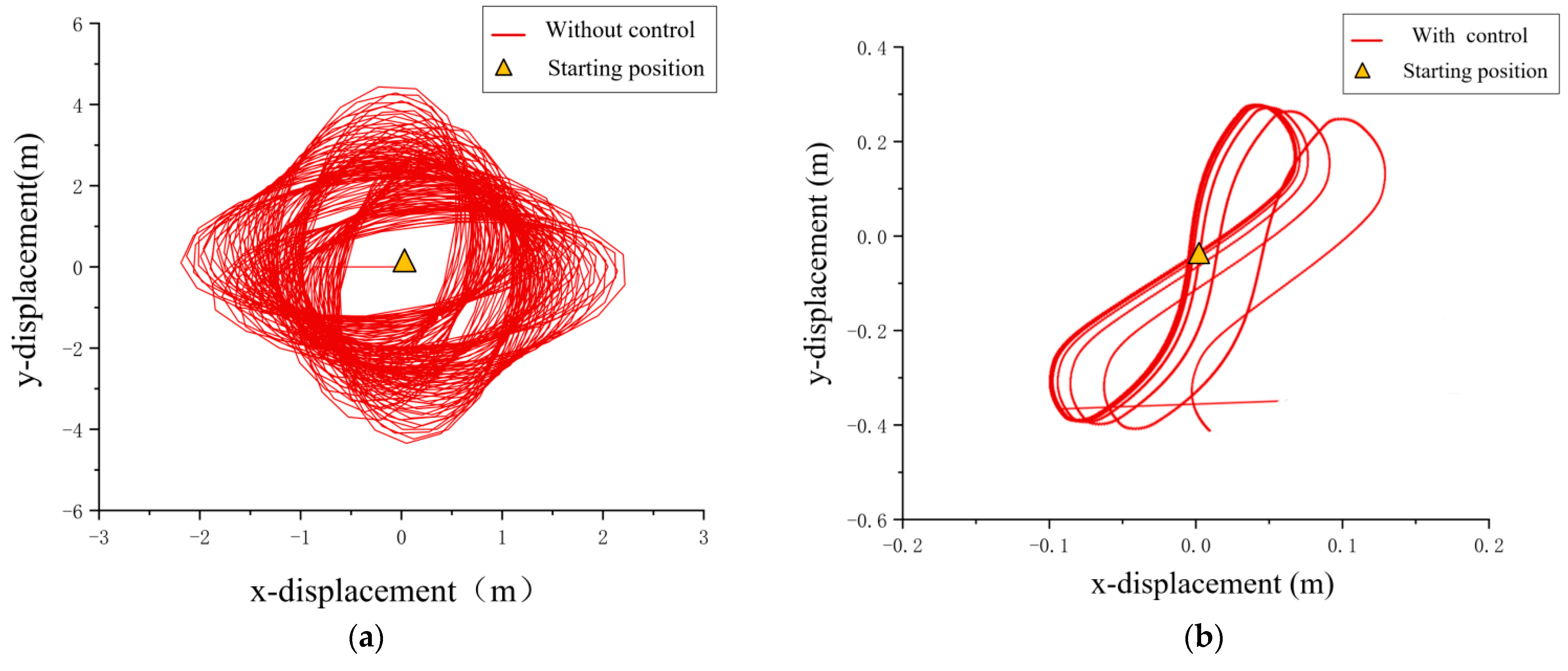
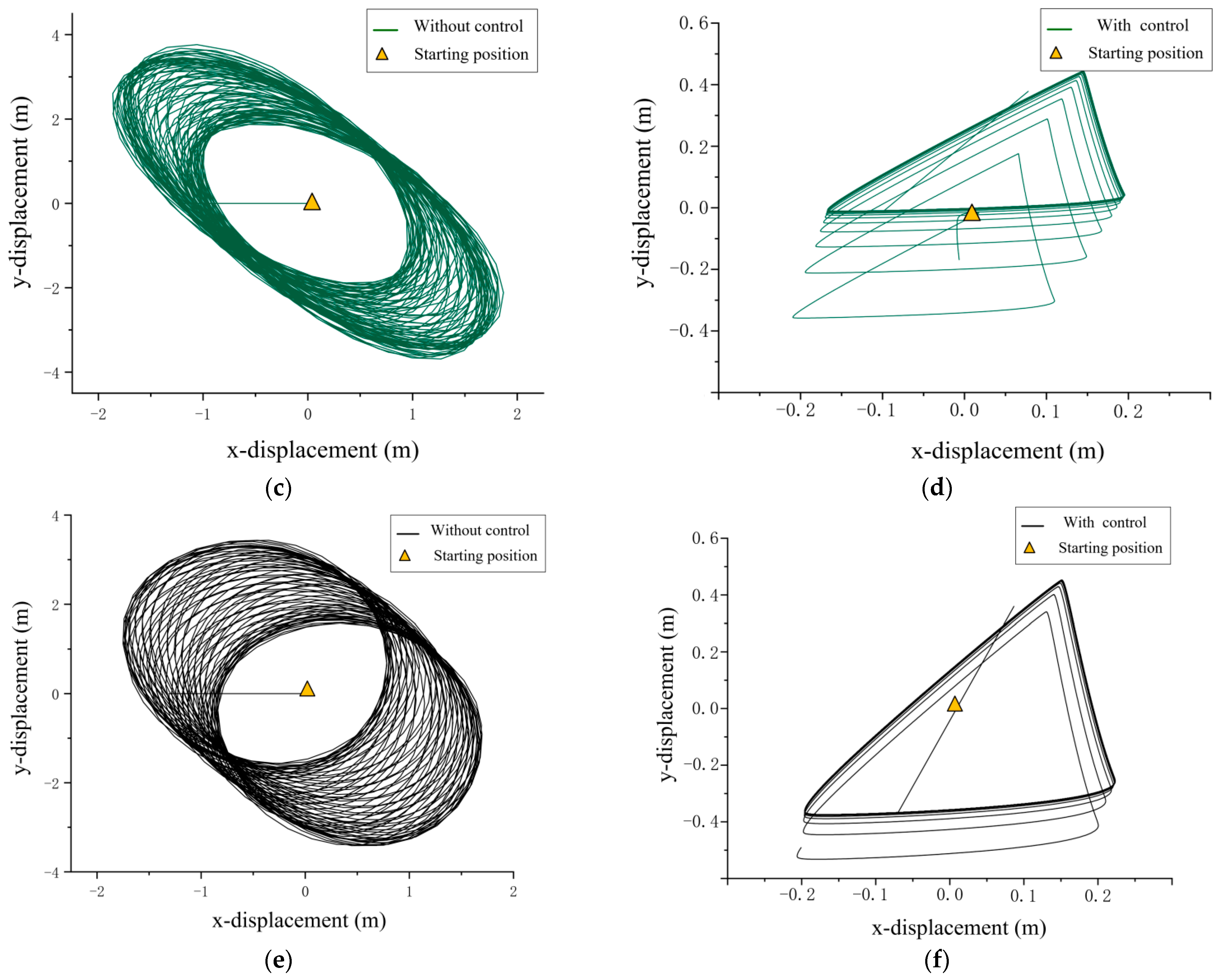
| Parameters | Value | Parameters | Value |
|---|---|---|---|
| l | 1.20 m | Lz | 0.42 m |
| LOD | 1.20 m | β1 | 0° |
| LOE | 1.20 m | β2 | 10° |
| LEF | 0.50 m | θ1x | 0 |
| LOH | 0.32 m | θ1y | 6sin (πt/3) |
| LHM | 0.25 m | θ2y | 45° |
| LMN | 0.75 m | θ2z | 0° |
| Lx | 0 m | m | 25 kg |
| Ly | 0 m | g | 9.8 m/s2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, M.; Wang, S.; Han, G.; An, L.; Chen, H.; Sun, Y. Modeling and Dynamic Analysis of a Triple-Tagline Anti-Swing System for Marine Cranes in an Offshore Environment. J. Mar. Sci. Eng. 2022, 10, 1146. https://doi.org/10.3390/jmse10081146
Sun M, Wang S, Han G, An L, Chen H, Sun Y. Modeling and Dynamic Analysis of a Triple-Tagline Anti-Swing System for Marine Cranes in an Offshore Environment. Journal of Marine Science and Engineering. 2022; 10(8):1146. https://doi.org/10.3390/jmse10081146
Chicago/Turabian StyleSun, Maokai, Shenghai Wang, Guangdong Han, Lin An, Haiquan Chen, and Yuqing Sun. 2022. "Modeling and Dynamic Analysis of a Triple-Tagline Anti-Swing System for Marine Cranes in an Offshore Environment" Journal of Marine Science and Engineering 10, no. 8: 1146. https://doi.org/10.3390/jmse10081146
APA StyleSun, M., Wang, S., Han, G., An, L., Chen, H., & Sun, Y. (2022). Modeling and Dynamic Analysis of a Triple-Tagline Anti-Swing System for Marine Cranes in an Offshore Environment. Journal of Marine Science and Engineering, 10(8), 1146. https://doi.org/10.3390/jmse10081146








