Hydroelastic Response to the Effect of Current Loads on Floating Flexible Offshore Platform
Abstract
:1. Introduction
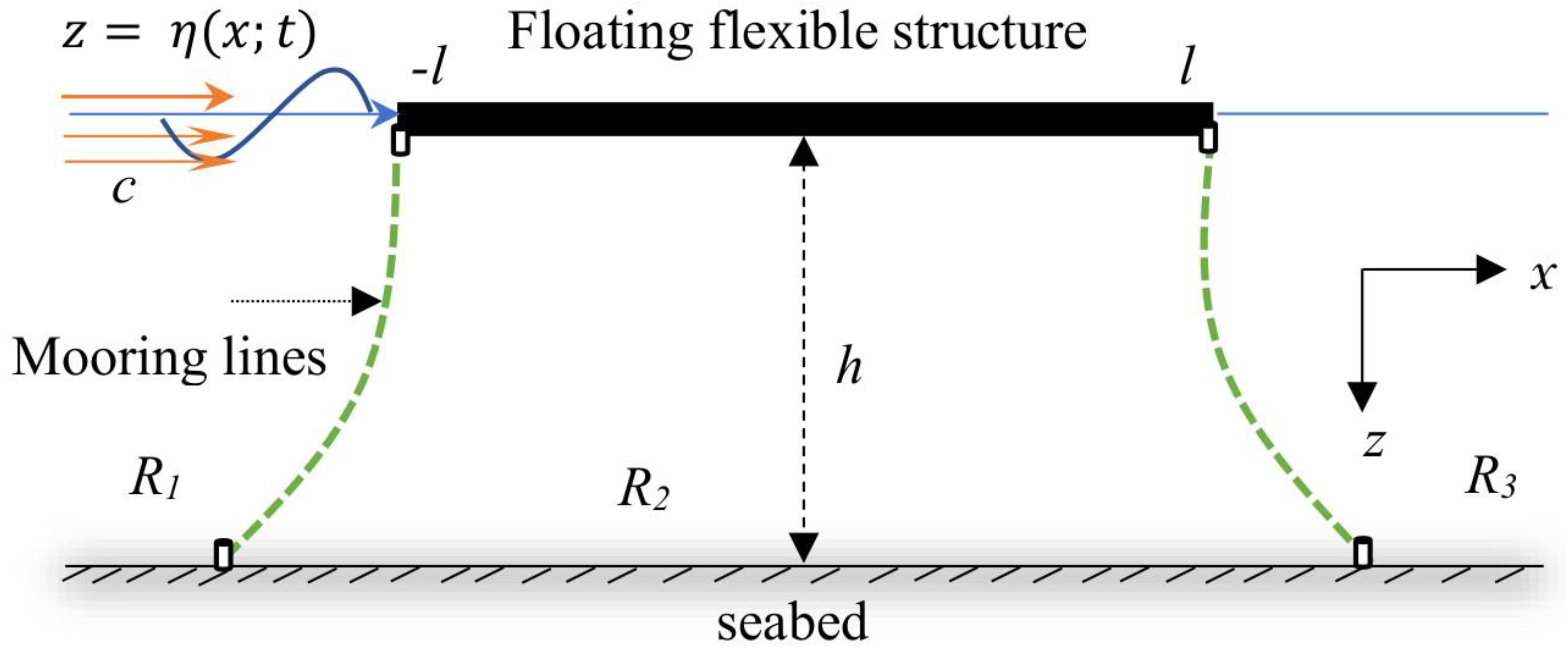
2. Model Definition
3. Method of Solution
Determination of Displacement and Shear Force
4. Numerical Results and Discussion
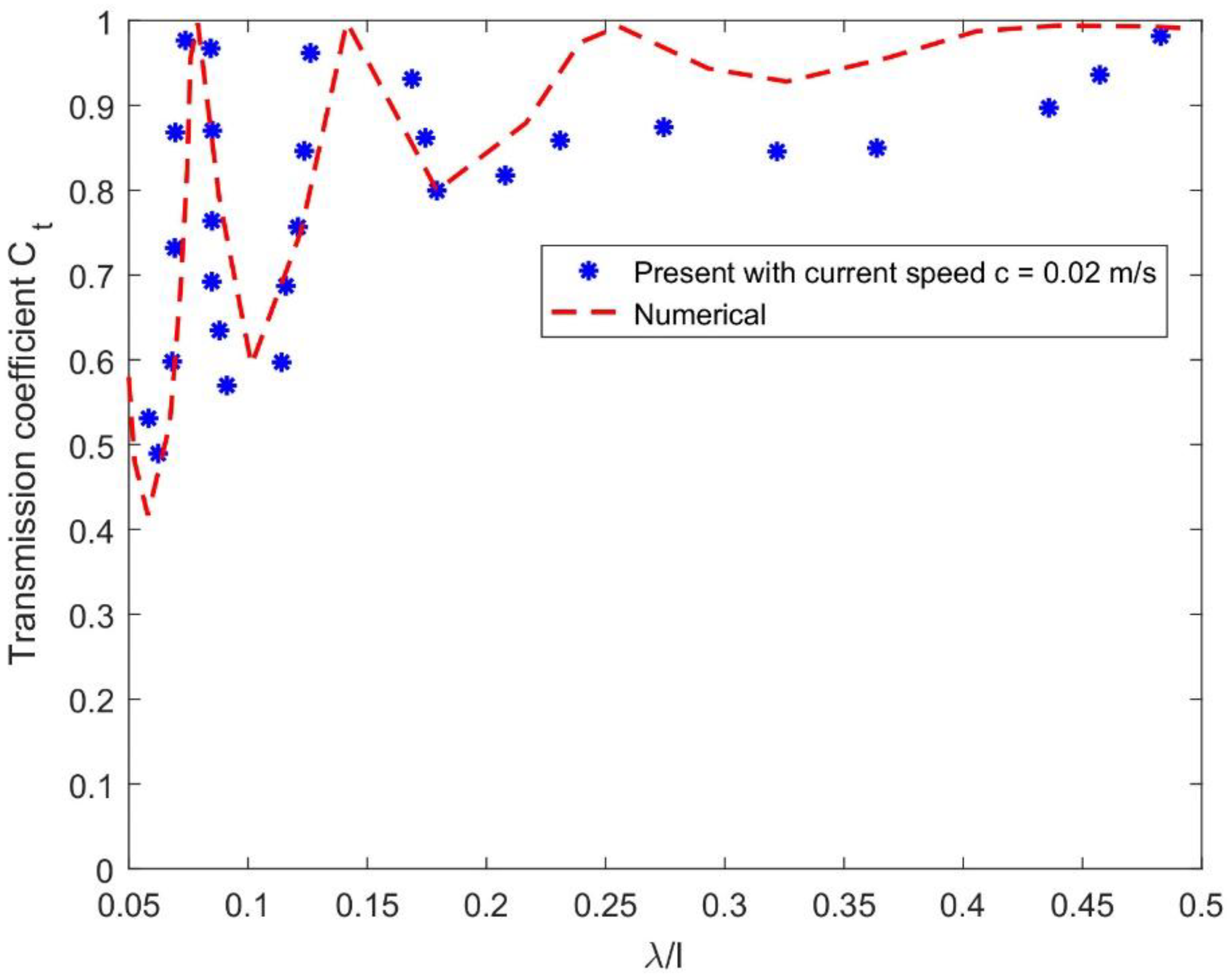
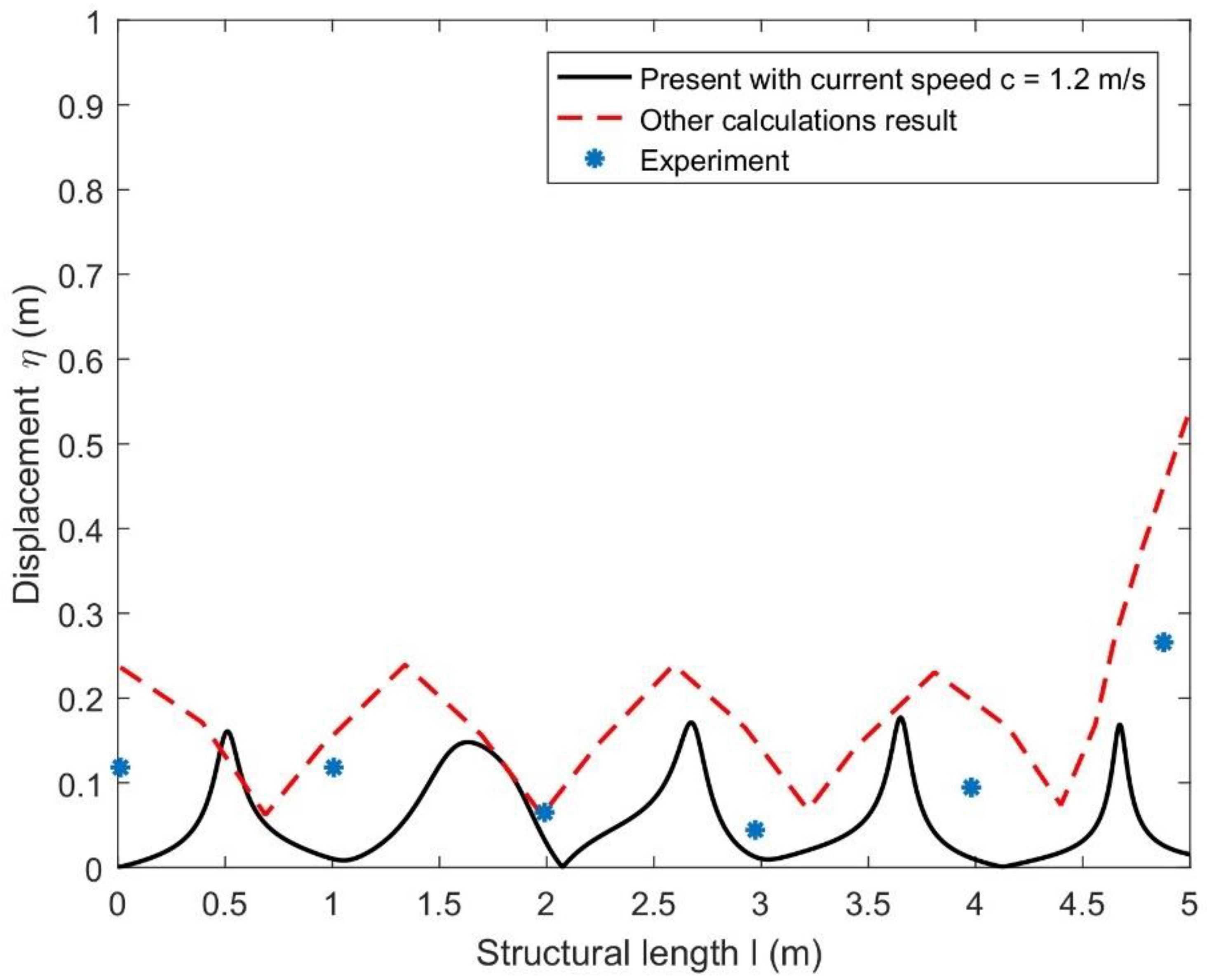
4.1. Comparison Results
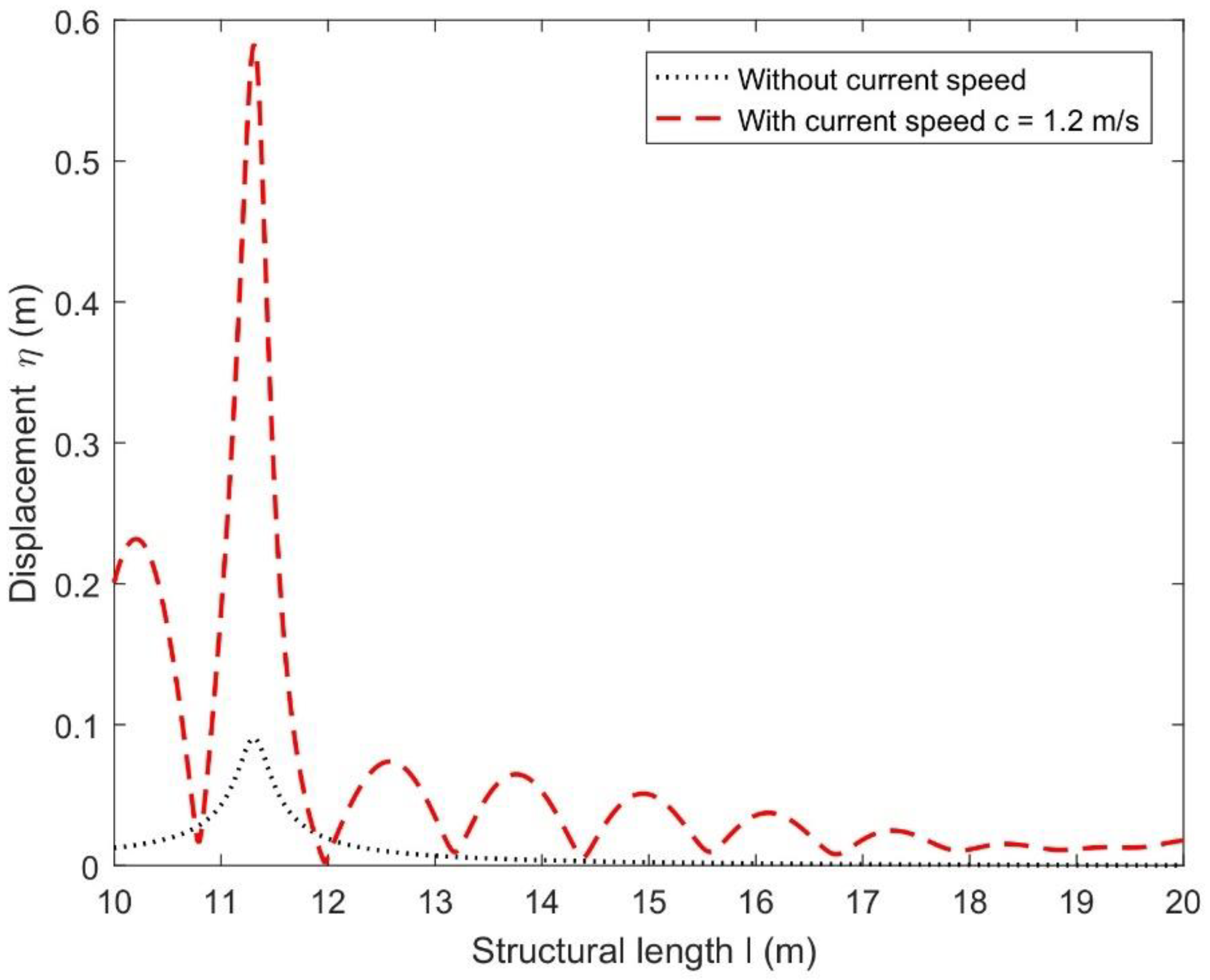
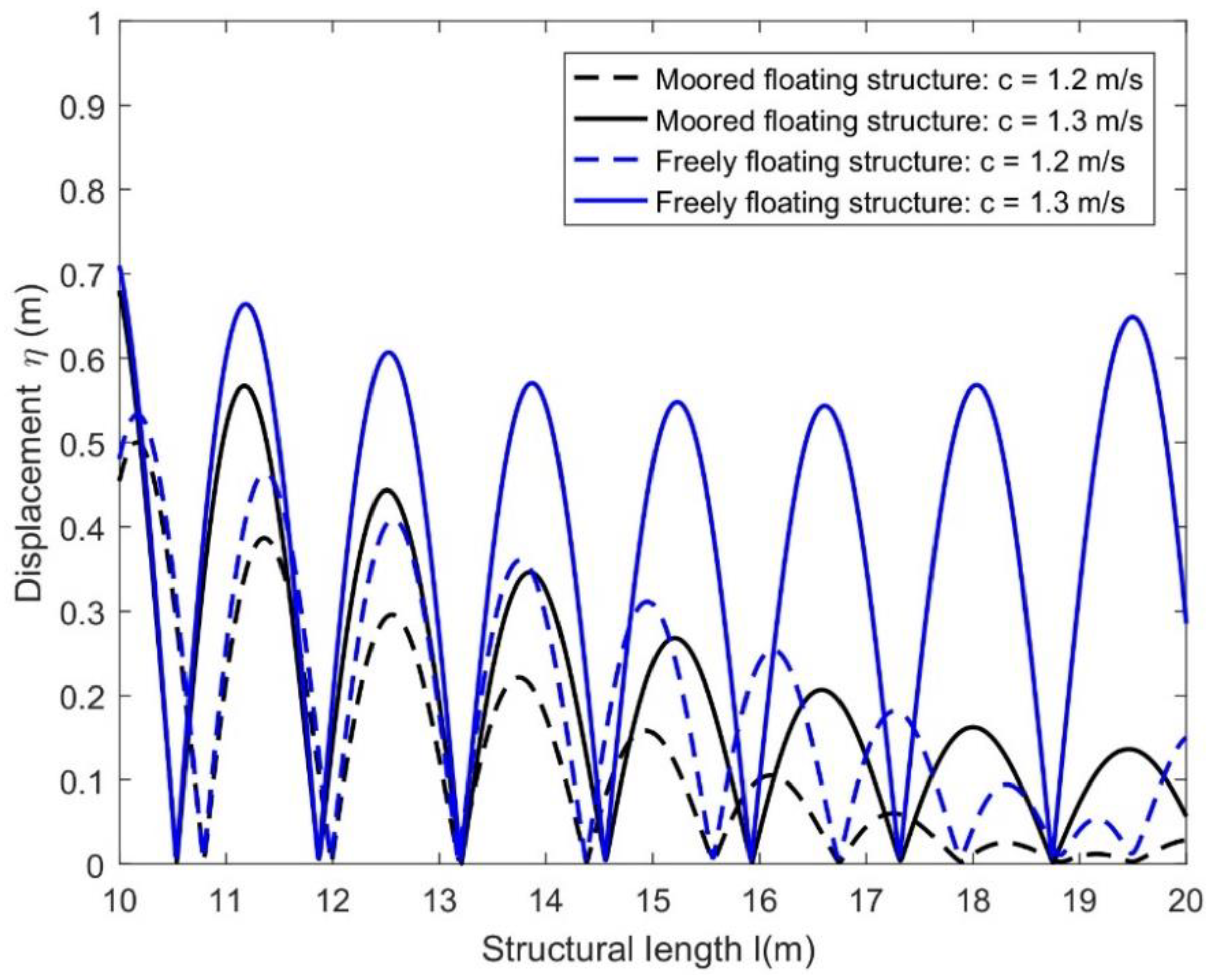
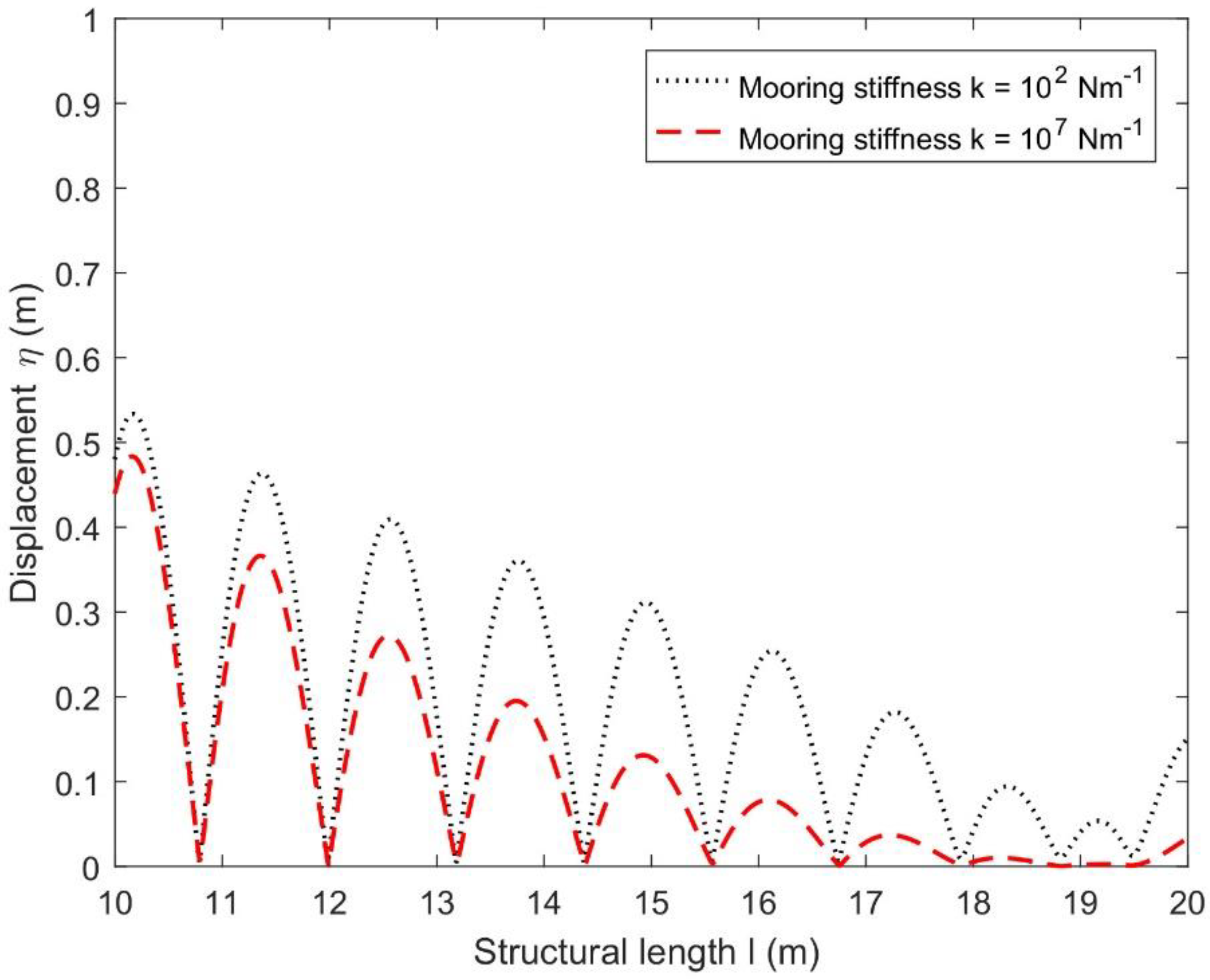
4.2. Hydroelastic Response Analysis via Displacements of Floating Structure
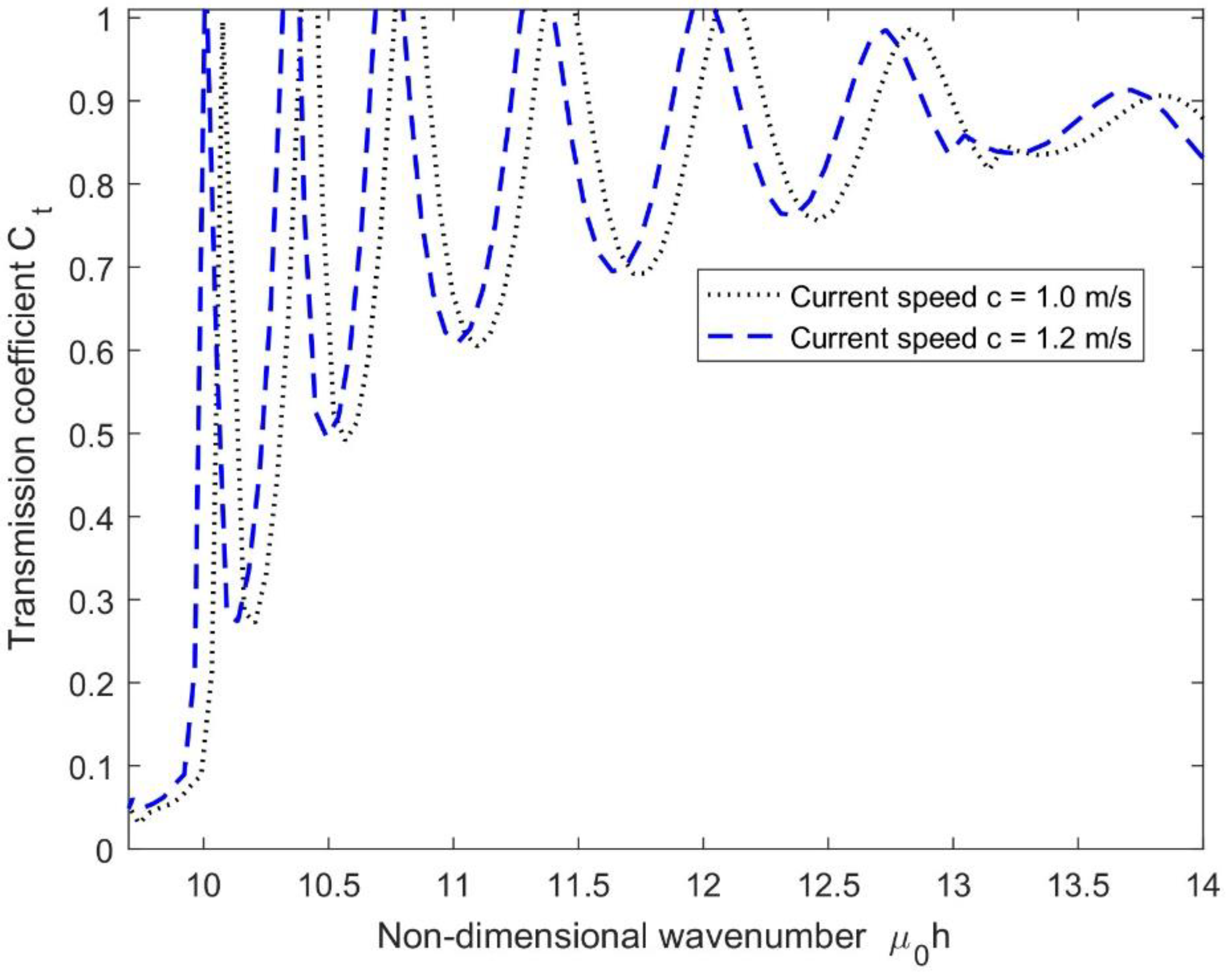
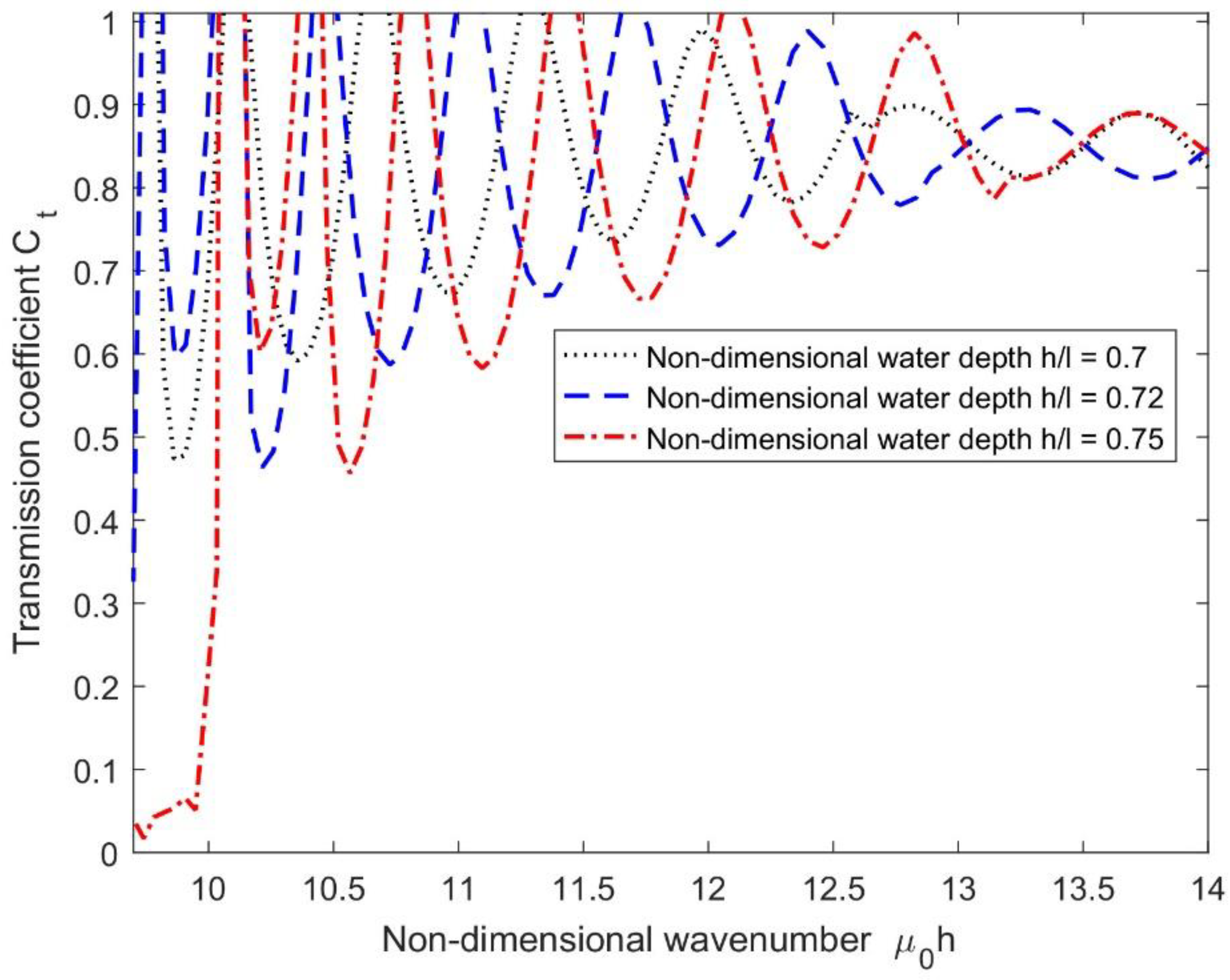
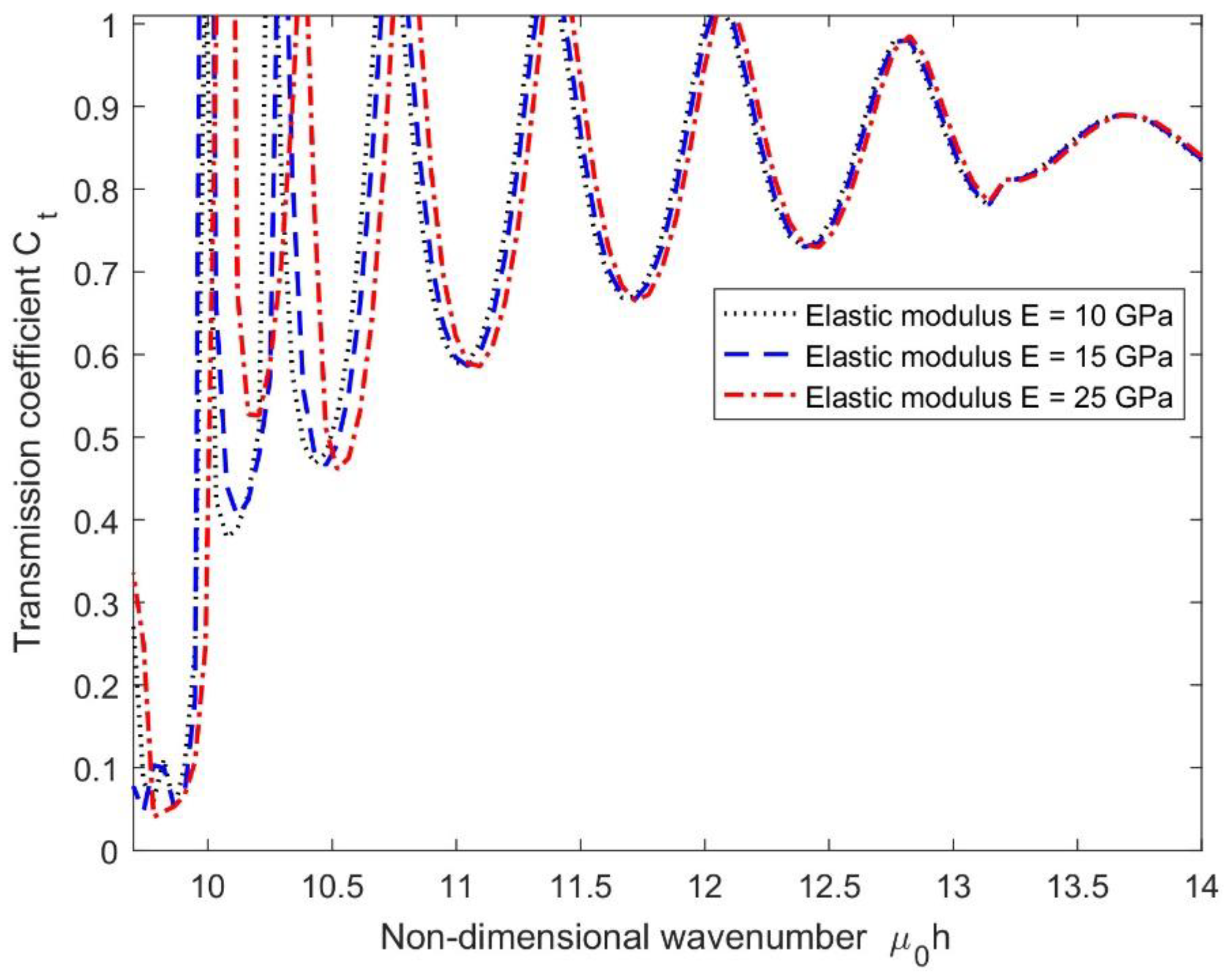
4.3. Effect of Current Speed on Transmission Coefficient
5. Conclusions
- The present result is supported by the existing numerical results published, other calculation results, and experimental datasets available in the literature, and the comparison between the moored and free-edge floating shows that the moored structure provides greater stability than that of the freely floating structure as it deflects more than that of the free one.
- The structural displacements increase for higher values of current speeds, which is due to the higher hydrodynamic loads on the structure in the upstream region.
- As the current speed increases, the transmission coefficients increase because the larger current provides larger hydrodynamic forces, which results in higher displacements that lead to more wave energy passing below the structure. Furthermore, the number of resonating patterns decreases as the wavenumber increases, which is expected as the wave reflection decreases in the upstream region.
- The analysis of the structural displacements and transmission coefficients for different current speeds suggested that the floating flexible structure became more stable for lower values of current speed and higher mooring stiffness.
- Therefore, the present study indicated that the mathematical model will be helpful to develop a three-dimensional analytical model by considering the opposite current to analyse the hydroelastic response and sensitivity analysis of floating flexible structures based on the Timoshenko–Mindlin beam theory.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| BIEM | Boundary Integral Equation Method |
| CFD | Computational Fluid Dynamics |
| FAST | Fourier Amplitude Sensitivity Test |
| FEM-BEM | Finite Element Method-Boundary Element Method |
| FOWT | Floating Offshore Wind Turbine |
| HDMR | High-Dimensional Model Representation |
| MEFEM | Matched Eigenfunction Expansion Method |
| VLFS | Very Large Floating Structure |
| Ct | Transmission Coefficient |
| Cr | Reflection Coefficient |
| 2D | Two-Dimensions |
| 3D | Three-Dimensions |
Appendix A. Equation System for Determining the Unknowns
References
- Thomas, G.; Klopman, G. Wave-current interactions in the near shore region. Int. Ser. Adv. Fluid Mech. 1997, 10, 255. [Google Scholar]
- Miao, Y.J.; Chen, X.J.; Ye, Y.L.; Ding, J.; Huang, H. Numerical modeling and dynamic analysis of a floating bridge subjected to wave, current and moving loads. Ocean Eng. 2021, 225, 108810. [Google Scholar] [CrossRef]
- Huang, J.; Teng, B.; Cong, P.W. Wave-current interaction with three-dimensional bodies in a channel. Ocean Eng. 2022, 249, 110952. [Google Scholar] [CrossRef]
- Latheef, M.; Abdulla, N.; Faieez, M.; Jupri, M.F.M. Wave current interaction: Effect on force prediction for fixed offshore structures. In Proceedings of the International Conference on Civil, Offshore & Environmental Engineering 2018 (ICCOEE 2018), Kuala Lumpur, Malaysia, 13–14 August 2018; Volume 203, p. 01011. [Google Scholar]
- Rey, V.; Touboul, J. Forces and moment on a horizontal plate due to regular and irregular waves in the presence of current. Appl. Ocean Res. 2011, 33, 88–99. [Google Scholar] [CrossRef]
- Chen, L.; Basu, B. Wave-current interaction effects on structural responses of floating offshore wind turbines. Wind Energy 2019, 22, 327–339. [Google Scholar] [CrossRef]
- Li, Y.; Lin, M. Hydrodynamic coefficients induced by waves and currents for submerged circular cylinder. Procedia Eng. 2010, 4, 253–261. [Google Scholar] [CrossRef] [Green Version]
- Viuff, T.; Xiang, X.; Øiseth, O.; Leira, B.J. Model uncertainty assessment for wave- and current-induced global response of a curved floating pontoon bridge. Appl. Ocean Res. 2020, 105, 102368. [Google Scholar] [CrossRef]
- Lu, D.Q.; Yeung, R.W. Hydroelastic waves generated by point loads in a current. Int. J. Offshore Polar Eng. 2015, 25, 8–12. [Google Scholar]
- Qua, X.; Li, Y.; Tang, Y.; Hu, Z.; Zhang, P.; Yin, T. Dynamic response of spar-type floating offshore wind turbine in freak wave considering the wave-current interaction effect. Appl. Ocean Res. 2020, 100, 102178. [Google Scholar] [CrossRef]
- Zhu, T.; Ke, S.; Li, W.; Chen, J.; Yun, Y.; Ren, H. WRF-CFD/CSD analytical method of hydroelastic responses of ultra-large floating body on maritime airport under typhoon-wave-current coupling effect. Ocean Eng. 2022, 261, 112022. [Google Scholar] [CrossRef]
- Ping, W.; Yongyan, W.; Chuanqi, S.; Yanzhao, Y. Nonlinear Hydroelastic Waves Generated due to a Floating Elastic Plate in a Current. Adv. Math. Phys. 2017, 2017, 2837603. [Google Scholar]
- Bhattacharjee, J.; Sahoo, T. Interaction of current and flexural gravity waves. Ocean Eng. 2007, 34, 1505–1515. [Google Scholar] [CrossRef]
- Bispo, I.B.S.; Mohapatra, S.C.; Guedes Soares, C. Numerical analysis of a moored very large floating structure composed by a set of hinged plates. Ocean Eng. 2022, 253, 110785. [Google Scholar] [CrossRef]
- Lamas-Pardo, M.; Iglesias, G.; Carral, L. A review of Very Large Floating Structures (VLFS) for coastal and offshore uses. Ocean Eng. 2015, 109, 677–690. [Google Scholar] [CrossRef]
- Mindlin, R.D. Influence of rotary inertia and shear on flexural motion of isotropic elastic plates. J. Appl. Mech. (ASME) 1951, 18, 31–38. [Google Scholar] [CrossRef]
- Mohapatra, S.C.; Guedes Soares, C. Interaction of ocean waves with floating and submerged horizontal flexible structures in three-dimensions. Appl. Ocean Res. 2019, 83, 136–154. [Google Scholar] [CrossRef]
- Mohapatra, S.C.; Guedes Soares, C. Hydroelastic behaviour of a submerged horizontal flexible porous structure in three-dimensions. J. Fluids Struct. 2021, 104, 103319. [Google Scholar] [CrossRef]
- Mohapatra, S.C.; Guedes Soares, C. Effect of mooring lines on the hydroelastic response of a floating flexible plate using the BIEM approach. J. Mar. Sci. Eng. 2021, 9, 941. [Google Scholar] [CrossRef]
- Mohapatra, S.C.; Guedes Soares, C. Surface gravity wave interaction with a horizontal flexible floating plate and submerged flexible porous plate. Ocean Eng. 2021, 237, 109621. [Google Scholar] [CrossRef]
- Mohapatra, S.C.; Guedes Soares, C. 3D hydroelastic modelling of fluid-structure interactions of porous flexible structures. J. Fluids Struct. 2022, 112, 103588. [Google Scholar] [CrossRef]
- Papathanasiou, T.K.; Belibassakis, K.A. Hydroelastic analysis of VLFS based on a consistent coupled-mode system and FEM. IES Part A Civ. Struct. Eng. 2014, 7, 195–206. [Google Scholar] [CrossRef]
- Andrianov, A.I.; Hermans, A.J. Hydroelastic analysis of a floating plate of finite draft. Appl. Ocean Res. 2006, 28, 313–325. [Google Scholar] [CrossRef]
- Zilman, G.; Miloh, T. Hydroelastic buoyant circular plate in shallow water: A closed form solution. Appl. Ocean Res. 2000, 22, 191–198. [Google Scholar] [CrossRef]
- Watanabe, E.; Utsunomiya, T.; Wang, C.M. Hydroelastic analysis of pontoon-type VLFS: A literature survey. Eng. Struct. 2004, 26, 245–256. [Google Scholar] [CrossRef]
- Cheng, Y.; Ji, C.; Zhai, G.; Gaidai, O. Hydroelastic analysis of oblique irregular waves with a pontoon-type VLFS edged with dual inclined perforated plates. Mar. Struct. 2016, 49, 31–57. [Google Scholar] [CrossRef]
- Yoon, J.S.; Cho, S.P.; Jiwinangun, R.G.; Lee, P.S. Hydroelastic analysis of floating plates with multiple hinge connections in regular waves. Mar. Struct. 2014, 36, 65–87. [Google Scholar] [CrossRef]
- Andrianov, A.I.; Hermans, A.J. The influence of water depth on the hydroelastic response of a very large floating platform. Mar. Struct. 2003, 16, 355–371. [Google Scholar] [CrossRef]
- Karperaki, A.E.; Belibassakis, K.A. Hydroelastic analysis of Very Large Floating Structures in variable bathymetry regions by multi-modal expansions and FEM. J. Fluids Struct. 2021, 102, 103236. [Google Scholar] [CrossRef]
- Nguyen, X.V.; Luong, V.; Cao, T.N.T.; Lieu, X.Q.; Nguyen, T.B. Hydroelastic responses of floating composite plates under moving loads using a hybrid moving element-boundary element method. Adv. Struct. Eng. 2020, 23, 2759–2775. [Google Scholar] [CrossRef]
- Lamei, A.; Hayatdavoodi, M.; Wong, C.; Tang, B. On Motion and Hydroelastic analysis of a Floating Offshore Wind Turbine. In Proceedings of the ASME 2019 38th International Conference on Ocean, Offshore and Arctic Engineering, Glasgow, UK, 9–14 June 2019. Paper No. OMAE2019-96034, V010T09A070. [Google Scholar]
- Amaechi, C.V.; Reda, A.; Butler, H.O.; Ahmed Ja’e, I.; An, C. Review on Fixed and Floating Offshore Structures. Part II: Sustainable Design Approaches and Project Management. J. Mar. Sci. Eng. 2022, 10, 973. [Google Scholar] [CrossRef]
- Shumin, C.; Swamidas, A.S.J.; Sharp, J.J. Similarity method for modelling hydroelastic offshore platforms. Ocean Eng. 1996, 23, 575–595. [Google Scholar] [CrossRef]
- Fu, S.; Moan, T.; Chen, X.; Cui, W. Hydroelastic analysis of flexible floating interconnected structures. Ocean Eng. 2007, 34, 1516–1531. [Google Scholar] [CrossRef]
- Ruzzo, C.; Failla, G.; Arena, F.; Collu, M.; Li, L.; Mariotti, C. Analysis of The Coupled Dynamics of an Offshore Floating Multi-Purpose Platform, Part B: Hydro-Elastic Analysis with Flexible Support Platform. In Proceedings of the ASME 2019 38th International Conference on Ocean, Offshore and Arctic Engineering, OMAE2019, Glasgow, Scotland, 9–14 June 2019. [Google Scholar]
- Kang, H.; Kim, M. Hydroelastic analysis and statistical assessment of flexible offshore platforms. Int. J. Offshore Polar Eng. 2014, 24, 35–44. [Google Scholar]
- Amouzadrad, P.; Mohapatra, S.C.; Guedes Soares, C. Hydroelastic response of a moored floating flexible offshore structure based on Timoshenko-Mindlin-Beam theory. In Trends in Renewable Energies Offshore, 1st ed.; Soares, G., Ed.; Taylor & Francis Group: London, UK, 2022; pp. 879–887. ISBN 9781003360773. [Google Scholar]
- Tay, Z.Y.; Wang, C.M. Reducing hydroelastic response of very large floating structures by altering their plan shapes. Ocean Syst. Eng. 2012, 2, 69–81. [Google Scholar] [CrossRef] [Green Version]
- Zhao, C.; Hu, C.; Wei, Y.; Zhang, J.; Huang, W. Diffraction of surface waves by floating elastic plates. J. Fluids Struct. 2008, 24, 231–249. [Google Scholar] [CrossRef]
- Loukogeorgaki, E.; Yagci, O.; Sedat Kabdasli, M. 3D Experimental investigation of the structural response and the effectiveness of a moored floating breakwater with flexibly connected modules. Coast. Eng. 2014, 91, 164–180. [Google Scholar] [CrossRef]
- Vengatesan, V.; Varyani, K.S.; Westlake, P.C. Drag and inertia coefficients for horizontally submerged rectangular cylinders in waves and currents. J. Eng. Marit. Environ. Part M 2009, 223, 121–136. [Google Scholar]
- Dai, J.; Abrahamsen, B.C.; Viuff, T.; Leira, B.J. Effect of wave-current interaction on a long fjord-crossing floating pontoon bridge. Eng. Struct. 2022, 266, 114549. [Google Scholar] [CrossRef]
- Liu, Z.; Mohapatra, S.C.; Guedes Soares, C. Finite Element Analysis of the Effect of Currents on the Dynamics of a Moored Flexible Cylindrical Net Cage. J. Mar. Sci. Eng. 2021, 9, 43. [Google Scholar]
- Wu, C.; Watanabe, E.; Utsunomiya, T. An eigenfunction expansion-matching method for analyzing the wave-induced responses of an elastic floating plate. Appl. Ocean Res. 1995, 17, 301–310. [Google Scholar] [CrossRef]
- Utsunomiya, T.; Watanabe, E.; Wu, C.; Hayashi, N.; Nakai, K.; Sekita, K. Wave Response Analysis of a Flexible Floating Structure By BE-FE Combination Method. In Proceedings of the Fifth International Offshore and Polar Engineering Conference, The Hague, The Netherlands, 11 June 1995. [Google Scholar]



| Model Parameters | Ranges of Values | Units |
|---|---|---|
| Non-dimensional water depth (h/l) | 0.5 | [-] |
| Non-dimensional wavenumber () | 0–14 | [-] |
| Non-dimensional thickness () | 0.03 | [-] |
| Current speed () | 0.02–1.3 | [m/s] |
| Mooring stiffness () | 0.25 | [N/m] |
| Water density () | 1025 | [kgm−3] |
| Gravitational constant () | 9.8 | [m/s] |
| Elastic modulus () | 10–50 | [GPa] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amouzadrad, P.; Mohapatra, S.C.; Guedes Soares, C. Hydroelastic Response to the Effect of Current Loads on Floating Flexible Offshore Platform. J. Mar. Sci. Eng. 2023, 11, 437. https://doi.org/10.3390/jmse11020437
Amouzadrad P, Mohapatra SC, Guedes Soares C. Hydroelastic Response to the Effect of Current Loads on Floating Flexible Offshore Platform. Journal of Marine Science and Engineering. 2023; 11(2):437. https://doi.org/10.3390/jmse11020437
Chicago/Turabian StyleAmouzadrad, Pouria, Sarat Chandra Mohapatra, and Carlos Guedes Soares. 2023. "Hydroelastic Response to the Effect of Current Loads on Floating Flexible Offshore Platform" Journal of Marine Science and Engineering 11, no. 2: 437. https://doi.org/10.3390/jmse11020437
APA StyleAmouzadrad, P., Mohapatra, S. C., & Guedes Soares, C. (2023). Hydroelastic Response to the Effect of Current Loads on Floating Flexible Offshore Platform. Journal of Marine Science and Engineering, 11(2), 437. https://doi.org/10.3390/jmse11020437









