Numerical Study on the Water Entry of a Freely Falling Unmanned Aerial-Underwater Vehicle
Abstract
1. Introduction
2. Materials and Methods
2.1. Governing Equations and Turbulence Model
2.2. Treatment of Free Surface
2.3. Numerical Procedure
3. Test Object and Computational Mesh
3.1. Description of the UAUV
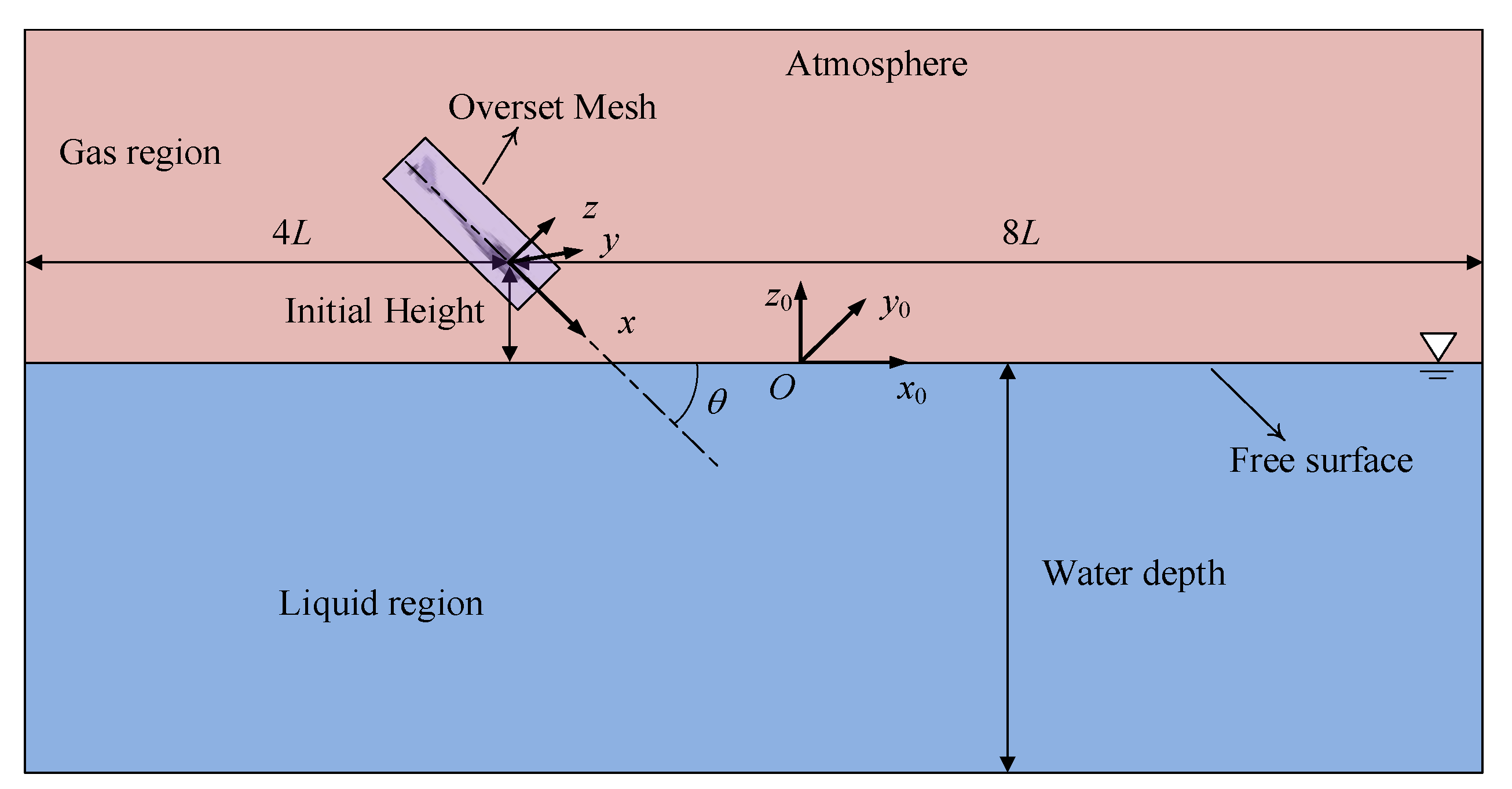
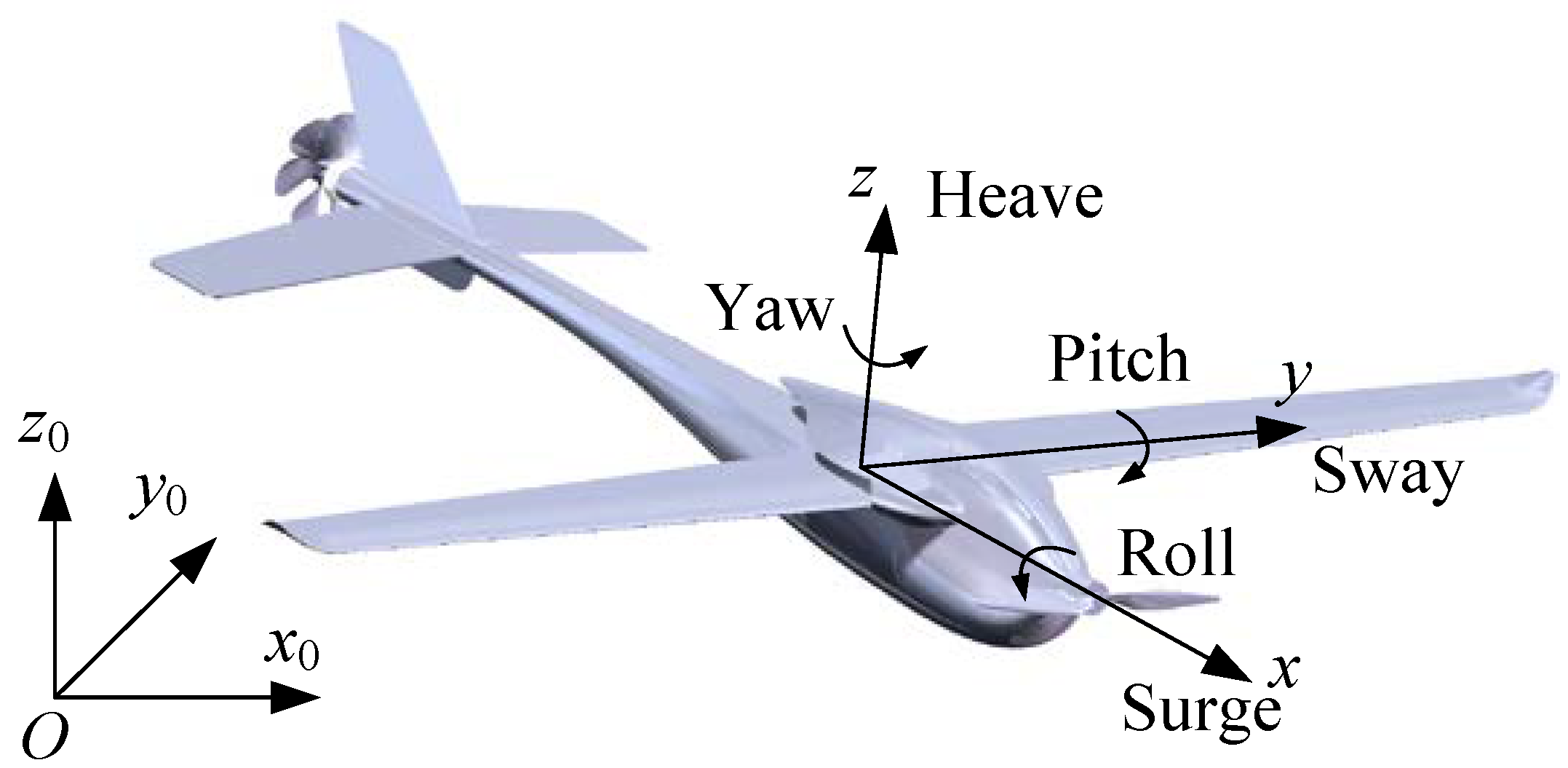
3.2. Coordinate Systems and Dimensionless Quantities
3.3. Computational Domain and Boundary Conditions
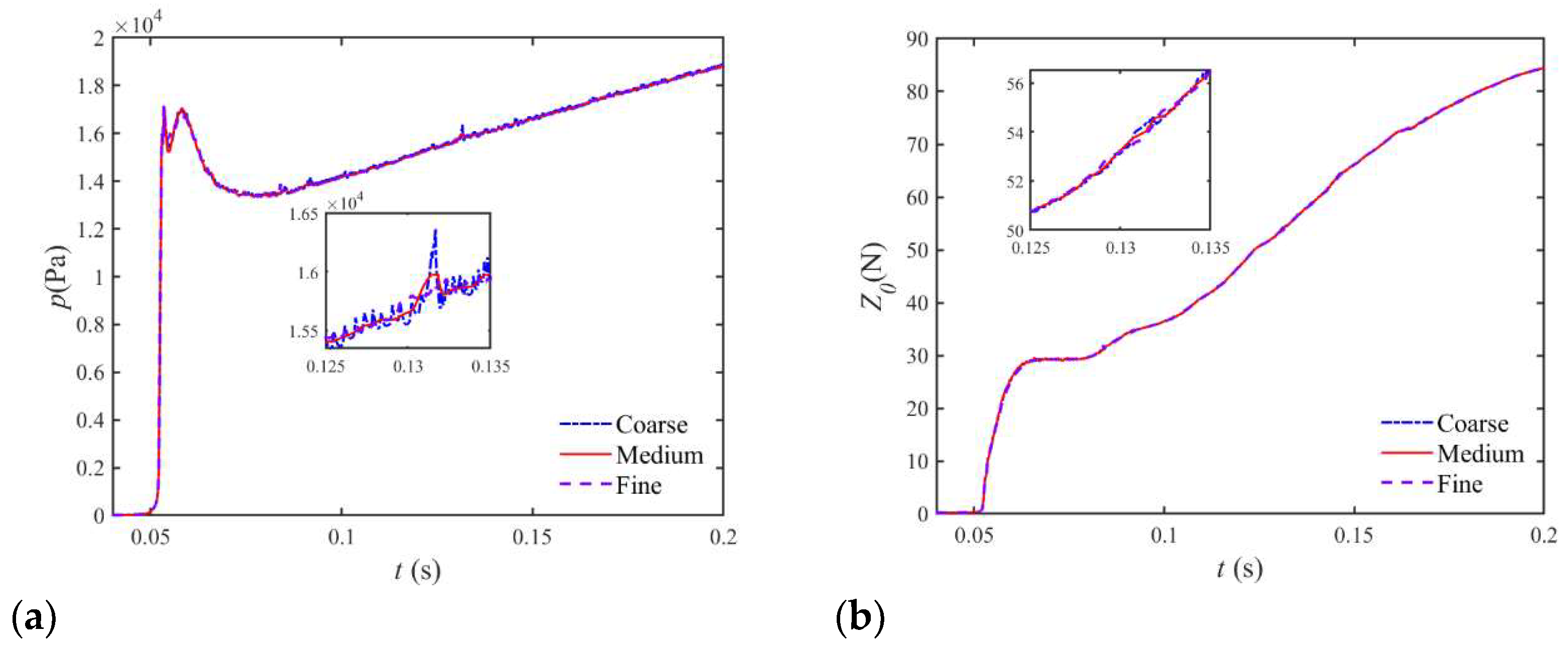
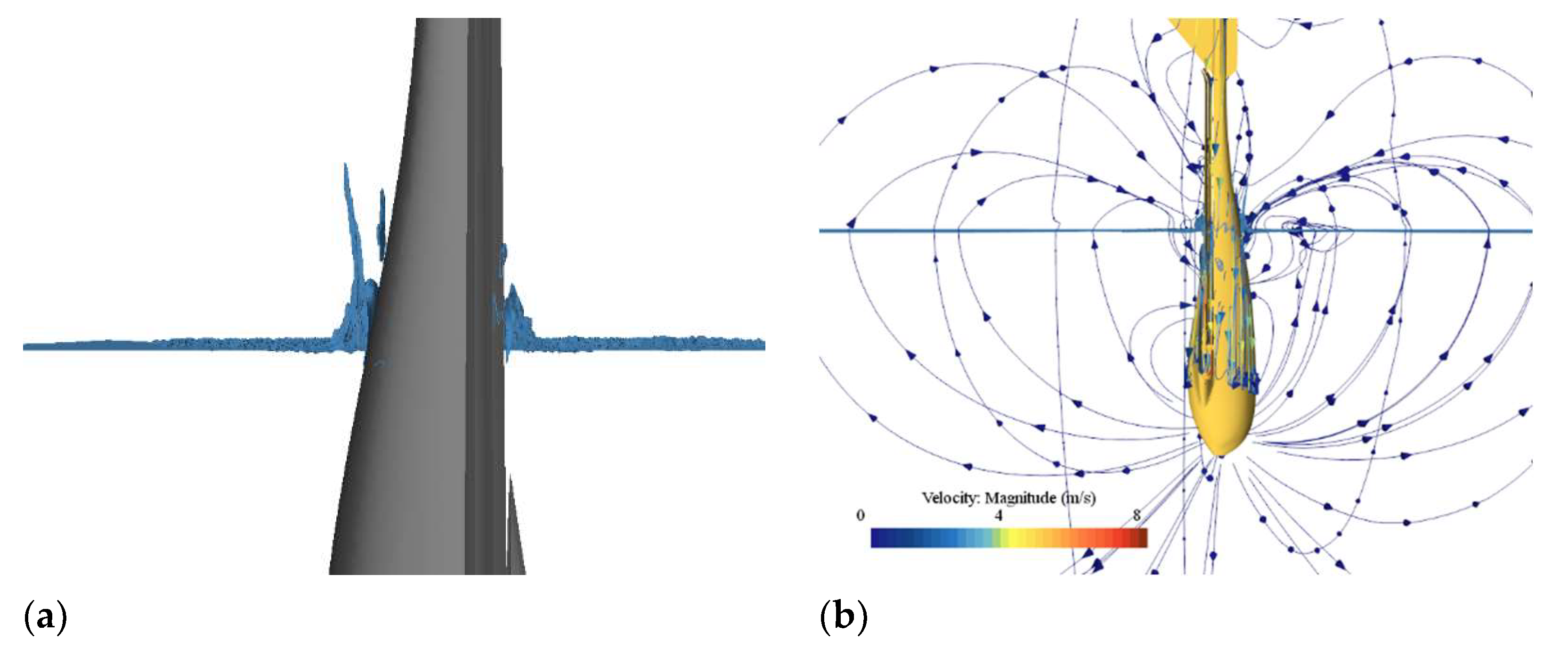
4. Convergence Study
5. Numerical Results and Discussions
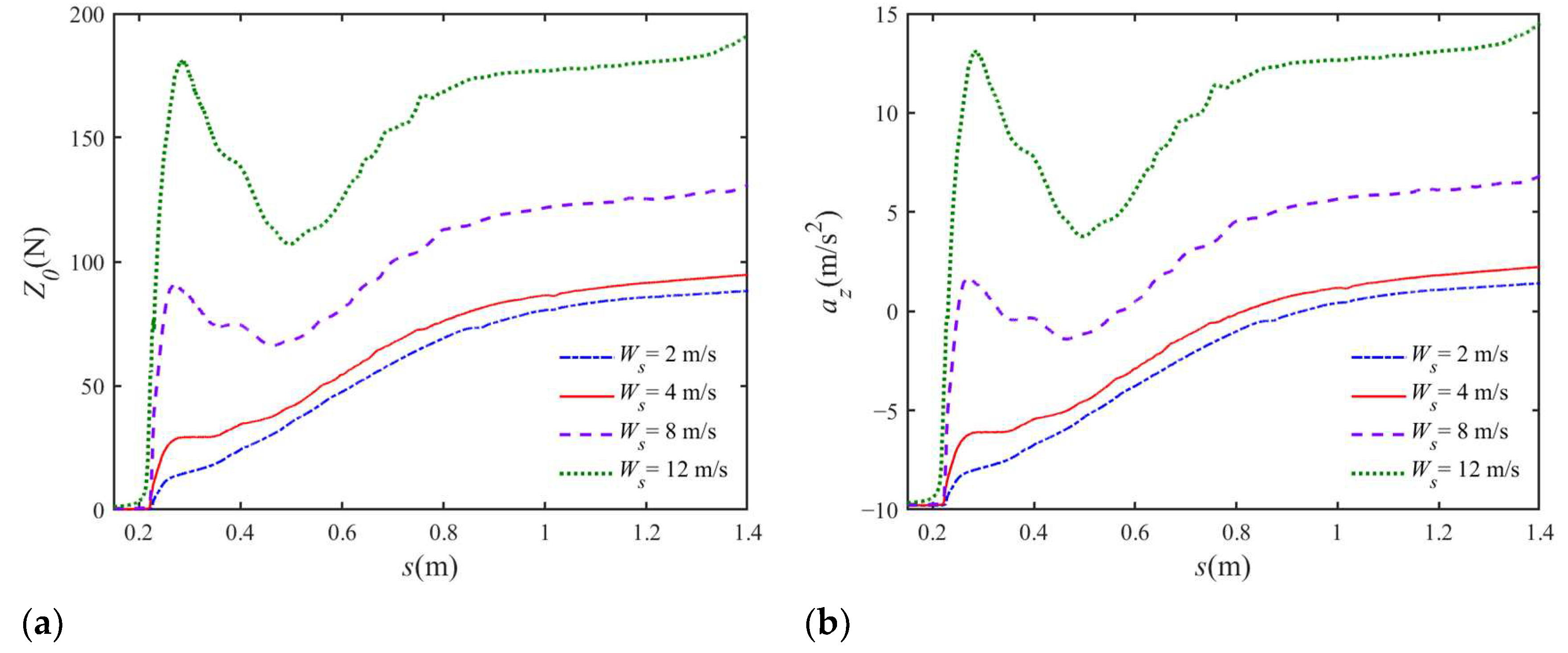
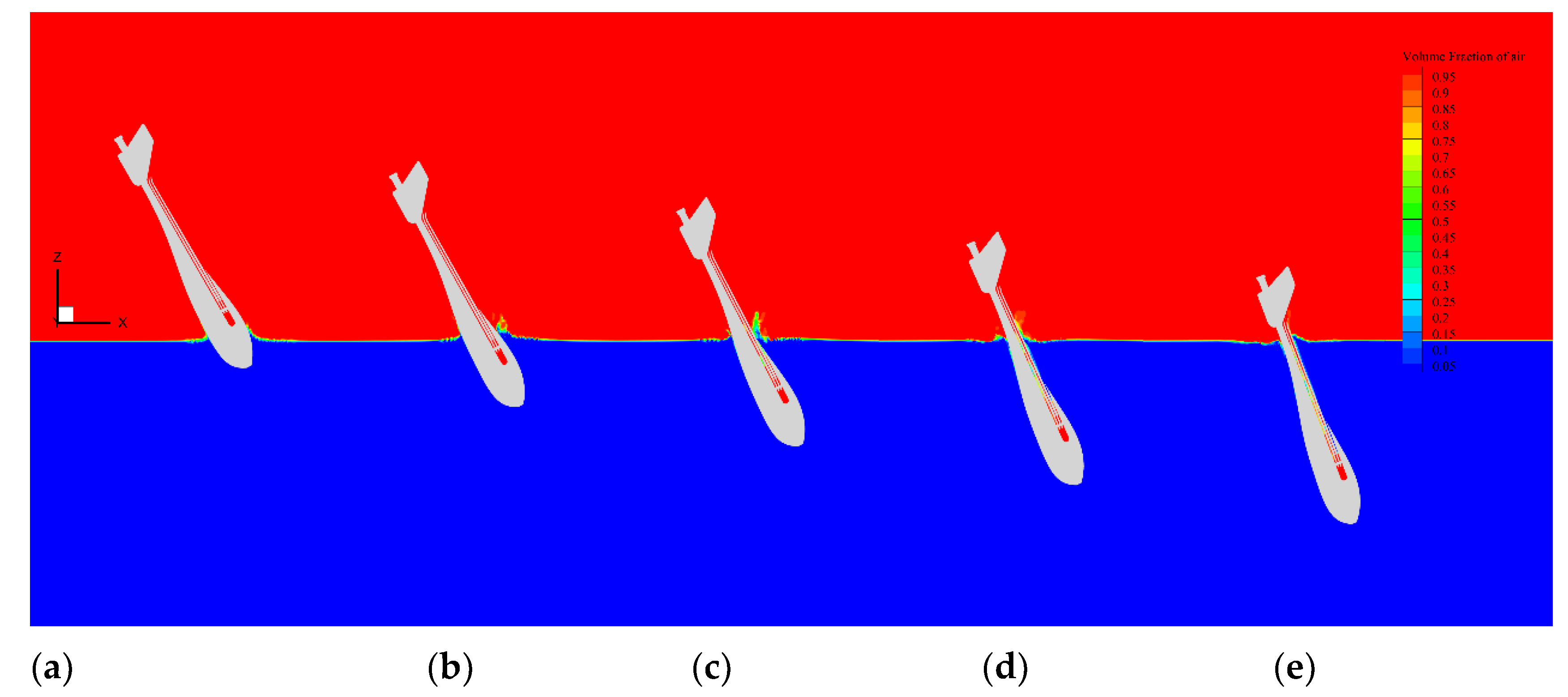
5.1. Effect of Initial Vertical Velocity
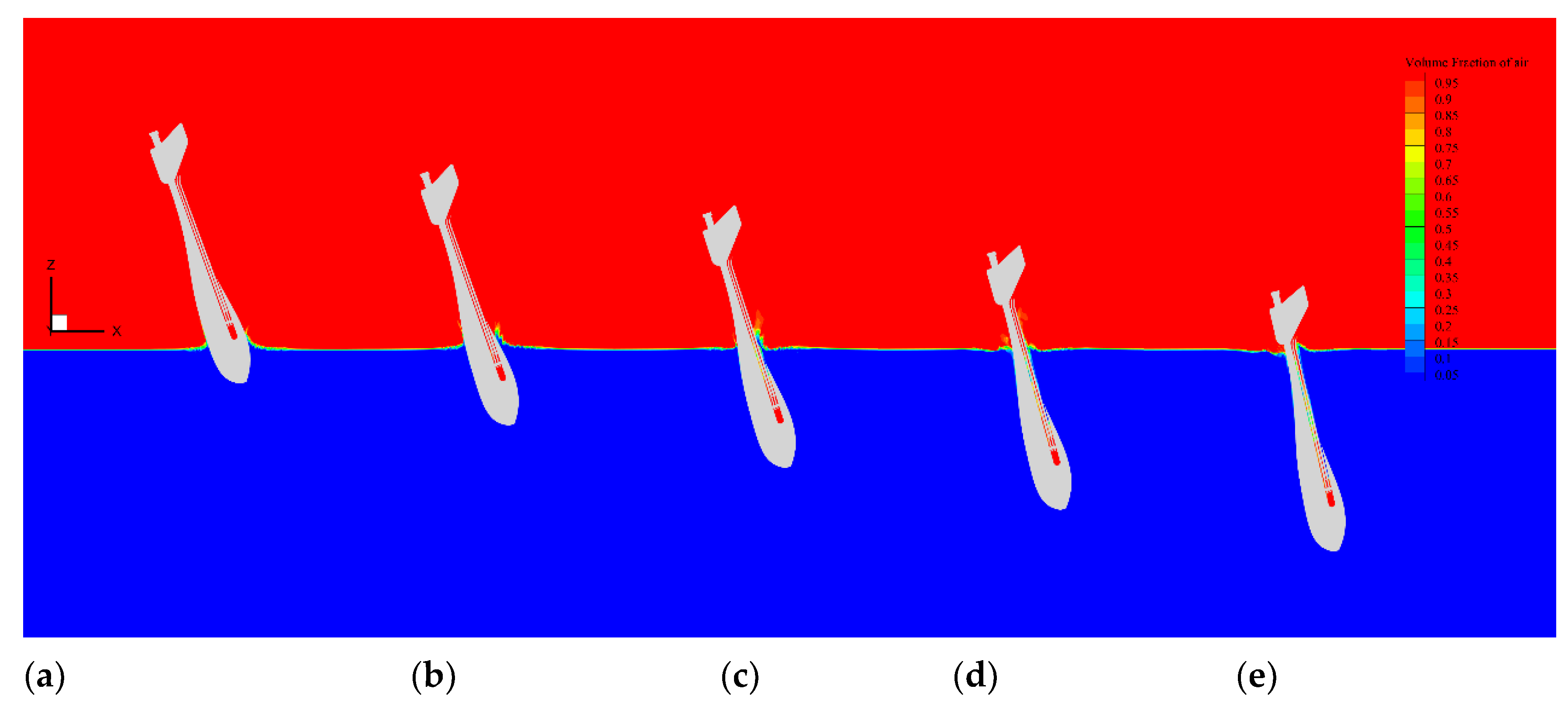
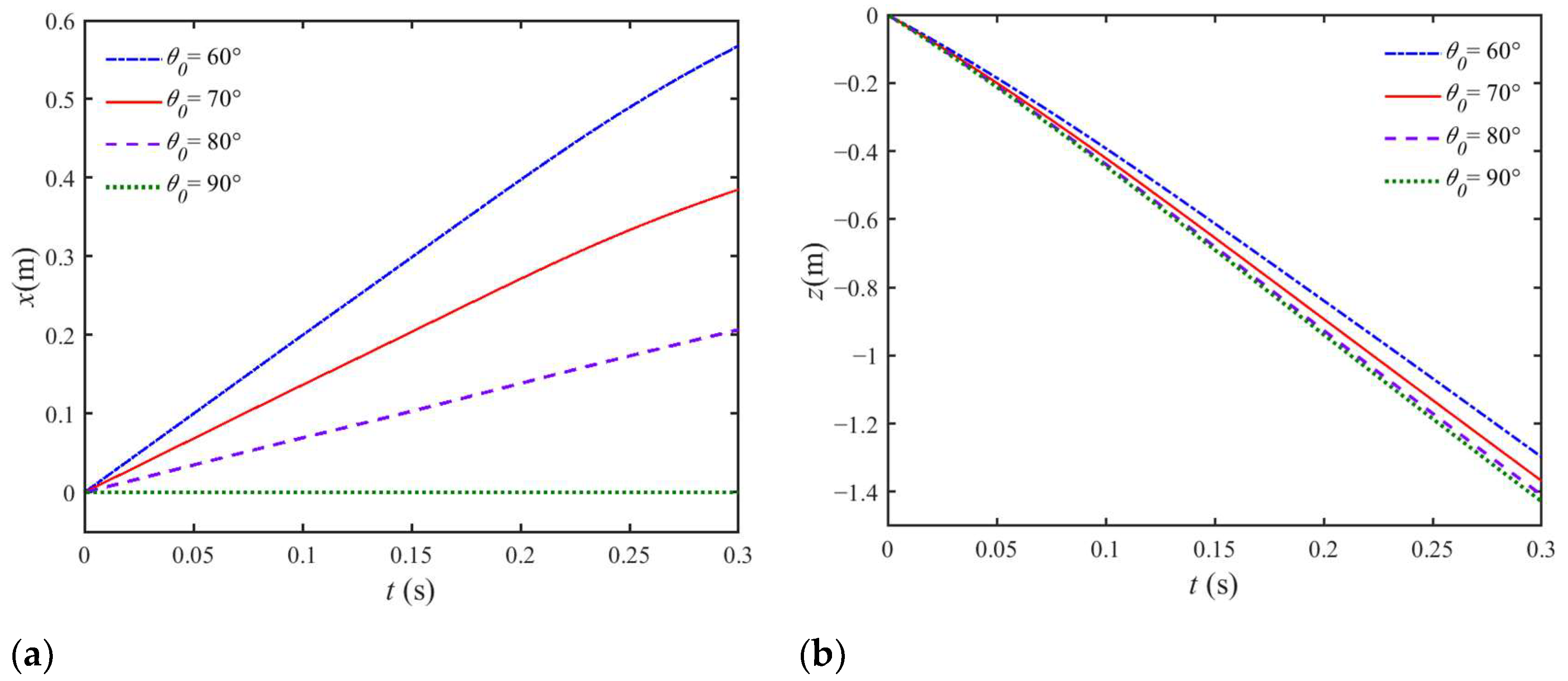
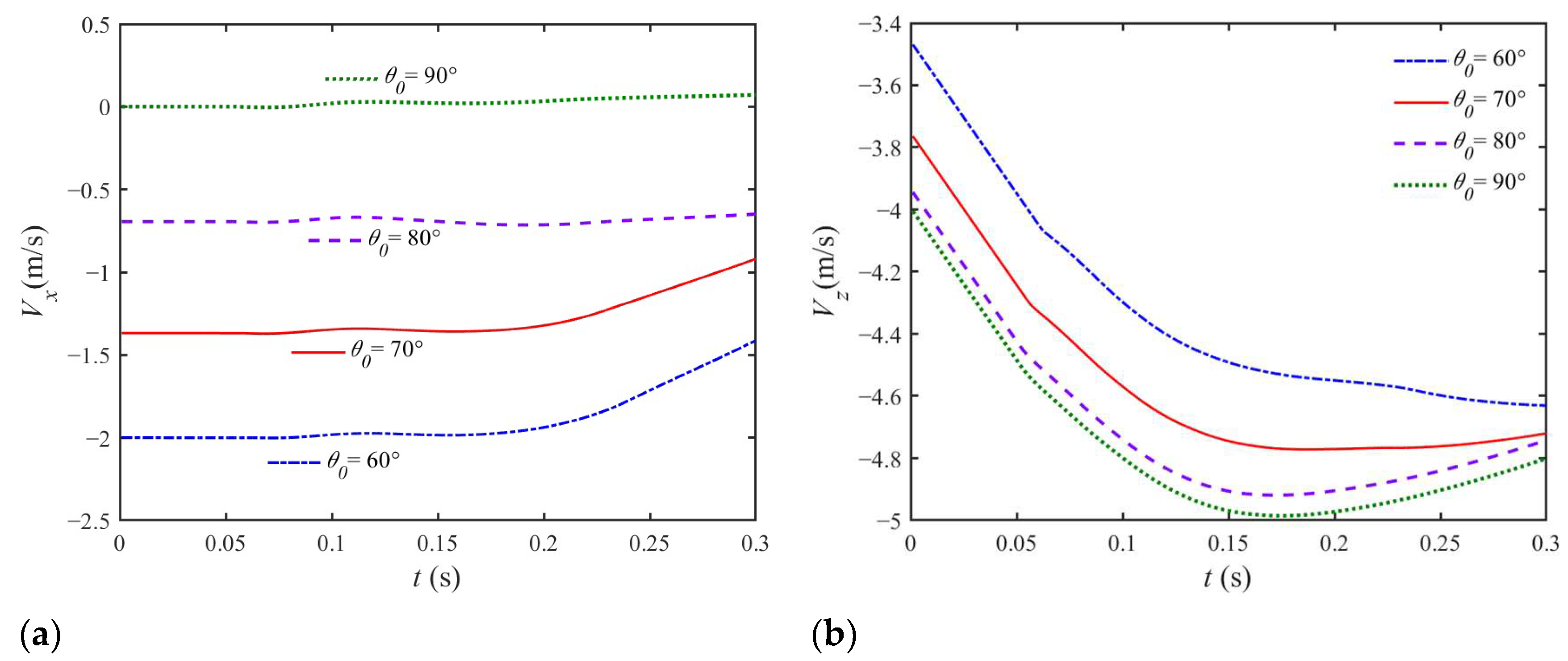
5.2. Effect of Initial Pitch Angle
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nicolaou, S. Flying Boats & Seaplanes: A History from 1905; Bay View Books Ltd.: Bideford, UK, 1996. [Google Scholar]
- Petrov, G. Flying submarine. J. Fleet. 1995, 3, 52–53. [Google Scholar]
- Li, Y.; Feng, J.; Hu, J.; Yang, J. Research on the motion characteristics of a trans-media vehicle when entering water obliquely at low speed. Int. J. Nav. Archit. Ocean Eng. 2018, 10, 188–200. [Google Scholar] [CrossRef]
- Oumeraci, H. Review and analysis of vertical breakwater failures—Lessons learned. Coast. Eng. 1994, 22, 3–29. [Google Scholar] [CrossRef]
- Wei, Z.; Teng, Y.; Meng, X.; Yao, B.; Lian, L. Lifting-principle-based design and implementation of fixed-wing unmanned aerial–underwater vehicle. J. Field Robot. 2022, 39, 694–711. [Google Scholar] [CrossRef]
- Von Karman, T. The Impact on Seaplane Floats during Landing; Tech. Rep. Arch. Image Librar: Washington, DC, USA, 1929; Available online: https://digital.library.unt.edu/ark:/67531/metadc54062/ (accessed on 15 March 2019).
- Wagner, H. Uber Stoss—und Gleitvorgange an der oberflacke flussigkiten. ZAMM 1932, 4, 193–235. [Google Scholar] [CrossRef]
- Dobrovol’skaya, Z.N. On some problems of similarity flow of fluid with a free surface. J. Fluid Mech. 1969, 36, 805–829. [Google Scholar] [CrossRef]
- Toyama, Y. Two-dimensional water impact of unsymmetrical bodies. J. Soc. Nav. Arch. Jpn. 1993, 173, 285–291. [Google Scholar] [CrossRef]
- Mei, X.; Liu, Y.; Yue, D.K. On the water impact of general two-dimensional sections. Appl. Ocean Res. 1999, 21, 1–15. [Google Scholar] [CrossRef]
- Vorus, W.S. A flat cylinder theory for vessel impact and steady planing resistance. J. Ship Res. 1996, 40, 89–106. [Google Scholar] [CrossRef]
- Semenov, Y.A.; Iafrati, A. On the nonlinear water entry problem of asymmetric wedges. J. Fluid Mech. 2006, 547, 231–256. [Google Scholar] [CrossRef]
- Xu, G.; Wu, G.; Duan, Y. Axisymmetric liquid block impact on a solid surface. Appl. Ocean Res. 2011, 33, 366–374. [Google Scholar] [CrossRef]
- Han, B.; Peng, Y.; Li, H.; Liu, S.; Sun, S.; Shan, Y.; Sun, Z. Numerical investigations of a 2D bow wedge asymmetric free-falling into still water. Ocean Eng. 2022, 266, 112905. [Google Scholar] [CrossRef]
- Wang, Y.; Shi, X.; Wang, P. Modeling and simulation of oblique water-entry of disk Ogive. Torpedo Technol. 2008, 16, 14–17. [Google Scholar]
- Afzal, A.; Ansari, Z.; Faizabadi, A.R.; Ramis, M.K. Parallelization Strategies for Computational Fluid Dynamics Software: State of the Art Review. Arch. Comput. Methods Eng. 2017, 24, 337–363. [Google Scholar] [CrossRef]
- Qiu, H.; Yuan, X.; Wang, Y.; Liu, C. Simulation on impact load and cavity shape in high speed vertical water entry for an axisymmetric body. Torpedo Technol. 2013, 21, 161–164. [Google Scholar]
- Guo, B.; Liu, P.; Qu, Q.; Wang, J. Effect of pitch angle on initial stage of a transport airplane ditching. Chin. J. Aeronaut. 2013, 26, 17–26. [Google Scholar] [CrossRef]
- Shi, Y.; Pan, G.; Huang, Q. Water entry impact cushioning performance of mitigator for AUV. In Oceans 2017-Aberdeen; IEEE: Aberdeen, UK, 2017. [Google Scholar]
- Chen, C.; Wang, T.; Feng, Z.; Lu, Y.; Huang, H.; Ji, D.; Chen, Y. Simulation research on water-entry impact force of an autonomous underwater helicopter. J. Mar. Sci. Technol. 2020, 25, 1166–1181. [Google Scholar] [CrossRef]
- Du, Y.; Wang, Z.; Wang, Y.; Wang, J.; Qiu, R.; Huang, C. Study on the cavity dynamics of water entry for horizontal objects with different geometrical shapes. Ocean Eng. 2022, 252, 111242. [Google Scholar] [CrossRef]
- Wu, Y.; Li, L.; Su, X.; Gao, B. Dynamics modeling and trajectory optimization for unmanned aerial-aquatic vehicle diving into the water. Aerosp. Sci. Technol. 2019, 89, 220–229. [Google Scholar] [CrossRef]
- Wu, Y.; Li, L.; Su, X.; Cui, J. Multi-phase trajectory optimization for an aerial-aquatic vehicle considering the influence of navigation error. Eng. Appl. Artif. Intel. 2020, 89, 103404. [Google Scholar] [CrossRef]
- Baldi, S.; Roy, S.; Yang, S. Towards adaptive autopilots for fixed-wing unmanned aerial vehicles. In Proceedings of the 2020 59th IEEE Conference on Decision and Control (CDC), Jeju, Korea, 14–18 December 2020; IEEE: New York, NY, USA, 2020. [Google Scholar]
- Wang, X.; Roy, S.; Farì, S.; Baldi, S. Adaptive Vector Field Guidance Without a Priori Knowledge of Course Dynamics and Wind. IEEE/ASME Trans. Mechatron. 2022, 27, 4597–4607. [Google Scholar] [CrossRef]
- Wang, Z.; Stern, F. Volume-of-fluid based two-phase flow methods on structured multiblock and overset grids. Int. J. Numer. Methods Fluids 2022, 94, 557–582. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Durbin, P.A.; Reif, B.P. Statistical Theory and Modeling for Turbulent Flows; John Wiley & Sons: Chichester, West Sussex, UK, 2011. [Google Scholar]
- Wilcox, D.C. Turbulence Modeling for CFD; DCW Industries: La Canada, CA, USA, 1998. [Google Scholar]
- Wilcox, D.C. Formulation of the k-w turbulence model revisited. AIAA J. 2008, 46, 2832–2838. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundary. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Nova Science Publishers. Multiphase Flow Research; Nova Science Publishers: New York, NY, USA, 2009. [Google Scholar]
- Zahle, F.; Sørensen, N.N.; Johansen, J. Wind turbine rotor-tower interaction using an incompressible overset grid method. Wind Energy Int. J. Prog. Appl. Wind Power Convers. Technol. 2009, 12, 594–619. [Google Scholar] [CrossRef]
- Siemens Digital Industries Software. STAR-CCM+ User Guide, Version.17.02; Siemens PLM Software: Plano, TX, USA, 2022; pp. 6882–7019. [Google Scholar]
- Song, Z.; Duan, W.; Xu, G.; Zhao, B. Experimental and numerical study of the water entry of projectiles at high oblique entry speed. Ocean Eng. 2020, 211, 107574. [Google Scholar] [CrossRef]
- Qiu, S.; Ren, H.; Li, H. Computational Model for Simulation of Lifeboat Free-Fall during Its Launching from Ship in Rough Seas. J. Mar. Sci. Eng. 2020, 8, 631. [Google Scholar] [CrossRef]
- Birkhoff, G.; Zarantonello, E. Jets, Wakes, and Cavities; Elsevier: New York, NY, USA, 1960; p. 261. [Google Scholar]
- Gurevich, M. The Theory of Jets in an Ideal Fluid; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar]
- Wu, G.; Sun, H.; He, Y. Numerical simulation and experimental study of water entry of a wedge in free fall motion. J. Fluids Struct. 2004, 19, 277–289. [Google Scholar] [CrossRef]
- Xiang, G.; Wang, S.; Soares, C.G. Study on the motion of a freely falling horizontal cylinder into water using OpenFOAM. Ocean Eng. 2020, 196, 106811.1–106811.13. [Google Scholar] [CrossRef]

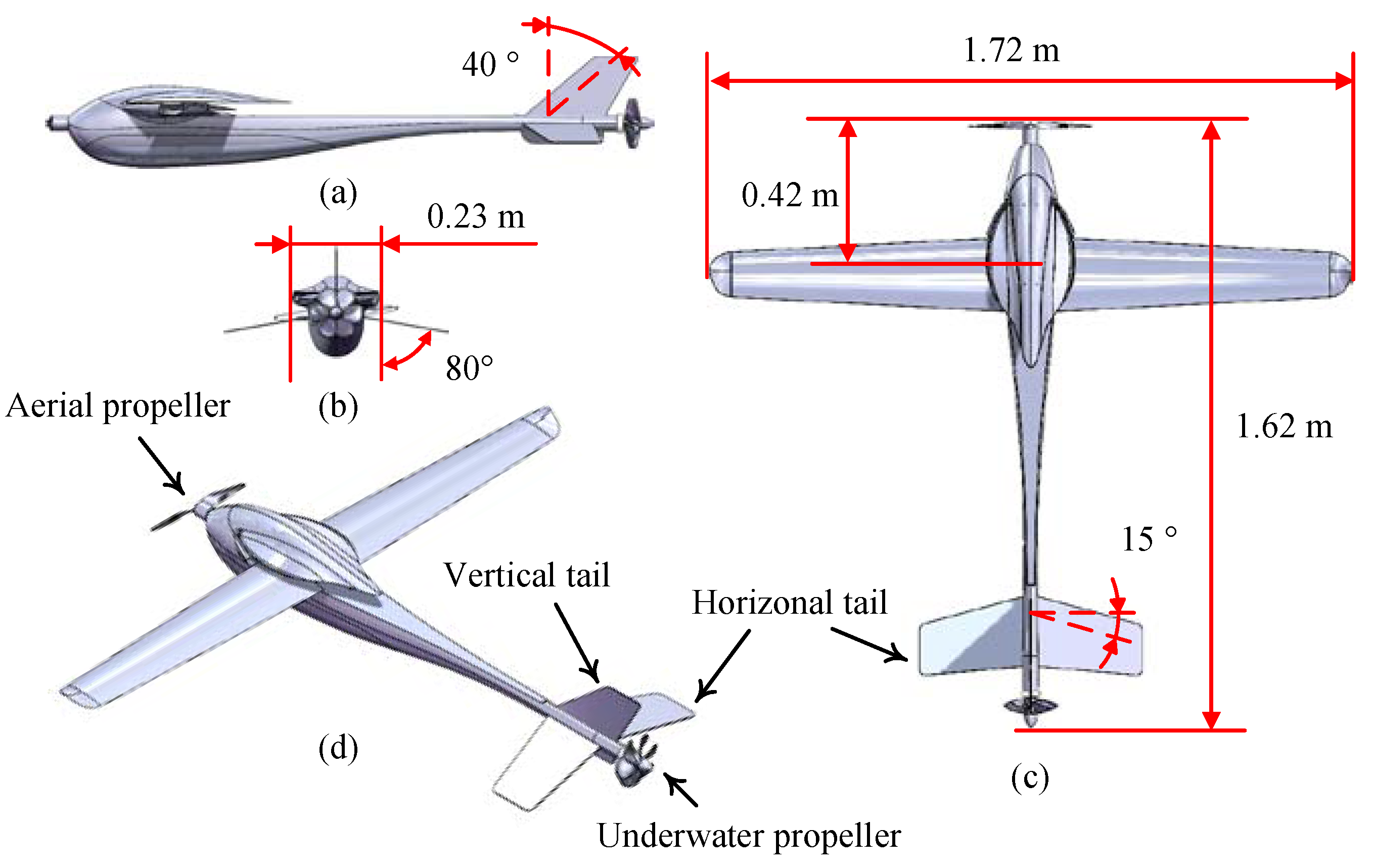










| Main Parameters | Symbol | Unit | Quantitative Values |
|---|---|---|---|
| Length of the vehicle | L | m | 1.62 |
| Span of the wing (UAV moed) | b | m | 1.72 |
| Width of the vehicle (AUV mode) | B | m | 0.23 |
| Weight of the vehicle | W | kg | 7.86 kg |
| Pitch angle of the vehicle | θ | ° | variable |
| Mesh | Basic Mesh Size dl (mm) | Time Interval Δt (s) | Initial Mesh Number NT |
|---|---|---|---|
| Coarse | 31.75 | 0.002 | 1225 k |
| Medium | 25.19 | 0.001 | 2451 k |
| Fine | 20 | 0.0005 | 4902 k |
| Initial Velocity | W0 [m/s] | W1 [m/s] | W2 [m/s] | ∆W0% | ∆W1% | ∆W2% |
|---|---|---|---|---|---|---|
| Ws = 2.0 m/s | 2.885 | 3.766 | 3.667 | 44.24 | 88.28% | 83.37% |
| Ws = 4.0 m/s | 4.486 | 4.985 | 4.814 | 12.16 | 24.63% | 20.35% |
| Ws = 8.0 m/s | 8.248 | 8.219 | 7.784 | 3.11 | 2.73% | −2.70% |
| Ws = 12.0 m/s | 12.148 | 11.793 | 11.117 | 1.24 | −1.73% | −7.36% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dong, L.; Wei, Z.; Zhou, H.; Yao, B.; Lian, L. Numerical Study on the Water Entry of a Freely Falling Unmanned Aerial-Underwater Vehicle. J. Mar. Sci. Eng. 2023, 11, 552. https://doi.org/10.3390/jmse11030552
Dong L, Wei Z, Zhou H, Yao B, Lian L. Numerical Study on the Water Entry of a Freely Falling Unmanned Aerial-Underwater Vehicle. Journal of Marine Science and Engineering. 2023; 11(3):552. https://doi.org/10.3390/jmse11030552
Chicago/Turabian StyleDong, Liyang, Zhaoyu Wei, Hangyu Zhou, Baoheng Yao, and Lian Lian. 2023. "Numerical Study on the Water Entry of a Freely Falling Unmanned Aerial-Underwater Vehicle" Journal of Marine Science and Engineering 11, no. 3: 552. https://doi.org/10.3390/jmse11030552
APA StyleDong, L., Wei, Z., Zhou, H., Yao, B., & Lian, L. (2023). Numerical Study on the Water Entry of a Freely Falling Unmanned Aerial-Underwater Vehicle. Journal of Marine Science and Engineering, 11(3), 552. https://doi.org/10.3390/jmse11030552








