Influence of Different Static Equilibrium Calculation Methods on the Dynamic Response of Marine Cables during the Releasing Process: Review and a Case Study
Abstract
:1. Review of Calculation Methods
1.1. Static Equilibrium Calculation of a Marine Cable
1.2. Solution of Marine Cables
2. Theory and Computation Modeling
2.1. Theory
2.2. Computation Modeling
3. Calculation Results
3.1. Fast Static Equilibrium Calculation Method

3.2. Analytical Catenary Calculation Method

3.3. Catenary Calculation Method
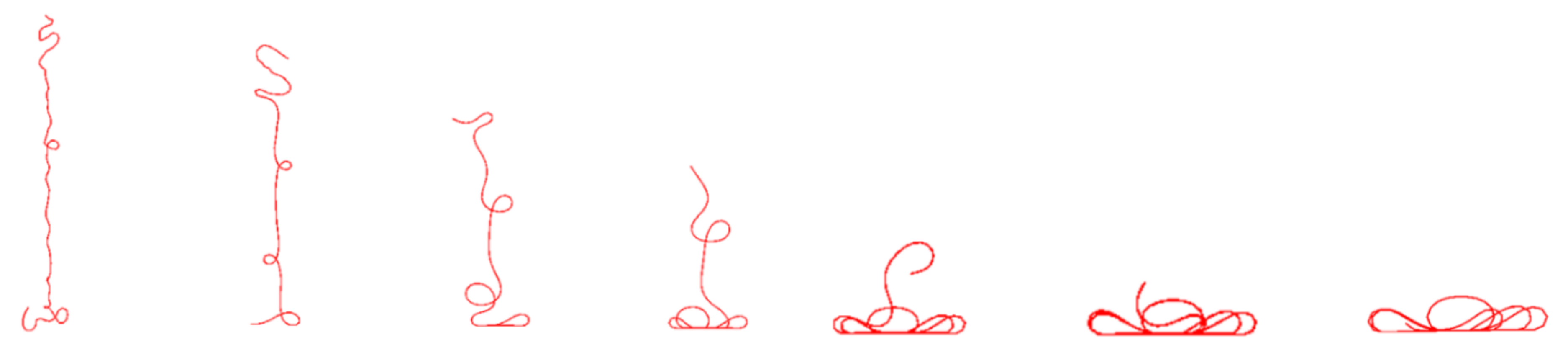
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lemon, S.G. Towed-array history, 1917–2003. IEEE J. Ocean. Eng. 2004, 29, 365–373. [Google Scholar] [CrossRef]
- Luo, Y.; Zhang, D. Dynamic analysis of an axially moving underwater pipe conveying pulsating fluid. Front. Mar. Sci. 2022, 9, 982374. [Google Scholar] [CrossRef]
- Simos, A.N.; Fujarra, A.L.C. Dynamic Tension on Mooring Lines. Comparison of Numerical and Experimental Results. In Proceedings of the Fourteenth International Offshore and Polar Engineering Conference, Toulin, France, 23–28 May 2004. [Google Scholar]
- Berteaux, H.O. Buoy Engineering; John Wiley and Sons: New York, NY, USA, 1976. [Google Scholar]
- Nakajima, T.; Motora, S.; Fujino, M. On the dynamic analysis of multi-component mooring lines. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 3–6 May 1982. [Google Scholar]
- Matha, D.; Schlipf, M.; Pereira, R.; Jonkman, J. Challenges in simulation of aerodynamics, hydrodynamics, and mooring-line dynamics of floating offshore wind turbines. In Proceedings of the Twenty-First International Offshore and Polar Engineering Conference, Maui, HI, USA, 19–24 June 2011. [Google Scholar]
- Matulea, I.C.; Năstase, A.; Tălmaciu, N.; Slămnoiu, G.; Gonçalves-Coelho, A.M. On the equilibrium configuration of mooring and towing cables. Appl. Ocean Res. 2008, 30, 81–91. [Google Scholar] [CrossRef]
- Abkowitz, M.A. Stability and Motion Control of Ocean Vehicles; Card Nr. 70-93041; Massachusetts Institute of Technology, The MIT Press: Cambridge, MA, USA, 1969. [Google Scholar]
- Chucheepsakul, S.; Huang, T. Static equilibrium of marine cables by a variational method. In Proceedings of the First ISOPE Pacific/Asia Offshore Mechanics Symposium, Seoul, Republic of Korea, 24–28 June 1990. [Google Scholar]
- Chen, L.; Basu, B.; Nielsen, S.R.K. Nonlinear periodic response analysis of mooring cables using harmonic balance method. J. Sound Vib. 2019, 438, 402–418. [Google Scholar] [CrossRef]
- Quan, W.; Chang, Q.; Zhang, Q.; Gong, J. Dynamics calculation for variable-length underwater cable with geometrically nonlinear motion. Ocean Eng. 2020, 212, 107695. [Google Scholar] [CrossRef]
- Zhanga, J.; Zhoua, X.L.; Guoa, J.J.; Wanga, J.H. Quasi-static Analysis of Mooring Line Tension Under Combined Impact of Wave, Current and Soil. In Proceedings of the Twenty-fourth International Ocean and Polar Engineering Conference, Busan, Republic of Korea, 15–20 June 2014. [Google Scholar]
- Kumar, P.; Ganguli, A.; Benipal, G.S. Empirical Validation of Free Vibration of Sagging Cables. ASPS Conf. Proc. 2022, 1, 1057–1063. [Google Scholar] [CrossRef]
- Such, M.; Jimenez-Octavio, J.R.; Carnicero, A.; Lopez-Garia, O. An approach based on the catenary equation to deal with static analysis of three dimensional cable structures. Eng. Struct. 2009, 31, 2162–2170. [Google Scholar] [CrossRef]
- Huang, T. A static equilibrium formulation including axial deformation for marine cables. In Proceedings of the Second International Offshore and Polar Engineering Conference, San Francisco, CA, USA, 14–19 June 1992. [Google Scholar]
- Watson, T.U.; Kuneman, J.E. Determination of the static equilibrium configuration of externally redundant submerged cable arrays. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 4–7 May 1975. [Google Scholar]
- Girón, A.R.C.; Corrêa, F.N.; Hernández, A.O.V.; Jacob, B.P. An integrated methodology for the design of mooring systems and risers. Mar. Struct. 2014, 39, 395–423. [Google Scholar] [CrossRef]
- Ja’e, I.A.; Ali, M.O.A.; Yenduri, A.; Nizamani, Z.; Nakayama, A. Optimisation of mooring line parameters for offshore floating structures: A review paper. Ocean Eng. 2022, 247, 110644. [Google Scholar]
- Nikan, O.; Golbabai, A.; Machado, J.T.; Nikazad, T. Numerical approximation of the time fractional cable model arising in neuronal dynamics. Eng. Comput. 2022, 38, 155–173. [Google Scholar] [CrossRef]
- da Silva, D.M.L.; Jacob, B.P.; Rodrigues, M.V. Implicit and explicit implementation of the dynamic relaxation method for the definition of initial equilibrium configurations of flexible lines. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, Hamburg, Germany, 4–9 June 2006. [Google Scholar]
- Neto, A.G.; de Arruda Martins, C. Structural stability of flexible lines in catenary configuration under torsion. Marine Struct. 2013, 4, 16–40. [Google Scholar] [CrossRef]
- Moe, G.; Arntsen, Ø. An analytic model for static analysis of catenary risers. In Proceedings of the Eleventh International Offshore and Polar Engineering Conference, Stavanger, Norway, 17–22 June 2001. [Google Scholar]
- Athisakul, C.; Phanyasahachart, T.; Klaycham, K.; Chucheepsakul, S. Static equilibrium configurations and appropriate applied top tension of extensible marine riser with specified total arc-length using finite element method. Eng. Struct. 2012, 34, 271–277. [Google Scholar] [CrossRef]
- Lepidi, M.; Gattulli, V. Static and dynamic response of elastic suspended cables with thermal effects. Int. J. Solids Struct. 2012, 49, 1103–1116. [Google Scholar] [CrossRef] [Green Version]
- Ma, G.; Sun, L.; Wang, H.; Ai, S. Nonlinear mechanical model for static analysis of stretched mooring line. In Proceedings of the Twenty-third International Offshore and Polar Engineering Conference, Anchorage, AK, USA, 30 June–5 July 2013. [Google Scholar]
- Yang, M.D.; Teng, B.I.N. Static and dynamic analysis of mooring lines by nonlinear finite element method. China Ocean Eng. 2010, 24, 417–430. [Google Scholar]
- Muhammad, N.; Ullah, Z.; Choi, D.H. A numerical procedure accounting for fluid drag forces and cable extensibility for the static response of mooring cables. Int. J. Steel Struct. 2018, 18, 293–303. [Google Scholar] [CrossRef]
- Sharma, A.K.; Sinha, S.S.; Kumar, R.; Saha, S.K. Semi-analytical solution for static and quasi-static analysis of an inextensible cable. Int. J. Solids Struct. 2022, 234, 111296. [Google Scholar] [CrossRef]
- Irvine, M. Cable Structures; (No. Sirsi) i9780486671277; Dover Publications: Mineola, NY, USA, 1992. [Google Scholar]
- Masciola, M.; Jonkman, J.; Robertson, A. Implementation of a multisegmented, quasi-static cable model. In Proceedings of the Twenty-Third International Offshore and Polar Engineering Conference, Anchorage, AK, USA, 30 June–5 July 2013. [Google Scholar]
- Wan, R.; Hu, F.; Tokai, T.; Matuda, K.O. A method for analyzing the static response of submerged rope systems based on a finite element method. Fish. Sci. 2002, 68, 65–70. [Google Scholar] [CrossRef] [Green Version]
- Wu, S. Adaptive dynamic relaxation technique for static analysis of catenary mooring. Mar. Struct. 1995, 8, 585–599. [Google Scholar] [CrossRef]
- Smith, R.J.; MacFarlane, C.J. Statics of a three component mooring line. Ocean Eng. 2001, 28, 899–914. [Google Scholar] [CrossRef]
- Zhu, X.; Yoo, W.S. Suggested new element reference frame for dynamic analysis of marine cables. Nonlinear Dynam. 2017, 87, 489–501. [Google Scholar] [CrossRef]
- Han, H.; Li, X.; Zhou, H.S. 3D mathematical model and numerical simulation for laying marine cable along prescribed trajectory on seabed. Appl. Math. Model. 2018, 60, 94–111. [Google Scholar] [CrossRef]
- Vaz, M.A.; Li, X.; Liu, J.; Ma, X. Analytical model for axial vibration of marine cables considering equivalent distributed viscous damping. Appl. Ocean Res. 2021, 113, 102733. [Google Scholar] [CrossRef]
- Bai, Y.; Zhang, D.; Zhu, K.; Zhang, T. Dynamic analysis of umbilical cable under interference with riser. Ships Offshore Struct. 2018, 13, 809–821. [Google Scholar] [CrossRef]
- Huang, S. Stability analysis of the heave motion of marine cable-body systems. Ocean Eng. 1999, 26, 531–546. [Google Scholar] [CrossRef]
- Chucheepsakul, S.; Srinil, N.; Petchpeart, P. A variational approach for three-dimensional model of extensible marine cables with specified top tension. Appl. Math. Model. 2003, 27, 781–803. [Google Scholar] [CrossRef] [Green Version]
- Zhu, Z.H. Dynamic modeling of cable system using a new nodal position finite element method. Int. J. Numer. Meth. Bio. Eng. 2010, 26, 692–704. [Google Scholar] [CrossRef]
- Zhu, K.; Zheng, D.; Cai, Y.; Yu, C.; Wang, R.; Liu, Y.; Zhang, F. Nonlinear hydrodynamic response of marine cable-body system under random dynamic excitations. J. Hydrodyn. 2009, 21, 851–855. [Google Scholar] [CrossRef]
- Xu, X.; Wang, S. A flexible-segment-model-based dynamics calculation method for free hanging marine risers in re-entry. China Ocean Eng. 2012, 26, 139–152. [Google Scholar] [CrossRef]
- Witz, J.A.; Tan, Z. Rotary bending of marine cables and umbilicals. Eng. Struct. 1995, 17, 267–275. [Google Scholar] [CrossRef]
- Witz, J.A.; Tan, Z. On the axial-torsional structural behaviour of flexible pipes, umbilicals and marine cables. Mar. Struct. 1992, 5, 205–227. [Google Scholar] [CrossRef]
- Wang, F.; Huang, G.; Deng, D. Dynamic response analysis of towed cable during deployment/retrieval. J. Shanghai Jiaotong Univ. (Sci.) 2008, 13, 245–251. [Google Scholar] [CrossRef]
- Vaz, M.A.; Patel, M.H. Three-dimensional behaviour of elastic marine cables in sheared currents. Appl. Ocean Res. 2000, 22, 45–53. [Google Scholar] [CrossRef]
- Jung, D.H.; Park, H.I.; Koterayama, W. A numerical and experimental study on dynamics of a towed low-tension cable. In Proceedings of the Twelfth International Offshore and Polar Engineering Conference, Kitakyushu, Japan, 26–31 May 2002. [Google Scholar]
- Niedzwecki, J.M.; Thampi, S.K. Snap loading of marine cable systems. Appl. Ocean Res. 1991, 13, 2–11. [Google Scholar] [CrossRef]
- Mavrakos, S.A.; Papazoglou, V.J.; Triantafyllou, M.S.; Hatjigeorgiou, J. Deep water mooring dynamics. Mar. Struct. 1996, 9, 181–209. [Google Scholar] [CrossRef]
- Kurniawan, A.; Yosi, M.; Arief, I.S.; Cahyagi, D. Design of marine cable installation for ocean current power plant in Toyopakeh Strait-Bali. In Proceedings of the 2017 International Conference on Advanced Mechatronics, Intelligent Manufacture, and Industrial Automation (ICAMIMIA), Surabaya, Indonesia, 12–14 October 2017. [Google Scholar]
- Huang, S. Dynamic analysis of three-dimensional marine cables. Ocean Eng. 1994, 21, 587–605. [Google Scholar] [CrossRef]
- Huang, S.; Vassalos, D. A semi-analytic treatment of three-dimensional statics of marine cables. Ocean Eng. 1993, 20, 409–420. [Google Scholar] [CrossRef]
- Ghadimi, R. A simple and efficient algorithm for the static and dynamic analysis of flexible marine risers. Comput. Struct. 1988, 29, 541–555. [Google Scholar] [CrossRef]
- Peltzer, R.D.; Rooney, D.M. Near wake properties of a strumming marine cable: An experimental study. J. Fluids Eng. 1985, 107, 86–91. [Google Scholar] [CrossRef]
- Chen, B.; Su, F.; Huo, C.F.; Zhang, R.B.; Yao, B.H.; Lian, L. Numerical investigation of the dynamics for low tension marine cables. J. Shanghai Jiaotong Univ. (Sci.) 2015, 20, 257–264. [Google Scholar] [CrossRef]
- Bian, A.; Zou, Z.; Zhou, H.W.; Zhang, J. Evaluation of multi-scale full waveform inversion with marine vertical cable data. J. Earth Sci. 2015, 26, 481–486. [Google Scholar] [CrossRef]
- Beasley, C.J.; Coates, R.T.; Flath, P.; Castellanos, C. Slanted cable marine acquisition and wave-equation receiver deghosting. In Proceedings of the 2015 SEG Annual Meeting, New Orleans, LA, USA, 18–23 October 2015. [Google Scholar]
- Peyrot, A.H. Marine cable structures. J. Struct. Div. 1980, 106, 2391–2404. [Google Scholar] [CrossRef]
- Yan, J.; Hu, H.; Lu, H.; Yin, Y.; Bu, Y.; Lu, Q. Experimental study on the influence of cross-section type of marine cable conductors on the bending performance. China Ocean Eng. 2022, 36, 629–637. [Google Scholar] [CrossRef]
- Marty, A.; Berhault, C.; Damblans, G.; Facq, J.V.; Gaurier, B.; Germain, G.; Soulard, T.; Schoefs, F. Experimental study of hard marine growth effect on the hydrodynamical behaviour of a submarine cable. Appl. Ocean Res. 2021, 114, 102810. [Google Scholar] [CrossRef]
- Chen, Y.; Li, J.; Wang, S.; Han, G.; Sun, Y.; Luo, W. Dynamic Modeling and Robust Adaptive Sliding Mode Controller for Marine Cable-Driven Parallel Derusting Robot. Appl. Sci. 2022, 12, 6137. [Google Scholar] [CrossRef]
- Cai, L.; Lin, J.; Liao, X. Residual life prediction of marine EPR cable under discontinuous operation. IET Gener. Transm. Distrib. 2020, 14, 6306–6311. [Google Scholar] [CrossRef]
- Rodríguez, L.Á.; Armesto, J.A.; Guanche, R.; Barrera, C.; Vidal, C. Simulation of marine towing cable dynamics using a finite elements method. J. Mar. Sci. Eng. 2020, 8, 140. [Google Scholar] [CrossRef] [Green Version]
- Casarella, M.J.; Parsons, M. Cable systems under hydrodynamic loading. Mar. Technol. Soc. J. 1970, 4, 27–44. [Google Scholar]
- Park, J.; Kim, N. Dynamics modeling of a semi-submersible autonomous underwater vehicle with a towfish towed by a cable. Int. J. Naval Arch. Ocean Eng. 2015, 7, 409–425. [Google Scholar] [CrossRef] [Green Version]
- Nguyen, T.D.; Egeland, O. Output Feedback Stabilization of Towed Marine Cable. In Proceedings of the OCEANS 2006—Asia Pacific, Singapore, 16–19 May 2006. [Google Scholar]
- Fukumoto, H.; Nakanishi, H.; Namita, Y. Dynamic response analysis of marine cable structure and its computational program. Doboku Gakkai Ronbunshu 1985, 356, 455–464. [Google Scholar] [CrossRef] [Green Version]
- Wildemann, E.R. Reduction of the Flow Induced Vibration Response of a Marine Cable by Altering the Cable Boundary Conditions; Massachusetts Institute of Technology: Cambridge, MA, USA, 1983. [Google Scholar]
- Westin, C.; Irani, R.A. Modeling dynamic cable–sheave contact and detachment during towing operations. Mar. Struct. 2021, 77, 102960. [Google Scholar] [CrossRef]
- Guo, L.; Yuan, Y.; Tang, W.; Xue, H. A numerical investigation on quasi-static configuration and nonlinear dynamic response characteristics of marine towing cable. Ocean Eng. 2021, 240, 110007. [Google Scholar] [CrossRef]
- Kamman, J.W.; Huston, R.L. Multibody dynamics modeling of variable length cable systems. Multibody Syst. Dyn. 2001, 5, 211–221. [Google Scholar] [CrossRef]
- Chatjigeorgiou, I.K.; Mavrakos, S.A. Cable dynamics for marine applications. In Springer Handbook of Ocean Engineering; Springer: Cham, Switzerland, 2016; pp. 875–906. [Google Scholar]
- Shin, H.K. Cable Dynamics for Marine Applications-Nonlinearities. J. Ocean Eng. Technol. 1990, 4, 35–40. [Google Scholar]
- Yang, N.; Jeng, D.S. Three-dimensional Analysis of Elastic Marine Cable during Laying. In Proceedings of the Eleventh ISOPE Pacific/Asia Offshore Mechanics Symposium, Shanghai, China, 12–14 October 2014. [Google Scholar]
- Prpić-Oršić, J.; Nabergoj, R. Nonlinear dynamics of an elastic cable during laying operations in rough sea. Appl. Ocean Res. 2005, 27, 255–264. [Google Scholar] [CrossRef]
- Athisakul, C.; Monprapussorn, T.; Chucheepsakul, S. A variational formulation for three-dimensional analysis of extensible marine riser transporting fluid. Ocean Eng. 2011, 38, 609–620. [Google Scholar] [CrossRef]
- Wang, Y.; Bian, X.; Zhang, X.; Xie, W. A study on the influence of cable tension on the movement of cable laying ship. In Proceedings of the OCEANS 2010 MTS/IEEE SEATTLE, Seattle, WA, USA, 20–23 September 2010; pp. 1–8. [Google Scholar]
- Delmer, T.N.; Stephens, T.C.; Tremills, J.A. Numerical simulation of cable-towed acoustic arrays. Ocean Eng. 1988, 15, 511–548. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, S.; Wang, D.; Lin, L.; Zhu, X. Synchronous clamping control of marine umbilical cable tensioner. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2013, 227, 2328–2336. [Google Scholar] [CrossRef]
- Wu, W.; Zhao, Y.; Gou, Y.; Lyu, B.C.; Lu, Q.Z.; Lu, Z.K.; Yan, J. An overview of structural design, analysis and common monitoring technologies for floating platform and flexible cable and riser. China Ocean Eng. 2022, 36, 511–531. [Google Scholar] [CrossRef]
- Zhu, X.; Yoo, W.S. Numerical modeling of a spar platform tethered by a mooring cable. Chin. J. Mech. Eng. 2015, 28, 785–792. [Google Scholar] [CrossRef]
- Bergdahl, L.; Palm, J.; Eskilsson, C.; Lindahl, J. Dynamically scaled model experiment of a mooring cable. J. Mar. Sci. Eng. 2016, 4, 5. [Google Scholar] [CrossRef] [Green Version]
- Orcina. OrcaFlex Manual. 2015. Available online: http://www.orcina.com/SoftwareProducts/OrcaFlex/Validation/index/.pdf (accessed on 1 January 2015).


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, D.; Zhao, B.; Sun, J.; Zhang, Y.; Zhu, K.; Jiang, H. Influence of Different Static Equilibrium Calculation Methods on the Dynamic Response of Marine Cables during the Releasing Process: Review and a Case Study. J. Mar. Sci. Eng. 2023, 11, 764. https://doi.org/10.3390/jmse11040764
Zhang D, Zhao B, Sun J, Zhang Y, Zhu K, Jiang H. Influence of Different Static Equilibrium Calculation Methods on the Dynamic Response of Marine Cables during the Releasing Process: Review and a Case Study. Journal of Marine Science and Engineering. 2023; 11(4):764. https://doi.org/10.3390/jmse11040764
Chicago/Turabian StyleZhang, Dapeng, Bowen Zhao, Jiyuan Sun, Yi Zhang, Keqiang Zhu, and Haoyu Jiang. 2023. "Influence of Different Static Equilibrium Calculation Methods on the Dynamic Response of Marine Cables during the Releasing Process: Review and a Case Study" Journal of Marine Science and Engineering 11, no. 4: 764. https://doi.org/10.3390/jmse11040764
APA StyleZhang, D., Zhao, B., Sun, J., Zhang, Y., Zhu, K., & Jiang, H. (2023). Influence of Different Static Equilibrium Calculation Methods on the Dynamic Response of Marine Cables during the Releasing Process: Review and a Case Study. Journal of Marine Science and Engineering, 11(4), 764. https://doi.org/10.3390/jmse11040764







