Analytical Solution for Negative Skin Friction in Offshore Wind Power Pile Foundations on Artificial Islands under the Influence of Soil Consolidation
Abstract
:1. Introduction
2. Calculation Model
2.1. Basic Assumptions
- (1)
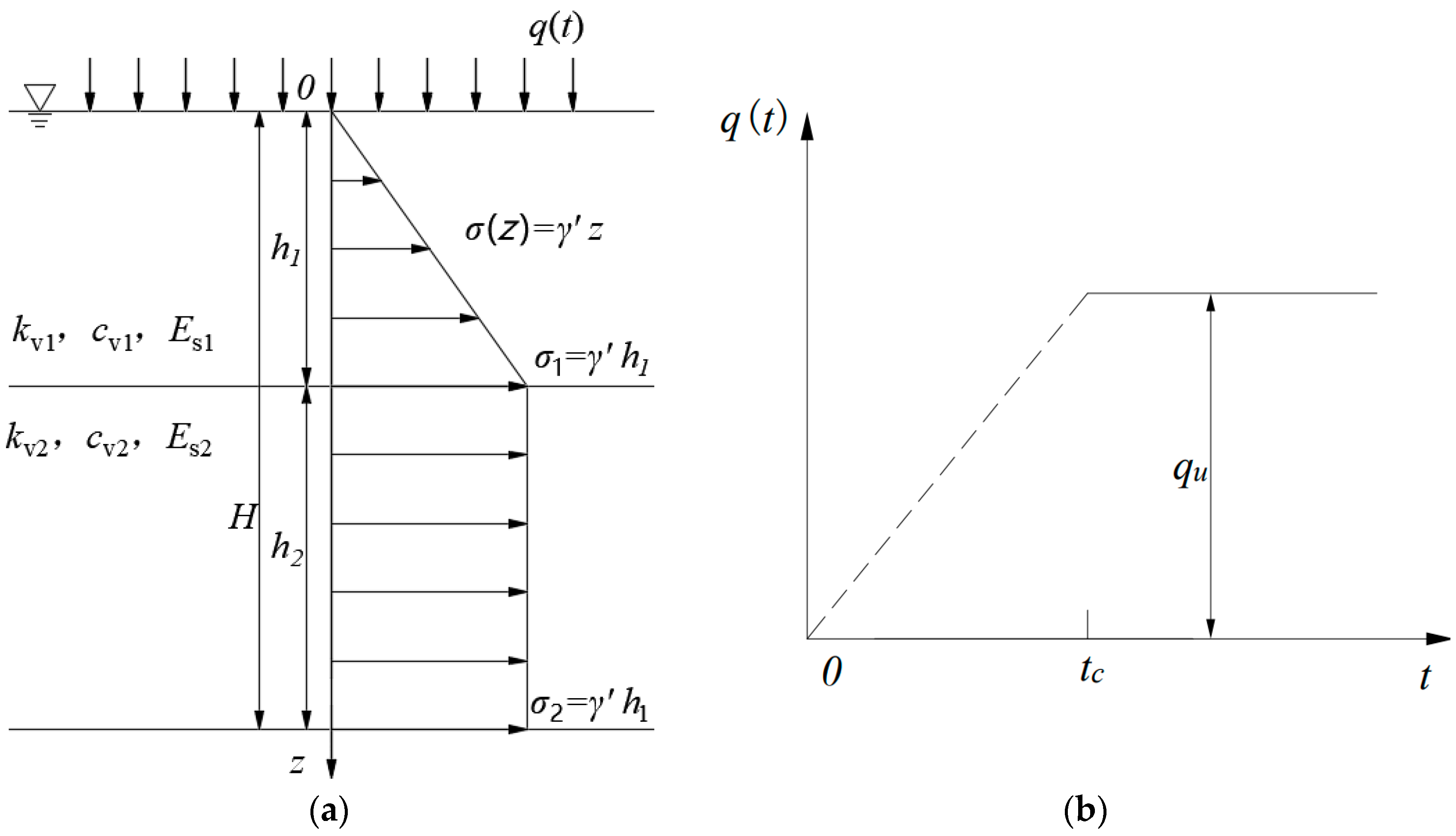
- The initial self-weight stress of the fill soil linearly distributes along the depth, as depicted in Figure 1;
- (2)
- The top surface of the soil layer is pervious, while the bottom surface is assumed to be impervious, resulting in a single-sided drainage state for the soil;
- (3)
- The disturbance caused by the installation of offshore wind power pile foundations on artificial islands is neglected, and the pile head load () remains constant during the consolidation process;
- (4)
- The load-transfer model is used to describe the relationship between the relative displacement of the pile–soil system and the skin friction acting on the pile shaft. The load transfer relationship along the pile shaft is assumed to be ideal elastoplastic behavior, as shown in Figure 2a, while the pile tip load-displacement relationship is described by a linear elastic model, as shown in Figure 2b. In Figure 2a, represents the skin friction acting on the pile shaft; represents the pile–soil relative displacement in the -th layer (); () represents the ultimate positive (negative) skin friction in the -th layer; and () represents the corresponding ultimate relative displacement. The coefficient represents the elastic shear stiffness and the same shear stiffness coefficient is used for calculating PSF and NSF, respectively, and they are assumed to be constant along the pile shaft. In Figure 2b, represents the tip resistance acting on the pile; represents the relative displacement of the tip soil; and represents the compressive stiffness coefficient at the pile tip;
- (5)
- Other assumptions are consistent with Terzaghi’s one-dimensional consolidation theory [25].
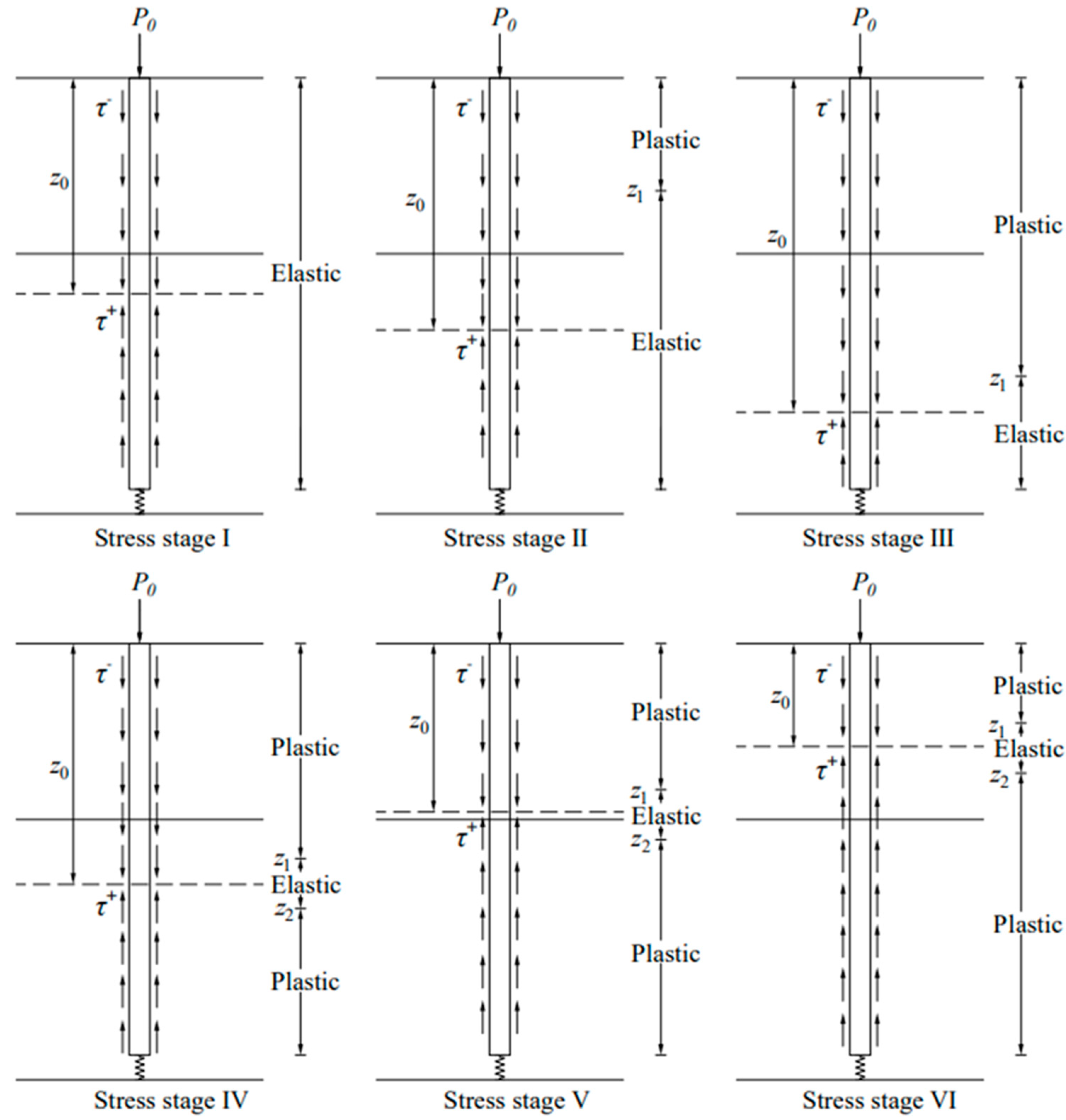
2.2. Calculation Models for Each Stage
- (1)
- Elastic shear stage: When the axial force at the top of the pile and soil consolidation is relatively small, the relative displacement of the pile–soil system is less than the ultimate relative displacement , and the ultimate skin friction cannot be fully mobilized. The soil surrounding the pile is in an elastic shear stage;
- (2)
- Plastic–elastic shear stage: When the axial force at the top of the pile is large or the soil is highly consolidated, the relative displacement of the pile–soil system in the upper part of the pile exceeds the ultimate relative displacement , and the ultimate skin friction is fully mobilized. Part of the soil surrounding the pile begins to transition into the plastic state, with the boundary depth of the plastic area denoted as ;
- (3)
- Plastic–elastic–plastic shear stage: As the axial force at the top of the pile or soil consolidation continues to increase, both the NSF and PSF of the pile transition into the plastic state. Due to the existence of the neutral plane, which is denoted as , the soil near the neutral plane remains in an elastic state. The boundary depth of the plastic area of NSF is denoted as , while the boundary depth of the plastic area of PSF is denoted as , and .
3. Governing Equations and Solutions for Load Transfer
3.1. Solution for One-Dimensional Consolidation of Double-Layer Foundation
3.2. Solution for Pile Foundation
3.2.1. Basic Governing Equations
3.2.2. Solution for Elastic Stage of the Pile–Soil System
3.2.3. Solution for Plastic–Elastic Stage of the Pile–Soil System
3.2.4. Solution for Plastic–Elastic–Plastic Stage of the Pile–Soil System
4. Verification
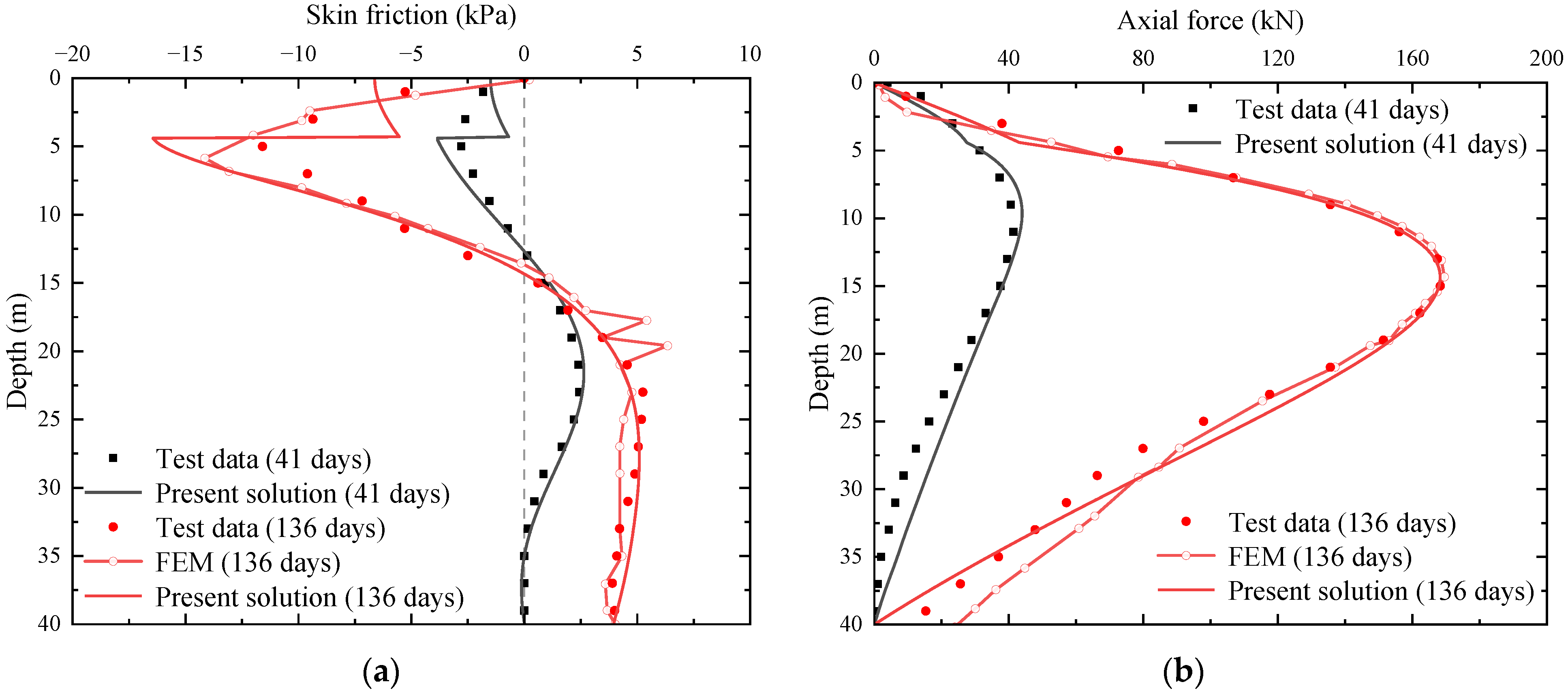
4.1. Case Histories: Yang [31]
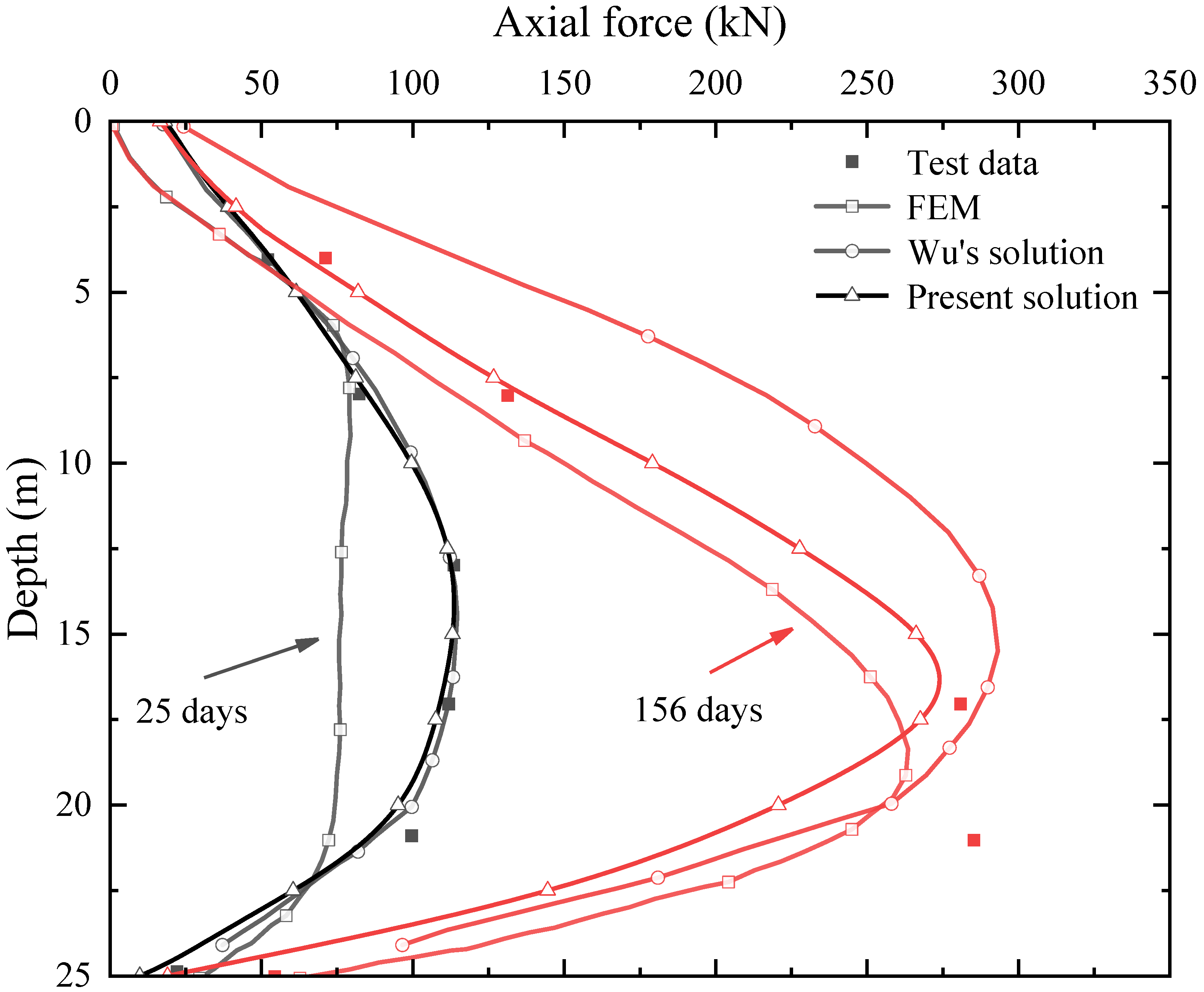
4.2. Case Histories: Indraratna et al. [32]
5. Parametric Study
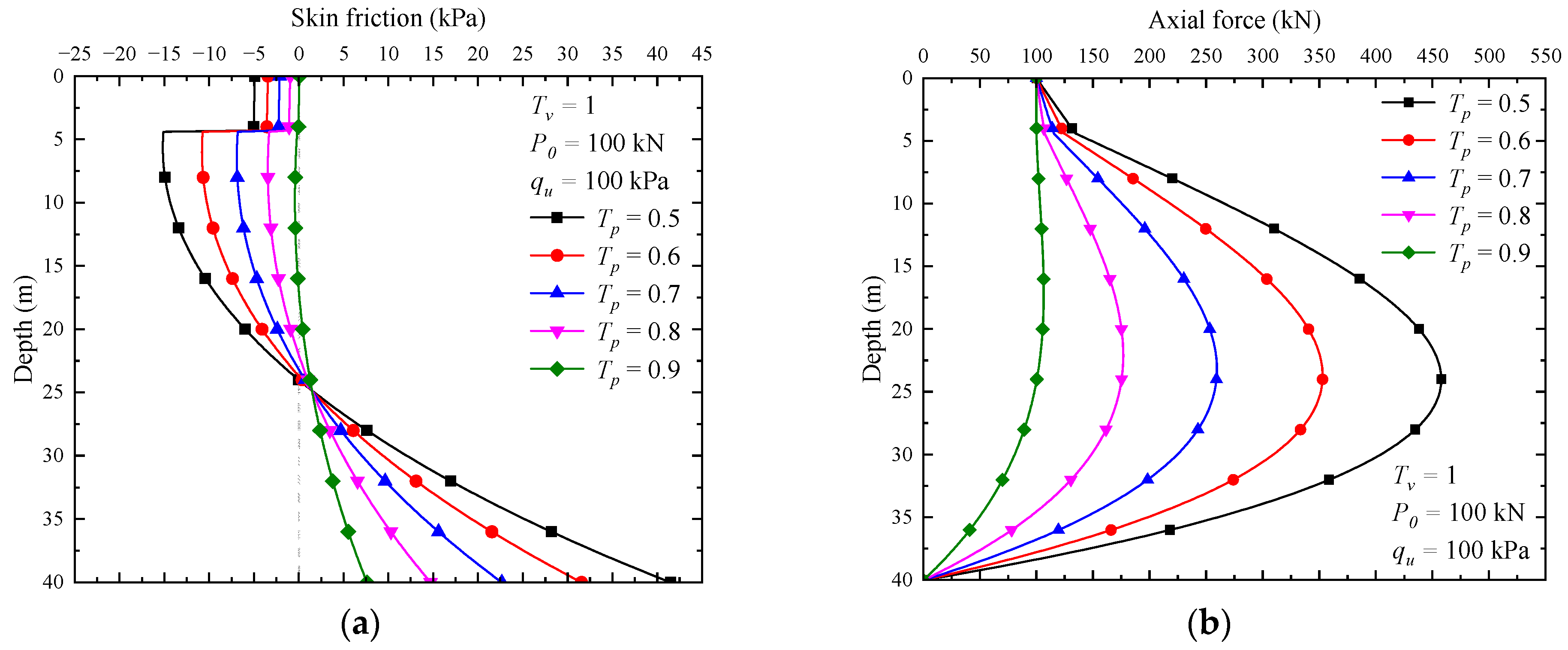
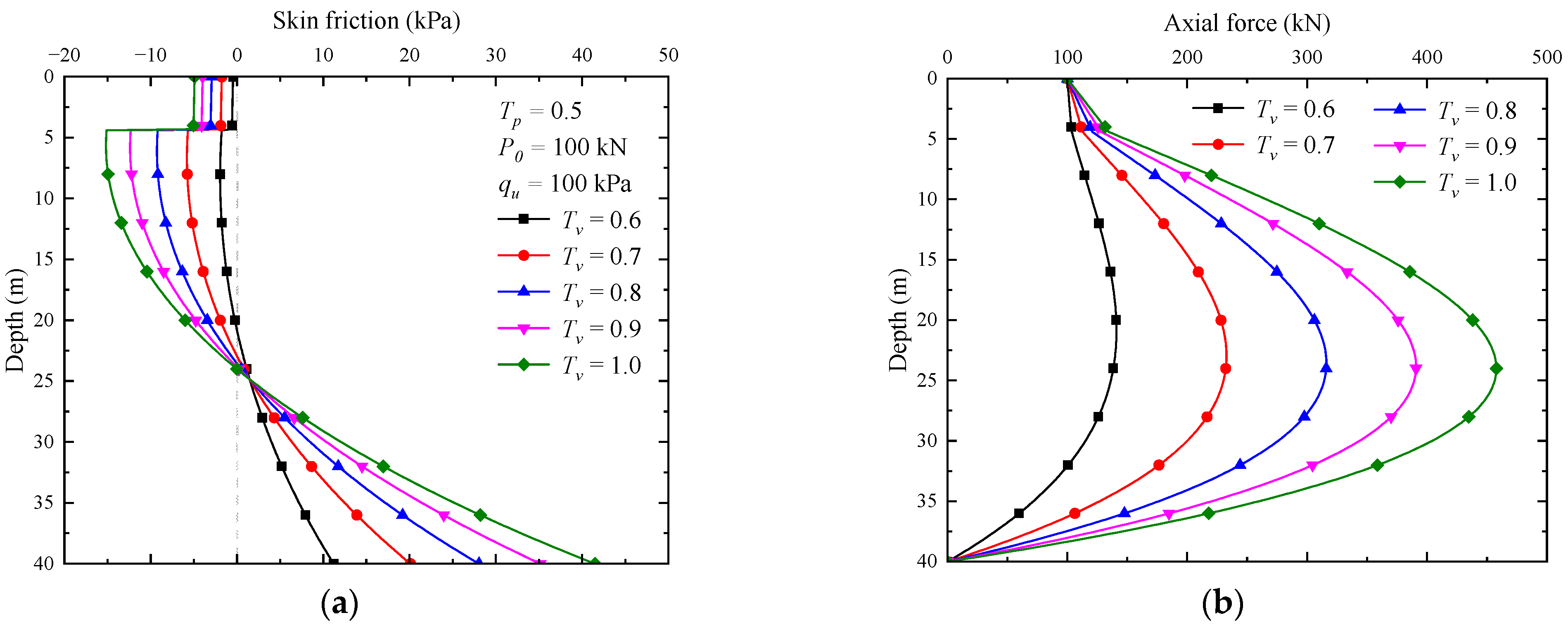
5.1. Case 1: Influence of the Pile Installation Time
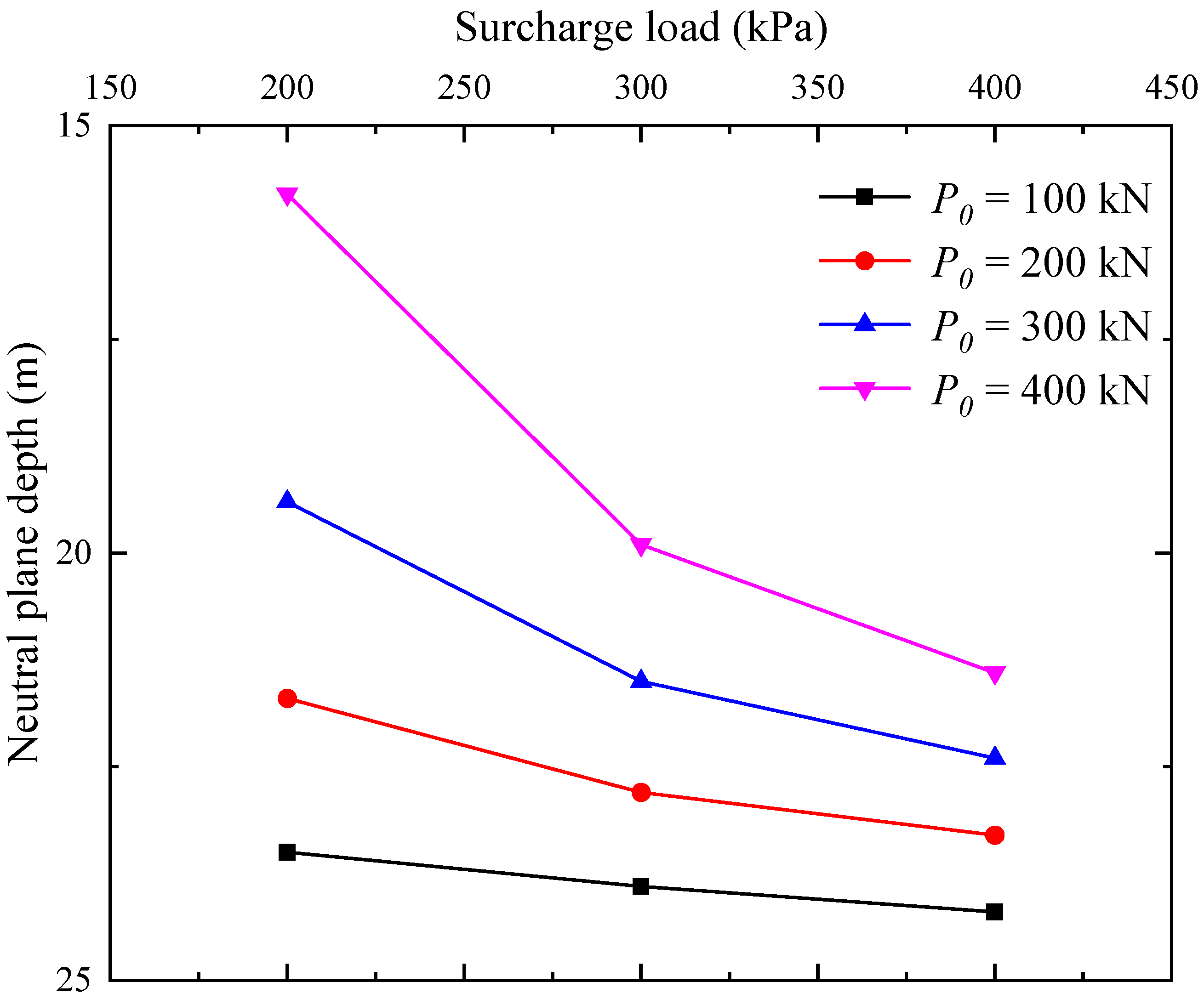
5.2. Case 2: Influence of Surcharge Load
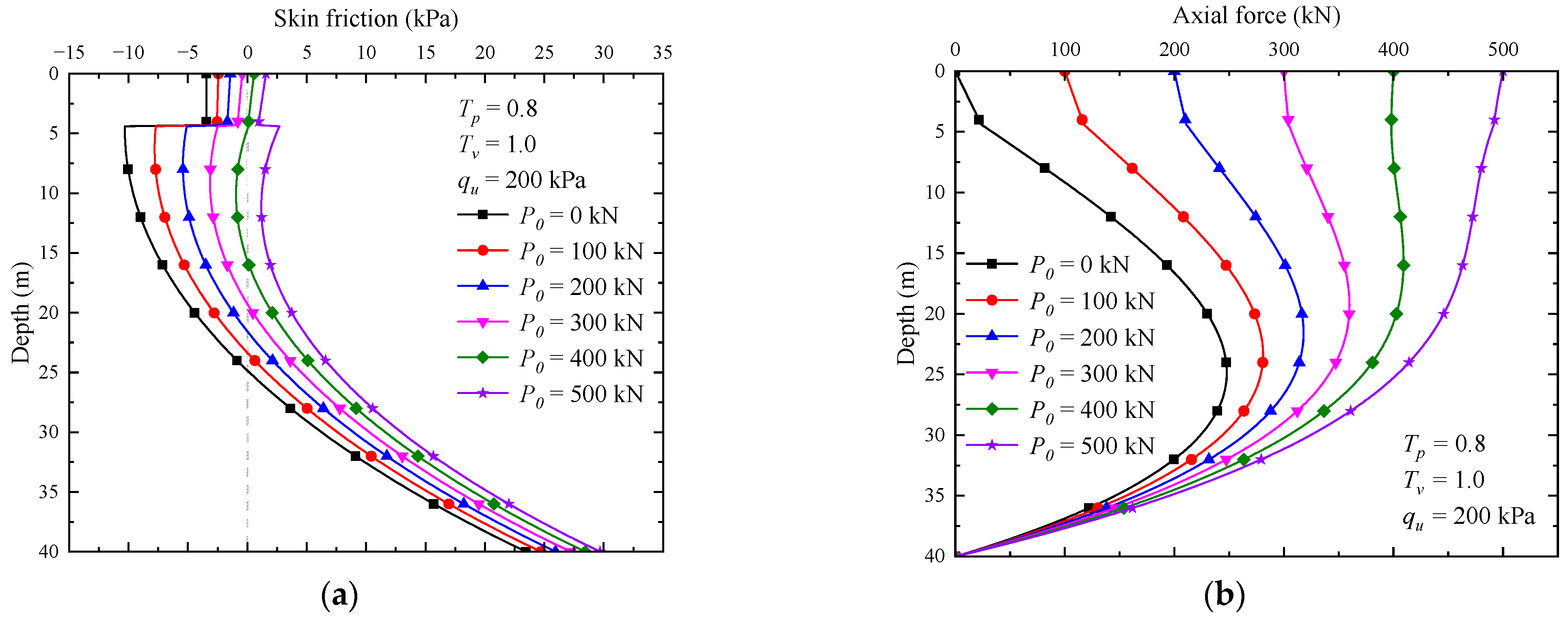
5.3. Case 3: Influence of Pile Head Load
6. Conclusions
- (1)
- Compared to existing methods, considering the elastic state of the soil near the neutral plane during the plastic stage of pile–soil interaction analysis provides a better prediction of the distribution of NSF under vertical loads considering consolidation;
- (2)
- Installing pile foundations immediately after soil filling results in NSF several times greater than that of installing piles after a period of consolidation. To balance the reduction of NSF and the shortening of construction time, pile installation can be carried out when = 90% ;
- (3)
- As the surcharge load increases, the increase in NSF inevitably leads to an increase in axial force on the pile, and the position of the neutral plane moves downward. Increasing the pile head load can reduce NSF and raise the neutral plane. This measure effectively mitigates the impact of soil consolidation on pile foundations. However, as the surcharge load increases, the influence of the pile top load gradually diminishes.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix C
Appendix D
Appendix E
Appendix F
References
- Soares-Ramos, E.P.; de Oliveira-Assis, L.; Sarrias-Mena, R.; Fernández-Ramírez, L.M. Current status and future trends of offshore wind power in Europe. Energy 2020, 202, 117787. [Google Scholar] [CrossRef]
- Hallak, T.S.; Guedes Soares, C.; Sainz, O.; Hernández, S.; Arévalo, A. Hydrodynamic Analysis of the WIND-Bos Spar Floating Offshore Wind Turbine. J. Mar. Sci. Eng. 2022, 10, 1824. [Google Scholar] [CrossRef]
- Pérez-Collazo, C.; Greaves, D.; Iglesias, G. A review of combined wave and offshore wind energy. Renew. Sustain. Energy Rev. 2015, 42, 141–153. [Google Scholar] [CrossRef]
- Salo, O.; Syri, S. What economic support is needed for Arctic offshore wind power? Renew. Sustain. Energy Rev. 2014, 31, 343–352. [Google Scholar] [CrossRef]
- Jansen, M.; Duffy, C.; Green, T.C.; Staffell, I. Island in the Sea: The prospects and impacts of an offshore wind power hub in the North Sea. Adv. Appl. Energy 2022, 6, 100090. [Google Scholar] [CrossRef]
- Lee, C.; Ng, C.; Jeong, S. The effect of negative skin friction on piles and pile groups. In Linear and Non-Linear Numerical Analysis of Foundations; CRC Press: Boca Raton, FL, USA, 2009; pp. 193–242. [Google Scholar]
- Liang, R.; Yin, Z.-Y.; Yin, J.-H.; Wu, P.-C. Numerical analysis of time-dependent negative skin friction on pile in soft soils. Comput. Geotech. 2023, 155, 105218. [Google Scholar] [CrossRef]
- Chiou, J.-S.; Wei, W.-T. Numerical investigation of pile-head load effects on the negative skin friction development of a single pile in consolidating ground. Acta Geotech. 2021, 16, 1867–1878. [Google Scholar] [CrossRef]
- Jeong, S.; Ko, J.; Lee, C.; Kim, J. Response of single piles in marine deposits to negative skin friction from long-term field monitoring. Mar. Georesources Geotechnol. 2014, 32, 239–263. [Google Scholar] [CrossRef]
- Rituraj, S.S.; Giridhar Rajesh, B. Negative skin friction on piles: State of the art. In Advances in Geo-Science and Geo-Structures: Select Proceedings of GSGS 2020; Springer: Berlin/Heidelberg, Germany, 2022; pp. 323–335. [Google Scholar]
- Abdrabbo, F.M.; Ali, N.A. Behaviour of single pile in consolidating soil. Alex. Eng. J. 2015, 54, 481–495. [Google Scholar] [CrossRef]
- Shen, K.; Wang, K.; Yao, J.; Yu, J. Numerical Investigation on Behavior of Compressive Piles in Coastal Tidal Flat with Fill. J. Mar. Sci. Eng. 2022, 10, 1742. [Google Scholar] [CrossRef]
- Chow, Y.; Lim, C.; Karunaratne, G. Numerical modelling of negative skin friction on pile groups. Comput. Geotech. 1996, 18, 201–224. [Google Scholar] [CrossRef]
- Wang, J.; Zhou, D.; Zhang, Y.; Cai, W. Vertical impedance of a tapered pile in inhomogeneous saturated soil described by fractional viscoelastic model. Appl. Math. Model. 2019, 75, 88–100. [Google Scholar] [CrossRef]
- Cheng, H.W.; Cheng, Z.; Jiang, C.; Li, Y. Influences of ultimate frictional resistance at low confining pressure on bearing characteristics of backfill piles. Yantu Gongcheng Xuebao/Chin. J. Geotech. Eng. 2018, 40, 87–90. [Google Scholar]
- Kim, H.-J.; Mission, J.L.; Park, T.-W.; Dinoy, P.R. Analysis of negative skin-friction on single piles by one-dimensional consolidation model test. Int. J. Civ. Eng. 2018, 16, 1445–1461. [Google Scholar] [CrossRef]
- Fellenius, B.H. Results from long-term measurement in piles of drag load and downdrag. Can. Geotech. J. 2006, 43, 409–430. [Google Scholar] [CrossRef]
- Ng, C.W.; Poulos, H.G.; Chan, V.S.; Lam, S.S.; Chan, G.C. Effects of tip location and shielding on piles in consolidating ground. J. Geotech. Geoenviron. Eng. 2008, 134, 1245–1260. [Google Scholar] [CrossRef]
- Yu, P.; Dong, J.; Liu, H.; Xu, R.; Wang, R.; Xu, M.; Liu, H. Analysis of cyclic shear stress–displacement mechanical properties of silt–steel interface in the Yellow River Delta. J. Mar. Sci. Eng. 2022, 10, 1704. [Google Scholar] [CrossRef]
- Mindlin, R.D. Force at a point in the interior of a semi-infinite solid. Physics 1936, 7, 195–202. [Google Scholar] [CrossRef]
- Cao, W.; Chen, Y.; Wolfe, W. New load transfer hyperbolic model for pile-soil interface and negative skin friction on single piles embedded in soft soils. Int. J. Geomech. 2014, 14, 92–100. [Google Scholar] [CrossRef]
- Wu, W.; Wang, Z.; Zhang, Y.; El Naggar, M.H.; Wu, T.; Wen, M. Semi-analytical solution for negative skin friction development on deep foundations in coastal reclamation areas. Int. J. Mech. Sci. 2023, 241, 107981. [Google Scholar] [CrossRef]
- Kim, H.J.; Mission, J.L.C. Negative skin friction on piles based on finite strain consolidation theory and the nonlinear load transfer method. KSCE J. Civ. Eng. 2009, 13, 107–115. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, P.; Xue, S.; Pan, Y. Influence of dredger fill self-consolidation on development of negative skin friction of piles. Arab. J. Geosci. 2020, 13, 725. [Google Scholar] [CrossRef]
- Terzaghi, K. Erdbaumechanik Auf Bodenphysikalischer Grundlage; F. Deuticke, Leipzig and Wien: Leipzig, Germany, 1925. [Google Scholar]
- Xie, K.H. Theory of One Dimensional Consolidation of Double-Layered Ground and its Applications. Chin. J. Geotech. Eng. 1994, 16, 24–35. [Google Scholar]
- Randolph, M.F.; Wroth, C.P. Analysis of deformation of vertically loaded piles. J. Geotech. Eng. Div. 1978, 104, 1465–1488. [Google Scholar] [CrossRef]
- Wong, K.; Teh, C. Negative skin friction on piles in layered soil deposits. J. Geotech. Eng. 1995, 121, 457–465. [Google Scholar] [CrossRef]
- Castelli, F.; Maugeri, M. Simplified nonlinear analysis for settlement prediction of pile groups. J. Geotech. Geoenviron. Eng. 2002, 128, 76–84. [Google Scholar] [CrossRef]
- Fellenius, B.H. Effective stress analysis and set-up for shaft capacity of piles in clay. In From Research to Practice in Geotechnical Engineering; ASCE: Reston, VA, USA, 2008; pp. 384–406. [Google Scholar]
- Yang, J.J. Bearing Performance and Computational Method of Piles in Hydraulic-Reclamation Area. Master’s Thesis, Shanghai Jiaotong University, Shanghai, China, 2014. [Google Scholar]
- Indraratna, B.; Balasubramaniam, A.; Phamvan, P.; Wong, Y. Development of negative skin friction on driven piles in soft Bangkok clay. Can. Geotech. J. 1992, 29, 393–404. [Google Scholar] [CrossRef]




| Soil | ||||||
|---|---|---|---|---|---|---|
| fill soil | 4.4 | 7.385 | 11,477 | 100 | 2207 | – |
| original soil | 45 | 9.527 | 34,364 | 3.48 | 6608 | 37,762 |
| 0.25 | 40 | 36 |
| Soil | ||||||
|---|---|---|---|---|---|---|
| fill soil | 2 | 7 | 15,000 | 7.82 | 2885 | – |
| original soil | 40 | 7.7 | 22,000 | 1.17 | 4231 | 24,176 |
| 0.2 | 27 | 30 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, C.; Shi, Z.; Pang, L. Analytical Solution for Negative Skin Friction in Offshore Wind Power Pile Foundations on Artificial Islands under the Influence of Soil Consolidation. J. Mar. Sci. Eng. 2023, 11, 1071. https://doi.org/10.3390/jmse11051071
Jiang C, Shi Z, Pang L. Analytical Solution for Negative Skin Friction in Offshore Wind Power Pile Foundations on Artificial Islands under the Influence of Soil Consolidation. Journal of Marine Science and Engineering. 2023; 11(5):1071. https://doi.org/10.3390/jmse11051071
Chicago/Turabian StyleJiang, Chong, Zexiong Shi, and Li Pang. 2023. "Analytical Solution for Negative Skin Friction in Offshore Wind Power Pile Foundations on Artificial Islands under the Influence of Soil Consolidation" Journal of Marine Science and Engineering 11, no. 5: 1071. https://doi.org/10.3390/jmse11051071
APA StyleJiang, C., Shi, Z., & Pang, L. (2023). Analytical Solution for Negative Skin Friction in Offshore Wind Power Pile Foundations on Artificial Islands under the Influence of Soil Consolidation. Journal of Marine Science and Engineering, 11(5), 1071. https://doi.org/10.3390/jmse11051071






