A 3D BEM-Coupled Mode Model for the Performance Analysis of Wave Energy Converter Parks in Nearshore-Coastal Regions
Abstract
:1. Introduction
2. Mathematical Formulation
2.1. Formulation of the Incident Field
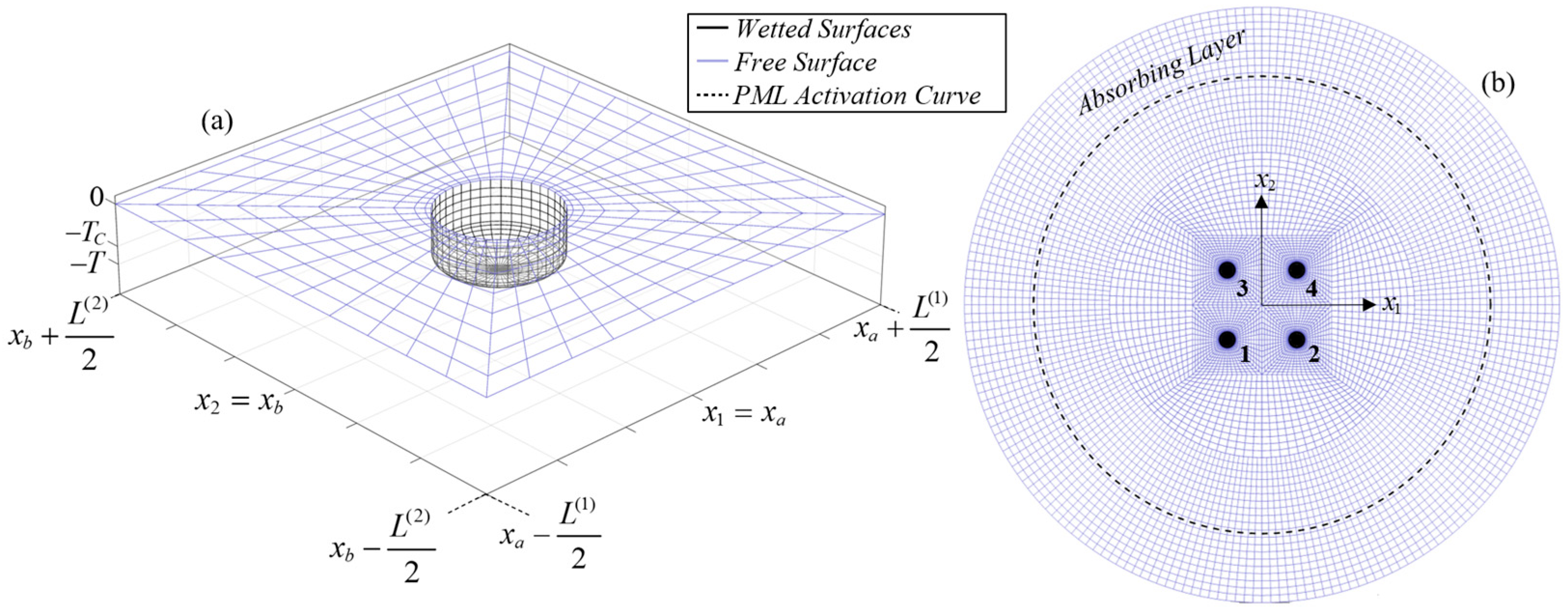
2.2. BEM Formulation for the Diffraction and Radiation Problems
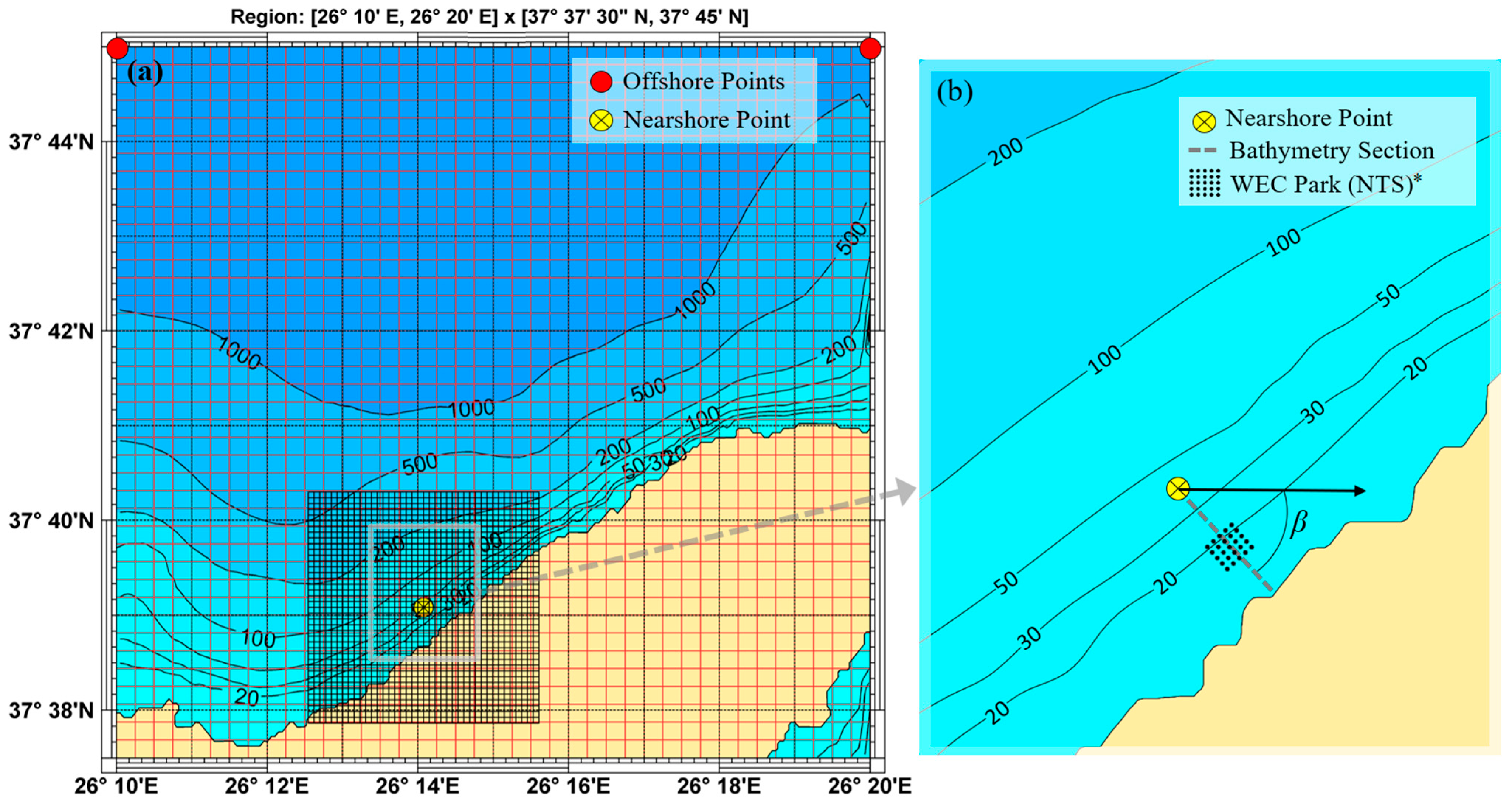
3. Case Study
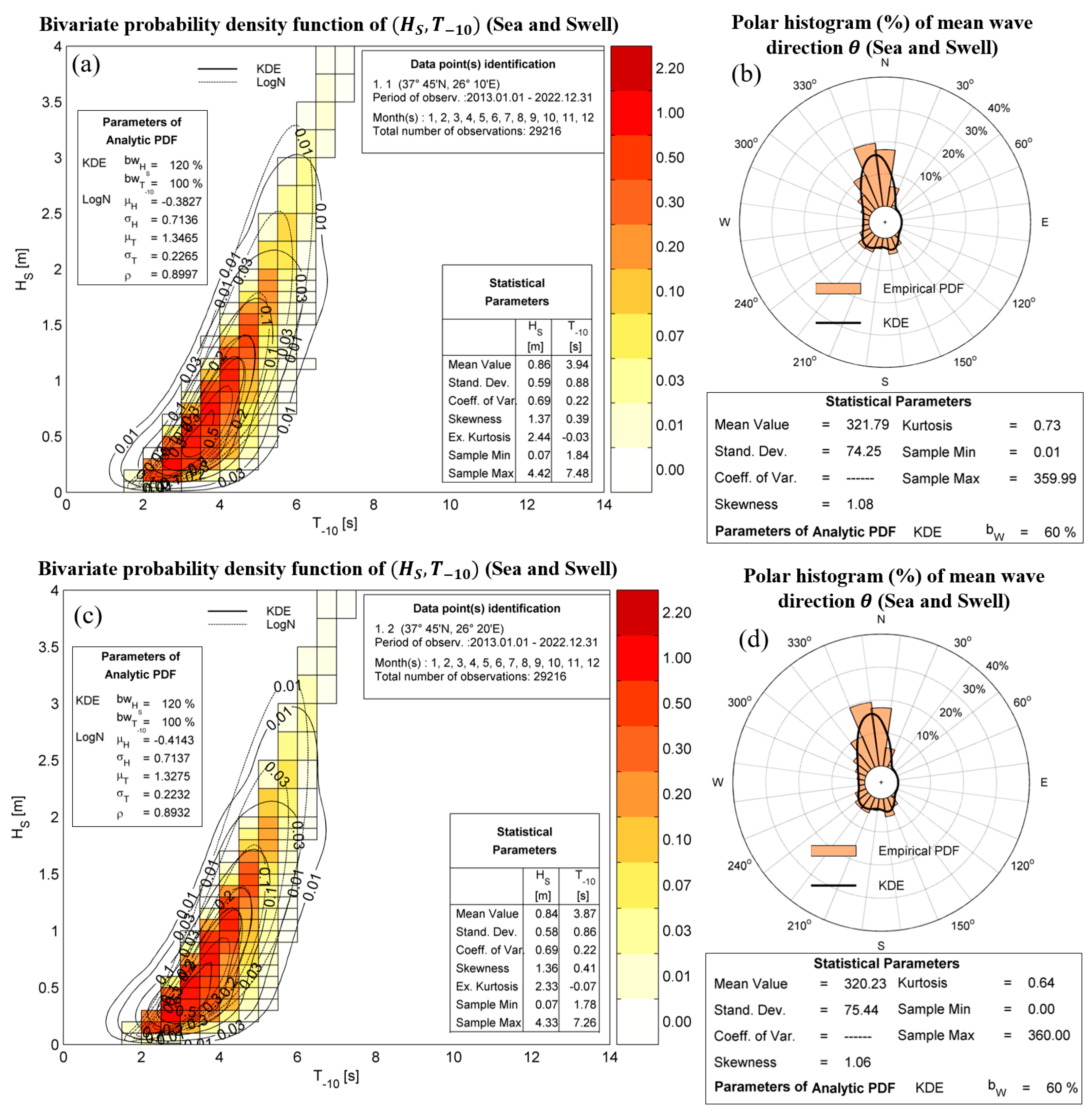
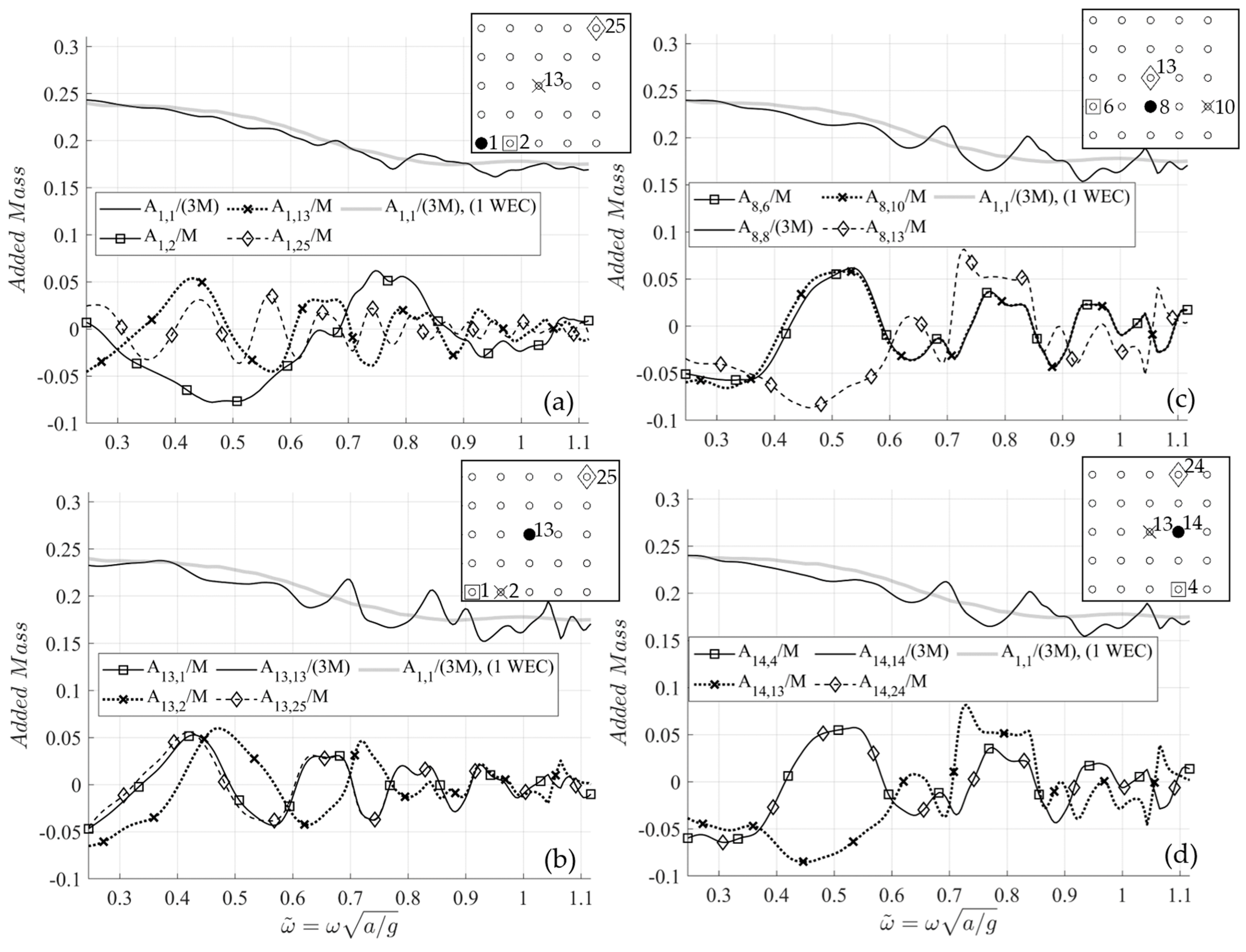
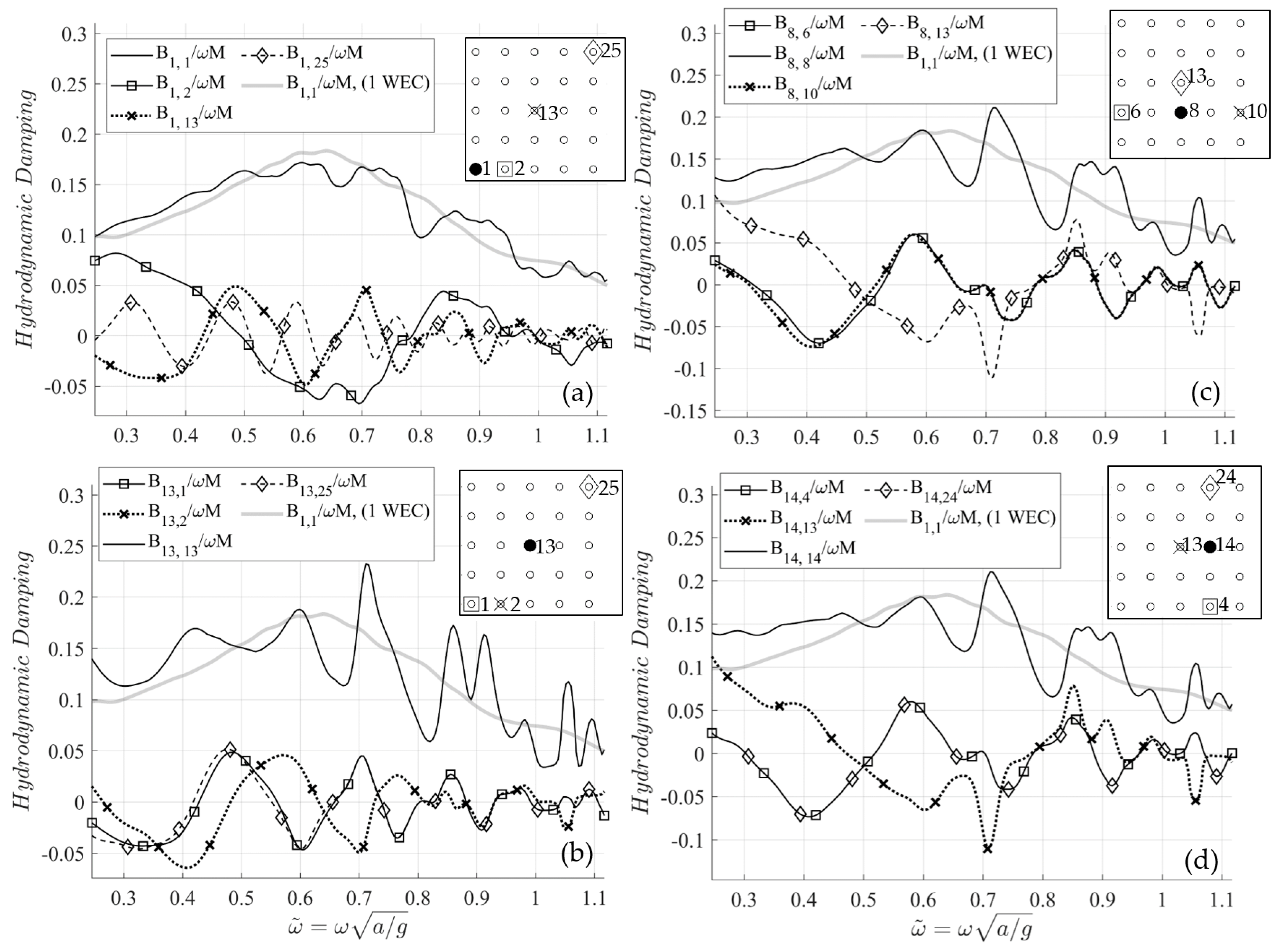
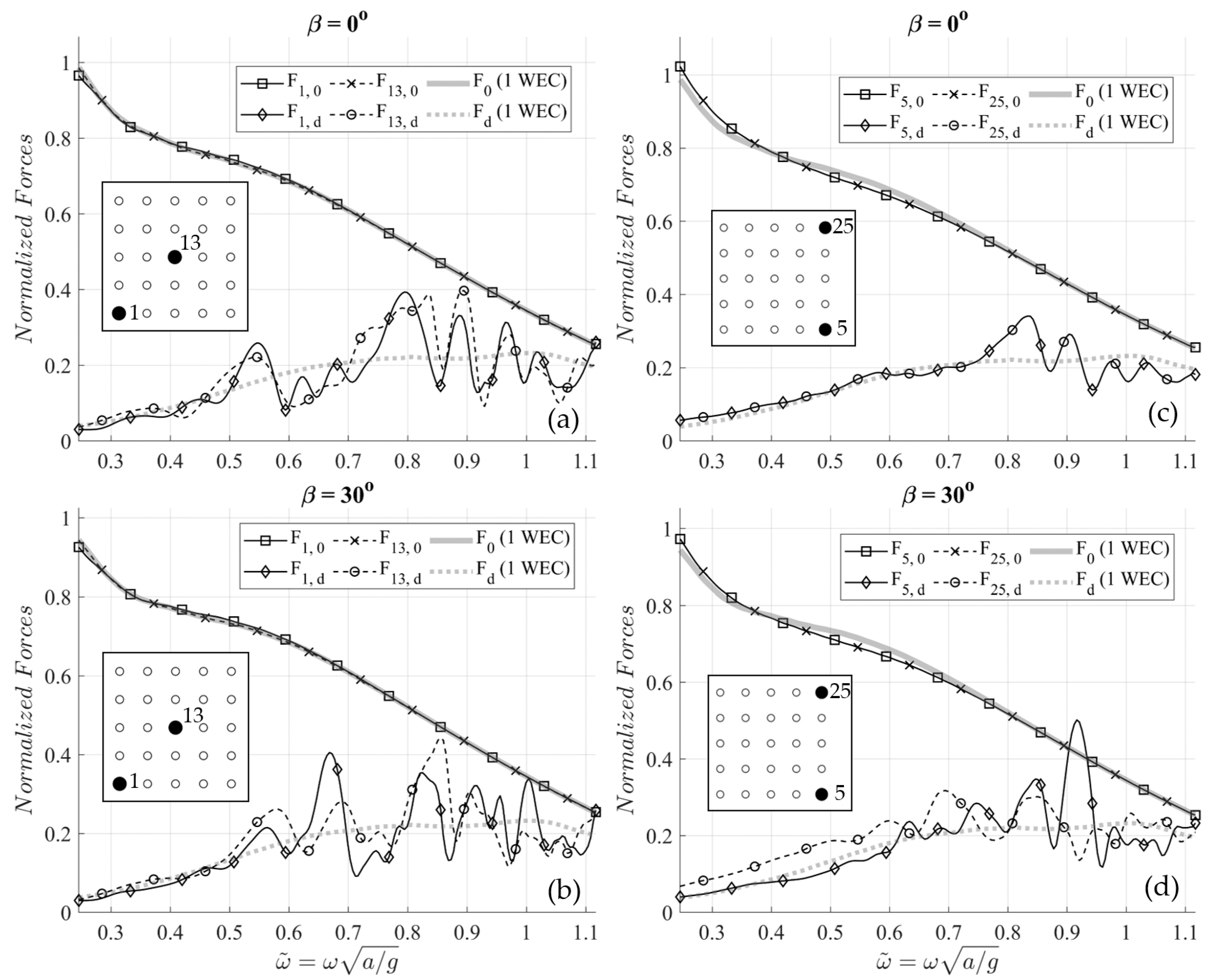
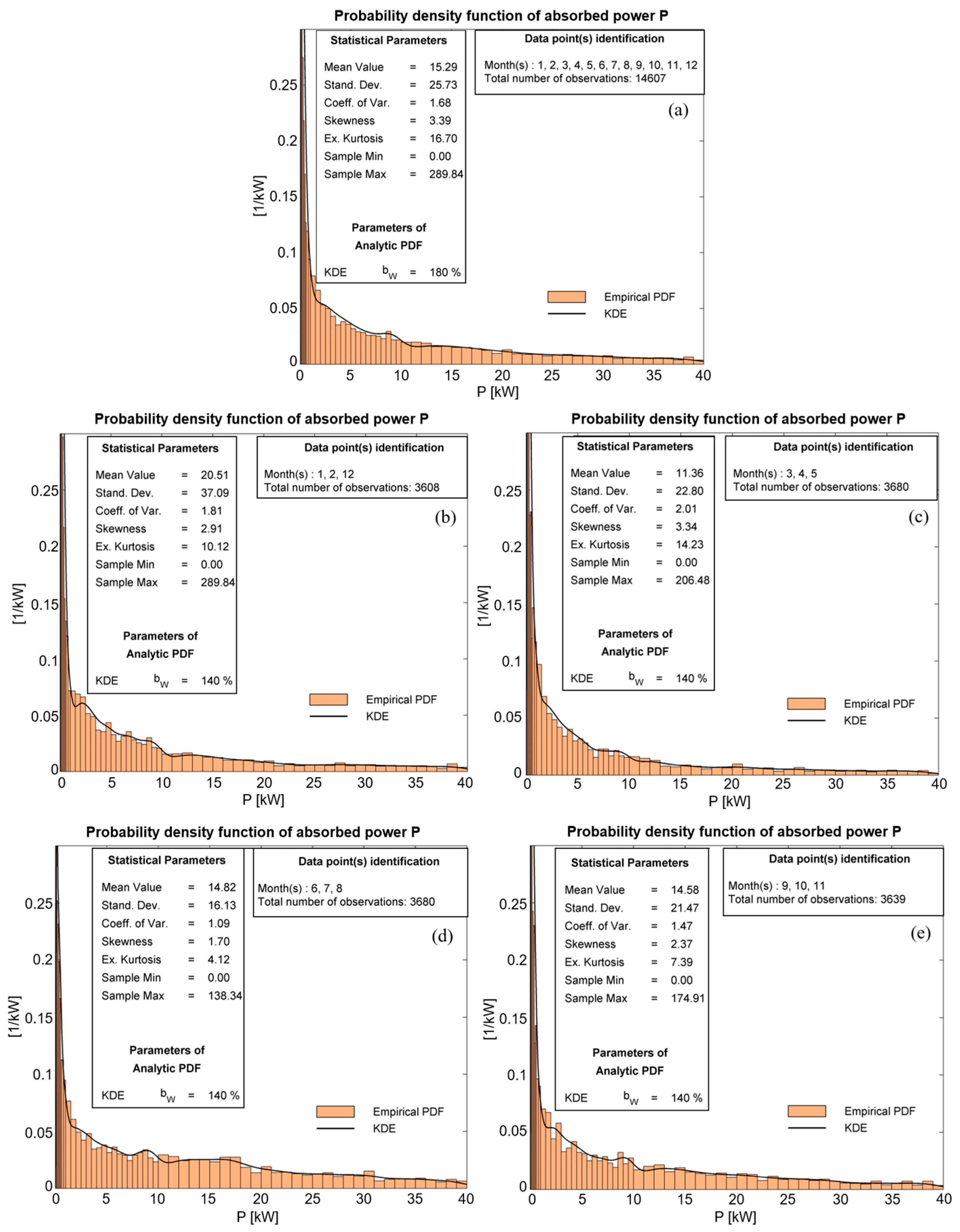
3.1. Application of the BEM-CMS Model
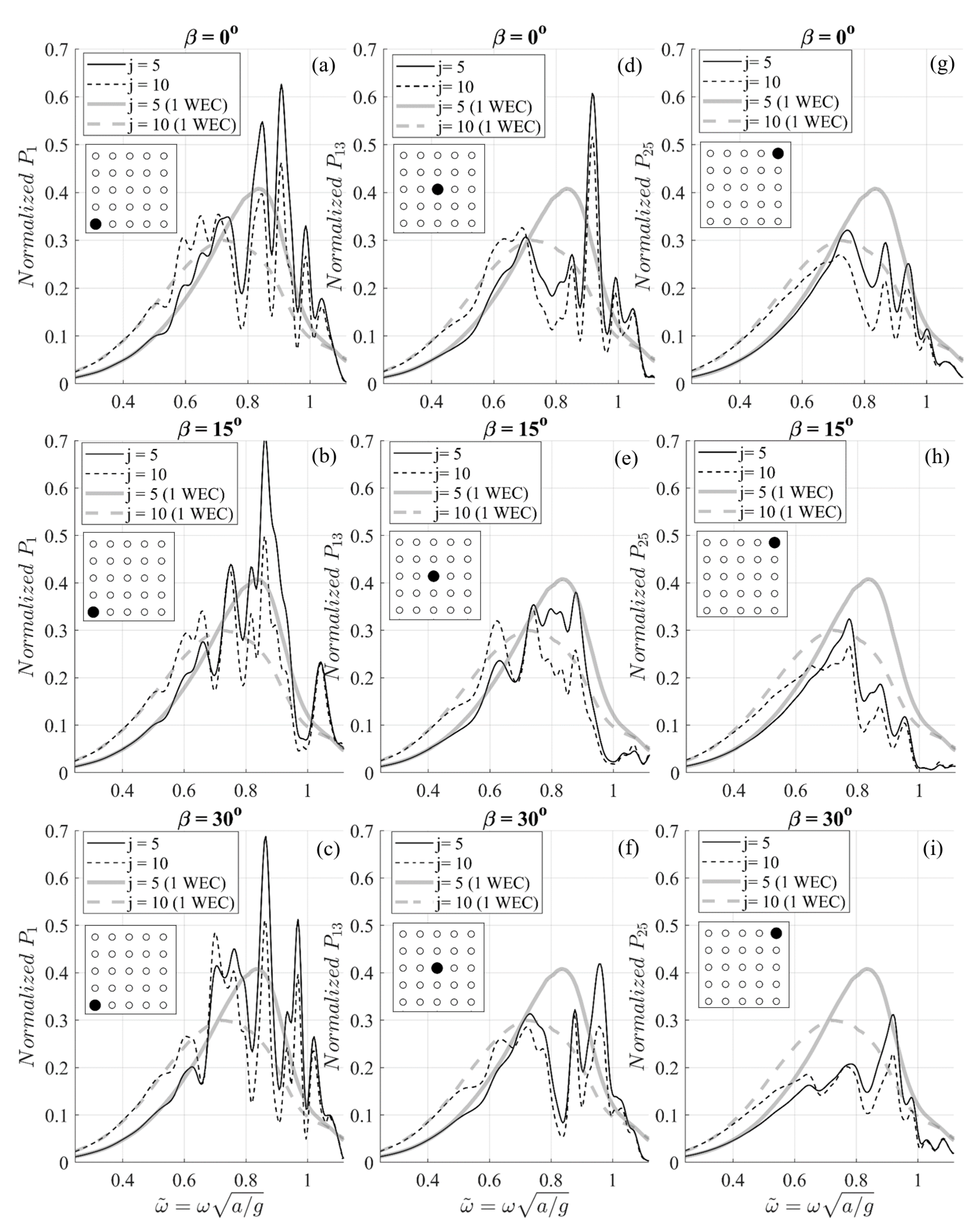
3.2. Estimation of Absorbed Power
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guo, B.; Ringwood, J.V. A review of wave energy technology from a research and commercial perspective. IET Renew. Power Gener. 2021, 15, 3065–3090. [Google Scholar] [CrossRef]
- Babarit, A. Ocean Wave Energy Conversion, 1st ed.; ISTE Press-Elsevier: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Stratigaki, V.; Troch, P.; Stallard, T.; Forehand, D.; Kofoed, J.P.; Folley, M.; Benoit, M.; Babarit, A.; Kirkegaard, J. Wave Basin Experiments with Large Wave Energy Converter Arrays to Study Interactions between the Converters and Effects on Other Users in the Sea and the Coastal Area. Energies 2014, 7, 701–734. [Google Scholar] [CrossRef]
- Belibassakis, K.; Bonovas, M.; Rusu, E. A Novel Method for Estimating Wave Energy Converter Performance in Variable Bathymetry Regions and Applications. Energies 2018, 11, 2092. [Google Scholar] [CrossRef]
- Charrayre, F.; Peyrard, C.; Benoit, M.; Babarit, A. A coupled methodology for wave body interactions at the scale of a farm of wave energy converters including irregular bathymetry. In Proceedings of the 33rd Intern Conference on Offshore Mechanics and Arctic Engineering (OMAE2014), San Francisco, CA, USA, 8 June 2014. [Google Scholar]
- Li, Y.; Yu, Y.H. A synthesis of numerical methods for modeling wave energy converter-point absorbers. Renew. Sust. Energ. Rev. 2012, 16, 4352–4364. [Google Scholar] [CrossRef]
- Bonovas, M.; Magkouris, A.; Belibassakis, K. A Modified Mild-Slope Model for the Hydrodynamic Analysis of Arrays of Heaving WECs in Variable Bathymetry Regions. Fluids 2022, 7, 183. [Google Scholar] [CrossRef]
- Gao, J.; Zang, J.; Chen, L.; Chen, Q.; Ding, H.; Liu, Y. On hydrodynamic characteristics of gap resonance between two fixed bod-ies in close proximity. Ocean Eng. 2019, 173, 28–44. [Google Scholar] [CrossRef]
- Gao, J.L.; He, Z.; Huang, X.; Liu, Q.; Zang, J.; Wang, G. Effects of free heave motion on wave resonance inside a narrow gap between two boxes under wave actions. Ocean Eng. 2021, 224, 108753. [Google Scholar] [CrossRef]
- Gao, J.; Gong, S.; He, Z.; Shi, H.; Zang, J.; Zou, T.; Bai, X. Study on Wave Loads during Steady-State Gap Resonance with Free Heave Motion of Floating Structure. J. Mar. Sci. Eng. 2023, 11, 448. [Google Scholar] [CrossRef]
- Bhinder, M.; Babarit, A.; Gentaz, L.; Ferrant, P. Effect of viscous forces on the performance of a surging wave energy converter. In Proceedings of the 22nd International Conference on Ocean, Offshore and Artic Engineering (ISOPE2012) hal-01202082f, Rhodes, Greece, 17–22 June 2012. [Google Scholar]
- Bhinder, M.; Babarit, A.; Gentaz, L.; Ferrant, P. Potential time domain model with viscous correction and CFD analysis of a generic surging floating wave energy converter. Int. J. Mar. 2015, 10, 70–96. [Google Scholar] [CrossRef]
- Verao Fernandez, G.; Balitsky, P.; Stratigaki, V.; Troch, P. Coupling Methodology for Studying the Far Field Effects of Wave Energy Converter Arrays over a Varying Bathymetry. Energies 2018, 11, 2899. [Google Scholar] [CrossRef]
- Belibassakis, K.A.; Athanassoulis, G.A.; Gerostathis, T.P. A coupled-mode model for the refraction-diffraction of linear waves over steep three-dimensional bathymetry. Appl. Ocean. Res. 2001, 23, 319–336. [Google Scholar] [CrossRef]
- Massel, S. Extended refraction-diffraction equation for surface waves. Coast. Eng. 1993, 19, 97–126. [Google Scholar] [CrossRef]
- Chamberlain, P.G.; Porter, D. The modified mild-slope equation. J. Fluid Mech. 1995, 291, 393–407. [Google Scholar] [CrossRef]
- Katz, J.; Plotkin, A. Low Speed Aerodynamics; McGraw-Hill: San Francisco, CA, USA, 2001. [Google Scholar]
- Newman, J. Distributions of sources and normal dipoles over a quadrilateral panel. J. Eng. Math. 1986, 20, 113–126. [Google Scholar] [CrossRef]
- Soukissian, T.; Gizari, N.; Chatzinaki, M. Wave Potential of the Greek Seas; WIT Transactions on Ecology and the Environment; WIT Press: Billerica, MA, USA, 2011. [Google Scholar]
- Dee, D.P.; Uppala, S.M.; Simmons, A.J.; Berrisford, P.; Poli, P.; Kobayashi, S. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- Booij, N.; Ris, R.C.; Holthuijsen, L.H. A third-generation wave model for coastal regions: 1. Model description and validation. J. Geophys. Res. Ocean 1999, 104, 7649–7666. [Google Scholar] [CrossRef]
- Massel, S.R. Ocean Surface Waves: Their Physics and Prediction. In Advanced Series on Ocean Engineering, 2nd ed.; World Scientific: Singapore, 2013; Volume 36. [Google Scholar]
- GEBCO Compilation Group (2023). GEBCO 2023 Grid. Available online: https://www.bodc.ac.uk/data/published_data_library/catalogue/10.5285/f98b053b-0cbc-6c23-e053-6c86abc0af7b/ (accessed on 20 January 2024).
- Wessel, P.; Smith, W. A global, self-consistent, hierarchical, high-resolution shoreline database. J. Geogr. Res. 1996, 101, 8741–8743. [Google Scholar] [CrossRef]
- Athanassoulis, G.A.; Belibassakis, K.A.; Gerostathis, T.P. The POSEIDON nearshore wave model and its application to the prediction of the wave conditions in the nearshore/coastal region of the Greek Seas. Glob. Atmos. Ocean Syst. 2002, 8, 201–217. [Google Scholar] [CrossRef]
- Anderlini, E.; Forehand, D.; Bannon, E.; Xiao, Q.; Abusara, M. Reactive control of a two-body point absorber using reinforcement learning. Ocean Eng. 2018, 148, 650–658. [Google Scholar] [CrossRef]
- Babarit, A. On the park effect in arrays of oscillating wave energy converters. Renew. Energy 2013, 68, 68–78. [Google Scholar] [CrossRef]
- Budal, K. Theory of absorption of wave power by a system of interacting bodies. J. Ship Res. 1977, 21, 248–253. [Google Scholar] [CrossRef]
- Jouybari, M.B.; Xing, Y. A practical design procedure for initial sizing of heaving point absorber wave energy converters. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1201, 012018. [Google Scholar] [CrossRef]
- Massel, S. Hydrodynamics of Coastal Zones; Elsevier: Amsterdam, The Netherlands, 1989. [Google Scholar]
- Göteman, M.; Giassi, M.; Engström, J.; Isberg, J. Advances and Challenges in Wave Energy Park Optimization—A Review. Front. Energy Res. 2020, 8, 26. [Google Scholar] [CrossRef]
- Falnes, J. Ocean Waves and Oscillating Systems: Linear Interactions Including Wave Energy Extraction; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Bonovas, M.; Belibassakis, K.; Rusu, E. Multi-DOF WEC Performance in Variable Bathymetry Regions Using a Hybrid 3D BEM and Optimization. Energies 2019, 12, 2108. [Google Scholar] [CrossRef]
- Tarrant, K.; Meskell, C. Investigation on parametrically excited motions of point absorbers in regular waves. Ocean Eng. 2011, 111, 67–81. [Google Scholar] [CrossRef]









Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gerostathis, T.; Magkouris, A.; Belibassakis, K. A 3D BEM-Coupled Mode Model for the Performance Analysis of Wave Energy Converter Parks in Nearshore-Coastal Regions. J. Mar. Sci. Eng. 2024, 12, 212. https://doi.org/10.3390/jmse12020212
Gerostathis T, Magkouris A, Belibassakis K. A 3D BEM-Coupled Mode Model for the Performance Analysis of Wave Energy Converter Parks in Nearshore-Coastal Regions. Journal of Marine Science and Engineering. 2024; 12(2):212. https://doi.org/10.3390/jmse12020212
Chicago/Turabian StyleGerostathis, Theodoros, Alexandros Magkouris, and Kostas Belibassakis. 2024. "A 3D BEM-Coupled Mode Model for the Performance Analysis of Wave Energy Converter Parks in Nearshore-Coastal Regions" Journal of Marine Science and Engineering 12, no. 2: 212. https://doi.org/10.3390/jmse12020212




