21 May 2003 Boumerdès Earthquake: Numerical Investigations of the Rupture Mechanism Effects on the Induced Tsunami and Its Impact in Harbors
Abstract
:1. Introduction
1.1. The Earthquake and Tsunami Event
1.2. Historical Occurrence of Tsunami Events Associated with Earthquakes at the North African Margin
1.3. Previous Modeling Studies of the 2003 Tsunami
1.4. Objectives of the Present Study
- simulation of the tsunami effects for a modified version of the fault mechanism originally proposed by Belabbès et al. (2009) [34], considering both a synchronous and asynchronous slip and justifying the need for an increased magnitude passing from seismic to tsunami modeling;
- highlighting for the analyzed tsunami case the effect of the bay/port resonance in relation to the observed periods and damages;
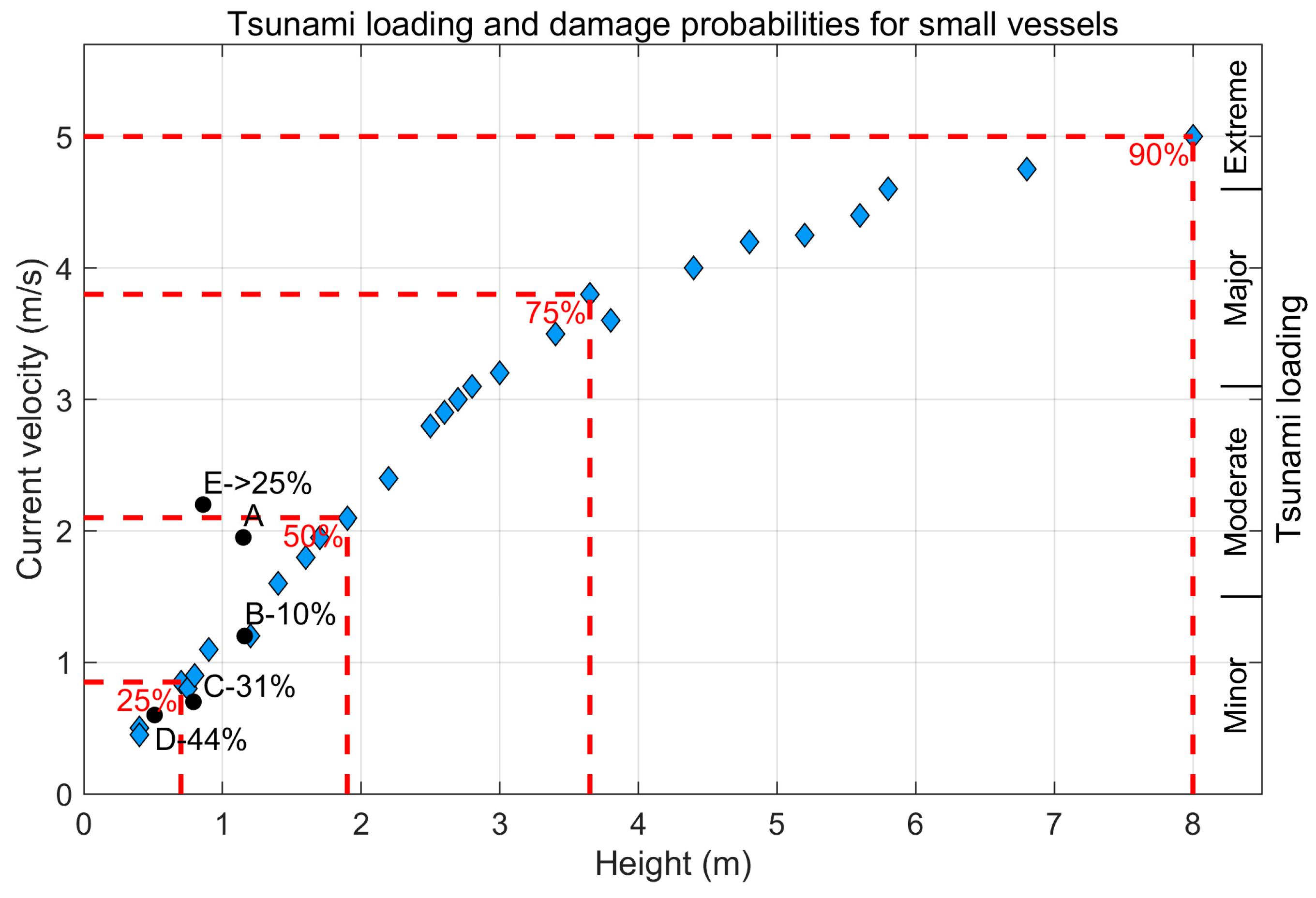
- analysis of the current intensity and tsunami height thresholds suggested in literature for small ships or boats at berth.
2. Details on the Earthquake and the Tsunami
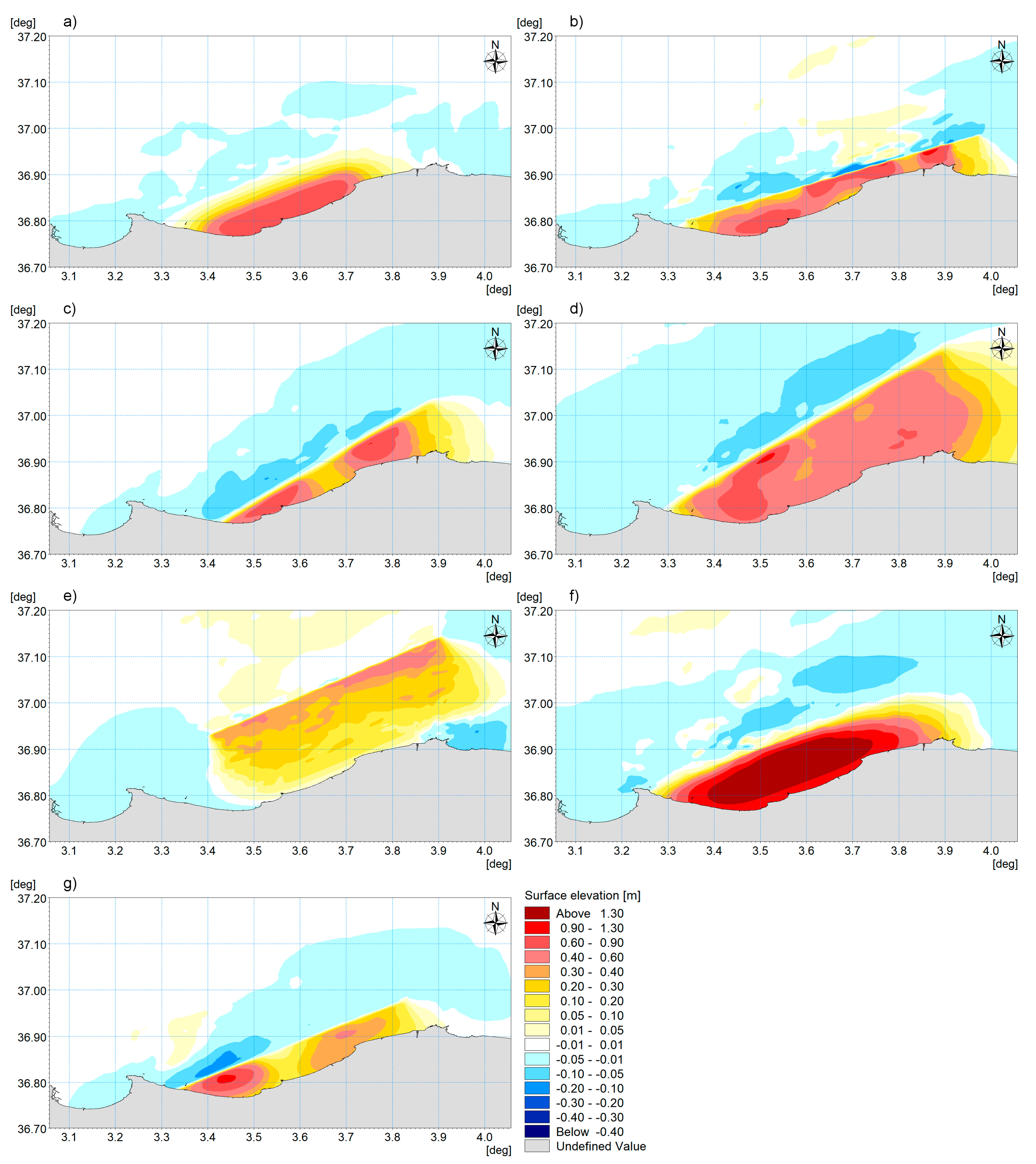
2.1. The Fault Process
2.2. The Generated Tsunami
3. Comparison between Hindcasted and Recorded Sea Levels
3.1. The Numerical Model Used
3.1.1. Seabed Displacement
3.1.2. Hydrodynamic Model
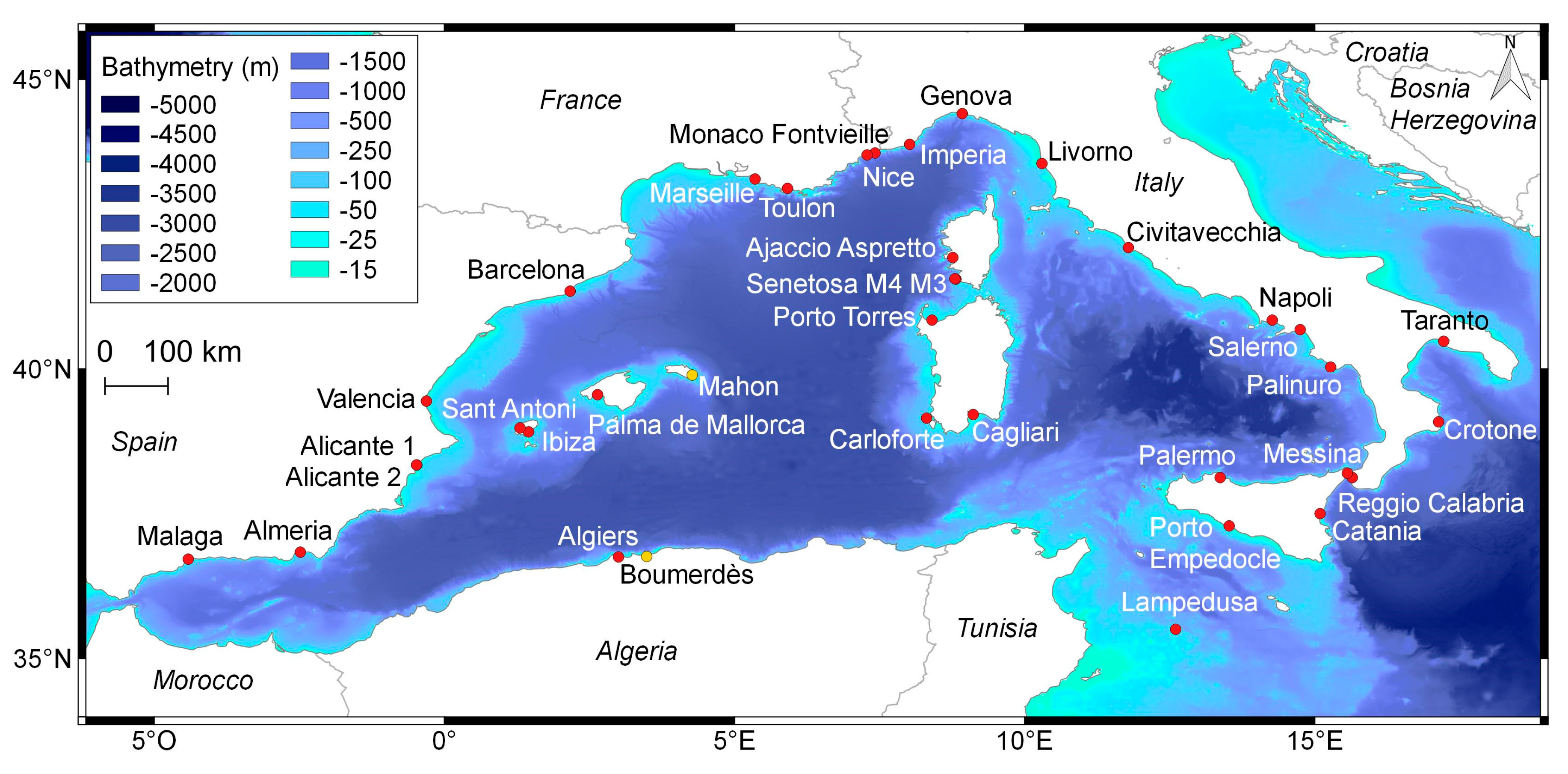
3.1.3. Computational Domain and Unstructured Grids
3.1.4. Bathymetry
3.1.5. Model Parameters
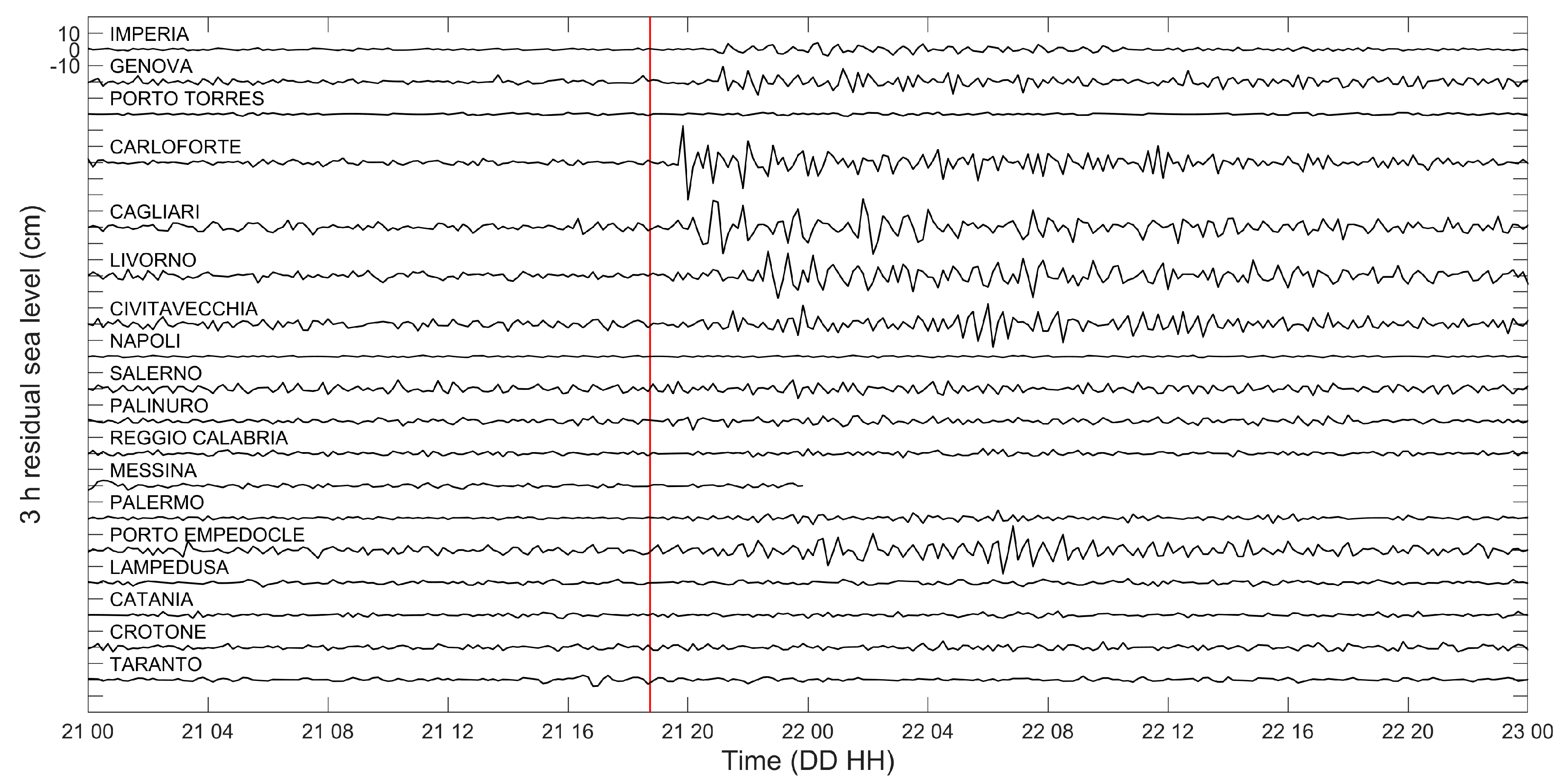
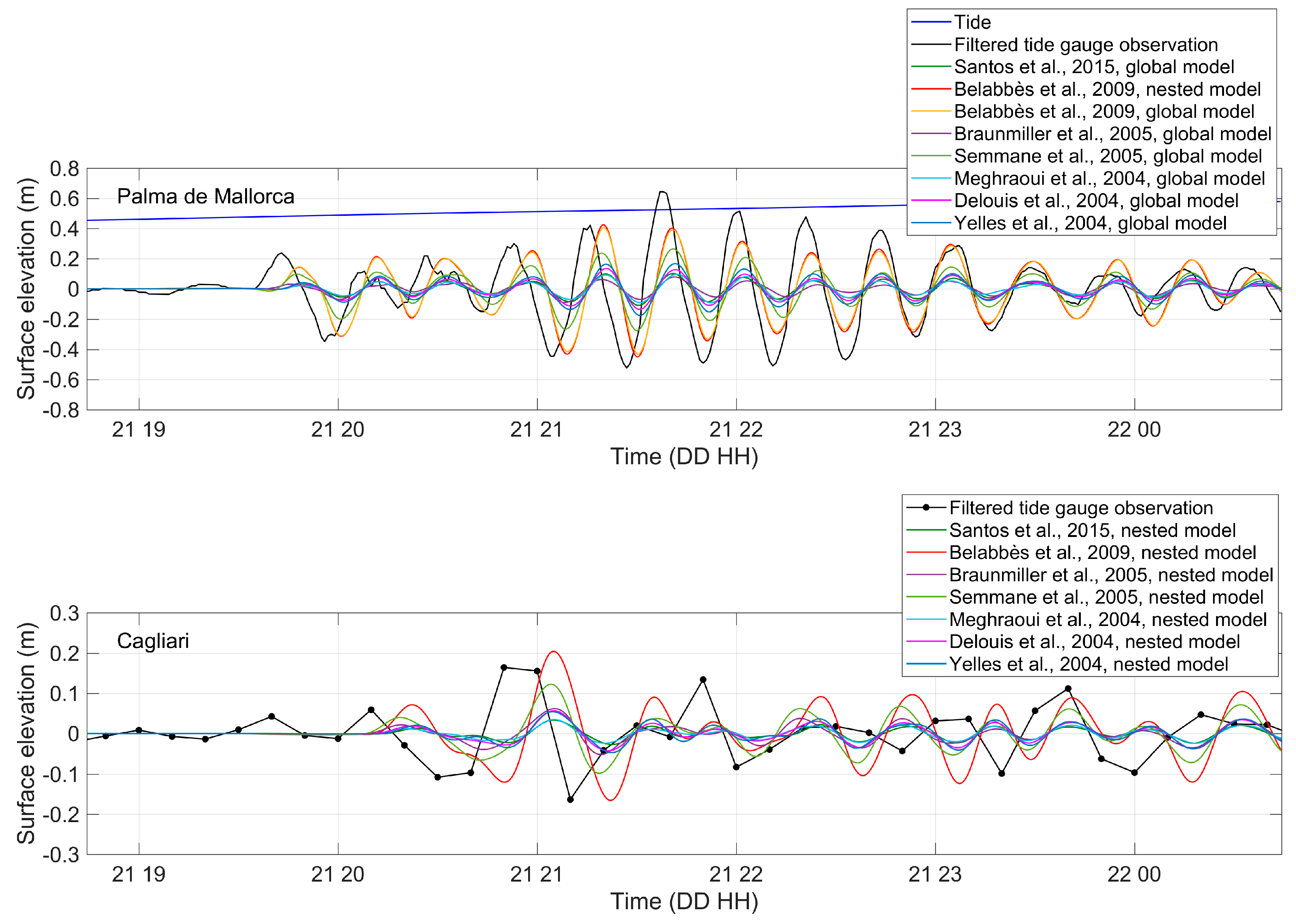
3.2. Comparison between Surface Elevation Results and Tidal Records
3.3. Comments
4. Damages in Bays and Ports Due to the 21 May 2003 Tsunami
- “the damages generated by the 2003 Western Mediterranean tsunami in the Palma harbour concentrated in the northern basin where several pleasure and sailing boats sank between Sant Magí dock and the Royal Yacht Club. The shallow water depth in this part of the harbour eased the sinking of the boats, as they were quickly moved up and down by the oscillations easily hitting the bottom and breaking” [31];
- the boats moored along the Paseo Maritimo close to La Riera hit the bottom due to the rapid lowering of the sea level [105];
- collisions among vessels occurred along the Paseo Maritimo [103];
- “in Palma, the first sea movement was an ingression and the Paseo Maritimo street was flooded” [106];
- “a second highly impacted area was the Espigón Consigna, in the commercial quays, where the tsunami waves took off an oil container and other objects” [31].
5. Relating Tsunami Hydrodynamic Features to Reported Damage
5.1. Tsunami Loading Factors
5.2. Port of Palma de Mallorca
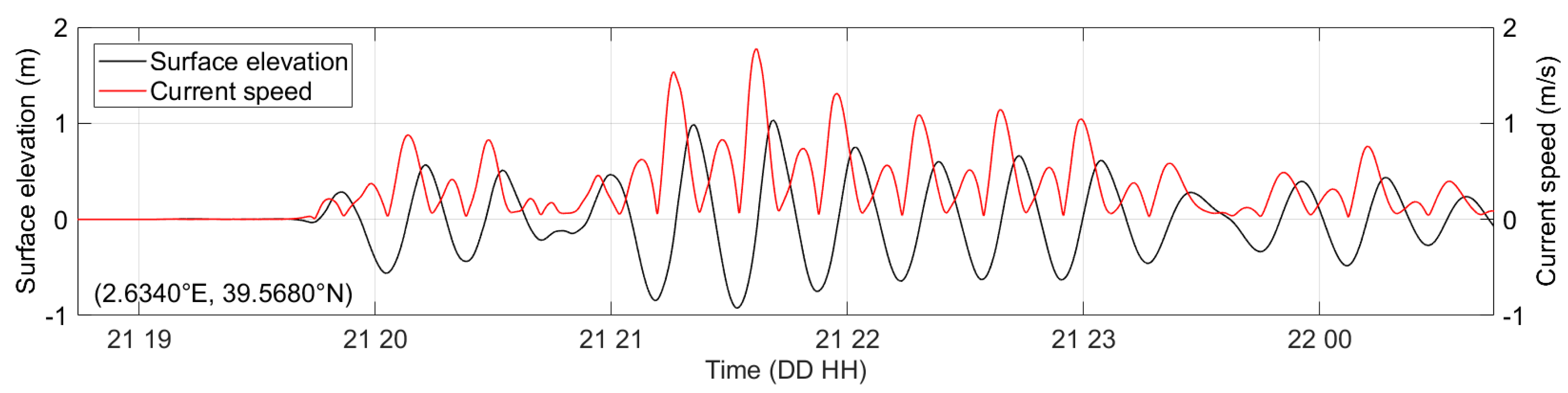
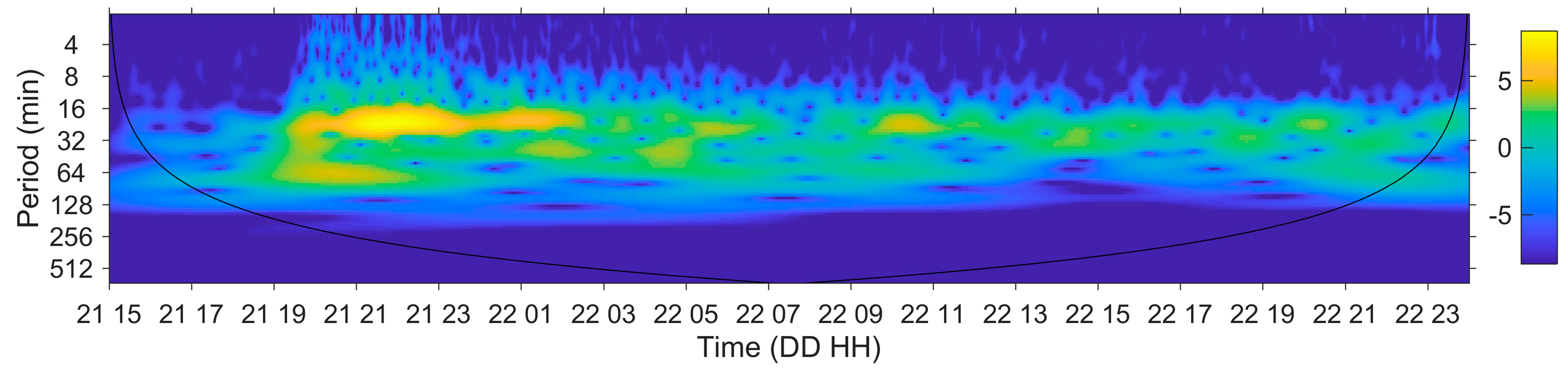
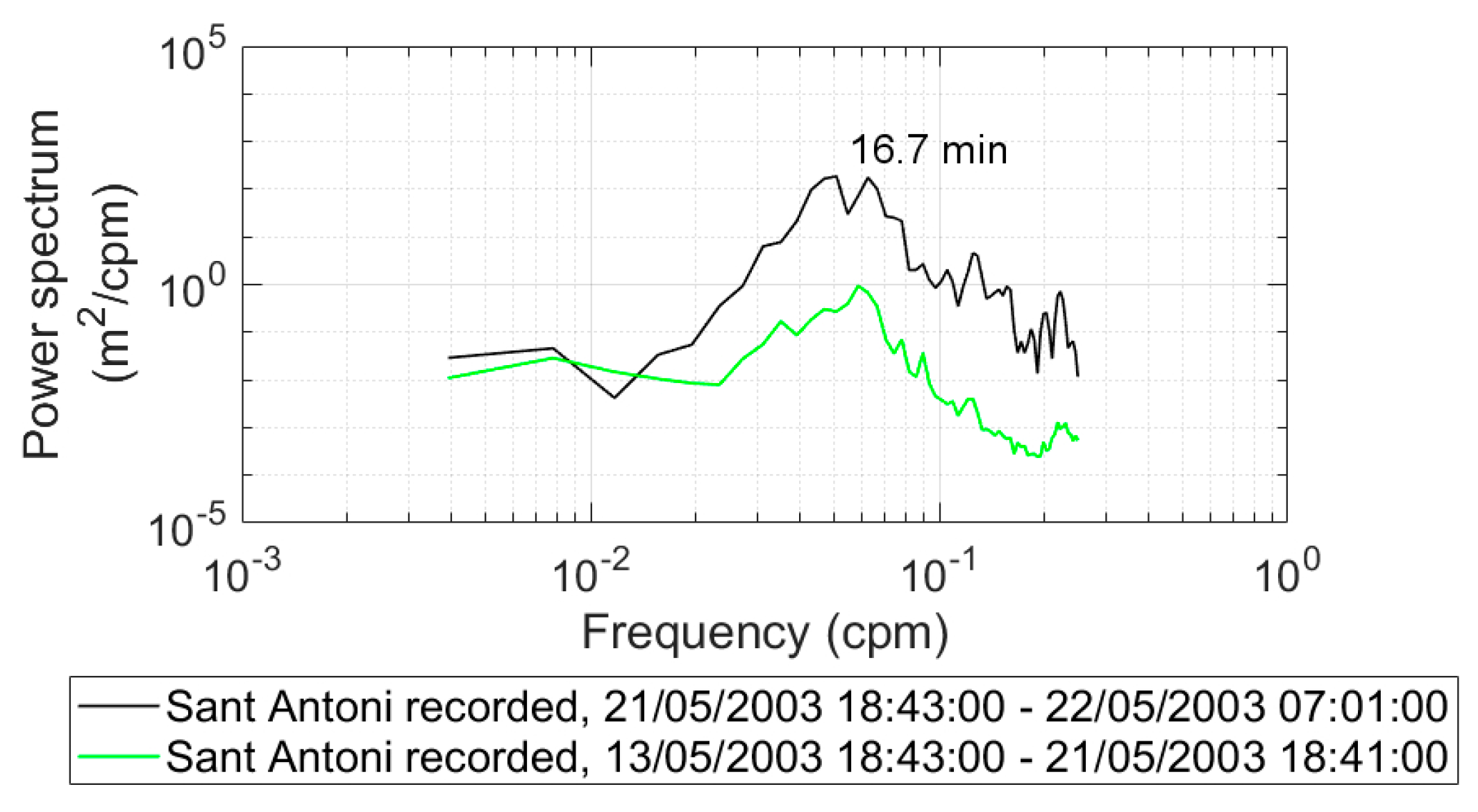
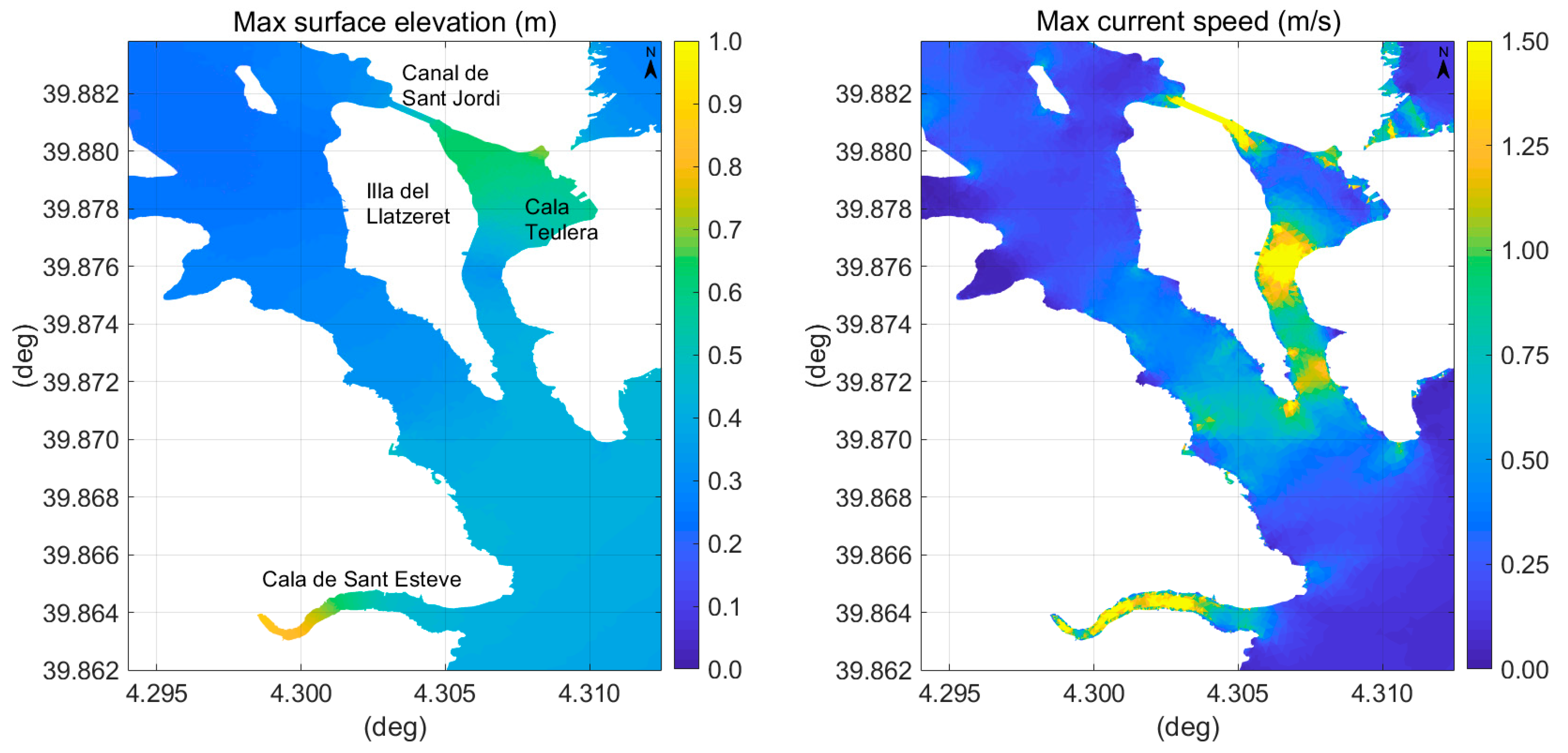
5.3. Port of Sant Antoni de Portmany
5.4. Port of Mahon
5.5. Damage Mechanisms and Thresholds
- mooring breakage and ship moved around hitting other ships or structures by (1) strong currents, (2) large water level oscillation with short and tight mooring;
- ship lowered or raised by water level (1) hitting the bottom with keel or rudder, (2) raised and transported over the wharf, (3) raised against a fixed pier or a bridge and then sunk by still rising sea level;
- ship caught in a breaker capsizes and sinks.
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dilley, M.; Chen, R.S.; Deichmann, U.; Lerner-Lam, A.L.; Arnold, M.; Agwe, J.; Buys, P.; Kjekstad, O.; Lyon, B.; Yetman, G. Natural Disaster Hotspots: A Global Risk Analysis. Disaster Risk Management Series No. 5-34423; The World Bank: Washington, DC, USA, 2005. [Google Scholar]
- International Tsunami Information Center. Available online: http://itic.ioc-unesco.org/index.php (accessed on 22 July 2020).
- NOAA National Centers for Environmental Information. National Geophysical Data Center/World Data Service: NCEI/WDS Global Historical Tsunami Database. Available online: https://www.ngdc.noaa.gov/hazard/tsu_db.shtml (accessed on 24 July 2020).
- Maramai, A.; Brizuela, B.; Graziani, L. The Euro-Mediterranean Tsunami Catalogue. Ann. Geophys. 2014, 57, S0435. [Google Scholar] [CrossRef]
- Goeldner-Gianella, L.; Grancher, D.; Robertsen, Ø.; Anselme, B.; Brunstein, D.; Lavigne, F. Perception of the risk of tsunami in a context of high-level risk assessment and management: The case of the fjord Lyngen in Norway. Geoenviron. Disasters 2017, 4, 227. [Google Scholar] [CrossRef] [Green Version]
- Cerase, A.; Crescimbene, M.; La Longa, F.; Amato, A. Tsunami risk perception in southern Italy: First evidence from a sample survey. Nat. Hazards Earth Syst. Sci. 2019, 19, 2887–2904. [Google Scholar] [CrossRef] [Green Version]
- Giles, J.; Marris, E. Indonesian tsunami-monitoring system lacked basic equipment. Nature 2004. [Google Scholar] [CrossRef]
- Melgar, D.; Bock, Y. Near-field tsunami models with rapid earthquake source inversions from land- and ocean-based observations: The potential for forecast and warning. J. Geophys. Res. Solid Earth 2013, 118, 5939–5955. [Google Scholar] [CrossRef]
- Lynett, P.J.; Borrero, J.; Son, S.; Wilson, R.; Miller, K. Assessment of the tsunami-induced current hazard. Geophys. Res. Lett. 2014, 41, 2048–2055. [Google Scholar] [CrossRef]
- Yelles-Chaouche, A.K.; Djellit, H.; Hamdache, M. The Boumerdes -Algiers (Algeria) Earthquake of May 21st, 2003 (Mw = 6.8). In CSEM/EMSC Newsletter N; 20 September 2003; pp. 3–5. Available online: https://www.emsc-csem.org/Files/docs/data/newsletters/newsletter_20.pdf (accessed on 26 July 2020).
- Laouami, N.; Slimani, A.; Bouhadad, Y.; Nour, A.; Larbes, S. Analysis of Strong Ground Motions Recorded during the 21st May, 2003 Boumerdes, Algeria, Earthquake. In CSEM/EMSC Newsletter N; 20 September 2003; pp. 5–7. Available online: https://www.emsc-csem.org/Files/docs/data/newsletters/newsletter_20.pdf (accessed on 26 July 2020).
- Laouami, N.; Slimani, A.; Bouhadad, Y.; Chatelain, J.-L.; Nour, A. Evidence for fault-related directionality and localized site effects from strong motion recordings of the 2003 Boumerdes (Algeria) earthquake: Consequences on damage distribution and the Algerian seismic code. Soil Dyn. Earthq. Eng. 2006, 26, 991–1003. [Google Scholar] [CrossRef]
- EERI, Earthquake Engineering Research Institute. The Boumerdes, Algeria, Earthquake of May 21, 2003. EERI Learning from Earthquakes, Reconnaissance Report; EERI Publication Number 2003-04; EERI: Oakland, CA, USA, 2003; Available online: https://www.eeri.org/site/images/lfe/pdf/algeria_20030521.pdf (accessed on 27 July 2020).
- Meghraoui, M.; Maouche, S.; Chemaa, B.; Cakir, Z.; Aoudia, A.; Harbi, A.; Alasset, P.-J.; Ayadi, A.; Bouhadad, Y.; Benhamouda, F. Coastal uplift and thrust faulting associated with the Mw = 6.8 Zemmouri (Algeria) earthquake of 21 May, 2003. Geophys. Res. Lett. 2004, 31, L19605. [Google Scholar] [CrossRef] [Green Version]
- Papadopoulos, G.A.; Gràcia, E.; Urgeles, R.; Sallares, V.; De Martini, P.M.; Pantosti, D.; González, M.; Yalciner, A.C.; Mascle, J.; Sakellariou, D.; et al. Historical and pre-historical tsunamis in the Mediterranean and its connected seas: Geological signatures, generation mechanisms and coastal impacts. Mar. Geol. 2014, 354, 81–109. [Google Scholar] [CrossRef]
- Peláez, J.A.; Chourak, M.; Tadili, B.A.; Aït Brahim, L.; Hamdache, M.; López Casado, C.; Martínez Solares, J.M. A catalog of main Moroccan earthquakes from 1045 to 2005. Seism. Res. Lett. 2007, 78, 614–621. [Google Scholar] [CrossRef]
- Hamdache, M.; Peláez, J.A.; Talbi, A.; López Casado, C. A unified catalog of main earthquakes for Northern Algeria from A.D. 856 to 2008. Seism. Res. Lett. 2010, 81, 732–739. [Google Scholar] [CrossRef] [Green Version]
- Soloviev, S.L.; Solovieva, O.N.; Go, C.N.; Kim, K.S.; Shchetnikov, N.A. Tsunamis in the Mediterranean Sea 2000 B.C.-2000 A.D. In Advances in Natural and Technological Hazards Research, Volume 13; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2000. [Google Scholar]
- Roger, J.; Hébert, H. The 1856 Djijelli (Algeria) earthquake and tsunami: Source parameters and implications for tsunami hazard in the Balearic Islands. Nat. Hazards Earth Syst. Sci. 2008, 8, 721–731. [Google Scholar] [CrossRef] [Green Version]
- Harbi, A.; Meghraoui, M.; Maouche, S. The Djidjelli (Algeria) earthquakes of 21 and 22 August 1856 (I0 VIII, IX) and related tsunami effects Revisited. J. Seism. 2011, 15, 105–129. [Google Scholar] [CrossRef]
- Maouche, S.; Harbi, A.; Meghraoui, M. Attenuation of intensity for the Zemmouri earthquake of 21 May 2003 (Mw 6.8): Insights for the seismic hazard and historical earthquake sources in Northern Algeria. In Historical Seismology. Interdisciplinary Studies of Past and Recent Earthquakes. Modern Approaches in Solid Earth Sciences; Fréchet, J., Meghraoui, M., Stucchi, M., Eds.; Springer: Dordrecht, The Netherlands, 2008; Volume 2, pp. 327–350. [Google Scholar] [CrossRef]
- Bezzeghoud, M.; Dimitro, D.; Ruegg, J.C.; Lammali, K. Faulting mechanism of the El Asnam (Algeria) 1954 and 1980 earthquakes from modelling of vertical movements. Tectonophysics 1995, 249, 249–266. [Google Scholar] [CrossRef]
- Hébert, H.; Alasset, P.-J. The tsunami triggered by the 21 May 2003 Algiers earthquake. In CSEM/EMSC Newsletter N.; 20 September 2003; pp. 10–12. Available online: https://www.emsc-csem.org/Files/docs/data/newsletters/newsletter_20.pdf (accessed on 26 July 2020).
- Wang, X.; Liu, P.L.-F. A numerical investigation of Boumerdes-Zemmouri (Algeria) earthquake and tsunami. Comput. Model. Eng. Sci. 2005, 10, 171–184. [Google Scholar] [CrossRef]
- Borrero, J.C. Preliminary Simulations of the Algerian Tsunami of 21 May, 2003 in the Balearic Islands; University of Southern California: Los Angeles, CA, USA, 2003. [Google Scholar]
- Alasset, P.-J.; Hébert, H.; Maouche, S.; Calbini, V.; Meghraoui, M. The tsunami induced by the 2003 Zemmouri earthquake (MW = 6.9, Algeria): Modelling and results. Geophys. J. Int. 2006, 166, 213–226. [Google Scholar] [CrossRef] [Green Version]
- Delouis, B.; Vallée, M.; Meghraoui, M.; Calais, E.; Maouche, S.; Lammali, K.; Mahsas, A.; Briole, P.; Benhamouda, F.; Yelles, K. Slip distribution of the 2003 Boumerdes-Zemmouri earthquake, Algeria, from teleseismic, GPS, and coastal uplift data. Geophys. Res. Lett. 2004, 31, L18607. [Google Scholar] [CrossRef] [Green Version]
- Yelles, K.; Lammali, K.; Mahsas, A.; Calais, E.; Briole, P. Coseismic deformation of the May 21st, 2003, Mw = 6.8 Boumerdes earthquake, Algeria, from GPS measurements. Geophys. Res. Lett. 2004, 31, L13610. [Google Scholar] [CrossRef]
- Semmane, F.; Campillo, M.; Cotton, F. Fault location and source process of the Boumerdes, Algeria, earthquake inferred from geodetic and strong motion data. Geophys. Res. Lett. 2005, 32, L01305. [Google Scholar] [CrossRef] [Green Version]
- Sahal, A.; Roger, J.; Allgeyer, S.; Lemaire, B.; Hébert, H.; Schindelé, F.; Lavigne, F. The tsunami triggered by the 21 May 2003 Boumerdès-Zemmouri (Algeria) earthquake: Field investigations on the French Mediterranean coast and tsunami modelling. Nat. Hazards Earth Syst. Sci. 2009, 9, 1823–1834. [Google Scholar] [CrossRef]
- Vela, J.; Pérez, B.; González, M.; Otero, L.; Olabarrieta, M.; Canals, M.; Casamor, J.L. Tsunami resonance in the Palma de Majorca bay and harbour induced by the 2003 Boumerdes-Zemmouri Algerian earthquake (Western Mediterranean). In Proceedings of the 32nd International Conference on Coastal Engineering 2010, Shanghai, China, 30 June–5 July 2010; McKee Smith, J., Lynett, P., Eds.; ASCE/COPRI Coastal Engineering Research Council: Reston, VA, USA, 2011. currents.7. [Google Scholar] [CrossRef] [Green Version]
- Vela, J.; Pérez, B.; González, M.; Otero, L.; Olabarrieta, M.; Canals, M.; Casamor, J.L. Tsunami resonance in Palma bay and harbor, Majorca Island, as induced by the 2003 Western Mediterranean earthquake. J. Geol. 2014, 122, 165–182. [Google Scholar] [CrossRef] [Green Version]
- Samaras, A.G.; Karambas, T.V.; Archetti, R. Simulation of tsunami generation, propagation and coastal inundation in the Eastern Mediterranean. Ocean Sci. 2015, 11, 643–655. [Google Scholar] [CrossRef] [Green Version]
- Belabbès, S.; Wicks, C.; Çakir, Z.; Meghraoui, M. Rupture parameters of the 2003 Zemmouri (Mw 6.8), Algeria, earthquake from joint inversion of interferometric synthetic aperture radar, coastal uplift, and GPS. J. Geophys. Res. Solid Earth 2009, 114, B03406. [Google Scholar] [CrossRef]
- Nocquet, J.-M.; Calais, E. Geodetic measurements of crustal deformation in the Western Mediterranean and Europe. Pure Appl. Geophys. 2004, 161, 661–681. [Google Scholar] [CrossRef]
- Serpelloni, E.; Vannucci, G.; Pondrelli, S.; Argnani, A.; Casula, G.; Anzidei, M.; Baldi, P.; Gasperini, P. Kinematics of the Western Africa-Eurasia plate boundary from focal mechanisms and GPS data. Geophys. J. Int. 2007, 169, 1180–1200. [Google Scholar] [CrossRef]
- Bounif, A.; Dorbath, C.; Ayadi, A.; Meghraoui, M.; Beldjoudi, H.; Laouami, N.; Frogneux, M.; Slimani, A.; Alasset, P.J.; Kharroubi, A.; et al. The 21 May 2003 Zemmouri (Algeria) earthquake Mw 6.8: Relocation and aftershock sequence analysis. Geophys. Res. Lett. 2004, 31, L19606. [Google Scholar] [CrossRef]
- Bourenane, H.; Bouhadad, Y.; Tas, M. Liquefaction hazard mapping in the city of Boumerdès, Northern Algeria. Bull. Eng. Geol. Environ. 2018, 77, 1473–1489. [Google Scholar] [CrossRef] [Green Version]
- Yagi, Y. Preliminary Results of Rupture Process. Available online: http://iisee.kenken.go.jp/staff/yagi/eq/algeria20030521/algeria2003521.html (accessed on 3 December 2016).
- Yagi, Y. Source process of large and significant earthquakes in 2003. Bull. Int. Inst. Seismol. Earthq. Eng. 2003, 37, 145–153. [Google Scholar]
- Delouis, B.; Vallée, M. The 2003 Boumerdes (Algeria) earthquake: Source process from teleseismic data. In CSEM/EMSC Newsletter N; 20 September 2003; pp. 8–9. Available online: https://www.emsc-csem.org/Files/docs/data/newsletters/newsletter_20.pdf (accessed on 26 July 2020).
- Bezzeghoud, M.; Caldeira, B.; Borges, J.F.; Beldjoudi, H.; Buforn, E.; Maouche, S.; Ousadou, F.; Kherroubi, A.; Harbi, A.; Ayadi, A. The Zemmouri-Boumerdes (Algeria) Earthquake of May 21st, 2003, Mw=6.8: Source Parameters and Rupture Propagation Study from Teleseismic Data; European Seismological Commission—XXIX General Assembly, University & GFZ: Potsdam, Germany, 2004. [Google Scholar]
- Braunmiller, J.; Bernardi, F. The 2003 Boumerdes, Algeria earthquake: Regional moment tensor analysis. Geophys. Res. Lett. 2005, 32, L06305. [Google Scholar] [CrossRef] [Green Version]
- Santos, R.; Caldeira, B.; Bezzeghoud, M.; Borges, J.F. The rupture process and location of the 2003 Zemmouri-Boumerdes earthquake (Mw 6.8) inferred from seismic and geodetic data. Pure Appl. Geophys. 2015, 172, 2421–2434. [Google Scholar] [CrossRef] [Green Version]
- Bossu, R.; Godey, S.; Mazet-Roux, G. EMSC actions concerning the Boumerdes-Zemmouri event. In CSEM/EMSC Newsletter N; 20 September 2003; p. 2. Available online: https://www.emsc-csem.org/Files/docs/data/newsletters/newsletter_20.pdf (accessed on 26 July 2020).
- Bernardi, F. Earthquake Source Parameters in the Alpine-Mediterranean Region from Surface Wave Analysis. Ph.D. Thesis, ETH Nr. 15652. Swiss Federal Institute of Technology, Zürich, Switzerland, 2004. [Google Scholar] [CrossRef]
- Déverchère, J.; Yelles, K.; Domzig, A.; Mercier de Lépinay, B.; Bouillin, J.-P.; Gaullier, V.; Bracène, R.; Calais, E.; Savoye, B.; Kherroubi, A.; et al. Active thrust faulting offshore Boumerdes, Algeria, and its relations to the 2003 Mw 6.9 earthquake. Geophys. Res. Lett. 2005, 32, L04311. [Google Scholar] [CrossRef] [Green Version]
- Déverchère, J.; Mercier de Lépinay, B.; Cattaneo, A.; Strzerzynski, P.; Calais, E.; Domzig, A.; Bracene, R. Comment on “Zemmouri earthquake rupture zone (Mw 6.8, Algeria): Aftershocks sequence relocation and 3D velocity model” by A. Ayadi et al. J. Geophys. Res. Solid Earth 2010, 115, B04320. [Google Scholar] [CrossRef] [Green Version]
- Kherroubi, A.; Yelles-Chaouche, A.; Koulakov, I.; Déverchère, J.; Beldjoudi, H.; Haned, A.; Semmane, F.; Aidi, C. Full aftershock sequence of the Mw 6.9 2003 Boumerdes earthquake, Algeria: Space-time distribution, local tomography and seismotectonic implications. Pure Appl. Geophys. 2017, 174, 2495–2521. [Google Scholar] [CrossRef]
- IRIS DMC. Data Services Products: EQEnergy Earthquake Energy & Rupture Duration; IRIS: Washington, DC, USA, 2013. [Google Scholar] [CrossRef]
- Convers, J.A.; Newman, A.V. Global evaluation of large earthquake energy from 1997 through mid-2010. J. Geophys. Res. Solid Earth 2011, 116, B08304. [Google Scholar] [CrossRef] [Green Version]
- Heezen, B.C.; Ewing, M. Orléansville earthquake and turbidity currents. AAPG Bull. 1955, 39, 2505–2514. [Google Scholar] [CrossRef]
- El-Robrini, M.; Gennesseaux, M.; Mauffret, A. Consequences of the El-Asnam earthquakes: Turbidity currents and slumps on the Algerian margin (Western Mediterranean). Geo-Mar. Lett. 1985, 5, 171–176. [Google Scholar] [CrossRef]
- Cattaneo, A.; Babonneau, N.; Ratzov, G.; Dan-Unterseh, G.; Yelles, K.; Bracène, R.; Mercier de Lépinay, B.; Boudiaf, A.; Déverchère, J. Searching for the seafloor signature of the 21 May 2003 Boumerdès earthquake offshore central Algeria. Nat. Hazards Earth Syst. Sci. 2012, 12, 2159–2172. [Google Scholar] [CrossRef] [Green Version]
- Bryant, E. Tsunami: The Underrated Hazard, 2nd ed.; Springer, Praxis Publishing Ltd.: Chichester, UK, 2008. [Google Scholar]
- Harbi, A.; Maouche, S.; Ousadou, F.; Rouchiche, Y.; Yelles-Chaouche, A.; Merahi, M.; Heddar, A.; Nouar, O.; Kherroubi, A.; Beldjoudi, H.; et al. Macroseismic Study of the Zemmouri Earthquake of 21 May 2003 (Mw 6.8, Algeria). Earthq. Spectra 2007, 23, 315–332. [Google Scholar] [CrossRef]
- Edwards, C.L. Zemmouri, Algeria, Mw 6.8 Earthquake of May 21, 2003. In Technical Council on Lifeline Earthquake Engineering, Monograph No. 27; Edwards, C.L., Ed.; The American Society of Civil Engineers: Reston, VA, USA, 2004. [Google Scholar]
- Vich, M.-d.-M.; Monserrat, S. Source spectrum for the Algerian tsunami of 21 May 2003 estimated from coastal tide gauge data. Geophys. Res. Lett. 2009, 36, L20610. [Google Scholar] [CrossRef] [Green Version]
- Heidarzadeh, M.; Satake, K. The 21 May 2003 tsunami in the Western Mediterranean Sea: Statistical and wavelet analyses. Pure Appl. Geophys. 2013, 170, 1449–1462. [Google Scholar] [CrossRef]
- Toda, S.; Stein, R.S.; Sevilgen, V.; Lin, J. Coulomb 3.3 Graphic-Rich Deformation and Stress-Change Software for Earthquake, Tectonic, and Volcano Research and Teaching-User Guide; US Geological Survey Open-File Report 2011-1060; 2011. Available online: https://pubs.usgs.gov/of/2011/1060/ (accessed on 29 July 2020).
- Okada, Y. Internal deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Am. 1992, 82, 1018–1040. [Google Scholar]
- Nosov, M.A.; Bolshakova, A.V.; Kolesov, S.V. Displaced water volume, potential energy of initial elevation, and tsunami intensity: Analysis of recent tsunami events. Pure Appl. Geophys. 2014, 171, 3515–3525. [Google Scholar] [CrossRef]
- Levin, B.W.; Nosov, M.A. Physics of Tsunamis, 2nd ed; Springer International Publishing: Cham, Switzerland, 2016. [Google Scholar] [CrossRef]
- Kajiura, K. The leading wave of a tsunami. Bull. Earthq. Res. Ins. Univ. Tokyo 1963, 41, 535–571. [Google Scholar]
- Saito, T.; Furumura, T. Three-dimensional tsunami generation simulation due to sea-bottom deformation and its interpretation based on the linear theory. Geophys. J. Int. 2009, 178, 877–888. [Google Scholar] [CrossRef]
- Lynett, P.J.; Borrero, J.C.; Weiss, R.; Son, S.; Greer, D.; Renteria, W. Observations and modeling of tsunami-induced currents in ports and harbors. Earth Planet. Sci. Lett. 2012, 327–328, 68–74. [Google Scholar] [CrossRef]
- Lynett, P.J.; Gately, K.; Wilson, R.; Montoya, L.; Arcas, D.; Aytore, B.; Bai, Y.; Bricker, J.D.; Castro, M.J.; Cheung, K.F.; et al. Inter-model analysis of tsunami-induced coastal currents. Ocean Model. 2017, 114, 14–32. [Google Scholar] [CrossRef]
- Arcos, M.E.M.; LeVeque, R.J. Validating velocities in the GeoClaw tsunami model using observations near Hawaii from the 2011 Tohoku tsunami. Pure Appl. Geophys. 2015, 172, 849–867. [Google Scholar] [CrossRef] [Green Version]
- Barberopoulou, A.; Legg, M.R.; Uslu, B.; Synolakis, C.E. Reassessing the tsunami risk in major ports and harbors of California I: San Diego. Nat. Hazards 2011, 58, 479–496. [Google Scholar] [CrossRef]
- Bolaños, R.; Sørensen, J.V.T.; Benetazzo, A.; Carniel, S.; Sclavo, M. Modelling ocean currents in the northern Adriatic Sea. Cont. Shelf Res. 2014, 87, 54–72. [Google Scholar] [CrossRef]
- Haigh, I.D.; Wijeratne, E.M.S.; MacPherson, L.R.; Pattiaratchi, C.B.; Mason, M.S.; Crompton, R.P.; George, S. Estimating present day extreme water level exceedance probabilities around the coastline of Australia: Tides, extra-tropical storm surges and mean sea level. Clim. Dyn. 2014, 42, 121–138. [Google Scholar] [CrossRef]
- Masina, M.; Archetti, R.; Besio, G.; Lamberti, A. Tsunami taxonomy and detection from recent Mediterranean tide gauge data. Coast. Eng. 2017, 127, 145–169. [Google Scholar] [CrossRef]
- Sarker, M.A. Numerical modelling of tsunami in the Makran Subduction Zone-A case study on the 1945 event. J. Oper. Oceanogr. 2019, 12, S212–S229. [Google Scholar] [CrossRef]
- Leschka, S.; Kongko, W.; Larsen, O. On the influence of nearshore bathymetry data quality on tsunami runup modelling, part II: Modelling. In Proceedings of the 5th International Conference on Asian and Pacific Coasts 2009; Tan, S.K., Huang, Z., Eds.; World Scientific Pub Co Pte Ltd.: Singapore, 2009; pp. 157–163. [Google Scholar] [CrossRef]
- Gayer, G.; Leschka, S.; Nöhren, I.; Larsen, O.; Günther, H. Tsunami inundation modelling based on detailed roughness maps of densely populated areas. Nat. Hazards Earth Syst. Sci. 2010, 10, 1679–1687. [Google Scholar] [CrossRef] [Green Version]
- Kaiser, G.; Scheele, L.; Kortenhaus, A.; Løvholt, F.; Römer, H.; Leschka, S. The influence of land cover roughness on the results of high resolution tsunami inundation modeling. Nat. Hazards Earth Syst. Sci. 2011, 11, 2521–2540. [Google Scholar] [CrossRef] [Green Version]
- Payande, A.R.; Niksokhan, M.H.; Naserian, H. Tsunami hazard assessment of Chabahar bay related to megathrust seismogenic potential of the Makran subduction zone. Nat. Hazards 2015, 76, 161–176. [Google Scholar] [CrossRef]
- Boswood, P.K. Tsunami Modelling along the East Queensland Coast, Report 1: Regional Modelling; Department of Science, Information Technology, Innovation and the Arts, Queensland Government: Brisbane, Queensland, Australia, 2013. Available online: https://publications.qld.gov.au/dataset/tsunami-modelling-east-queensland-coast (accessed on 26 July 2020).
- Barua, D.K.; Allyn, N.F.; Quick, M.C. Modeling tsunami and resonance response of Alberni Inlet, British Columbia. In Proceedings of the 30th International Conference on Coastal Engineering 2006, San Diego, CA, USA, 3–8 September 2006; McKee Smith, J., Ed.; World Scientific Pub Co Pte Ltd.: Singapore, 2007; pp. 1590–1602. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Onodera, M.; Carr, C. Modelling of resonance response of Dawei Seaport to tsunami waves. In Proceedings of the 34th International Conference on Coastal Engineering 2014, Seoul, Korea, 15–20 June 2014; Lynett, P., Ed.; ASCE/COPRI Coastal Engineering Research Council: Reston, VA, USA, 2014. currents.29. [Google Scholar] [CrossRef] [Green Version]
- Winckler, P.; Sepúlveda, I.; Aron, F.; Contreras-López, M. How do tides and tsunamis interact in a highly energetic channel? The case of Canal Chacao, Chile. J. Geophys. Res. Oceans 2017, 122, 9605–9624. [Google Scholar] [CrossRef] [Green Version]
- DHI. MIKE 21 Flow Model FM, Hydrodynamic Module, User Guide; Danish Hydraulic Institute: Hørsholm, Denmark, 2011. [Google Scholar]
- Tintoré, J.; Vizoso, G.; Casas, B.; Heslop, E.; Pascual, A.; Orfila, A.; Ruiz, S.; Martínez-Ledesma, M.; Torner, M.; Cusí, S.; et al. SOCIB: The Balearic Islands Coastal Ocean Observing and Forecasting System responding to science, technology and society needs. Mar. Technol. Soc. J. 2013, 47, 101–117. [Google Scholar] [CrossRef]
- Pagine Azzurre. Il Portolano dei Mari d’Italia, 2014 ed.; Pagine Azzurre s.r.l.: Rome, Italy, 2014. [Google Scholar]
- Smagorinsky, J. General circulation experiments with the primitive equations: I. The basic experiment. Mon. Weather Rev. 1963, 91, 99–164. [Google Scholar] [CrossRef]
- Kowalik, Z. Introduction to Numerical Modeling of Tsunami Waves; Institute of Marine Science, University of Alaska: Fairbanks, AK, USA, 2012; Available online: https://uaf.edu/cfos/files/research-projects/people/kowalik/book_sum.pdf (accessed on 28 July 2020).
- Flather, R.A. A tidal model of the northwest European continental shelf. Mem. Soc. R. Sci. Liège 1976, 6, 141–164. [Google Scholar]
- Palma, E.D.; Matano, R.P. On the implementation of passive open boundary conditions for a general circulation model: The barotropic mode. J. Geophys. Res. Oceans 1998, 103, 1319–1341. [Google Scholar] [CrossRef]
- Marchesiello, P.; McWilliams, J.C.; Shchepetkin, A. Open boundary conditions for long-term integration of regional oceanic models. Ocean Model. 2001, 3, 1–20. [Google Scholar] [CrossRef]
- Roe, P.L. Approximate Riemann solvers, parameter vectors, and difference schemes. J. Comput. Phys. 1981, 43, 357–372. [Google Scholar] [CrossRef]
- DHI. MIKE 21 & MIKE 3 Flow Model FM—Hydrodynamic and Transport Module. Scientific Documentation; Danish Hydraulic Institute: Hørsholm, Denmark, 2017. [Google Scholar]
- Jawahar, P.; Kamath, H. A high-resolution procedure for Euler and Navier-Stokes computations on unstructured grids. J. Comput. Phys. 2000, 164, 165–203. [Google Scholar] [CrossRef]
- Hirsch, C. Numerical Computation of Internal and External Flows, Volume 2: Computational Methods for Inviscid and Viscous Flows; Wiley: Chichester, UK, 1990. [Google Scholar]
- Darwish, M.S.; Moukalled, F. TVD schemes for unstructured grids. Int. J. Heat Mass Transf. 2003, 46, 599–611. [Google Scholar] [CrossRef]
- Díaz del Río, V. El ‘tsunami’ que llegó de Argelia. El País. 25 June 2003. Available online: https://elpais.com/diario/2003/06/25/futuro/1056492007_850215.html (accessed on 16 February 2020).
- Tel, E.; González, M.-J.; Ruiz, C.; García, M.-J. Sea level data archaeology: Tsunamis and Seiches and other phenomena. In Proceedings of the 4a Assembleia Luso Espanhola de Geodesia e Geofísica, Figueira da Foz, Portugal, 3–7 February 2004. [Google Scholar]
- Basterretxea, G.; Orfila, A.; Jordi, A.; Casas, B.; Lynett, P.; Liu, P.L.F.; Duarte, C.M.; Tintoré, J. Seasonal dynamics of a microtidal pocket beach with posidonia oceanica seabeds (Mallorca, Spain). J. Coast. Res. 2004, 20, 1155–1164. [Google Scholar] [CrossRef] [Green Version]
- Gailler, A.; Hébert, H.; Schindelé, F.; Reymond, D. Coastal amplification laws for the French Tsunami Warning Center: Numerical modeling and fast estimate of tsunami wave heights along the French Riviera. Pure Appl. Geophys. 2018, 175, 1429–1444. [Google Scholar] [CrossRef]
- Luger, S.A.; Harris, R.L. Modelling tsunamis generated by earthquakes and submarine slumps using Mike 21. In Proceedings of the International MIKE by DHI Conference ‘Modelling in a World of Change’, Copenhagen, Denmark, 6–8 September 2010; p. 17. [Google Scholar]
- ABC (Madrid). 23 May 2003, p. 27. Available online: https://www.abc.es/archivo/periodicos/abc-madrid-20030523-27.html (accessed on 3 September 2020).
- Bureau de Recherches Géologiques et Minières (BRGM). Base de données des tsunamis observés en France. Available online: http://tsunamis.brgm.fr/fiche_biblio.asp?NUMEVT=60004 (accessed on 31 December 2016).
- www.belt.es. 26 May 2003. Available online: http://www.belt.es/noticias/2003/mayo/26/baleares.htm (accessed on 31 December 2016).
- La Vanguardia. 23 May 2003, p. 37. Available online: http://hemeroteca-paginas.lavanguardia.com/LVE01/PUB/2003/05/23/LVG200305230371LB.pdf (accessed on 3 September 2020).
- Roig-Munar, F.X.; Martín-Prieto, J.Á.; Rodríguez-Perea, A.; Gelabert Ferrer, B.; Vilaplana Fernández, J.M. Bloques en plataformas rocosas y acantilados del SE de Menorca: Tipología y procesos. In Geomorfología Litoral de Menorca: Dinámica, evolución y prácticas de gestión, Monografies de la Societat d’Història Natural de les Balears; Gómez-Pujol, L., Pons, G.X., Eds.; Societat d’Història Natural de les Balears: Palma, Illes Balears, 2017; Volume 25, pp. 251–262. [Google Scholar]
- Libertad Digital. 22 May 2003. Available online: http://www.libertaddigital.com/sociedad/numerosas-embarcaciones-y-puertos-de-baleares-han-sufrido-danos-a-causa-del-terremoto-1275760565/ (accessed on 3 September 2020).
- Papadopoulos, G.A. Tsunamis in the European-Mediterranean Region: From Historical Record to Risk Mitigation; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar]
- Consell d’Eivissa. Plan territorial insular de protección civil de la isla de Eivissa -Platerei- Documento de Consulta. Available online: http://www.conselldeivissa.es/portal/RecursosWeb/DOCUMENTOS/1/0_5186_1.pdf (accessed on 3 September 2020).
- Periódico de Ibiza y Formentera. 24 May 2003. Available online: https://www.periodicodeibiza.es/sucesos/ultimas/2003/05/24/722787/las-pitiuses-fueron-las-%20islas-que-menos-sufrieron-el-maremoto.html (accessed on 3 September 2020).
- Diario de Ibiza. 26 August 2011. Available online: http://www.diariodeibiza.es/pitiuses-balears/2011/08/26/sistema-alerta-maremotos-mediterraneoafronta-primer-gran-ensayo/503113.html (accessed on 3 September 2020).
- Azurseisme. Site sur la sismicité historique régionale. Available online: https://www.azurseisme.com/Tsunami-english-version.html (accessed on 3 September 2020).
- Periódico de Ibiza y Formentera, 28 October 2010. Available online: https://www.periodicodeibiza.es/pitiusas/ibiza/2010/10/28/24008/el-peligro-que-llega-del-mar.html (accessed on 3 September 2020).
- El Mundo, 22 May 2003. Available online: https://www.elmundo.es/elmundo/2003/05/22/sociedad/1053590416.html (accessed on 3 September 2020).
- Fritz, H.M.; Phillips, D.A.; Okayasu, A.; Shimozono, T.; Liu, H.; Mohammed, F.; Skanavis, V.; Synolakis, C.E.; Takahashi, T. The 2011 Japan tsunami current velocity measurements from survivor videos at Kesennuma Bay using LiDAR. Geophys. Res. Lett. 2012, 39, L00G23. [Google Scholar] [CrossRef]
- Okal, E.A.; Fritz, H.M.; Raad, P.E.; Synolakis, C.; Al-Shijbi, Y.; Al-Saifi, M. Oman field survey after the December 2004 Indian Ocean tsunami. Earthq. Spectra 2006, 22, 203–218. [Google Scholar] [CrossRef]
- Okal, E.A.; Fritz, H.M.; Raveloson, R.; Joelson, G.; Pančošková, P.; Rambolamanana, G. Madagascar field survey after the December 2004 Indian Ocean tsunami. Earthq. Spectra 2006, 22, 263–283. [Google Scholar] [CrossRef]
- Okal, E.A.; Sladen, A.; Okal, E.A.-S. Rodrigues, Mauritius, and Réunion Islands field survey after the December 2004 Indian Ocean tsunami. Earthq. Spectra 2006, 22, 241–261. [Google Scholar] [CrossRef]
- Wilson, R.I.; Admire, A.R.; Borrero, J.C.; Dengler, L.A.; Legg, M.R.; Lynett, P.; McCrink, T.P.; Miller, K.M.; Ritchie, A.; Sterling, K.; et al. Observations and impacts from the 2010 Chilean and 2011 Japanese tsunamis in California (USA). Pure Appl. Geophys. 2013, 170, 1127–1147. [Google Scholar] [CrossRef]
- Admire, A.R.; Dengler, L.A.; Crawford, G.B.; Uslu, B.U.; Borrero, J.C.; Greer, S.D.; Wilson, R.I. Observed and modeled currents from the Tohoku-oki, Japan and other recent tsunamis in Northern California. Pure Appl. Geophys. 2014, 171, 3385–3403. [Google Scholar] [CrossRef]
- Borrero, J.C.; Lynett, P.J.; Kalligeris, N. Tsunami currents in ports. Phil. Trans. R. Soc. A 2015, 373, 20140372. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lynett, P.; Weiss, R.; Renteria, W.; De La Torre Morales, G.; Son, S.; Arcos, M.E.M.; MacInnes, B.T. Coastal impacts of the March 11th Tohoku, Japan tsunami in the Galapagos Islands. Pure Appl. Geophys. 2013, 170, 1189–1206. [Google Scholar] [CrossRef] [Green Version]
- Borrero, J.C.; Bell, R.; Csato, C.; DeLange, W.; Goring, D.; Greer, S.D.; Pickett, V.; Power, W. Observations, effects and real time assessment of the March 11, 2011 Tohoku-oki tsunami in New Zealand. Pure Appl. Geophys. 2013, 170, 1229–1248. [Google Scholar] [CrossRef]
- Wilson, R.I.; Miller, K.M. Tsunami Emergency Response Playbooks and FASTER Tsunami Height Calculation: Background Information and Guidance for Use. California Geological Survey Special Report 236; California Department of Conservation, California Geological Survey: Sacramento, CA, USA, 2014. [Google Scholar]
- Suppasri, A.; Muhari, A.; Futami, T.; Imamura, F.; Shuto, N. Loss functions for small marine vessels based on survey data and numerical simulation of the 2011 Great East Japan tsunami. J. Waterw. Port Coast. Ocean Eng. 2014, 140, 04014018. [Google Scholar] [CrossRef]
- Muhari, A.; Charvet, I.; Tsuyoshi, F.; Suppasri, A.; Imamura, F. Assessment of tsunami hazards in ports and their impact on marine vessels derived from tsunami models and the observed damage data. Nat. Hazards 2015, 78, 1309–1328. [Google Scholar] [CrossRef] [Green Version]
- Rabinovich, A.B.; Thomson, R.E. The 26 December 2004 Sumatra tsunami: Analysis of tide gauge data from the World Ocean Part 1. Indian Ocean and South Africa. Pure Appl. Geophys. 2007, 164, 261–308. [Google Scholar] [CrossRef]
- Torrence, C.; Compo, G.P. A practical guide to wavelet analysis. Bull. Am. Meteor. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef] [Green Version]
- RCC Pilotage Foundation; Baggaley, D.; Baggaley, S. Islas Baleares: Ibiza, Formentera, Mallorca, Cabrera and Menorca, 11th ed.; Imray Laurie Norie & Wilson Ltd.: Cambridgeshire, UK, 2018. [Google Scholar]
- Rabinovich, A.B. Seiches and harbor oscillations. In Handbook of Coastal and Ocean Engineering; Kim, Y.C., Ed.; World Scientific Pub Co Pte Ltd.: Singapore, 2009; pp. 193–236. [Google Scholar]




















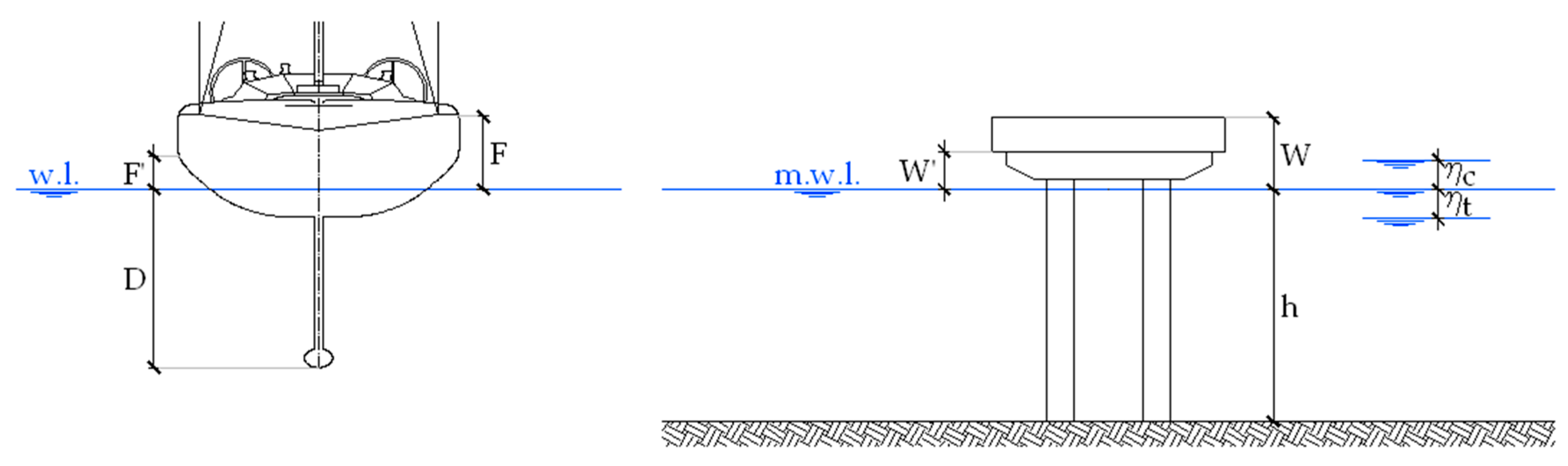
| Source | Sea Level Station | Simulated Fault Mechanism | Mw | Arrival Time | First Peak Elevation (m) | First Peak to Trough Height (m) |
|---|---|---|---|---|---|---|
| Sea level record | Sant Antoni | 19:45 | 0.37 | 0.99 | ||
| Wang & Liu [24] | Sant Antoni | Meghraoui et al. [14] | 6.8–6.9 | 19:47 | 0.19 | 0.54 |
| Wang & Liu [24] | Sant Antoni | Wang & Liu [24] | 7.2 | 19:45 | 0.37 | 1.08 |
| Alasset et al. [26] | Sant Antoni | Meghraoui et al. [14] | 6.8–6.9 | 19:47 | 0.07 | 0.19 |
| Alasset et al. [26] | Sant Antoni | Semmane et al. [29] | 7.1 | 19:45 | 0.22 | 0.57 |
| Sea level record | Palma | 19:35 | 0.24 | 0.59 | ||
| Alasset et al. [26] | Palma | Meghraoui et al. [14] | 6.8–6.9 | 19:45 | 0.04 | 0.08 |
| Alasset et al. [26] | Palma | Semmane et al. [29] | 7.1 | 19:41 | 0.16 | 0.36 |
| Vela et al. [31] | Palma | Meghraoui et al. [14] | 6.8–6.9 | 19:41 | 0.09 | 0.24 |
| Vela et al. [31] | Palma | Wang & Liu [24] | 7.2 | 19:41 | 0.12 | 0.30 |
| Source | Time (UTC) | Lon (°E) | Lat (°N) | Depth (km) | M0 (N·m) | Mw | S/D/R (°) | Fault Length (km) | Fault Width (km) | Data Type |
|---|---|---|---|---|---|---|---|---|---|---|
| CRAAG | 18:44:19 | 3.58 | 36.91 | 10 | 6.8 | |||||
| CGS | 18:44:40 | 3.53 | 36.81 | 7.0 | ||||||
| EMSC | 18:44:22 | 3.76 | 37.02 | 21 | 6.8 | |||||
| USGS NEIC | 18:44:19 | 3.78 | 36.89 | 10 | 6.7 | |||||
| USGS (2014) | 18:44:20 | 3.634 | 36.964 | 12 | 2.15 × 1019 | 6.8 | 55/30/90 | |||
| Harvard CMT | 18:44:30 | 3.58 | 36.93 | 15 | 2.01 × 1019 | 6.8 | 57/44/71 | |||
| CEA-DASE | 18:44:22 | 3.78 | 36.71 | 18 | 6.9 | |||||
| ETH | 3.74 | 37.04 | 10 | 6.74 | 63/35/48 | |||||
| INGV | 18:44:29 | 3.61 | 36.90 | 15 | 1.8 × 1019 | 6.8 | 65/27/86 | |||
| Yagi [39,40] | 3.78 | 36.89 | 10 | 2.4 × 1019 | 6.9 | 54/47/86 | 60 | 10 | T | |
| Delouis & Vallée [41] | 10 | 2.38 × 1019 | 6.9 | 57/39/83 | 50 | T | ||||
| Yelles et al. [28] | 2.4 × 1019 | 6.9 | 55/42/84 | 32 | 14 | G | ||||
| Delouis et al. [27] | 3.65 (B) | 36.83 (B) | 6.5 | 2.9 × 1019 | 6.9 | 70/45/95 | 60 | 24 | T, G, U | |
| Meghraoui et al. [14] | 3.65 (B) | 36.83 (B) | 2.75 × 1019 | 6.9 | 54/50/(90) | 54 | U | |||
| Bezzeghoud et al. [42] | 3.65 (B) | 36.83 (B) | 7 | 1.3 × 1019 | 6.8 | 64/50/90 | T | |||
| Braunmiller & Bernardi [43] | 18:44 | 3.65 (B) | 36.83 (B) | 7.0 | 62/25/82 | 50 | T | |||
| Semmane et al. [29] | 3.65 (B) | 36.83 (B) | 5.9 × 1019 | 7.1 | 54/47/88 | 64 | 32 | G, U, M | ||
| Belabbès et al. [34], planar | 3.65 (B) | 36.83 (B) | 8 | 1.78 × 1019 | 6.8 | 65/40/90 | 60 | 30 | S, U, G | |
| Belabbès et al. [34], curved | 3.65 (B) | 36.83 (B) | 10 | 2.15 × 1019 | 6.8 | 65/40/90 | S, U, G | |||
| Santos et al. [44], sol 1 | 3.660 | 36.846 | 8 | 1.40 × 1019 | 6.7 | 64/50/97 | 60 | 20 | T, U, S |
| Tide Gauge | Yelles et al. | Delouis et al. | Meghraoui et al. | Semmane et al. | Braunmiller & Bernardi | Santos et al. | Belabbès et al. Modified | Belabbès et al. Modified | |||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Palma | 19:30–24:00 | Model | global | global | global | global | global | global | global | nested | |
| Arrival time | 19:35 | 19:43:04 | 19:42:52 | 19:43:34 | 19:41:30 | 19:38:36 | 19:42:56 | 19:41:36 | 19:41:38 | ||
| Max elevation (m) | 0.64 | 0.17 | 0.14 | 0.10 | 0.27 | 0.08 | 0.10 | 0.41 | 0.43 | ||
| Max drawdown (m) | −0.52 | −0.17 | −0.14 | −0.09 | −0.27 | −0.09 | −0.11 | −0.43 | −0.45 | ||
| RMS elevation (m) | 0.251 | 0.072 | 0.057 | 0.041 | 0.114 | 0.035 | 0.044 | 0.189 | 0.196 | ||
| Mean period (min) | 22.7 | 22.4 | 22.4 | 22.3 | 22.5 | 22.7 | 22.4 | 22.5 | 22.5 | ||
| Cagliari | 19:50–24:00 | Model | nested | nested | nested | nested | nested | nested | nested | ||
| Arrival time | 20:00 | 20:12:42 | 20:10:56 | 20:11:12 | 20:08:32 | 20:08:50 | 20:14:06 | 20:11:24 | |||
| Max elevation (m) | 0.16 | 0.05 | 0.06 | 0.03 | 0.12 | 0.06 | 0.03 | 0.20 | |||
| Max drawdown (m) | −0.16 | −0.05 | −0.04 | −0.02 | −0.10 | −0.05 | −0.03 | −0.17 | |||
| RMS elevation (m) | 0.082 | 0.022 | 0.020 | 0.013 | 0.043 | 0.022 | 0.013 | 0.070 | |||
| Mean period (min) | (33.3) | 28.1 | 28.4 | 28.1 | 28.6 | 28.5 | 28.0 | 28.4 | |||
| Sant Antoni | 19:35–24:00 | Model | nested | ||||||||
| Arrival time | 19:45 | 19:44:14 | |||||||||
| Max elevation (m) | 1.11 | 1.10 | |||||||||
| Max drawdown (m) | −0.85 | −1.18 | |||||||||
| RMS elevation (m) | 0.314 | 0.306 | |||||||||
| Mean period (min) | 19.5 | 19.1 | |||||||||
| Ibiza | 19:25–24:00 | Model | nested | ||||||||
| Arrival time | 19:30 | 19:32:24 | |||||||||
| Max elevation (m) | 0.34 | 0.41 | |||||||||
| Max drawdown (m) | −0.31 | −0.36 | |||||||||
| RMS elevation (m) | 0.149 | 0.154 | |||||||||
| Mean period (min) | 22.1 | 16.4 | |||||||||
| Valencia | 19:55–24:00 | Model | nested | ||||||||
| Arrival time | 20:10 | 20:05:52 | |||||||||
| Max elevation (m) | 0.35 | 0.14 | |||||||||
| Max drawdown (m) | −0.31 | −0.13 | |||||||||
| RMS elevation (m) | 0.123 | 0.053 | |||||||||
| Mean period (min) | 20.0 | 20.5 | |||||||||
| Synchronous Slip | Asynchronous Slip | Observation | ||||
|---|---|---|---|---|---|---|
| Zero-Crossing Time (UTC) | Extreme Elevation (m) | Zero-Crossing Time (UTC) | Extreme Elevation (m) | Zero-Crossing Time (UTC) | Extreme Elevation (m) | |
| Palma de Mallorca | 19:41:36 | 19:42:06 | 19:35 | |||
| 0.145 | 0.147 | 0.24 | ||||
| 19:54:28 | 19:54:54 | 19:49 | ||||
| −0.308 | −0.301 | −0.35 | ||||
| 20:07:26 | 20:08:00 | 20:04 | ||||
| 0.209 | 0.201 | 0.12 | ||||
| 20:18:08 | 20:18:42 | 20:14 | ||||
| −0.184 | −0.173 | −0.13 | ||||
| 20:26:38 | 20:27:12 | 20:22 | ||||
| Sant Antoni | 19:44:14 | 19:44:44 | 19:45 | |||
| 0.292 | 0.287 | 0.37 | ||||
| 19:52:18 | 19:52:54 | 19:54 | ||||
| −0.589 | −0.574 | −0.62 | ||||
| 20:02:34 | 20:03:04 | 20:04 | ||||
| 1.101 | 1.089 | 1.11 | ||||
| 20:11:24 | 20:11:52 | 20:12 | ||||
| −1.176 | −1.160 | −0.85 | ||||
| 20:20:42 | 20:21:02 | 20:23 | ||||
| Mahon | 19:38:24 | 19:39:20 | ||||
| 0.655 | 0.767 | |||||
| 19:44:12 | 19:44:50 | |||||
| −0.521 | −0.614 | |||||
| 19:56:16 | 19:56:36 | |||||
| 0.618 | 0.690 | |||||
| 20:04:54 | 20:03:50 | |||||
| −0.114 | −0.110 | |||||
| 20:07:20 | 20:07:44 | |||||
| Damage Mechanism | Source | Image |
|---|---|---|
| Boats touching the dry bottom of the harbor | https://www.youtube.com/watch?v=AxFNXRTXgHw Santa Cruz Harbor, California, USA, 11 March 2011 tsunami | around 1:40/16:48 |
| Boats captured in a tsunami bore | https://www.youtube.com/watch?v=jltIeWB1XH8 Santa Cruz Harbor, California, USA, 11 March 2011 tsunami | around 1:17/5:50 |
| Boat wedged under a floating dock | https://www.youtube.com/watch?v=AxFNXRTXgHw Santa Cruz Harbor, California, USA, 11 March 2011 tsunami | around 8:52/16:48 |
| Stranded ships | http://www.cargolaw.com/2011nightmare_sendai_ships.html Sendai, Japan, 11 March 2011 tsunami | several examples |
| Id | Port, Bay | Peak Surface Elevation (m) | Peak Drawdown (m) | Current Speed (m/s) | Estimated n. of Boats in Harbor | Reported Damage |
|---|---|---|---|---|---|---|
| A | Palma de Mallorca-Between Sant Magí dock and Royal Yacht Club up to La Riera | +1.15 | −1.07 | 1.95 (2.1) | 1500 | Flooding, several boats damaged by grounding and collision |
| B | Sant Antoni-Near the fishermen pier | +1.16 | −1.33 | 1.2 (1.4) | 150 | Flooding, 15 boats, port facilities |
| C | Port Mahon-Colàrsega | +0.79 | −0.64 | 0.7 (1.6) | 120 | Flooding, 37 boats, port facilities |
| D | Port Mahon-Cala Llonga | +0.51 | −0.47 | 0.6 (1.0) | 45 | 20 boats, port facilities |
| E | Cala de Sant Esteve | +0.86 | −1.37 | 2.2 (3.0) | 20 | Flooding, at least 5 boats stranded |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Masina, M.; Archetti, R.; Lamberti, A. 21 May 2003 Boumerdès Earthquake: Numerical Investigations of the Rupture Mechanism Effects on the Induced Tsunami and Its Impact in Harbors. J. Mar. Sci. Eng. 2020, 8, 933. https://doi.org/10.3390/jmse8110933
Masina M, Archetti R, Lamberti A. 21 May 2003 Boumerdès Earthquake: Numerical Investigations of the Rupture Mechanism Effects on the Induced Tsunami and Its Impact in Harbors. Journal of Marine Science and Engineering. 2020; 8(11):933. https://doi.org/10.3390/jmse8110933
Chicago/Turabian StyleMasina, Marinella, Renata Archetti, and Alberto Lamberti. 2020. "21 May 2003 Boumerdès Earthquake: Numerical Investigations of the Rupture Mechanism Effects on the Induced Tsunami and Its Impact in Harbors" Journal of Marine Science and Engineering 8, no. 11: 933. https://doi.org/10.3390/jmse8110933
APA StyleMasina, M., Archetti, R., & Lamberti, A. (2020). 21 May 2003 Boumerdès Earthquake: Numerical Investigations of the Rupture Mechanism Effects on the Induced Tsunami and Its Impact in Harbors. Journal of Marine Science and Engineering, 8(11), 933. https://doi.org/10.3390/jmse8110933







