Bragg Reflections of Oblique Water Waves by Periodic Surface-Piercing and Submerged Breakwaters
Abstract
1. Introduction
2. Materials and Methods
2.1. The Mathematical Model
2.2. Eigenfunction Matching Method
3. Results
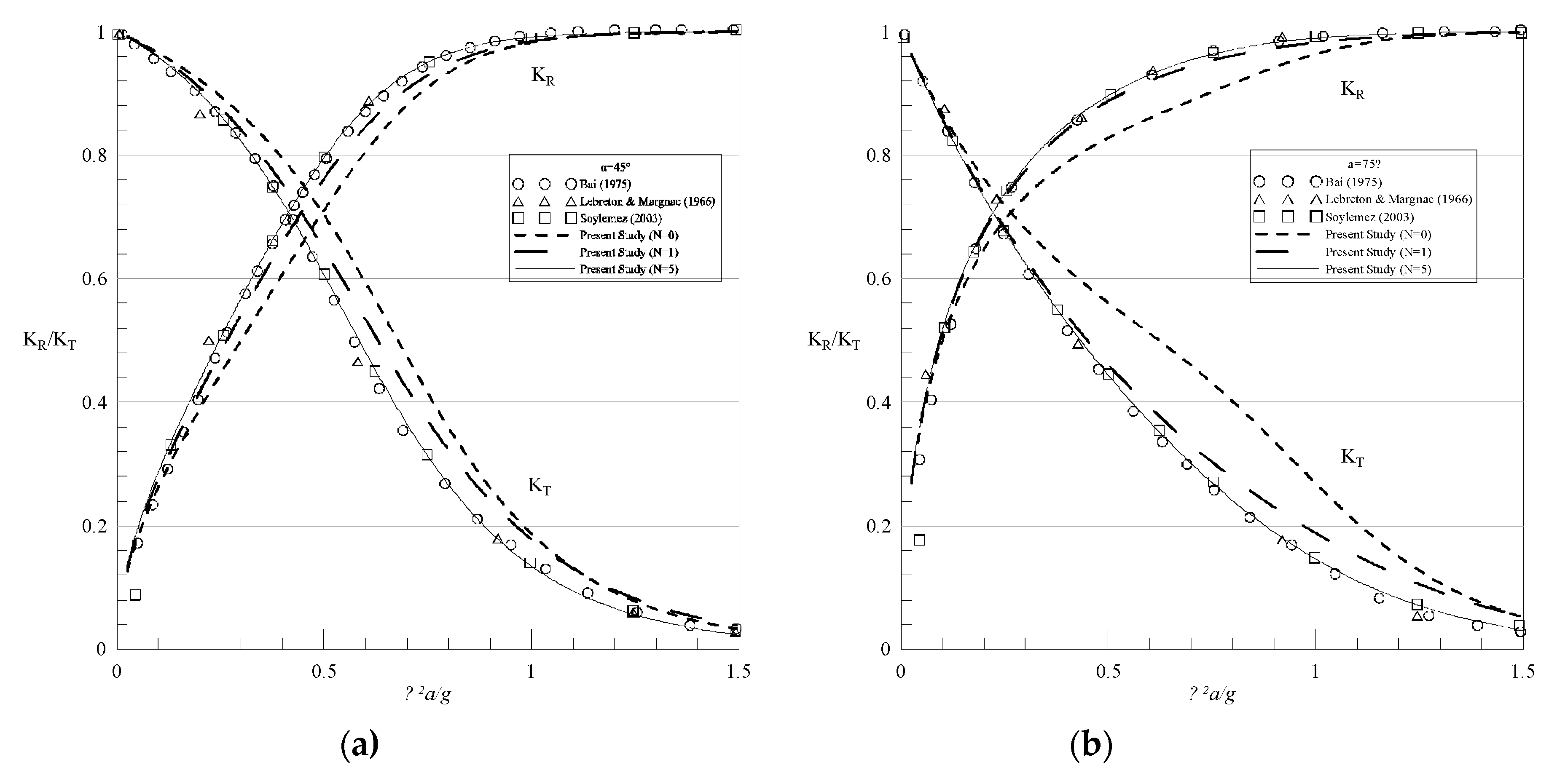
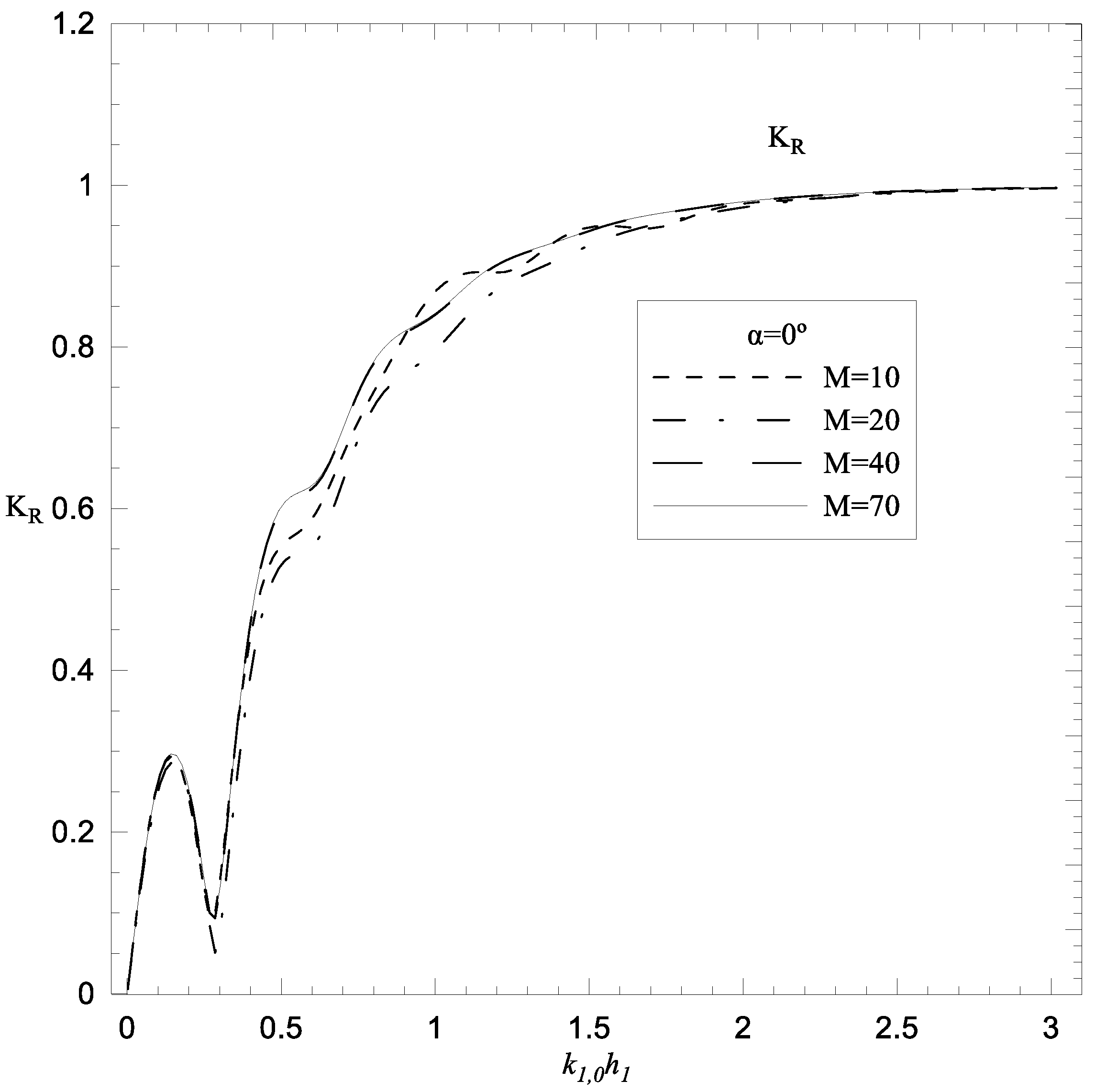
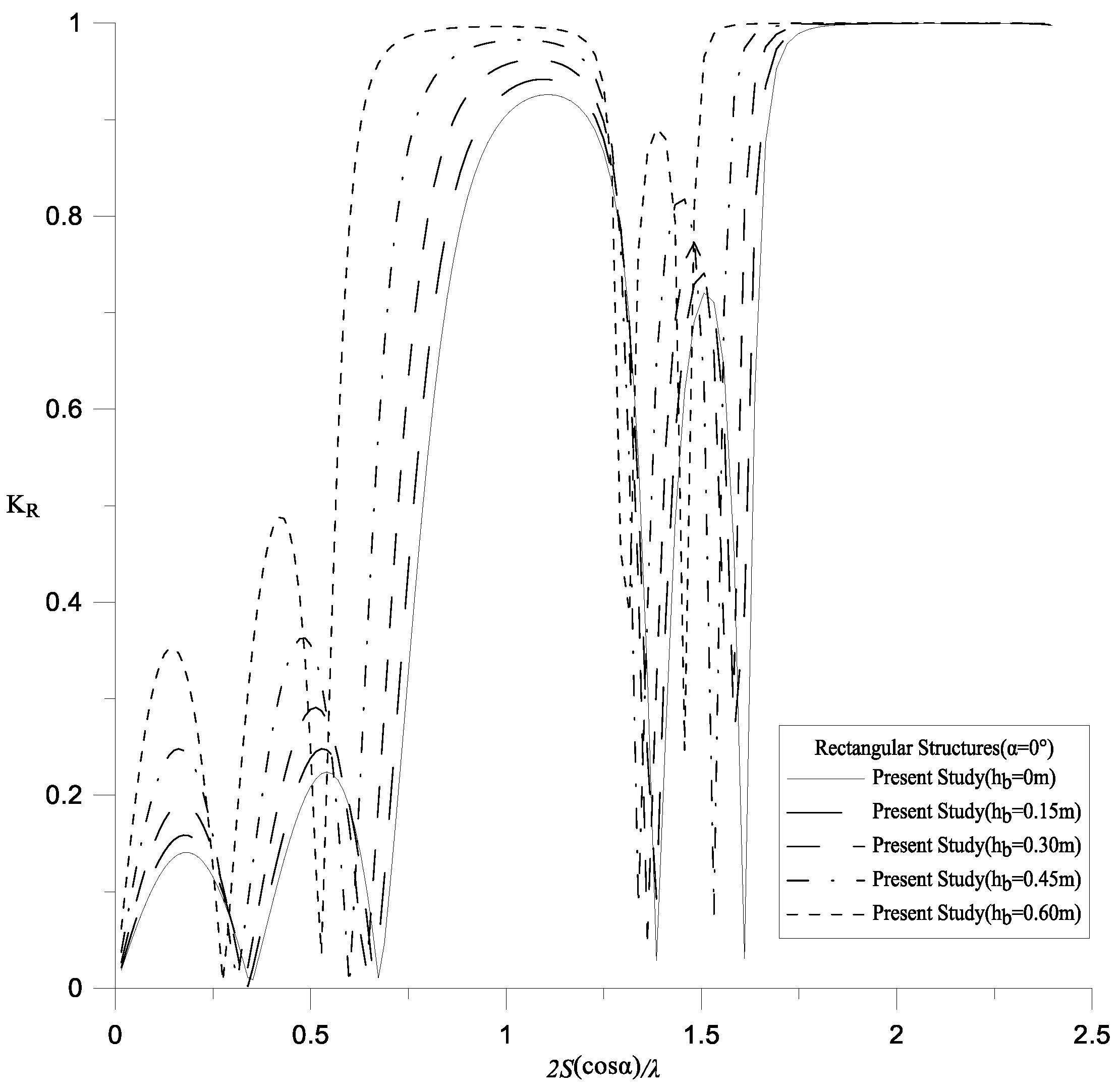
3.1. Rectangular Surface-Piercing Structure over a Flat Bottom
3.2. Rectangular Surface-Piercing Structure behind Parabolic Breakwater
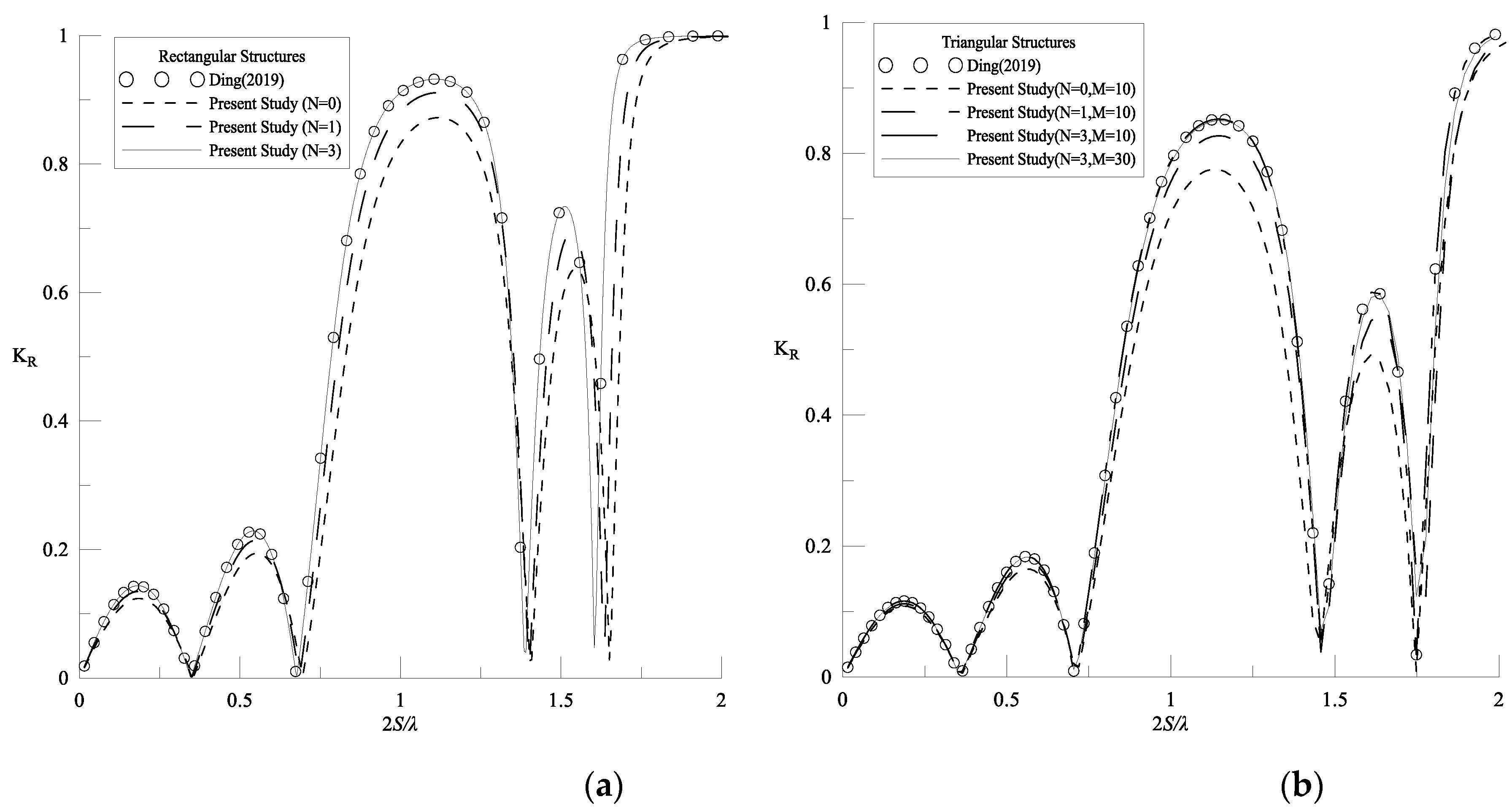
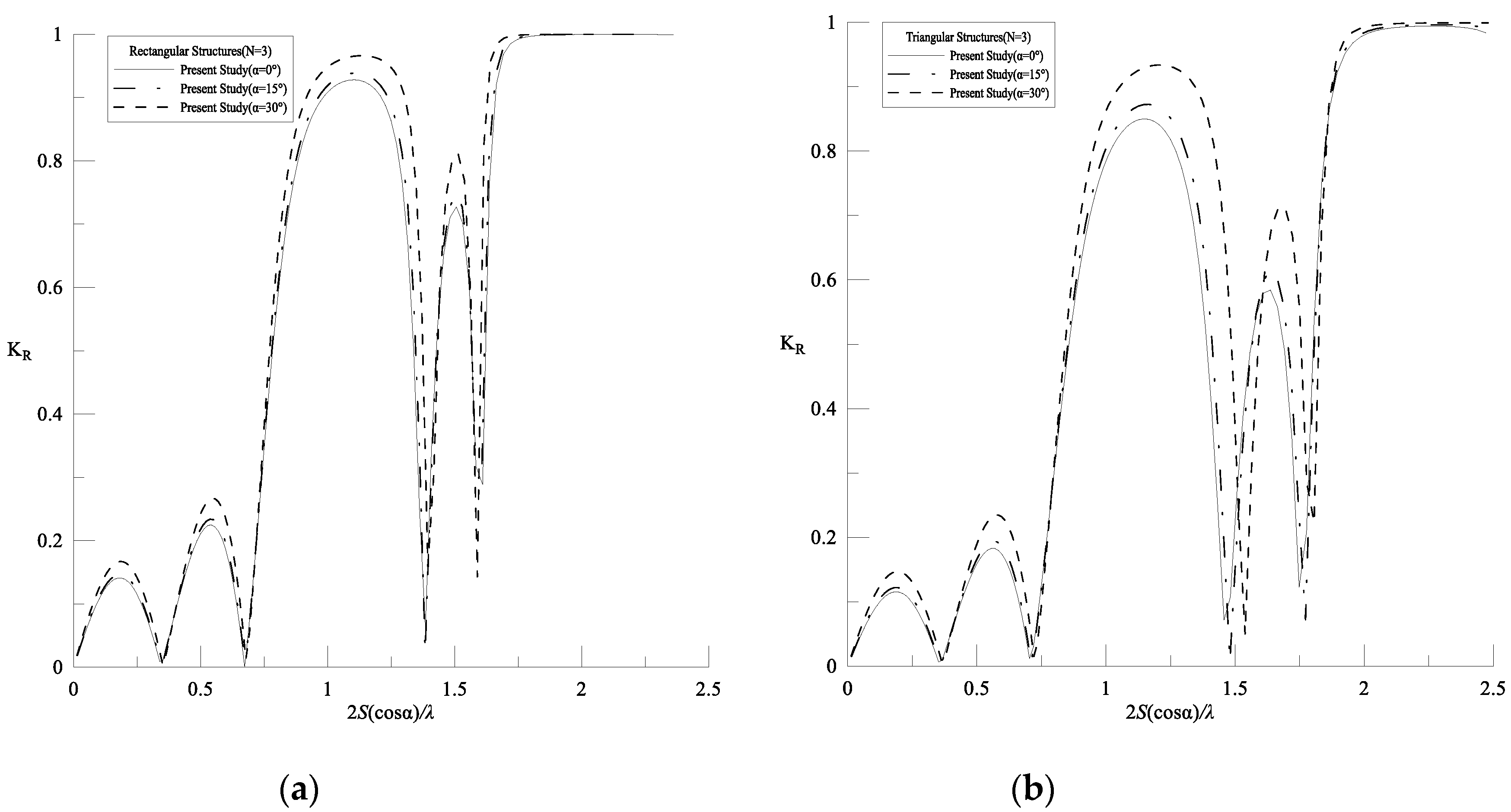
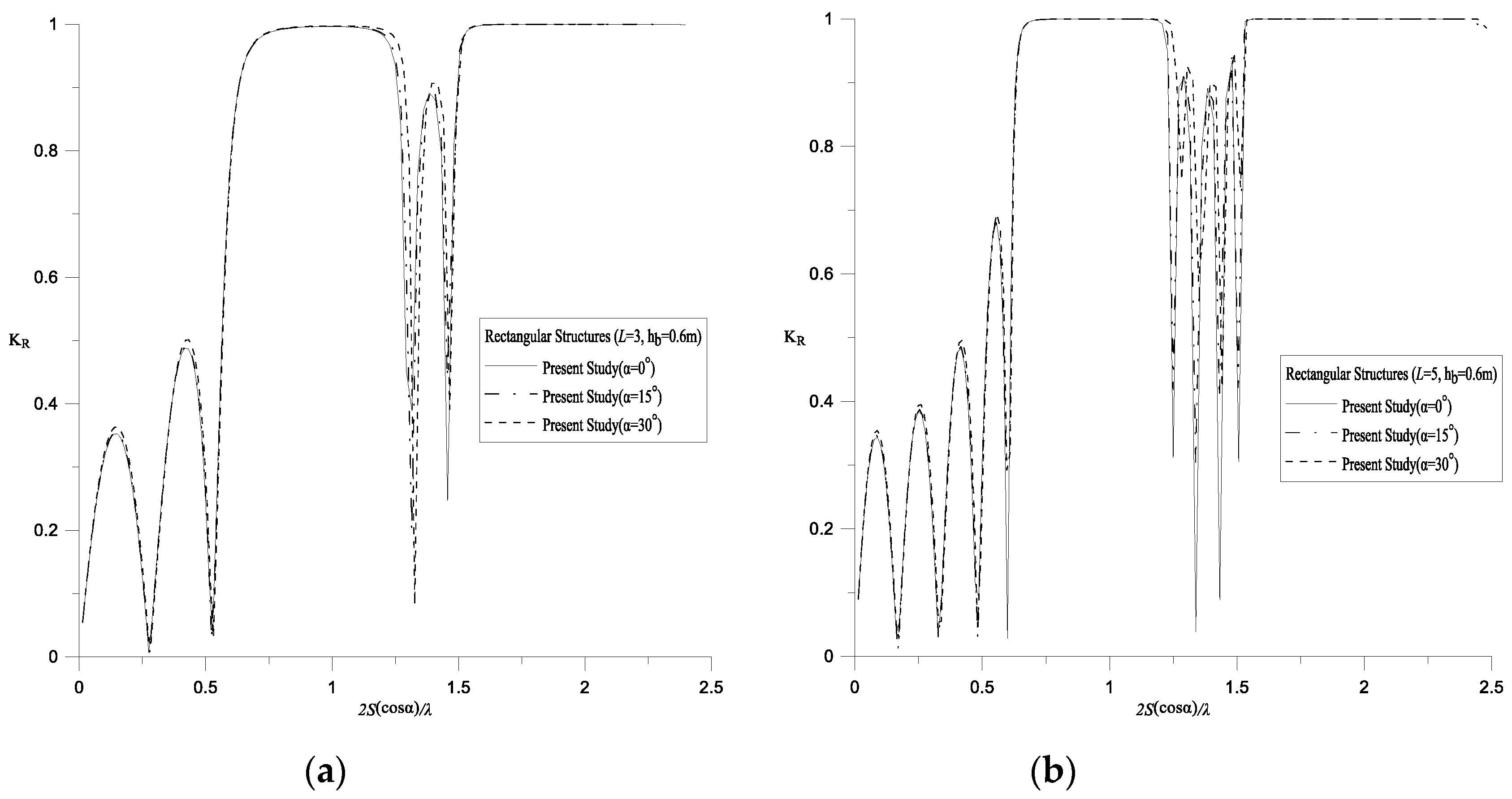
3.3. Bragg Reflections by Periodic Surface-Piercing Structures over Flat Bottom
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Belibassakis, K.; Athanassoulis, G. A coupled-mode technique for weakly nonlinear wave interaction with large floating structures lying over variable bathymetry regions. Appl. Ocean Res. 2006, 28, 59–76. [Google Scholar] [CrossRef]
- Shih, R.-S. Experimental study on the performance characteristics of porous perpendicular pipe breakwaters. Ocean Eng. 2012, 50, 53–62. [Google Scholar] [CrossRef]
- Chung, J.S. Motion of a floating structure in water of uniform depth. J. Hydronautics 1976, 10, 65–73. [Google Scholar] [CrossRef]
- Kagemoto, H.; Fujino, M.; Murai, M. Theoretical and experimental predictions of the hydroelastic response of a very large floating structure in waves. Appl. Ocean Res. 1998, 20, 135–144. [Google Scholar] [CrossRef]
- Mei, C.C.; Hara, T.; Naciri, M. Note on Bragg scattering of water waves by parallel bars on the seabed. J. Fluid Mech. 1988, 186, 147–162. [Google Scholar] [CrossRef]
- Bailard, J.A.; DeVries, J.W.; Kirby, J.T. Considerations in using Bragg reflection for storm erosion protection. J. Waterw. Port Coast. Ocean Eng. 1992, 118, 62–74. [Google Scholar] [CrossRef]
- Tsai, L.-H.; Wen, C.-C. Design of a series of submerged breakwaters for coastal protection against waves. China Ocean Eng. 2010, 24, 553–564. [Google Scholar]
- Hsu, T.-W.; Lin, J.-F.; Hsiao, S.-C.; Ou, S.-H.; Babanin, A.V.; Wu, Y.-T. Wave reflection and vortex evolution in Bragg scattering in real fluids. Ocean Eng. 2014, 88, 508–519. [Google Scholar] [CrossRef]
- Ouyang, H.-T.; Chen, K.-H.; Tsai, C.-M. Investigation on bragg reflection of surface water waves induced by a train of fixed floating pontoon breakwaters. Int. J. Nav. Archit. Ocean Eng. 2015, 7, 951–963. [Google Scholar] [CrossRef]
- Ding, W.-W.; Zou, Z.-J.; Wu, J.-P.; Huang, B.-G. Investigation of surface-piercing fixed structures with different shapes for Bragg reflection of water waves. Int. J. Nav. Archit. Ocean Eng. 2019, 11, 819–827. [Google Scholar] [CrossRef]
- Roseau, M. Asymptotic Wave Theory; North-Holland Pub. Co.: Amsterdam, NY, USA, 1976. [Google Scholar]
- Berkhoff, J.C.W. Computation of combined refraction-diffraction. In Proceedings of the 13th International Conference on Coastal Engineering, Vancouver, BC, Canada, 10–14 July 1972; pp. 471–490. [Google Scholar]
- Kirby, J.T. A general wave equation for waves over rippled beds. J. Fluid Mech. 1986, 162, 171–186. [Google Scholar] [CrossRef]
- Chamberlain, P.G.; Porter, D. The modified mild-slope equation. J. Fluid Mech. 1995, 291, 393–407. [Google Scholar] [CrossRef]
- Massel, S.R. Extended refraction-diffraction equation for surface waves. Coast. Eng. 1993, 19, 97–126. [Google Scholar] [CrossRef]
- Porter, D.; Staziker, D.J. Extensions of the mild-slope equation. J. Fluid Mech. 1995, 300, 367–382. [Google Scholar] [CrossRef]
- Athanassoulis, G.; Belibassakis, K. A consistent coupled-mode theory for the propagation of small-amplitude water waves over variable bathymetry regions. J. Fluid Mech. 1999, 389, 275–301. [Google Scholar] [CrossRef]
- Belibassakis, K.; Athanassoulis, G. Extension of second-order Stokes theory to variable bathymetry. J. Fluid Mech. 2002, 464, 35–80. [Google Scholar] [CrossRef]
- Belibassakis, K.A.; Athanassoulis, G.A.; Gerostathis, T.P. A Coupled-Mode Model for the Refraction–Diffraction of Linear Waves over Steep Three-Dimensional Bathymetry. Appl. Ocean Res. 2001, 23, 319–336. [Google Scholar] [CrossRef]
- Belibassakis, K. A coupled-mode model for the scattering of water waves by shearing currents in variable bathymetry. J. Fluid Mech. 2007, 578, 413–434. [Google Scholar] [CrossRef]
- Belibassakis, K.; Touboul, J. A nonlinear coupled-mode model for waves propagating in vertically sheared currents in variable bathymetry—Collinear waves and currents. Fluids 2019, 4, 61. [Google Scholar] [CrossRef]
- Toledo, Y.; Agnon, Y. Three dimensional application of the complementary mild-slope equation. Coast. Eng. 2011, 58, 1–8. [Google Scholar] [CrossRef]
- Belibassakis, K.; Touboul, J.; Laffitte, E.; Rey, V. A mild-slope system for bragg scattering of water waves by sinusoidal bathymetry in the presence of vertically sheared currents. J. Mar. Sci. Eng. 2019, 7, 9. [Google Scholar] [CrossRef]
- Suh, K.D.; Lee, C.; Park, W.S. Time-dependent equations for wave propagation on rapidly varying topography. Coast. Eng. 1997, 32, 91–117. [Google Scholar] [CrossRef]
- Belibassakis, K.; Athanassoulis, G. A coupled-mode model for the hydroelastic analysis of large floating bodies over variable bathymetry regions. J. Fluid Mech. 2005, 531, 221–249. [Google Scholar] [CrossRef]
- Porter, D.; Porter, R. Approximations to wave scattering by an ice sheet of variable thickness over undulating bed topography. J. Fluid Mech. 2004, 509, 145–179. [Google Scholar] [CrossRef]
- Porter, D. The mild-slope equations: A unified theory. J. Fluid Mech. 2020, 887, A29. [Google Scholar] [CrossRef]
- Takano, K. Effets d’un obstacle parallelepipedique sur la propagation de la houle. La Houille Blanche 1960, 15, 247–267. [Google Scholar] [CrossRef]
- Kirby, J.T.; Dalrymple, R.A.; Seo, S.N. Propagation of obliquely incident water waves over a trench. Part 2. Currents flowing along the trench. J. Fluid Mech. 1987, 176, 95–116. [Google Scholar] [CrossRef]
- Kirby, J.T.; Dalrymple, R.A. Propagation of obliquely incident water waves over a trench. J. Fluid Mech. 1983, 133, 47–63. [Google Scholar] [CrossRef]
- Devillard, P.; Dunlop, F.; Souillard, B. Localization of gravity waves on a channel with a random bottom. J. Fluid Mech. 1988, 186, 521–538. [Google Scholar] [CrossRef]
- O’Hare, T.J.; Davies, A.G. A comparison of two models for surface-wave propagation over rapidly varying topography. Appl. Ocean Res. 1993, 15, 1–11. [Google Scholar] [CrossRef]
- O’Hare, T.J.; Davies, A.G. A new model for surface wave propagation over undulating topography. Coast. Eng. 1992, 18, 251–266. [Google Scholar] [CrossRef]
- Tsai, C.-C.; Lin, Y.-T.; Hsu, T.-W. On step approximation of water-wave scattering over steep or undulated slope. Int. J. Offshore Polar Eng. 2014, 24, 98–105. [Google Scholar]
- Tsai, C.C.; Hsu, T.-W.; Lin, Y.-T. On Step Approximation for Roseau’s Analytical Solution of Water Waves. Math. Probl. Eng. 2011, 2011. [Google Scholar] [CrossRef]
- Tsai, C.-C.; Lin, Y.-T.; Chang, J.-Y.; Hsu, T.-W. A coupled-mode study on weakly viscous Bragg scattering of surface gravity waves. Ocean Eng. 2016, 122, 136–144. [Google Scholar] [CrossRef]
- Tsai, C.C.; Lin, Y.-T.; Hsu, T.-W. On the weak viscous effect of the reflection and transmission over an arbitrary topography. Phys. Fluids 2013, 25, 043103–043121. [Google Scholar] [CrossRef]
- Tsai, C.-C.; Lin, Y.-T.; Hsu, T.-W. Propagating of obliquely incident, weakly viscous waves over variable bathymetry. J. Coast. Res. 2016, 32, 974–982. [Google Scholar] [CrossRef]
- Tsai, C.-C.; Tai, W.; Hsu, T.-W.; Hsiao, S.-C. Step approximation of water wave scattering caused by tension-leg structures over uneven bottoms. Ocean Eng. 2018, 166, 208–225. [Google Scholar] [CrossRef]
- Liu, X.; Wang, X.; Xu, S. A DMM-EMM-RSM hybrid technique on two-dimensional frequency-domain hydroelasticity of floating structures over variable bathymetry. Ocean Eng. 2020, 201, 107135. [Google Scholar] [CrossRef]
- Tsai, C.-C.; Chou, W.-R. Comparison between consistent coupled-mode system and eigenfunction matching method for solving water wave scattering. J. Mar. Sci. Tech. Taiw. 2015, 23, 870–881. [Google Scholar] [CrossRef]
- Katō, J.; Hagino, S.; Uekita, Y. Damping effect of floating breakwater to which anti-rolling system is applied. In Proceedings of the Tenth Conference Coastal Engineering, Tokyo, Japan, September 1966; pp. 1068–1078. [Google Scholar]
- Bai, K.J. Diffraction of oblique waves by an infinite cylinder. J. Fluid Mech. 1975, 68, 513–535. [Google Scholar] [CrossRef]
- Kanoria, M.; Dolai, D.P.; Mandal, B.N. Water-wave Scattering by Thick Vertical Barriers. J. Eng. Math. 1999, 35, 361–384. [Google Scholar] [CrossRef]
- Söylemez, M.; Gören, Ö. Diffraction of oblique waves by thick rectangular barriers. Appl. Ocean Res. 2003, 25, 345–353. [Google Scholar] [CrossRef]
- Garrison, C.J. Interaction of oblique waves with an infinite cylinder. Appl. Ocean Res. 1984, 6, 4–15. [Google Scholar] [CrossRef]
- Manisha; Kaligatla, R.B.; Sahoo, T. Effect of bottom undulation for mitigating wave-induced forces on a floating bridge. Wave Motion 2019, 89, 166–184. [Google Scholar] [CrossRef]
- Bragg, W.H.; Bragg, W.L. The reflection of X-rays by crystals. Proc. R. Soc. Lond. Ser. A 1913, 88, 428–438. [Google Scholar] [CrossRef]
- Mei, C.C. Resonant reflection of surface water waves by periodic sandbars. J. Fluid Mech. 1985, 152, 315–335. [Google Scholar] [CrossRef]
- Dalrymple, R.A.; Kirby, J.T. Water waves over ripples. J. Waterw. Port Coast. Ocean Eng. 1986, 112, 309–319. [Google Scholar] [CrossRef]
- Zettl, A. Sturm-Liouville Theory; American Mathematical Society: Rhode Island, RI, USA, 2005. [Google Scholar]
- Li, X.S. An overview of SuperLU: Algorithms, implementation, and user interface. ACM Trans. Math. Softw. 2005, 31, 302–325. [Google Scholar] [CrossRef]
- Lebreton, J.; Margnac, M. Traitement sur ordinateur de quelques problèmes concernant l’action de la houle sur les corps flottants en théorie bidimensionelle. In Bulletin du Centre de Recherches et D’Essais de Chatou; Chatou: Yvelines, France, 1966; pp. 3–43. [Google Scholar]
- Lee, C.-P.; Lee, J.-F. Wave-induced surge motion of a tension leg structure. Ocean Eng. 1993, 20, 171–186. [Google Scholar] [CrossRef]
- Lee, C.-P. Dragged surge motion of a tension leg structure. Ocean Eng. 1994, 21, 311–328. [Google Scholar] [CrossRef]
- Lee, J.-F. On the heave radiation of a rectangular structure. Ocean Eng. 1995, 22, 19–34. [Google Scholar] [CrossRef]
- Zheng, Y.H.; Shen, Y.M.; You, Y.G.; Wu, B.J.; Jie, D.S. On the radiation and diffraction of water waves by a rectangular structure with a sidewall. Ocean Eng. 2004, 31, 2087–2104. [Google Scholar] [CrossRef]








© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tseng, I.-F.; You, C.-S.; Tsai, C.-C. Bragg Reflections of Oblique Water Waves by Periodic Surface-Piercing and Submerged Breakwaters. J. Mar. Sci. Eng. 2020, 8, 522. https://doi.org/10.3390/jmse8070522
Tseng I-F, You C-S, Tsai C-C. Bragg Reflections of Oblique Water Waves by Periodic Surface-Piercing and Submerged Breakwaters. Journal of Marine Science and Engineering. 2020; 8(7):522. https://doi.org/10.3390/jmse8070522
Chicago/Turabian StyleTseng, I-Fan, Chi-Shian You, and Chia-Cheng Tsai. 2020. "Bragg Reflections of Oblique Water Waves by Periodic Surface-Piercing and Submerged Breakwaters" Journal of Marine Science and Engineering 8, no. 7: 522. https://doi.org/10.3390/jmse8070522
APA StyleTseng, I.-F., You, C.-S., & Tsai, C.-C. (2020). Bragg Reflections of Oblique Water Waves by Periodic Surface-Piercing and Submerged Breakwaters. Journal of Marine Science and Engineering, 8(7), 522. https://doi.org/10.3390/jmse8070522






