Reanalysis of the Sydney Harbor RiverCat Ferry
Abstract
:1. Introduction
1.1. Previous Studies
1.2. Current Investigation
2. Hydrodynamic Theory
2.1. Decomposition of Resistance
2.2. Wave Resistance
2.3. Hydrostatic Resistance
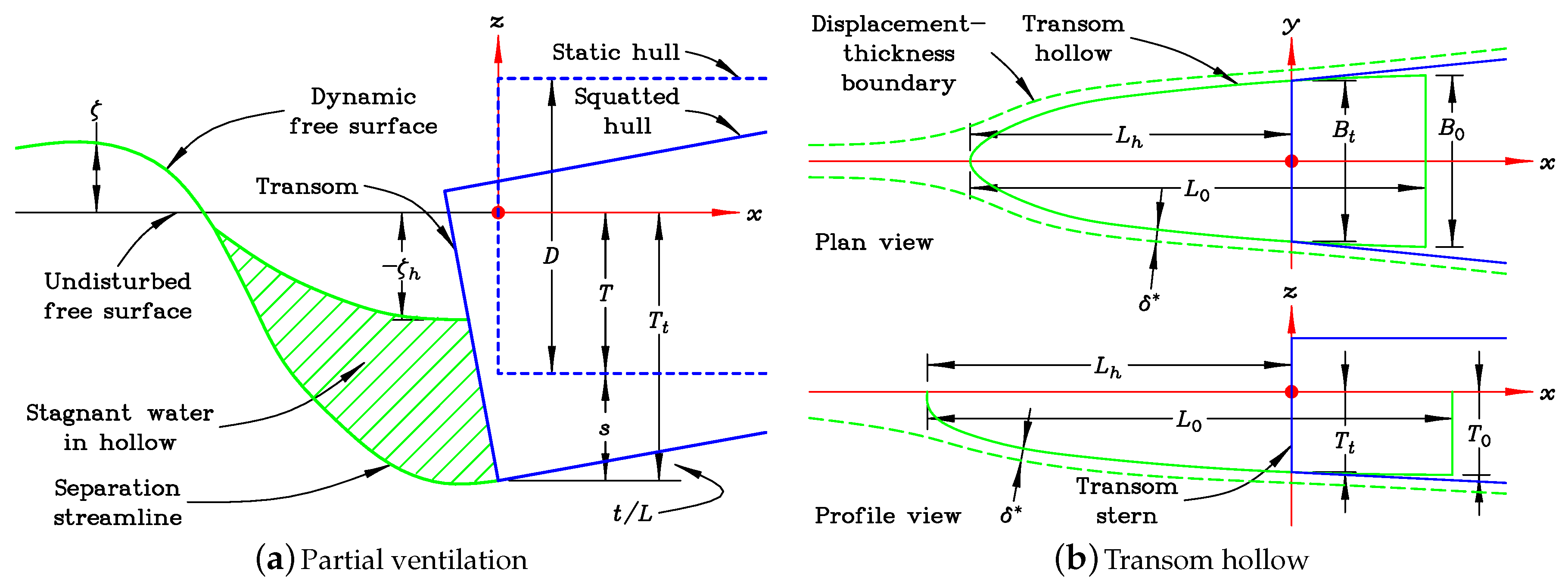
2.4. Transom Hollow
2.5. Frictional Resistance
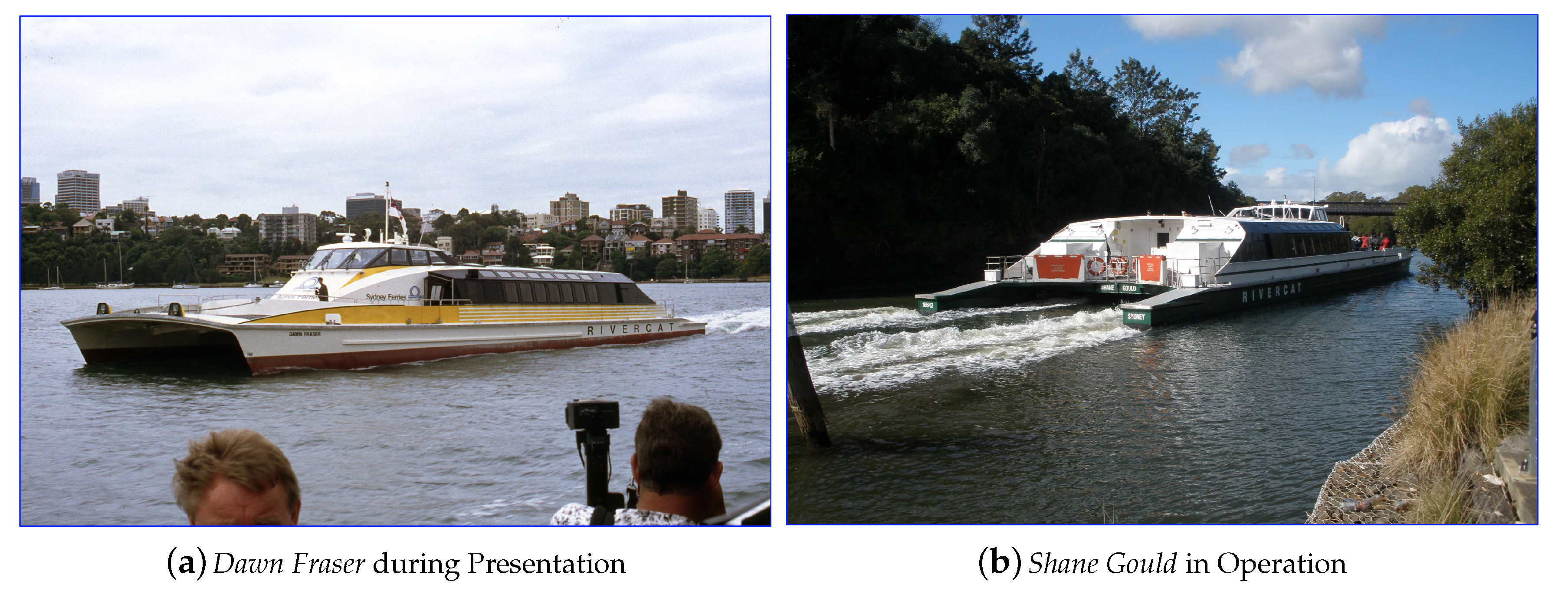
3. Characteristics of the RiverCat
3.1. General Layout
3.2. Demihulls
4. Numerical Computations
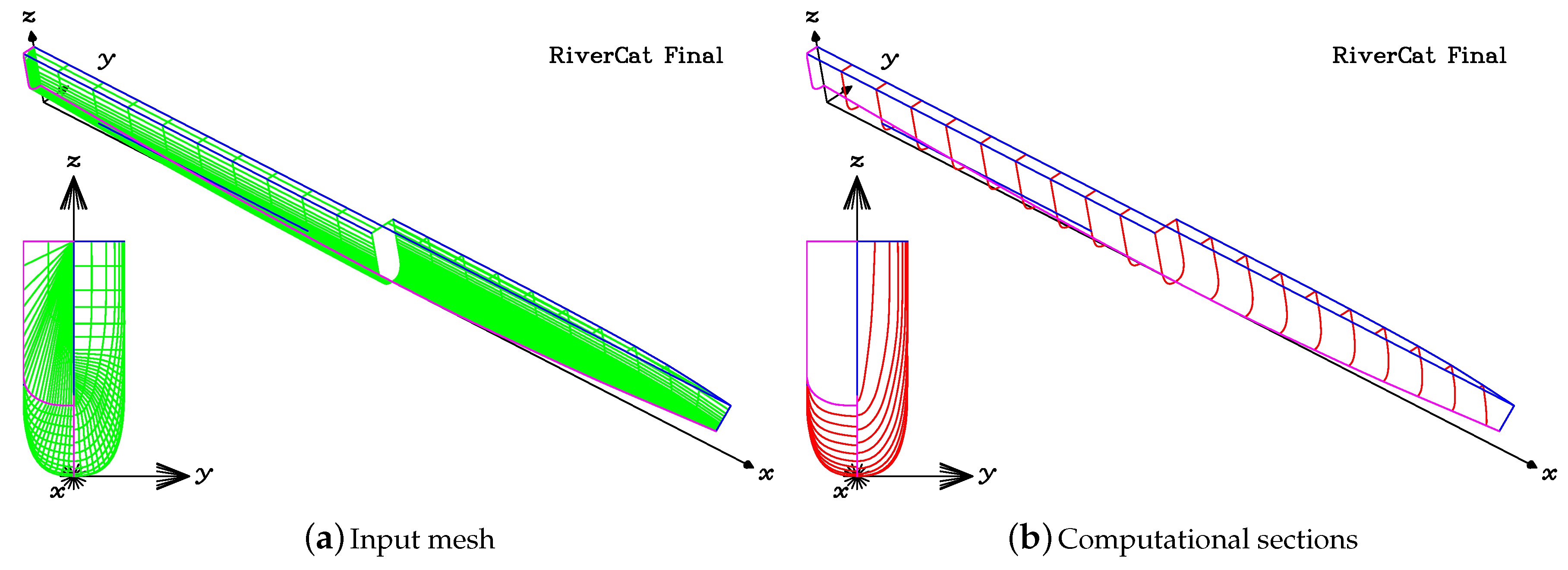
4.1. Finalized Vessel
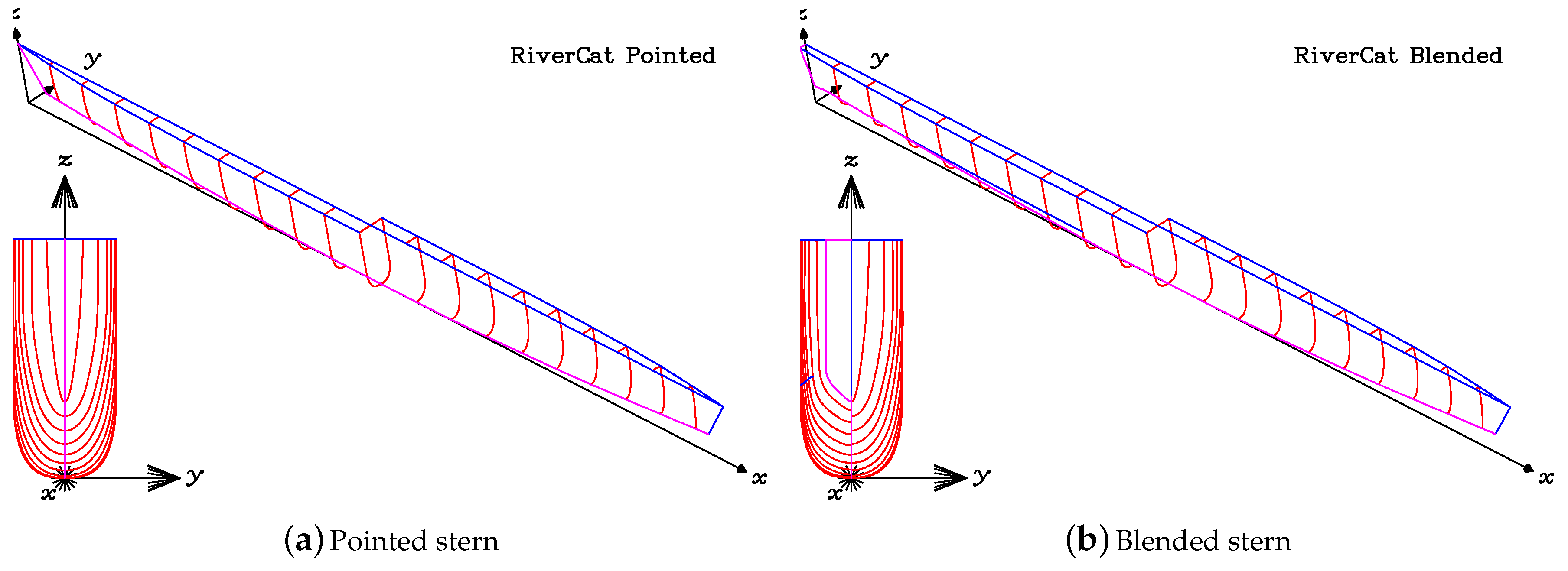
4.2. Transformations of the Finalized Demihull
4.3. Wave Resistance
4.4. Model Total Resistance
4.5. Prototype Total Resistance
4.6. Hull Finish
5. Conclusions
5.1. Current Investigation
5.2. Future Studies
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
List of Symbols
| Transom area | T | Draft | |
| Midship-section area | U | Ship velocity | |
| B | Overall beam | W | Displacement weight |
| Demihull beam | Demihull local beam | ||
| Correlation allowance | d | Depth of water | |
| Block coefficient | Frictional-resistance form factor | ||
| Prismatic coefficient | g | Acceleration due to gravity | |
| F | Froude number | k | Circular wave number |
| Depth Froude number | Longitudinal wave number | ||
| Volumetric Froude number | Transverse wave number | ||
| L | Length | s | Demihull centerplane separation |
| Prototype nominal length | w | Towing-tank or canal width | |
| Slenderness ratio | x | Longitudinal coordinate | |
| P | Power | Longitudinal coordinate at transom | |
| Correlation resistance | y | Transverse coordinate | |
| Frictional resistance | z | Vertical coordinate | |
| Hydrostatic resistance | Model displacement mass | ||
| Total resistance | Prototype displacement mass | ||
| Wave resistance | Free-surface elevation on face of transom | ||
| Aerodynamic resistance | Propulsion efficiency | ||
| Reynolds number | Kinematic viscosity of water | ||
| S | Overall wetted-surface area | Density of water | |
| Demihull wetted-surface area | ∇ | Displacement volume |
References
- Doctors, L.J.; Renilson, M.R.; Parker, G.; Hornsby, N. Waves and Wave Resistance of a High-Speed River Catamaran. In Proceedings of the First International Conference on Fast Sea Transportation (FAST ’91), Norges Tekniske Høgskole, Trondheim, Norway, June 1991; Volume 1, pp. 35–52. Available online: https://repository.tudelft.nl/islandora/object/uuid%3A133e695e-1a70-4a0d-b99a-17c25aeace92 (accessed on 8 February 2021).
- Hornsby, N.; Parker, G.; Doctors, L.J.; Renilson, M.R. The Design, Development, and Construction of a 35-Metre Low-Wash Fast Catamaran River Ferry. In Proceedings of the Sixth International Maritime and Shipping Conference (IMAS ’91), The University of New South Wales, Sydney, Australia, 11–13 November 1991; pp. 41–47. [Google Scholar]
- Renilson, M.R. Resistance Tests, Powering Estimates and Wake Wave Prediction for a 35 m Ferry; Report 89/T/13; Australian Maritime College, AMC Search Limited: Mowbray, TAS, Australia, 1989; p. 63+i. [Google Scholar]
- Doctors, L.J. On the Great Trimaran-Catamaran Debate. In Proceedings of the Fifth International Conference on Fast Sea Transportation (FAST ’99), Seattle, WA, USA, 31 August–2 September 1999; pp. 283–296. [Google Scholar]
- Froude, W. On Experiments with H.M.S. ‘Greyhound’. Trans. Inst. Nav. Archit. 1874, 15, 36–59. [Google Scholar]
- Michell, J.H. The Wave Resistance of a Ship. Philos. Mag. 1898, 45, 106–123. [Google Scholar] [CrossRef]
- Sretensky, L.N. On the Wave-Making Resistance of a Ship Moving along in a Canal. Philos. Mag. 1936, 22, 1005–1013. [Google Scholar] [CrossRef]
- Newman, J.N.; Poole, F.A.P. The Wave Resistance of a Moving Pressure Distribution in a Canal. Schiffstechnik 1962, 9, 21–26. [Google Scholar]
- Doctors, L.J.; Day, A.H. Resistance Prediction for Transom-Stern Vessels”. In Proceedings of the Fourth International Conference on Fast Sea Transportation (FAST ’97), Sydney, Australia, 21–23 July 1997; Volume 2, pp. 743–750. [Google Scholar]
- Doctors, L.J. Hydrodynamics of High-Performance Marine Vessels, 2nd ed.; CreateSpace: Charleston, SC, USA, 2018; Volume 1, pp. 1–421+li. [Google Scholar]
- Toby, A.S. The Evolution of Round Bilge Fast Attack Craft Hull Forms. Nav. Eng. J. 1987, 99, 52–62. [Google Scholar] [CrossRef]
- Toby, A.S. U.S. High Speed Destroyers, 1919–1942: Hull Proportions (to the Edge of the Possible). Nav. Eng. J. 1997, 109, 155–177. [Google Scholar] [CrossRef]
- Toby, A.S. The Edge of the Possible: U.S. High Speed Destroyers, 1919–1942. Part 2: Secondary Hull Form Parameters. Nav. Eng. J. 2002, 114, 55–76. [Google Scholar] [CrossRef]
- Clements, R.E. An Analysis of Ship-Model Correlation Data Using the 1957 I.T.T.C. Line. Trans. R. Inst. Nav. Archit. 1959, 101, 373–385. [Google Scholar]
- Lewis, E.V.Q.Q. Principles of Naval Architecture: Volume II. Resistance, Propulsion and Vibration; Society of Naval Architects and Marine Engineers: Jersey City, NJ, USA, 1988; p. 327+vi. [Google Scholar]
- de Vahl Davis, G. Numerical Methods in Engineering and Science; Allen & Unwin (Publishers) Ltd.: London, UK, 1986; pp. 286+xvi. [Google Scholar]
- Day, A.H.; Clelland, D.; Doctors, L.J. Unsteady Finite-Depth Effects during Resistance Tests in a Towing Tank. J. Mar. Sci. Technol. 2009, 14, 387–397. [Google Scholar] [CrossRef]
- Doctors, L.J. A Versatile Hull-Generator Program. In Proceedings of the Twenty-First Century Shipping Symposium, The University of New South Wales, Sydney, Australia, 6 November 1995; pp. 140–158. [Google Scholar]
- Oosterveld, M.W.C.Q.Q. Report of Performance Committee. In Proceedings of the Fifteenth International Towing Tank Conference, The Hague, The Netherlands, September 1978; pp. 359–404. Available online: https://repository.tudelft.nl/islandora/object/uuid%3Ad7ad3bc5-40f8-42b1-a997-be238d791b56 (accessed on 8 February 2021).
- ITTC. Procedure 7.5-02-03-01.4—Recommended Procedures: Performance, Propulsion 1978 ITTC Performance Prediction Method. In Proceedings of the Twenty-Second International Towing Tank Conference, Seoul, Japan, Shanghai, China, 5–11 September 1999; p. 31. [Google Scholar]
- Candries, M.; Atlar, M. On the Drag and Roughness Characteristics of Antifoulings. Trans. R. Inst. Nav. Archit. 2003, 145, 107–132. [Google Scholar]
- ITTC. Procedure 7.5-03-03-01 Recommended Procedures and Guidelines: Practical Guidelines for Ship Self-Propulsion CFD. In Proceedings of the Twenty-Seventh International Towing Tank Conference, Copenhagen, Denmark, 31 August–5 September 2014; p. 9. [Google Scholar]







| Quantity | Symbol * | Value |
|---|---|---|
| Length on waterline | L | 35.00 m |
| Demihull beam | 1.000 m | |
| Beam overall | B | 10.06 m |
| Draft | T | 1.226 m |
| Block coefficient | 0.6262 | |
| Prismatic coefficient | 0.6958 | |
| Slenderness ratio | 11.68 | |
| Transom–area ratio | 0.4311 | |
| Displacement mass | 55.00 t | |
| Power | P | kW |
| Speed | U | 23 kn |
| Index | Parameter | Symbol | Values | ||
|---|---|---|---|---|---|
| Affine 0 | Affine 1 | Affine 2 | |||
| 1 * | Slenderness ratio | 11.68 | 14.60 | 17.52 | |
| 2 | Beam-to-draft ratio | 0.8159 | 1.275 | 1.836 | |
| 3 | Demihull separation | 0.2589 | 0.3160 | 0.3731 | |
| 4 | Transom-area ratio | 0.4311 | 0.2156 | 0 | |
| Assumption | Transport Factor TF * |
|---|---|
| ITTC (1957) with = 0 | 11.03 |
| ITTC (1957) with = 0.0004 | 09.52 |
| Measured | 09.52 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Doctors, L.J. Reanalysis of the Sydney Harbor RiverCat Ferry. J. Mar. Sci. Eng. 2021, 9, 215. https://doi.org/10.3390/jmse9020215
Doctors LJ. Reanalysis of the Sydney Harbor RiverCat Ferry. Journal of Marine Science and Engineering. 2021; 9(2):215. https://doi.org/10.3390/jmse9020215
Chicago/Turabian StyleDoctors, Lawrence J. 2021. "Reanalysis of the Sydney Harbor RiverCat Ferry" Journal of Marine Science and Engineering 9, no. 2: 215. https://doi.org/10.3390/jmse9020215
APA StyleDoctors, L. J. (2021). Reanalysis of the Sydney Harbor RiverCat Ferry. Journal of Marine Science and Engineering, 9(2), 215. https://doi.org/10.3390/jmse9020215






