Coupled Interactions Analysis of a Floating Tidal Current Power Station in Uniform Flow
Abstract
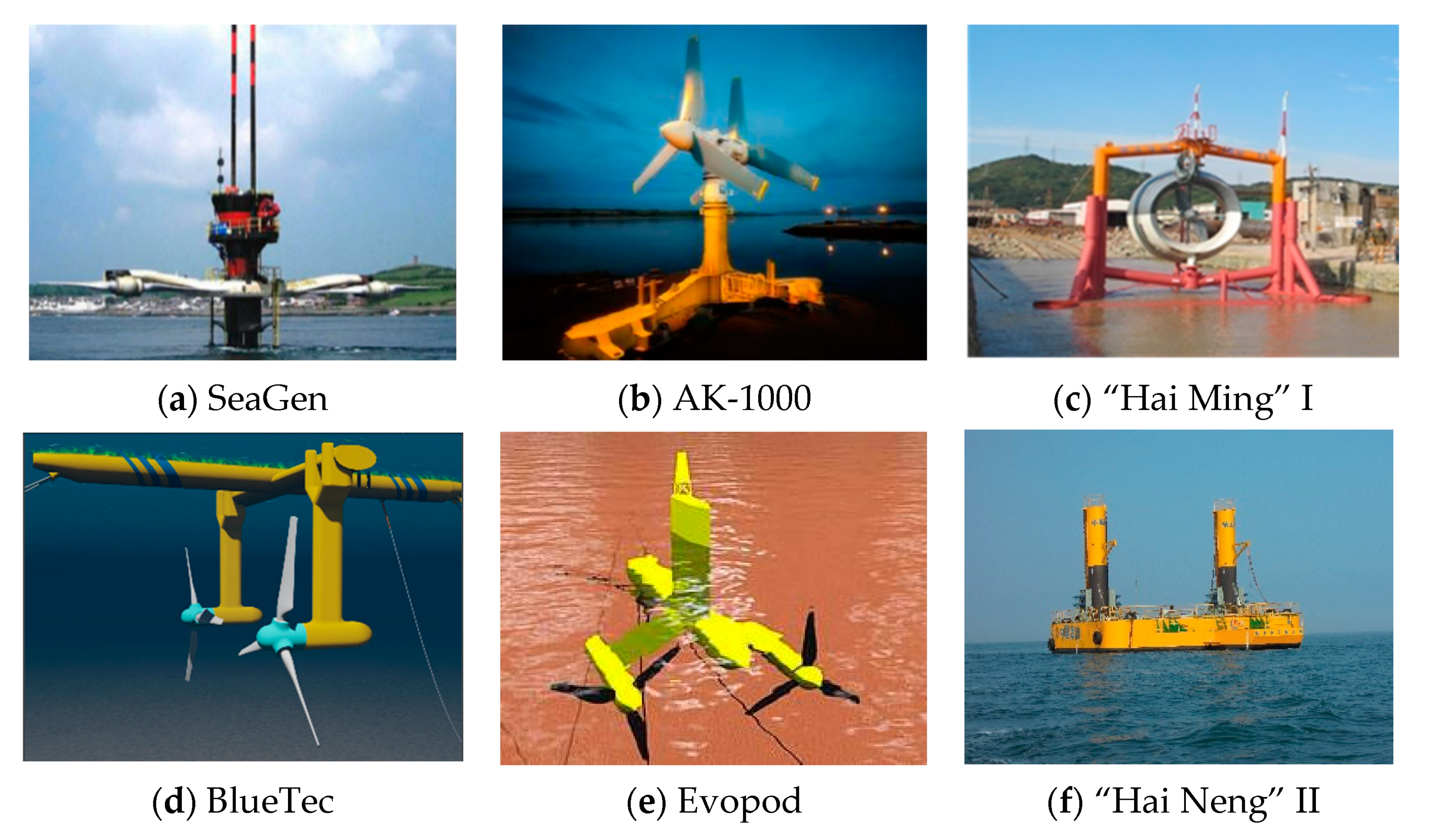
:1. Introduction
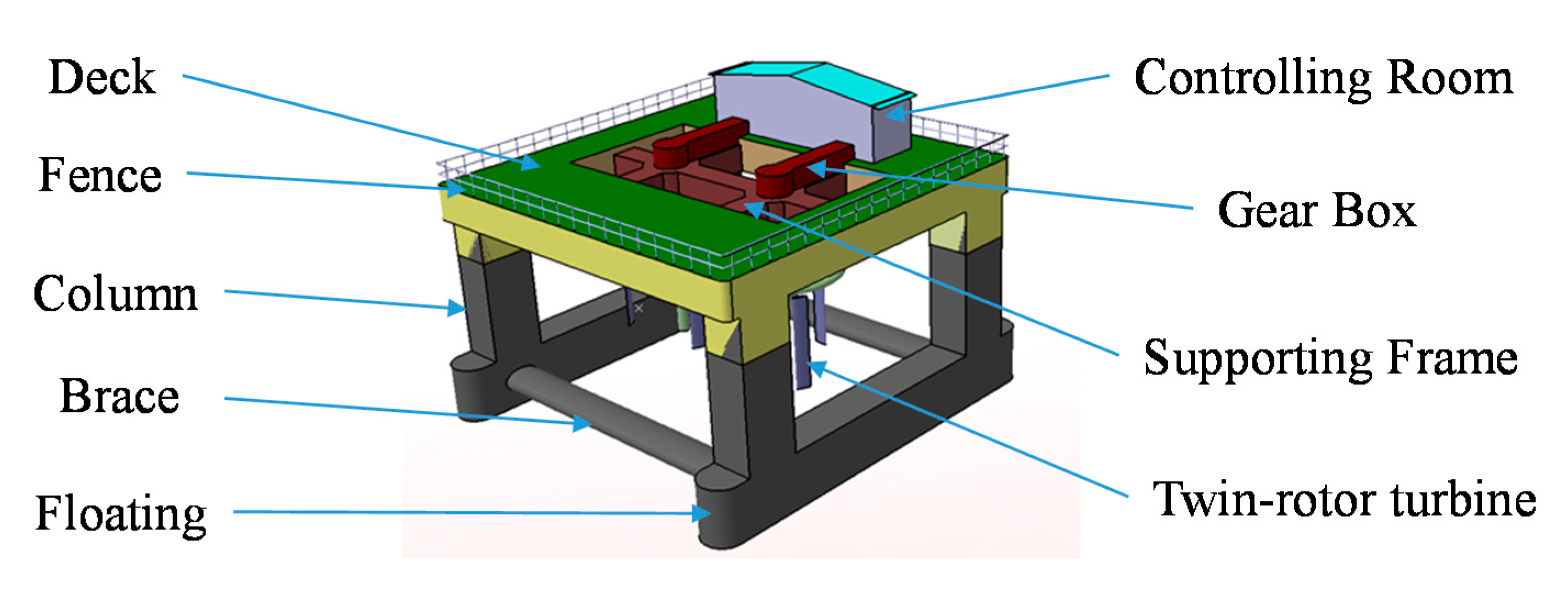
2. Floating Tidal Current Power Station Design
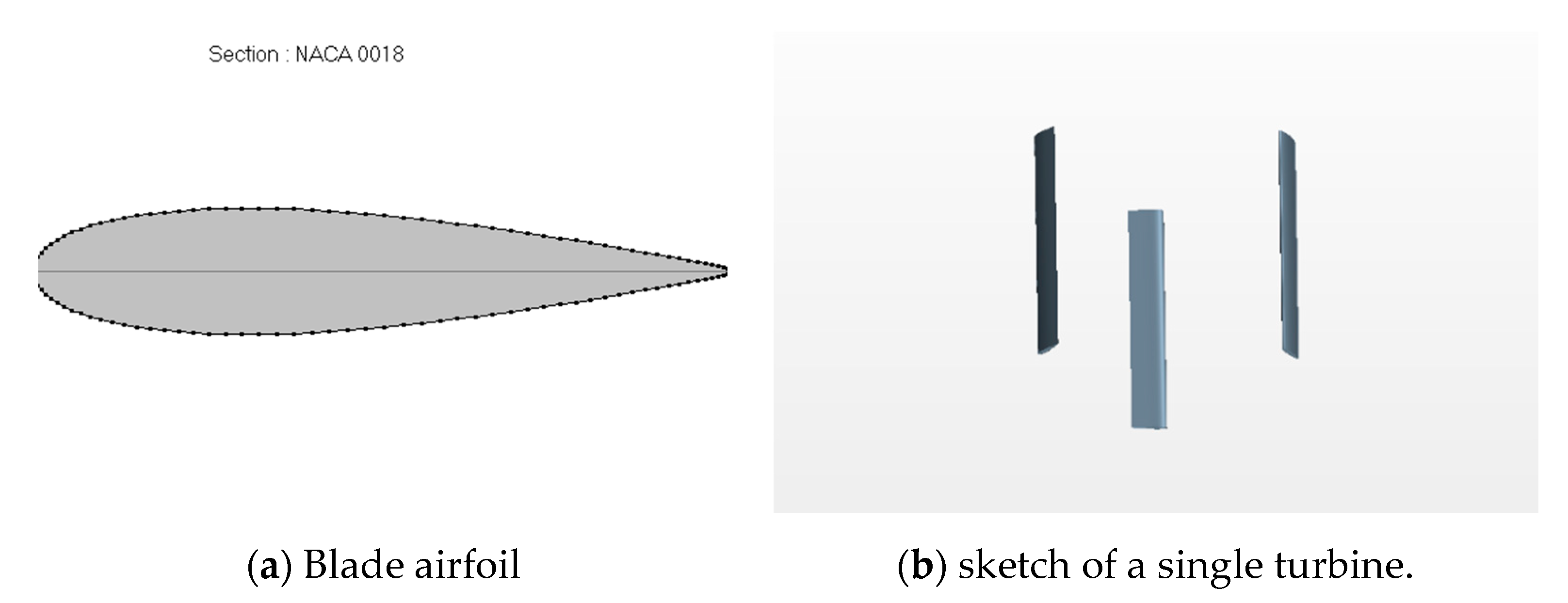
3. Numerical Research on the Performance of Vertical-Axis Twin-Rotors Tidal Current Turbine
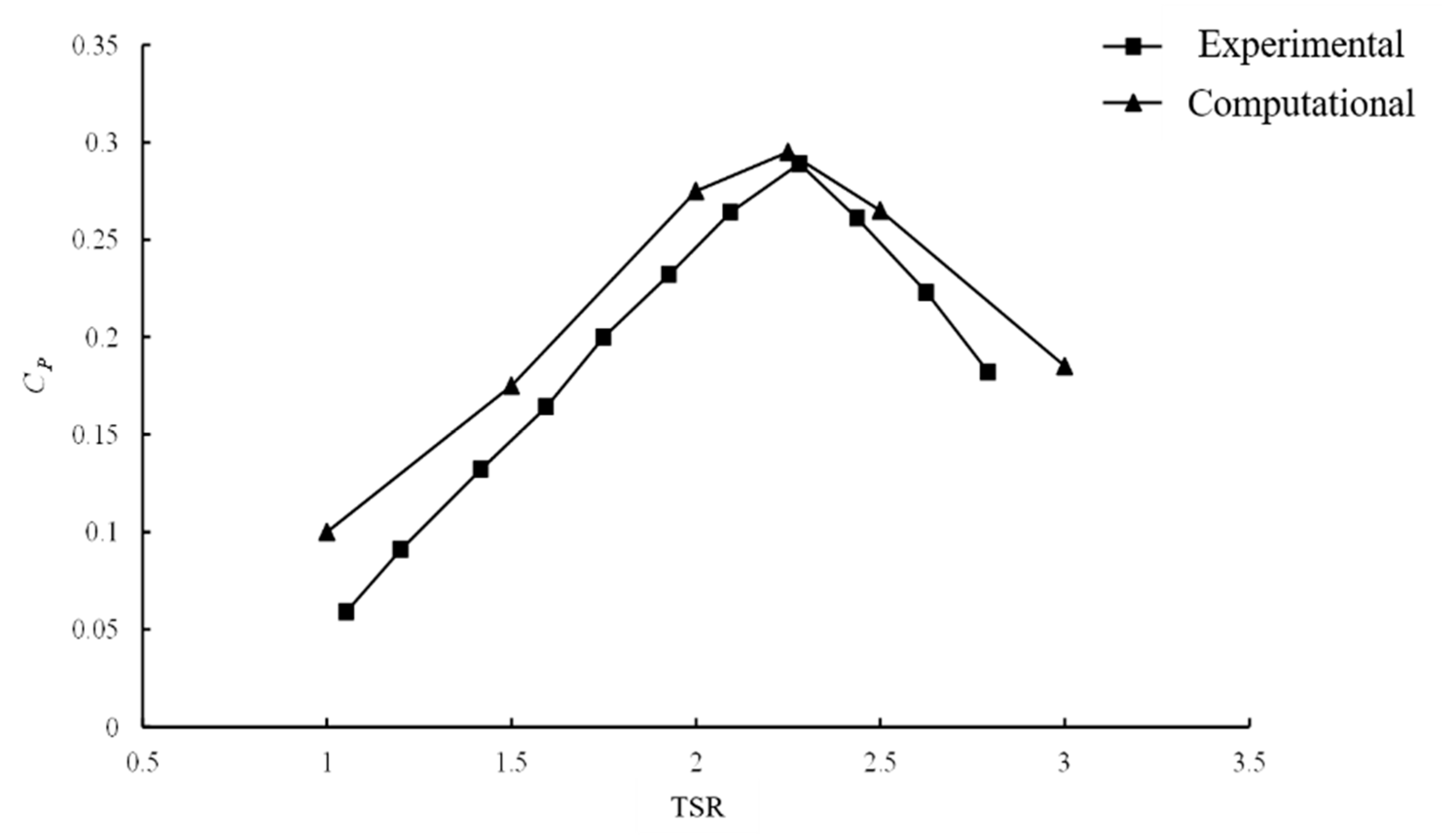
3.1. Numerical Verification of CFD Simulation
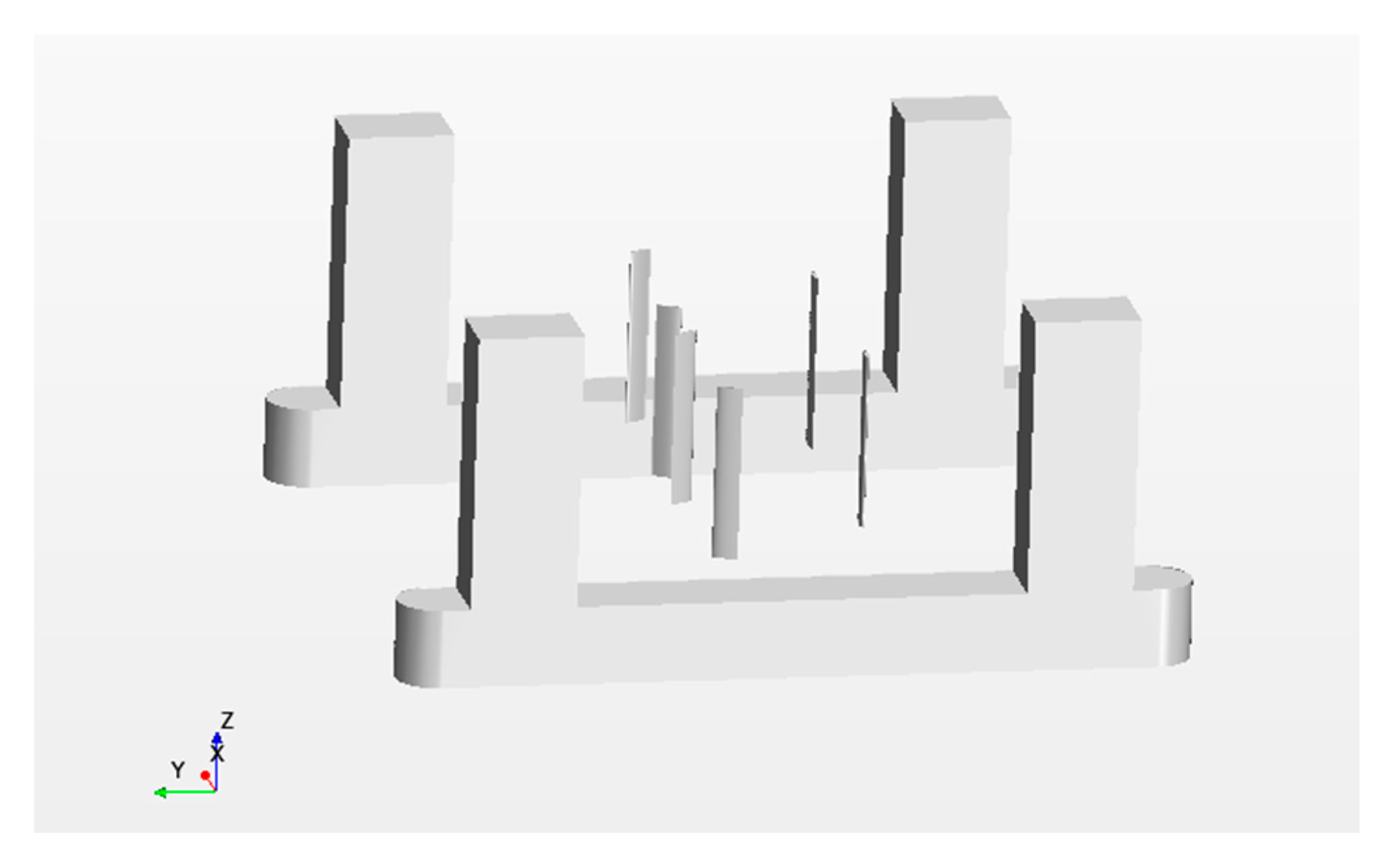
3.2. Computational Domain Settings
3.3. Validation of the Computational Model
4. Results and Discussions
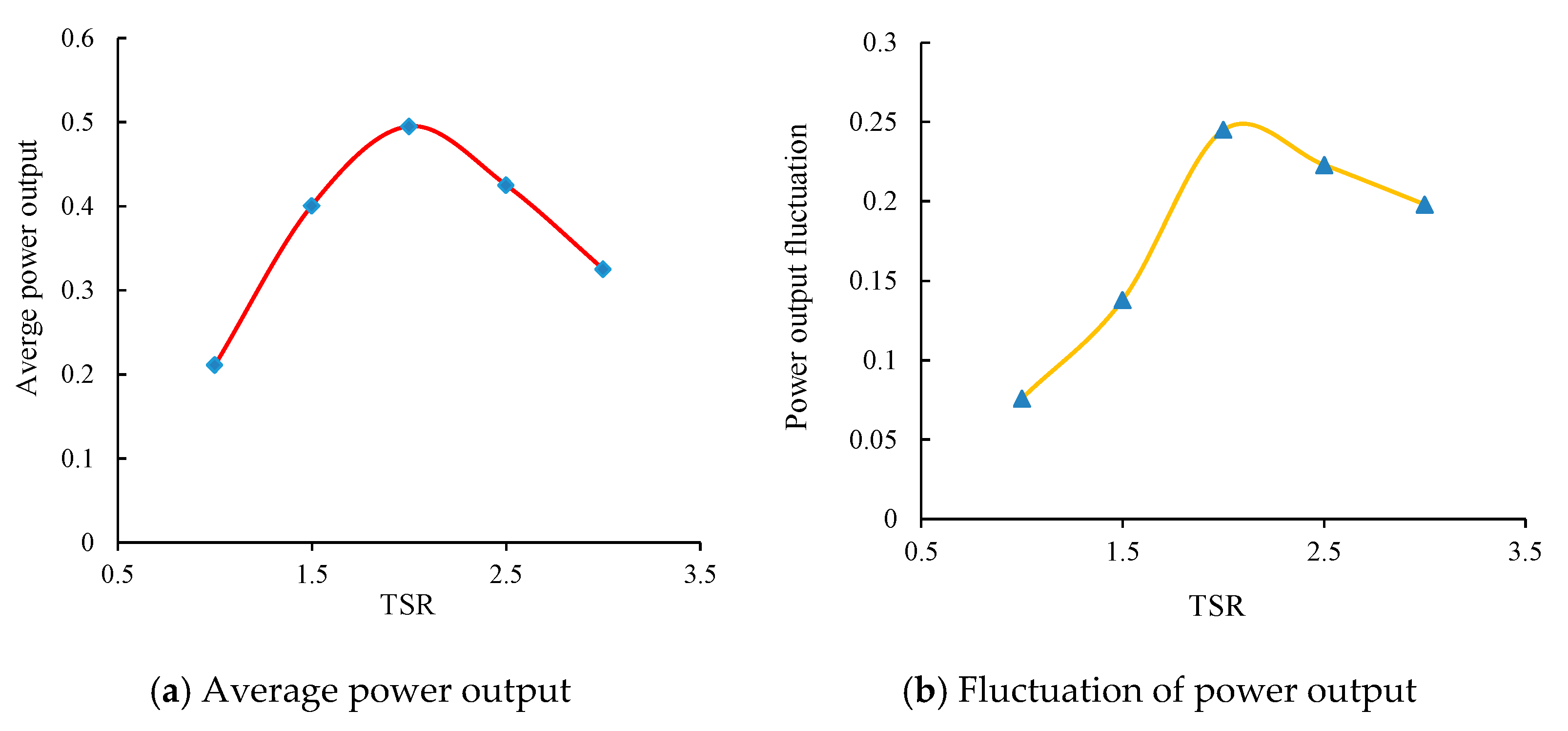
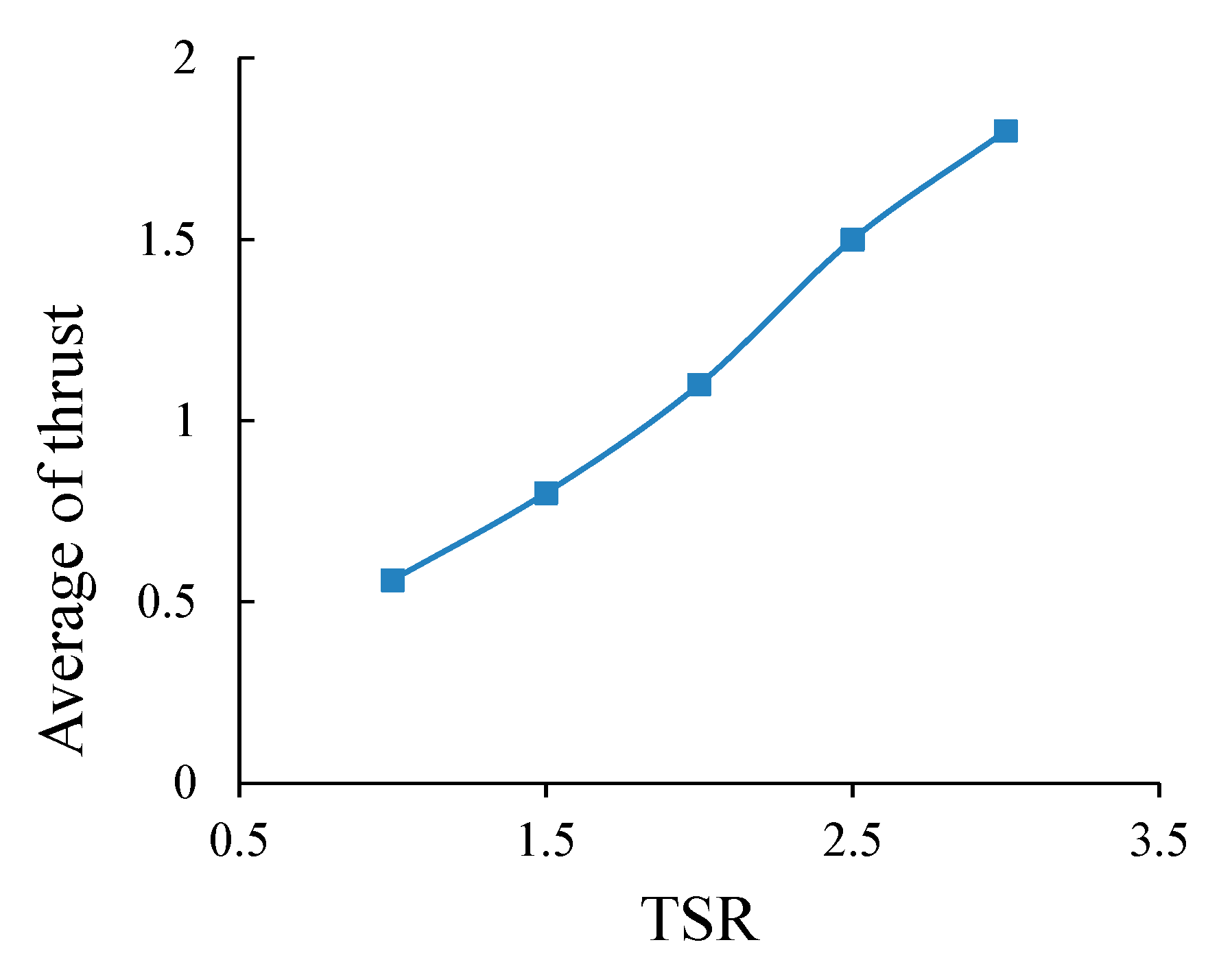
4.1. Influence of Speed Ratio on the Hydrodynamic Characteristics of Hydraulic Turbine
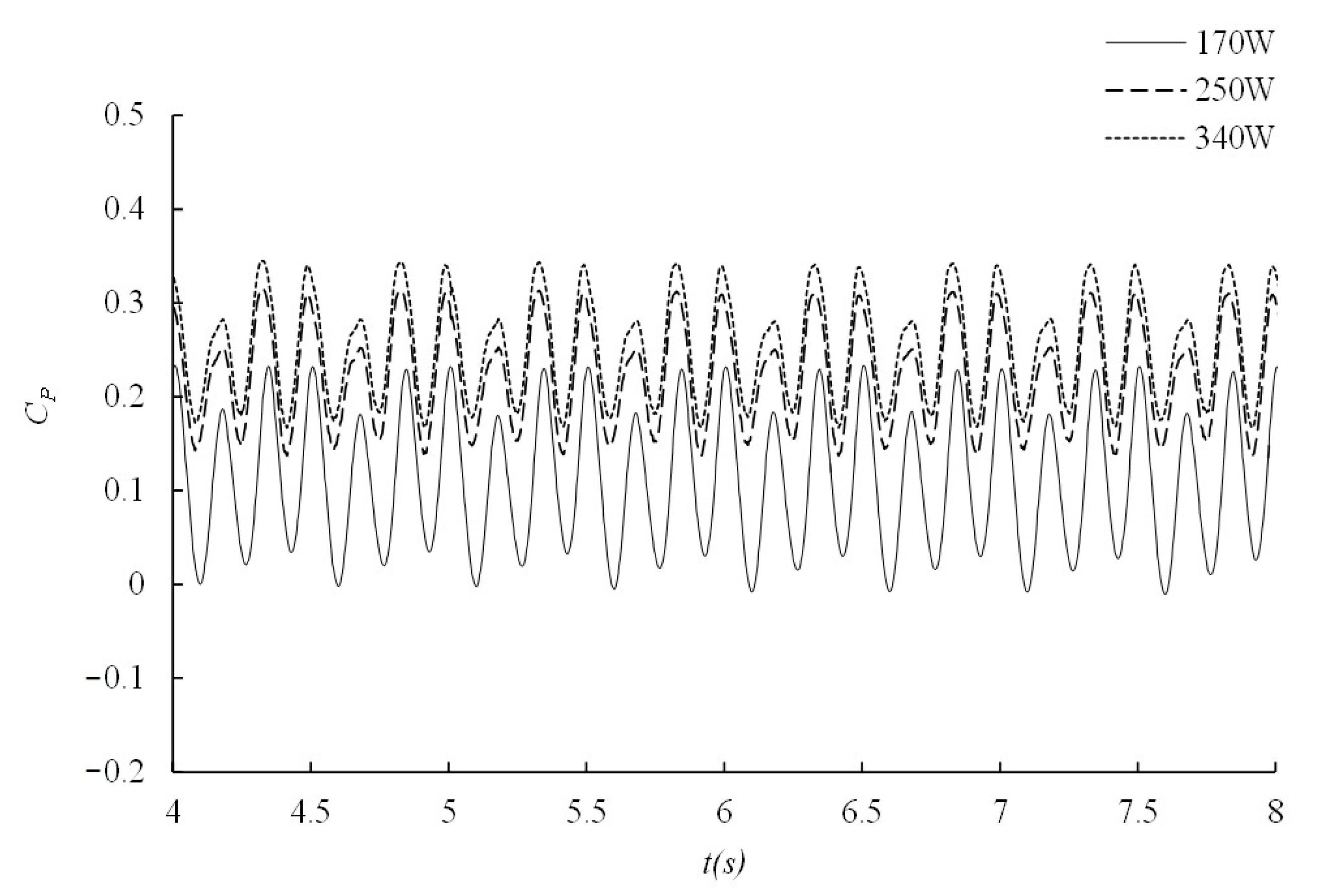
4.1.1. Power Output
4.1.2. Thrust Coefficient
4.1.3. Lateral Force Coefficient
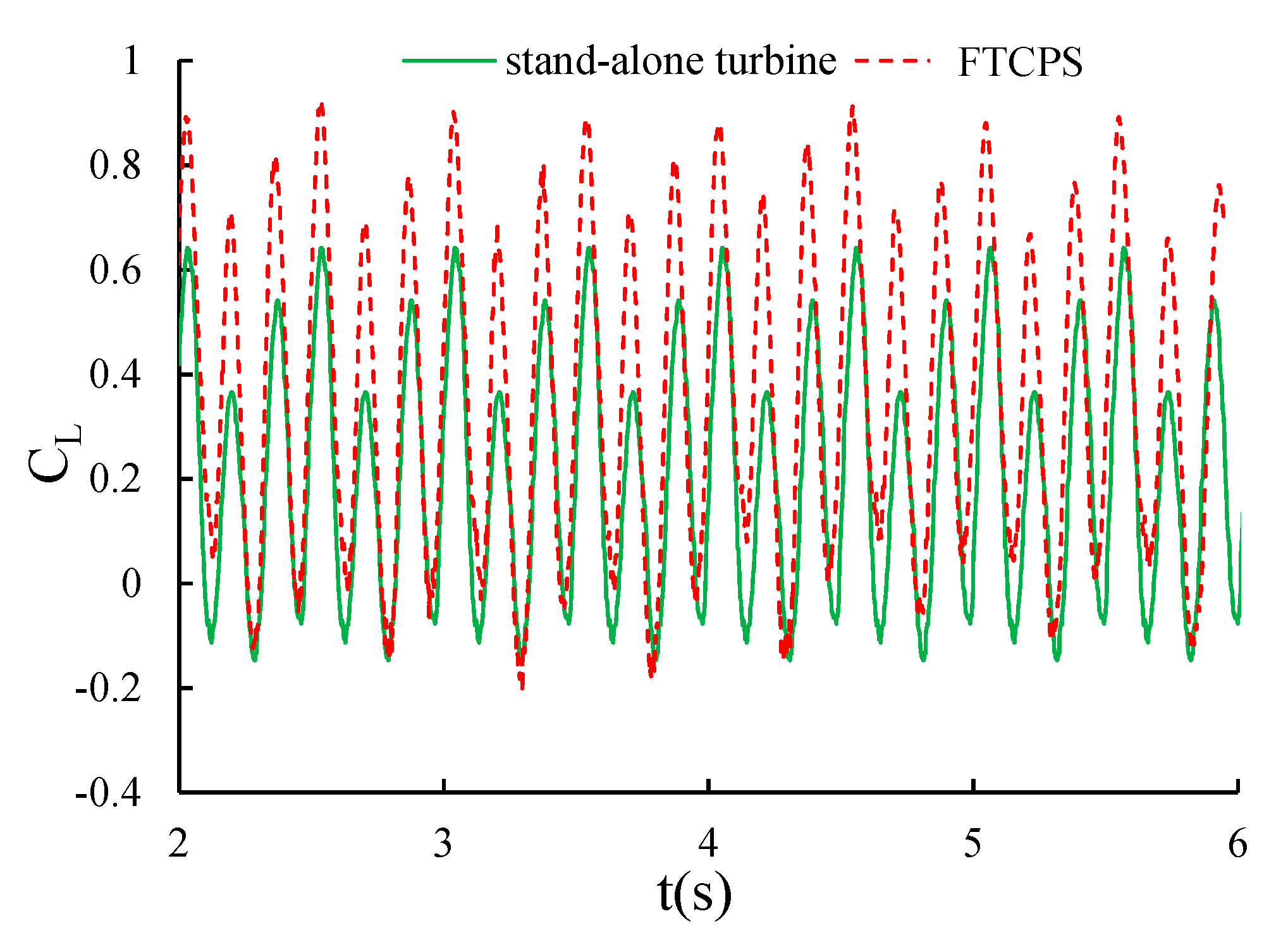
4.2. Influence of Carrier Motion on the Hydrodynamic Characteristics of Hydraulic Turbine
4.2.1. Power Output
4.2.2. Thrust Coefficient
4.2.3. Lateral Force Coefficient
4.3. Influence of the Turbine on the Hydrodynamic Response of the Carrier
4.3.1. Surge Motion of the Carrier
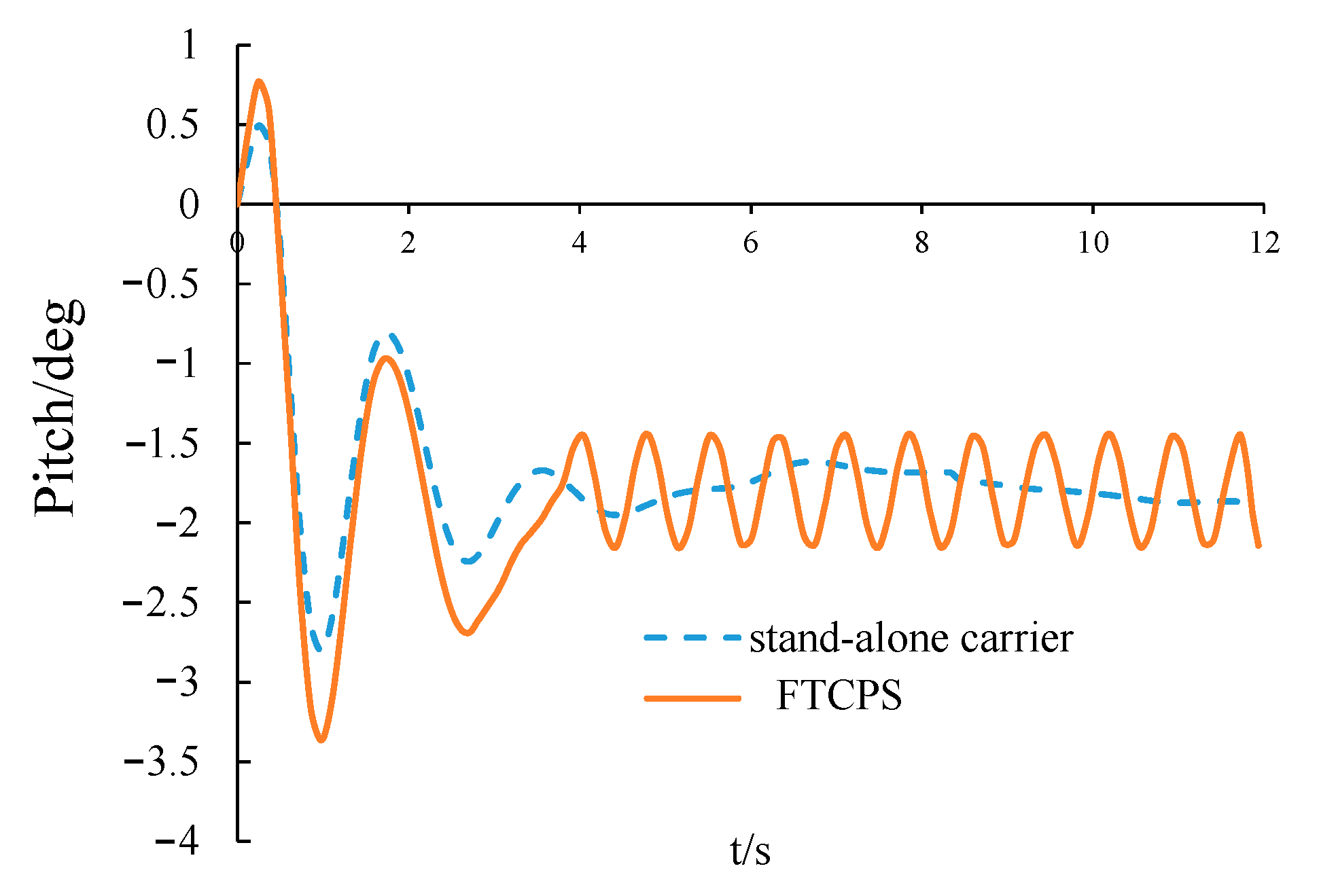
4.3.2. Pitch Motion of the Carrier
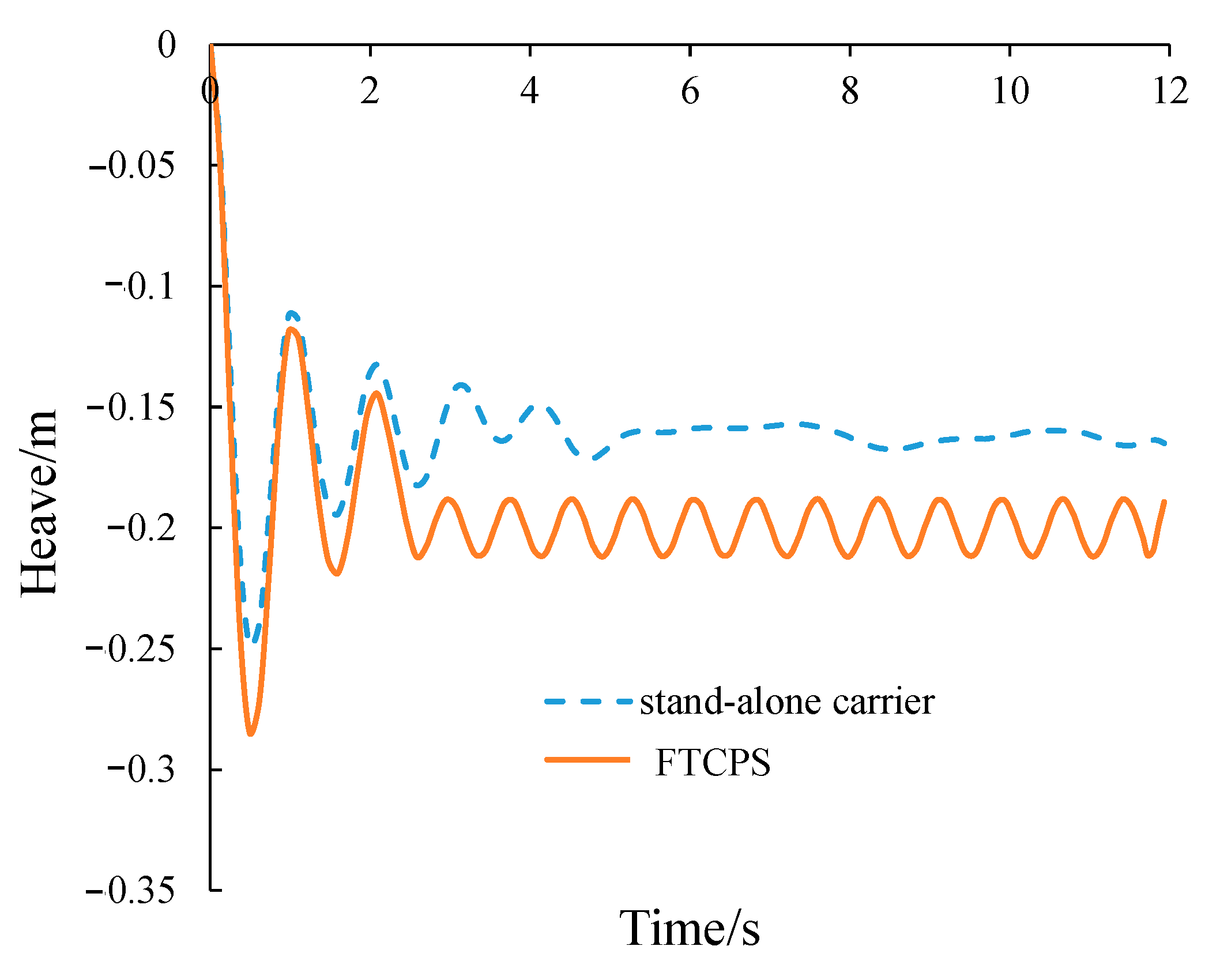
4.3.3. Heave Motion of the Carrier
4.4. Flow Field Analysis
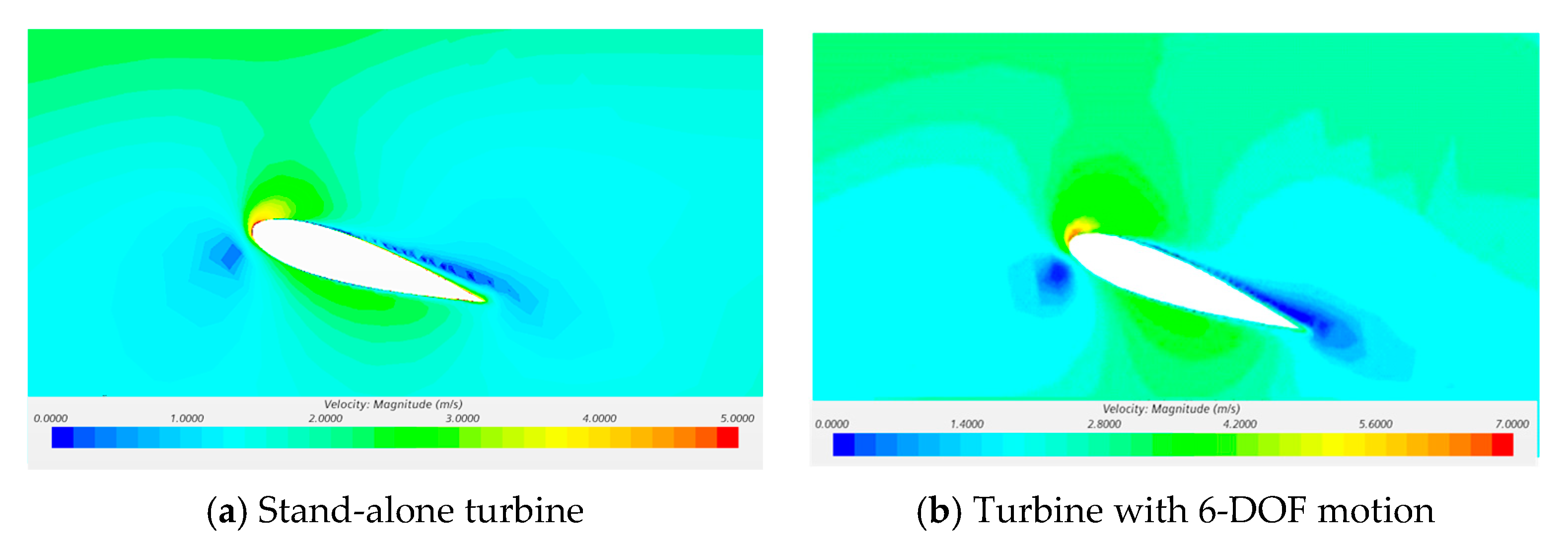
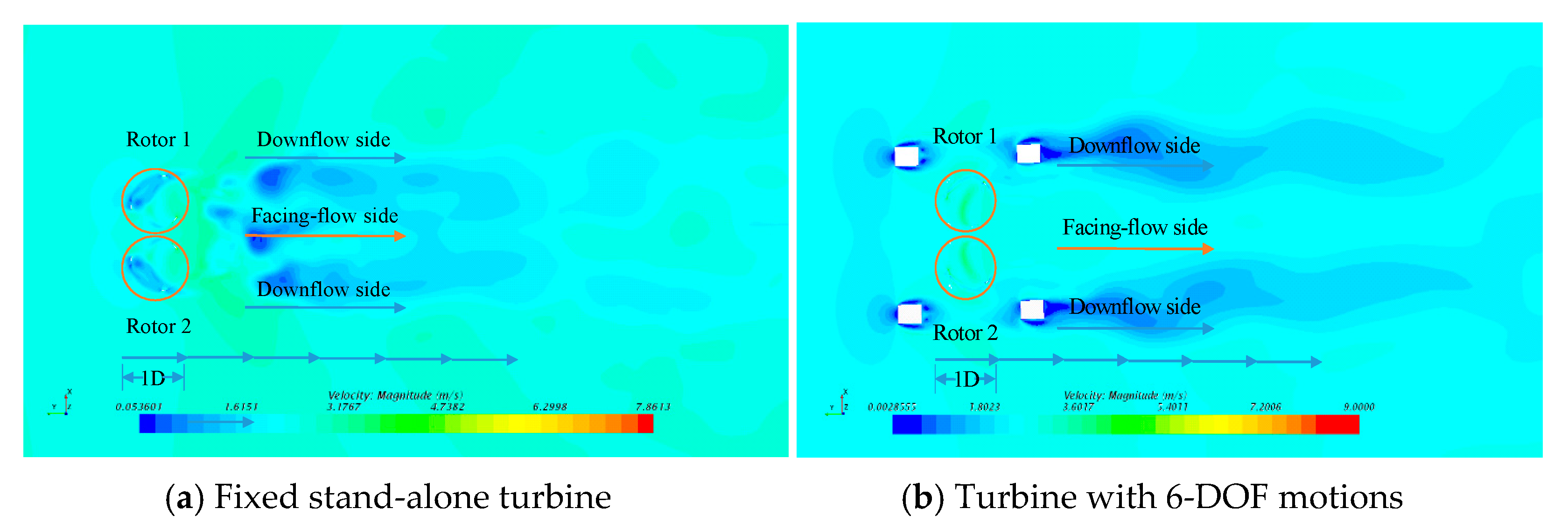
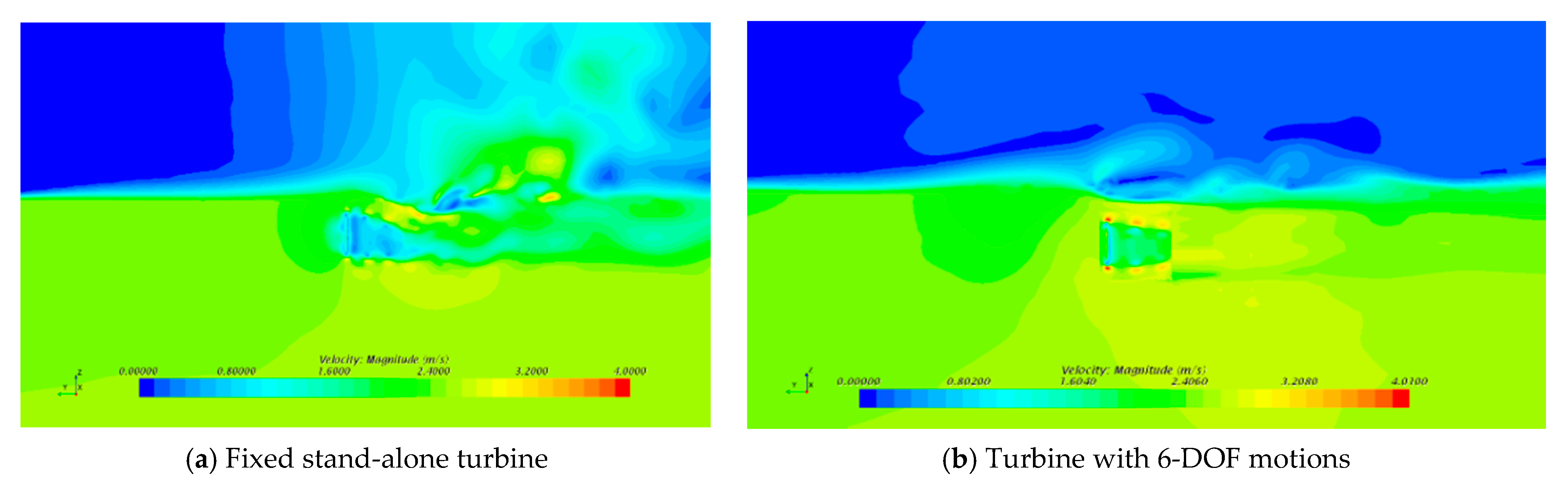
4.4.1. Flow Velocity
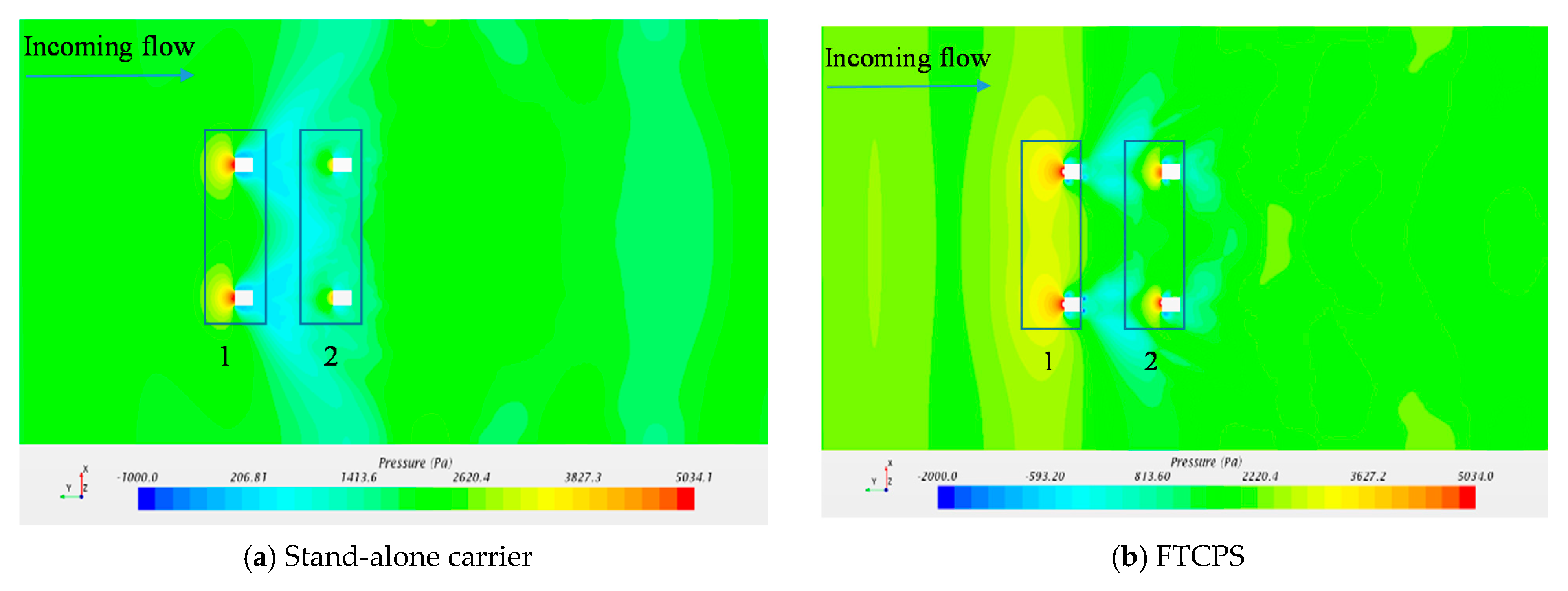
4.4.2. Flow Pressure
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khan, N.; Kalair, A.; Abas, N.; Haider, A. Review of ocean tidal, wave and thermal energy technologies. Renew. Sustain. Energy Rev. 2017, 72, 590–604. [Google Scholar] [CrossRef]
- Nachtane, M.; Tarfaoui, M.; Goda, I.; Rouway, M. A review on the technologies, design considerations and numerical models of tidal current turbines. Renew. Energy 2020, 157, 1274–1288. [Google Scholar] [CrossRef]
- Zhang, L.; Li, X.; Jing, G.; Zhang, X. Tidal enery updates 2013. Adv. New Renew. Energy 2013, 1, 53–68. [Google Scholar]
- Zhou, Z.; Benbouzid, M.; Charpentier, J.-F.; Scuiller, F.; Tang, T. Developments in large marine current turbine technologies—A review. Renew. Sustain. Energy Rev. 2017, 71, 852–858. [Google Scholar] [CrossRef]
- Ma, Y.; Li, B.; Xu, Y.; Dong, Y. Design and structural strength analysis of the gate-type tidal current energy converter. In International Conference on Offshore Mechanics and Arctic Engineering; American Society of Mechanical Engineers: New York, NY, USA, 2016. [Google Scholar]
- Uihlein, A.; Magagna, D. Wave and tidal current energy—A review of the current state of research beyond technology. Renew. Sustain. Energy Rev. 2016, 58, 1070–1081. [Google Scholar] [CrossRef]
- Jing, F.; Sheng, Q.; Zhang, L. Experimental research on tidal current vertical axis turbine with variable-pitch blades. Ocean Eng. 2014, 88, 228–241. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, J.; Lin, X.; Wang, R.; Zhang, C.; Zhao, J. Experimental investigation into downstream field of a horizontal axis tidal stream turbine supported by a mono pile. Appl. Ocean Res. 2020, 101, 102257. [Google Scholar] [CrossRef]
- Tran, T.; Kim, D.; Song, J. Computational Fluid Dynamic Analysis of a Floating Offshore Wind Turbine Experiencing Platform Pitching Motion. Energies 2014, 7, 5011–5026. [Google Scholar] [CrossRef]
- Fu, S.; Jin, Y.; Zheng, Y.; Chamorro, L. Wake and power fluctuations of a model wind turbine subjected to pitch and roll oscillations. Appl. Energy 2019, 253, 113605. [Google Scholar] [CrossRef]
- Tran, T.; Kim, D.-H. Fully coupled aero-hydrodynamic analysis of a semi-submersible FOWT using a dynamic fluid body interaction approach. Renew. Energy 2016, 92, 244–261. [Google Scholar] [CrossRef]
- Guo, X.; Yang, J.; Lu, W.; Li, X. Dynamic responses of a floating tidal turbine with 6-DOF prescribed floater motions. Ocean Eng. 2018, 165, 426–437. [Google Scholar] [CrossRef]
- Guo, X.; Yang, J.; Li, X.; Lu, W.; Peng, T.; Li, J. Dynamic responses of a floating tidal turbine considering prescribed wave-frequency motion. In International Conference on Offshore Mechanics and Arctic Engineering; American Society of Mechanical Engineers: New York, NY, USA, 2018; Volume 10. [Google Scholar]
- Brown, S.; Ransley, E.; Greaves, D. Developing a coupled turbine thrust methodology for floating tidal stream concepts: Verification under prescribed motion. Renew. Energy 2020, 147, 529–540. [Google Scholar] [CrossRef]
- Wang, S.-Q.; Sun, K.; Zhang, J.-H.; Zhang, L. The effects of roll motion of the floating platform on hydrodynamics performance of horizontal-axis tidal current turbine. J. Mar. Sci. Technol. 2017, 22, 259–269. [Google Scholar] [CrossRef]
- Wang, K.; Sun, K.; Sheng, Q.-H.; Zhang, L.; Wang, S.-Q. The effects of yawing motion with different frequencies on the hydrodynamic performance of floating vertical-axis tidal current turbines. Appl. Ocean Res. 2016, 59, 224–235. [Google Scholar] [CrossRef] [Green Version]
- Xu, G.; Sun, S.; Liu, F. Motion response analysis of floating tidal current power station. Zhong Guo Shui Yun. J. 2015, 15, 137–140. [Google Scholar]
- Lynch, C.; Smith, M. Unstructured overset incompressible computational fluid dynamics for unsteady wind turbine simulations. Wind. Energy 2012, 16, 1033–1048. [Google Scholar] [CrossRef]
- Rogers, S.E.; Suhs, N.E.; Dietz, W.E. PEGASUS 5: An Automated Preprocessor for Overset-Grid Computational Fluid Dynamics. AIAA J. 2003, 41, 1037–1045. [Google Scholar] [CrossRef]
- Park, S.; Jeun, G. Coupling of rigid body dynamics and moving particle semi-implicit method for simulating isothermal multi-phase fluid interactions. Comput. Methods Appl. Mech. Eng. 2011, 200, 130–140. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics Free Boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Xie, Y. Hydrodynamic Performance Research and Experiment of Vertical Axis Tidal Current Turbine; Dalian University of Technology: Dalian, China, 2016. [Google Scholar]
- Zhang, Y.; Zhang, Z.; Zheng, J.; Zhang, J.; Zheng, Y.; Zang, W.; Lin, X.; Fernandez-Rodriguez, E. Experimental investigation into effects of boundary proximity and blockage on horizontal-axis tidal turbine wake. Ocean Eng. 2021, 225, 108829. [Google Scholar] [CrossRef]
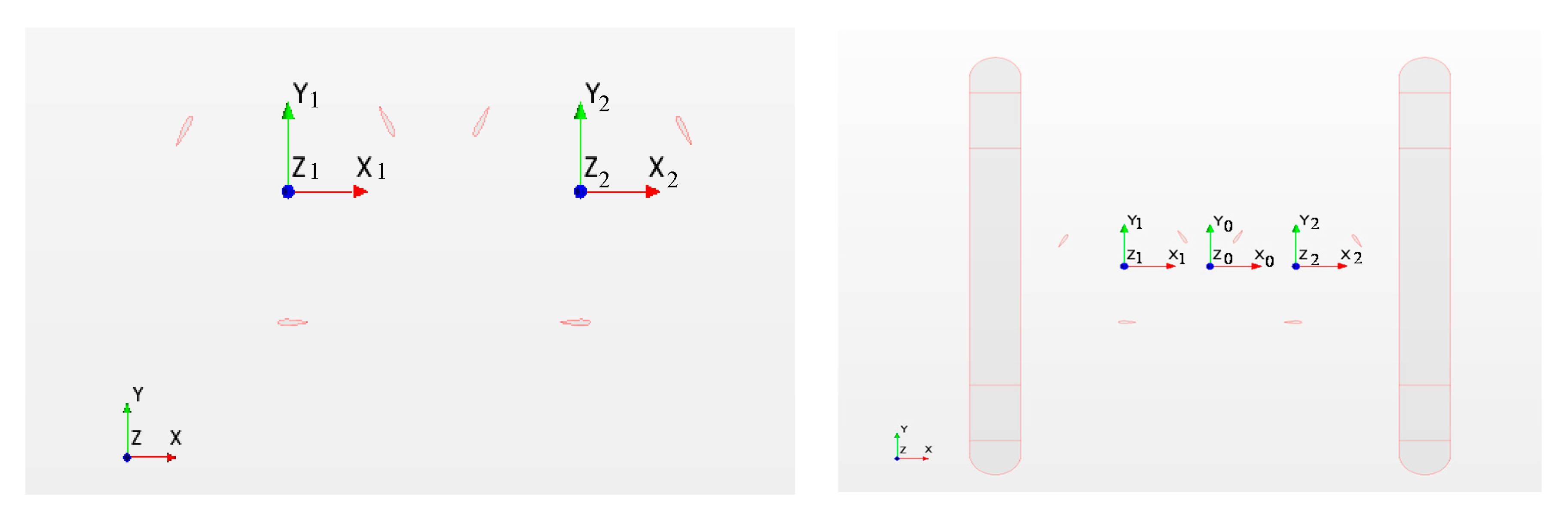

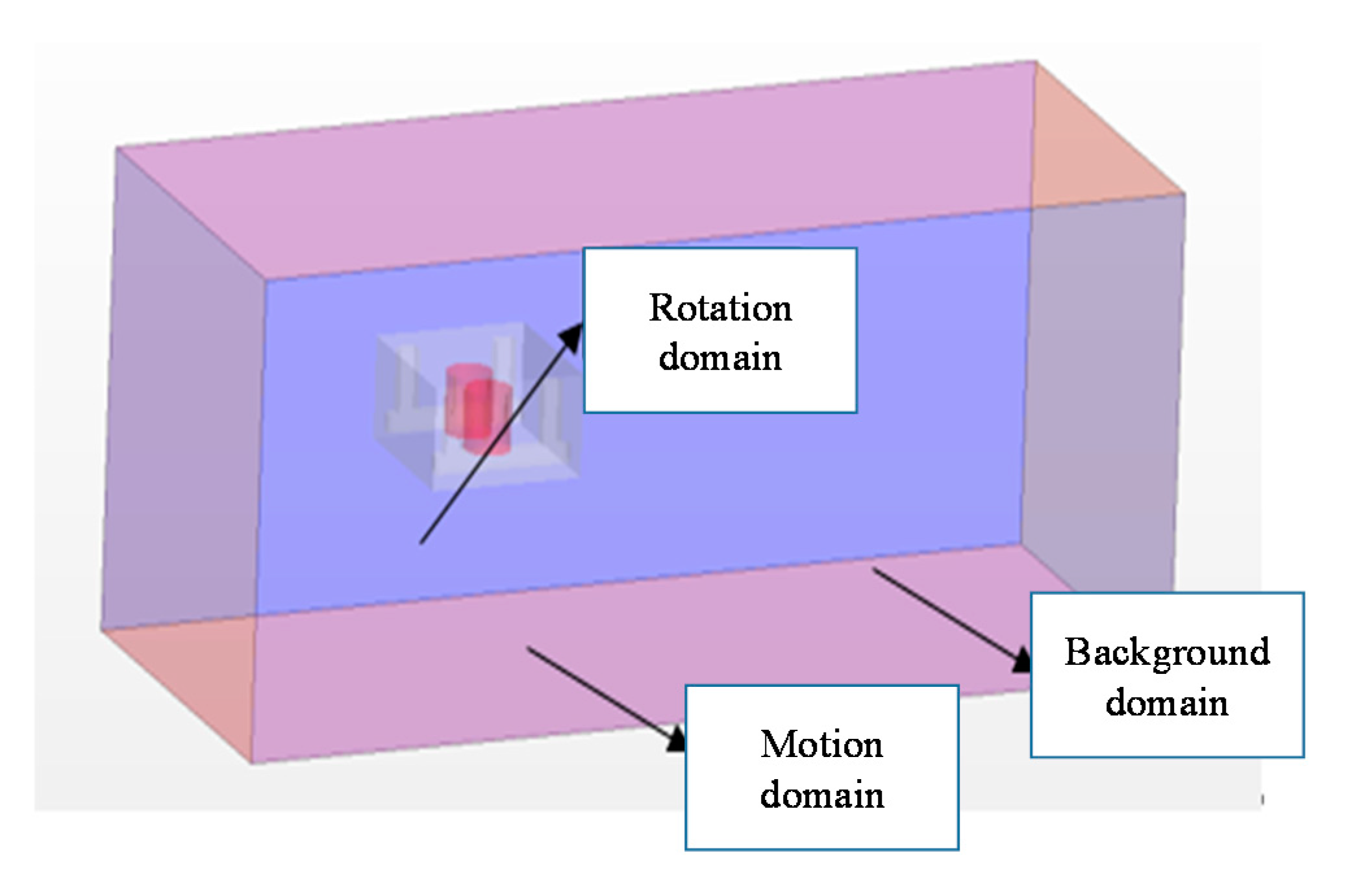


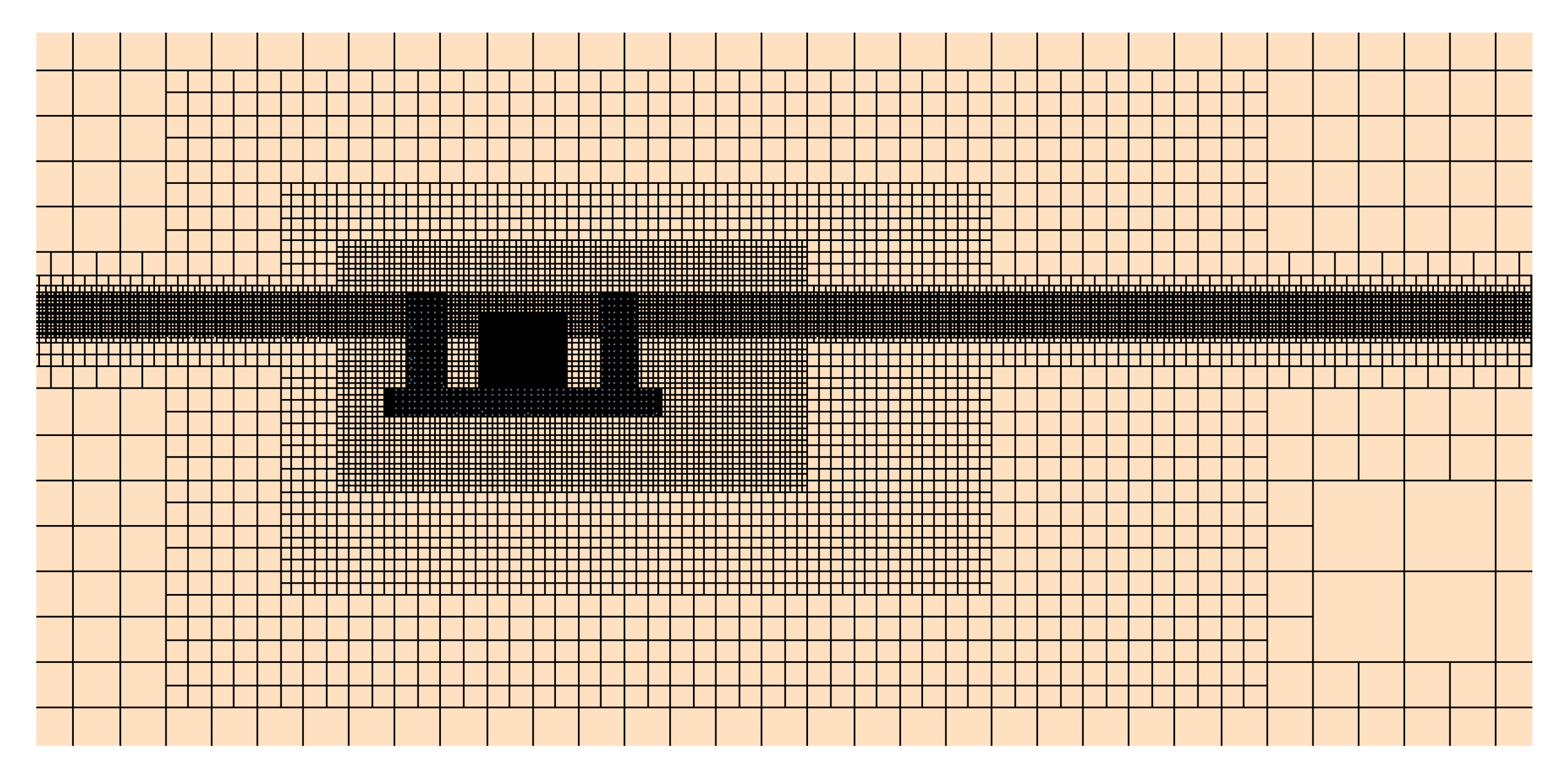









| Case | Velocity (m/s) | TSR | Computational Domain |
|---|---|---|---|
| A | 2.5 | 2.0 | Stand-alone turbine |
| B | 2.5 | 2.0 | FTCPS |
| Case | Velocity (m/s) | TSR | Computational Domain |
|---|---|---|---|
| C | 2.5 | 2.0 | Stand-alone carrier |
| D | 2.5 m/s | 2.0 | FTCPS |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, C.; Tang, C.; Yuwen, C.; Ma, Y. Coupled Interactions Analysis of a Floating Tidal Current Power Station in Uniform Flow. J. Mar. Sci. Eng. 2021, 9, 958. https://doi.org/10.3390/jmse9090958
Hu C, Tang C, Yuwen C, Ma Y. Coupled Interactions Analysis of a Floating Tidal Current Power Station in Uniform Flow. Journal of Marine Science and Engineering. 2021; 9(9):958. https://doi.org/10.3390/jmse9090958
Chicago/Turabian StyleHu, Chao, Chenxuan Tang, Chenyang Yuwen, and Yong Ma. 2021. "Coupled Interactions Analysis of a Floating Tidal Current Power Station in Uniform Flow" Journal of Marine Science and Engineering 9, no. 9: 958. https://doi.org/10.3390/jmse9090958
APA StyleHu, C., Tang, C., Yuwen, C., & Ma, Y. (2021). Coupled Interactions Analysis of a Floating Tidal Current Power Station in Uniform Flow. Journal of Marine Science and Engineering, 9(9), 958. https://doi.org/10.3390/jmse9090958






