Diffusion Kinetics Theory of Removal of Assemblies’ Surface Deposits with Flushing Oil
Abstract
:1. Introduction
2. The Models and Solutions
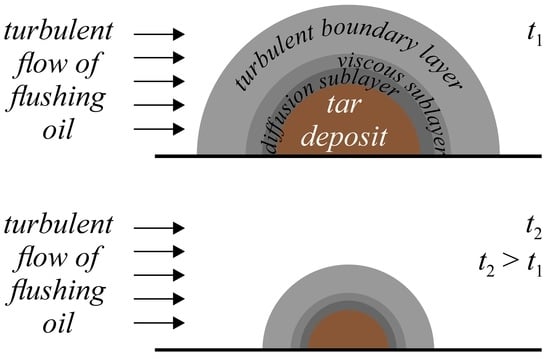
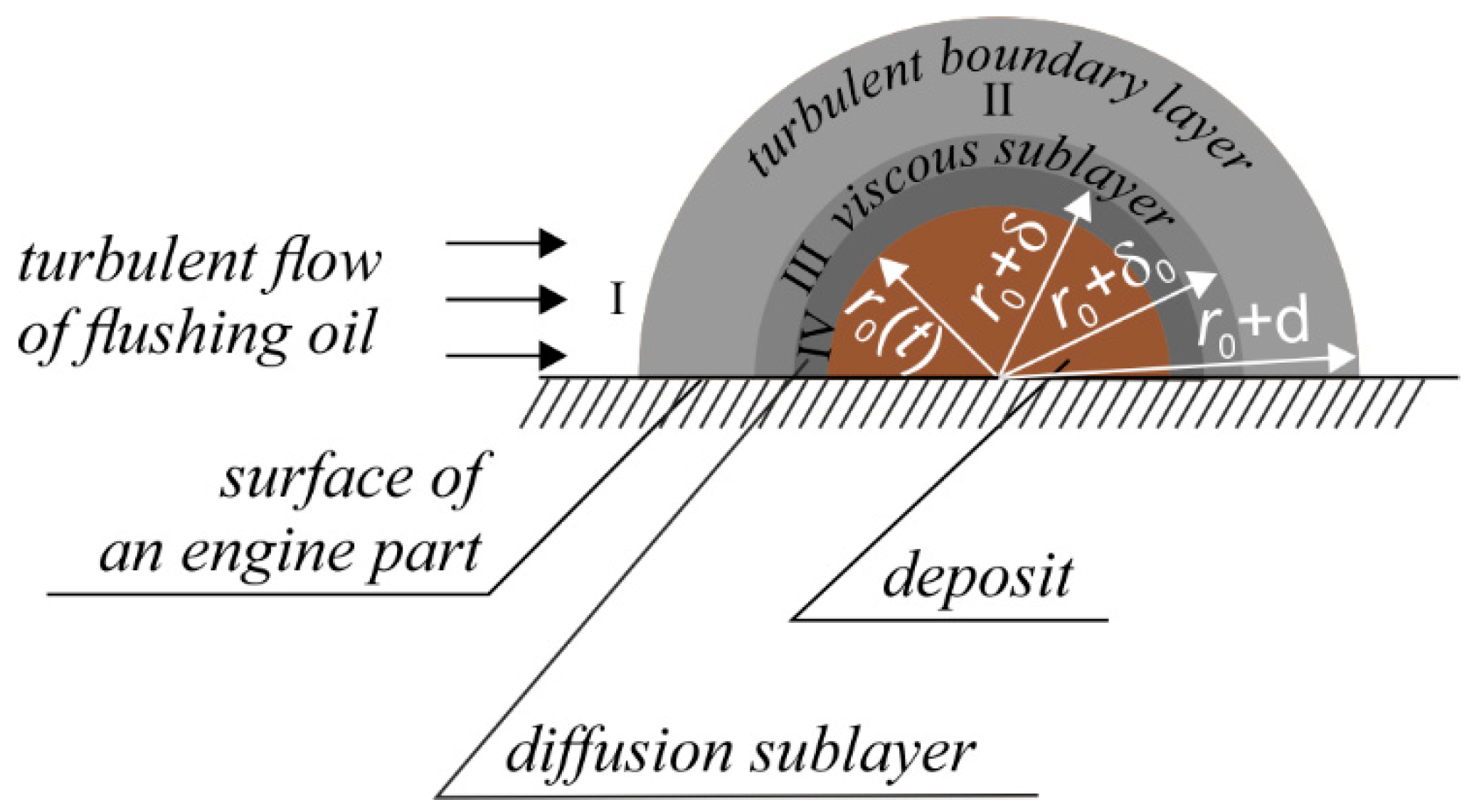
2.1. Hydrodynamic Scouring Model of Tar Deposit Removal
2.1.1. Distribution of Contaminant Concentrations
2.1.2. Dynamics of the Deposit Scouring
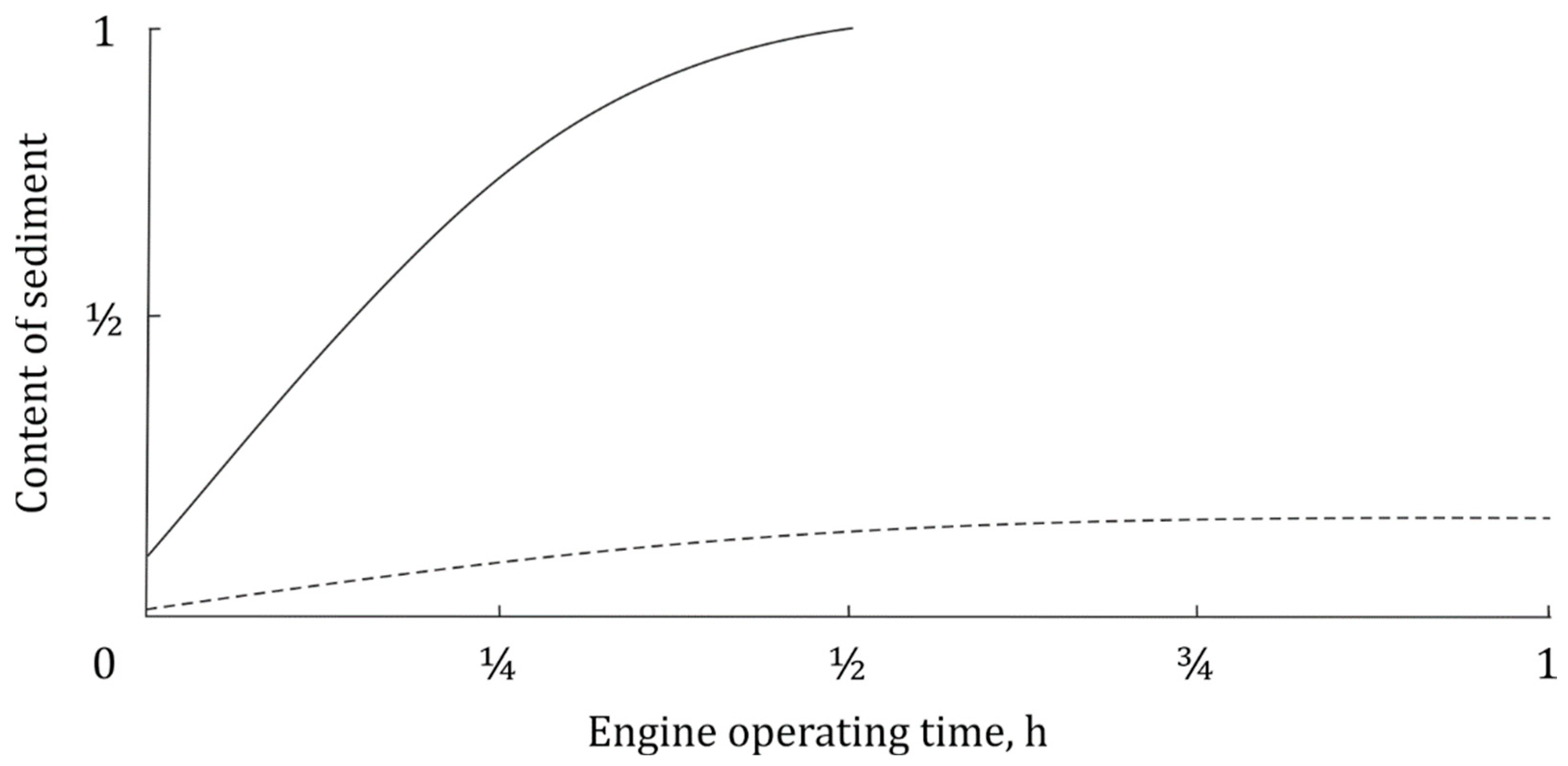
2.2. Softening and Flushing of Tar Deposits in the Chemical Kinetics Model
2.3. Discussion
3. Macroscopic Cleaning of Combustion Engine Part Surfaces
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cortazar, M.; Santamaria, L.; Lopez, G.; Alvarez, J.; Zhang, L.; Wang, R.; Bi, X.; Olazar, M. A comprehensive review of primary strategies for tar removal in biomass gasification. Energy Convers. Manag. 2023, 276, 116496. [Google Scholar] [CrossRef]
- Rabou, L.P.L.M.; Zwart, R.W.R.; Vreugdenhil, B.J.; Bos, L. Tar in Biomass Producer Gas, the Energy research Centre of The Netherlands (ECN) Experience: An Enduring Challenge. Energy Fuels 2009, 23, 6189–6198. [Google Scholar] [CrossRef]
- Palma, C.F. Modelling of tar formation and evolution for biomass gasification: A review. Appl. Energy 2013, 111, 129–141. [Google Scholar] [CrossRef]
- Evans, R.J.; Milne, T.A. Chemistry of Tar Formation and Maturation in the Thermochemical Conversion of Biomass. In Developments in Thermochemical Biomass Conversion; Bridgwater, A.V., Boocock, D.G.B., Eds.; Springer: Dordrecht, The Netherlands, 1997. [Google Scholar] [CrossRef]
- Shukla, B.; Koshi, M. Comparative study on the growth mechanisms of PAHs. Combust. Flame 2011, 158, 369–375. [Google Scholar] [CrossRef]
- Zhou, H.; Wu, C.; Onwudili, J.A.; Meng, A.; Zhang, Y.; Williams, P.T. Polycyclic aromatic hydrocarbon formation from the pyrolysis/gasification of lignin at different reaction conditions. Energy Fuels 2014, 28, 6371–6379. [Google Scholar] [CrossRef]
- Qin, Y.; Campen, A.; Wiltowski, T.; Feng, J.; Li, W. The influence of different chemical compositions in biomass on gasification tar formation. Biomass Bioenergy 2015, 83, 77–84. [Google Scholar] [CrossRef]
- Arena, U.; Di Gregorio, F.; Santonastasi, M. A techno-economic comparison between two design configurations for a small scale, biomass-to-energy gasification based system. Chem. Eng. J. 2010, 162, 580–590. [Google Scholar] [CrossRef]
- Situmorang, Y.A.; Zhao, Z.; Yoshida, A.; Abudula, A.; Guan, G. Small-scale biomass gasification systems for power generation: A review. Renew. Sustain. Energy Rev. 2020, 117, 109486. [Google Scholar] [CrossRef]
- Asadullah, M. Biomass gasification gas cleaning for downstream applications: A comparative critical review. Renew. Sustain. Energy Rev. 2014, 40, 118–132. [Google Scholar] [CrossRef]
- Prando, D.; Ail, S.S.; Chiaramonti, D.; Baratieri, M.; Dasappa, S. Characterisation of the producer gas from an open top gasifier: Assessment of different tar analysis approaches. Fuel 2016, 181, 566–572. [Google Scholar] [CrossRef]
- Li, Y.; Pang, Y.; Tu, H.; Torrigino, F.; Biollaz, S.M.A.; Li, Z.; Huang, Y.; Yin, X.; Grimm, F.; Karl, J. Impact of syngas from biomass gasification on solid oxide fuel cells: A review study for the energytransition. Energy Convers. Manag. 2021, 250, 114894. [Google Scholar] [CrossRef]
- Molino, A.; Chianese, S.; Musmarra, D. Biomass gasification technology: The state of the art overview. J. Energy Chem. 2016, 25, 10–25. [Google Scholar] [CrossRef]
- Sikarwar, V.S.; Zhao, M.; Clough, P.; Yao, J.; Zhong, X.; Memon, M.Z.; Shah, N.; Anthony, E.J.; Fennell, P.S. An overview of advances in biomass gasification. Energy Environ. Sci. 2016, 9, 2939–2977. [Google Scholar] [CrossRef]
- Gredinger, A.; Spörl, R.; Scheffknecht, G. Comparison measurements of tar content in gasification systems between an online method and the tar protocol. Biomass Bioenergy 2018, 111, 301–307. [Google Scholar] [CrossRef]
- Ostrikov, V.V.; Vigdorovich, V.I.; Safonov, V.V.; Kartoshkin, A.P. Development of a Technological Process and Composition of Flushing Oil for Diesel Engines. Chem. Technol. Fuels Oils 2018, 54, 24–28. [Google Scholar] [CrossRef]
- Al-Saadi, D.A.Y.; Ostrikov, V.V.; Sazonov, S.N.; Zabrodskaya, A.V. Evaluating performance of cleaning the diesel engine lubrication system from pollution. Plant Arch. 2020, 20, 2704–2707. [Google Scholar]
- Koshelev, A. Cleaning of tractor engine lubrication system. Sci. Cent. Russ. 2022, 56, 142–147. [Google Scholar] [CrossRef]
- Siddig, O.; Mahmoud, A.A.; Elkatatny, S. A review of different approaches for water-based drilling fluid filter cake removal. J. Pet. Sci. Eng. 2020, 192, 107346. [Google Scholar] [CrossRef]
- Arens, V.; Offenberg, I. Physical-chemical geotechnology. Instrument for the superior valuation of mineral resources and environmental protection. MATEC Web Conf. 2021, 342, 02017. [Google Scholar] [CrossRef]
- Levich, V.G. Physicochemical Hydrodynamics; Prentice-Hall: Englewood Cliffs, NJ, USA, 1962. [Google Scholar]
- Eyring, H.; Lin, S.H.; Lin, S.M. Basic Chemical Kinetics; Wiley: New York, NY, USA, 1980. [Google Scholar]
- Salvestrini, S. A modification of the Langmuir rate equation for diffusion-controlled adsorption kinetics. React. Kinet. Mech. Catal. 2019, 128, 571–586. [Google Scholar] [CrossRef]
- Vigdorowitsch, M.; Tsygankova, L.E.; Vigdorovich, V.I.; Knyazeva, L.G. To the thermodynamic properties of nano-ensembles. Mater. Sci. Eng. B 2021, 263, 114897. [Google Scholar] [CrossRef]
- Vigdorowitsch, M. Thermodynamics and stability of metallic nano-ensembles. In Mechanics and Physics of Structured Media; Andrianov, I., Gluzman, S., Mityushev, V., Eds.; Academic Press: Cambridge, MA, USA, 2022; pp. 357–394. [Google Scholar] [CrossRef]
- Salvestrini, S. Analysis of the Langmuir rate equation in its differential and integrated form for adsorption processes and a comparison with the pseudo first and pseudo second order models. React. Kinet. Mech. Catal. 2018, 123, 455–472. [Google Scholar] [CrossRef]
- Fenti, A.; Salvestrini, S. Analytical solution of the Langmuir-based linear driving force model and its application to the adsorption kinetics of boscalid onto granular activated carbon. React. Kinet. Mech. Catal. 2018, 125, 1–13. [Google Scholar] [CrossRef]

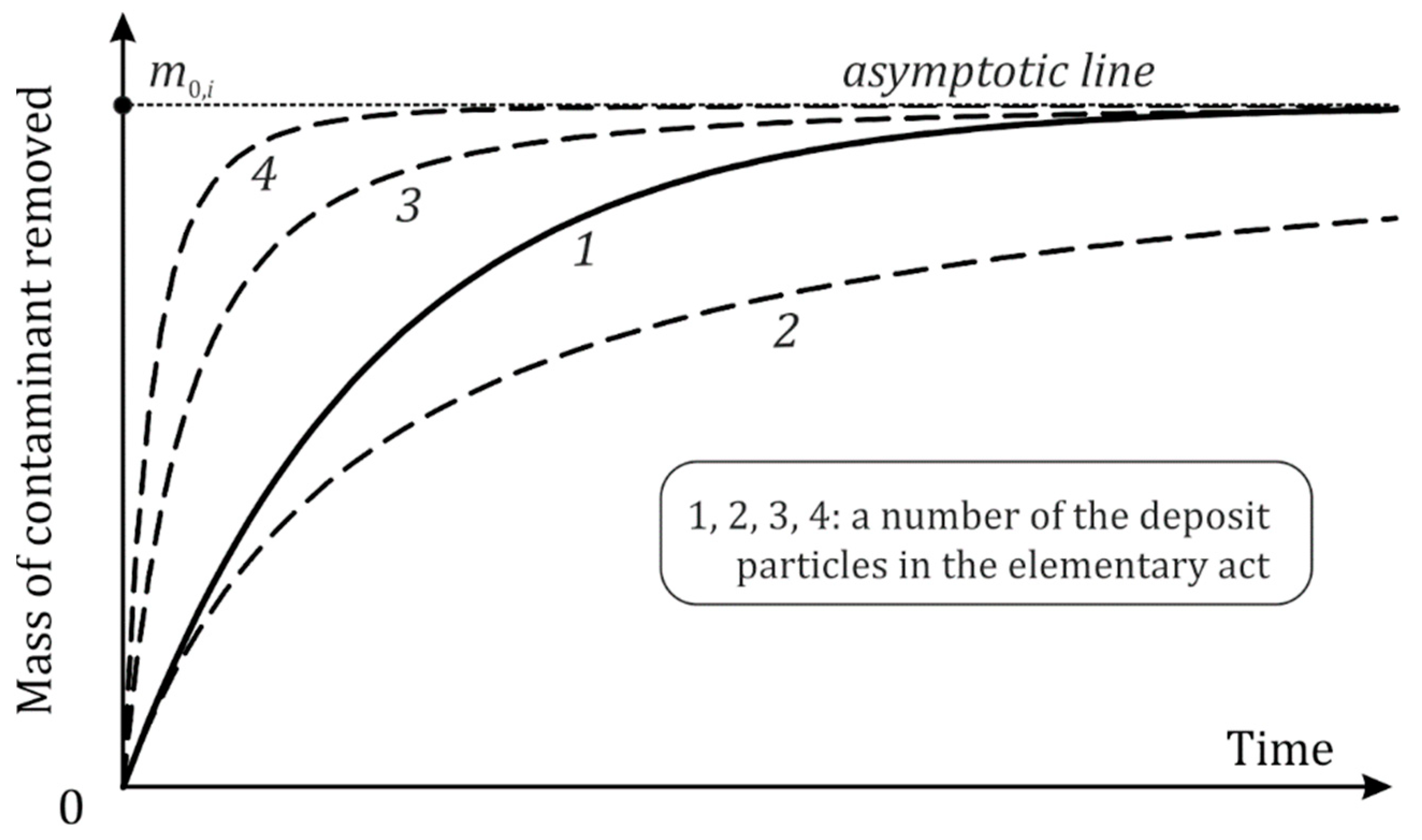
| Structure of an Elementary Act from the Deposit Side. Cases: | |||
|---|---|---|---|
| 1 Particle | 2 Particles (Example) | ||
| Kinetic equation | |||
| Solution | |||
| Deposit substance mass removed | |||
| Deposit erosion rate | |||
| for small t ♣ | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vigdorowitsch, M.; Ostrikov, V.V.; Pchelintsev, A.N.; Pchelintseva, I.Y. Diffusion Kinetics Theory of Removal of Assemblies’ Surface Deposits with Flushing Oil. Computation 2023, 11, 164. https://doi.org/10.3390/computation11080164
Vigdorowitsch M, Ostrikov VV, Pchelintsev AN, Pchelintseva IY. Diffusion Kinetics Theory of Removal of Assemblies’ Surface Deposits with Flushing Oil. Computation. 2023; 11(8):164. https://doi.org/10.3390/computation11080164
Chicago/Turabian StyleVigdorowitsch, Michael, Valery V. Ostrikov, Alexander N. Pchelintsev, and Irina Yu. Pchelintseva. 2023. "Diffusion Kinetics Theory of Removal of Assemblies’ Surface Deposits with Flushing Oil" Computation 11, no. 8: 164. https://doi.org/10.3390/computation11080164
APA StyleVigdorowitsch, M., Ostrikov, V. V., Pchelintsev, A. N., & Pchelintseva, I. Y. (2023). Diffusion Kinetics Theory of Removal of Assemblies’ Surface Deposits with Flushing Oil. Computation, 11(8), 164. https://doi.org/10.3390/computation11080164