Computational Representation of Fractional Inequalities Through 2D and 3D Graphs with Applications
Abstract
:1. Introduction
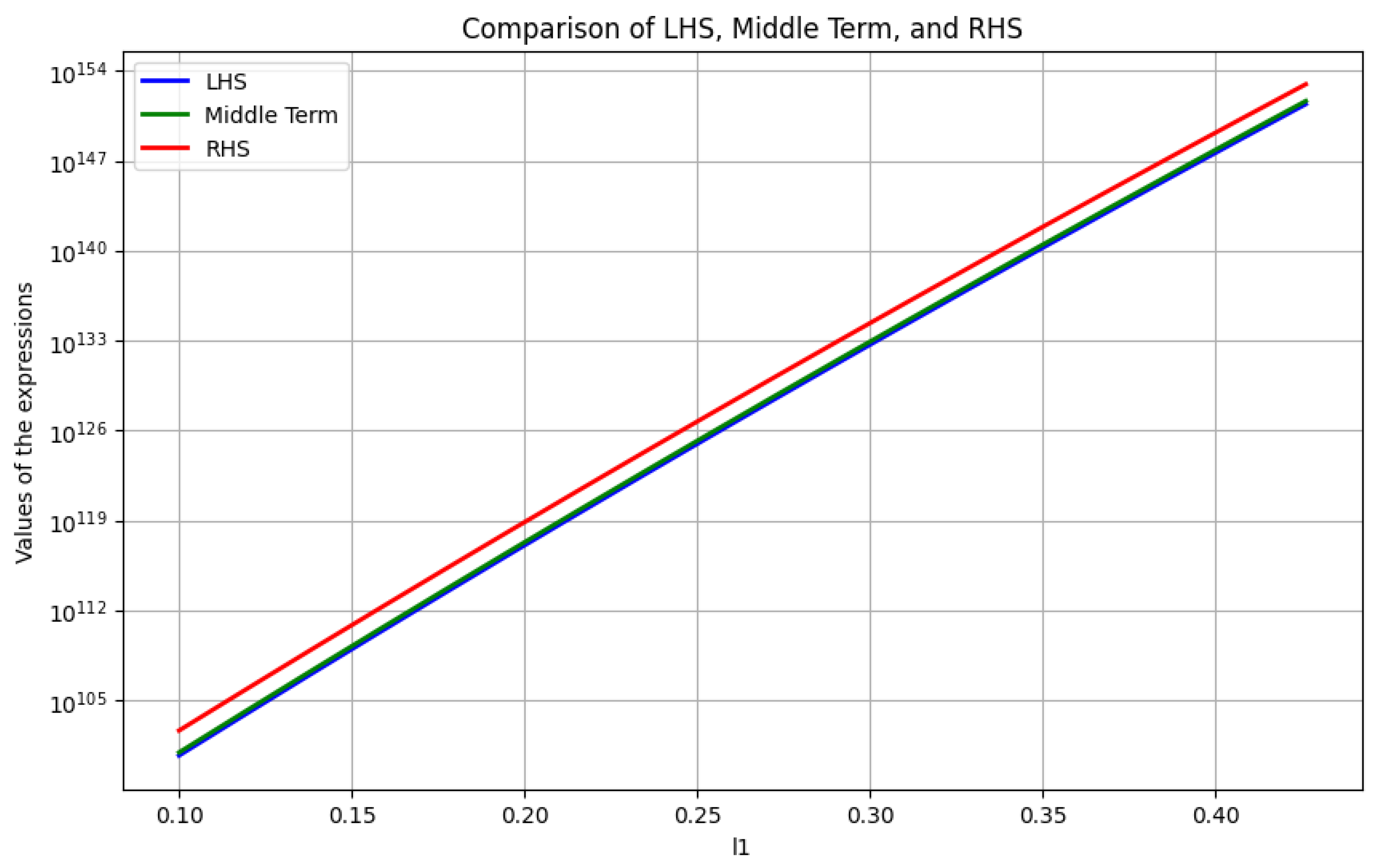
2. A Class of Some Results
| / | ||
|---|---|---|
| (4.25 × , 1.23 × , 2.19 × ) | (5.56 × , 1.39 × , 3.72 × ) | |
| (6.51 × , 2.14 × , 2.87 × ) | (7.83 × , 2.26 × , 4.91 × ) |
| / | ||
|---|---|---|
| (7.48 × , 1.68 × , 4.51 × ) | (8.74 × , 1.95 × , 5.63 × ) | |
| (9.66 × , 2.72 × , 5.74 × ) | (1.12 × , 3.06 × , 6.85 × ) |
3. Generalized H-H-F Type Inequalities Involving h-Convexity Associated with an Extended Fractional Operator and M-M-L Function
| / | |||
|---|---|---|---|
| 0.10 | (4.12 × , 3.14 × ) | (5.23 × , 4.16 × ) | (6.98 × , 5.69 × ) |
| 0.30 | (5.29 × , 4.21 × ) | (6.43 × , 5.25 × ) | (8.44 × , 7.07 × ) |
| 0.50 | (6.47 × , 5.29 × ) | (7.62 × , 6.34 × ) | (9.89 × , 8.08 × ) |
| 0.70 | (7.65 × , 6.36 × ) | (8.82 × , 7.42 × ) | (1.10 × , 9.09 × ) |
| 0.90 | (8.83 × , 7.43 × ) | (1.00 × , 8.51 × ) | (1.24 × , 1.04 × ) |
| 1.00 | (1.00 × , 8.57 × ) | (1.13 × , 9.45 × ) | (1.39 × , 1.17 × ) |
| / | |||
|---|---|---|---|
| 0.10 | (8.72 × , 7.22 × ) | (1.04 × , 8.75 × ) | (1.16 × , 9.87 × ) |
| 0.30 | (1.03 × , 8.78 × ) | (1.21 × , 1.05 × ) | (1.34 × , 1.17 × ) |
| 0.50 | (1.20 × , 9.89 × ) | (1.40 × , 1.16 × ) | (1.55 × , 1.30 × ) |
| 0.70 | (1.32 × , 1.10 × ) | (1.53 × , 1.29 × ) | (1.69 × , 1.43 × ) |
| 0.90 | (1.48 × , 1.27 × ) | (1.72 × , 1.49 × ) | (1.88 × , 1.64 × ) |
| 1.00 | (1.64 × , 1.38 × ) | (1.90 × , 1.58 × ) | (2.06 × , 1.72 × ) |
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abel, N. Solution de quelques problemes l’aide d’integrales definies. Mag. Naturv. 1823, 55–63. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.H.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier B.V.: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Oldham, K.B.; Spanier, J. The Fractional Calculus; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Prog. Frac. Differ. Appl. 2015, 2, 73–85. [Google Scholar]
- Losada, J.; Nieto, J.J. Properties of a new fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 2015, 1, 87–92. [Google Scholar]
- Yang, X.J.; Srivastava, H.M.; Machado, J.A.T. A new fractional derivative without a singular kernel: Application to the moddeling of the steady heat flow. Therm. Sci. 2016, 20, 753–756. [Google Scholar] [CrossRef]
- Fernandez, A.; Ozarslan, M.A.; Baleanu, D. On fractional calculus with general analytic kernels. Appl. Math. Comput. 2019, 354, 248–265. [Google Scholar] [CrossRef]
- Teodoro, G.S.; Machado, J.A.T.; de Oliveira, E.C. A review of definitions of fractional derivatives and other operators. J. Comput. Phys. 2019, 388, 195–208. [Google Scholar] [CrossRef]
- Wu, S.; Samraiz, M.; Mehmood, A.; Jarad, J.; Naheed, S. Some symmetric propertise and applications of weighted fractional integral operator. Fractals 2023, 31, 2340011. [Google Scholar] [CrossRef]
- Samraiz, M.; Mehmood, A.; Iqbal, S.; Naheed, S.; Rehman, G.; Chu, Y.M. Generalized fractional operator with applications in mathematical physics. Chaos Solition Fractals 2022, 16, 112830. [Google Scholar] [CrossRef]
- Samraiz, M.; Mehmood, A.; Naheed, S.; Rehman, G.; Kashuri, A.; Nonlaopon, K. On novel fractional Operators involving the multivariate M-L function. Mathematics 2022, 10, 3991. [Google Scholar] [CrossRef]
- Huang, W.H.; Samraiz, M.; Mehmood, A.; Baleanu, D.; Rehman, G.; Naheed, S. Modified Atangana- Baleanu Fractional operators Involving generalized Mittag-Leffler function. Alexanderia Eng. J. 2023, 75, 639–648. [Google Scholar] [CrossRef]
- Cho, Y.; Kim, I.; Sheen, D. A fractional-order model for MINMOD Millennium. Math. Biosci. 2015, 262, 36–45. [Google Scholar] [CrossRef] [PubMed]
- Magin, R.L.; Ovadia, M. Modeling the cardiac tissue electrode interface using fractional calculus. J. Vib. Control 2008, 1, 1431–1442. [Google Scholar] [CrossRef]
- Chen, W.; Pang, G. A new definition of fractional Laplacian with application to modeling three-dimensional nonlocal heat conduction. J. Comput. Phys. 2016, 309, 350–367. [Google Scholar] [CrossRef]
- Grahovac, N.M.; Zigic, M.M. Modelling of the hamstring muscle group by use of fractional derivatives. Comput. Math. Appl. 2010, 59, 1695–1700. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.Y. Analysis of two colliding fractionally damped spherical shells in modelling blunt human head impacts. Cent. Eur. J. Phys. 2013, 11, 760–778. [Google Scholar] [CrossRef]
- Balci, M.A. Fractional virus epidemic model on financial networks. Open Math. 2016, 14, 1074–1086. [Google Scholar] [CrossRef]
- Roman, H.E.; Porto, M. Fractional derivatives of random walks: Time series with long-time memory. Phys. Rev. E 2008, 78, 031127. [Google Scholar] [CrossRef]
- Moreles, M.A.; Lainez, R. Mathematical modelling of fractional order circuit elements and bioimpedance applications. Commun. Nonlinear Sci. Numer. Simul. 2017, 46, 81–88. [Google Scholar] [CrossRef]
- Yalçın, S.; Bayram, H. On harmonic univalent functions involving q-Poisson distribution series. MJPS 2021, 8, 105–111. [Google Scholar]
- Reyes-Melo, E.; Martinez-Vega, J.; Guerrero-Salazar, C.; Ortiz-Mendez, U. Application of fractional calculus to the modeling of dielectric relaxation phenomena in polymeric materials. J. Appl. Polym. Sci. 2005, 98, 923–935. [Google Scholar] [CrossRef]
- Carcione, J.M. Theory and modeling of constant-QP- and S -waves using fractional time derivatives. Geophysics 2009, 74, 1–11. [Google Scholar] [CrossRef]
- Jensen, J.L.W.V. Sur les fonctions convexes et les inegalites entre les valeurs moyennes. Acta Math. 1905, 30, 175–193. [Google Scholar] [CrossRef]
- Breckner, W.W. Stetigkeitsaussagen fur eine Klasse verallgemein konvexer Funktionen in topologischen linearen Raumen. Publ. Inst. Math. 1978, 23, 13–20. [Google Scholar]
- Jarad, F.; Ugurlu, E.; Abdeljawad, T.; Baleanu, D. On a new class of fractional operators. Adv. Differ. Equ. 2017, 2017, 247. [Google Scholar] [CrossRef]
- Set, E.; Iscan, I.; Sarikaya, M.Z.; Ozdemir, M.E. On new inequalities of Hermite-Hadamard-Fejer’ type for convex functions via fractional integrals. Appl. Math. Comput. 2015, 259, 875–881. [Google Scholar] [CrossRef]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals Series and Products, 7 Eds.; Academic Press: San Diego, CA, USA, 1980. [Google Scholar]
- Sarikaya, M.Z.; Dahmani, Z.; Kiris, M.E.; Ahmad, F. (k, s)-Riemann-Liouville fractional integral and applications. Hacet. J. Math. Stat. 2016, 45, 77–89. [Google Scholar] [CrossRef]
- Gorenflo, R.; Kilbas, A.A.; Mainardi, F.; Rogosin, S.V. Mittag-Leffler Functions, Related Topics and Applications; Springer Monographs in Mathematics; Springer: New York, NY, USA, 2014. [Google Scholar]
- Akgül, E.K.; Akgül, A.; Yavuz, M. New illustrative application of integral transform to financial models with different fractional derivatives. Chaos Solitions Fractals 2021, 146, 110877. [Google Scholar] [CrossRef]
- Yavuz, M.; Sene, N. Approximation solution of the model describing fluid flow using generalized laplace transform method and heat balance integral method. Axioms 2020, 9, 123. [Google Scholar] [CrossRef]
- Yavuz, M.; Abdeljawad, T. Nonlinear regularized long-wave with a new integral transformation applied to the fractional derivative with power and Mittag-Leffler kernel. Adv. Differ. Equ. 2020, 2020, 367. [Google Scholar] [CrossRef]
- Yavuz, M.; Ozdemir, N. Comparison the new fractional derivative operators involving exponential and Mittag-Leffler kernal. Discrete Contin. Dyn. Syst. Ser. S. 2020, 13, 995–1006. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Nisar, K.S.; Rahman, G.; Baleanu, D.; Mubeen, S.; Arshad, M. The (k, s)-fractional calculus of k Mittag-Leffler function. Adv. Differ. Equ. 2017, 2017, 118. [Google Scholar] [CrossRef]
- Baleanu, D.; Diethelm, K.; Scalas, E.; Trujillo, J.J. Fractional Calculus: Models and Numerical Methods, Series on Complexity, Nonlinearity and Chaos; World Scientific: Singapore, 2012. [Google Scholar]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models; World Scientific Publishing Company: Singapore; Hackensack, NJ, USA; London, UK, 2010. [Google Scholar]
- Zhao, D.; Luo, M. Representations of acting processes and memory effects: General fractional derivative and its application to theory of heat conduction with finite wave speeds. Appl. Math. Comput. 2019, 49, 531–544. [Google Scholar] [CrossRef]
- Chen, S.B.; Rashid, S.; Noor, M.A.; Ashraf, R.; Chu, Y.M. A new approach on fractional calculus and probability density function. AIMS Math. 2020, 5, 7041–7054. [Google Scholar] [CrossRef]
- Samraiz, M.; Umer, M.; Abduljawad, T.; Naheed, S.; Rahman, G.; Shah, K. On Riemann-type weighted fractional operator and solution to cauchy problems. Comput. Model. Eng. Sci. 2022, 136, 901–917. [Google Scholar] [CrossRef]
- Mittag-Leffler, M.G. Sur la nouvelle fonction E(x). C. R. Acad. Sci. 1903, 137, 5548. [Google Scholar]
- Garg, M.; Manohar, P.; Kalla, S.L. A Mittag-Leffler-type function of two variables. Int. Transf. Spec. Funct. 2013, 24, 9344. [Google Scholar] [CrossRef]
- Saxena, R.K.; Kalla, S.L.; Saxena, R. Multivariate analogue of generalised Mittag-Leffler function. Int. Transf. Spec. Funct. 2011, 22, 533–548. [Google Scholar] [CrossRef]
- Fej, L. Über die Fourierreihen. II. Math. Naturwiss Anz. Ungar. Akad. Wiss. 1906, 24, 369–390. [Google Scholar]
- Hadamard, J. Étude sur les propriétés des fonctions entières et en particulier d’une fonction considérée par Riemann. J. Mathématiques Pures Appliquées 1893, 9, 171–215. [Google Scholar]
- Tseng, K.L.; Yang, G.S.; Hsu, K.C. Some inequalities for differentiable mappings and applications to Fejér inequality and weighted trapezoidal formula. Taiwan J. Math. 2011, 15, 1737–1747. [Google Scholar] [CrossRef]
- Delavar, M.R.; Dargomir, S.S. Trapezoidal type inequalities related to h-convex functions with applications. Rev. R. Acad. Cienc Exactas Fís Nat. Ser.-A Math. 2019, 113, 1487–1498. [Google Scholar] [CrossRef]
- Matloka, M. Weighted Simpson type inequalities for h-convex functions. J. Non-Linear Sci. Appl. 2017, 10, 5770–5780. [Google Scholar] [CrossRef]
- Varošanec, S. On h-convexity. J. Math. Anal. Appl. 2007, 326, 303–311. [Google Scholar] [CrossRef]
- Chen, H.; Katugampola, U.N. Hermite-Hadamard and Hermite-Hadamard-Fejer type inequalities for generalized fractional integrals. J. Math. Anal. Appl. 2017, 446, 1274–1291. [Google Scholar] [CrossRef]
- Khan, T.U.; Khan, M.A. Generalized conformable fractional operators. J. Comput. Appl. Math. 2019, 346, 378–389. [Google Scholar] [CrossRef]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives, Theory and Applications; Gordon and Breach: Montreux, Switzerland, 1993. [Google Scholar]
- Agarwal, P.; Nieto, J.J. Some fractional integral formulas for the Mittag-Leffler type function with four parameters. Open Math. 2015, 13, 537–546. [Google Scholar] [CrossRef]
- Hölder, O.L. Nachrichten von der Königl Gesel Schaft der Wissenschaften und der Georg-August-Universität zu Göttingen; Dieterichsche Verlags-Buchhandlung: Gottingen, Germany, 1889; pp. 38–47. [Google Scholar]
- Alqudah, M.A.; Mohammed, P.O.; Abdeljawad, T. Solution of Singular integral equation via Riemann-Liouville fractional integral. Math. Prob. Eng. 2020, 1, 1250970. [Google Scholar] [CrossRef]
- Hakiki, M.T.; Wibowo, A. Hermite-Hadamard-Fejer type inequalities for s-convex functions in the second sense via Riemann-Liouville fractional integral. J. Phys. Conf. Ser. 2020, 1442, 012039. [Google Scholar] [CrossRef]
- Sarikaya, M.Z.; Set, E.; Özdemir, M.E. On some new inequalities of Hadamard type involving h-convex functions. Acta Math. Univ. Comeniane 2010, 79, 265–272. [Google Scholar]



| / | |||
|---|---|---|---|
| 1.00 | (6.93 × , 2.77 × ) | (2.37 × , 9.49 × ) | (9.60 × , 3.84 × ) |
| 1.20 | (4.76 × , 1.91 × ) | (1.64 × , 6.55 × ) | (6.46 × , 2.58 × ) |
| 1.40 | (3.08 × , 1.23 × ) | (1.09 × , 4.37 × ) | (4.25 × , 1.70 × ) |
| 1.60 | (1.81 × , 7.23 × ) | (7.02 × , 2.81 × ) | (2.72 × , 1.09 × ) |
| 1.80 | (8.48 × , 3.39 × ) | (4.25 × , 1.70 × ) | (1.69 × , 6.76 × ) |
| 2.00 | (0.00 × , 0.00 × ) | (2.34 × , 9.35 × ) | (1.01 × , 4.05 × ) |
| / | |||
|---|---|---|---|
| 1.00 | (4.80 × , 1.92 × ) | (3.03 × , 1.21 × ) | (2.43 × , 9.70 × ) |
| 1.20 | (3.12 × , 1.25 × ) | (1.89 × , 7.57 × ) | (1.46 × , 5.83 × ) |
| 1.40 | (1.98 × , 7.94 × ) | (1.16 × , 4.64 × ) | (8.57 × , 3.43 × ) |
| 1.60 | (1.24 × , 4.94 × ) | (6.96 × , 2.78 × ) | (4.95 × , 1.98 × ) |
| 1.80 | (7.52 × , 3.01 × ) | (4.09 × , 1.64 × ) | (2.80 × , 1.12 × ) |
| 2.00 | (4.47 × , 1.79 × ) | (2.35 × , 9.42 × ) | (1.55 × , 6.20 × ) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Younis, M.; Mehmood, A.; Samraiz, M.; Rahman, G.; Haque, S.; Aloqaily, A.; Mlaiki, N. Computational Representation of Fractional Inequalities Through 2D and 3D Graphs with Applications. Computation 2025, 13, 46. https://doi.org/10.3390/computation13020046
Younis M, Mehmood A, Samraiz M, Rahman G, Haque S, Aloqaily A, Mlaiki N. Computational Representation of Fractional Inequalities Through 2D and 3D Graphs with Applications. Computation. 2025; 13(2):46. https://doi.org/10.3390/computation13020046
Chicago/Turabian StyleYounis, Muhammad, Ahsan Mehmood, Muhammad Samraiz, Gauhar Rahman, Salma Haque, Ahmad Aloqaily, and Nabil Mlaiki. 2025. "Computational Representation of Fractional Inequalities Through 2D and 3D Graphs with Applications" Computation 13, no. 2: 46. https://doi.org/10.3390/computation13020046
APA StyleYounis, M., Mehmood, A., Samraiz, M., Rahman, G., Haque, S., Aloqaily, A., & Mlaiki, N. (2025). Computational Representation of Fractional Inequalities Through 2D and 3D Graphs with Applications. Computation, 13(2), 46. https://doi.org/10.3390/computation13020046











