Innovative Quantum Encryption Method for RGB Images Based on Bit-Planes and Logistic Maps
Abstract
1. Introduction
2. Preliminaries
2.1. Bit and Qubits
2.2. Quantum Gates and Quantum Circuits
3. Our Proposed Model for Quantum Encryption Method for RGB Images Based on Bit-Planes and Logistic Maps
3.1. BRQI for RGB Color Images


3.2. Our Model for Rgb Images Encryption Based on Bit-Planes and Logistic Maps
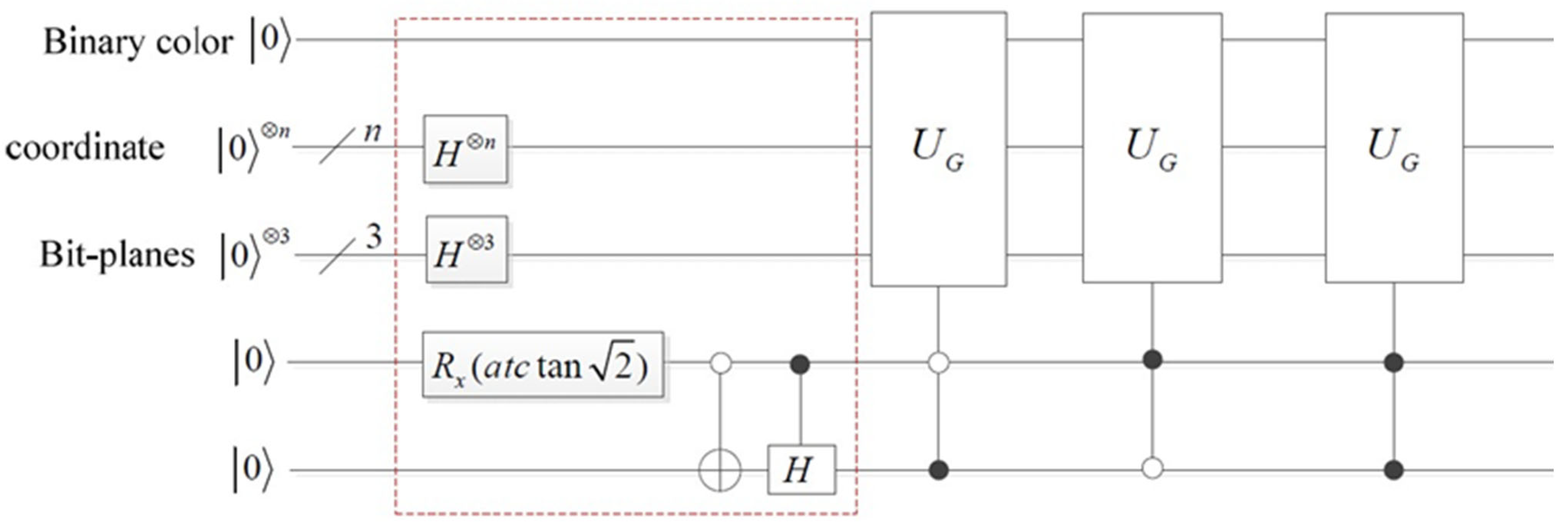
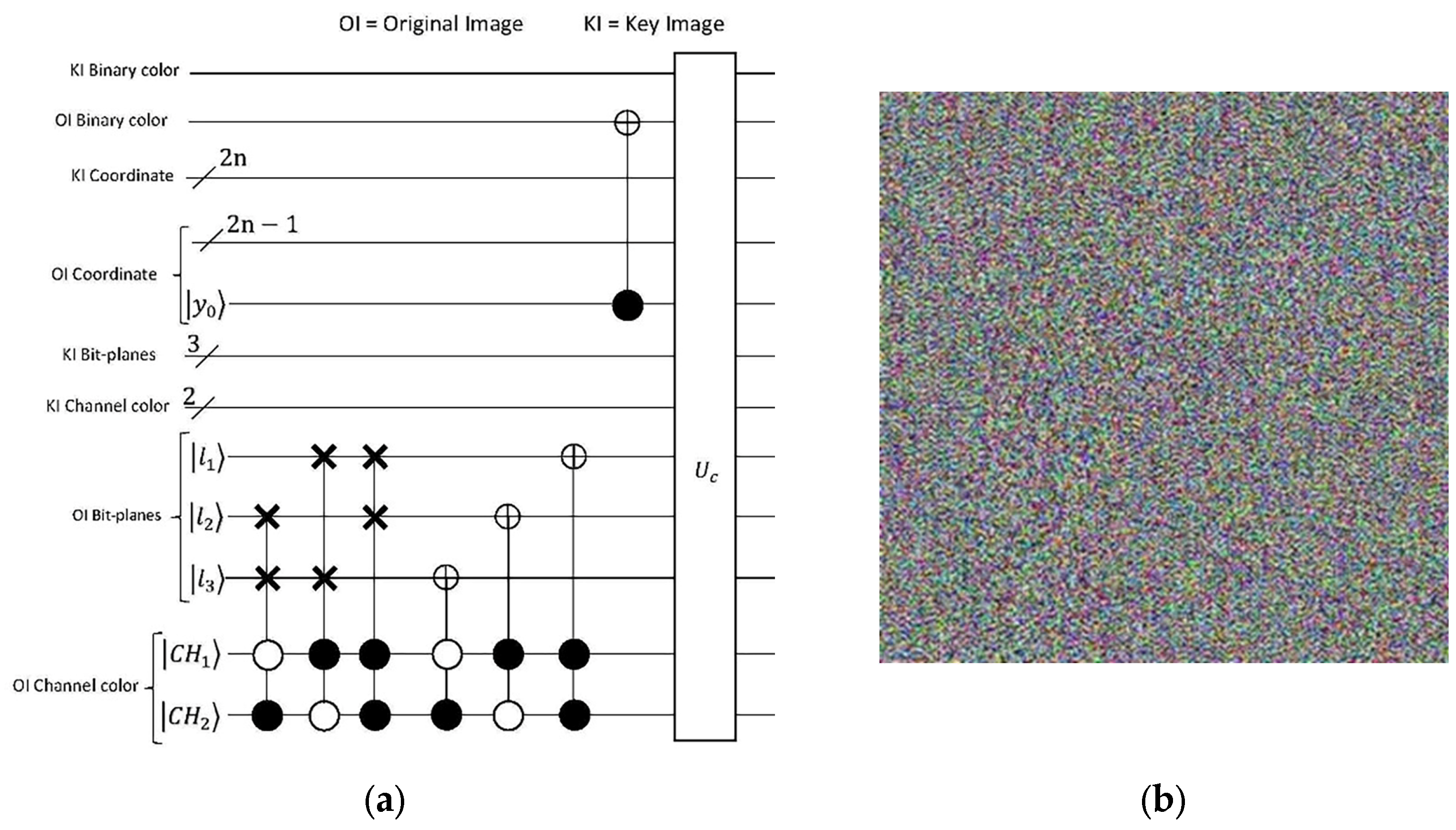
3.2.1. Image Scrambling Process
- 1.
- Swapping bit-planes.
- 2.
- Transferring image bit-planes.
- 3.
- Color complement.
- 4.
- Applying logistic map:
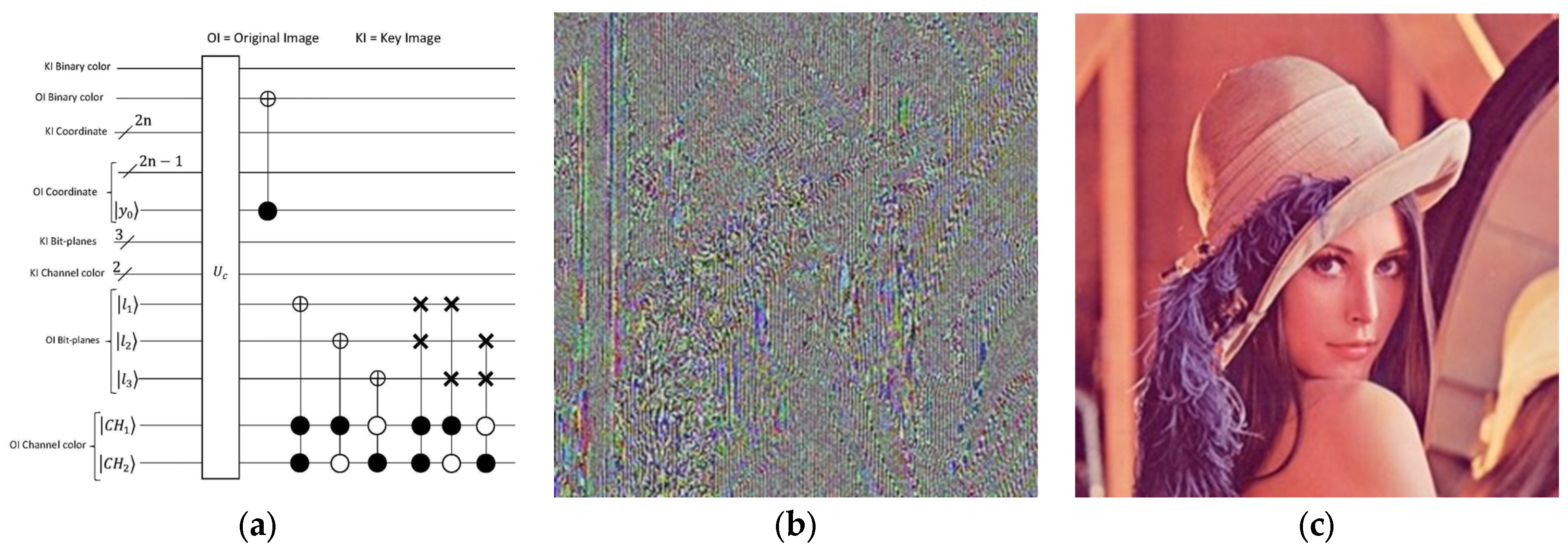
3.2.2. Recover Encrypted Image
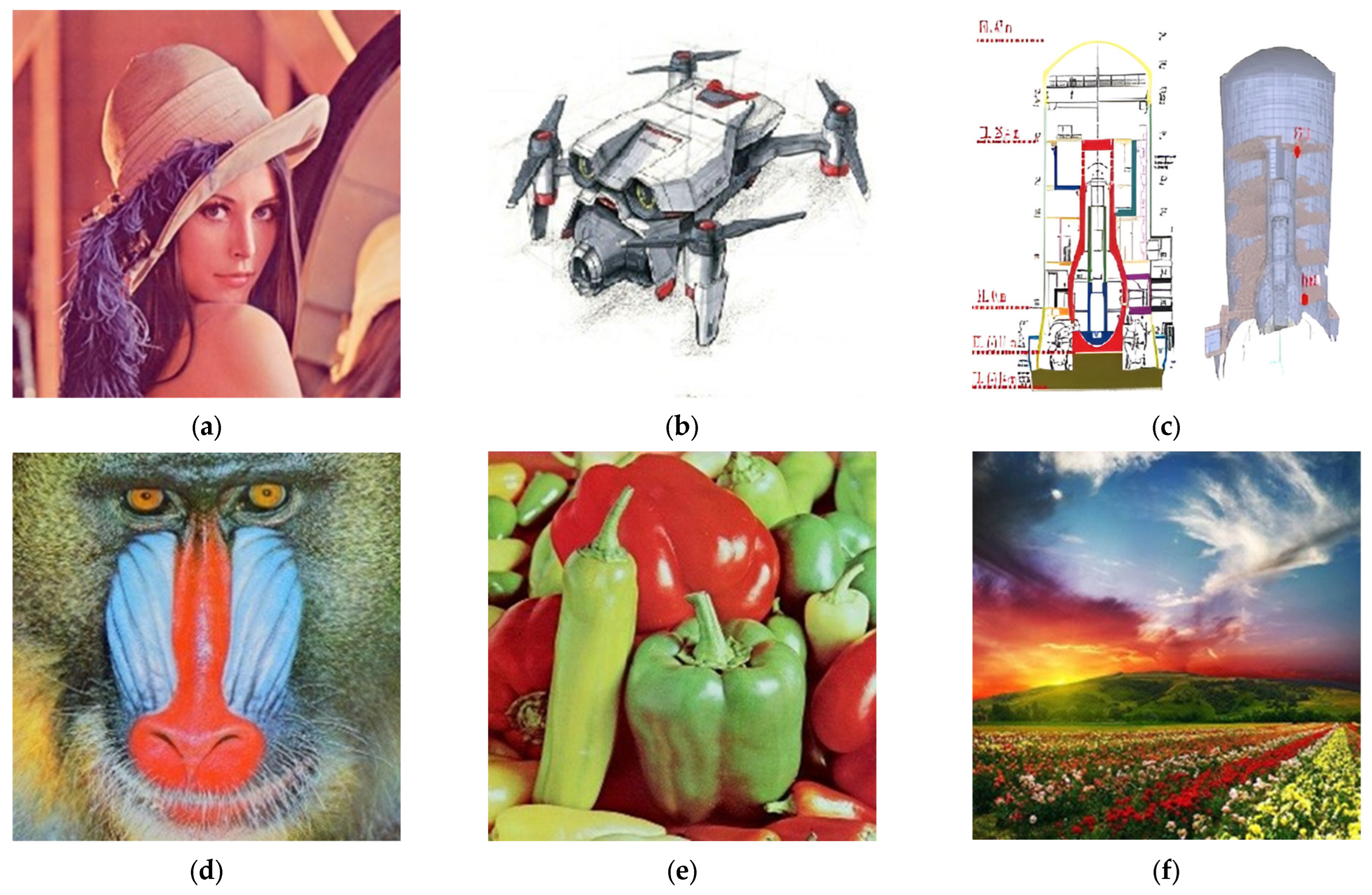
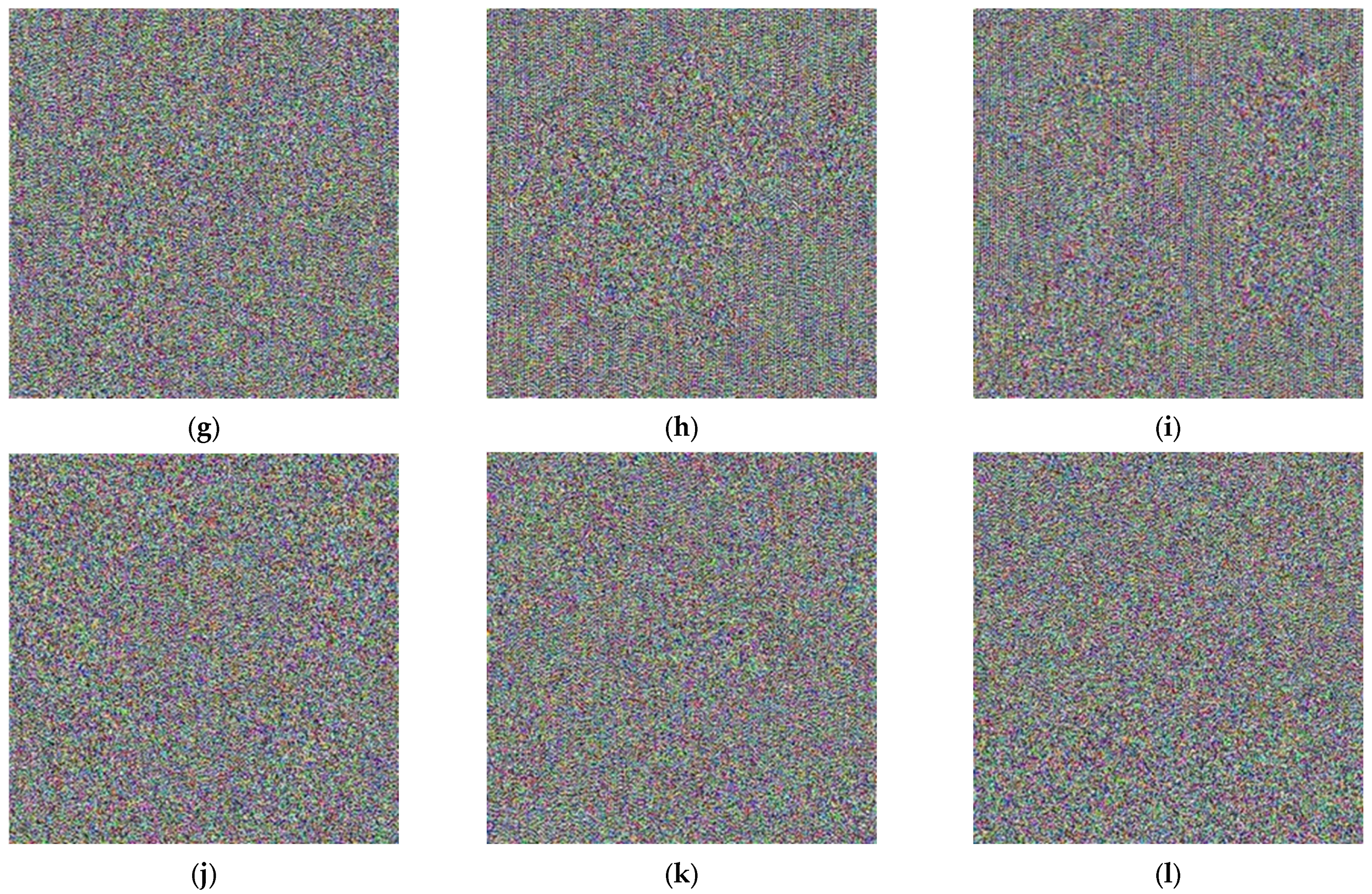
4. Analyzing the Proposed Method
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Morkel, T.; Eloff, .H.; Olivier, M.S. An Overview of Image Steganography. In Information Security South Africa; University of Pretoria: Pretoria, South Africa, 2005; pp. 1–11. [Google Scholar]
- Pahati, O.J. Confounding Carnivore: How to Protect Your Online Privacy. AlterNet 2001, 7–16. [Google Scholar]
- Singh, L.D.; Singh, K.M. Image Encryption Using Elliptic Curve Cryptography. Procedia Comput. Sci. 2015, 54, 287–294. [Google Scholar] [CrossRef]
- Lukac, R.; Plataniotis, K.N. Bit-Level-Based Secret Sharing for Image Encryption. Pattern Recognit. 2005, 38, 873–882. [Google Scholar] [CrossRef]
- Mishra, R.; Bhanodiya, P. A review on steganography and cryptography. In Proceedings of the 2015 International Conference on Advances in Computer Engineering and Applications, Ghaziabad, India, 19–20 March 2015; pp. 119–122. [Google Scholar] [CrossRef]
- Kamali, S.H.; Shakerian, R.; Hedayati, M.; Rahmani, M. A New Modified Version of Advanced Encryption Standard Based Algorithm for Image Encryption. In Proceedings of the International Conference on Electronics and Information Engineering, Kyoto, Japan, 1–3 August 2010. [Google Scholar]
- Jiang, N.; Wang, L.; Wu, W. Quantum Hilbert Image Scrambling. Int. J. Theor. Phys. 2014, 53, 2655–2663. [Google Scholar] [CrossRef]
- Jiang, N.; Wu, W.; Wang, L. The Quantum Realization of Arnold and Fibonacci Image Scrambling. Quantum Inf. Process. 2014, 13, 2179–2192. [Google Scholar] [CrossRef]
- Sankpal, P.R.; Vijaya, P.A. Image Encryption Using Chaotic Maps: A Survey. In Proceedings of the Fifth International Conference on Signal and Image Processing, Bangalore, India, 8–10 January 2014. [Google Scholar]
- Chen, J.X.; Zhu, Z.L.; Liu, Z.; Fu, C.; Zhang, L.B.; Yu, H. A Novel Double-Image Encryption Scheme Based on Cross-Image Pixel Scrambling in Gyrator Domains. Opt. Express 2014, 22, 10343–10356. [Google Scholar] [CrossRef] [PubMed]
- Wong, K.W.; Kwok, B.S.H.; Yuen, C.H. An Efficient Diffusion Approach for Chaos-Based Image Encryption. Chaos Solitons Fractals 2009, 42, 1877–1888. [Google Scholar] [CrossRef]
- Yang, Y.; Jia, X.; Xu, P.; Tian, J. Analysis and Improvement of the Watermark Strategy for Quantum Images Based on Quantum Fourier Transform. Quantum Inf. Process. 2013, 12, 2971–2990. [Google Scholar] [CrossRef]
- Li, H.; Chen, X.; Xia, H.; Liang, Y.; Zhou, Z. A Quantum Image Representation Based on Bitplanes. Proc. IEEE 2018, 6, 62396–62404. [Google Scholar] [CrossRef]
- Yan, F.; Iliyasu, A.M.; Venegas-Andraca, S.E. A Survey of Quantum Image Representations. Quantum Inf. Process. 2016, 15, 1065–1085. [Google Scholar] [CrossRef]
- Erhard, M.; Krenn, M.; Zeilinger, A. Advances in High-Dimensional Quantum Entanglement. Nat. Rev. Phys. 2020, 2, 365–381. [Google Scholar] [CrossRef]
- Vedral, V. Quantum Entanglement. Nat. Phys. 2014, 10, 256–258. [Google Scholar] [CrossRef]
- Delaubert, V.; Treps, N.; Fabre, C.; Bachor, H.A.; Réfrégier, P. Quantum Limits in Image Processing. EPL (Europhys. Lett.) 2008, 81, 44001. [Google Scholar] [CrossRef]
- Venegas-Andraca, S.E.; Bose, S. Storing, Processing, and Retrieving an Image Using Quantum Mechanics. In Quantum Information and Computation; SPIE: Bellingham, DC, USA, 2003; Volume 5105, pp. 137–147. [Google Scholar]
- Chakraborty, S.; Mandal, S.B.; Shaikh, S.H. Quantum Image Processing: Challenges and Future Research Issues. Int. J. Inf. Technol. 2018, 10, 475–489. [Google Scholar] [CrossRef]
- Ventura, D.; Martinez, T. Quantum Associative Memory with Exponential Capacity. In Proceedings of the IEEE International Joint Conference on Neural Networks, World Congress on Computational Intelligence, Anchorage, AK, USA, 4–9 May 1998; Volume 1, pp. 509–513. [Google Scholar]
- Le, P.Q.; Dong, F.; Hirota, K. A Flexible Representation of Quantum Images for Polynomial Preparation, Image Compression, and Processing Operations. Quantum Inf. Process. 2011, 10, 63–84. [Google Scholar] [CrossRef]
- Li, H.S.; Zhu, Q.; Li, M.C.; Ian, H. Multidimensional color image storage, retrieval, and compression based on quantum amplitudes and phases. Inf. Sci. 2014, 273, 212–232. [Google Scholar] [CrossRef]
- Sun, B.; Iliyasu, A.M.; Yan, F.; Dong, F.; Hirota, K. An RGB Multi-Channel Representation for Images on Quantum Computers. J. Adv. Comput. Intell. Intell. Inform. 2013, 17, 404–417. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, K.; Gao, Y.; Wang, M. NEQR: A Novel Enhanced Quantum Representation of Digital Images. Quantum Inf. Process. 2013, 12, 2833–2860. [Google Scholar] [CrossRef]
- Abdolmaleky, M.; Naseri, M.; Batle, J.; Farouk, A.; Gong, L.-H. Red-Green-Blue multi-channel quantum representation of digital images. Optik 2017, 128, 121–132. [Google Scholar] [CrossRef]
- Li, H.S.; Fan, P.; Xia, H.Y.; Peng, H.; Song, S. Quantum Implementation Circuits of Quantum Signal Representation and Type Conversion. IEEE Trans. Circuits Syst. I Regul. Pap. 2018, 65, 341–354. [Google Scholar] [CrossRef]
- Heidari, S.; Naseri, M. A Novel LSB Based Quantum Watermarking. Int. J. Theor. Phys. 2016, 55, 4205–4218. [Google Scholar] [CrossRef]
- Naseri, M.; Heidari, S.; Baghfalaki, M.; Fatahi, N.; Gheibi, R.; Batle, J.; Farouk, A.; Habibi, A. A new secure quantum watermarking scheme. Optik 2017, 139, 77–86. [Google Scholar] [CrossRef]
- U.S. District Court Rules in Favor of Copyright Protection for Standards Incorporated by Reference into Federal Regulations. Available online: www.ansi.org (accessed on 24 November 2017).
- Zhang, L.; Liao, X.; Wang, X. An Image Encryption Approach Based on Chaotic Maps. Inf. Sci. 2005, 24, 237–245. [Google Scholar] [CrossRef]
- Fridrich, J. Image Encryption Based on Chaotic Maps. In Proceedings of the IEEE International Conference on Systems, Man, and Cybernetics, Orlando, FL, USA, 12–15 October 1997; pp. 1105–1110. [Google Scholar]
- Peng, J.; Jin, S.; Chen, G.; Yang, Z.; Liao, X. An Image Encryption Scheme Based on Chaotic Map. In Proceedings of the 2008 Fourth International Conference on Natural Computation, Jinan, China, 18–20 October 2008; pp. 595–599. [Google Scholar] [CrossRef]
- Jakobson, M.V. Absolutely Continuous Invariant Measures for One-Parameter Families of One-Dimensional Maps. Commun. Math. Phys. 1981, 81, 39–88. [Google Scholar] [CrossRef]
- Kwok, H.S.; Tang, W.K.S. A Fast Image Encryption System Based on Chaotic Maps with Finite Precision Representation. Chaos Solitons Fractals 2007, 33, 1919–1928. [Google Scholar] [CrossRef]
- May, R.M. Simple Mathematical Models with Very Complicated Dynamics. Nature 1976, 261, 459–467. [Google Scholar] [CrossRef] [PubMed]
- Pareek, N.K.; Patidar, V.; Sud, K.K. Image Encryption Using Chaotic Logistic Map. Image Vis. Comput. 2006, 24, 926–934. [Google Scholar] [CrossRef]
- Thum, C. Measurement of the Entropy of an Image with Application to Image Focusing. IEEE Trans. Pattern Anal. Mach. Intell. 1984, 31, 203–211. [Google Scholar] [CrossRef]
- Wu, X.; Wang, K.; Wang, X.; Kan, H. Lossless Chaotic Color Image Cryptosystem Based on DNA Encryption and Entropy. Nonlinear Dyn. 2017, 87, 855–875. [Google Scholar] [CrossRef]
- Cheng, H.D.; Jiang, X.H.; Wang, J. Color Image Segmentation Based on Histogram Thresholding and Region Merging. Pattern Recognit. 2002, 35, 373–393. [Google Scholar] [CrossRef]
- Wu, Y.; Zhou, Y.; Saveriades, G.; Agaian, S.; Noonan, J.P.; Natarajan, P. Local Shannon Entropy Measure with Statistical Tests for Image Randomness. Inf. Sci. 2013, 222, 323–342. [Google Scholar] [CrossRef]
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Pisarchik, A.N.; Zanin, M. Image Encryption with Chaotically Coupled Chaotic Maps. Phys. D Nonlinear Phenom. 2008, 237, 2638–2648. [Google Scholar] [CrossRef]
- Rhouma, R.; Meherzi, S.; Belghith, S. OCML-Based Colour Image Encryption. Inf. Sci. 2009, 40, 309–318. [Google Scholar] [CrossRef]
- Mackenzie, C.E. Coded Character Sets: Overview of Image Steganography, 1st ed.; The Systems Programming Series; Addison-Wesley Publishing Company, Inc.: Boston, MA, USA, 1980. [Google Scholar]
- Abdullah, A.H.; Enayatifar, R.; Lee, M. A Hybrid Genetic Algorithm and Chaotic Function Model for Image Encryption. Appl. Math. Comput. 2012, 66, 185–198. [Google Scholar] [CrossRef]















| QIR | Qubits (GI) | Qubits (CI) | Pixel Encoding |
|---|---|---|---|
| FRQI | 2n + 1 | --- | Amplitude |
| MCQI | --- | 2n + 3 | Amplitude |
| NASS | 2n | 2n | Amplitude |
| NEQR | 2n + 8 | --- | Basis states |
| QMCR | --- | 2n + 24 | Basis states |
| GNEQR | 2n + 8 | 2n + 24 | Basis states |
| BRQI | 2n + 4 | 2n + 6 | Basis states |
| Gate Type | Circuit | Matrix |
|---|---|---|
| NOT |  | |
| Identity |  | |
| Hadamard |  | |
| Pauli-X |  | |
| Pauli-Y |  | |
| Pauli-Z |  | |
 |
| Gate Type | Circuit | Matrix | |
|---|---|---|---|
| CNOT |  | ||
| Swap |  | ||
| 0CNOT |  | ||
| Toffoli |  | ||
| Image | Red | Green | Blue | Average | |
|---|---|---|---|---|---|
| Lena | Plain | 7.26647529 | 7.57641548 | 6.99698477 | 7.75230230 |
| encrypted | 7.99706260 | 7.99747581 | 7.99666707 | 7.99914383 | |
| Image-1 | Plain | 4.78364119 | 4.73370893 | 5.05956128 | 4.86826736 |
| encrypted | 7.99166776 | 7.99227987 | 7.99282306 | 7.99439984 | |
| Image-2 | Plain | 4.67421401 | 4.85111012 | 5.01229852 | 4.87986000 |
| encrypted | 7.99187429 | 7.99281031 | 7.99301983 | 7.99462144 | |
| Image-3 | Plain | 7.64643957 | 7.33613706 | 7.64931401 | 7.66549665 |
| encrypted | 7.99729804 | 7.99722871 | 7.99718760 | 7.99893469 | |
| Image-4 | Plain | 7.36835372 | 7.63346757 | 7.14444474 | 7.74964698 |
| encrypted | 7.99633432 | 7.99722933 | 7.99718431 | 7.99910385 | |
| Image-5 | Plain | 7.91513367 | 7.73168494 | 7.64447837 | 7.88854042 |
| encrypted | 7.99727711 | 7.99705858 | 7.99729900 | 7.99914117 | |
| Image | Red | Green | Blue | ||
|---|---|---|---|---|---|
| Lena | Plain | horizontal | 0.94496092 | 0.94394613 | 0.90382255 |
| vertical | 0.97160053 | 0.97138740 | 0.94575043 | ||
| diagonal | 0.92060182 | 0.92063101 | 0.86947909 | ||
| Encrypted | horizontal | 0.04684634 | 0.05272363 | 0.05873554 | |
| vertical | −0.08600054 | −0.07969069 | −0.06718450 | ||
| diagonal | 0.03889228 | 0.03631901 | 0.05433776 | ||
| Image-1 | Plain | horizontal | 0.92722854 | 0.92541146 | 0.92171877 |
| vertical | 0.93122544 | 0.92875145 | 0.92504564 | ||
| diagonal | 0.88693262 | 0.88339981 | 0.87809024 | ||
| Encrypted | horizontal | −0.04400776 | −0.04404985 | −0.03493985 | |
| vertical | −0.02690263 | −0.02770975 | −0.01463662 | ||
| diagonal | −0.04237329 | −0.04804702 | −0.03113912 | ||
| Image-2 | Plain | horizontal | 0.79642455 | 0.81825466 | 0.82329247 |
| vertical | 0.85224205 | 0.86751412 | 0.86920629 | ||
| diagonal | 0.70727947 | 0.73512158 | 0.74101511 | ||
| Encrypted | horizontal | −0.03844896 | −0.03945518 | −0.02958604 | |
| vertical | −0.03513343 | −0.04033299 | −0.02344154 | ||
| diagonal | −0.03173505 | −0.03945518 | −0.02184033 | ||
| Image-3 | Plain | horizontal | 0.93243145 | 0.89115097 | 0.93705562 |
| vertical | 0.91626012 | 0.86801252 | 0.92639075 | ||
| diagonal | 0.89600855 | 0.83303664 | 0.90363931 | ||
| Encrypted | horizontal | 0.05921598 | 0.06870314 | 0.07175162 | |
| vertical | −0.01380835 | −0.00194525 | 0.00106863 | ||
| diagonal | 0.05774048 | 0.06055552 | 0.07260252 | ||
| Image-4 | Plain | horizontal | 0.95116615 | 0.97288558 | 0.94465043 |
| vertical | 0.95408423 | 0.97791858 | 0.95067943 | ||
| diagonal | 0.92085361 | 0.95553869 | 0.91182653 | ||
| Encrypted | horizontal | 0.03727045 | 0.04323486 | 0.04399785 | |
| vertical | −0.08462279 | −0.07850834 | −0.07398065 | ||
| diagonal | 0.03837588 | 0.03645186 | 0.04947882 | ||
| Image-5 | Plain | horizontal | 0.91795308 | 0.90593869 | 0.95240375 |
| vertical | 0.89809097 | 0.88392991 | 0.94136339 | ||
| diagonal | 0.87193455 | 0.86353650 | 0.93517466 | ||
| Encrypted | horizontal | 0.04486794 | 0.05486874 | 0.05032741 | |
| vertical | −0.05127246 | −0.04212630 | −0.04436001 | ||
| diagonal | 0.03982164 | 0.04169260 | 0.04825240 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Basiri, S.; Farhang Matin, L.; Naseri, M. Innovative Quantum Encryption Method for RGB Images Based on Bit-Planes and Logistic Maps. Computation 2025, 13, 56. https://doi.org/10.3390/computation13020056
Basiri S, Farhang Matin L, Naseri M. Innovative Quantum Encryption Method for RGB Images Based on Bit-Planes and Logistic Maps. Computation. 2025; 13(2):56. https://doi.org/10.3390/computation13020056
Chicago/Turabian StyleBasiri, Saeed, Laleh Farhang Matin, and Mosayeb Naseri. 2025. "Innovative Quantum Encryption Method for RGB Images Based on Bit-Planes and Logistic Maps" Computation 13, no. 2: 56. https://doi.org/10.3390/computation13020056
APA StyleBasiri, S., Farhang Matin, L., & Naseri, M. (2025). Innovative Quantum Encryption Method for RGB Images Based on Bit-Planes and Logistic Maps. Computation, 13(2), 56. https://doi.org/10.3390/computation13020056







