Co-Solvent Exfoliation of Hexagonal Boron Nitride: Effect of Raw Bulk Boron Nitride Size and Co-Solvent Composition
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
2.3. Characterizations
2.4. Statistical Analysis
3. Results and Discussion
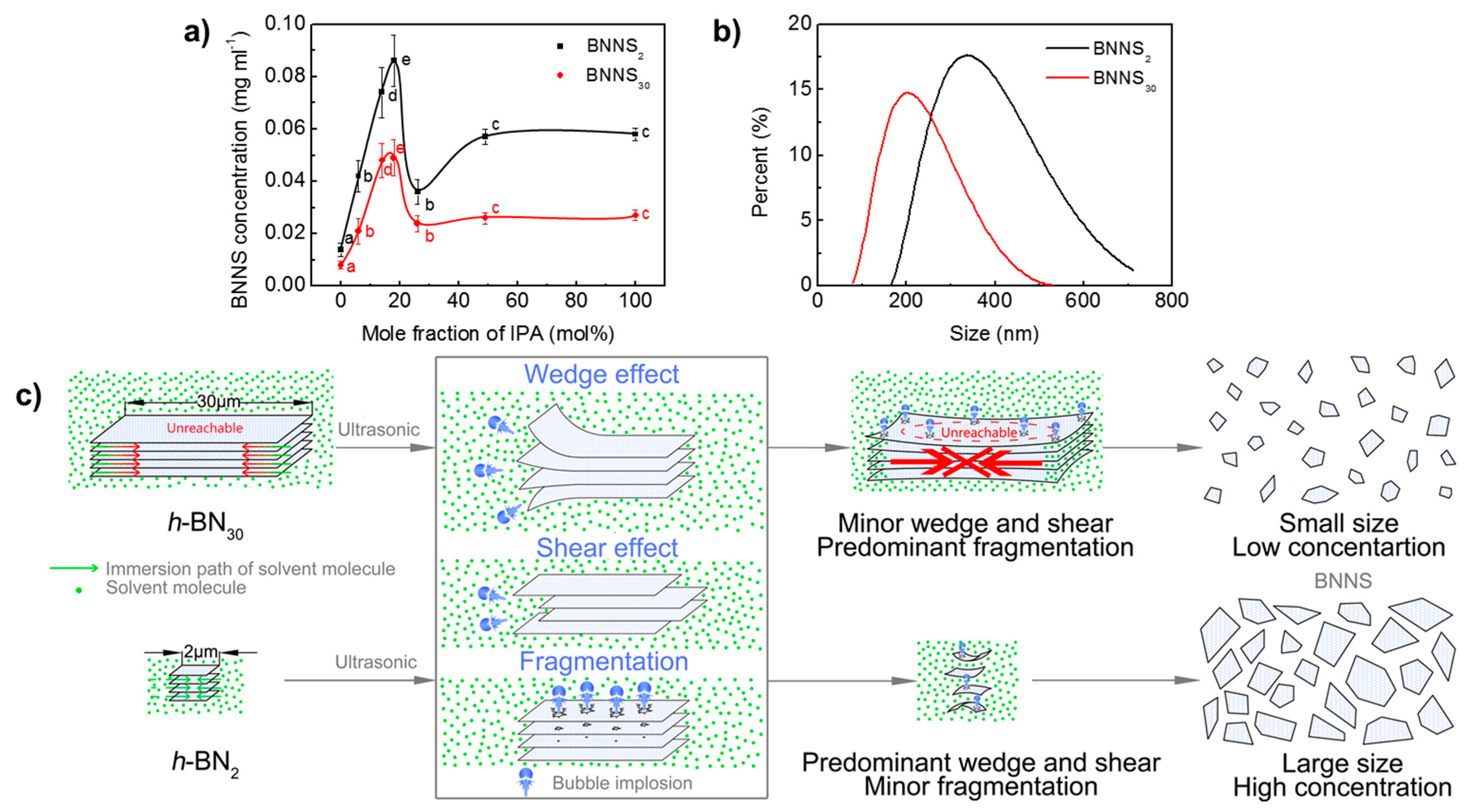
3.1. Effect of Raw h-BN Size
3.2. Effect of Centrifugation Speed and Ultrasonic Time
3.3. Effect of Co-Solvent Compositions
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Vijayaraghavan, V.; Zhang, L. Effective mechanical properties and thickness determination of boron nitride nanosheets using molecular dynamics simulation. Nanomaterials 2018, 8, 546. [Google Scholar] [CrossRef]
- Gonzalez Ortiz, D.; Pochat-Bohatier, C.; Cambedouzou, J.; Bechelany, M.; Miele, P. Exfoliation of hexagonal boron nitride (h-BN) in liquide phase by ion intercalation. Nanomaterials 2018, 8, 716. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.; Connell, J.W. Advances in 2D boron nitride nanostructures: Nanosheets, nanoribbons, nanomeshes, and hybrids with graphene. Nanoscale 2012, 4, 6908–6939. [Google Scholar] [CrossRef] [PubMed]
- Suzuki, S.; Pallares, R.M.; Hibino, H. Growth of atomically thin hexagonal boron nitride films by diffusion through a metal film and precipitation. J. Physi. D Appl. Phys. 2012, 45, 385304. [Google Scholar] [CrossRef]
- Kim, K.K.; Hsu, A.; Jia, X.; Kim, S.M.; Shi, Y.; Dresselhaus, M.; Palacios, T.; Kong, J. Synthesis and characterization of hexagonal boron nitride film as a dielectric layer for graphene devices. ACS Nano 2012, 6, 8583–8590. [Google Scholar] [CrossRef] [PubMed]
- Zeng, X.; Yao, Y.; Gong, Z.; Wang, F.; Sun, R.; Xu, J.; Wong, C.P. Ice-templated assembly strategy to construct 3D boron nitride nanosheet networks in polymer composites for thermal conductivity improvement. Small 2015, 11, 6205–6213. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Gong, Y.; Zhou, W.; Ma, L.; Yu, J.; Idrobo, J.C.; Jung, J.; MacDonald, A.H.; Vajtai, R.; Lou, J. Ultrathin high-temperature oxidation-resistant coatings of hexagonal boron nitride. Nat. Commun. 2013, 4, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Xu, L.; Yang, Z.; Xie, H.; Jiang, P.; Dai, J.; Luo, W.; Yao, Y.; Hitz, E.; Yang, R. High temperature thermal management with boron nitride nanosheets. Nanoscale 2018, 10, 167–173. [Google Scholar] [CrossRef] [PubMed]
- Fu, L.; Wang, T.; Yu, J.; Dai, W.; Sun, H.; Liu, Z.; Sun, R.; Jiang, N.; Yu, A.; Lin, C.-T. An ultrathin high-performance heat spreader fabricated with hydroxylated boron nitride nanosheets. 2D Mater. 2017, 4, 025047. [Google Scholar] [CrossRef]
- Yao, Y.; Zeng, X.; Wang, F.; Sun, R.; Xu, J.-b.; Wong, C.-P. Significant enhancement of thermal conductivity in bioinspired freestanding boron nitride papers filled with graphene oxide. Chem. Mater. 2016, 28, 1049–1057. [Google Scholar] [CrossRef]
- Wang, M.; Wang, H.; An, L.; Zhang, B.; Huang, X.; Wen, G.; Zhong, B.; Yu, Y. Facile fabrication of Hildewintera-colademonis-like hexagonal boron nitride/carbon nanotube composite having light weight and enhanced microwave absorption. J. Colloid Interface Sci. 2020, 564, 454–466. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Yao, Y.; Zeng, X.; Huang, T.; Sun, R.; Xu, J.; Wong, C.-P. Highly thermally conductive polymer nanocomposites based on boron nitride nanosheets decorated with silver nanoparticles. RSC Adv. 2016, 6, 41630–41636. [Google Scholar] [CrossRef]
- Sajjad, M.; Makarov, V.; Mendoza, F.; Sultan, M.S.; Aldalbahi, A.; Feng, P.X.; Jadwisienczak, W.M.; Weiner, B.R.; Morell, G. Synthesis, Characterization and fabrication of graphene/boron nitride nanosheets heterostructure tunneling devices. Nanomaterials 2019, 9, 925. [Google Scholar] [CrossRef] [PubMed]
- Aldalbahi, A.; Rivera, M.; Rahaman, M.; Zhou, A.F.; Mohammed Alzuraiqi, W.; Feng, P. High-performance and self-powered deep UV photodetectors based on high quality 2D boron nitride nanosheets. Nanomaterials 2017, 7, 454. [Google Scholar] [CrossRef]
- Luo, W.; Wang, Y.; Hitz, E.; Lin, Y.; Yang, B.; Hu, L. Solution processed boron nitride nanosheets: Synthesis, assemblies and emerging applications. Adv. Funct. Mater. 2017, 27, 1701450. [Google Scholar] [CrossRef]
- Halim, U.; Zheng, C.R.; Chen, Y.; Lin, Z.; Jiang, S.; Cheng, R.; Huang, Y.; Duan, X. A rational design of cosolvent exfoliation of layered materials by directly probing liquid–solid interaction. Nat. Commun. 2013, 4, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Ciesielski, A.; Samorì, P. Graphene via sonication assisted liquid-phase exfoliation. Chem. Soc. Rev. 2014, 43, 381–398. [Google Scholar] [CrossRef]
- Manna, K.; Huang, H.-N.; Li, W.-T.; Ho, Y.-H.; Chiang, W.-H. Toward understanding the efficient exfoliation of layered materials by water-assisted cosolvent liquid-phase exfoliation. Chem. Mater. 2016, 28, 7586–7593. [Google Scholar] [CrossRef]
- Coleman, J.N.; Lotya, M.; O’Neill, A.; Bergin, S.D.; King, P.J.; Khan, U.; Young, K.; Gaucher, A.; De, S.; Smith, R.J. Two-dimensional nanosheets produced by liquid exfoliation of layered materials. Science 2011, 331, 568–571. [Google Scholar] [CrossRef]
- Duan, Z.Q.; Liu, Y.T.; Xie, X.M.; Ye, X.Y.; Zhu, X.D. h-BN Nanosheets as 2D Substrates to Load 0D Fe3O4 Nanoparticles: A Hybrid Anode Material for Lithium-Ion Batteries. Chem. Asian J. 2016, 11, 828–833. [Google Scholar] [CrossRef]
- Wu, K.; Liao, P.; Du, R.; Zhang, Q.; Chen, F.; Fu, Q. Preparation of a thermally conductive biodegradable cellulose nanofiber/hydroxylated boron nitride nanosheet film: The critical role of edge-hydroxylation. J. Mater. Chem. A 2018, 6, 11863–11873. [Google Scholar] [CrossRef]
- Lei, Z.; Xu, S.; Wan, J.; Wu, P. Facile preparation and multifunctional applications of boron nitride quantum dots. Nanoscale 2015, 7, 18902–18907. [Google Scholar] [CrossRef] [PubMed]
- Ye, H.; Lu, T.; Xu, C.; Han, B.; Meng, N.; Xu, L. Liquid-Phase exfoliation of hexagonal boron nitride into boron nitride nanosheets in common organic solvents with hyperbranched polyethylene as stabilizer. Macromol. Chem. Phys. 2018, 219, 1700482. [Google Scholar] [CrossRef]
- Zhu, H.; Li, Y.; Fang, Z.; Xu, J.; Cao, F.; Wan, J.; Preston, C.; Yang, B.; Hu, L. Highly thermally conductive papers with percolative layered boron nitride nanosheets. ACS Nano 2014, 8, 3606–3613. [Google Scholar] [CrossRef]
- Habib, T.; Sundaravadivelu Devarajan, D.; Khabaz, F.; Parviz, D.; Achee, T.C.; Khare, R.; Green, M.J. Cosolvents as liquid surfactants for boron nitride nanosheet (BNNS) dispersions. Langmuir 2016, 32, 11591–11599. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.; Williams, T.V.; Xu, T.-B.; Cao, W.; Elsayed-Ali, H.E.; Connell, J.W. Aqueous dispersions of few-layered and monolayered hexagonal boron nitride nanosheets from sonication-assisted hydrolysis: Critical role of water. J. Phys. Chem. C 2011, 115, 2679–2685. [Google Scholar] [CrossRef]
- Kim, J.; Kwon, S.; Cho, D.-H.; Kang, B.; Kwon, H.; Kim, Y.; Park, S.O.; Jung, G.Y.; Shin, E.; Kim, W.-G. Direct exfoliation and dispersion of two-dimensional materials in pure water via temperature control. Nat. Commun. 2015, 6, 1–9. [Google Scholar] [CrossRef]
- Zhou, K.G.; Mao, N.N.; Wang, H.X.; Peng, Y.; Zhang, H.L. A mixed-solvent strategy for efficient exfoliation of inorganic graphene analogues. Angew. Chem. Int. Ed. 2011, 50, 10839–10842. [Google Scholar] [CrossRef]
- Marsh, K.; Souliman, M.; Kaner, R.B. Co-solvent exfoliation and suspension of hexagonal boron nitride. Chem. Commun. 2015, 51, 187–190. [Google Scholar] [CrossRef] [PubMed]
- Hernandez, Y.; Nicolosi, V.; Lotya, M.; Blighe, F.M.; Sun, Z.; De, S.; McGovern, I.; Holland, B.; Byrne, M.; Gun’Ko, Y.K. High-yield production of graphene by liquid-phase exfoliation of graphite. Nat. Nanotechnol. 2008, 3, 563. [Google Scholar] [CrossRef]
- Hernandez, Y.; Lotya, M.; Rickard, D.; Bergin, S.D.; Coleman, J.N. Measurement of multicomponent solubility parameters for graphene facilitates solvent discovery. Langmuir 2010, 26, 3208–3213. [Google Scholar] [CrossRef] [PubMed]
- Zhang, B.; Wu, Q.; Yu, H.; Bulin, C.; Sun, H.; Li, R.; Ge, X.; Xing, R. High-Efficient Liquid Exfoliation of Boron Nitride Nanosheets Using Aqueous Solution of Alkanolamine. Nanoscale Res. Lett. 2017, 12, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Wang, M.; Xu, X.; Ge, Y.; Dong, P.; Baines, R.; Ajayan, P.M.; Ye, M.; Shen, J. Surface tension components ratio: An efficient parameter for direct liquid phase exfoliation. ACS Appl. Mater. Interfaces 2017, 9, 9168–9175. [Google Scholar] [CrossRef] [PubMed]
- Shen, J.; Wu, J.; Wang, M.; Dong, P.; Xu, J.; Li, X.; Zhang, X.; Yuan, J.; Wang, X.; Ye, M. Surface tension components based selection of cosolvents for efficient liquid phase exfoliation of 2D materials. Small 2016, 12, 2741–2749. [Google Scholar] [CrossRef] [PubMed]
- Liu, F.; Li, Q.; Li, Z.; Liu, Y.; Dong, L.; Xiong, C.; Wang, Q. Poly (methyl methacrylate)/boron nitride nanocomposites with enhanced energy density as high temperature dielectrics. Compos. Sci. Technol. 2017, 142, 139–144. [Google Scholar] [CrossRef]
- Yang, Z.; Zhou, L.; Luo, W.; Wan, J.; Dai, J.; Han, X.; Fu, K.; Henderson, D.; Yang, B.; Hu, L. Thermally conductive, dielectric PCM–boron nitride nanosheet composites for efficient electronic system thermal management. Nanoscale 2016, 8, 19326–19333. [Google Scholar] [CrossRef]
- Gorbachev, R.V.; Riaz, I.; Nair, R.R.; Jalil, R.; Britnell, L.; Belle, B.D.; Hill, E.W.; Novoselov, K.S.; Watanabe, K.; Taniguchi, T. Hunting for monolayer boron nitride: Optical and Raman signatures. Small 2011, 7, 465–468. [Google Scholar] [CrossRef]
- Li, L.H.; Cervenka, J.; Watanabe, K.; Taniguchi, T.; Chen, Y. Strong oxidation resistance of atomically thin boron nitride nanosheets. ACS Nano 2014, 8, 1457–1462. [Google Scholar] [CrossRef]
- Li, T.; Li, C.; Cai, Y.; Lin, J.; Long, X.; Wang, L.; Xu, Y.; Sun, J.; Tang, L.; Zhang, Y.-W. Highly efficient mass production of boron nitride nanosheets via a borate nitridation method. J. Phys. Chem. C 2018, 122, 17370–17377. [Google Scholar] [CrossRef]
- Lastoskie, C.; Gubbins, K.E.; Quirke, N. Pore size heterogeneity and the carbon slit pore: A density functional theory model. Langmuir 1993, 9, 2693–2702. [Google Scholar] [CrossRef]
- Guo, F.; Creighton, M.; Chen, Y.; Hurt, R.; Külaots, I. Porous structures in stacked, crumpled and pillared graphene-based 3D materials. Carbon 2014, 66, 476–484. [Google Scholar] [CrossRef] [PubMed]
- Witomska, S.; Leydecker, T.; Ciesielski, A.; Samorì, P. Production and patterning of liquid phase–exfoliated 2D sheets for applications in optoelectronics. Adv. Funct. Mater. 2019, 29, 1901126. [Google Scholar] [CrossRef]
- Tao, H.; Zhang, Y.; Gao, Y.; Sun, Z.; Yan, C.; Texter, J. Scalable exfoliation and dispersion of two-dimensional materials–an update. Phys. Chem. Chem. Phys. 2017, 19, 921–960. [Google Scholar] [CrossRef] [PubMed]
- Shen, J.; He, Y.; Wu, J.; Gao, C.; Keyshar, K.; Zhang, X.; Yang, Y.; Ye, M.; Vajtai, R.; Lou, J. Liquid phase exfoliation of two-dimensional materials by directly probing and matching surface tension components. Nano Lett. 2015, 15, 5449–5454. [Google Scholar] [CrossRef] [PubMed]
- Gao, W.; Zhao, Y.; Yin, H. Lateral size selection of liquid exfoliated hexagonal boron nitride nanosheets. RSC Adv. 2018, 8, 5976–5983. [Google Scholar] [CrossRef]
- Yi, M.; Shen, Z.; Liu, L.; Liang, S. Size-selected boron nitride nanosheets as oxygen-atom corrosion resistant fillers. RSC Adv. 2015, 5, 2983–2987. [Google Scholar] [CrossRef]
- Du, Z.; Zeng, X.; Zhu, M.; Kanta, A.; Liu, Q.; Li, J.; Kong, L.B. Alkyl ethoxylate assisted liquid phase exfoliation of BN nanosheet and its application as interphase for oxide/oxide composites. Ceram. Int. 2018, 44, 21461–21469. [Google Scholar] [CrossRef]
- Liscio, A.; Kouroupis-Agalou, K.; Betriu, X.D.; Kovtun, A.; Treossi, E.; Pugno, N.M.; De Luca, G.; Giorgini, L.; Palermo, V. Evolution of the size and shape of 2D nanosheets during ultrasonic fragmentation. 2D Mater. 2017, 4, 025017. [Google Scholar] [CrossRef]
- Wu, Y.G.; Tabata, M.; Takamuku, T. A Rayleigh light scattering study on mixing states of 2-propanol–water binary mixtures widely used as mobile phase in separation. Talanta 2001, 54, 69–77. [Google Scholar] [CrossRef]
- Ruckenstein, E.; Shulgin, I. Hydrophobic self-assembling in dilute aqueous solutions of alcohols and hydrocarbons. Chem. Eng. Sci. 2001, 56, 5675–5680. [Google Scholar] [CrossRef]
- Dixit, S.; Crain, J.; Poon, W.; Finney, J.L.; Soper, A.K. Molecular segregation observed in a concentrated alcohol–water solution. Nature 2002, 416, 829–832. [Google Scholar] [CrossRef] [PubMed]
- Großmann, G.H.; Ebert, K.H. Formation of clusters in 1-Propanol/Water-mixtures. Berichte Bunsenges. Phys. Chem. 1981, 85, 1026–1029. [Google Scholar] [CrossRef]
- Hayashi, H.; Nishikawa, K.; Iijima, T. Small-angle X-ray scattering study of fluctuations in 1-propanol-water and 2-propanol-water systems. J. Phys. Chem. 1990, 94, 8334–8338. [Google Scholar] [CrossRef]
- Takamuku, T.; Maruyama, H.; Watanabe, K.; Yamaguchi, T. Structure of 1-propanol–water mixtures investigated by large-angle X-ray scattering technique. J. Sol. Chem. 2004, 33, 641–660. [Google Scholar] [CrossRef]
- Jora, M.Z.; Cardoso, M.V.; Sabadini, E. Correlation between viscosity, diffusion coefficient and spin-spin relaxation rate in 1H NMR of water-alcohols solutions. J. Mol. Liq. 2017, 238, 341–346. [Google Scholar] [CrossRef]
- Mialdun, A.; Yasnou, V.; Shevtsova, V.; Königer, A.; Köhler, W.; Alonso de Mezquia, D.; Bou-Ali, M. A comprehensive study of diffusion, thermodiffusion, and Soret coefficients of water-isopropanol mixtures. J. Chem. Phys. 2012, 136, 244512. [Google Scholar] [CrossRef]
- Pang, F.-M.; Seng, C.-E.; Teng, T.-T.; Ibrahim, M. Densities and viscosities of aqueous solutions of 1-propanol and 2-propanol at temperatures from 293.15 K to 333.15 K. J. Mol. Liq. 2007, 136, 71–78. [Google Scholar] [CrossRef]
- Cappelezzo, M.; Capellari, C.; Pezzin, S.; Coelho, L. Stokes-Einstein relation for pure simple fluids. J. Chem. Phys. 2007, 126, 224516. [Google Scholar] [CrossRef] [PubMed]
- Alavi, S.; Takeya, S.; Ohmura, R.; Woo, T.K.; Ripmeester, J.A. Hydrogen-bonding alcohol-water interactions in binary ethanol, 1-propanol, and 2-propanol+ methane structure II clathrate hydrates. J. Chem. Phys. 2010, 133, 074505. [Google Scholar] [CrossRef] [PubMed]
- Iwasaki, K.; Fujiyama, T. Light-scattering study of clathrate hydrate formation in binary mixtures of tert-butyl alcohol and water. J. Phys. Chem. 1977, 81, 1908–1912. [Google Scholar] [CrossRef]
- Atkins, P.W.; De Paula, J.; Keeler, J. Atkins’ Physical Chemistry; Oxford University Press: Oxford, UK, 2018. [Google Scholar]
- Pratt, K.; Wakeham, W. The mutual diffusion coefficient for binary mixtures of water and the isomers of propanol. Proc. R. Soc. Lond. A Math. Phys. Sci. 1975, 342, 401–419. [Google Scholar]
- Li, R.; D’Agostino, C.; McGregor, J.; Mantle, M.D.; Zeitler, J.A.; Gladden, L.F. Mesoscopic structuring and dynamics of alcohol/water solutions probed by terahertz time-domain spectroscopy and pulsed field gradient nuclear magnetic resonance. J. Phys. Chem. B 2014, 118, 10156–10166. [Google Scholar] [CrossRef]
- Micali, N.; Trusso, S.; Vasi, C.; Blaudez, D.; Mallamace, F. Dynamical properties of water-methanol solutions studied by depolarized Rayleigh scattering. Phys. Rev. E 1996, 54, 1720. [Google Scholar] [CrossRef] [PubMed]
- Mukhopadhyay, T.K.; Datta, A. Deciphering the role of solvents in the liquid phase exfoliation of hexagonal boron nitride: A molecular dynamics simulation study. J. Phys. Chem. C 2017, 121, 811–822. [Google Scholar] [CrossRef]
- Gerchman, D.; Alves, A.K. Solution-processable exfoliation and suspension of atomically thin WSe2. J. Colloid Interface Sci. 2016, 468, 247–252. [Google Scholar] [CrossRef] [PubMed]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nie, X.; Li, G.; Jiang, Z.; Li, W.; Ouyang, T.; Wang, J. Co-Solvent Exfoliation of Hexagonal Boron Nitride: Effect of Raw Bulk Boron Nitride Size and Co-Solvent Composition. Nanomaterials 2020, 10, 1035. https://doi.org/10.3390/nano10061035
Nie X, Li G, Jiang Z, Li W, Ouyang T, Wang J. Co-Solvent Exfoliation of Hexagonal Boron Nitride: Effect of Raw Bulk Boron Nitride Size and Co-Solvent Composition. Nanomaterials. 2020; 10(6):1035. https://doi.org/10.3390/nano10061035
Chicago/Turabian StyleNie, Xiang, Guo Li, Zhao Jiang, Wei Li, Ting Ouyang, and Jianfeng Wang. 2020. "Co-Solvent Exfoliation of Hexagonal Boron Nitride: Effect of Raw Bulk Boron Nitride Size and Co-Solvent Composition" Nanomaterials 10, no. 6: 1035. https://doi.org/10.3390/nano10061035
APA StyleNie, X., Li, G., Jiang, Z., Li, W., Ouyang, T., & Wang, J. (2020). Co-Solvent Exfoliation of Hexagonal Boron Nitride: Effect of Raw Bulk Boron Nitride Size and Co-Solvent Composition. Nanomaterials, 10(6), 1035. https://doi.org/10.3390/nano10061035





