Dependencies of Surface Condensation on the Wettability and Nanostructure Size Differences
Abstract
:1. Introduction
2. Simulation Method
3. Results and Discussion
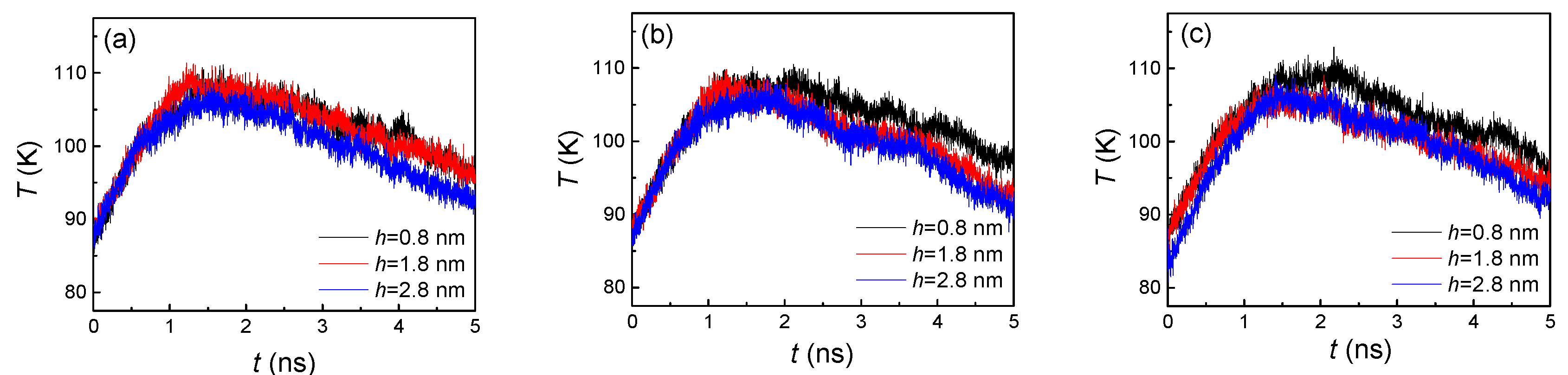
3.1. Effect of the Nanostructure Size on Hydrophilic Surface Condensation
3.2. Effect of Nanostructure Size on Hydrophobic Surface Condensation
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zheng, Y.; Bai, H.; Huang, Z.; Tian, X.; Nie, F.Q.; Zhao, Y.; Zhai, J.; Jiang, L. Directional water collection on wetted spider silk. Nature 2010, 463, 640–643. [Google Scholar] [CrossRef]
- Rose, J.W. Condensation heat transfer fundamentals. Chem. Eng. Res. Des. 1998, 76, 143–152. [Google Scholar] [CrossRef]
- Miljkovic, N.; Preston, D.J.; Enright, R.; Wang, E.N. Electric-field-enhanced condensation on superhydrophobic nanostructured surfaces. ACS Nano 2013, 7, 11043–11054. [Google Scholar] [CrossRef]
- Leach, R.N.; Stevens, F.; Langford, S.C.; Dickinson, J.T. Dropwise condensation: Experiments and simulations of nucleation and growth of water drops in a cooling system. Langmuir 2006, 22, 8864–8872. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Wu, J.; Ma, R.; Hua, M.; Koratkar, N.; Yao, S.; Wang, Z. Nanograssed micropyramidal architectures for continuous dropwise condensation. Adv. Funct. Mater. 2011, 21, 4617–4623. [Google Scholar] [CrossRef]
- Jin, H.; Lin, G.; Bai, L.; Zeiny, A.; Wen, D. Steam generation in a nanoparticle-based solar receiver. Nano Energy 2016, 28, 397–406. [Google Scholar] [CrossRef] [Green Version]
- Wang, S.; Chen, J.; Hu, Y.; Zhang, W. Effect of evaporation section and condensation section length on thermal performance of flat plate heat pipe. Appl. Therm. Eng. 2011, 31, 2367–2373. [Google Scholar] [CrossRef]
- Lips, S.; Bonjour, J.; Lefèvre, F. Investigation of evaporation and condensation processes specific to grooved flat heat pipes. Front. Heat Pipes 2010, 1, 023001. [Google Scholar]
- Liu, Z.H.; Li, Y.Y.; Bao, R. Thermal performance of inclined grooved heat pipes using nanofluids. Int. J. Therm. Sci. 2010, 49, 1680–1687. [Google Scholar] [CrossRef]
- Luo, H.; Lu, Y.; Yin, S.; Huang, S.; Song, J.; Chen, F.; Carmalt, C.J.; Parkin, I.P. Robust platform for water harvesting and directional transport. J. Mater. Chem. A 2018, 6, 5635–5643. [Google Scholar] [CrossRef] [Green Version]
- Miljkovic, N.; Enright, R.; Nam, Y.; Lopez, K.; Dou, N.; Sack, J.; Wang, E.N. Jumping-droplet-enhanced condensation on scalable superhydrophobic nanostructured surfaces. Nano Lett. 2013, 13, 179–187. [Google Scholar] [CrossRef]
- Wen, R.F.; Xu, S.S.; Zhao, D.L.; Lee, Y.C.; Ma, X.H.; Yang, R.G. Hierarchical superhydrophobic surfaces with micropatterned nanowire arrays for high-efficiency jumping droplet condensation. ACS Appl. Mater. Interfaces 2017, 9, 44911–44921. [Google Scholar] [CrossRef] [PubMed]
- Bian, J.C.; Li, Q.; Huang, C.; Li, J.F.; Guo, Y.; Zaw, M.; Zhang, R.Q. Thermal vapor condensation of uniform graphitic carbon nitride films with remarkable photocurrent density for photoelectrochemical applications. Nano Energy 2015, 15, 353–361. [Google Scholar] [CrossRef]
- Attinger, D.; Frankiewicz, C.; Betz, A.R.; Schutzius, T.M.; Ganguly, R.; Das, A.; Kim, C.J.; Megaridis, C.M. Surface engineering for phase change heat transfer: A review. MRS Energy Sustain. 2014, 15, E4. [Google Scholar] [CrossRef] [Green Version]
- Nagayama, G.; Kawagoe, M.; Tokunaga, A.; Tsuruta, T. On the evaporation rate of ultra-thin liquid film at the nanostructured surface: A molecular dynamics study. Int. J. Therm. Sci. 2010, 49, 59–66. [Google Scholar] [CrossRef] [Green Version]
- Gao, S.; Liao, Q.; Liu, W.; Liu, Z.C. Effects of solid fraction on droplet wetting and vapor condensation: A molecular dynamic simulation study. Langmuir 2017, 33, 12379–12388. [Google Scholar] [CrossRef]
- Hasan, M.N.; Shavik, S.M.; Mukut, K.M.; Rabbi, K.F.; Faisal, A.H.M. Atomistic modelling of thin film argon evaporation over different solid surfaces at different wetting conditions. Micro Nano Lett. 2018, 13, 351–356. [Google Scholar] [CrossRef]
- Ou, X.; Wang, X.; Lin, Z.; Li, J. Heterogeneous condensation of water on the mica (001) surface: A molecular dynamics simulation work. J. Phys. Chem. C 2017, 121, 6813–6819. [Google Scholar] [CrossRef]
- Niu, D.; Tang, G.H. The effect of surface wettability on water vapor condensation in nanoscale. Sci. Rep. 2016, 6, 19192. [Google Scholar] [CrossRef] [Green Version]
- Hiratsuka, M.; Emoto, M.; Konno, A.; Ito, S. Molecular dynamics simulation of the influence of nanoscale structure on water wetting and condensation. Micromachines 2019, 10, 587. [Google Scholar] [CrossRef] [Green Version]
- Wang, Q.; Xie, H.; Hu, Z.; Liu, C. The impact of the electric field on surface condensation of water vapor: Insight from molecular dynamics simulation. Nanomaterials 2019, 9, 64. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lu, Z.; Salamon, T.R.; Narayanan, S.; Bagnall, K.R.; Hanks, D.F.; Antao, D.S.; Barabadi, B.; Sircar, J.; Simon, M.E.; Wang, E.N. Design and modeling of membranebased evaporative cooling devices for thermal management of high heat fluxe. IEEE Trans. Compon. Packag. Technol. 2016, 6, 1056–1065. [Google Scholar]
- Humplik, T.; Lee, J.; O’Hern, S.; Fellman, B.; Baig, M.; Hassan, S.; Atieh, M.; Rahman, F.; Laoui, T.; Karnik, R. Nanostructured materials for water desalination. Nanotechnology 2011, 22, 292001. [Google Scholar] [CrossRef] [PubMed]
- Sefiane, K. On the formation of regular patterns from drying droplets and their potential use for bio-medical applications. J. Bionic Eng. 2010, 7, S82–S93. [Google Scholar] [CrossRef]
- Goto, E.; Matsumoto, Y.; Kamoi, M.; Endo, K.; Ishida, R.; Dogru, M.; Kaido, M.; Kojima, T.; Tsubota, K. Tear evaporation rates in Sjögren syndrome and nonSjögren dry eye patients. Am. J. Ophthalmol. 2007, 144, 81–85. [Google Scholar] [CrossRef]
- Yu, J.; Wang, H. A molecular dynamics investigation on evaporation of thin liquid films. Int. J. Heat Mass Transf. 2012, 55, 1218–1225. [Google Scholar] [CrossRef]
- Li, L.; Ji, P.; Zhang, Y. Molecular dynamics simulation of condensation on nanostructured surface in a confined space. Appl. Phys. A Mater. Sci. Process. 2016, 122, 496. [Google Scholar] [CrossRef] [Green Version]
- Yi, P.; Poulikakos, D.; Walther, J.; Yadigaroglu, G. Molecular dynamics simulation of vaporization of an ultra-thin liquid argon layer on a surface. Int. J. Heat Mass Transf. 2002, 145, 2087–2100. [Google Scholar] [CrossRef]
- Kuri, S.K.; Rakibuzzaman, S.M.; Sabah, A.; Ahmed, J.; Hasan, M.N. Effect of nanostructured surface configuration on evaporation and condensation characteristics of thin film liquid argon in a nano-scale confinement. AIP Conf. Proc. 2017, 1919, 020049. [Google Scholar]
- Liang, Z.; Biben, T.; Keblinski, P. Molecular simulation of steady-state evaporation and condensation: Validity of the Schrage relationships. Int. J. Heat Mass Transf. 2017, 114, 105–114. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Nosé, S. Constant-temperature molecular dynamics. J. Phys.-Cond. Matter 1990, 2, 115–119. [Google Scholar] [CrossRef]
- Nosé, S.U.I. A molecular dynamics method for simulations in the canonical ensemble. Mol. Phys. 2002, 100, 191–198. [Google Scholar] [CrossRef]
- Ding, W.Y.; Han, D.; Zhang, J.Z.; Ma, Q.M.; Li, X.Y.; Zhang, J.C. Molecular dynamics study of anisotropic behaviours of water droplet on textured surfaces with various energies. Mol. Phys. 2020. [Google Scholar] [CrossRef]
- Wang, B.B.; Wang, X.D.; Wang, T.H.; Lu, G.; Yan, W.M. Enhancement of boiling heat transfer of thin water film on an electrified solid surface. Int. J. Heat Mass Transf. 2017, 109, 410–416. [Google Scholar] [CrossRef]
- Zhang, K.; Wang, F.; Zhao, X. The self-propelled movement of the water nanodroplet in different surface wettability gradients: A contact angle view. Comput. Mater. Sci. 2016, 124, 190–194. [Google Scholar] [CrossRef]
- Werder, T.; Walther, J.H.; Jaffe, R.L.; Halicioglu, T.; Koumoutsakos, P. On the Water-Carbon Interaction for Use in Molecular Dynamics Simulations of Graphite and Carbon Nanotubes. J. Phys. Chem. B 2003, 107, 1345–1352. [Google Scholar] [CrossRef]
- Wang, Y.H.; Wang, S.Y.; Lu, G.; Wang, X.D. Explosive boiling of nano-liquid argon films on high temperature platinum walls: Effects of surface wettability and film thickness. Int. J. Therm. Sci. 2018, 132, 610–617. [Google Scholar] [CrossRef]
- Liao, M.J.; Duan, L.Q. Explosive boiling of liquid argon films on flat and nanostructured surfaces. Numer. Heat Tranf. A Appl. 2020, 78, 94–105. [Google Scholar] [CrossRef]









© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liao, M.-J.; Duan, L.-Q. Dependencies of Surface Condensation on the Wettability and Nanostructure Size Differences. Nanomaterials 2020, 10, 1831. https://doi.org/10.3390/nano10091831
Liao M-J, Duan L-Q. Dependencies of Surface Condensation on the Wettability and Nanostructure Size Differences. Nanomaterials. 2020; 10(9):1831. https://doi.org/10.3390/nano10091831
Chicago/Turabian StyleLiao, Ming-Jun, and Li-Qiang Duan. 2020. "Dependencies of Surface Condensation on the Wettability and Nanostructure Size Differences" Nanomaterials 10, no. 9: 1831. https://doi.org/10.3390/nano10091831
APA StyleLiao, M.-J., & Duan, L.-Q. (2020). Dependencies of Surface Condensation on the Wettability and Nanostructure Size Differences. Nanomaterials, 10(9), 1831. https://doi.org/10.3390/nano10091831



