Atomistic Simulations on Metal Rod Penetrating Thin Target at Nanoscale Caused by High-Speed Collision
Abstract
:1. Introduction
2. Simulation Details
3. Results and Discussion
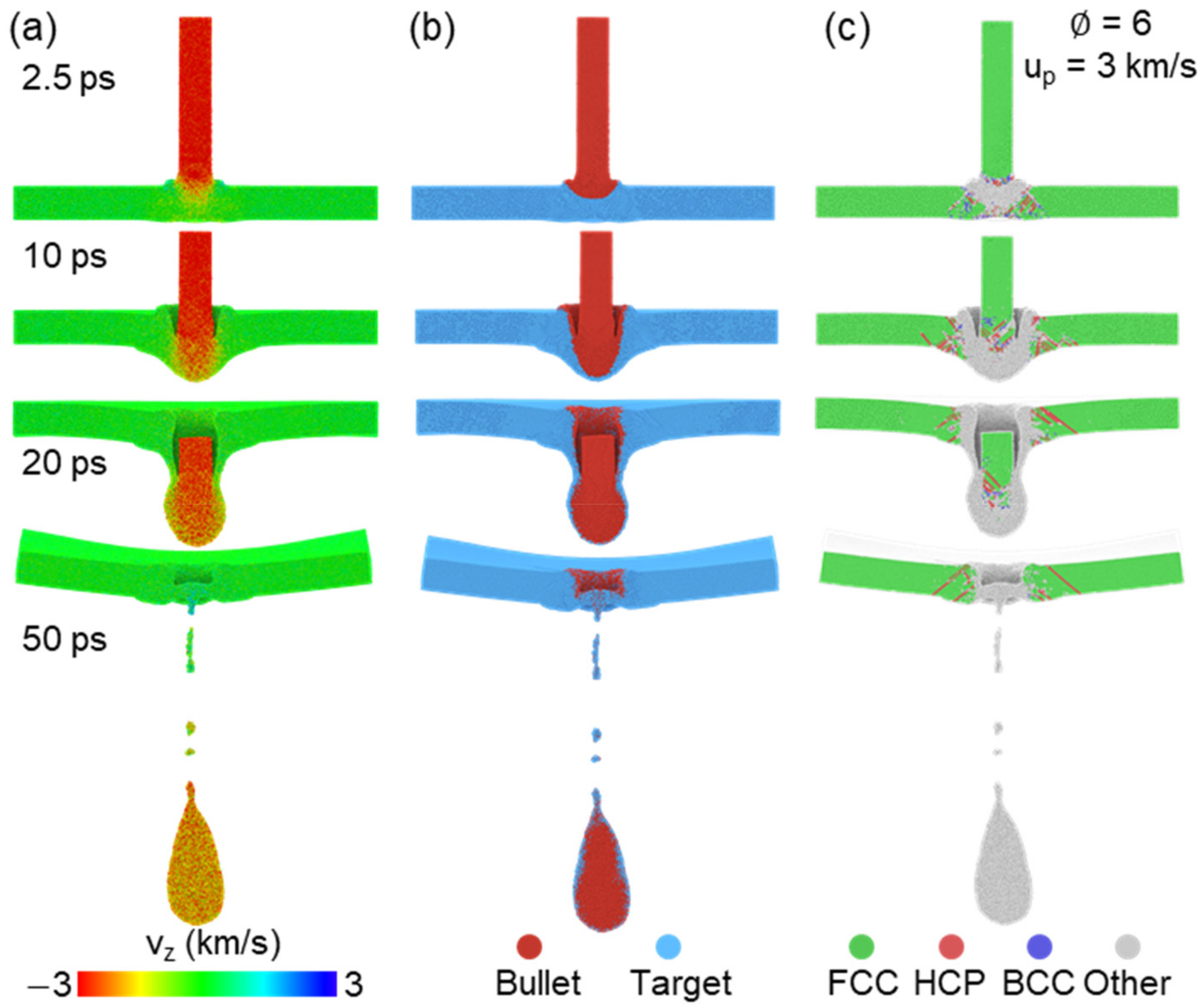
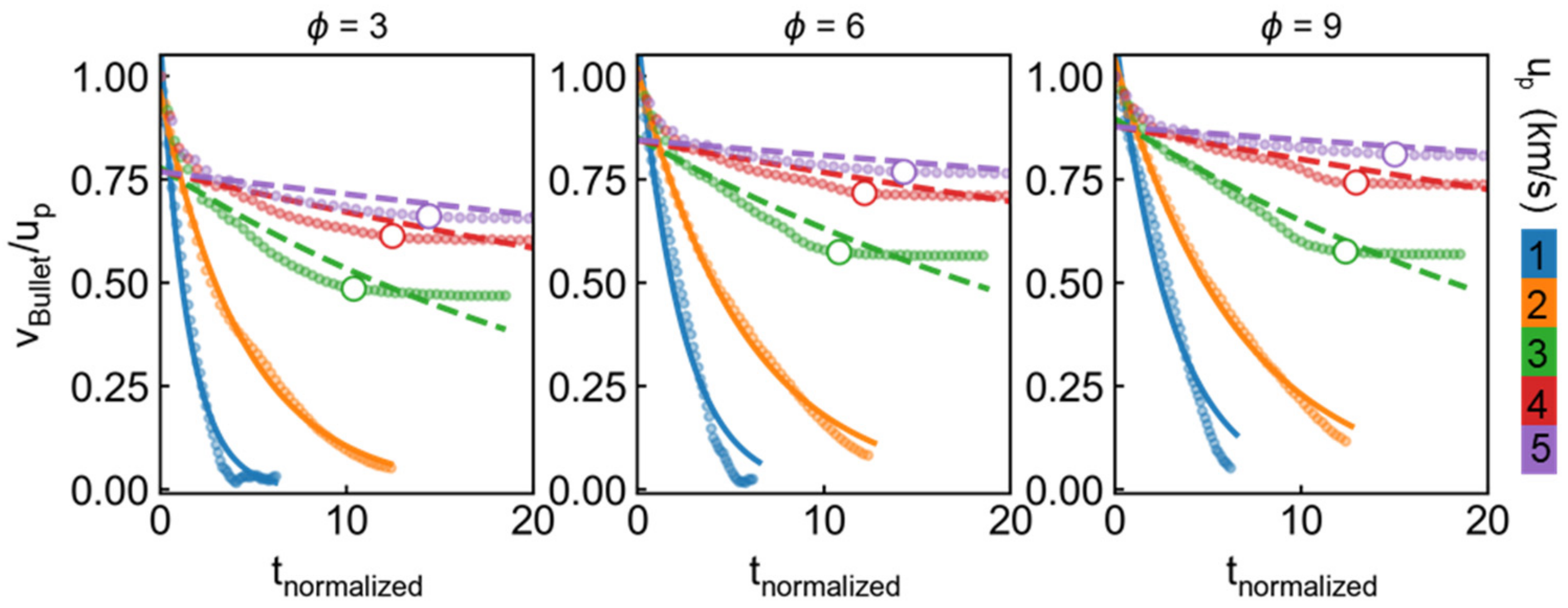
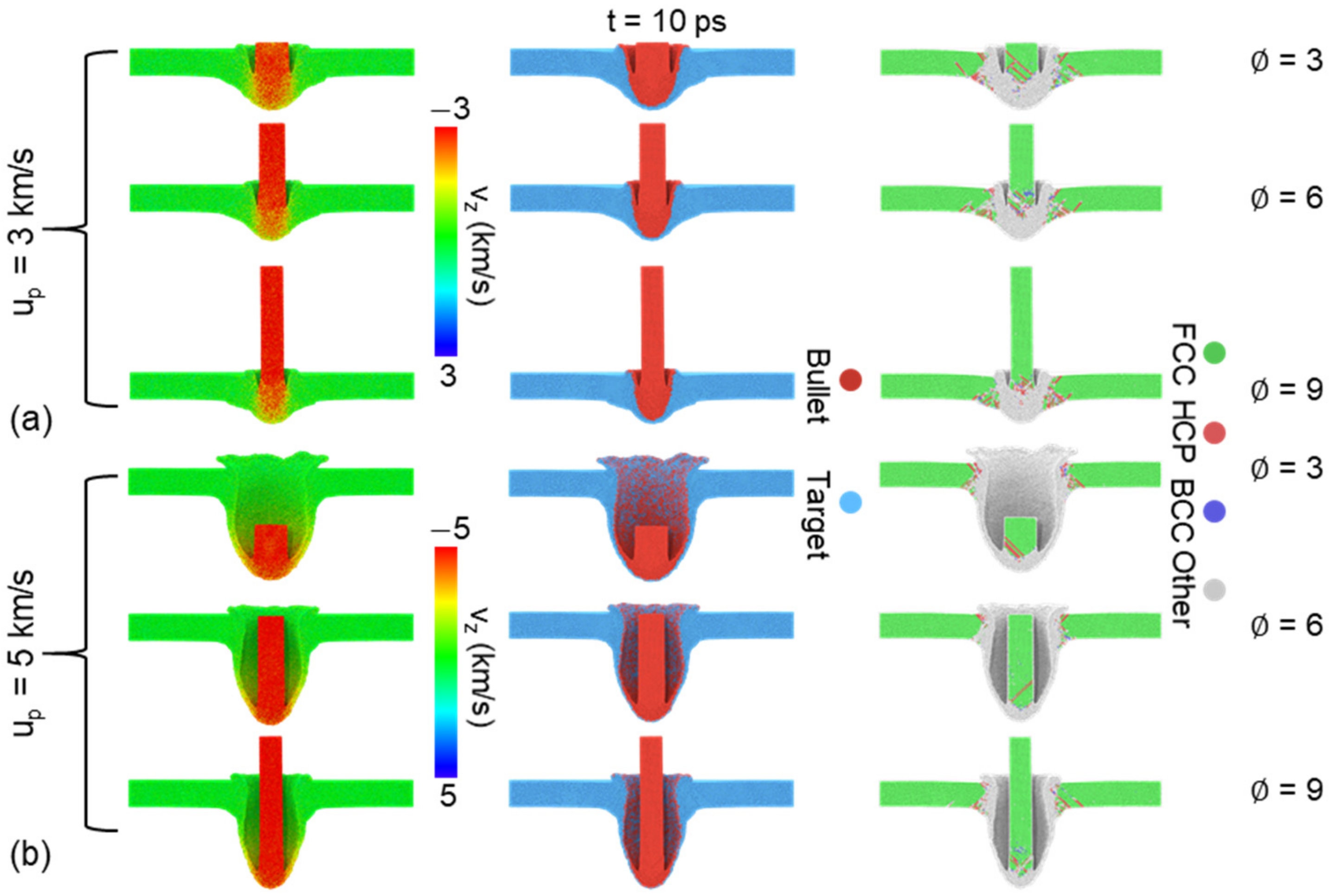
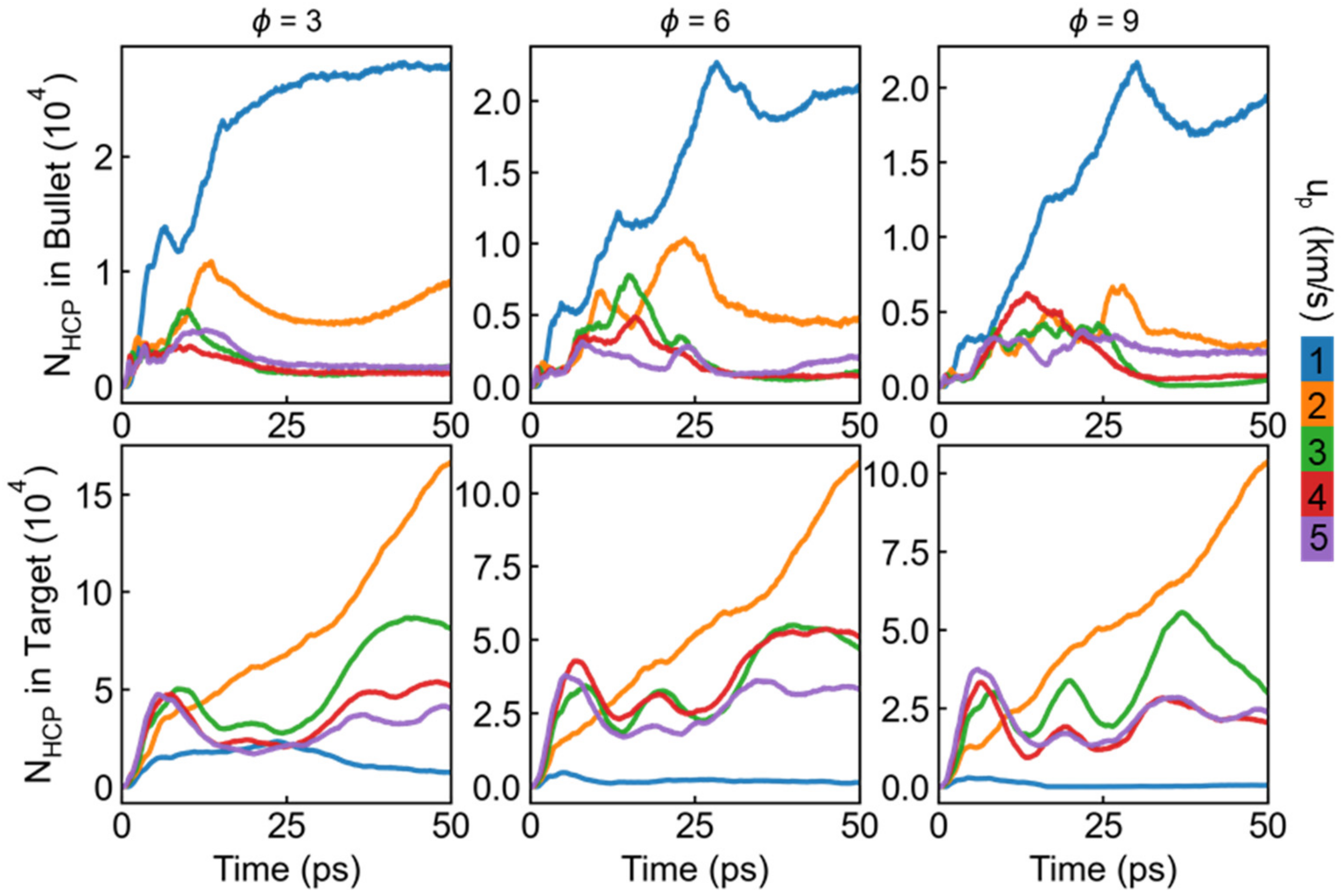
3.1. Characteristics of Penetration at Nanoscale
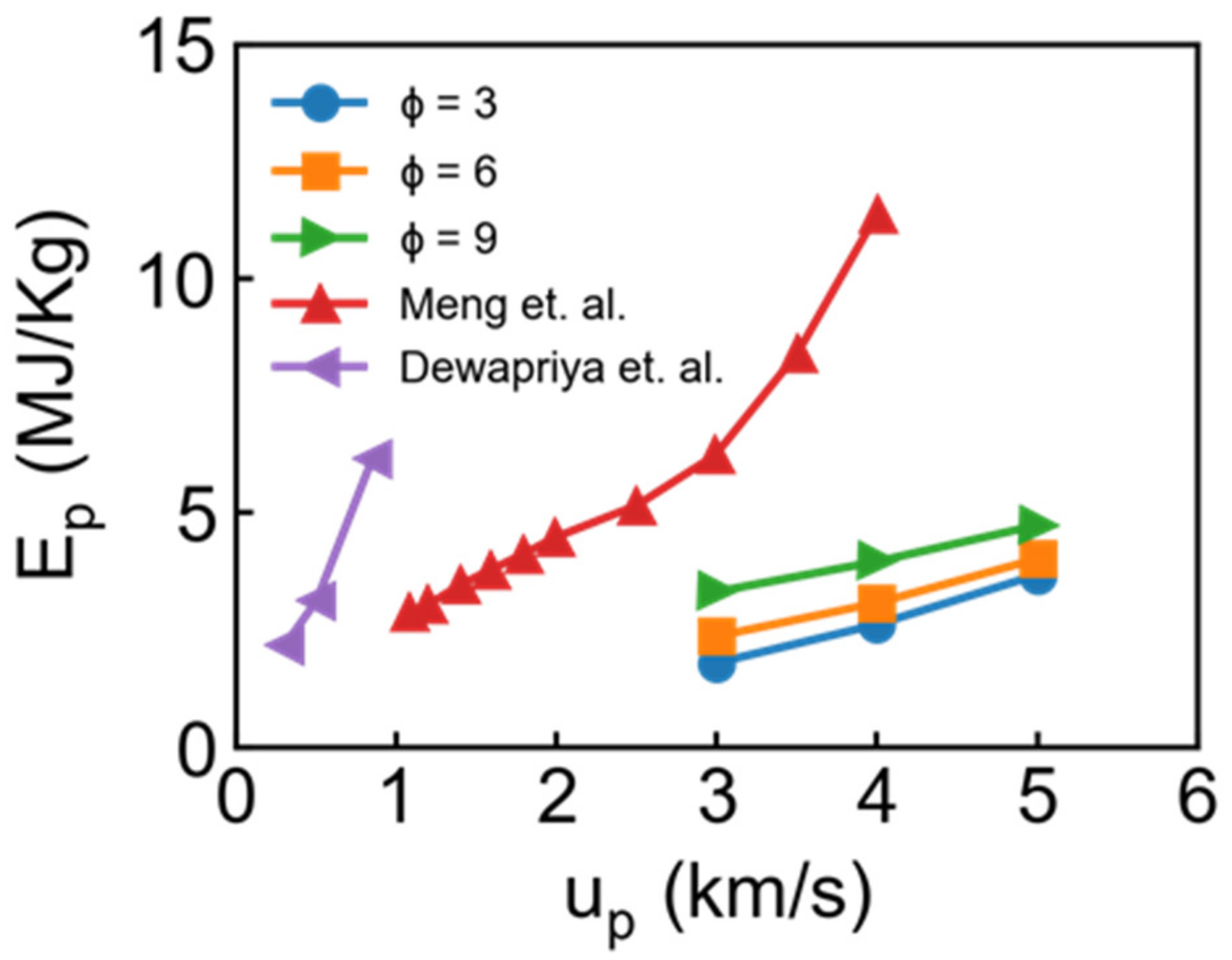
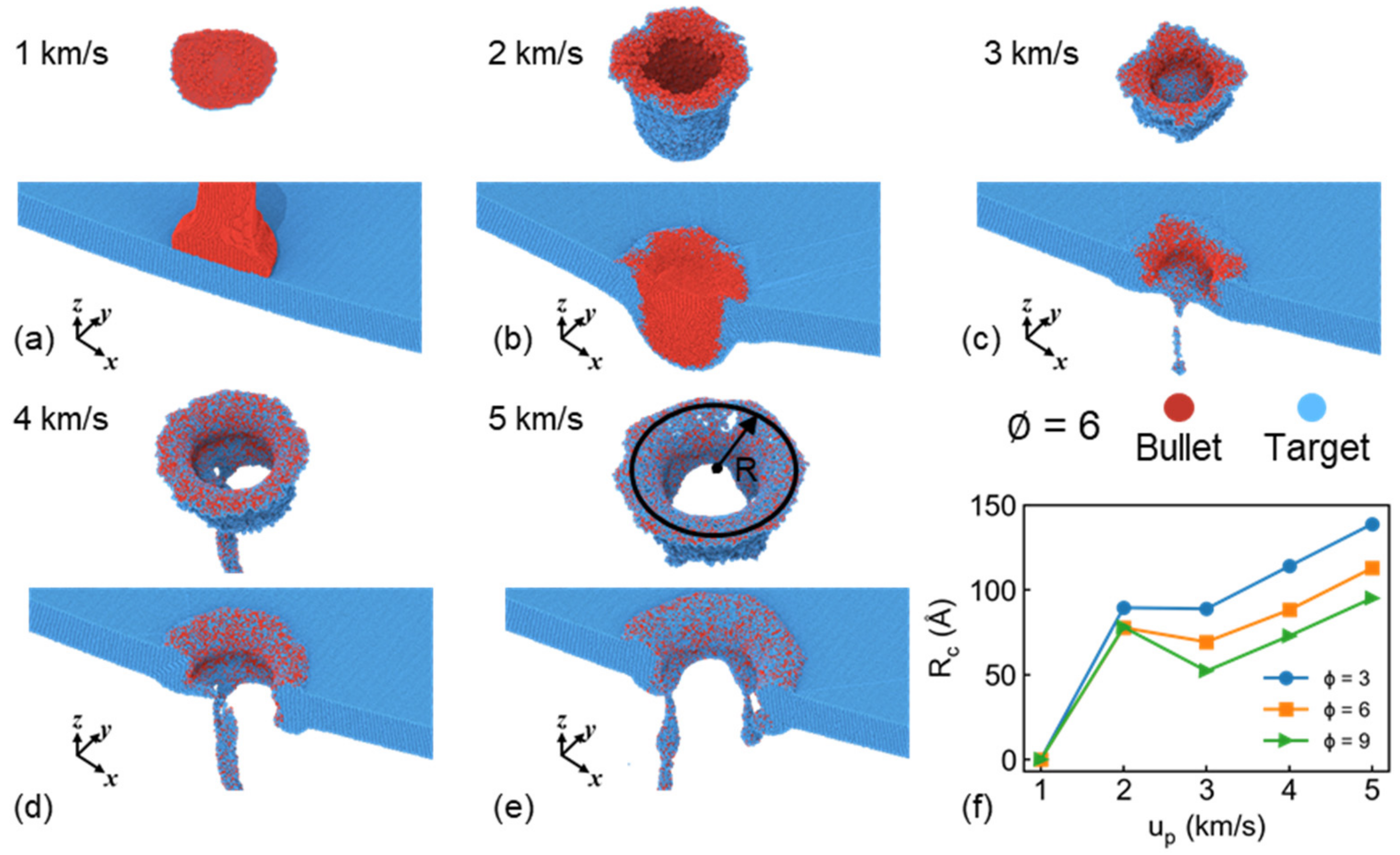
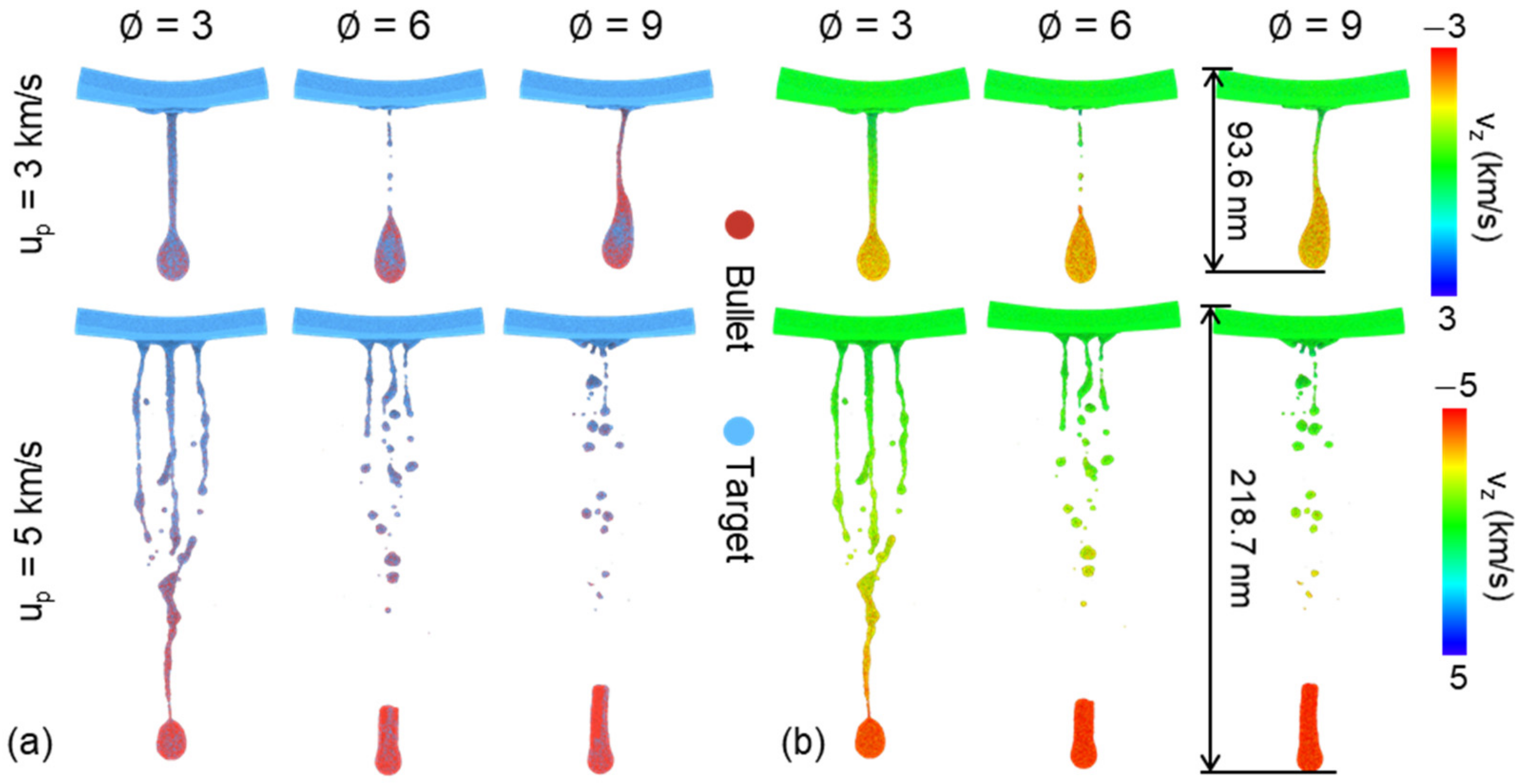
3.2. Crater and Fragematation Process
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Grossman, E.; Gouzman, I.; Verker, R. Debris/Micrometeoroid Impacts and Synergistic Effects on Spacecraft Materials. MRS Bull. 2010, 35, 41–47. [Google Scholar] [CrossRef]
- Wen, K.; Chen, X.-W.; Lu, Y.-G. Research and development on hypervelocity impact protection using Whipple shield: An overview. Def. Technol. 2020. [Google Scholar] [CrossRef]
- Schonberg, W.P. Studies of hypervelocity impact phenomena as applied to the protection of spacecraft operating in the MMOD environment. Procedia Eng. 2017, 204, 4–42. [Google Scholar] [CrossRef]
- Schonberg, W.; Schäfer, F.; Putzar, R. Hypervelocity impact response of honeycomb sandwich panels. Acta Astronaut. 2010, 66, 455–466. [Google Scholar] [CrossRef]
- Kanel, G.I.; Fortov, V.E.; Razorenov, S.V. Shock-Wave Phenomena and the Properties of Condensed Matter; Springer: New York, NY, USA, 2004. [Google Scholar]
- Ramjaun, D.; Kato, I.; Takayama, K.; Jagadeesh, G. Hypervelocity Impacts on Thin Metallic and Composite Space Debris Bumper Shields. AIAA J. 2003, 41, 1564–1572. [Google Scholar] [CrossRef]
- Liu, M.; Su, Z.; Zhang, Q.; Long, R. Modeling Hypervelocity-Impact-Induced Shock Waves for Characterizing Orbital Debris-Produced Damage. J. Appl. Mech. 2016, 83, 081010. [Google Scholar] [CrossRef]
- dos Santos, T.; Rodríguez-Martínez, J.A. Size effects on the plastic shock formation in steady-state cavity expansion in porous ductile materials. Mech. Res. Commun. 2021, 113, 103690. [Google Scholar] [CrossRef]
- Li, S.; Duan, Z.; Gao, T.; Wang, X.; Ou, Z.; Huang, F. Size effect of explosive particle on shock initiation of aluminized 2,4-dinitroanisole (DNAN)-based melt-cast explosive. J. Appl. Phys. 2020, 128, 125903. [Google Scholar] [CrossRef]
- Wu, Y.-C.; Shao, J.-L.; Zhan, H. Damage characteristics of aluminum nanorod under hypervelocity impact. Comput. Mater. Sci. 2020, 174, 109490. [Google Scholar] [CrossRef]
- Dremov, V.; Petrovtsev, A.; Sapozhnikov, P.; Smirnova, M.; Preston, D.L.; Zocher, M.A. Molecular dynamics simulations of the initial stages of spall in nanocrystalline copper. Phys. Rev. B 2006, 74, 144110. [Google Scholar] [CrossRef]
- Dongare, A.M.; Rajendran, A.M.; LaMattina, B.; Zikry, M.A.; Brenner, D.W. Atomic scale simulations of ductile failure micromechanisms in nanocrystalline Cu at high strain rates. Phys. Rev. B 2009, 80, 104108. [Google Scholar] [CrossRef] [Green Version]
- Hahn, E.N.; Fensin, S.J.; Germann, T.C.; Gray, G.T. Orientation dependent spall strength of tantalum single crystals. Acta Mater. 2018, 159, 241–248. [Google Scholar] [CrossRef]
- Kuksin, A.; Norman, G.; Stegailov, V.; Yanilkin, A.; Zhilyaev, P. Dynamic fracture kinetics, influence of temperature and microstructure in the atomistic model of aluminum. Int. J. Fract. 2009, 162, 127–136. [Google Scholar] [CrossRef]
- Shao, J.-L.; Wang, C.; Wang, P.; He, A.-M.; Zhang, F.-G. Atomistic simulations and modeling analysis on the spall damage in lead induced by decaying shock. Mech. Mater. 2019, 131, 78–83. [Google Scholar] [CrossRef]
- Kanel, G.I. Spall fracture: Methodological aspects, mechanisms and governing factors. Int. J. Fract. 2010, 163, 173–191. [Google Scholar] [CrossRef]
- Remington, T.P.; Remington, B.A.; Hahn, E.N.; Meyers, M.A. Deformation and failure in extreme regimes by high-energy pulsed lasers: A review. Mater. Sci. Eng. A 2017, 688, 429–458. [Google Scholar] [CrossRef] [Green Version]
- Turley, W.D.; Fensin, S.J.; Hixson, R.S.; Jones, D.R.; La Lone, B.M.; Stevens, G.D.; Thomas, S.A.; Veeser, L.R. Spall response of single-crystal copper. J. Appl. Phys. 2018, 123, 055102. [Google Scholar] [CrossRef] [Green Version]
- Neogi, A.; Mitra, N. Shock induced deformation response of single crystal copper: Effect of crystallographic orientation. Comput. Mater. Sci. 2017, 135, 141–151. [Google Scholar] [CrossRef]
- Mayer, A.E.; Mayer, P.N. Evolution of pore ensemble in solid and molten aluminum under dynamic tensile fracture: Molecular dynamics simulations and mechanical models. Int. J. Mech. Sci. 2019, 157–158, 816–832. [Google Scholar] [CrossRef]
- Zhu, Q.; Shao, J.-L.; Pan, H.; Wang, P. Collapse of stacking fault tetrahedron and dislocation evolution in copper under shock compression. J. Nucl. Mater. 2021, 554, 153081. [Google Scholar] [CrossRef]
- Wu, W.-D.; Shao, J.-L. Atomistic study on the dynamic response of the void or helium bubble in aluminum under compression and tension. J. Appl. Phys. 2020, 127, 154902. [Google Scholar] [CrossRef] [Green Version]
- Remington, T.P.; Hahn, E.N.; Zhao, S.; Flanagan, R.; Mertens, J.C.E.; Sabbaghianrad, S.; Langdon, T.G.; Wehrenberg, C.E.; Maddox, B.R.; Swift, D.C.; et al. Spall strength dependence on grain size and strain rate in tantalum. Acta Mater. 2018, 158, 313–329. [Google Scholar] [CrossRef] [Green Version]
- Li, W.; Hahn, E.N.; Yao, X.; Germann, T.C.; Feng, B.; Zhang, X. On the grain size dependence of shock responses in nanocrystalline sic ceramics at high strain rates. Acta Mater. 2020, 200, 632–651. [Google Scholar] [CrossRef]
- Branicio, P.S.; Kalia, R.K.; Nakano, A.; Vashishta, P.; Shimojo, F.; Rino, J.P. Atomistic damage mechanisms during hypervelocity projectile impact on AlN: A large-scale parallel molecular dynamics simulation study. J. Mech. Phys. Solids 2008, 56, 1955–1988. [Google Scholar] [CrossRef]
- Dewapriya, M.A.N.; Miller, R.E. Molecular dynamics study of the penetration resistance of multilayer polymer/ceramic nanocomposites under supersonic projectile impacts. Extrem. Mech. Lett. 2021, 44, 101238. [Google Scholar] [CrossRef]
- Mayer, A.E.; Ebel, A.A. Influence of free surface nanorelief on the rear spallation threshold: Molecular-dynamics investigation. J. Appl. Phys. 2016, 120, 165903. [Google Scholar] [CrossRef]
- Pogorelko, V.V.; Mayer, A.E.; Krasnikov, V.S. High-speed collision of copper nanoparticle with aluminum surface: Molecular dynamics simulation. Appl. Surf. Sci. 2016, 390, 289–302. [Google Scholar] [CrossRef]
- Wu, Y.-C.; Shao, J.-L.; Zhan, H. Damage and self-healing characteristics of monolayer graphene enhanced Cu under ballistic impact. Mech. Mater. 2021, 155, 103736. [Google Scholar] [CrossRef]
- Meng, Z.; Keten, S. Unraveling the Effect of Material Properties and Geometrical Factors on Ballistic Penetration Energy of Nanoscale Thin Films. J. Appl. Mech. 2018, 85. [Google Scholar] [CrossRef]
- Dewapriya, M.A.N.; Miller, R.E. Superior Dynamic Penetration Resistance of Nanoscale Multilayer Polymer/Metal Films. J. Appl. Mech. 2020, 87. [Google Scholar] [CrossRef]
- Lee, J.H.; Loya, P.E.; Lou, J.; Thomas, E.L. Dynamic mechanical behavior of multilayer graphene via supersonic projectile penetration. Science 2014, 346, 1092–1096. [Google Scholar] [CrossRef]
- Dewapriya, M.A.N.; Miller, R.E. Molecular dynamics study of the mechanical behaviour of ultrathin polymer–metal multilayers under extreme dynamic conditions. Comput. Mater. Sci. 2020, 184, 109951. [Google Scholar] [CrossRef]
- Mishin, Y.; Farkas, D.; Mehl, M.J.; Papaconstantopoulos, D.A. Interatomic potentials for monoatomic metals from experimental data andab initiocalculations. Phys. Rev. B 1999, 59, 3393–3407. [Google Scholar] [CrossRef] [Green Version]
- Zhakhovskii, V.V.; Inogamov, N.A.; Petrov, Y.V.; Ashitkov, S.I.; Nishihara, K. Molecular dynamics simulation of femtosecond ablation and spallation with different interatomic potentials. Appl. Surf. Sci. 2009, 255, 9592–9596. [Google Scholar] [CrossRef]
- Jiang, D.-D.; Chen, P.-Y.; Wang, P.; He, A.-M. Spallation Characteristics of Single Crystal Aluminum with Copper Nanoparticles Based on Atomistic Simulations. Nanomaterials 2021, 11, 2603. [Google Scholar] [CrossRef]
- Wu, L.; Zhu, Y.; Wang, H.; Li, M. Crystal–melt coexistence in fcc and bcc metals: A molecular-dynamics study of kinetic coefficients. Model. Simul. Mater. Sci. Eng. 2021, 29. [Google Scholar] [CrossRef]
- Evans, D.J.; Holian, B.L. The Nose–Hoover thermostat. J. Chem. Phys. 1985, 83, 4069–4074. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2010, 18. [Google Scholar] [CrossRef]
- Stukowski, A. Structure identification methods for atomistic simulations of crystalline materials. Model. Simul. Mater. Sci. Eng. 2012, 20. [Google Scholar] [CrossRef]
- Amendola, V.; Meneghetti, M. Self-healing at the nanoscale. Nanoscale 2009, 1, 74–88. [Google Scholar] [CrossRef]
- Liu, X.; Wang, F.; Wu, H.; Wang, W. Strengthening metal nanolaminates under shock compression through dual effect of strong and weak graphene interface. Appl. Phys. Lett. 2014, 104, 231901. [Google Scholar] [CrossRef]
- He, A.-M.; Wang, P.; Shao, J.-L. Molecular dynamics simulations of ejecta size distributions for shock-loaded Cu with a wedged surface groove. Comput. Mater. Sci. 2015, 98, 271–277. [Google Scholar] [CrossRef]
- Durand, O.; Soulard, L. Large-scale molecular dynamics study of jet breakup and ejecta production from shock-loaded copper with a hybrid method. J. Appl. Phys. 2012, 111, 044901. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Y.-C.; Liu, J.-M.; Xie, W.; Yin, Q.; Shao, J.-L. Atomistic Simulations on Metal Rod Penetrating Thin Target at Nanoscale Caused by High-Speed Collision. Nanomaterials 2021, 11, 3160. https://doi.org/10.3390/nano11113160
Wu Y-C, Liu J-M, Xie W, Yin Q, Shao J-L. Atomistic Simulations on Metal Rod Penetrating Thin Target at Nanoscale Caused by High-Speed Collision. Nanomaterials. 2021; 11(11):3160. https://doi.org/10.3390/nano11113160
Chicago/Turabian StyleWu, Yong-Chao, Jin-Ming Liu, Wei Xie, Qing Yin, and Jian-Li Shao. 2021. "Atomistic Simulations on Metal Rod Penetrating Thin Target at Nanoscale Caused by High-Speed Collision" Nanomaterials 11, no. 11: 3160. https://doi.org/10.3390/nano11113160
APA StyleWu, Y.-C., Liu, J.-M., Xie, W., Yin, Q., & Shao, J.-L. (2021). Atomistic Simulations on Metal Rod Penetrating Thin Target at Nanoscale Caused by High-Speed Collision. Nanomaterials, 11(11), 3160. https://doi.org/10.3390/nano11113160





