Double Narrow Fano Resonances via Diffraction Coupling of Magnetic Plasmon Resonances in Embedded 3D Metamaterials for High-Quality Sensing
Abstract
:1. Introduction
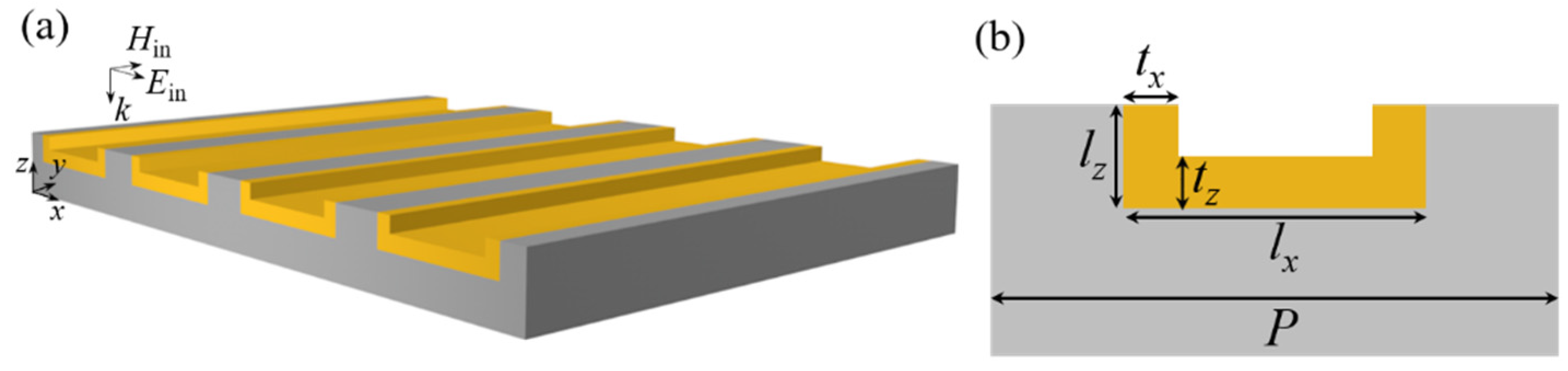
2. Materials and Methods
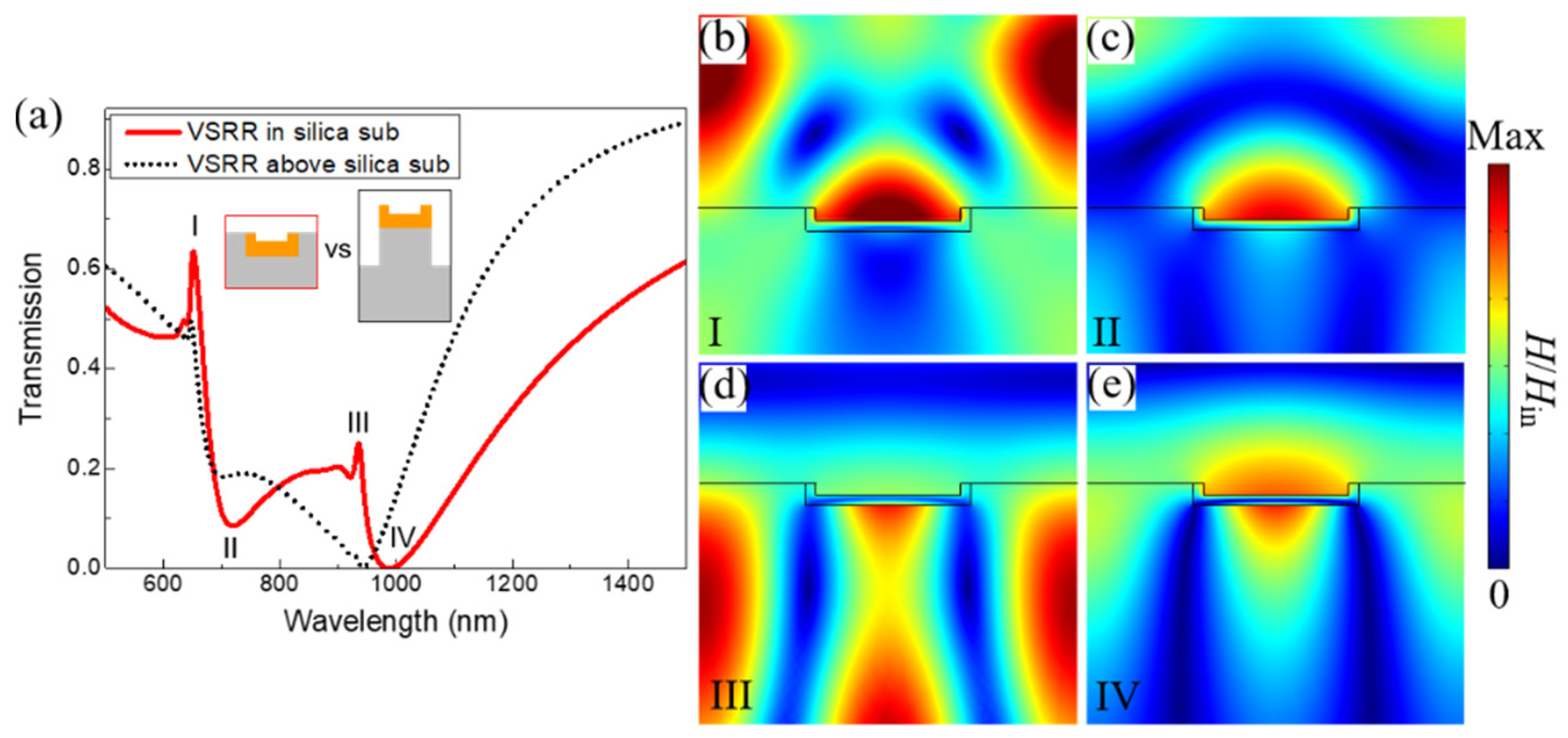
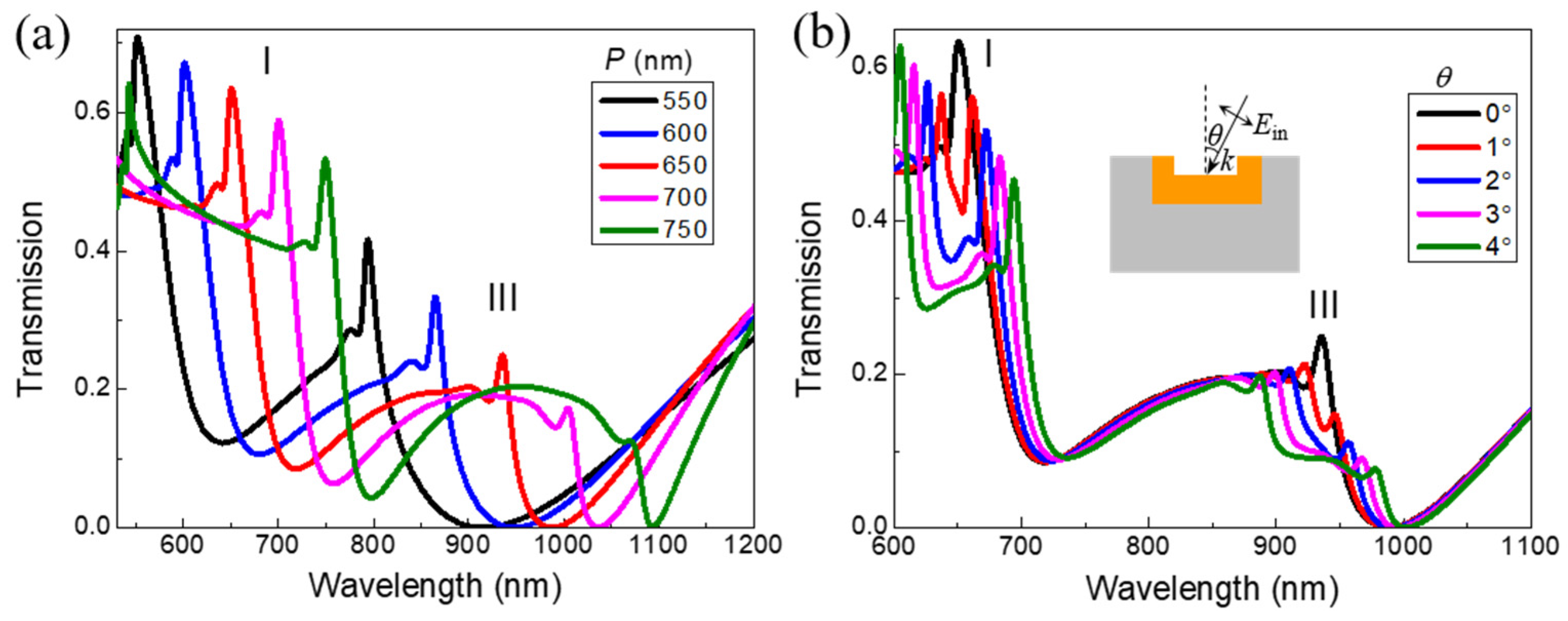
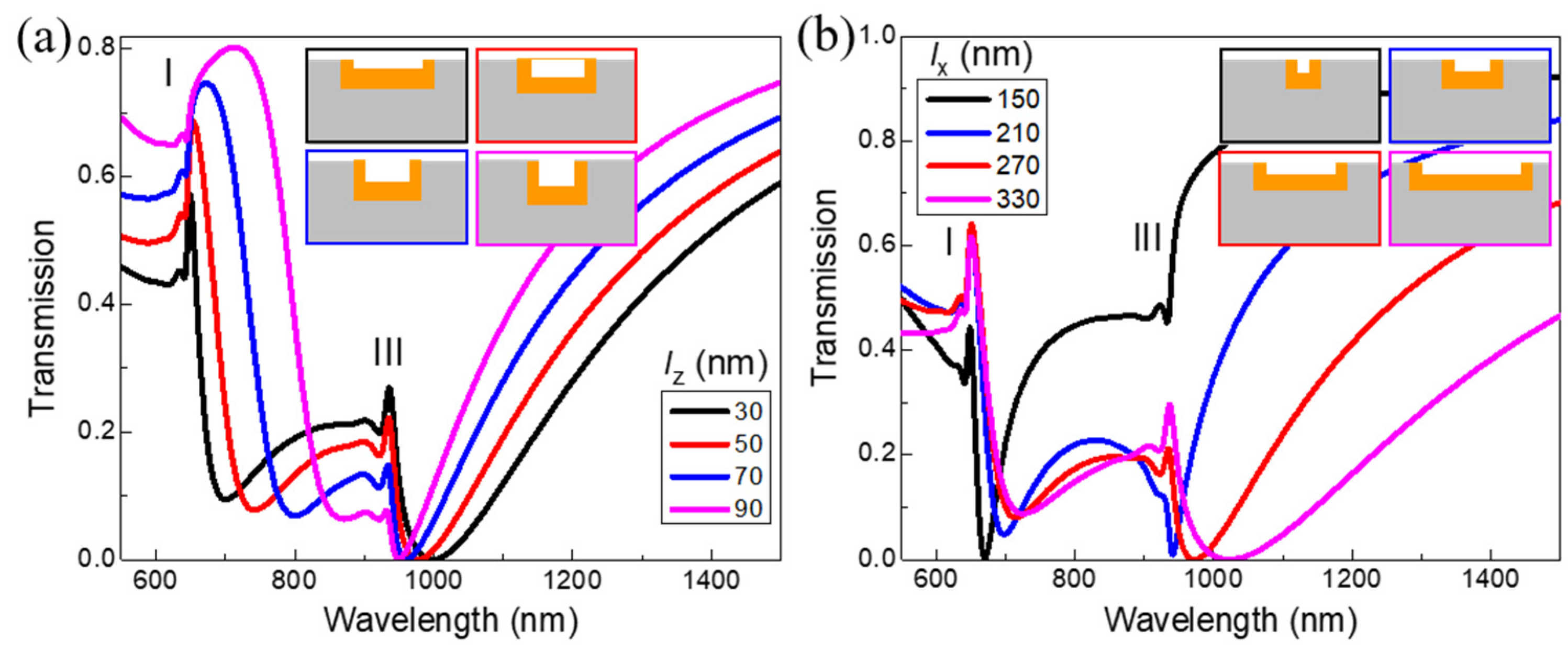
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer: New York, NY, USA, 2007. [Google Scholar]
- Halas, N.J.; Lal, S.; Chang, W.S.; Link, S.; Nordlander, P. Plasmons in Strongly Coupled Metallic Nanostructures. Chem. Rev. 2011, 111, 3913–3961. [Google Scholar] [CrossRef]
- Grigorenko, A.N.; Polini, M.; Novoselov, K.S. Graphene plasmonics. Nat. Photonics 2012, 6, 749–758. [Google Scholar] [CrossRef]
- Jahani, S.; Jacob, Z. All-dielectric metamaterials. Nat. Nanotechnol. 2016, 11, 23–36. [Google Scholar] [CrossRef] [PubMed]
- Lin, Z.; He, L. Recent advance in SERS techniques for food safety and quality analysis: A brief review. Curr. Opin. Food Sci. 2019, 28, 82. [Google Scholar] [CrossRef]
- Azmin, S.N.H.M.; Hayat, N.A.B.M.; Nor, M.S.M. Development and Characterization of Food Packaging Bioplastic Film from Cocoa Pod Husk Cellulose Incorporated with Sugarcane Bagasse Fibre. J. Bioresour. Bioprod. 2020, 5, 248–255. [Google Scholar] [CrossRef]
- Joseph, B.; Sagarika, V.K.; Lamstein, J.; Liang, Y.; Bezryadina, A.; Liang, G.; Hansson, T.; Wetzel, B.; Preece, D.; White, A.; et al. Cellulose Nanocomposites: Fabrication and Biomedical Applications. J. Bioresour. Bioprod. 2020, 5, 223–237. [Google Scholar] [CrossRef]
- Fatima, A.; Yasir, S.; Khan, M.S.; Manan, S.; Ullah, M.W.; Ul-Islam, M. Plant extract-loaded bacterial cellulose composite membrane for potential biomedical applications. J. Bioresour. Bioprod. 2021, 6, 26–32. [Google Scholar] [CrossRef]
- Izquierdo-Lorenzo, I.; Sanchez-Cortes, S.; Garcia-Ramos, J.V. Adsorption of Beta-Adrenergic Agonists Used in Sport Doping on Metal Nanoparticles: A Detection Study Based on Surface-Enhanced Raman Scattering. Langmuir 2010, 26, 14663–14670. [Google Scholar] [CrossRef]
- Lee, H.J.; Yook, J.G. Biosensing using split-ring resonators at microwave regime. Appl. Phys. Lett. 2008, 92, 25. [Google Scholar] [CrossRef]
- Bahadori, M.; Eshaghian, A.; Mehrany, K. A Circuit Model for Analysis of Metal-Insulator-Metal Plasmonic Complementary Split-Ring Resonators. J. Lightw. Technol. 2014, 32, 2659–2665. [Google Scholar] [CrossRef]
- Ma, F.S.; Lin, Y.S.; Zhang, X.H.; Lee, C. Tunable multiband terahertz metamaterials using a reconfigurable electric split-ring resonator array. Light Sci. Appl. 2014, 3, e171. [Google Scholar] [CrossRef] [Green Version]
- Klein, M.W.; Enkrich, C.; Wegener, M.; Linden, S. Second-harmonic generation from magnetic metamaterials. Science 2006, 313, 502–504. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ciraci, C.; Poutrina, E.; Scalora, M.; Smith, D.R. Origin of secondharmonic generation enhancement in optical split-ring resonators. Phys. Rev. B 2012, 85, 201403. [Google Scholar] [CrossRef] [Green Version]
- Shadrivov, I.V.; Kozyrev, A.B.; Van der Weide, D.W.; Kivshar, Y.S. Nonlinear magnetic metamaterials. Opt. Express 2008, 16, 20266–20271. [Google Scholar] [CrossRef] [Green Version]
- Clark, A.W.; Glidle, A.; Cumming, D.R.S.; Cooper, J.M. Plasmonic split-ring resonators as dichroic nanophotonic DNA biosensors. J. Amer. Chem. Soc. 2009, 131, 7615–17619. [Google Scholar] [CrossRef] [PubMed]
- Pol, L.; Eckstein, C.; Acosta, L.K.; Xifré-Pérez, E.; Ferré-Borrull, J.; Marsal, L.F. Real-Time Monitoring of Biotinylated Molecules Detection Dynamics in Nanoporous Anodic Alumina for Bio-Sensing. Nanomaterials 2019, 9, 478. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tobing, L.Y.M.; Tjahjana, L.; Zhang, D.H.; Zhang, Q.; Xiong, Q.H. Deep subwavelength fourfold rotationally symmetric split-ring-resonator metamaterials for highly sensitive and robust biosensing platform. Sci. Rep. 2013, 3, 2437. [Google Scholar] [CrossRef] [Green Version]
- Yang, J.; Lin, Y.S. Design of Tunable Terahertz Metamaterial Sensor with Single- and Dual-Resonance Characteristic. Nanomaterials 2021, 11, 2212. [Google Scholar] [CrossRef]
- Zhong, J.T.; Xu, X.C.; Lin, Y.S. Tunable Terahertz Metamaterial with Electromagnetically Induced Transparency Characteristic for Sensing Application. Nanomaterials 2021, 11, 2175. [Google Scholar] [CrossRef]
- Wu, P.C.; Sun, G.; Chen, W.T.; Yang, K.Y.; Huang, Y.W.; Chen, Y.H.; Huang, H.L.; Hsu, W.L.; Chiang, H.P.; Tsai, D.P. Vertical split-ring resonator based nanoplasmonic sensor. Appl. Phys. Lett. 2014, 105, 033105. [Google Scholar] [CrossRef]
- Dmitriev, A.; Hagglund, C.; Chen, S.; Fredriksson, H.; Pakizeh, T.; Kall, M.; Sutherland, D.S. Enhanced Nanoplasmonic Optical Sensors with Reduced Substrate Effect. Nano Lett. 2008, 8, 3893–3898. [Google Scholar] [CrossRef] [PubMed]
- Wu, P.C.; Hsu, W.L.; Chen, W.T.; Huang, Y.W.; Liao, C.Y.; Liu, A.Q.; Zheludev, N.I.; Sun, G.; Tsai, D.P. Plasmon coupling in vertical split-ring resonator metamolecules. Sci. Rep. 2015, 5, 9726. [Google Scholar] [CrossRef] [PubMed]
- Wu, P.C.; Liao, C.Y.; Chen, J.W.; Tsai, D.P. Isotropic Absorption and Sensor of Vertical Split-Ring Resonator. Adv. Opt. Mater. 2017, 5, 1600581. [Google Scholar] [CrossRef]
- Chen, W.T.; Chen, C.J.; Wu, P.C.; Sun, S.L.; Zhou, L.; Guo, G.Y.; Hsiao, C.T.; Yang, K.Y.; Zheludev, N.I.; Tsai, D.P. Optical magnetic response in three-dimensional metamaterial of upright plasmonic meta-molecules. Opt. Express 2011, 19, 12837–12842. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, P.C.; Chen, W.T.; Yang, K.Y.; Hsiao, C.T.; Sun, G.; Liu, A.Q.; Zheludev, N.I.; Tsai, D.P. Magnetic plasmon induced transparency in three-dimensional metamolecules. Nanophotonics 2012, 1, 131–138. [Google Scholar] [CrossRef]
- Chen, J.; Qi, S.; Hong, X.; Gu, P.; Wei, R.; Tang, C.; Huang, Y.; Zhao, C. Highly sensitive 3D metamaterial sensor based on diffraction coupling of magnetic plasmon resonances. Results Phys. 2019, 15, 102791. [Google Scholar] [CrossRef]
- Chen, J.; Nie, H.; Peng, C.; Qi, S.; Tang, C.; Zhang, Y.; Wang, L. Enhancing the Magnetic Plasmon Resonance of Three-Dimensional Optical Metamaterials via Strong Coupling for High-Sensitivity Sensing. J. Lightw. Technol. 2018, 36, 3481–3485. [Google Scholar] [CrossRef]
- Yan, Z.D.; Zhang, Z.X.; Du, W.; Wu, W.J.; Hu, T.P.; Yu, Z.; Gu, P.; Chen, J.; Tang, C.J. Graphene Multiple Fano Resonances Based on Asymmetric Hybrid Metamaterial. Nanomaterials 2020, 10, 2408. [Google Scholar] [CrossRef]
- Yan, Z.D.; Lu, X.; Chen, K.; Lv, Z.Q.; Pu, X.T.; Tang, C.J.; Cai, P.G. Ultranarrow Dual-Band Perfect Absorption in Visible and Near-infrared Regimes Based on Three-Dimensional Metamaterials for Ultrahigh-Sensitivity Sensing. J. Lightw. Technol. 2021, 39, 7217–7222. [Google Scholar] [CrossRef]
- Gu, P.; Cai, X.F.; Wu, G.H.; Xue, C.P.; Chen, J.; Zhang, Z.X.; Yan, Z.D.; Liu, F.X.; Tang, C.J.; Du, W.; et al. Ultranarrow and Tunable Fano Resonance in Ag Nanoshells and a Simple Ag Nanomatryushka. Nanomaterials 2021, 11, 2039. [Google Scholar] [CrossRef]
- Yan, Z.D.; Lu, X.; Du, W.; Lv, Z.Q.; Tang, C.J.; Cai, P.G.; Gu, P.; Chen, J.; Yu, Z. Ultraviolet graphene ultranarrow absorption engineered by lattice plasmon resonance. Nanotechnology 2021, 32, 465202. [Google Scholar] [CrossRef] [PubMed]
- Yan, Z.D.; Zhu, Q.; Wan, M.J.; Lu, X.; Pu, X.T.; Tang, C.J.; Yu, L.L. Graphene ultraviolet ultrahigh-Q perfect absorption for nanoscale optical sensing. Opt. Express 2020, 28, 6095–6101. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Fan, W.F.; Zhang, T.; Tang, C.J.; Chen, X.Y.; Wu, J.J.; Li, D.Y.; Yu, Y. Engineering the magnetic plasmon resonances of metamaterials for high-quality sensing. Opt. Express 2020, 25, 3675–3681. [Google Scholar] [CrossRef] [PubMed]
- Kravets, V.G.; Kabashin, A.V.; Barnes, W.L.; Grigorenko, A.N. Plasmonic Surface Lattice Resonances: A Review of Properties and Applications. Chem. Rev. 2018, 118, 5912. [Google Scholar] [CrossRef]
- Wang, J.Q.; Fan, C.Z.; He, J.N.; Ding, P.; Liang, E.J.; Xue, Q.Z. Double Fano resonances due to interplay of electric and magnetic plasmon modes in planar plasmonic structure with high sensing sensitivity. Opt. Express 2013, 21, 2236–2244. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Wen, X.L.; Li, G.Y.; Ruan, Q.F.; Wang, J.F.; Xiong, Q.H. Multiple Magnetic Mode-Based Fano Resonance in Split-Ring Resonator/Disk Nanocavities. ACS Nano 2013, 7, 11071–11078. [Google Scholar] [CrossRef] [PubMed]
- Lopez-Ortega, A.; Zapata-Herrera, M.; Maccaferri, N.; Pancaldi, M.; Garcia, M.; Chuvilin, A.; Vavassori, P. Enhanced magnetic modulation of light polarization exploiting hybridization with multipolar dark plasmons in magnetoplasmonic nanocavities. Light Sci. Appl. 2020, 9, 49. [Google Scholar] [CrossRef] [Green Version]
- Luk’yanchuk, B.; Zheludev, N.I.; Maier, S.A.; Halas, N.J.; Nordlander, P.; Giessen, H.; Chong, C.T. The Fano resonance in plasmonic nanostructures and metamaterials. Nat. Mater. 2010, 9, 707–715. [Google Scholar] [CrossRef] [PubMed]
- He, Z.H.; Xue, W.W.; Cui, W.; Li, C.J.; Li, Z.X.; Pu, L.H.; Feng, J.J.; Xiao, X.T.; Wang, X.Y.; Li, G. Tunable Fano Resonance and Enhanced Sensing in a Simple Au/TiO2 Hybrid Metasurface. Nanomaterials 2020, 10, 687. [Google Scholar] [CrossRef] [Green Version]
- Adato, R.; Yanik, A.A.; Wu, C.H.; Shvets, G.; Altug, H. Radiative engineering of plasmon lifetimes in embedded nanoantenna arrays. Opt. Express 2010, 18, 4526–4537. [Google Scholar] [CrossRef] [Green Version]
- Liu, N.; Liu, H.; Zhu, S.; Giessen, H. Stereometamaterials. Nat. Photonics 2009, 3, 157–162. [Google Scholar] [CrossRef]
- Yan, Z.D.; Chen, X.; Du, W.; Chen, Z.; Zhan, P.; Wang, H.T.; Wang, Z.L. Near-field plasmonic coupling for enhanced nonlinear absorption by femtosecond pulses in bowtie nanoantenna arrays. Appl. Phys. A 2014, 117, 1841–1848. [Google Scholar] [CrossRef]
- Yan, Z.D.; Du, W.; Tu, L.L.; Gu, P.; Huang, Z.; Zhan, P.; Liu, F.X.; Wang, Z.L. A facile high-performance SERS substrate based on broadband near-perfect optical absorption. J. Raman Spectrosc. 2015, 46, 795–801. [Google Scholar] [CrossRef]
- Auguié, B.; Barnes, B.L. Collective resonances in gold nanoparticle arrays. Phys. Rev. Lett. 2008, 101, 143902. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mayer, K.M.; Hafner, J.H. Localized surface plasmon resonance sensors. Chem. Rev. 2011, 111, 3828–3857. [Google Scholar] [CrossRef] [PubMed]
- Chao, C.T.C.; Chau, Y.F.C.; Chen, S.H.; Huang, J.H.; Lim, C.M.; Kooh, M.R.R.; Thotagamuge, R.; Chiang, H.P. Ultrahigh Sensitivity of a Plasmonic Pressure Sensor with a Compact Size. Nanomaterials 2021, 11, 3147. [Google Scholar] [CrossRef] [PubMed]
- Runowski, M.; Sobczak, S.; Marciniakn, J.; Bukalska, I.; Lis, S.; Katrusiak, A. Gold nanorods as a high-pressure sensor of phase transitions and refractive-index gauge. Nanoscale 2019, 11, 8718–8726. [Google Scholar] [CrossRef] [PubMed]
- Martin-Sanchez, C.; Sanchez-Iglesias, A.; Mulvaney, P.; Liz-Marzan, L.M.; Rodriguez, F. Plasmonic Sensing of Refractive Index and Density in Methanol-Ethanol Mixtures at High Pressure. J. Phys. Chem. C 2020, 124, 8978–8983. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, H.; Lu, X.; Huang, J.; Chen, K.; Su, J.; Yan, Z.; Tang, C.; Cai, P. Double Narrow Fano Resonances via Diffraction Coupling of Magnetic Plasmon Resonances in Embedded 3D Metamaterials for High-Quality Sensing. Nanomaterials 2021, 11, 3361. https://doi.org/10.3390/nano11123361
Hu H, Lu X, Huang J, Chen K, Su J, Yan Z, Tang C, Cai P. Double Narrow Fano Resonances via Diffraction Coupling of Magnetic Plasmon Resonances in Embedded 3D Metamaterials for High-Quality Sensing. Nanomaterials. 2021; 11(12):3361. https://doi.org/10.3390/nano11123361
Chicago/Turabian StyleHu, Haitao, Xue Lu, Jianhua Huang, Kai Chen, Jun Su, Zhendong Yan, Chaojun Tang, and Pingen Cai. 2021. "Double Narrow Fano Resonances via Diffraction Coupling of Magnetic Plasmon Resonances in Embedded 3D Metamaterials for High-Quality Sensing" Nanomaterials 11, no. 12: 3361. https://doi.org/10.3390/nano11123361
APA StyleHu, H., Lu, X., Huang, J., Chen, K., Su, J., Yan, Z., Tang, C., & Cai, P. (2021). Double Narrow Fano Resonances via Diffraction Coupling of Magnetic Plasmon Resonances in Embedded 3D Metamaterials for High-Quality Sensing. Nanomaterials, 11(12), 3361. https://doi.org/10.3390/nano11123361




