The Role of Hydrogen on the Behavior of Intergranular Cracks in Bicrystalline α-Fe Nanowires
Abstract
1. Introduction
2. Simulation Methodology
3. Results
3.1. Theoretical Model for Embrittlement
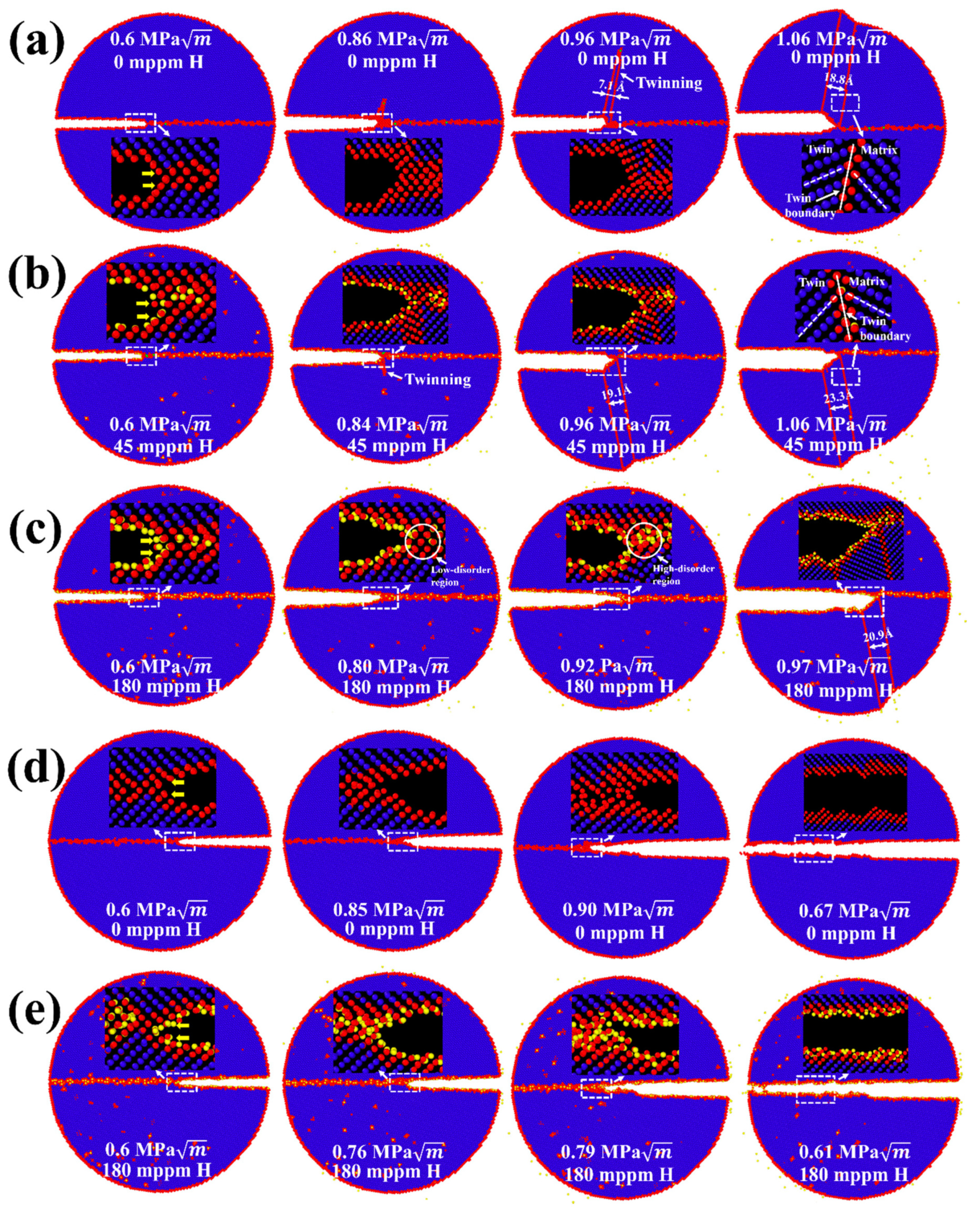
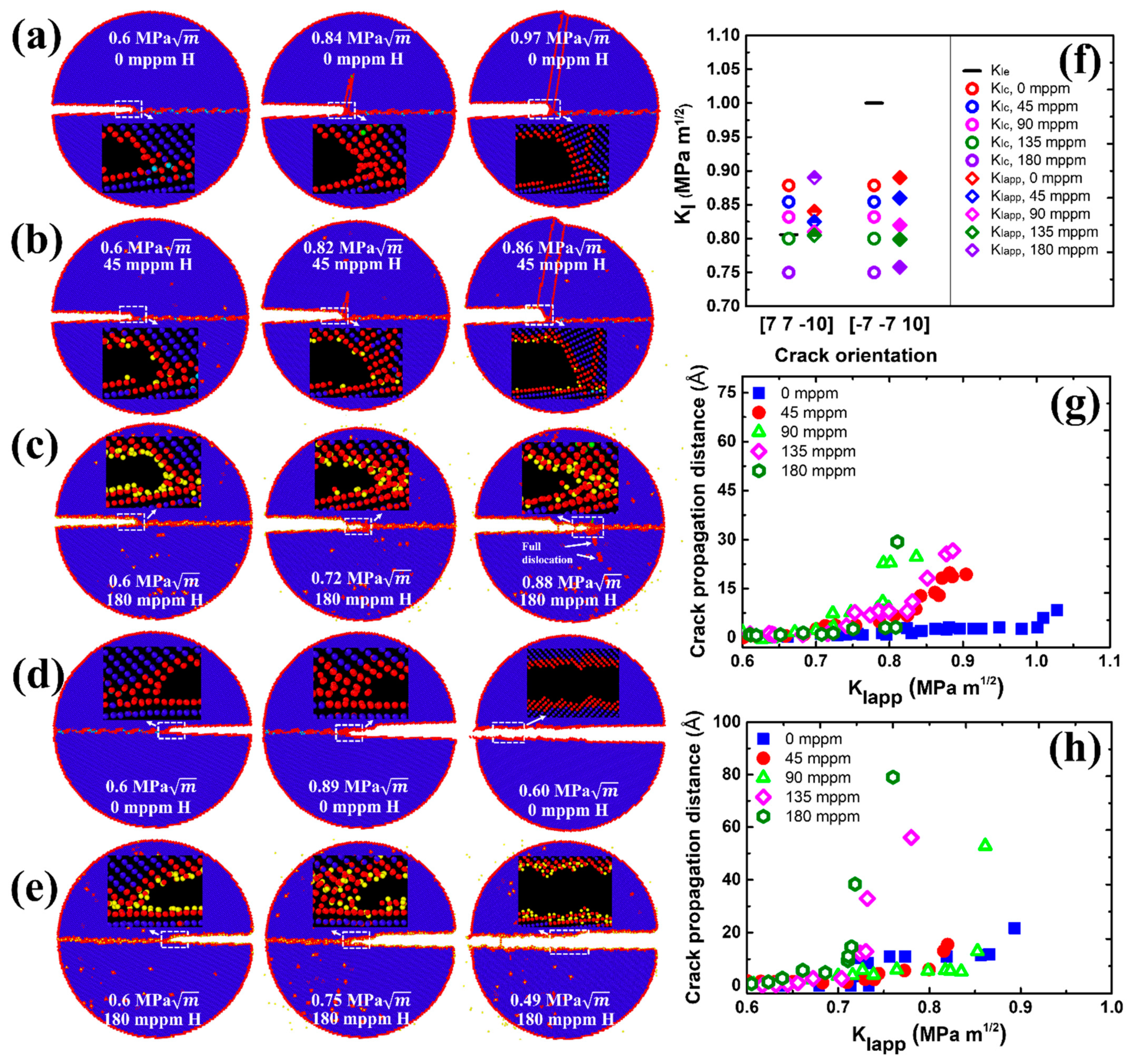
3.2. Dislocation Emission and Cleavage of Crack Tip under Monotonic Loading
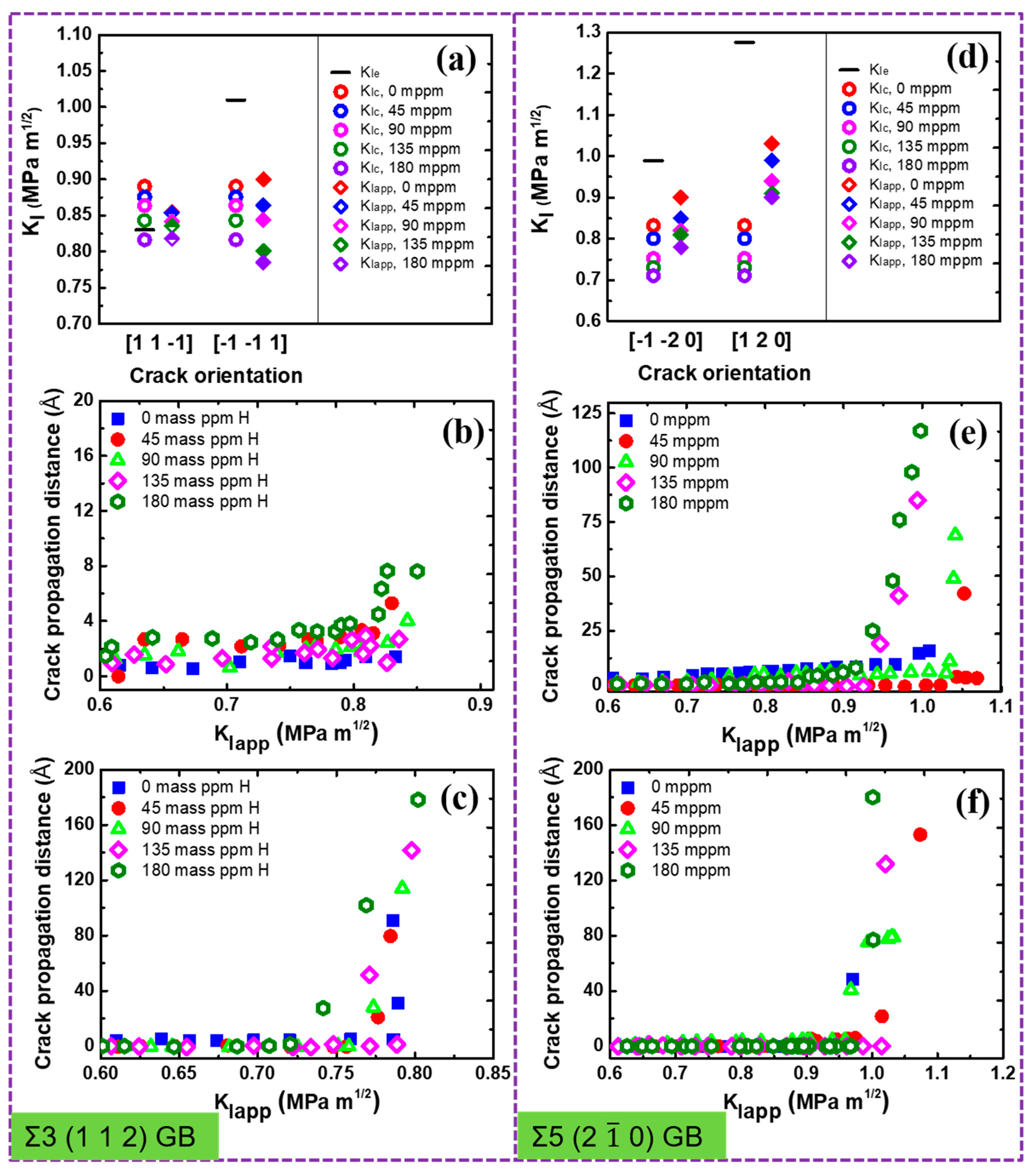
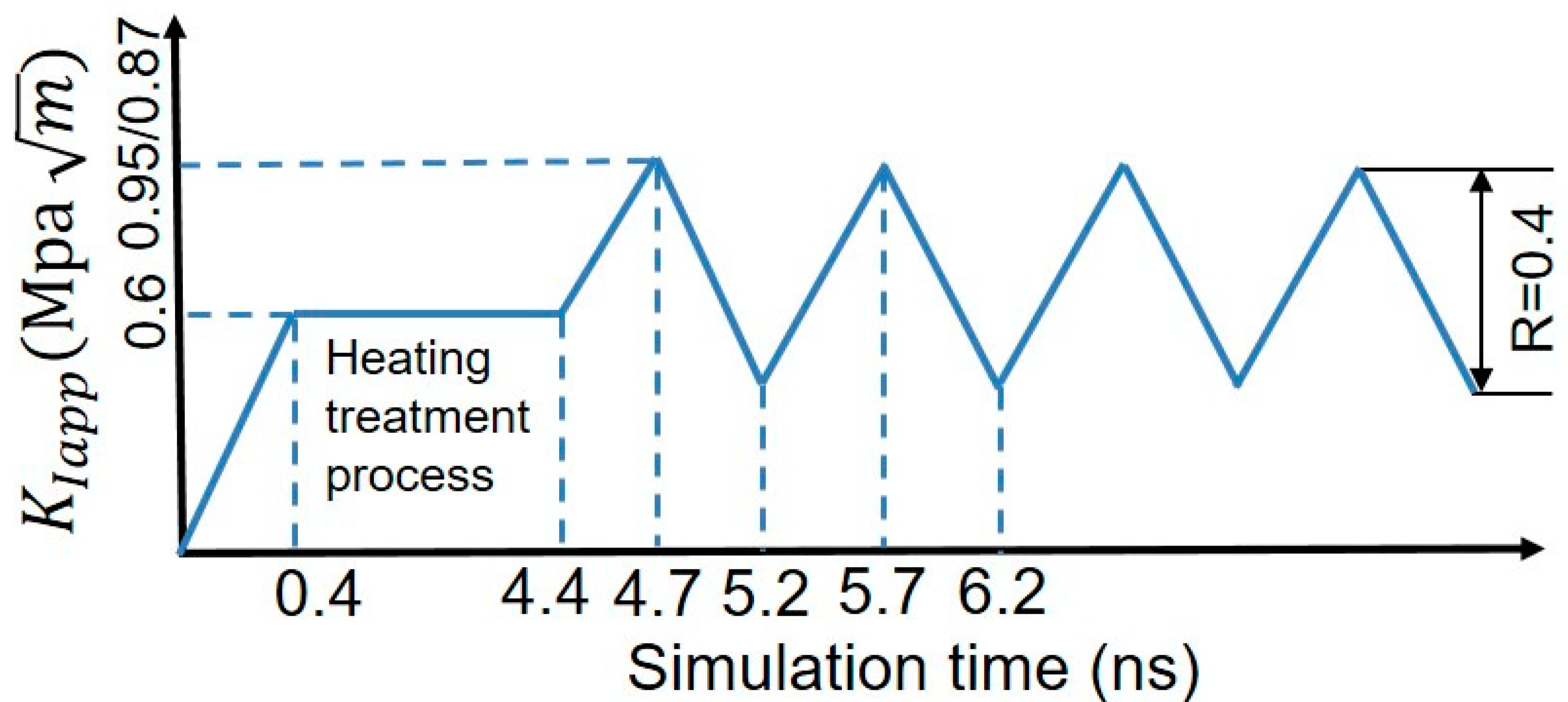
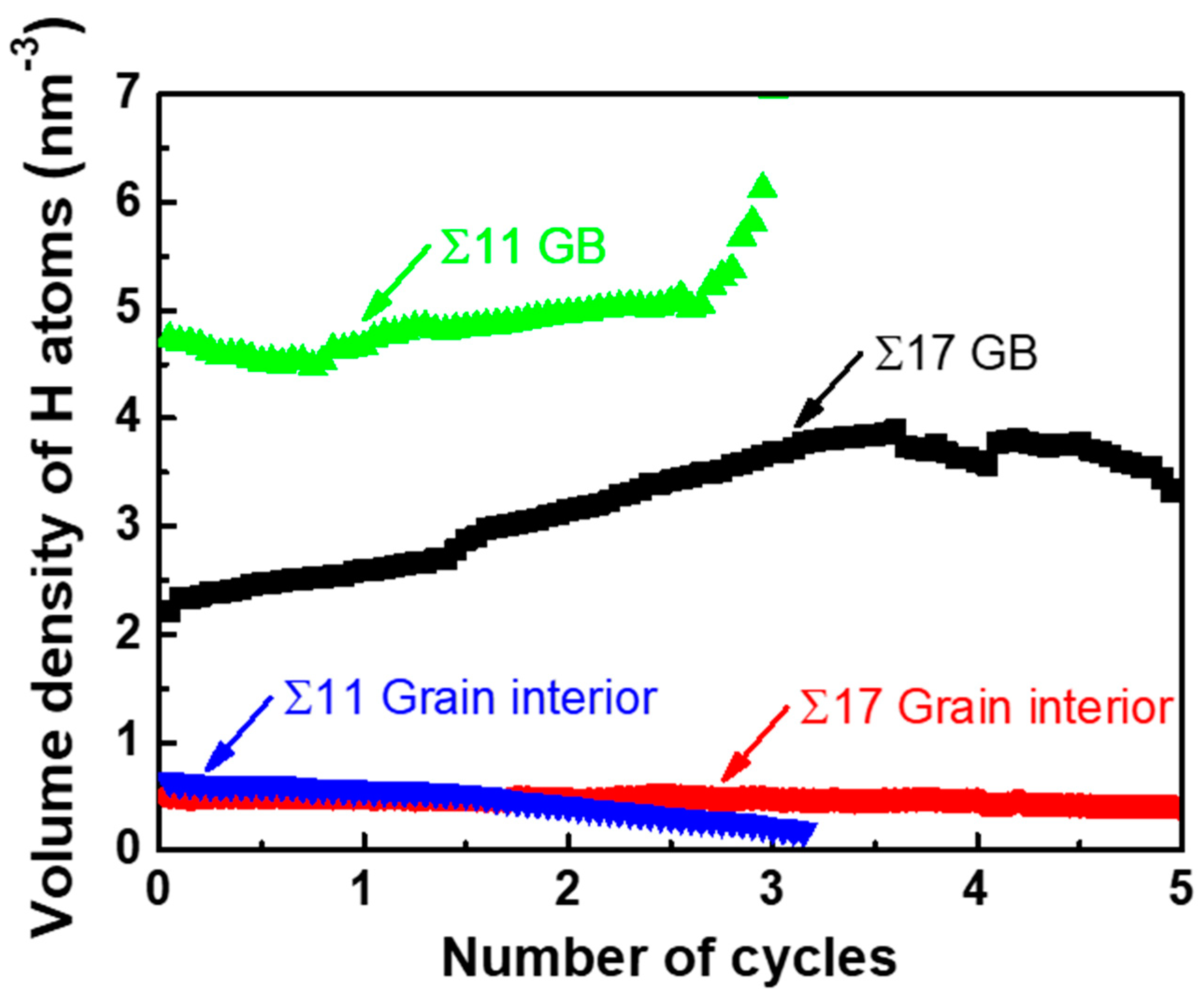
3.3. Dislocation Emission and Cleavage of Crack Tip under Cyclic Loading
4. Discussion and Summary
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Johnson, W.H.; Thomson, W., II. On some remarkable changes produced in iron and steel by the action of hydrogen and acids. Proc. R. Soc. Lond. 1875, 23, 168–179. [Google Scholar] [CrossRef]
- Barthélémy, H. Hydrogen storage—Industrial prospectives. Int. J. Hydrog. Energy 2012, 37, 17364–17372. [Google Scholar] [CrossRef]
- Li, X.; Chen, L.; Liu, H.; Shi, C.; Wang, D.; Mi, Z.; Qiao, L. Prevention of hydrogen damage using MoS₂ coating on iron surface. Nanomaterials 2019, 9, 382. [Google Scholar] [CrossRef] [PubMed]
- Barrera, O.; Bombač, D.; Chen, Y.; Daff, T.D.; Galindo-Nava, E.; Gong, P.; Haley, D.; Horton, R.; Katzarov, I.; Kermode, J.R.; et al. Understanding and mitigating hydrogen embrittlement of steels: A review of experimental, modelling and design progress from atomistic to continuum. J. Mater. Sci. 2018, 53, 6251–6290. [Google Scholar] [CrossRef] [PubMed]
- Olden, V.; Alvaro, A.; Akselsen, O.M. Hydrogen diffusion and hydrogen influenced critical stress intensity in an API X70 pipeline steel welded joint—Experiments and FE simulations. Int. J. Hydrog. Energy 2012, 37, 11474–11486. [Google Scholar] [CrossRef]
- Gangloff, R.P.; Wei, R.P. Gaseous hydrogen embrittlement of high strength steels. Met. Mater. Trans. A 1977, 8, 1043–1053. [Google Scholar] [CrossRef]
- Barthélémy, H. Effects of pressure and purity on the hydrogen embrittlement of steels. Int. J. Hydrog. Energy 2011, 36, 2750–2758. [Google Scholar] [CrossRef]
- Chen, Y.-S.; Lu, H.; Liang, J.; Rosenthal, A.; Liu, H.; Sneddon, G.; McCarroll, I.; Zhao, Z.; Li, W.; Guo, A.; et al. Observation of hydrogen trapping at dislocations, grain boundaries, and precipitates. Science 2020, 367, 171–175. [Google Scholar]
- Troiano, A.R. The role of hydrogen and other interstitials in the mechanical behavior of metals. Trans. ASM 1960, 52, 54–80. [Google Scholar] [CrossRef]
- Oriani, R.; Josephic, P. Equilibrium aspects of hydrogen-induced cracking of steels. Acta Met. 1974, 22, 1065–1074. [Google Scholar] [CrossRef]
- Lassila, D.; Birnbaum, H. The effect of diffusive hydrogen segregation on fracture of polycrystalline nickel. Acta Met. 1986, 34, 1237–1243. [Google Scholar] [CrossRef]
- Li, J.; Lu, C.; Pei, L.; Zhang, C.; Wang, R. Atomistic investigation of hydrogen induced decohesion of Ni grain boundaries. Mech. Mater. 2020, 150, 103586. [Google Scholar] [CrossRef]
- Beachem, C.D. A new model for hydrogen-assisted cracking (hydrogen “embrittlement”). Met. Mater. Trans. B 1972, 3, 441–455. [Google Scholar] [CrossRef]
- Birnbaum, H.; Sofronis, P. Hydrogen-enhanced localized plasticity—A mechanism for hydrogen-related fracture. Mater. Sci. Eng. A 1994, 176, 191–202. [Google Scholar] [CrossRef]
- Robertson, I. The effect of hydrogen on dislocation dynamics. Eng. Fract. Mech. 2001, 68, 671–692. [Google Scholar] [CrossRef]
- Nagumo, M.; Takai, K. The predominant role of strain-induced vacancies in hydrogen embrittlement of steels: Overview. Acta Mater. 2019, 165, 722–733. [Google Scholar] [CrossRef]
- Nagumo, M. Hydrogen related failure of steels—A new aspect. Mater. Sci. Technol. 2004, 20, 940–950. [Google Scholar] [CrossRef]
- Lynch, S. Hydrogen embrittlement phenomena and mechanisms. Corros. Rev. 2012, 30, 105–123. [Google Scholar] [CrossRef]
- Lynch, S. Interpreting hydrogen-induced fracture surfaces in terms of deformation processes: A new approach. Scr. Mater. 2011, 65, 851–854. [Google Scholar] [CrossRef]
- Kirchheim, R. Reducing grain boundary, dislocation line and vacancy formation energies by solute segregation. I. Theoretical background. Acta Mater. 2007, 55, 5129–5138. [Google Scholar] [CrossRef]
- Kirchheim, R. Revisiting hydrogen embrittlement models and hydrogen-induced homogeneous nucleation of dislocations. Scr. Mater. 2010, 62, 67–70. [Google Scholar] [CrossRef]
- Martin, M.L.; Fenske, J.A.; Liu, G.S.; Sofronis, P.; Robertson, I.M. On the formation and nature of quasi-cleavage fracture surfaces in hydrogen embrittled steels. Acta Mater. 2011, 59, 1601–1606. [Google Scholar] [CrossRef]
- Taketomim, S.; Matsumoto, R.; Miyazaki, N. Atomistic simulation of the effects of hydrogen on the mobility of edge dislocation in alpha iron. J. Mater. Sci. 2008, 43, 1166–1169. [Google Scholar] [CrossRef]
- Tehranchi, A.; Curtin, W.A. The role of atomistic simulations in probing hydrogen effects on plasticity and embrittlement in metals. Eng. Fract. Mech. 2019, 216, 106502. [Google Scholar] [CrossRef]
- Barnoush, A.; Vehoff, H. Recent developments in the study of hydrogen embrittlement: Hydrogen effect on dislocation nucleation. Acta Mater. 2010, 58, 5274–5285. [Google Scholar] [CrossRef]
- Djukic, M.; Bakic, G.M.; Zeravcic, V.S.; Sedmak, A.; Rajicic, B. The synergistic action and interplay of hydrogen embrittlement mechanisms in steels and iron: Localized plasticity and decohesion. Eng. Fract. Mech. 2019, 216, 106528. [Google Scholar] [CrossRef]
- Robertson, I.M.; Sofronis, P.; Nagao, A.; Martin, M.L.; Wang, S.; Gross, D.W.; Nygren, K.E. Hydrogen embrittlement understood. Met. Mater. Trans. B 2015, 46, 1085–1103. [Google Scholar] [CrossRef]
- Song, J.; Curtin, W.A. Atomic mechanism and prediction of hydrogen embrittlement in iron. Nat. Mater. 2012, 12, 145–151. [Google Scholar] [CrossRef]
- Song, J.; Curtin, W. A nanoscale mechanism of hydrogen embrittlement in metals. Acta Mater. 2011, 59, 1557–1569. [Google Scholar] [CrossRef]
- Xie, D.; Li, S.; Li, M.; Wang, Z.; Gumbsch, P.; Sun, J.; Ma, E.; Li, J.; Shan, Z. Hydrogenated vacancies lock dislocations in aluminium. Nat. Commun. 2016, 7, 13341. [Google Scholar] [CrossRef]
- Pei, L.; Lu, C.; Zhao, X.; Zhang, L.; Cheng, K.; Michal, G.; Tieu, K. Brittle versus ductile behavior of nanotwinned copper: A molecular dynamics study. Acta Mater. 2015, 89, 1–13. [Google Scholar] [CrossRef]
- Rice, J.R. Dislocation nucleation from a crack tip: An analysis based on the Peierls concept. J. Mech. Phys. Solids 1992, 40, 239–271. [Google Scholar] [CrossRef]
- Adlakha, I.; Solanki, K. Role of hydrogen on the incipient crack tip deformation behavior in α-Fe: An atomistic perspective. J. Appl. Phys. 2018, 123, 014304. [Google Scholar] [CrossRef]
- Lee, G.H.; Shim, J.S.; Cui, C.Y.; Beom, H.G. Hydrogen-induced cracking of an aluminum single crystal: An atomistic simulation. Comput. Mater. Sci. 2019, 169, 109084. [Google Scholar] [CrossRef]
- Wen, M.; Li, Z.; Barnoush, A. Atomistic study of hydrogen effect on dislocation nucleation at crack tip. Adv. Eng. Mater. 2013, 15, 1146–1151. [Google Scholar] [CrossRef]
- Matsumoto, R.; Taketomi, S.; Matsumoto, S.; Miyazaki, N. Atomistic simulations of hydrogen embrittlement. Int. J. Hydrog. Energy 2009, 34, 9576–9584. [Google Scholar] [CrossRef]
- Solanki, K.; Ward, D.K.; Bammann, D.J. A nanoscale study of dislocation nucleation at the crack tip in the nickel-hydrogen system. Met. Mater. Trans. A 2011, 42, 340–347. [Google Scholar] [CrossRef]
- Song, H.; Zhang, L.; Xiao, M. Molecular dynamics simulation of effect of hydrogen atoms on crack propagation behavior of α -Fe. Phys. Lett. A 2016, 380, 4049–4056. [Google Scholar] [CrossRef]
- Sun, Y.; Peng, Q.; Lu, G. Quantum mechanical modeling of hydrogen assisted cracking in aluminum. Phys. Rev. B 2013, 88, 104109. [Google Scholar] [CrossRef]
- Zhou, X.-Y.; Yang, X.-S.; Zhu, J.-H.; Xing, F. Atomistic simulation study of the grain-size effect on hydrogen embrittlement of nanograined Fe. Int. J. Hydrog. Energy 2020, 45, 3294–3306. [Google Scholar] [CrossRef]
- Fan, Y.; Cui, F.; Lu, L.; Zhang, B. A nanotwinned austenite stainless steel with high hydrogen embrittlement resistance. J. Alloy. Compd. 2019, 788, 1066–1075. [Google Scholar] [CrossRef]
- Koyama, M.; Akiyama, E.; Tsuzaki, K.; Raabe, D. Hydrogen-assisted failure in a twinning-induced plasticity steel studied under in situ hydrogen charging by electron channeling contrast imaging. Acta Mater. 2013, 61, 4607–4618. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Cherepanov, G.P.; Balankin, A.S.; Ivanova, V.S. Fractal fracture mechanics: A review. Eng. Fract. Mech. 1995, 51, 997–1033. [Google Scholar] [CrossRef]
- Metropolis, N.; Rosenbluth, A.W.; Rosenbluth, M.N.; Teller, A.H.; Teller, E. Equation of state calculations by fast computing machines. J. Chem. Phys. 1953, 21, 1087–1092. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A 1985, 31, 1695–1697. [Google Scholar] [CrossRef] [PubMed]
- Nosé, S. A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 1984, 81, 511–519. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2009, 18, 015012. [Google Scholar] [CrossRef]
- Wu, Z.; Curtin, W. Brittle and ductile crack-tip behavior in magnesium. Acta Mater. 2015, 88, 1–12. [Google Scholar] [CrossRef]
- Xing, X.; Yu, M.; Chen, W.; Zhang, H. Atomistic simulation of hydrogen-assisted ductile-to-brittle transition in α-iron. Comput. Mater. Sci. 2017, 127, 211–221. [Google Scholar] [CrossRef]
- Li, J.; Lu, C.; Pei, L.; Zhang, C.; Wang, R.; Tieu, K. Influence of hydrogen environment on dislocation nucleation and fracture response of 〈1 1 0〉 grain boundaries in nickel. Comput. Mater. Sci. 2019, 165, 40–50. [Google Scholar] [CrossRef]
- Song, J.; Soare, M.; Curtin, W.A. Testing continuum concepts for hydrogen embrittlement in metals using atomistics. Model. Simul. Mater. Sci. Eng. 2010, 18, 045003. [Google Scholar] [CrossRef]
- Li, J.; Lu, C.; Pei, L.; Zhang, C.; Tieu, K. Influence of solute hydrogen on the interaction of screw dislocations with vicinal twin boundaries in nickel. Scr. Mater. 2019, 173, 115–119. [Google Scholar] [CrossRef]
- Yu, M.; Chen, W.; Kania, R.; Van Boven, G.; Been, J. Crack propagation of pipeline steel exposed to a near-neutral pH environment under variable pressure fluctuations. Int. J. Fatigue 2016, 82, 658–666. [Google Scholar] [CrossRef]
- Yu, M.; Chen, W.; Kania, R.; Van Boven, G.; Been, J. Underload-induced crack growth behavior of minor cycles of pipeline steel in near-neutral pH environment. Fatigue Fract. Eng. M 2015, 38, 681–692. [Google Scholar] [CrossRef]
- Murakami, Y.; Kanezaki, T.; Mine, Y.; Matsuoka, S. Hydrogen Embrittlement Mechanism in Fatigue of Austenitic Stainless Steels. Met. Mater. Trans. A 2008, 39, 1327–1339. [Google Scholar] [CrossRef]
- Yu, M.; Xing, X.; Zhang, H.; Zhao, J.; Eadie, R.; Chen, W.; Been, J.; Van Boven, G.; Kania, R. Corrosion fatigue crack growth behavior of pipeline steel under underload-type variable amplitude loading schemes. Acta Mater. 2015, 96, 159–169. [Google Scholar] [CrossRef]
- Wang, S.; Martin, M.L.; Robertson, I.M.; Sofronis, P. Effect of hydrogen environment on the separation of Fe grain boundaries. Acta Mater. 2016, 107, 279–288. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Lu, C.; Wang, L.; Pei, L.; Godbole, A.; Michal, G. The Role of Hydrogen on the Behavior of Intergranular Cracks in Bicrystalline α-Fe Nanowires. Nanomaterials 2021, 11, 294. https://doi.org/10.3390/nano11020294
Li J, Lu C, Wang L, Pei L, Godbole A, Michal G. The Role of Hydrogen on the Behavior of Intergranular Cracks in Bicrystalline α-Fe Nanowires. Nanomaterials. 2021; 11(2):294. https://doi.org/10.3390/nano11020294
Chicago/Turabian StyleLi, Jiaqing, Cheng Lu, Long Wang, Linqing Pei, Ajit Godbole, and Guillaume Michal. 2021. "The Role of Hydrogen on the Behavior of Intergranular Cracks in Bicrystalline α-Fe Nanowires" Nanomaterials 11, no. 2: 294. https://doi.org/10.3390/nano11020294
APA StyleLi, J., Lu, C., Wang, L., Pei, L., Godbole, A., & Michal, G. (2021). The Role of Hydrogen on the Behavior of Intergranular Cracks in Bicrystalline α-Fe Nanowires. Nanomaterials, 11(2), 294. https://doi.org/10.3390/nano11020294






