Strain Sensor via Wood Anomalies in 2D Dielectric Array
Abstract
:1. Instruction
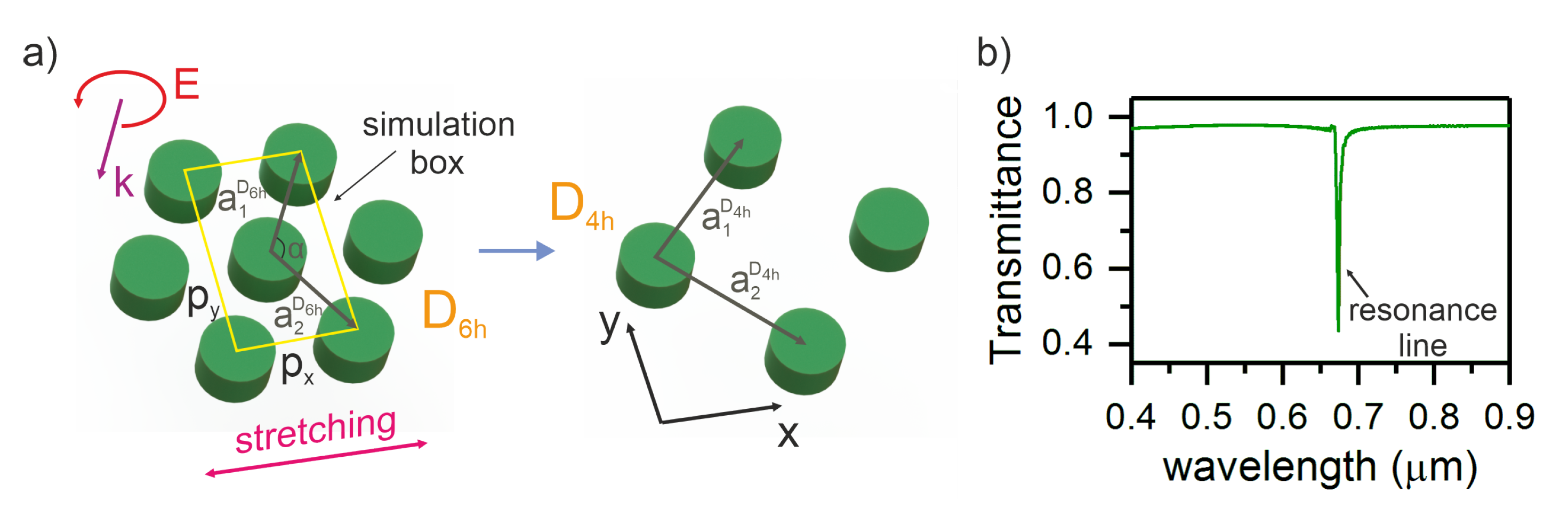
2. Description of the Structure
3. Wood’s Anomalies in Two-Dimensional Periodic Array
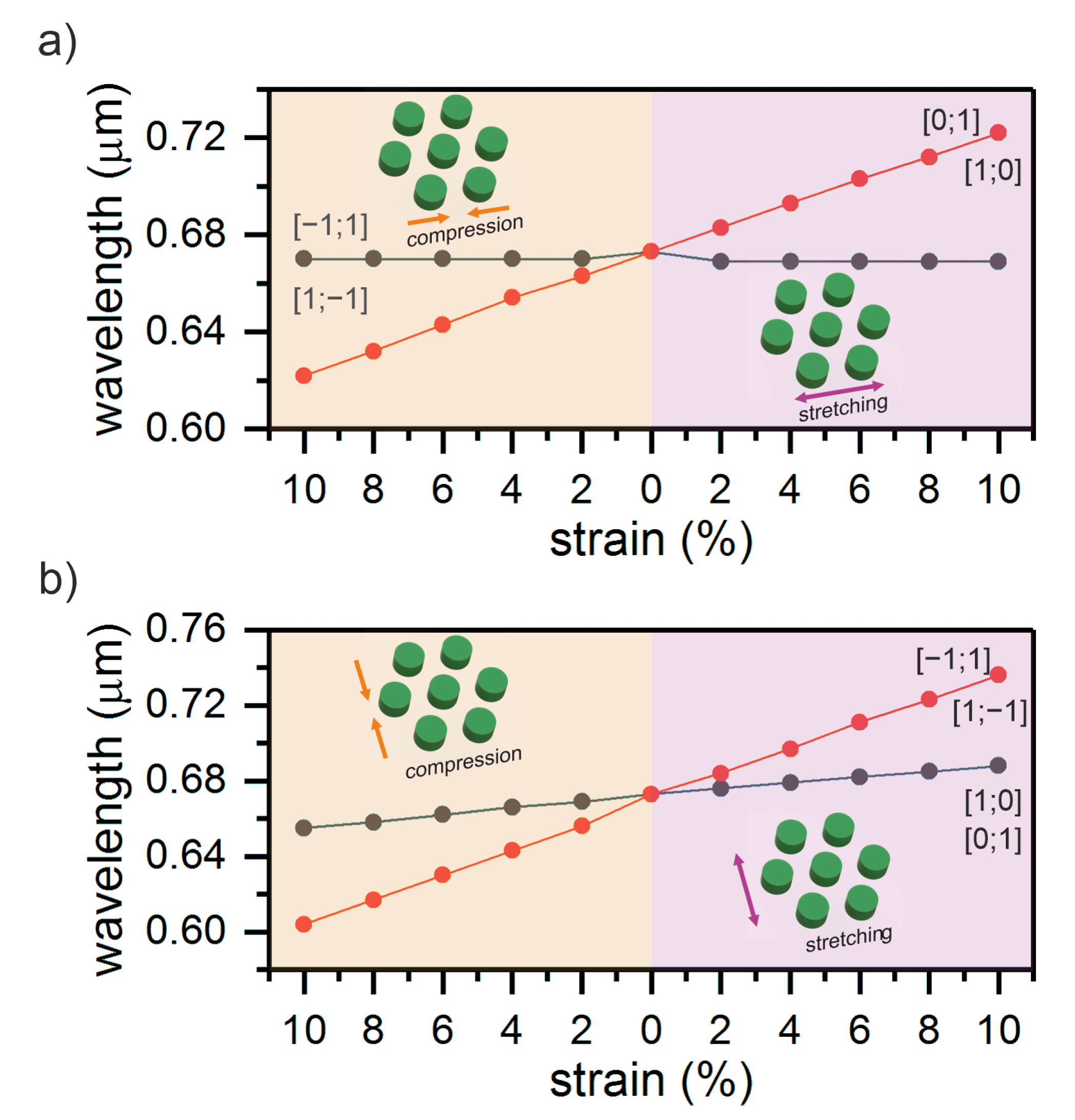
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Špačková, B.; Homola, J. Sensing properties of lattice resonances of 2D metal nanoparticle arrays: An analytical model. Opt. Express 2013, 21, 27490. [Google Scholar] [CrossRef]
- Špačková, B.; Ermini, M.L.; Homola, J. High-performance biosensor exploiting a light guidance in sparse arrays of metal nanoparticles. Opt. Lett. 2019, 44, 1568. [Google Scholar] [CrossRef] [PubMed]
- Mejía-Salazar, J.R.; Oliveira, O.N. Plasmonic Biosensing. Chem. Rev. 2018, 118, 10617–10625. [Google Scholar] [CrossRef]
- Spackova, B.; Wrobel, P.; Bockova, M.; Homola, J. Optical Biosensors Based on Plasmonic Nanostructures: A Review. Proc. IEEE 2016, 104, 2380–2408. [Google Scholar] [CrossRef]
- Utyushev, A.D.; Zakomirnyi, V.I.; Rasskazov, I.L. Collective lattice resonances: Plasmonics and beyond. Rev. Phys. 2021, 6, 100051. [Google Scholar] [CrossRef]
- Kravets, V.G.; Kabashin, A.V.; Barnes, W.L.; Grigorenko, A.N. Plasmonic surface lattice resonances: A review of properties and applications. Chem. Rev. 2018, 118, 5912–5951. [Google Scholar] [CrossRef] [PubMed]
- Limonov, M.F.; Rybin, M.V.; Poddubny, A.N.; Kivshar, Y.S. Fano resonances in photonics. Nat. Photon. 2017, 11, 543–554. [Google Scholar] [CrossRef]
- Su, W.; Ding, Y.; Luo, Y.; Liu, Y. A high figure of merit refractive index sensor based on Fano resonance in all-dielectric metasurface. Results Phys. 2020, 16, 102833. [Google Scholar] [CrossRef]
- Li, Y.; Yuan, Y.; Peng, X.; Song, J.; Liu, J.; Qu, J. An ultrasensitive Fano resonance biosensor using two dimensional hexagonal boron nitride nanosheets: Theoretical analysis. RSC Adv. 2019, 9, 29805–29812. [Google Scholar] [CrossRef] [Green Version]
- Romano, S.; Zito, G.; Yépez, S.N.L.; Cabrini, S.; Penzo, E.; Coppola, G.; Rendina, I.; Mocellaark, V. Tuning the exponential sensitivity of a bound-state-in-continuum optical sensor. Opt. Express 2019, 27, 18776. [Google Scholar] [CrossRef]
- Romano, S.; Zito, G.; Torino, S.; Calafiore, G.; Penzo, E.; Coppola, G.; Cabrini, S.; Rendina, I.; Mocella, V. Label-free sensing of ultralow-weight molecules with all-dielectric metasurfaces supporting bound states in the continuum. Photon. Res. 2018, 6, 726. [Google Scholar] [CrossRef]
- Maksimov, D.N.; Gerasimov, V.S.; Romano, S.; Polyutov, S.P. Refractive index sensing with optical bound states in the continuum. Opt. Express 2020, 28, 38907. [Google Scholar] [CrossRef] [PubMed]
- Wood, R.W. On a remarkable case of uneven distribution of light in a diffraction grating spectrum. Proc. Phys. Soc. Lond. 1902, 18, 269–275. [Google Scholar] [CrossRef]
- Rayleigh, L. On the Dynamical Theory of Gratings. Proc. R. Soc. A Math. Phys. Eng. Sci. 1907, 79, 399–416. [Google Scholar] [CrossRef]
- Fano, U. The Theory of Anomalous Diffraction Gratings and of Quasi-Stationary Waves on Metallic Surfaces (Sommerfeld’s Waves). J. Opt. Soc. Am. 1941, 31, 213. [Google Scholar] [CrossRef]
- Gutha, R.R.; Sadeghi, S.M.; Wing, W.J. Ultrahigh refractive index sensitivity and tunable polarization switching via infrared plasmonic lattice modes. Appl. Phys. Lett. 2017, 110, 153103. [Google Scholar] [CrossRef]
- Danilov, A.; Tselikov, G.; Wu, F.; Kravets, V.G.; Ozerov, I.; Bedu, F.; Grigorenko, A.N.; Kabashin, A.V. Ultra-narrow surface lattice resonances in plasmonic metamaterial arrays for biosensing applications. Biosens. Bioelectron. 2018, 104, 102–112. [Google Scholar] [CrossRef] [Green Version]
- Charconnet, M.; Kuttner, C.; Matricardi, C.; Mihi, A.; Liz-Marzan, L.M.; Seifert, A. Tunable Plasmonics by Self-Assembled Stretchable Superlattices on Macroscopic Scale. In Proceedings of the 2019 International Conference on Optical MEMS and Nanophotonics (OMN), Daejeon, Korea, 28 July–1 August 2019. [Google Scholar] [CrossRef]
- Matricardi, C.; Hanske, C.; Garcia-Pomar, J.L.; Langer, J.; Mihi, A.; Liz-Marzán, L.M. Gold Nanoparticle Plasmonic Superlattices as Surface-Enhanced Raman Spectroscopy Substrates. ACS Nano 2018, 12, 8531–8539. [Google Scholar] [CrossRef] [Green Version]
- Chang, K.H.; Cheng, J.S.; Lu, T.W.; Lee, P.T. Engineering surface lattice resonance of elliptical gold nanodisk array for enhanced strain sensing. Opt. Express 2018, 26, 33215. [Google Scholar] [CrossRef] [PubMed]
- Yang, A.; Hryn, A.J.; Bourgeois, M.R.; Lee, W.K.; Hu, J.; Schatz, G.C.; Odom, T.W. Programmable and reversible plasmon mode engineering. Proc. Natl. Acad. Sci. USA 2016, 113, 14201–14206. [Google Scholar] [CrossRef] [Green Version]
- Andueza, A.; Pérez-Conde, J.; Sevilla, J. Strain sensing based on resonant states in 2D dielectric photonic quasicrystals. Opt. Express 2021, 29, 6980. [Google Scholar] [CrossRef]
- Feng, D.; Zhang, H.; Xu, S.; Tian, L.; Song, N. Fabrication of Plasmonic Nanoparticles on a Wave Shape PDMS Substrate. Plasmonics 2017, 12, 1627–1631. [Google Scholar] [CrossRef]
- Kahraman, M.; Daggumati, P.; Kurtulus, O.; Seker, E.; Wachsmann-Hogiu, S. Fabrication and Characterization of Flexible and Tunable Plasmonic Nanostructures. Sci. Rep. 2013, 3, 3396. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cui, Y.; Zhou, J.; Tamma, V.A.; Park, W. Dynamic Tuning and Symmetry Lowering of Fano Resonance in Plasmonic Nanostructure. ACS Nano 2012, 6, 2385–2393. [Google Scholar] [CrossRef]
- Yoo, D.; Johnson, T.W.; Cherukulappurath, S.; Norris, D.J.; Oh, S.H. Template-Stripped Tunable Plasmonic Devices on Stretchable and Rollable Substrates. ACS Nano 2015, 9, 10647–10654. [Google Scholar] [CrossRef]
- Ponomareva, E.; Volk, K.; Mulvaney, P.; Karg, M. Surface Lattice Resonances in Self-Assembled Gold Nanoparticle Arrays: Impact of Lattice Period, Structural Disorder, and Refractive Index on Resonance Quality. Langmuir 2020, 36, 13601–13612. [Google Scholar] [CrossRef] [PubMed]
- Capretti, A.; Ringsmuth, A.K.; van Velzen, J.F.; Rosnik, A.; Croce, R.; Gregorkiewicz, T. Nanophotonics of higher-plant photosynthetic membranes. Light Sci. Appl. 2019, 8. [Google Scholar] [CrossRef] [PubMed]
- Taflove, A.; Hagness, S.C. Computational Electrodynamics: The Finite-Difference Time-Domain Method, 3rd ed.; Artech House Antennas and Propagation Library, Artech House: Boston, MA, USA, 2005. [Google Scholar]
- Zakomirnyi, V.I.; Ershov, A.E.; Gerasimov, V.S.; Karpov, S.V.; Ågren, H.; Rasskazov, I.L. Collective lattice resonances in arrays of dielectric nanoparticles: A matter of size. Opt. Lett. 2019, 44, 5743. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bikbaev, R.G.; Timofeev, I.V.; Shabanov, V.F. Strain Sensor via Wood Anomalies in 2D Dielectric Array. Nanomaterials 2021, 11, 1022. https://doi.org/10.3390/nano11041022
Bikbaev RG, Timofeev IV, Shabanov VF. Strain Sensor via Wood Anomalies in 2D Dielectric Array. Nanomaterials. 2021; 11(4):1022. https://doi.org/10.3390/nano11041022
Chicago/Turabian StyleBikbaev, Rashid G., Ivan V. Timofeev, and Vasiliy F. Shabanov. 2021. "Strain Sensor via Wood Anomalies in 2D Dielectric Array" Nanomaterials 11, no. 4: 1022. https://doi.org/10.3390/nano11041022
APA StyleBikbaev, R. G., Timofeev, I. V., & Shabanov, V. F. (2021). Strain Sensor via Wood Anomalies in 2D Dielectric Array. Nanomaterials, 11(4), 1022. https://doi.org/10.3390/nano11041022







