Ultra-Broadband Refractory All-Metal Metamaterial Selective Absorber for Solar Thermal Energy Conversion
Abstract
:1. Introduction
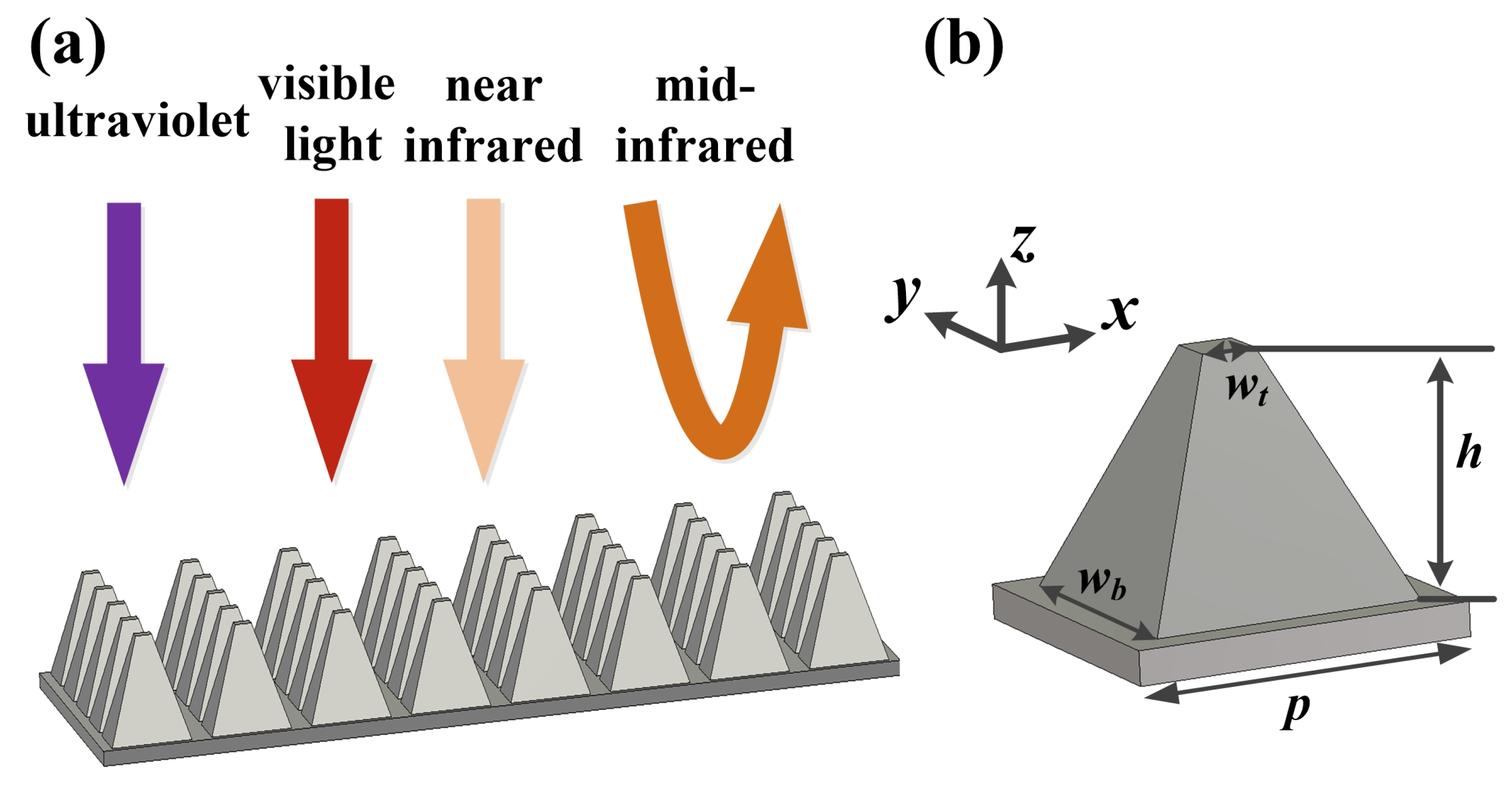
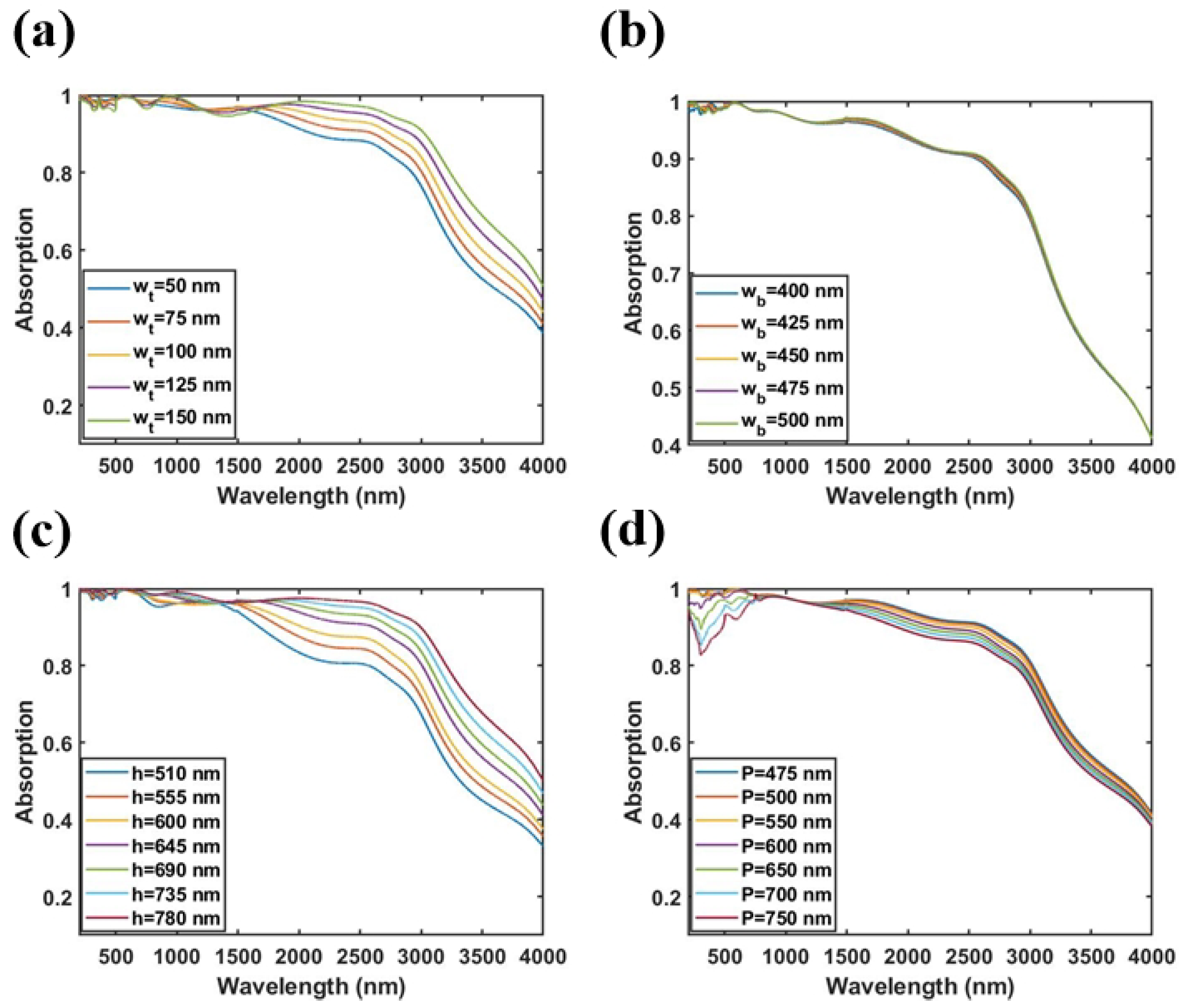
2. Structure and Results
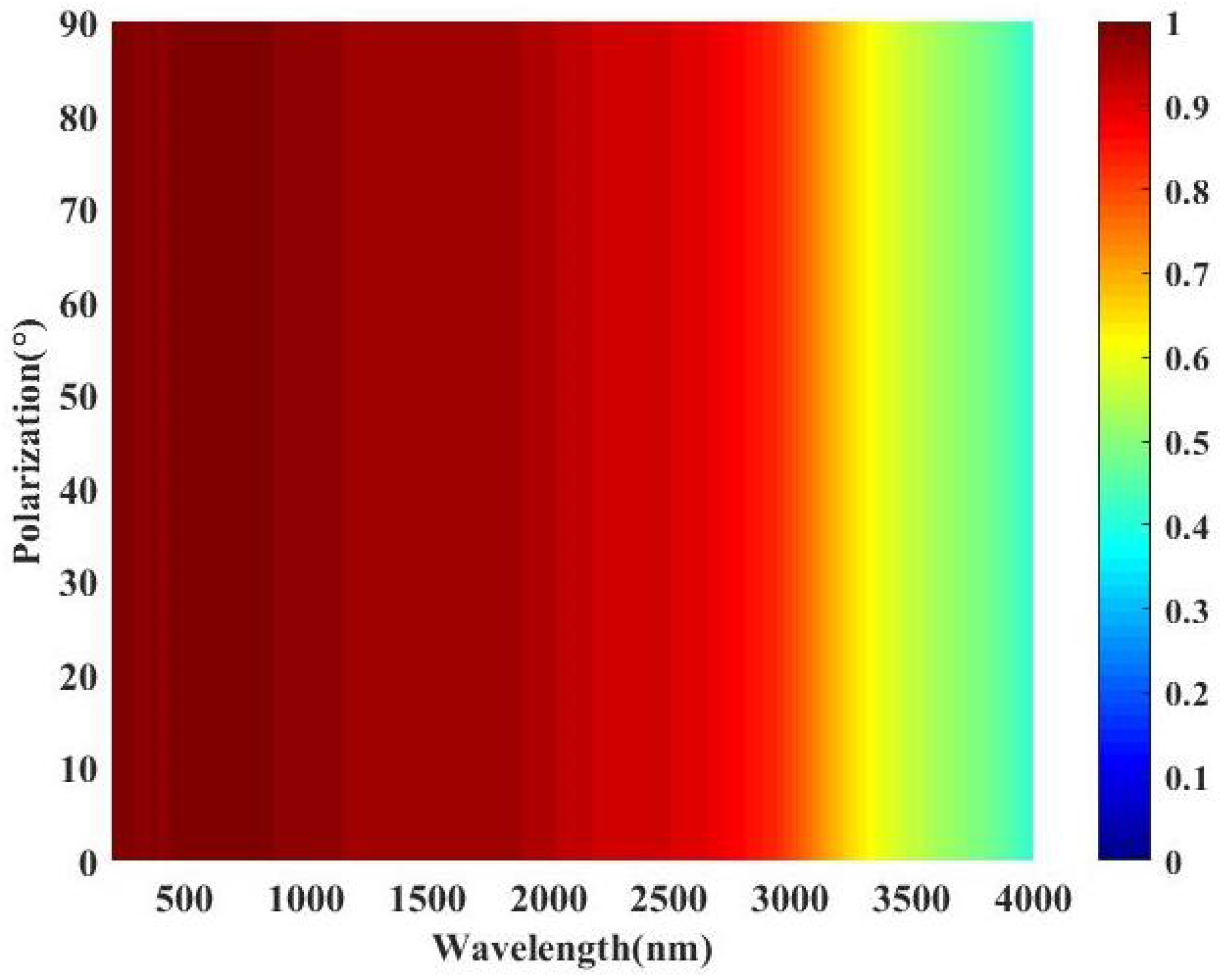
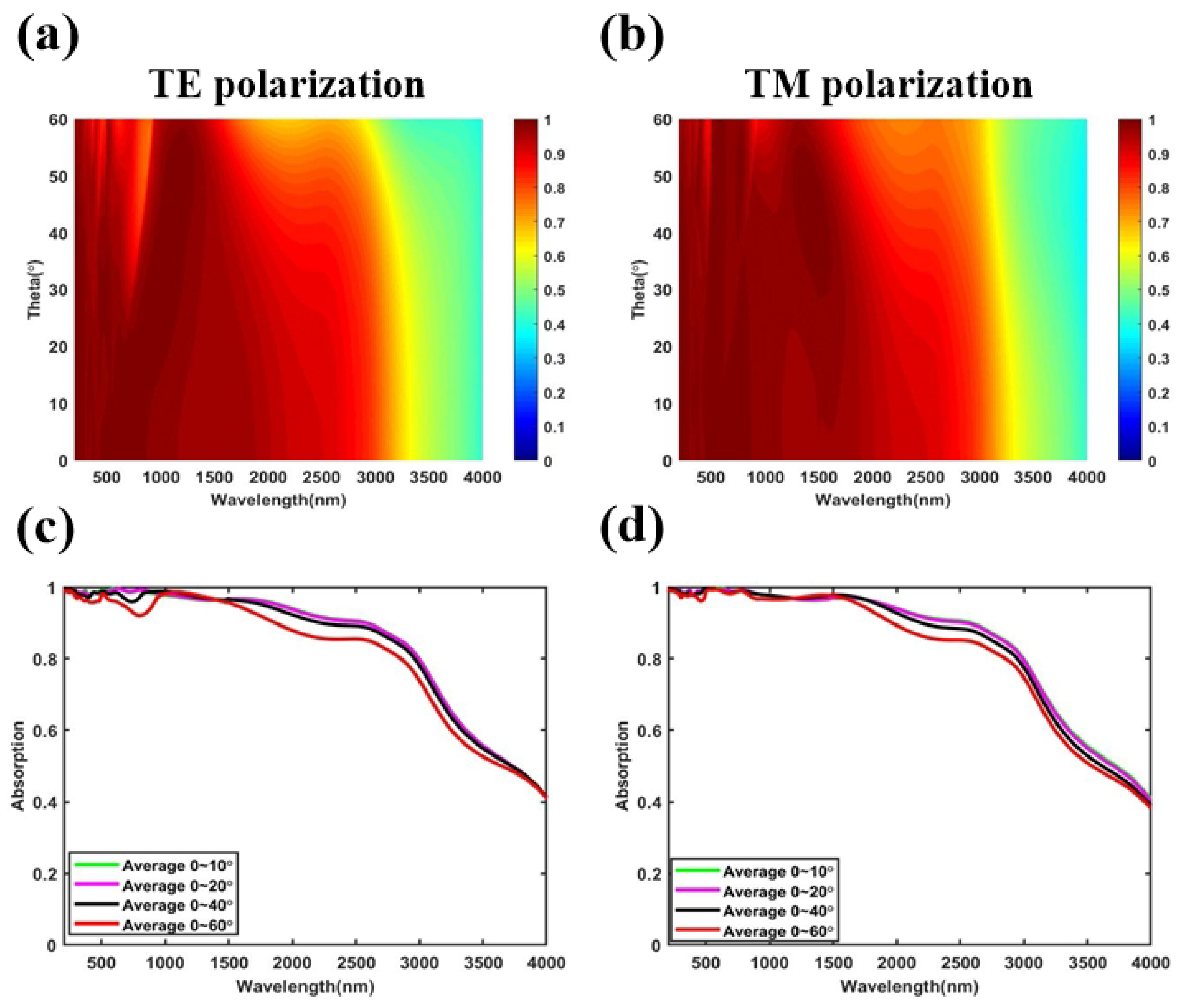
3. Principle, Analysis, and Discussions
4. Solar Energy Harvesting and Conversion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Landy, N.I.; Sajuyigbe, S.; Mock, J.J.; Smith, D.R.; Padilla, W.J. Perfect metamaterial absorber. Phys. Rev. Lett. 2008, 100, 207402. [Google Scholar] [CrossRef]
- Li, Y.; Lin, C.; Wu, Z.; Chen, Z.; Chi, C.; Cao, F.; Mei, D.; Yan, H.; Tso, C.Y.; Chao, C.Y.; et al. Solution-Processed All-Ceramic Plasmonic Metamaterials for Efficient Solar–Thermal Conversion over 100–727 °C. Adv. Mater. 2021, 33, 2005074. [Google Scholar] [CrossRef] [PubMed]
- Lin, K.T.; Lin, H.; Yang, T.; Jia, B. Structured graphene metamaterial selective absorbers for high efficiency and omnidirectional solar thermal energy conversion. Nat. Commun. 2020, 11, 1389. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Li, Y.; Li, D.; Zhou, D.; Chi, C.; Yang, S.; Huang, B. Efficient, Scalable, and High-Temperature Selective Solar Absorbers Based on Hybrid-Strategy Plasmonic Metamaterials. Sol. RRL 2018, 2, 1800057. [Google Scholar] [CrossRef]
- Vora, A.; Gwamuri, J.; Pala, N.; Kulkarni, A.; Pearce, J.M.; Güney, D.Ö. Exchanging ohmic losses in metamaterial absorbers with useful optical absorption for photovoltaics. Sci. Rep. 2014, 4, 4901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, N.; Mesch, M.; Weiss, T.; Hentschel, M.; Giessen, H. Infrared perfect absorber and its application as plasmonic sensor. Nano Lett. 2010, 10, 2342–2348. [Google Scholar] [CrossRef]
- Cong, L.; Tan, S.; Yahiaoui, R.; Yan, F.; Zhang, W.; Singh, R. Experimental demonstration of ultrasensitive sensing with terahertz metamaterial absorbers: A comparison with the metasurfaces. Appl. Phys. Lett. 2015, 106, 031107. [Google Scholar] [CrossRef]
- Long, Y.; Li, Y.; Shen, L.; Liang, W.; Deng, H.; Xu, H. Dually guided-mode-resonant graphene perfect absorbers with narrow bandwidth for sensors. J. Phys. D Appl. Phys. 2016, 49, 32LT01. [Google Scholar] [CrossRef]
- Wen, D.; Yue, F.; Li, G.; Zheng, G.; Chan, K.; Chen, S.; Chen, M.; Li, K.F.; Wong, P.W.H.; Cheah, K.W.; et al. Helicity multiplexed broadband metasurface holograms. Nat. Commun. 2015, 6, 8241. [Google Scholar] [CrossRef]
- Wen, D.; Yue, F.; Ardron, M.; Chen, X. Multifunctional metasurface lens for imaging and Fourier transform. Sci. Rep. 2016, 6, 27628. [Google Scholar] [CrossRef] [Green Version]
- Yu, W.; Lu, Y.; Chen, X.; Xu, H.; Shao, J.; Chen, X.; Sun, Y.; Hao, J.; Dai, N. Large-Area, Broadband, Wide-Angle Plasmonic Metasurface Absorber for Midwavelength Infrared Atmospheric Transparency Window. Adv. Opt. Mater. 2019, 7, 1900841. [Google Scholar] [CrossRef] [Green Version]
- Diem, M.; Koschny, T.; Soukoulis, C.M. Wide-angle perfect absorber/thermal emitter in the terahertz regime. Phys. Rev. B 2009, 79, 033101. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Tyler, T.; Starr, T.; Starr, A.F.; Jokerst, N.M.; Padilla, W.J. Taming the blackbody with infrared metamaterials as selective thermal emitters. Phys. Rev. Lett. 2011, 107, 045901. [Google Scholar] [CrossRef] [Green Version]
- Rephaeli, E.; Fan, S. Absorber and emitter for solar thermo-photovoltaic systems to achieve efficiency exceeding the Shockley-Queisser limit. Opt. Express 2009, 17, 15145–15159. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Shen, S. Broadband near-field radiative thermal emitter/absorber based on hyperbolic metamaterials: Direct numerical simulation by the Wiener chaos expansion method. Phys. Rev. B 2013, 87, 115403. [Google Scholar] [CrossRef] [Green Version]
- Yuan, H.; Liu, X.; Afshinmanesh, F.; Li, W.; Xu, G.; Sun, J.; Lian, B.; Curto, A.G.; Ye, G.; Hikita, Y.; et al. Polarization-sensitive broadband photodetector using a black phosphorus vertical p–n junction. Nat. Nanotechnol. 2015, 10, 707–713. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, H.; Wang, L. Tailoring thermal radiative properties with film-coupled concave grating metamaterials. J. Quant. Spectrosc. Radiat. Transf. 2015, 158, 127–135. [Google Scholar] [CrossRef] [Green Version]
- Zhao, B.; Chen, K.; Buddhiraju, S.; Bhatt, G.; Lipson, M.; Fan, S. High-performance near-field thermophotovoltaics for waste heat recovery. Nano Energy 2017, 41, 344–350. [Google Scholar] [CrossRef]
- Qing, Y.M.; Ma, H.F.; Yu, S.; Cui, T.J. Tunable dual-band perfect metamaterial absorber based on a graphene-SiC hybrid system by multiple resonance modes. J. Phys. D Appl. Phys. 2018, 52, 015104. [Google Scholar] [CrossRef]
- Zhao, B.; Zhang, Z.M. Study of magnetic polaritons in deep gratings for thermal emission control. J. Quant. Spectrosc. Radiat. Transf. 2014, 135, 81–89. [Google Scholar] [CrossRef]
- Kim, S.J.; Choi, H.K.; Lee, H.; Hong, S.H. Solution-processable nanocrystal-based broadband Fabry–Perot absorber for reflective vivid color generation. ACS Appl. Mater. Interfaces 2019, 11, 7280–7287. [Google Scholar] [CrossRef]
- Park, C.S.; Lee, S.S. Narrowband and flexible perfect absorber based on a thin-film nano-resonator incorporating a dielectric overlay. Sci. Rep. 2020, 10, 17727. [Google Scholar] [CrossRef] [PubMed]
- Khonina, S.; Butt, M.; Kazanskiy, N. Numerical investigation of metasurface narrowband perfect absorber and a plasmonic sensor for a near-infrared wavelength range. J. Opt. 2021, 23, 065102. [Google Scholar] [CrossRef]
- Pan, M.; Su, Z.; Yu, Z.; Wu, P.; Jile, H.; Yi, Z.; Chen, Z. A narrowband perfect absorber with high Q-factor and its application in sensing in the visible region. Results Phys. 2020, 19, 103415. [Google Scholar] [CrossRef]
- Kazanskiy, N.L.; Butt, M.A.; Khonina, S.N. Carbon dioxide gas sensor based on polyhexamethylene biguanide polymer deposited on silicon nano-cylinders metasurface. Sensors 2021, 21, 378. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.; Chen, Z.; Liu, J.; Cheng, J.; Zhou, Y.; Xiao, L.; Chen, K. Ultra-narrow band mid-infrared perfect absorber based on hybrid dielectric metasurface. Nanomaterials 2019, 9, 1350. [Google Scholar] [CrossRef] [Green Version]
- Zhang, K.; Luo, W.; Huang, S.; Bai, X.; Shuai, Y.; Zhao, Y.; Zeng, X.; Wu, C.; Zhang, W. Wavelength-selective infrared detector fabricated by integrating LiTaO3 with a metamaterial perfect absorber. Sens. Actuator A Phys. 2020, 313, 112186. [Google Scholar] [CrossRef]
- Zhou, J.; Liu, Z.; Liu, G.; Pan, P.; Liu, X.; Tang, C.; Liu, Z.; Wang, J. Ultra-broadband solar absorbers for high-efficiency thermophotovoltaics. Opt. Express 2020, 28, 36476–36486. [Google Scholar] [CrossRef]
- Feng, H.; Li, X.; Wang, M.; Xia, F.; Zhang, K.; Kong, W.; Dong, L.; Yun, M. Ultrabroadband metamaterial absorbers from ultraviolet to near-infrared based on multiple resonances for harvesting solar energy. Opt. Express 2021, 29, 6000–6010. [Google Scholar] [CrossRef]
- Lei, L.; Li, S.; Huang, H.; Tao, K.; Xu, P. Ultra-broadband absorber from visible to near-infrared using plasmonic metamaterial. Opt. Express 2018, 26, 5686–5693. [Google Scholar] [CrossRef]
- Gong, J.; Yang, F.; Zhang, X. A novel wideband optical absorber based on all-metal 2D gradient nanostructures. J. Phys. D Appl. Phys. 2017, 50, 455105. [Google Scholar] [CrossRef] [Green Version]
- Zhou, L.; Tan, Y.; Wang, J.; Xu, W.; Yuan, Y.; Cai, W.; Zhu, S.; Zhu, J. 3D self-assembly of aluminium nanoparticles for plasmon-enhanced solar desalination. Nat. Photonics 2016, 10, 393–398. [Google Scholar] [CrossRef]
- Bağmancı, M.; Karaaslan, M.; Ünal, E.; Akgol, O.; Sabah, C. Extremely-broad band metamaterial absorber for solar energy harvesting based on star shaped resonator. Opt. Quantum Electron. 2017, 49, 1–14. [Google Scholar] [CrossRef]
- Xiong, H.; Wu, Y.B.; Dong, J.; Tang, M.C.; Jiang, Y.N.; Zeng, X.P. Ultra-thin and broadband tunable metamaterial graphene absorber. Opt. Express 2018, 26, 1681–1688. [Google Scholar] [CrossRef]
- Abdelatif, G.; Hameed, M.F.O.; Obayya, S.; Hussein, M. Ultrabroadband absorber based on a funnel-shaped anisotropic metamaterial. JOSA B 2019, 36, 2889–2895. [Google Scholar] [CrossRef]
- Wu, D.; Liu, C.; Liu, Y.; Xu, Z.; Yu, Z.; Yu, L.; Chen, L.; Ma, R.; Zhang, J.; Ye, H. Numerical study of a wide-angle polarization-independent ultra-broadband efficient selective metamaterial absorber for near-ideal solar thermal energy conversion. RSC Adv. 2018, 8, 21054–21064. [Google Scholar] [CrossRef] [Green Version]
- Wang, B.; Wang, W.; Ashalley, E.; Zhang, X.; Yu, P.; Xu, H.; Wang, Z.M. Broadband refractory plasmonic absorber without refractory metals for solar energy conversion. J. Phys. D Appl. Phys. 2020, 54, 094001. [Google Scholar] [CrossRef]
- Taflove, A.; Hagness, S.C. Computational Electrodynamics: The Finite-Difference Time-Domain Method; Artech House: Boston, MA, USA, 2005. [Google Scholar]
- Edward, D.P.; Palik, I. Handbook of Optical Constants of Solids; Academic: Orlando, FL, USA, 1985. [Google Scholar]
- Wang, H.; Wang, L. Perfect selective metamaterial solar absorbers. Opt. Express 2013, 21, A1078–A1093. [Google Scholar] [CrossRef]
- Li, P.; Liu, B.; Ni, Y.; Liew, K.K.; Sze, J.; Chen, S.; Shen, S. Large-Scale Nanophotonic Solar Selective Absorbers for High-Efficiency Solar Thermal Energy Conversion. Adv. Mater. 2015, 27, 4585–4591. [Google Scholar] [CrossRef]
- Liao, Z.; Liu, Z.; Wu, Q.; Zhan, X.; Liu, M.; Liu, G. Ultra-broadband solar light wave trapping by gradient cavity-thin-film metasurface. J. Phys. D Appl. Phys. 2021, 54, 284002. [Google Scholar] [CrossRef]
- 5 Spectra, American Society for Testing and Materials (ASTM). Available online: http://rredc.nrel.gov/solar/spectra/am1.5/ (accessed on 20 July 2021).
- Riordan, C.; Hulstron, R. What is an air mass 1.5 spectrum? (solar cell performance calculations). In Proceedings of the IEEE Conference on Photovoltaic Specialists, Kissimmee, FL, USA, 21–25 May 1990; pp. 1085–1088. [Google Scholar]
- Duffie, J.A.; Beckman, W.A. Solar Engineering of Thermal Processes; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Khodasevych, I.E.; Wang, L.; Mitchell, A.; Rosengarten, G. Micro-and nanostructured surfaces for selective solar absorption. Adv. Opt. Mater. 2015, 3, 852–881. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, B.; Chen, W.; Niu, T.; Mei, Z. Ultra-Broadband Refractory All-Metal Metamaterial Selective Absorber for Solar Thermal Energy Conversion. Nanomaterials 2021, 11, 1872. https://doi.org/10.3390/nano11081872
Qi B, Chen W, Niu T, Mei Z. Ultra-Broadband Refractory All-Metal Metamaterial Selective Absorber for Solar Thermal Energy Conversion. Nanomaterials. 2021; 11(8):1872. https://doi.org/10.3390/nano11081872
Chicago/Turabian StyleQi, Buxiong, Wenqiong Chen, Tiaoming Niu, and Zhonglei Mei. 2021. "Ultra-Broadband Refractory All-Metal Metamaterial Selective Absorber for Solar Thermal Energy Conversion" Nanomaterials 11, no. 8: 1872. https://doi.org/10.3390/nano11081872
APA StyleQi, B., Chen, W., Niu, T., & Mei, Z. (2021). Ultra-Broadband Refractory All-Metal Metamaterial Selective Absorber for Solar Thermal Energy Conversion. Nanomaterials, 11(8), 1872. https://doi.org/10.3390/nano11081872





