High Q Resonant Sb2S3-Lithium Niobate Metasurface for Active Nanophotonics
Abstract
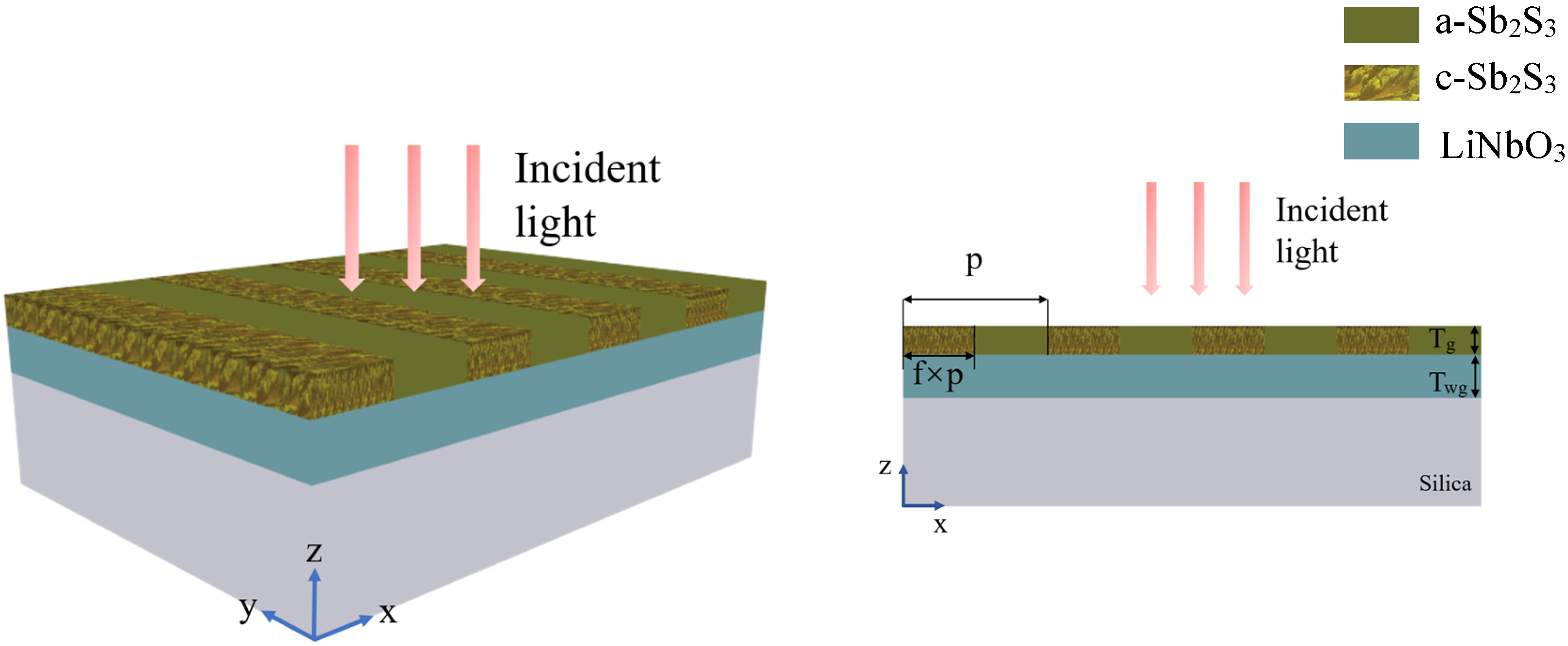
:1. Introduction
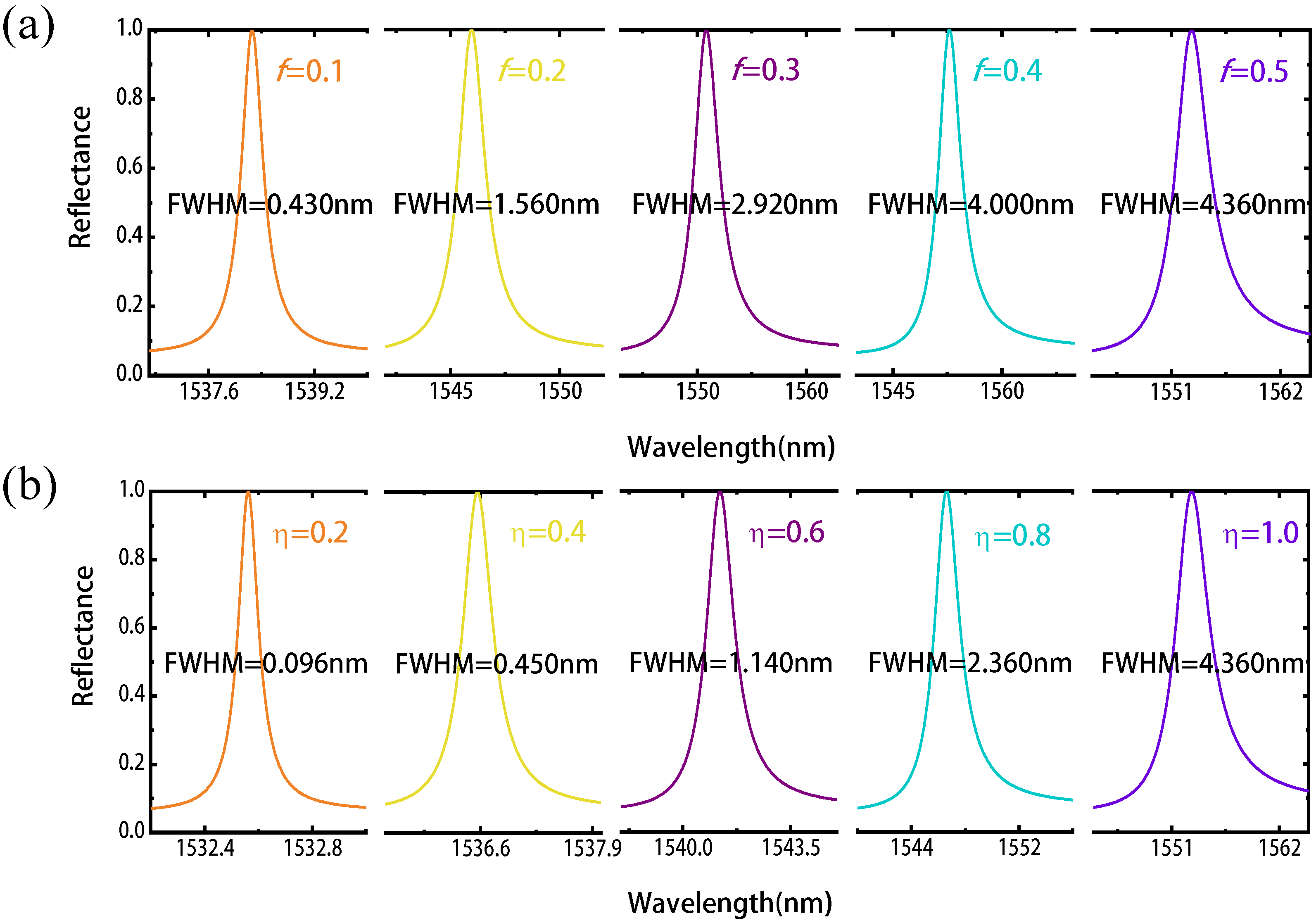
2. Results and Discussion
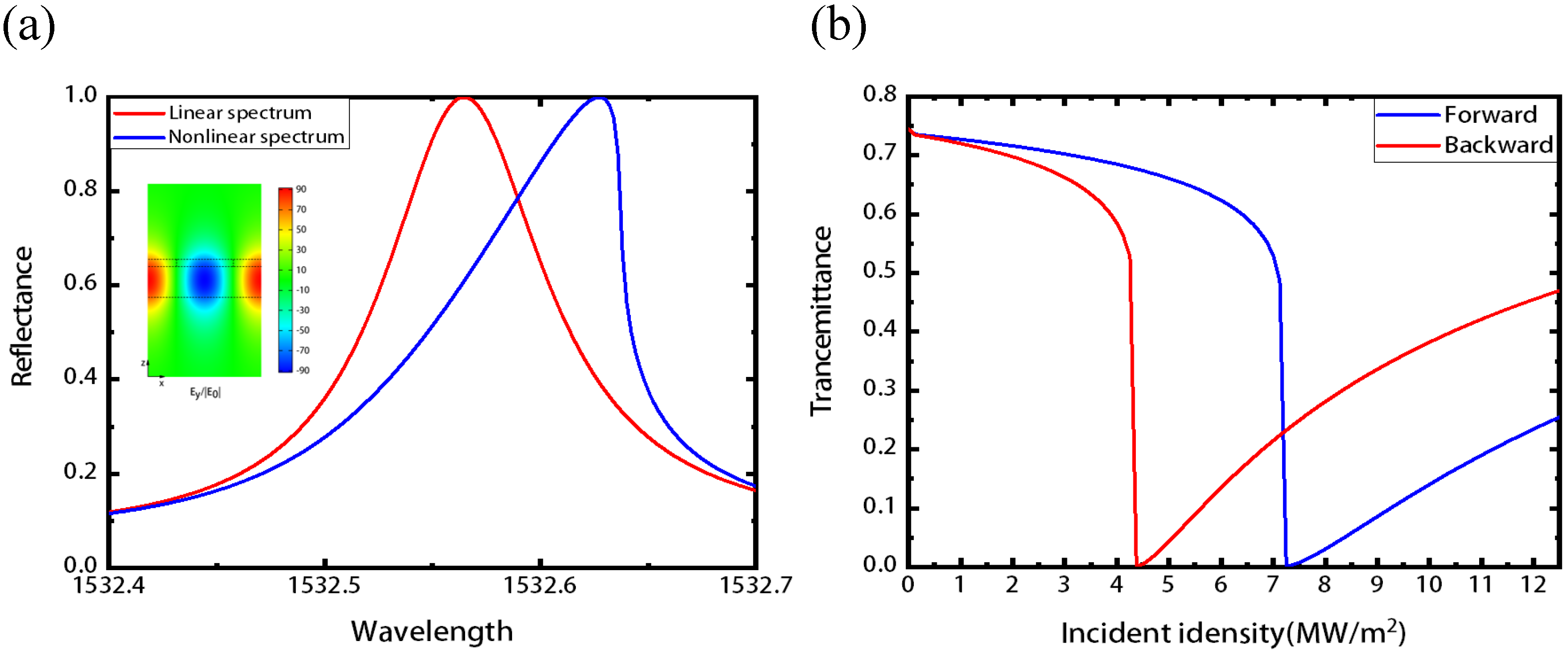
2.1. Nonlinear Optics with the -LN Resonant Metasurface
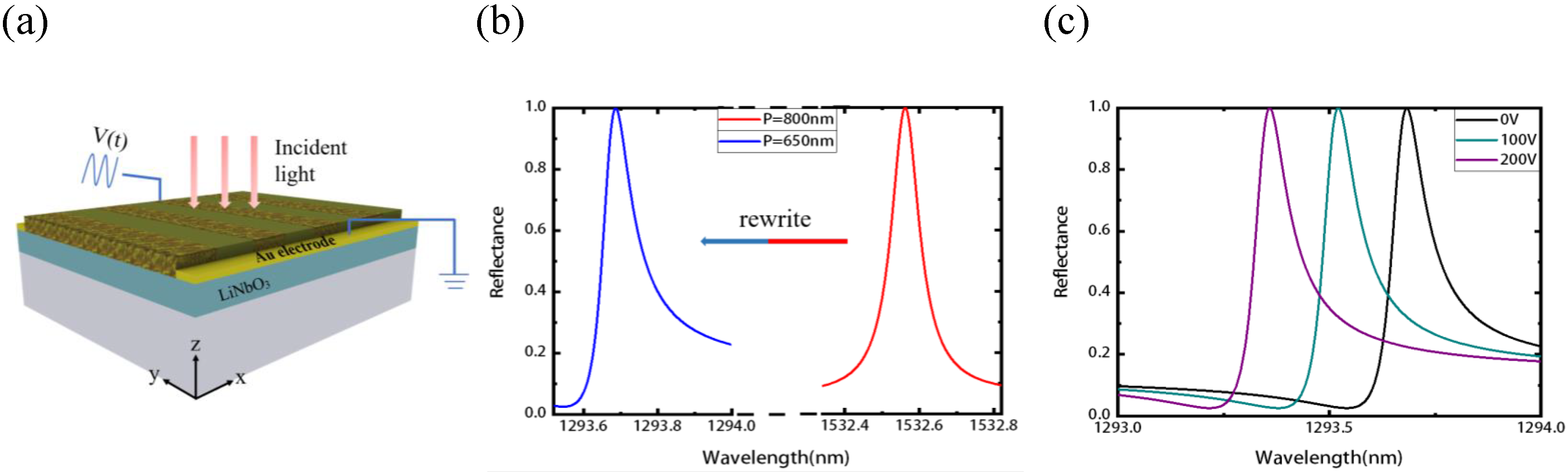
2.2. Electro-Optical Tunability of the -LN Resonant Metasurface
3. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wuttig, M.; Bhaskaran, H.; Taubner, T. Phase-change materials for non-volatile photonic applications. Nat. Photonics 2017, 11, 465–476. [Google Scholar] [CrossRef]
- Abdollahramezani, S.; Hemmatyar, O.; Taghinejad, H.; Krasnok, A.; Kiarashinejad, Y.; Zandehshahvar, M.; Alù, A.; Adibi, A. Tunable nanophotonics enabled by chalcogenide phase-change materials. Nanophotonics 2020, 9, 1189–1241. [Google Scholar] [CrossRef]
- Lepeshov, S.; Krasnok, A. Tunable phase-change metasurfaces. Nat. Nanotechnol. 2021, 16, 615–616. [Google Scholar] [CrossRef]
- Driscoll, T.; Kim, H.T.; Chae, B.G.; Kim, B.J.; Lee, Y.W.; Jokerst, N.M.; Palit, S.; Smith, D.R.; Di Ventra, M.; Basov, D.N. Memory metamaterials. Science 2009, 325, 1518–1521. [Google Scholar] [CrossRef] [Green Version]
- Dicken, M.J.; Aydin, K.; Pryce, I.M.; Sweatlock, L.A.; Boyd, E.M.; Walavalkar, S.; Ma, J.; Atwater, H.A. Frequency tunable near-infrared metamaterials based on VO2 phase transition. Opt. Express 2009, 17, 18330–18339. [Google Scholar] [CrossRef] [Green Version]
- Gholipour, B.; Zhang, J.; MacDonald, K.F.; Hewak, D.W.; Zheludev, N.I. An all-optical, non-volatile, bidirectional, phase-change meta-switch. Adv. Mater. 2013, 25, 3050–3054. [Google Scholar] [CrossRef] [PubMed]
- Qu, Y.; Li, Q.; Du, K.; Cai, L.; Lu, J.; Qiu, M. Dynamic Thermal Emission Control Based on Ultrathin Plasmonic Metamaterials Including Phase-Changing Material GST. Laser Photonics Rev. 2017, 11, 1700091. [Google Scholar] [CrossRef]
- Qu, Y.; Li, Q.; Cai, L.; Pan, M.; Ghosh, P.; Du, K.; Qiu, M. Thermal camouflage based on the phase-changing material GST. Light Sci. Appl. 2018, 7, 26. [Google Scholar] [CrossRef] [PubMed]
- Xu, Z.; Li, Q.; Du, K.; Long, S.; Yang, Y.; Cao, X.; Luo, H.; Zhu, H.; Ghosh, P.; Shen, W.; et al. Spatially Resolved Dynamically Reconfigurable Multilevel Control of Thermal Emission. Laser Photonics Rev. 2019, 14, 1900162. [Google Scholar] [CrossRef]
- Kang, Q.; Li, D.; Guo, K.; Gao, J.; Guo, Z. Tunable Thermal Camouflage Based on GST Plasmonic Metamaterial. Nanomaterials 2021, 11, 260. [Google Scholar] [CrossRef]
- Tanaka, D.; Shoji, Y.; Kuwahara, M.; Wang, X.; Kintaka, K.; Kawashima, H.; Toyosaki, T.; Ikuma, Y.; Tsuda, H. Ultra-small, self-holding, optical gate switch using Ge2Sb2Te5 with a multi-mode Si waveguide. Opt. Express 2012, 20, 10283–10294. [Google Scholar] [CrossRef] [PubMed]
- Carrillo, S.G.C.; Nash, G.R.; Hayat, H.; Cryan, M.J.; Klemm, M.; Bhaskaran, H.; Wright, C.D. Design of practicable phase-change metadevices for near-infrared absorber and modulator applications. Opt. Express 2016, 24, 13563–13573. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Miller, K.J.; Hallman, K.A.; Haglund, R.F.; Weiss, S.M. Silicon waveguide optical switch with embedded phase change material. Opt. Express 2017, 25, 26527–26536. [Google Scholar] [CrossRef] [PubMed]
- Stegmaier, M.; Ríos, C.; Bhaskaran, H.; Wright, C.D.; Pernice, W.H. Nonvolatile all-optical 1 × 2 switch for chipscale photonic networks. Adv. Opt. Mater. 2017, 5, 1600346. [Google Scholar] [CrossRef]
- Kim, Y.; Wu, P.C.; Sokhoyan, R.; Mauser, K.; Glaudell, R.; Kafaie Shirmanesh, G.; Atwater, H.A. Phase modulation with electrically tunable vanadium dioxide phase-change metasurfaces. Nano Lett. 2019, 19, 3961–3968. [Google Scholar] [CrossRef] [Green Version]
- Sreekanth, K.V.; Medwal, R.; Das, C.M.; Gupta, M.; Mishra, M.; Yong, K.T.; Rawat, R.S.; Singh, R. Electrically Tunable All-PCM Visible Plasmonics. Nano Lett. 2021, 21, 4044–4050. [Google Scholar] [CrossRef] [PubMed]
- Fang, Z.; Zheng, J.; Saxena, A.; Whitehead, J.; Chen, Y.; Majumdar, A. Non-volatile Reconfigurable Integrated Photonics Enabled by Broadband Low-loss Phase Change Material. Adv. Opt. Mater. 2021, 9, 2002049. [Google Scholar] [CrossRef]
- Yin, X.; Steinle, T.; Huang, L.; Taubner, T.; Wuttig, M.; Zentgraf, T.; Giessen, H. Beam switching and bifocal zoom lensing using active plasmonic metasurfaces. Light Sci. Appl. 2017, 6, e17016. [Google Scholar] [CrossRef]
- de Galarreta, C.R.; Alexeev, A.M.; Au, Y.Y.; Lopez-Garcia, M.; Klemm, M.; Cryan, M.; Bertolotti, J.; Wright, C.D. Nonvolatile reconfigurable phase-change metadevices for beam steering in the near infrared. Adv. Funct. Mater. 2018, 28, 1704993. [Google Scholar] [CrossRef] [Green Version]
- Zhu, W.; Yang, R.; Fan, Y.; Fu, Q.; Wu, H.; Zhang, P.; Shen, N.H.; Zhang, F. Controlling optical polarization conversion with Ge2Sb2Te5-based phase-change dielectric metamaterials. Nanoscale 2018, 10, 12054–12061. [Google Scholar] [CrossRef] [Green Version]
- Hosseini, P.; Wright, C.D.; Bhaskaran, H. An optoelectronic framework enabled by low-dimensional phase-change films. Nature 2014, 511, 206–211. [Google Scholar] [CrossRef] [Green Version]
- Carrillo, S.G.C.; Trimby, L.; Au, Y.Y.; Nagareddy, V.K.; Rodriguez-Hernandez, G.; Hosseini, P.; Ríos, C.; Bhaskaran, H.; Wright, C.D. A nonvolatile phase-change metamaterial color display. Adv. Opt. Mater. 2019, 7, 1801782. [Google Scholar] [CrossRef] [Green Version]
- Tao, S.; Li, Q.; Wang, J.; Wang, X.; Cai, J.; Li, S.; Xu, W.; Zhang, K.; Hu, C. Phase Change Materials for Nonvolatile, Solid-State Reflective Displays: From New Structural Design Rules to Enhanced Color-Changing Performance. Adv. Opt. Mater. 2020, 8, 2000062. [Google Scholar] [CrossRef]
- Chu, C.H.; Tseng, M.L.; Chen, J.; Wu, P.C.; Chen, Y.H.; Wang, H.C.; Chen, T.Y.; Hsieh, W.T.; Wu, H.J.; Sun, G.; et al. Active dielectric metasurface based on phase-change medium. Laser Photonics Rev. 2016, 10, 986–994. [Google Scholar] [CrossRef]
- Chen, K.; Feng, Y.; Monticone, F.; Zhao, J.; Zhu, B.; Jiang, T.; Zhang, L.; Kim, Y.; Ding, X.; Zhang, S.; et al. A Reconfigurable Active Huygens’ Metalens. Adv. Mater. 2017, 29. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gerislioglu, B.; Ahmadivand, A.; Karabiyik, M.; Sinha, R.; Pala, N. VO2 based Reconfigurable Antenna Platform with Addressable Microheater Matrix. Adv. Electron. Mater. 2017, 3, 1700170. [Google Scholar] [CrossRef]
- Zhu, Z.; Evans, P.G.; Haglund, R.F.; Valentine, J.G. Dynamically Reconfigurable Metadevice Employing Nanostructured Phase-Change Materials. Nano Lett. 2017, 17, 4881–4885. [Google Scholar] [CrossRef] [PubMed]
- Bai, W.; Yang, P.; Huang, J.; Chen, D.; Zhang, J.; Zhang, Z.; Yang, J.; Xu, B. Near-infrared tunable metalens based on phase change material Ge2Se2Te5. Sci. Rep. 2019, 9, 5368. [Google Scholar] [CrossRef] [Green Version]
- Gholipour, B.; Piccinotti, D.; Karvounis, A.; MacDonald, K.F.; Zheludev, N.I. Reconfigurable ultraviolet and high-energy visible dielectric metamaterials. Nano Lett. 2019, 19, 1643–1648. [Google Scholar] [CrossRef] [PubMed]
- Cueff, S.; Taute, A.; Bourgade, A.; Lumeau, J.; Monfray, S.; Song, Q.; Genevet, P.; Devif, B.; Letartre, X.; Berguiga, L. Reconfigurable Flat Optics with Programmable Reflection Amplitude Using Lithography Free Phase Change Material Ultra Thin Films. Adv. Opt. Mater. 2020, 9, 2001291. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, Y.; Hong, Q.; Xu, W.; Zhu, Z.; Yuan, X. Near-Infrared Rewritable, Non-Volatile Subwavelength Absorber Based on Chalcogenide Phase Change Materials. Nanomaterials 2020, 10, 1222. [Google Scholar] [CrossRef]
- Shalaginov, M.Y.; An, S.; Zhang, Y.; Yang, F.; Su, P.; Liberman, V.; Chou, J.B.; Roberts, C.M.; Kang, M.; Rios, C.; et al. Reconfigurable all-dielectric metalens with diffraction-limited performance. Nat. Commun. 2021, 12, 1225. [Google Scholar] [CrossRef]
- Qin, S.; Xu, N.; Huang, H.; Jie, K.; Liu, H.; Guo, J.; Meng, H.; Wang, F.; Yang, X.; Wei, Z. Near-infrared thermally modulated varifocal metalens based on the phase change material Sb2S3. Opt. Express 2021, 29, 7925–7934. [Google Scholar] [CrossRef]
- Xiao, S.; Wang, T.; Liu, T.; Zhou, C.; Jiang, X.; Zhang, J. Active metamaterials and metadevices: A review. J. Phys. D Appl. Phys. 2020, 53, 503002. [Google Scholar] [CrossRef]
- Zhang, Y.; Fowler, C.; Liang, J.; Azhar, B.; Shalaginov, M.Y.; An, S.; Chou, J.B.; Roberts, C.M.; Liberman, V.; Kang, M.; et al. Electrically Reconfigurable Nonvolatile Metasurface Using Low-Loss Optical Phase Change Material. Nat. Nanotechnol. 2021, 16, 661–666. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.; Rogers, E.T.F.; Gholipour, B.; Wang, C.M.; Yuan, G.; Teng, J.; Zheludev, N.I. Optically reconfigurable metasurfaces and photonic devices based on phase change materials. Nat. Photonics 2016, 10, 60–65. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y.; Landreman, P.; Schoen, D.; Okabe, K.; Marshall, A.; Celano, U.; Wong, H.S.P.; Park, J.; Brongersma, M.L. Electrical Tuning of Phase Change Antennas and Metasurfaces. Nat. Nanotechnol. 2021, 16, 667–672. [Google Scholar] [CrossRef]
- Abdollahramezani, S.; Hemmatyar, O.; Taghinejad, M.; Taghinejad, H.; Krasnok, A.; Eftekhar, A.A.; Teichrib, C.; Deshmukh, S.; El-Sayed, M.; Pop, E.; et al. Electrically driven programmable phase-change meta-switch reaching 80% efficiency. arXiv 2021, arXiv:2104.10381. [Google Scholar]
- Taghinejad, H.; Abdollahramezani, S.; Eftekhar, A.A.; Fan, T.; Hosseinnia, A.H.; Hemmatyar, O.; Dorche, A.E.; Gallmon, A.; Adibi, A. ITO-based microheaters for reversible multi-stage switching of phase-change materials: Towards miniaturized beyond-binary reconfigurable integrated photonics. Opt. Express 2021, 29, 20449–20462. [Google Scholar] [CrossRef]
- Delaney, M.; Zeimpekis, I.; Lawson, D.; Hewak, D.W.; Muskens, O.L. A New Family of Ultralow Loss Reversible Phase change Materials for Photonic Integrated Circuits: Sb2S3 and Sb2Se3. Adv. Funct. Mater. 2020, 30, 2002447. [Google Scholar] [CrossRef]
- Weis, R.S.; Gaylord, T.K. Lithium niobate: Summary of physical properties and crystal structure. Appl. Phys. Solids Surfaces 1985, 37, 191–203. [Google Scholar] [CrossRef]
- Boes, A.; Corcoran, B.; Chang, L.; Bowers, J.; Mitchell, A. Status and Potential of Lithium Niobate on Insulator (LNOI) for Photonic Integrated Circuits. Laser Photonics Rev. 2018, 12, 1700256. [Google Scholar] [CrossRef]
- Kong, Y.; Bo, F.; Wang, W.; Zheng, D.; Liu, H.; Zhang, G.; Rupp, R.; Xu, J. Recent Progress in Lithium Niobate: Optical Damage, Defect Simulation, and On-Chip Devices. Adv. Mater. 2020, 32, e1806452. [Google Scholar] [CrossRef]
- Chen, F. Photonic guiding structures in lithium niobate crystals produced by energetic ion beams. J. Appl. Phys. 2009, 106, 081101. [Google Scholar] [CrossRef]
- Sayem, A.A.; Cheng, R.; Wang, S.; Tang, H.X. Lithium-niobate-on-insulator waveguide-integrated superconducting nanowire single-photon detectors. Appl. Phys. Lett. 2020, 116, 151102. [Google Scholar] [CrossRef] [Green Version]
- Zhang, M.; Wang, C.; Cheng, R.; Shams-Ansari, A.; Lonar, M. Monolithic ultra-high-Q lithium niobate microring resonator. Optica 2017, 4, 1536–1537. [Google Scholar] [CrossRef]
- Liang, H.; Luo, R.; He, Y.; Jiang, H.; Lin, Q. High-quality lithium niobate photonic crystal nanocavities. Optica 2017, 4, 1251–1258. [Google Scholar] [CrossRef]
- Shao, L.; Maity, S.; Zheng, L.; Wu, L.; Shams-Ansari, A.; Sohn, Y.I.; Puma, E.; Gadalla, M.N.; Zhang, M.; Wang, C.; et al. Phononic Band Structure Engineering for High-Q Gigahertz Surface Acoustic Wave Resonators on Lithium Niobate. Phys. Rev. Appl. 2019, 12, 014022. [Google Scholar] [CrossRef] [Green Version]
- Gao, B.; Ren, M.; Wu, W.; Hu, H.; Cai, W.; Xu, J. Lithium niobate metasurfaces. Laser Photonics Rev. 2019, 13, 1800312. [Google Scholar] [CrossRef] [Green Version]
- Gao, B.; Ren, M.; Wu, W.; Cai, W.; Xu, J. Electro-optic lithium niobate metasurfaces. Sci. China Phys. Mech. Astron. 2021, 64, 240362. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, M.; Chen, X.; Bertrand, M.; Shams-Ansari, A.; Chandrasekhar, S.; Winzer, P.; Loncar, M. Integrated lithium niobate electro-optic modulators operating at CMOS-compatible voltages. Nature 2018, 562, 101–104. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Buscaino, B.; Wang, C.; Shams-Ansari, A.; Reimer, C.; Zhu, R.; Kahn, J.M.; Loncar, M. Broadband electro-optic frequency comb generation in a lithium niobate microring resonator. Nature 2019, 568, 373–377. [Google Scholar] [CrossRef] [PubMed]
- Xu, M.; He, M.; Zhang, H.; Jian, J.; Pan, Y.; Liu, X.; Chen, L.; Meng, X.; Chen, H.; Li, Z.; et al. High-performance coherent optical modulators based on thin-film lithium niobate platform. Nat. Commun. 2020, 11, 3911. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Ling, J.; He, Y.; Javid, U.A.; Xue, S.; Lin, Q. Lithium niobate photonic-crystal electro-optic modulator. Nat. Commun. 2020, 11, 4123. [Google Scholar] [CrossRef] [PubMed]
- Sarabalis, C.J.; Van Laer, R.; Patel, R.N.; Dahmani, Y.D.; Jiang, W.; Mayor, F.M.; Safavi-Naeini, A.H. Acousto-optic modulation of a wavelength-scale waveguide. Optica 2021, 8, 477–483. [Google Scholar] [CrossRef]
- Jin, M.; Chen, J.; Sua, Y.; Kumar, P.; Huang, Y. Efficient electro-optical modulation on thin-film lithium niobate. Opt. Lett. 2021, 46, 1884–1887. [Google Scholar] [CrossRef]
- Lehr, D.; Reinhold, J.; Thiele, I.; Hartung, H.; Dietrich, K.; Menzel, C.; Pertsch, T.; Kley, E.B.; Tünnermann, A. Enhancing Second Harmonic Generation in Gold Nanoring Resonators Filled with Lithium Niobate. Nano Lett. 2015, 15, 1025–1030. [Google Scholar] [CrossRef] [PubMed]
- Lu, J.; Surya, J.B.; Liu, X.; Bruch, A.W.; Gong, Z.; Xu, Y.; Tang, H.X. Periodically poled thin-film lithium niobate microring resonators with a second-harmonic generation efficiency of 250,000%W. Optica 2019, 6, 1455–1460. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Huang, Z.; Sui, Z.; Chen, H.; Zhang, X.; Huang, W.; Guan, H.; Qiu, W.; Dong, J.; Zhu, W.; et al. Optical anapole mode in nanostructured lithium niobate for enhancing second harmonic generation. Nanophotonics 2020, 9, 3575–3585. [Google Scholar] [CrossRef]
- Fang, B.; Li, H.; Zhu, S.; Li, T. Second-harmonic generation and manipulation in lithium niobate slab waveguides by grating metasurfaces. Photonics Res. 2020, 8, 1296–1300. [Google Scholar] [CrossRef]
- Hafermann, M.; Zapf, M.; Ritzer, M.; Printschler, A.; Luo, Y.; Ambrosio, A.; Wilson, W.L.; Ronning, C. Grayscale Nanopatterning of Phase-Change Materials for Subwavelength-Scaled, Inherently Planar, Nonvolatile, and Reconfigurable Optical Devices. ACS Appl. Nano Mater. 2020, 3, 4486–4493. [Google Scholar] [CrossRef]
- Sharon, A.; Rosenblatt, D.; Friesem, A.A. Narrow spectral bandwidths with grating waveguide structures. Appl. Phys. Lett. 1996, 69, 4154–4156. [Google Scholar] [CrossRef]
- Huang, Z.; Lu, H.; Xiong, H.; Li, Y.; Chen, H.; Qiu, W.; Guan, H.; Dong, J.; Zhu, W.; Yu, J.; et al. Fano Resonance on Nanostructured Lithium Niobate for Highly Efficient and Tunable Second Harmonic Generation. Nanomaterials 2019, 9, 69. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lawrence, M.; Barton, D.R.; Dionne, J.A. Nonreciprocal Flat Optics with Silicon Metasurfaces. Nano Lett. 2018, 18, 1104–1109. [Google Scholar] [CrossRef] [PubMed]
- Yin, L.; Jiang, J.; Huo, Y.; Wang, S.; Ning, T. Third-order optical nonlinearity of niobium-rich lithium niobate thin films. Opt. Mater. 2021, 114, 110914. [Google Scholar] [CrossRef]
- Zhu, M.; Abdollahramezani, S.; Hemmatyar, O.; Adibi, A. Linear and Nonlinear Focusing Using Reconfigurable All-Dielectric Metalens Based on Phase-Change Materials. In Frontiers in Optics/Laser Science; Lee, B.M.C.C.K., Jason, J.R., Eds.; Optical Society of America: Washington, DC, USA, 2020; p. JW6B.6. [Google Scholar]
- Wang, C.; Li, Z.; Kim, M.H.; Xiong, X.; Ren, X.F.; Guo, G.C.; Yu, N.; Lončar, M. Metasurface-assisted phase-matching-free second harmonic generation in lithium niobate waveguides. Nat. Commun. 2017, 8, 2098. [Google Scholar] [CrossRef]
- Ma, J.; Xie, F.; Chen, W.; Chen, J.; Wu, W.; Liu, W.; Chen, Y.; Cai, W.; Ren, M.; Xu, J. Nonlinear lithium niobate metasurfaces for second harmonic generation. Laser Photonics Rev. 2021, 15, 2000521. [Google Scholar] [CrossRef]
- Arizmendi, L. Photonic applications of lithium niobate crystals. Phys. Status Solidi (A) 2004, 201, 253–283. [Google Scholar] [CrossRef]
- Zhang, Y.; Chou, J.B.; Li, J.; Li, H.; Du, Q.; Yadav, A.; Zhou, S.; Shalaginov, M.Y.; Fang, Z.; Zhong, H.; et al. Broadband transparent optical phase change materials for high-performance nonvolatile photonics. Nat. Commun. 2019, 10, 4279. [Google Scholar] [CrossRef] [PubMed]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, Q.; Chen, X.; Xu, W.; Zhu, Z.; Yuan, X.; Zhang, J. High Q Resonant Sb2S3-Lithium Niobate Metasurface for Active Nanophotonics. Nanomaterials 2021, 11, 2373. https://doi.org/10.3390/nano11092373
Meng Q, Chen X, Xu W, Zhu Z, Yuan X, Zhang J. High Q Resonant Sb2S3-Lithium Niobate Metasurface for Active Nanophotonics. Nanomaterials. 2021; 11(9):2373. https://doi.org/10.3390/nano11092373
Chicago/Turabian StyleMeng, Qi, Xingqiao Chen, Wei Xu, Zhihong Zhu, Xiaodong Yuan, and Jianfa Zhang. 2021. "High Q Resonant Sb2S3-Lithium Niobate Metasurface for Active Nanophotonics" Nanomaterials 11, no. 9: 2373. https://doi.org/10.3390/nano11092373
APA StyleMeng, Q., Chen, X., Xu, W., Zhu, Z., Yuan, X., & Zhang, J. (2021). High Q Resonant Sb2S3-Lithium Niobate Metasurface for Active Nanophotonics. Nanomaterials, 11(9), 2373. https://doi.org/10.3390/nano11092373







