Silicon Nanosheets: An Emerging 2D Photonic Material with a Large Transient Nonlinear Optical Response beyond Graphene
Abstract
:1. Introduction
2. Materials and Methods
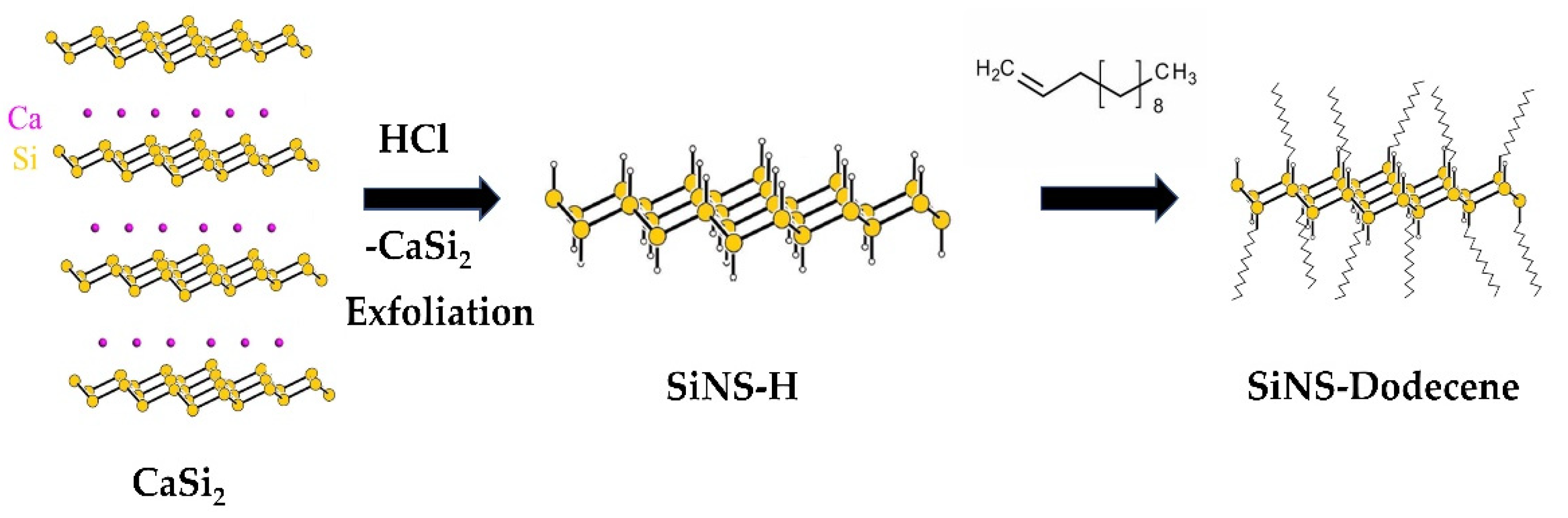
2.1. Synthesis of SiNS–H and SiNS–Dodecene
2.2. Samples Preparation
2.3. Z-Scan Measurements
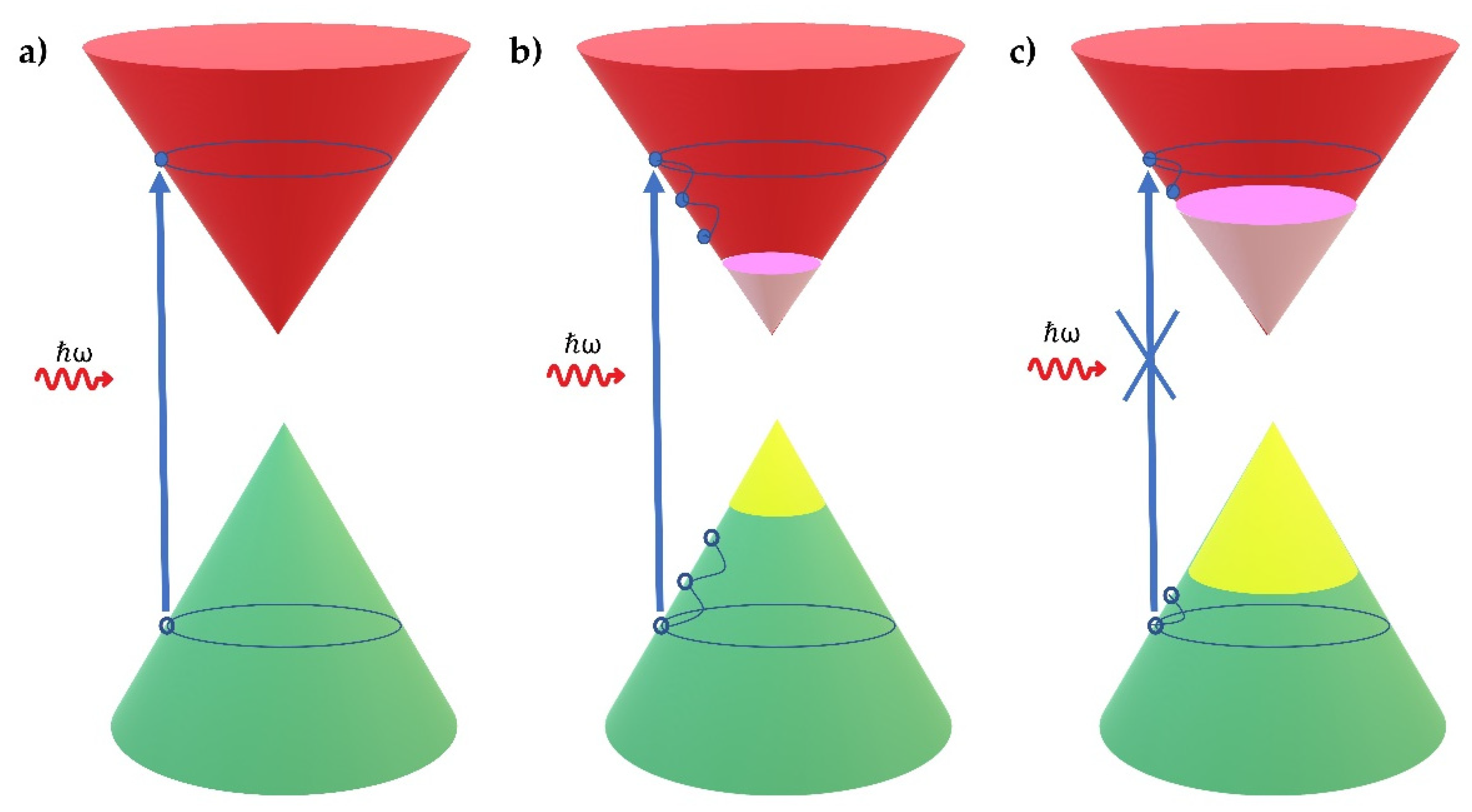
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Geim, A.K.; Novoselov, K.S. The Rise of Graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef]
- Tan, C.; Cao, X.; Wu, X.J.; He, Q.; Yang, J.; Zhang, X.; Chen, J.; Zhao, W.; Han, S.; Nam, G.H.; et al. Recent Advances in Ultrathin Two-Dimensional Nanomaterials. Chem. Rev. 2017, 117, 6225–6331. [Google Scholar] [CrossRef]
- Stavrou, M.; Dalamaras, I.; Karampitsos, N.; Couris, S. Determination of the Nonlinear Optical Properties of Single- and Few-Layered Graphene Dispersions under Femtosecond Laser Excitation: Electronic and Thermal Origin Contributions. J. Phys. Chem. C 2020, 124, 27241–27249. [Google Scholar] [CrossRef]
- Li, S.; Wang, C.; Yin, Y.; Lewis, E.; Wang, P. Novel Layered 2D Materials for Ultrafast Photonics. Nanophotonics 2020, 9, 1743–1786. [Google Scholar] [CrossRef] [Green Version]
- Stavrou, M.; Papadakis, I.; Bawari, S.; Narayanan, T.; Couris, S. Giant Broadband (450–2300 nm) Optical Limiting and Enhancement of the Nonlinear Optical Response of Some Graphenes by Defect Engineering. J. Phys. Chem. C 2021, 125, 16075–16085. [Google Scholar] [CrossRef]
- Papadakis, I.; Stavrou, M.; Bawari, S.; Narayanan, T.; Couris, S. Outstanding Broadband (532 nm to 2.2 μm) and Very Efficient Optical Limiting Performance of Some Defect-Engineered Graphenes. J. Phys. Chem. Lett. 2020, 11, 9515–9520. [Google Scholar] [CrossRef]
- Stathis, A.; Stavrou, M.; Papadakis, I.; Obratzov, I.; Couris, S. Enhancing and Tuning the Nonlinear Optical Response and Wavelength-Agile Strong Optical Limiting Action of N-octylamine Modified Fluorographenes. Nanomaterials 2020, 10, 2319. [Google Scholar] [CrossRef]
- Varma, S.J.; Kumar, J.; Liu, Y.; Layne, K.; Wu, J.; Liang, C.; Nakanishi, Y.; Aliyan, A.; Yang, W.; Ajayan, P.M.; et al. 2D TiS2 Layers: A Superior Nonlinear Optical Limiting Material. Adv. Opt. Mater. 2017, 5, 1700713. [Google Scholar] [CrossRef]
- Deng, D.; Novoselov, K.; Fu, Q.; Zheng, N.; Tian, Z.; Bao, X. Catalysis with Two-Dimensional Materials and their Heterostructures. Nat. Nanotechnol. 2016, 11, 218–230. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.W.; Suh, J.M.; Jang, H.W. Chemical Sensors Based on Two-Dimensional (2D) Materials for Selective Detection of Ions and Molecules in Liquid. Front. Chem. 2019, 7, 708. [Google Scholar] [CrossRef]
- Zhao, K.; Zhu, W.; Liu, S.; Wei, X.; Ye, G.; Su, Y.; He, Z. Two-Dimensional Metal–Organic Frameworks and their Derivatives for Electrochemical Energy Storage and Electrocatalysis. Nanoscale Adv. 2020, 2, 536–562. [Google Scholar] [CrossRef] [Green Version]
- Akhtar, M.; Anderson, G.; Zhao, R.; Alruqi, A.; Mroczkowska, J.E.; Sumanasekera, G.; Jasinski, J.B. Recent Advances in Synthesis, Properties, and Applications of Phosphorene. NPJ 2D Mater. Appl. 2017, 1, 5. [Google Scholar] [CrossRef]
- Molle, A.; Goldberger, J.; Houssa, M.; Xu, Y.; Zhang, S.C.; Akinwande, D. Buckled Two-Dimensional Xene Sheets. Nat. Mater. 2017, 16, 163–169. [Google Scholar] [CrossRef] [PubMed]
- Voiry, D.; Mohite, A.; Chhowalla, M. Phase Engineering of Transition Metal Dichalcogenides. Chem. Soc. Rev. 2015, 44, 2702–2712. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.J.; Yoshida, M.; Suzuki, R.; Iwasa, Y. 2D Crystals of Transition Metal Dichalcogenide and their Iontronic Functionalities. 2D Mater. 2015, 2, 044004. [Google Scholar] [CrossRef]
- Kannan, P.K.; Late, D.J.; Morgan, H.; Rout, C.S. Recent Developments in 2D Layered Inorganic Nanomaterials for Sensing. Nanoscale 2015, 7, 13293–13312. [Google Scholar] [CrossRef]
- Onga, M.; Zhang, Y.; Ideue, T.; Iwasa, Y. Exciton Hall Effect in Monolayer MoS2. Nat. Mater. 2017, 16, 1193–1197. [Google Scholar] [CrossRef]
- Zhang, Z.; Niu, J.; Yang, P.; Gong, Y.; Ji, Q.; Shi, J.; Fang, Q.; Jiang, S.; Li, H.; Zhou, X.; et al. Van der Waals Epitaxial Growth of 2D Metallic Vanadium Diselenide Single Crystals and their Extra-High Electrical Conductivity. Adv. Mater. 2017, 29, 1702359. [Google Scholar] [CrossRef]
- Naguib, M.; Mochalin, V.N.; Barsoum, M.W.; Gogotsi, Y. 25th Anniversary Article: MXenes: A New Family of Two-Dimensional Materials. Adv. Mater. 2013, 26, 992–1005. [Google Scholar] [CrossRef] [PubMed]
- Kim, K.K.; Hsu, A.; Jia, X.; Kim, S.M.; Shi, Y.; Hofmann, M.; Nezich, D.; Rodriguez-Nieva, J.F.; Dresselhaus, M.; Palacios, T.; et al. Synthesis of Monolayer Hexagonal Boron Nitride on Cu Foil Using Chemical Vapor Deposition. Nano Lett. 2012, 12, 161–166. [Google Scholar] [CrossRef]
- Cheng, Z.; Lin, J. Layered Organic–Inorganic Hybrid Perovskites: Structure, Optical Properties, Film Preparation, Patterning and Templating Engineering. Cryst. Eng. Comm. 2010, 12, 2646–2662. [Google Scholar] [CrossRef]
- Dou, L.; Wong, A.B.; Yu, Y.; Lai, M.; Kornienko, N.; Eaton, S.W.; Fu, A.; Bischak, C.G.; Ma, J.; Ding, T.; et al. Atomically Thin Two-Dimensional Organic-Inorganic Hybrid Perovskites. Science 2015, 349, 1518–1521. [Google Scholar] [CrossRef] [Green Version]
- Guzman-Verri, G.G.; Lew Yan Voon, L.C. Electronic Structure of Silicon-Based Nanostructures. Phys. Rev. B 2007, 76, 075131. [Google Scholar] [CrossRef] [Green Version]
- Cahangirov, S.; Topsakal, M.; Aktürk, E.; Şahin, H.; Ciraci, S. Two- and One-Dimensional Honeycomb Structures of Silicon and Germanium. Phys. Rev. Lett. 2009, 102, 236804. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Houssa, M.; Dimoulas, A.; Molle, A. Silicene: A Review of Recent Experimental and Theoretical Investigations. J. Phys. Condens. Matter 2015, 27, 253002. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.C.; Feng, W.; Yao, Y. Quantum Spin Hall Effect in Silicene and Two-Dimensional Germanium. Phys. Rev. Lett. 2011, 107, 076802. [Google Scholar] [CrossRef] [Green Version]
- Le Lay, G. Silicene Transistors. Nat. Nanotechnol. 2015, 10, 202–203. [Google Scholar] [CrossRef] [PubMed]
- Ni, Z.; Zhong, H.; Jiang, X.; Quhe, R.; Luo, G.; Wang, Y.; Ye, M.; Yang, J.; Shi, J.; Lu, J. Tunable Band Gap and Doping Type in Silicene by Surface Adsorption: Towards Tunneling Transistors. Nanoscale 2014, 6, 7609–7618. [Google Scholar] [CrossRef] [Green Version]
- Vogt, P.; De Padova, P.; Quaresima, C.; Avila, J.; Frantzeskakis, E.; Asensio, M.C.; Resta, A.; Ealet, B.; Le Lay, G. Silicene: Compelling Experimental Evidence for Graphenelike Two-Dimensional Silicon. Phys. Rev. Lett. 2012, 108, 155501. [Google Scholar] [CrossRef]
- Lin, C.L.; Arafune, R.; Kawahara, K.; Tsukahara, N.; Minamitani, E.; Kim, Y.; Takagi, N.; Kawai, M. Structure of Silicene Grown on Ag(111). Appl. Phys. Express 2012, 5, 045802. [Google Scholar] [CrossRef]
- Chiappe, D.; Grazianetti, C.; Tallarida, G.; Fanciulli, M.; Molle, A. Local Electronic Properties of Corrugated Silicene Phases. Adv. Mater. 2012, 24, 5088–5093. [Google Scholar] [CrossRef]
- Fleurence, A.; Friedlein, R.; Ozaki, T.; Kawai, H.; Wang, Y.; Yamada-Takamura, Y. Experimental Evidence for Epitaxial Silicene on Diboride Thin Films. Phys. Rev. Lett. 2012, 108, 245501. [Google Scholar] [CrossRef] [PubMed]
- Meng, L.; Wang, Y.; Zhang, L.; Du, S.; Wu, R.; Li, L.; Zhang, Y.; Li, G.; Zhou, H.; Hofer, W.A.; et al. Buckled Silicene Formation on Ir(111). Nano Lett. 2013, 13, 685–690. [Google Scholar] [CrossRef]
- Helbich, T.; Lyuleeva, A.; Höhlein, I.M.D.; Marx, P.; Scherf, L.M.; Kehrle, J.; Fässler, T.F.; Lugli, P.; Rieger, B. Radical-Induced Hydrosilylation Reactions for the Functionalization of Two-Dimensional Hydride Terminated Silicon Nanosheets. Chem. Eur. J. 2016, 22, 6194–6198. [Google Scholar] [CrossRef] [PubMed]
- Feng, M.; Zhan, H.; Chen, Y. Nonlinear Optical and Optical Limiting Properties of Graphene Families. Appl. Phys. Lett. 2010, 96, 033107. [Google Scholar] [CrossRef]
- Wang, G.; Marie, X.; Gerber, I.; Amand, T.; Lagarde, D.; Bouet, L.; Vidal, M.; Balocchi, A.; Urbaszek, B. Giant Enhancement of the Optical Second-Harmonic Emission of WSe2 Monolayers by Laser Excitation at Exciton Resonances. Phys. Rev. Lett. 2015, 114, 097403. [Google Scholar] [CrossRef] [Green Version]
- Wei, C.; Luo, H.; Zhang, H.; Li, C.; Xie, J.; Li, J.; Liu, Y. Passively Q-switched Mid-Infrared Fluoride Fiber Laser Around 3µm Using a Tungsten Disulfide (WS2) Saturable Absorber. Laser Phys. Lett. 2016, 13, 105108. [Google Scholar] [CrossRef]
- Wang, Y.; Li, J.; Han, L.; Lu, R.; Hu, Y.; Li, Z.; Liu, Y. Q-Switched Tm3+-Doped Fiber Laser with a Micro-Fiber Based Black Phosphorus Saturable Absorber. Laser Phys. 2016, 26, 065104. [Google Scholar] [CrossRef]
- Bonaccorso, F.; Sun, Z.; Hasan, T.; Ferrari, A.C. Graphene Photonics and Optoelectronics. Nat. Photonics 2010, 4, 611–622. [Google Scholar] [CrossRef] [Green Version]
- Bao, Q.; Zhang, H.; Wang, Y.; Ni, Z.; Yan, Y.; Shen, Z.X.; Loh, K.P.; Tang, D.Y. Atomic-Layer Graphene as a Saturable Absorber for Ultrafast Pulsed Lasers. Adv. Funct. Mater. 2009, 19, 3077–3083. [Google Scholar] [CrossRef]
- Stavrou, M.; Papadakis, I.; Stathis, A.; Kloberg, M.J.; Mock, J.; Kratky, T.; Günther, S.; Rieger, B.; Becherer, M.; Lyuleeva-Husemann, A.; et al. Silicon Nanosheets versus Graphene Nanosheets: A Comparison of Their Nonlinear Optical Response. J. Phys. Chem. Lett. 2021, 12, 815–821. [Google Scholar] [CrossRef] [PubMed]
- Helbich, T.; Lyuleeva, A.; Marx, P.; Scherf, L.M.; Purkait, T.K.; Fassler, T.F.; Lugli, P.; Veinot, J.G.C.; Rieger, B. Lewis Acid Induced Functionalization of Photoluminescent 2D Silicon Nano-sheets for the Fabrication of Functional Hybrid Films. Adv. Funct. Mater. 2017, 27, 1606764. [Google Scholar] [CrossRef]
- Sheik-bahae, M.; Said, A.A.; Van Stryland, E.W. High-Sensitivity, Single-Beam n2 Measurements. Opt. Lett. 1989, 14, 955–957. [Google Scholar] [CrossRef]
- Papagiannouli, I.; Iliopoulos, K.; Gindre, D.; Sahraoui, B.; Krupka, O.; Smokal, V.; Kolendo, A.; Couris, S. Third-order Nonlinear Optical Response of Push-Pull Azobenzene Polymers. Chem. Phys. Lett. 2012, 554, 107–112. [Google Scholar] [CrossRef] [Green Version]
- Mark, J.E.; Allcock, H.R.; West, R. Inorganic Polymers; Oxford University Press: New York, NY, USA, 2005. [Google Scholar]
- Zheng, X.; Feng, M.; Zhan, H. Giant Optical Limiting Effect in Ormosil Gel Glasses Doped with Graphene Oxide Materials. J. Mater. Chem. C 2013, 1, 6759–6766. [Google Scholar] [CrossRef]
- Stathis, A.; Stavrou, M.; Papadakis, I.; Mock, J.; Kloberg, M.J.; Becherer, M.; Lyuleeva-Husemann, A.; Couris, S. Silicon Nanosheets: A Promising 2D Material with Strong Ultrafast Nonlinear Optical Response. J. Phys. Chem. C 2021, 125, 18510–18516. [Google Scholar] [CrossRef]
- Boyd, R.W. Nonlinear Optics; Academic Press: Orlando, FL, USA, 2008. [Google Scholar]
- Christodoulides, D.N.; Khoo, I.C.; Salamo, G.J.; Stegeman, G.I.; Van Stryland, E.W. Nonlinear Refraction and Absorption: Mechanisms and Magnitudes. Adv. Opt. Photonics 2010, 2, 60–200. [Google Scholar] [CrossRef]
- You, J.W.; Bongu, S.R.; Bao, Q.; Panoiu, N.C. Nonlinear Optical Properties and Applications of 2D Materials: Theoretical and Experimental Aspects. Nanophotonics 2018, 8, 63–97. [Google Scholar] [CrossRef]
- Xing, G.; Guo, H.; Zhang, X.; Sum, T.C.; Huan, C.H.A. The Physics of Ultrafast Saturable Absorption in Graphene. Opt. Express 2010, 18, 4564–4573. [Google Scholar] [CrossRef] [PubMed]
- Newson, R.W.; Dean, J.; Schmidt, B.; van Driel, H.M. Ultrafast Carrier Kinetics in Exfoliated Graphene and Thin Graphite Films. Opt. Express 2009, 17, 2326–2333. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cinquanta, E.; Fratesi, G.; dal Conte, S.; Grazianetti, C.; Scotognella, F.; Stagira, S.; Vozzi, C.; Onida, G.; Molle, A. Ultrafast Carrier Dynamics of Epitaxial Silicene. In Proceedings of the SPIE 10102, Ultrafast Phenomena and Nanophotonics XXI, San Francisco, CA, USA, 30 January–2 February 2017; p. 101020J. [Google Scholar]
- Papadakis, I.; Bouza, Z.; Couris, S.; Mouselimis, V.; Bourlinos, A.B. Dramatic Enhancement of the Nonlinear Optical Response of Hydrogenated Fluorographene: The Effect of Midgap States. J. Phys. Chem. C 2018, 122, 25573–25579. [Google Scholar] [CrossRef]
- Liaros, N.; Aloukos, P.; Kolokithas-Ntoukas, A.; Bakandritsos, A.; Szabo, T.; Zboril, R.; Couris, S. Nonlinear Optical Properties and Broadband Optical Power Limiting Action of Graphene Oxide Colloids. J. Phys. Chem. C 2013, 117, 6842–6850. [Google Scholar] [CrossRef]
- Stathis, A.; Papadakis, I.; Karampitsos, N.; Couris, S.; Potsi, G.; Bourlinos, A.B.; Otyepka, M.; Zboril, R. Thiophenol-Modified Fluorographene Derivatives for Nonlinear Optical Applications. ChemPlusChem 2019, 84, 1288–1298. [Google Scholar] [CrossRef] [PubMed]
- Pfanner, G.; Freysoldt, C.; Neugebauer, J.; Inam, F.; Drabold, D.; Jarolimek, K.; Zeman, M. Dangling-Bond Defect in a-Si:H: Characterization of Network and Strain Effects by First-Principles Calculation of the EPR Parameters. Phys. Rev. B 2013, 87, 125308. [Google Scholar] [CrossRef] [Green Version]
- Kamiyama, E.; Sueoka, K. Effect of Dangling Bonds of Ultra-Thin Silicon Film Surface on Electronic States of Internal Atoms. Appl. Surf. Sci. 2012, 258, 5265–5269. [Google Scholar] [CrossRef]
- John, S.; Soukoulis, C.; Cohen, M.H.; Economou, E.N. Theory of Electron Band Tails and the Urbach Optical-Absorption Edge. Phys. Rev. Lett. 1986, 57, 1777–1780. [Google Scholar] [CrossRef] [PubMed]
- Sieval, A.B.; Opitz, R.; Maas, H.P.A.; Schoeman, M.G.; Meijer, G.; Vergeldt, F.J.; Zuilhof, H.; Sudhölter, E.J.R. Monolayers of 1-Alkynes on the H-Terminated Si(100) Surface. Langmuir 2000, 16, 10359–10368. [Google Scholar] [CrossRef]
- Ikeda, K.; Shen, Y.; Fainman, Y. Enhanced Optical Nonlinearity in Amorphous Silicon and its Application to Waveguide Devices. Opt. Express 2007, 15, 17761. [Google Scholar] [CrossRef]
- Wathen, J.J.; Pagán, V.R.; Suess, R.J.; Wang, K.Y.; Foster, A.C.; Murphy, T.E. Non-instantaneous Optical Nonlinearity of an a-Si:H Nanowire Waveguide. Opt. Express 2014, 22, 22730–27742. [Google Scholar] [CrossRef] [Green Version]
- Miller, A.; Welford, K.; Daino, B. Nonlinear Optical Materials and Devices for Applications in Information Technology; Springer: Dordrecht, The Netherlands, 1995. [Google Scholar]


| λ (nm) | Sample | β (×10−11 m/W) | γ’ (×10−18 m2/W) | |χ|(3) (×10−13 esu) |
|---|---|---|---|---|
| 532 | SiNS–H in toluene | - | 1990 ± 360 | 2820 ± 520 |
| SiNS–dodecene in toluene | −1243 ± 140 | 4014 ± 540 | 5724 ± 764 | |
| G in DMF a | 1240 ± 149 | 1440 ± 173 | 2000 ± 313 | |
| GO in H2O b | 43.5 ± 4.0 | −81 ± 8 | 93 ± 9 | |
| N–GO in DMF c | 453 ± 70 | 235 ± 32 | 388 ± 52 | |
| B–GO in DMF c | 524 ± 52 | 304 ± 32 | 481 ± 48 | |
| CF in DMF d | 601 ± 72 | −1265 ± 177 | 1769 ± 230 | |
| 1064 | SiNS–H in toluene | - | 297 ± 40 | 423 ± 56 |
| SiNS–dodecene in toluene | −110 ± 21 | 425 ± 80 | 621 ± 127 | |
| G in DMF a | 526 ± 65 | 401 ± 54 | 778 ± 96 | |
| GO in H2O b | - | - | - | |
| N-GO in DMF c | 331 ± 40 | 229 ± 38 | 344 ± 54 | |
| B-GO in DMF c | 429 ± 35 | 254 ± 38 | 398 ± 51 | |
| CF in DMF d | 100 ± 17 | −193 ± 28 | 270 ± 39 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stavrou, M.; Stathis, A.; Papadakis, I.; Lyuleeva-Husemann, A.; Koudoumas, E.; Couris, S. Silicon Nanosheets: An Emerging 2D Photonic Material with a Large Transient Nonlinear Optical Response beyond Graphene. Nanomaterials 2022, 12, 90. https://doi.org/10.3390/nano12010090
Stavrou M, Stathis A, Papadakis I, Lyuleeva-Husemann A, Koudoumas E, Couris S. Silicon Nanosheets: An Emerging 2D Photonic Material with a Large Transient Nonlinear Optical Response beyond Graphene. Nanomaterials. 2022; 12(1):90. https://doi.org/10.3390/nano12010090
Chicago/Turabian StyleStavrou, Michalis, Aristeidis Stathis, Ioannis Papadakis, Alina Lyuleeva-Husemann, Emmanouel Koudoumas, and Stelios Couris. 2022. "Silicon Nanosheets: An Emerging 2D Photonic Material with a Large Transient Nonlinear Optical Response beyond Graphene" Nanomaterials 12, no. 1: 90. https://doi.org/10.3390/nano12010090
APA StyleStavrou, M., Stathis, A., Papadakis, I., Lyuleeva-Husemann, A., Koudoumas, E., & Couris, S. (2022). Silicon Nanosheets: An Emerging 2D Photonic Material with a Large Transient Nonlinear Optical Response beyond Graphene. Nanomaterials, 12(1), 90. https://doi.org/10.3390/nano12010090








