Nanoparticles to Enhance Melting Performance of Phase Change Materials for Thermal Energy Storage
Abstract
:1. Introduction
- (a)
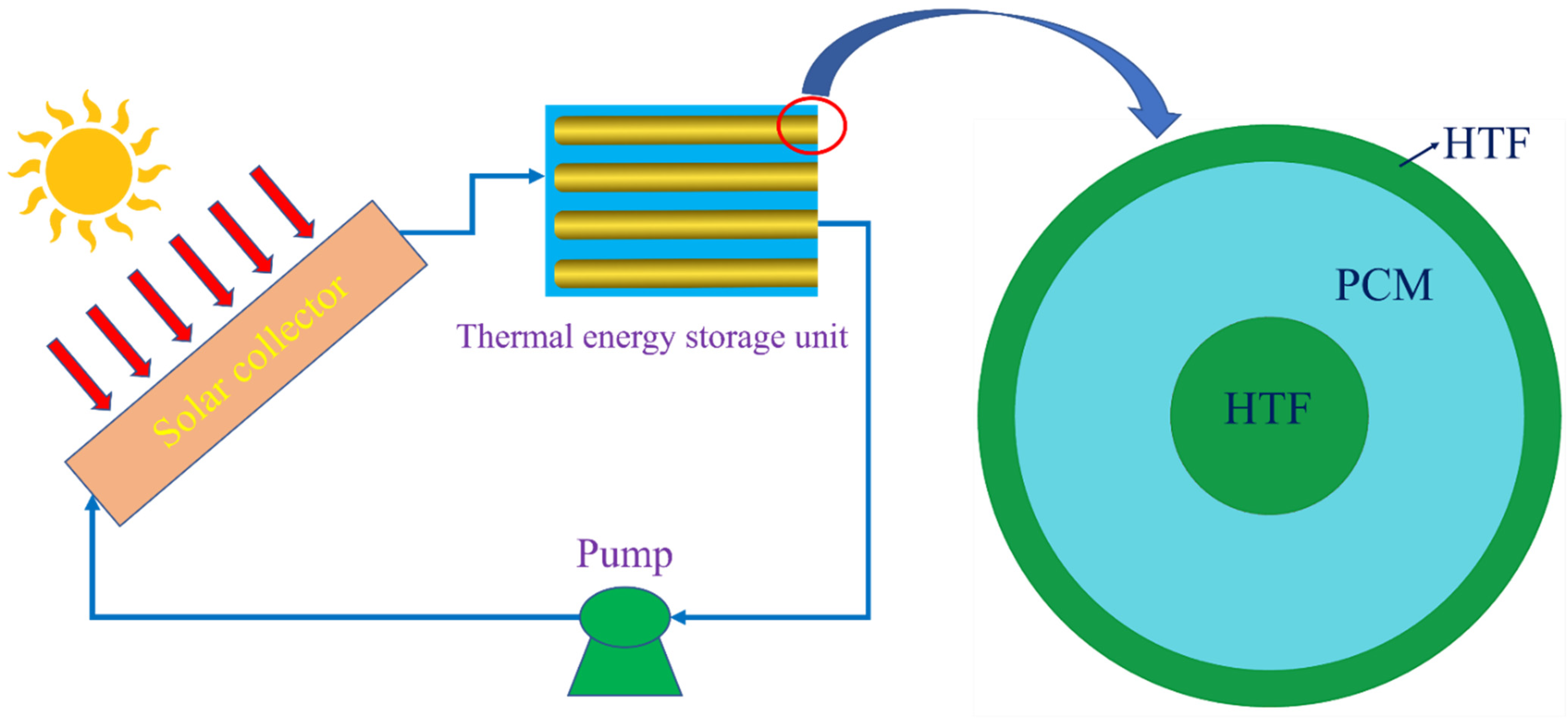
- We propose to integrate PCM into a solar-energy-based heating and cooling system as a thermal energy storage unit, which is expected to ensure the stability and flexibility of the system and mitigate the carbon emissions from the built environment, helping to achieve a net-zero society. The melting process of PCM in a tubular unit is described in detail, considering natural convection due to the buoyancy effect.
- (b)
- The performance of nanoparticles during the melting process of phase change materials is analysed in a tubular energy storage unit. We use an experimentally validated formula for the thermal conductivity of nano-PCM. Factors considered in this study include those usually neglected in previous studies, such as the effects of the Brownian motion of nanoparticles, size, volume fraction and temperature dependence [27,28,29].
- (c)
- We determine the mechanism of performance enhancement of the melting process of PCM using nanoparticles with various thermal conductivities, based on the analysis of Al2O3-, copper- and graphene-based nanofluids.
2. Problem Statements
2.1. Phase Change Material Thermal Energy Storage System
2.2. Governing Equations
2.3. Thermophysical Properties
2.4. Numerical Implementations
3. Results and Discussion
3.1. Model Validation and Verification
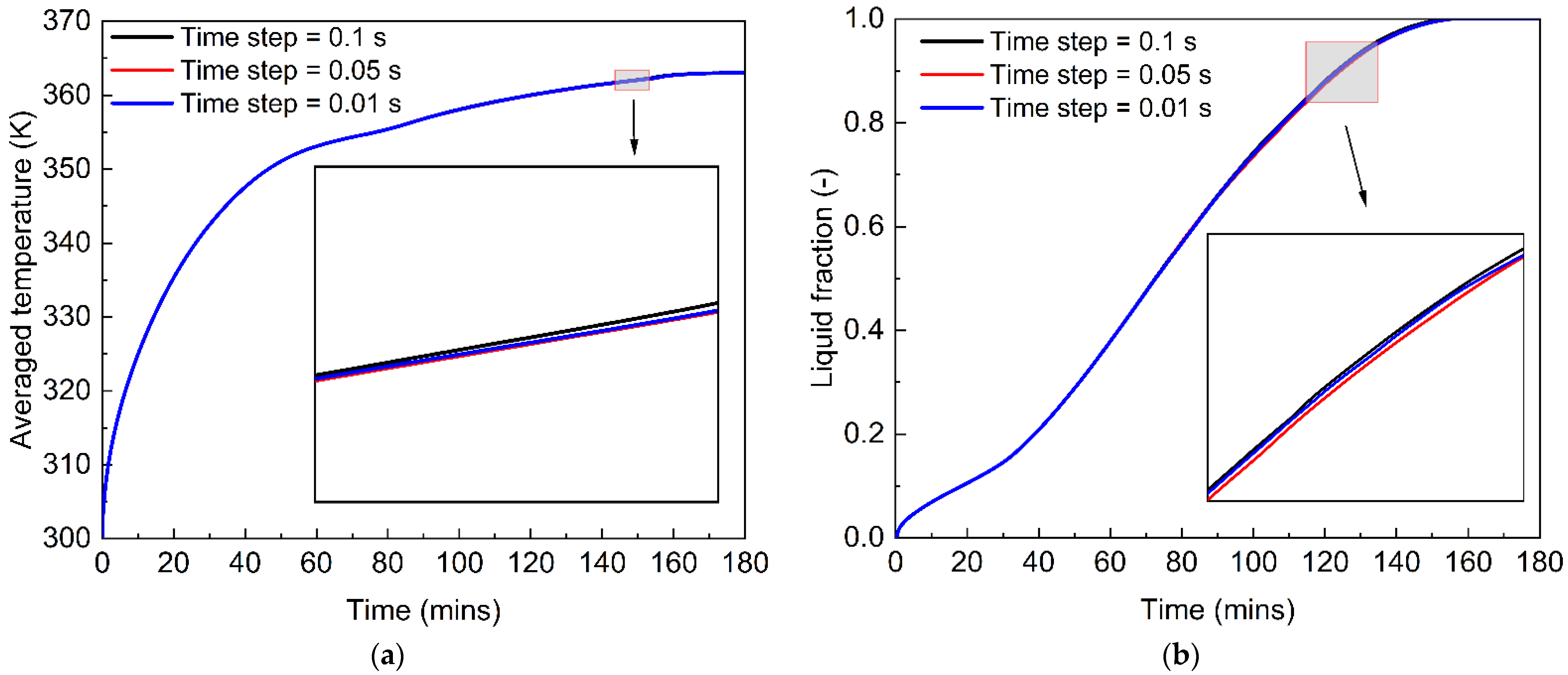
3.1.1. Model Sensitivity of Grid Density and Time Step
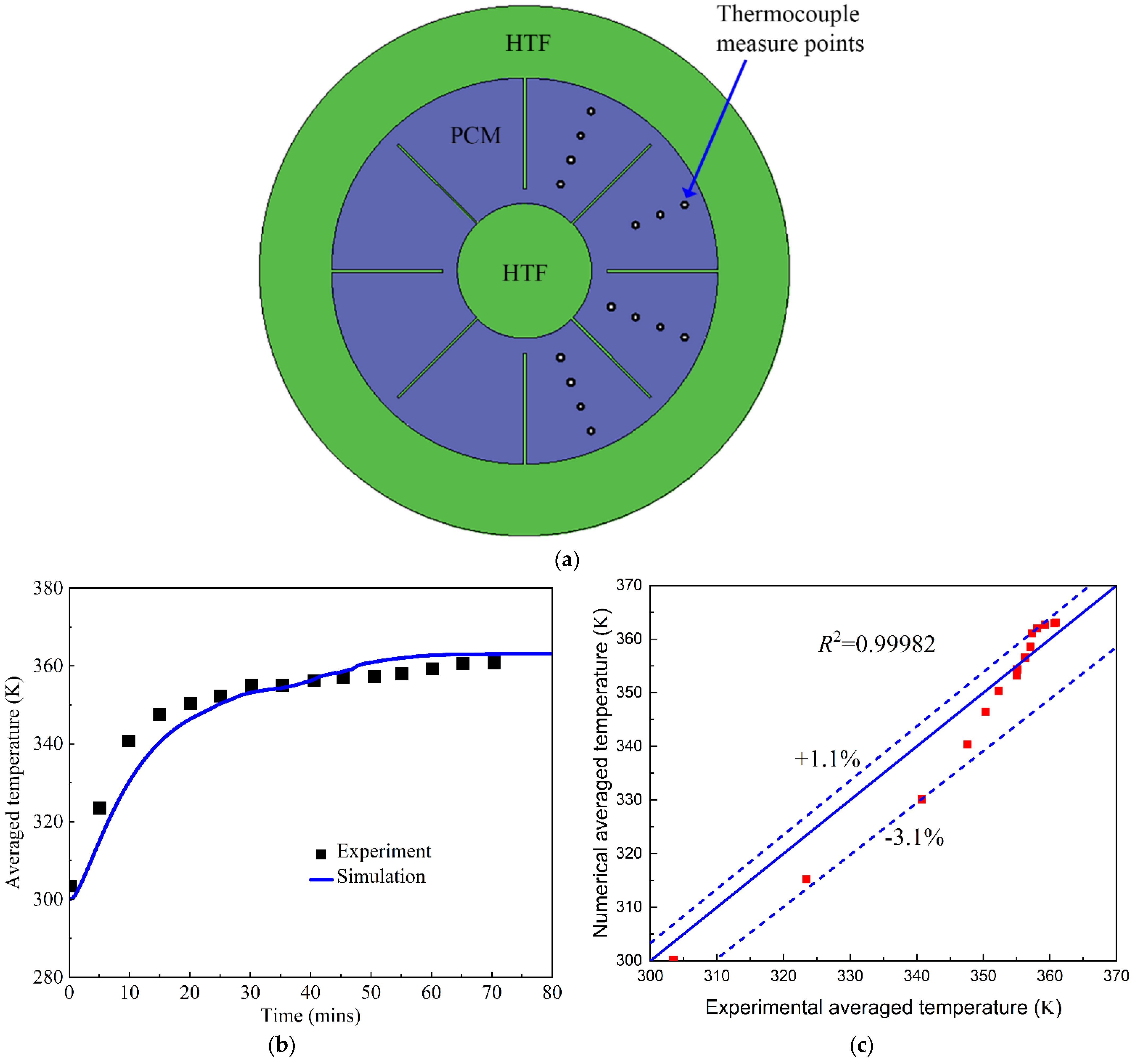
3.1.2. Model Validation against Experimental Data
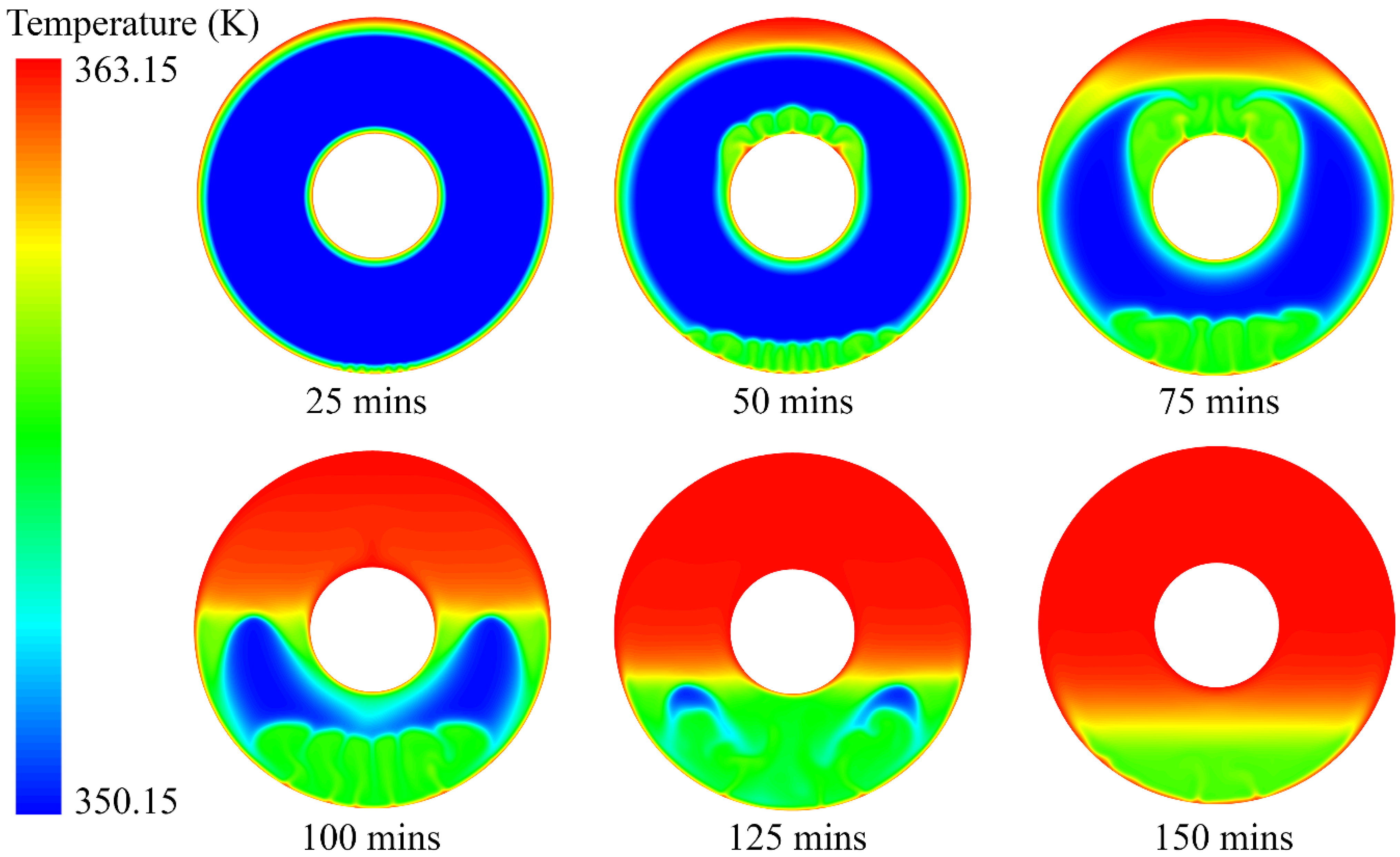
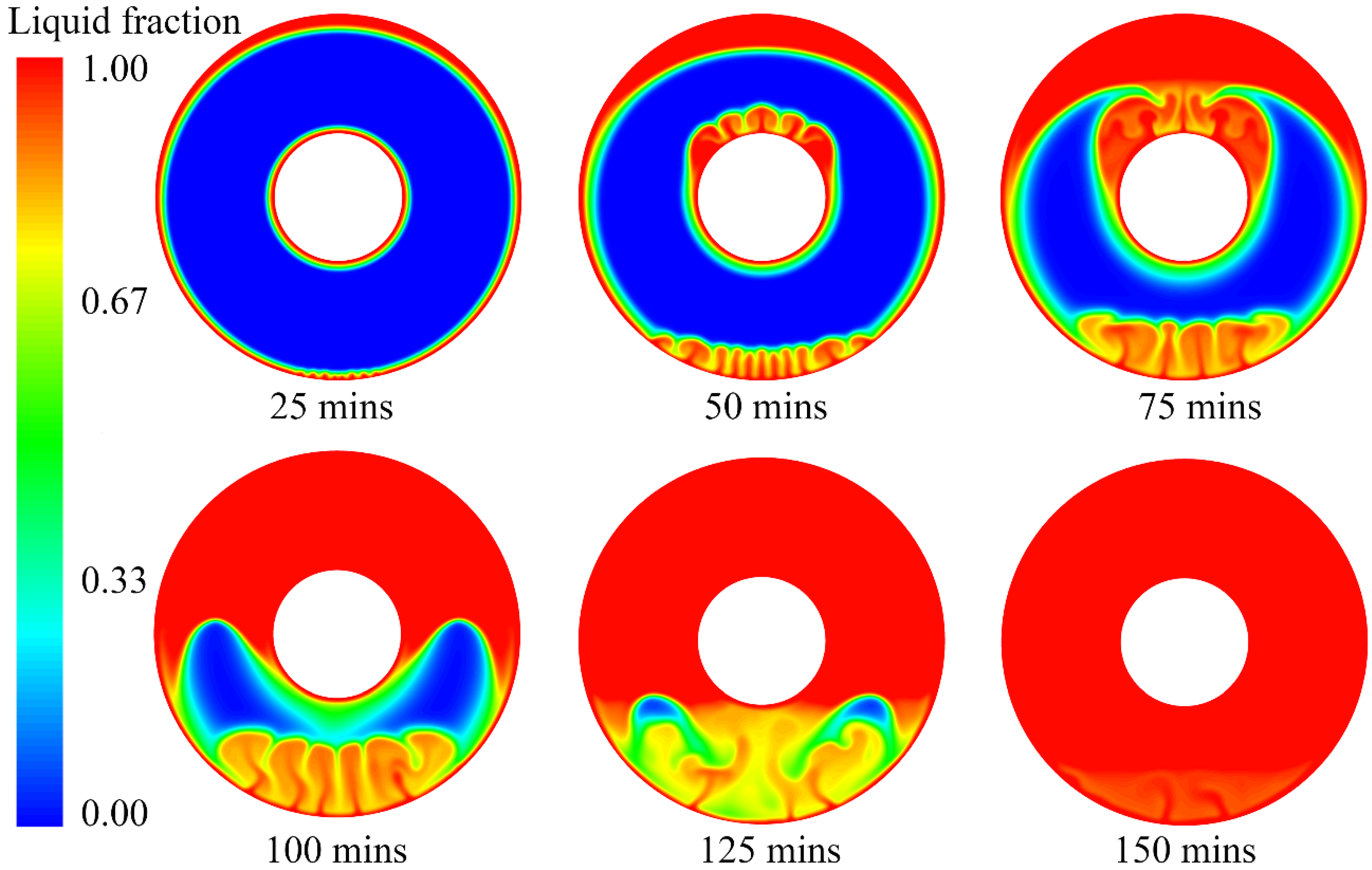
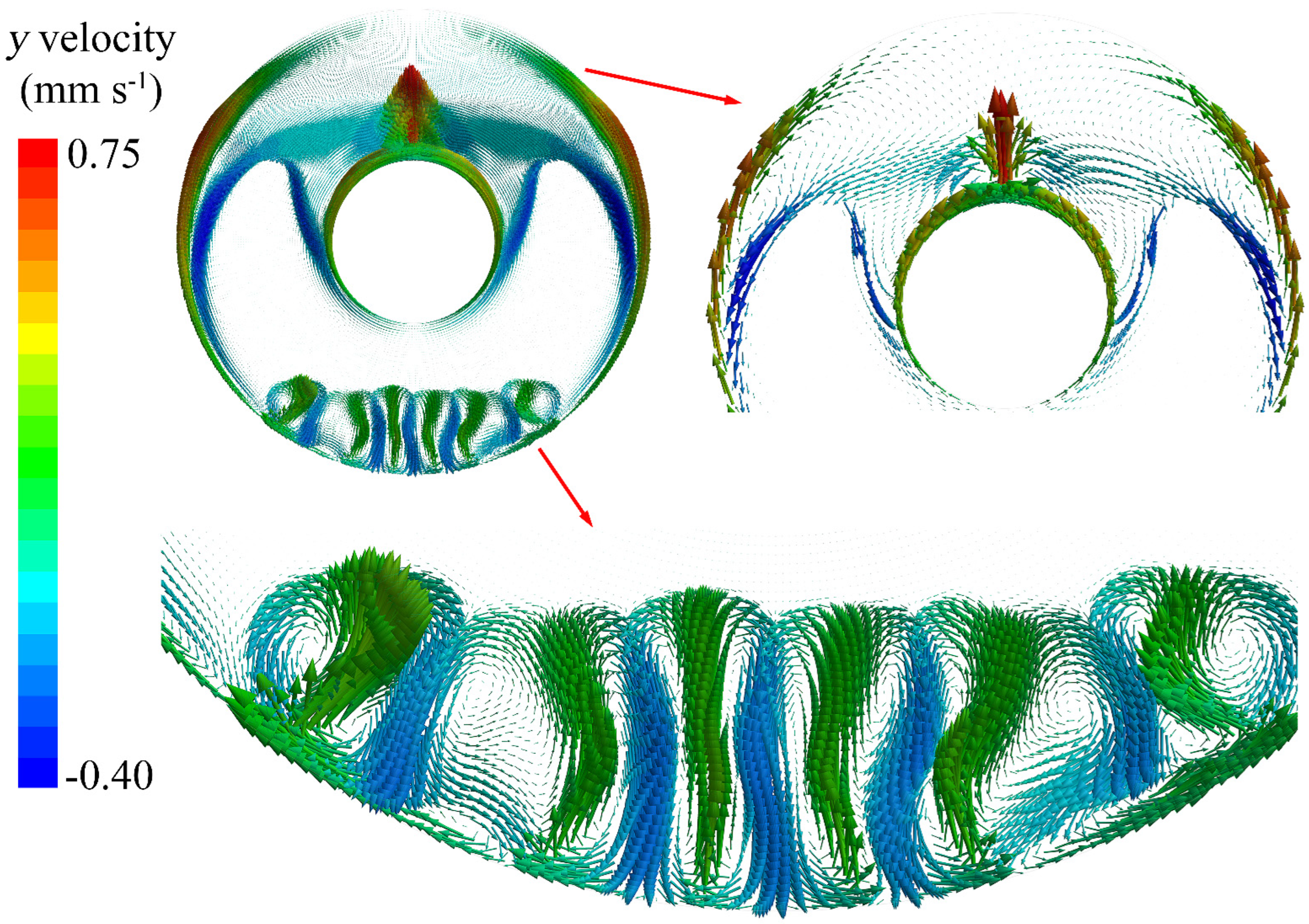
3.2. Melting Process and Interface Evolution of PCM
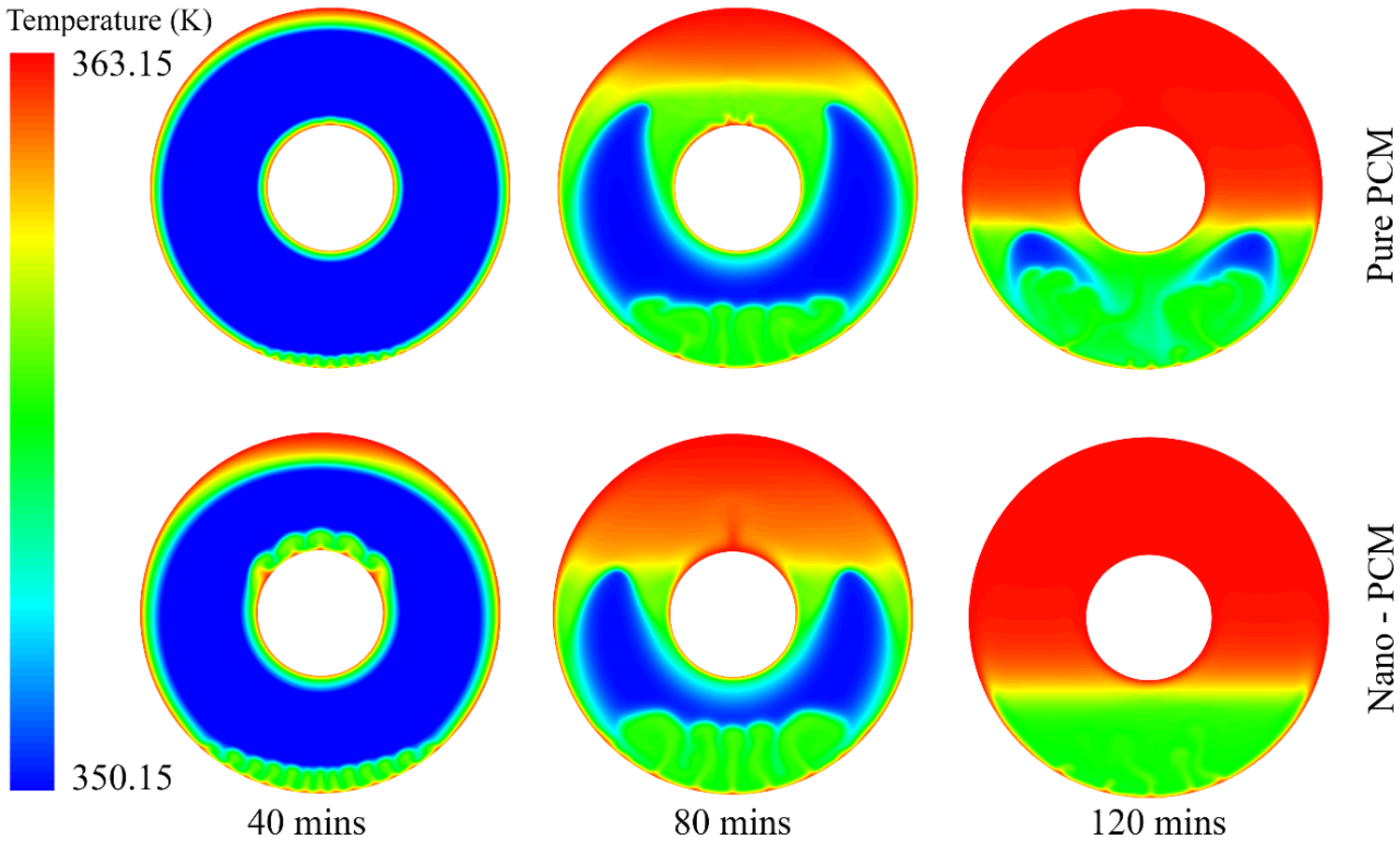
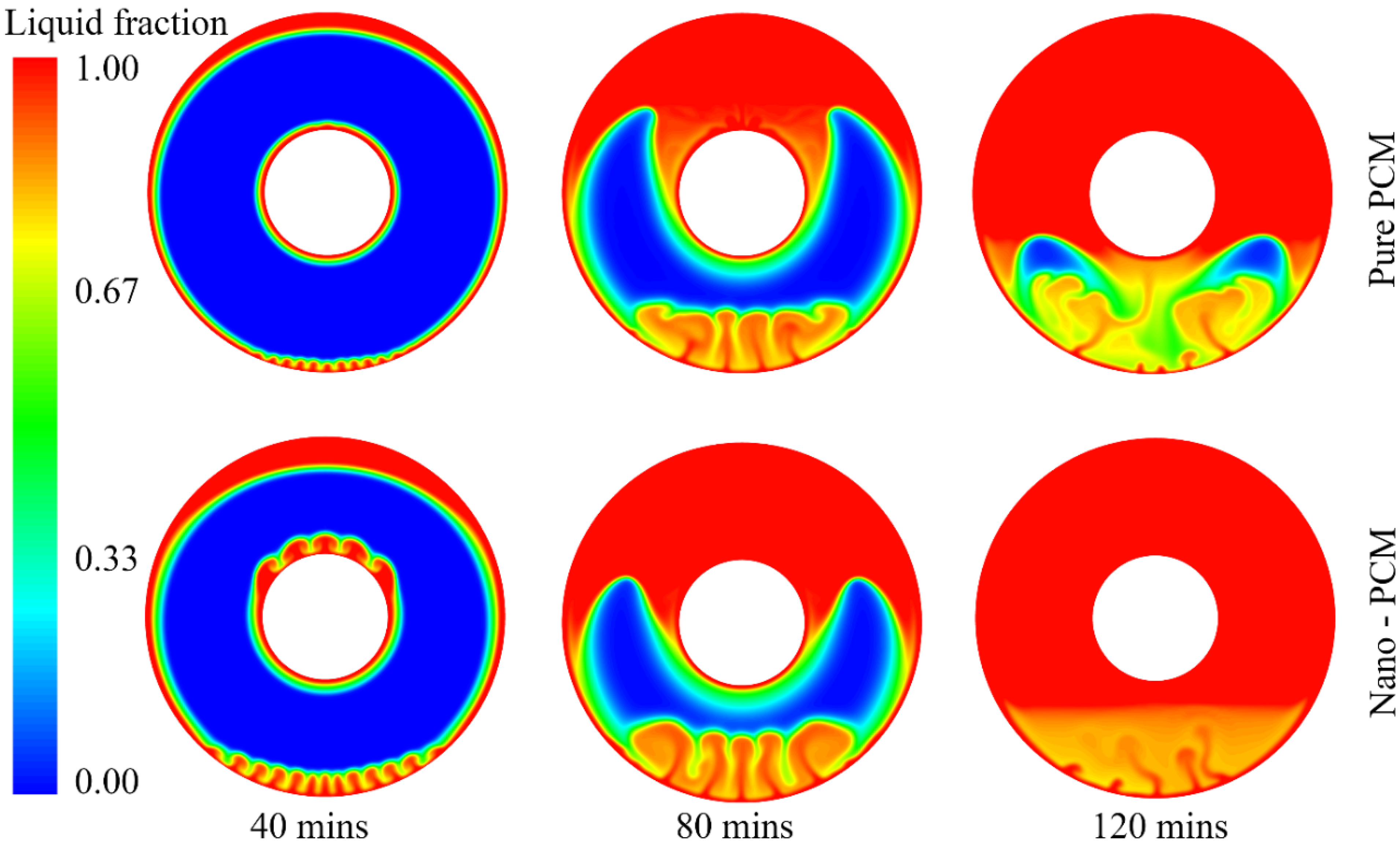
3.3. Role of Nanoparticles in the PCM Melting Processes
3.4. Effect of Nanoparticle Material on PCM Melting Performance
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Junaid, M.F.; ur Rehman, Z.; Čekon, M.; Čurpek, J.; Farooq, R.; Cui, H.; Khan, I. Inorganic phase change materials in thermal energy storage: A review on perspectives and technological advances in building applications. Energy Build. 2021, 252, 111443. [Google Scholar] [CrossRef]
- Tyagi, V.; Chopra, K.; Kalidasan, B.; Chauhan, A.; Stritih, U.; Anand, S.; Pandey, A.; Sarı, A.; Kothari, R. Phase change material based advance solar thermal energy storage systems for building heating and cooling applications: A prospective research approach. Sustain. Energy Technol. Assess. 2021, 47, 101318. [Google Scholar] [CrossRef]
- Tan, P.; Lindberg, P.; Eichler, K.; Löveryd, P.; Johansson, P.; Kalagasidis, A.S. Thermal energy storage using phase change materials: Techno-economic evaluation of a cold storage installation in an office building. Appl. Energy 2020, 276, 115433. [Google Scholar] [CrossRef]
- Du, K.; Calautit, J.; Eames, P.; Wu, Y. A state-of-the-art review of the application of phase change materials (PCM) in Mobilized-Thermal Energy Storage (M-TES) for recovering low-temperature industrial waste heat (IWH) for distributed heat supply. Renew. Energy 2021, 168, 1040–1057. [Google Scholar] [CrossRef]
- Zahir, M.; Rahman, M.M.; Basamad, S.K.; Mohaisen, K.O.; Irshad, K.; Rahman, M.M.; Aziz, M.; Ali, A.; Hossain, M.M. Preparation of a Sustainable Shape-Stabilized Phase Change Material for Thermal Energy Storage Based on Mg2+-Doped CaCO3/PEG Composites. Nanomaterials 2021, 11, 1639. [Google Scholar] [CrossRef]
- Bouzennada, T.; Mechighel, F.; Ghachem, K.; Kolsi, L. Numerical Simulation of the Impact of the Heat Source Position on Melting of a Nano-Enhanced Phase Change Material. Nanomaterials 2021, 11, 1425. [Google Scholar] [CrossRef]
- Choudhari, V.; Dhoble, A.; Panchal, S. Numerical analysis of different fin structures in phase change material module for battery thermal management system and its optimization. Int. J. Heat Mass Transf. 2020, 163, 120434. [Google Scholar] [CrossRef]
- Desai, A.N.; Gunjal, A.; Singh, V. Numerical investigations of fin efficacy for phase change material (PCM) based thermal control module. Int. J. Heat Mass Transf. 2020, 147, 118855. [Google Scholar] [CrossRef]
- Mostafavi, A.; Parhizi, M.; Jain, A. Theoretical modeling and optimization of fin-based enhancement of heat transfer into a phase change material. Int. J. Heat Mass Transf. 2019, 145, 118698. [Google Scholar] [CrossRef]
- Ali, H.M. Analysis of heat pipe-aided graphene-oxide based nanoparticle-enhanced phase change material heat sink for passive cooling of electronic components. J. Therm. Anal. Calorim. 2021, 146, 277–286. [Google Scholar] [CrossRef]
- Ren, Q. Enhancement of nanoparticle-phase change material melting performance using a sinusoidal heat pipe. Energy Convers. Manag. 2019, 180, 784–795. [Google Scholar] [CrossRef]
- Ghalambaz, M.; Zhang, J. Conjugate solid-liquid phase change heat transfer in heatsink filled with phase change material-metal foam. Int. J. Heat Mass Transf. 2020, 146, 118832. [Google Scholar] [CrossRef]
- Xu, Y.; Li, M.-J.; Zheng, Z.-J.; Xue, X.-D. Melting performance enhancement of phase change material by a limited amount of metal foam: Configurational optimization and economic assessment. Appl. Energy 2018, 212, 868–880. [Google Scholar] [CrossRef]
- Nie, C.; Liu, J.; Deng, S. Effect of geometry modification on the thermal response of composite metal foam/phase change material for thermal energy storage. Int. J. Heat Mass Transf. 2021, 165, 120652. [Google Scholar] [CrossRef]
- Kamkari, B.; Shokouhmand, H. Experimental investigation of phase change material melting in rectangular enclosures with horizontal partial fins. Int. J. Heat Mass Transf. 2014, 78, 839–851. [Google Scholar] [CrossRef]
- Sciacovelli, A.; Gagliardi, F.; Verda, V. Maximization of performance of a PCM latent heat storage system with innovative fins. Appl. Energy 2015, 137, 707–715. [Google Scholar] [CrossRef]
- Gharebaghi, M.; Sezai, I. Enhancement of heat transfer in latent heat storage modules with internal fins. Numer. Heat Transf. Part A Appl. 2007, 53, 749–765. [Google Scholar] [CrossRef]
- Rathod, M.K.; Banerjee, J. Thermal performance enhancement of shell and tube Latent Heat Storage Unit using longitudinal fins. Appl. Therm. Eng. 2015, 75, 1084–1092. [Google Scholar] [CrossRef]
- Agyenim, F.; Eames, P.; Smyth, M. A comparison of heat transfer enhancement in a medium temperature thermal energy storage heat exchanger using fins. Sol. Energy 2009, 83, 1509–1520. [Google Scholar] [CrossRef]
- Williams, J.D.; Peterson, G. A Review of Thermal Property Enhancements of Low-Temperature Nano-Enhanced Phase Change Materials. Nanomaterials 2021, 11, 2578. [Google Scholar] [CrossRef]
- Cabaleiro, D.; Hamze, S.; Fal, J.; Marcos, M.A.; Estellé, P.; Żyła, G. Thermal and physical characterization of PEG phase change materials enhanced by carbon-based nanoparticles. Nanomaterials 2020, 10, 1168. [Google Scholar] [CrossRef] [PubMed]
- Khodadadi, J.; Hosseinizadeh, S. Nanoparticle-enhanced phase change materials (NEPCM) with great potential for improved thermal energy storage. Int. Commun. Heat Mass Transf. 2007, 34, 534–543. [Google Scholar] [CrossRef]
- Sciacovelli, A.; Colella, F.; Verda, V. Melting of PCM in a thermal energy storage unit: Numerical investigation and effect of nanoparticle enhancement. Int. J. Energy Res. 2013, 37, 1610–1623. [Google Scholar] [CrossRef] [Green Version]
- Lin, S.C.; Al-Kayiem, H.H. Evaluation of copper nanoparticles–Paraffin wax compositions for solar thermal energy storage. Sol. Energy 2016, 132, 267–278. [Google Scholar] [CrossRef]
- Mahdi, J.M.; Nsofor, E.C. Solidification of a PCM with nanoparticles in triplex-tube thermal energy storage system. Appl. Therm. Eng. 2016, 108, 596–604. [Google Scholar] [CrossRef]
- Mahdi, J.M.; Lohrasbi, S.; Ganji, D.D.; Nsofor, E.C. Accelerated melting of PCM in energy storage systems via novel configuration of fins in the triplex-tube heat exchanger. Int. J. Heat Mass Transf. 2018, 124, 663–676. [Google Scholar] [CrossRef]
- Lohrasbi, S.; Sheikholeslami, M.; Ganji, D.D. Multi-objective RSM optimization of fin assisted latent heat thermal energy storage system based on solidification process of phase change Material in presence of copper nanoparticles. Appl. Therm. Eng. 2017, 118, 430–447. [Google Scholar] [CrossRef]
- Ma, X.; Sheikholeslami, M.; Jafaryar, M.; Shafee, A.; Nguyen-Thoi, T.; Li, Z. Solidification inside a clean energy storage unit utilizing phase change material with copper oxide nanoparticles. J. Clean. Prod. 2020, 245, 118888. [Google Scholar] [CrossRef]
- Zarma, I.; Ahmed, M.; Ookawara, S. Enhancing the performance of concentrator photovoltaic systems using Nanoparticle-phase change material heat sinks. Energy Convers. Manag. 2019, 179, 229–242. [Google Scholar] [CrossRef]
- Kant, K.; Shukla, A.; Sharma, A.; Henry Biwole, P. Heat transfer study of phase change materials with graphene nano particle for thermal energy storage. Sol. Energy 2017, 146, 453–463. [Google Scholar] [CrossRef]
- Vajjha, R.S.; Das, D.K. Experimental determination of thermal conductivity of three nanofluids and development of new correlations. Int. J. Heat Mass Transf. 2009, 52, 4675–4682. [Google Scholar] [CrossRef]
- Al-Abidi, A.A.; Mat, S.; Sopian, K.; Sulaiman, M.; Mohammad, A.T. Internal and external fin heat transfer enhancement technique for latent heat thermal energy storage in triplex tube heat exchangers. Appl. Therm. Eng. 2013, 53, 147–156. [Google Scholar] [CrossRef]
- Gürbüz, H.; Buran, D. Experimental study on the effect of concentrated turbulence around the spark plug zone in a swirling flow on a hydrogen SI engine performance and combustion parameters. J. Energy Eng. 2016, 142, 04015031. [Google Scholar] [CrossRef]
- Gürbüz, H. Experimental evaluation of combustion parameters with ion-current sensor integrated to fast response thermocouple in SI engine. J. Energy Eng. 2017, 143, 04016046. [Google Scholar] [CrossRef]
- Solmaz, Ö.; Gürbüz, H.; Karacor, M. Comparison of artificial neural network and fuzzy logic approaches for the prediction of in-cylinder pressure in a spark ignition engine. J. Dyn. Syst. Meas. Control 2020, 142, 091005. [Google Scholar] [CrossRef]
- Nazari, M.A.; Maleki, A.; Assad, M.E.; Rosen, M.A.; Haghighi, A.; Sharabaty, H.; Chen, L. A review of nanomaterial incorporated phase change materials for solar thermal energy storage. Sol. Energy 2021, 228, 725–743. [Google Scholar] [CrossRef]
- Joshi, V.; Rathod, M.K. Constructal enhancement of thermal transport in metal foam-PCM composite-assisted latent heat thermal energy storage system. Numer. Heat Transf. Part A Appl. 2019, 75, 413–433. [Google Scholar] [CrossRef]
- Hernández-Acosta, M.A.; Martines-Arano, H.; Soto-Ruvalcaba, L.; Martínez-González, C.L.; Martínez-Gutiérrez, H.; Torres-Torres, C. Fractional thermal transport and twisted light induced by an optical two-wave mixing in single-wall carbon nanotubes. Int. J. Therm. Sci. 2020, 147, 106136. [Google Scholar] [CrossRef]




| Thermophysical Properties | RT82 |
|---|---|
| Solid density (kg m−3) | 950 |
| Liquid density (kg m−3) | 770 |
| Specific heat (J kg−1 K−1) | 2000 |
| Thermal conductivity (W m−1 K−1) | 0.2 |
| Latent heat (J/kg) | 176,000 |
| Dynamic viscosity (kg/m s) | 0.03499 |
| Solidus temperature (K) | 350.15 |
| Liquidus temperature (K) | 358.15 |
| Thermal expansion coefficient (1/K) | 0.001 |
| Thermophysical Properties | Al2O3 | Copper | Graphene |
|---|---|---|---|
| Solid density (kg m−3) | 3600 | 8920 | 2200 |
| Specific heat (J kg−1 K−1) | 765 | 380 | 790.1 |
| Thermal conductivity (W m−1 K−1) | 36 | 400 | 5000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, Y.; Yang, Y.; Mallick, T.; Wen, C. Nanoparticles to Enhance Melting Performance of Phase Change Materials for Thermal Energy Storage. Nanomaterials 2022, 12, 1864. https://doi.org/10.3390/nano12111864
Han Y, Yang Y, Mallick T, Wen C. Nanoparticles to Enhance Melting Performance of Phase Change Materials for Thermal Energy Storage. Nanomaterials. 2022; 12(11):1864. https://doi.org/10.3390/nano12111864
Chicago/Turabian StyleHan, Yu, Yan Yang, Tapas Mallick, and Chuang Wen. 2022. "Nanoparticles to Enhance Melting Performance of Phase Change Materials for Thermal Energy Storage" Nanomaterials 12, no. 11: 1864. https://doi.org/10.3390/nano12111864
APA StyleHan, Y., Yang, Y., Mallick, T., & Wen, C. (2022). Nanoparticles to Enhance Melting Performance of Phase Change Materials for Thermal Energy Storage. Nanomaterials, 12(11), 1864. https://doi.org/10.3390/nano12111864








