Improving the Insulating Capacity of Polyurethane Foams through Polyurethane Aerogel Inclusion: From Insulation to Superinsulation
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Synthesis of Polyurethane Aerogels and PUF–PUA Composites
2.3. Characterization Techniques
2.3.1. Density, Shrinkage, and Aerogel Mass
2.3.2. Nitrogen Adsorption–Desorption
2.3.3. Scanning Electron Microscopy (SEM)
2.3.4. Mechanical Properties
2.3.5. Thermal Conductivity
3. Results and Discussion
3.1. Polyurethane Foams and Polyurethane Aerogel Characterization
3.2. PUF–PUA Composites Characterization
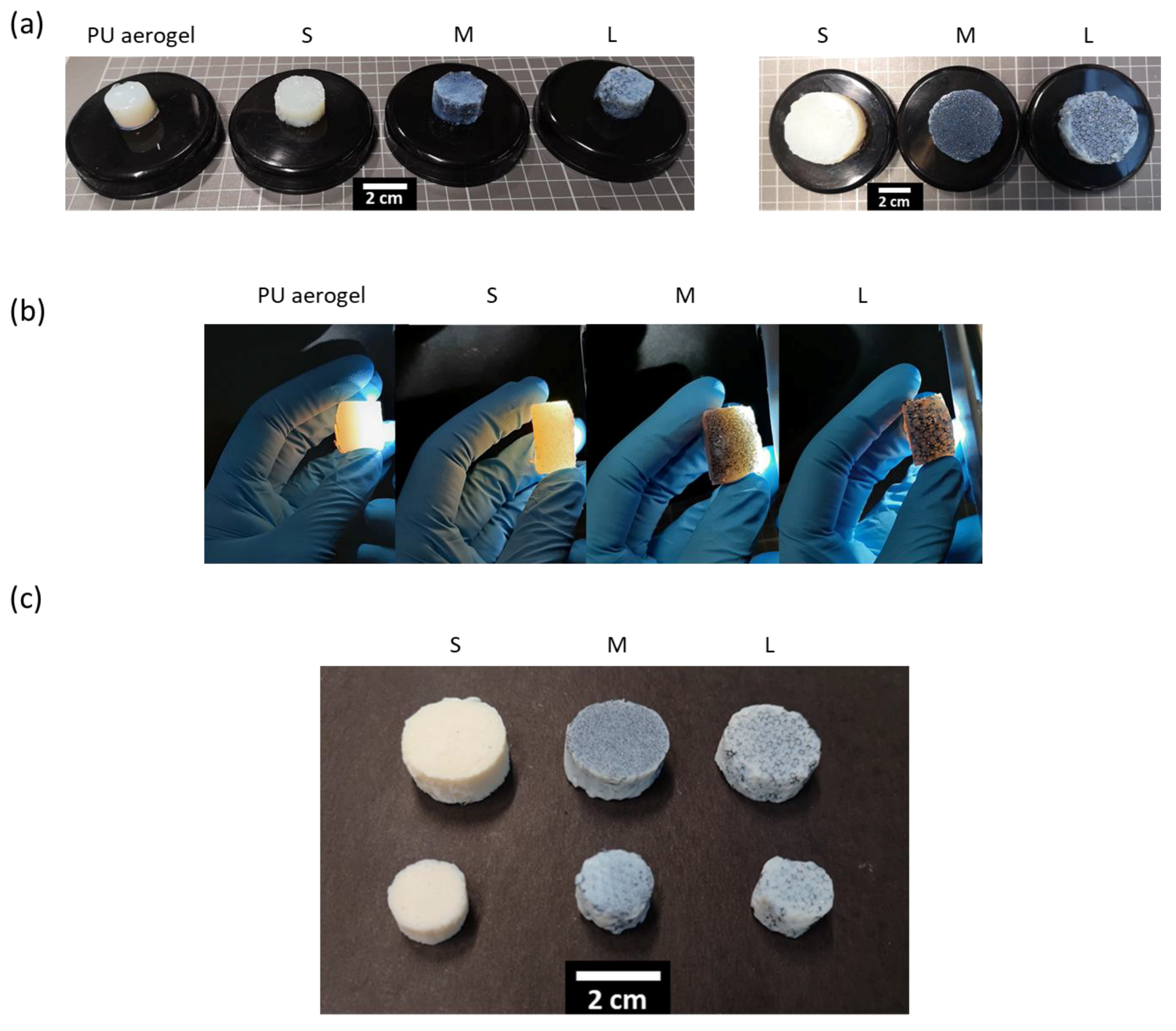
3.2.1. Density and Aerogel Mass
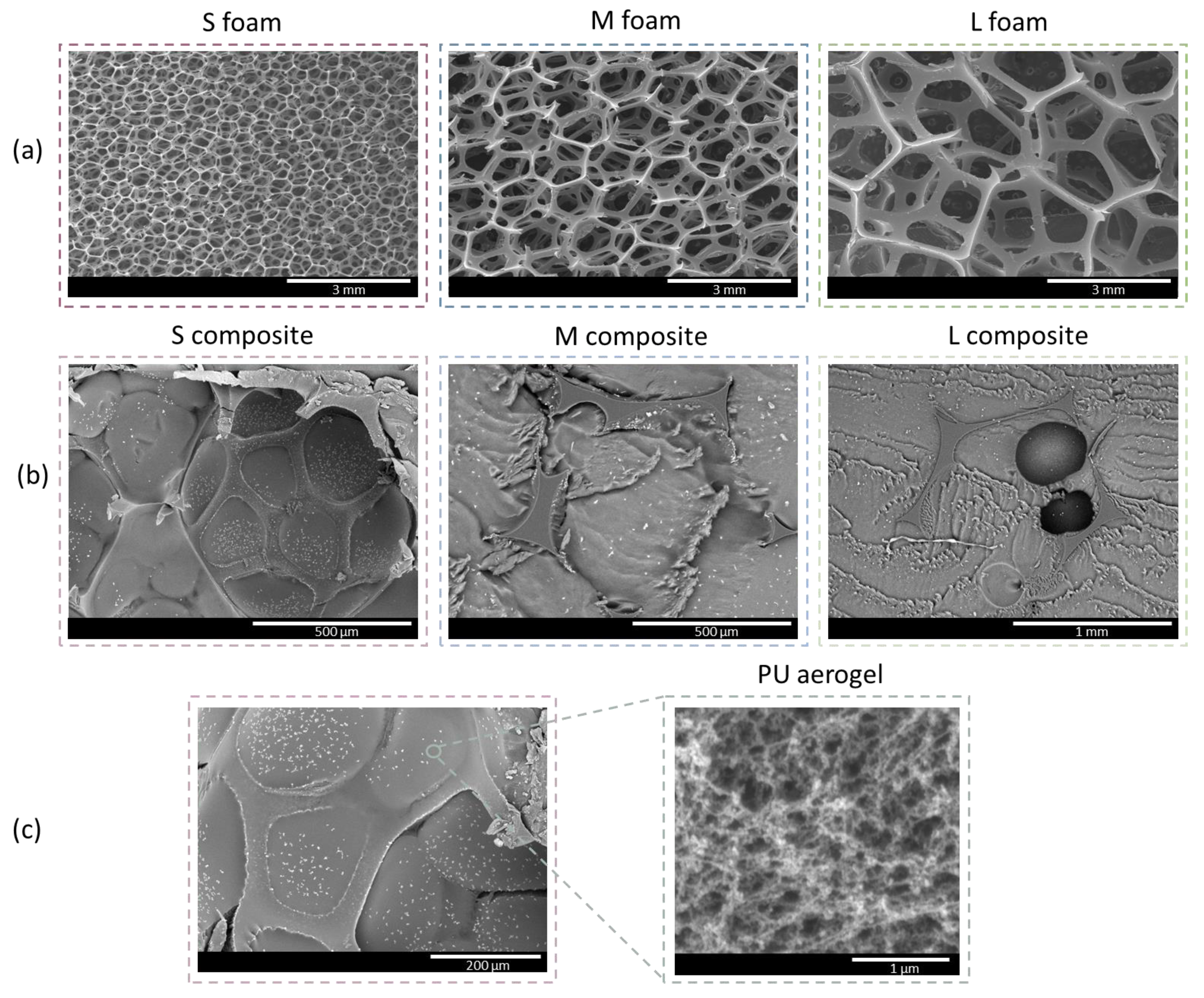
3.2.2. Porous Structure
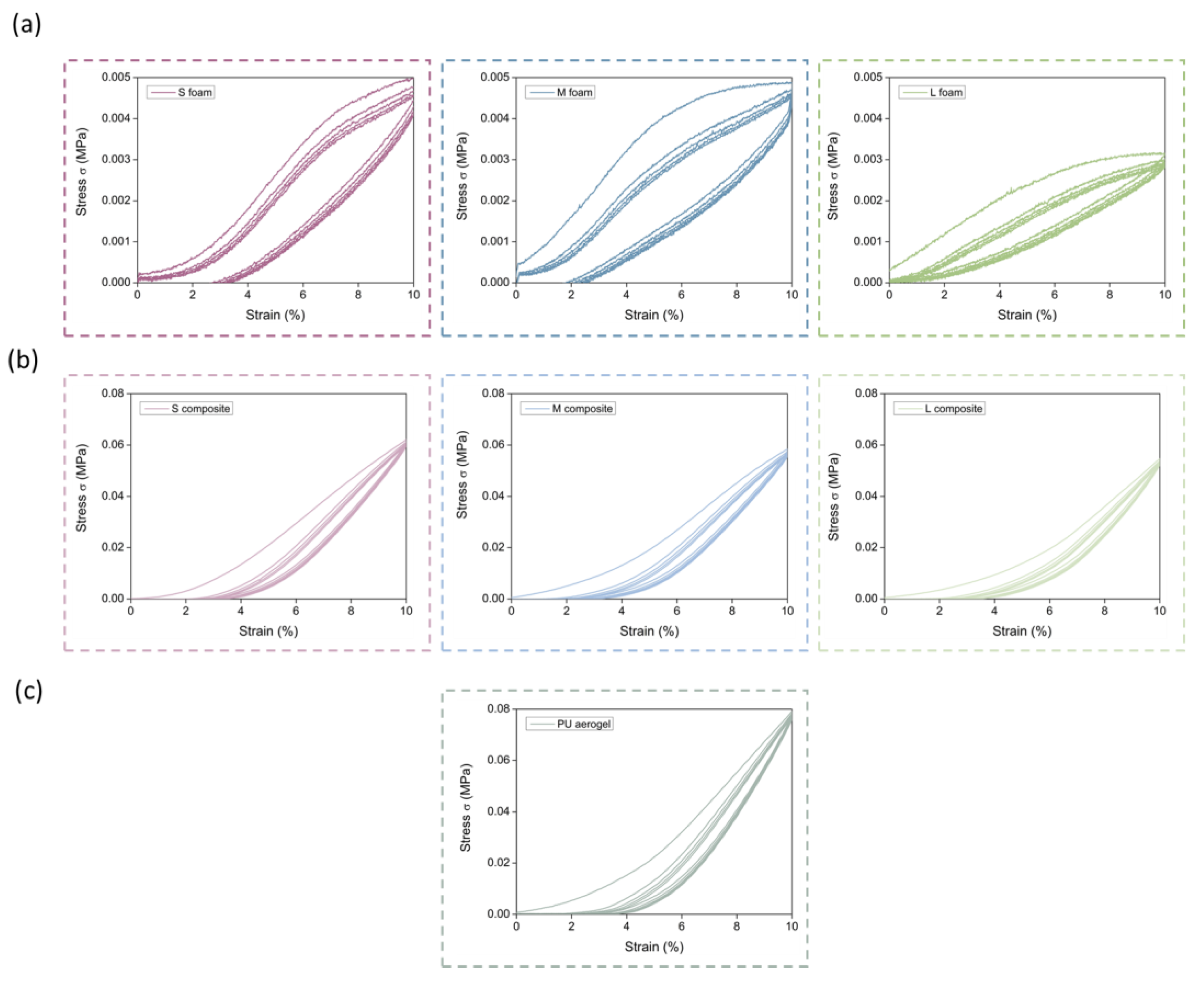
3.2.3. Mechanical Properties
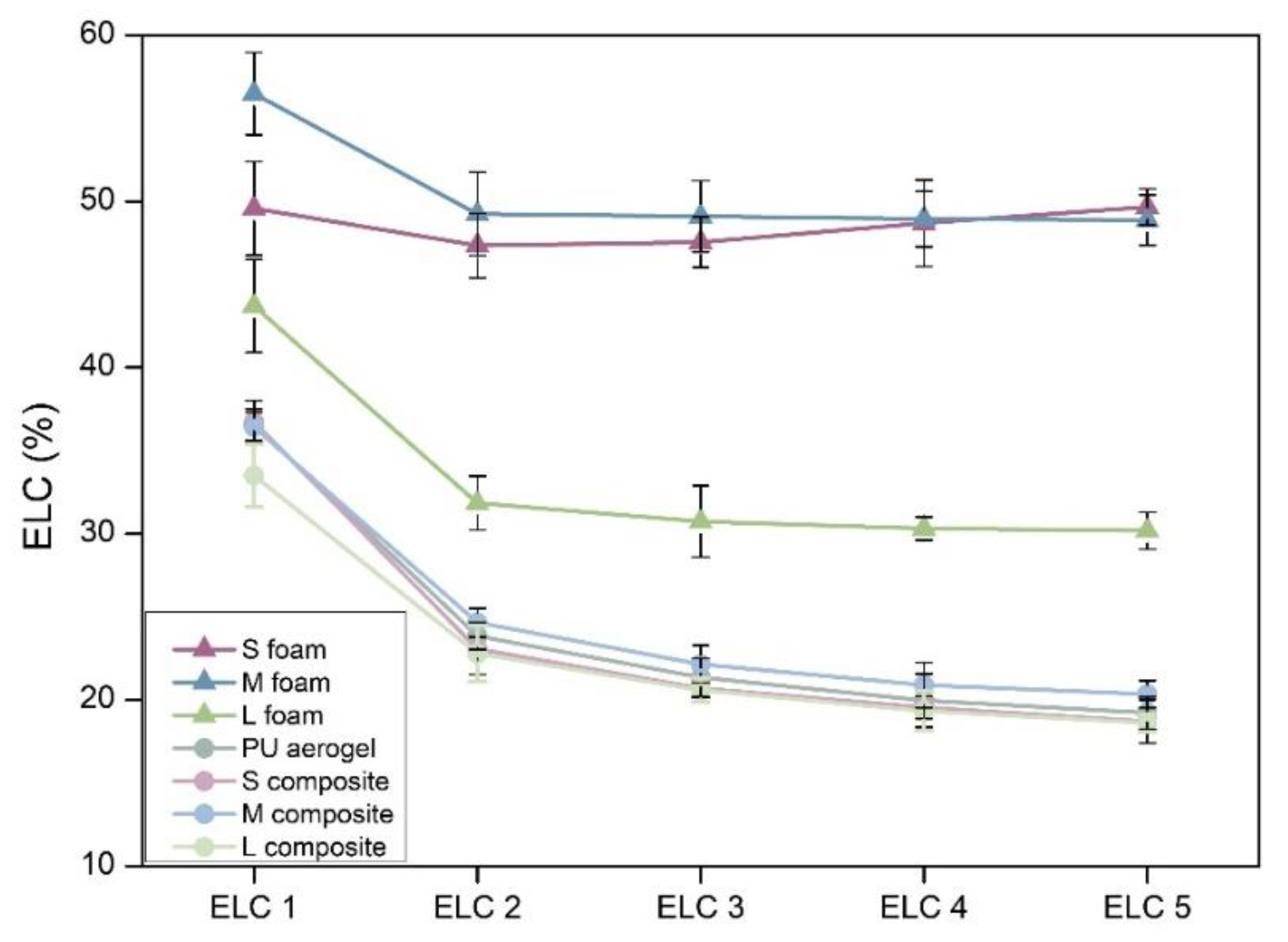
Elasticity
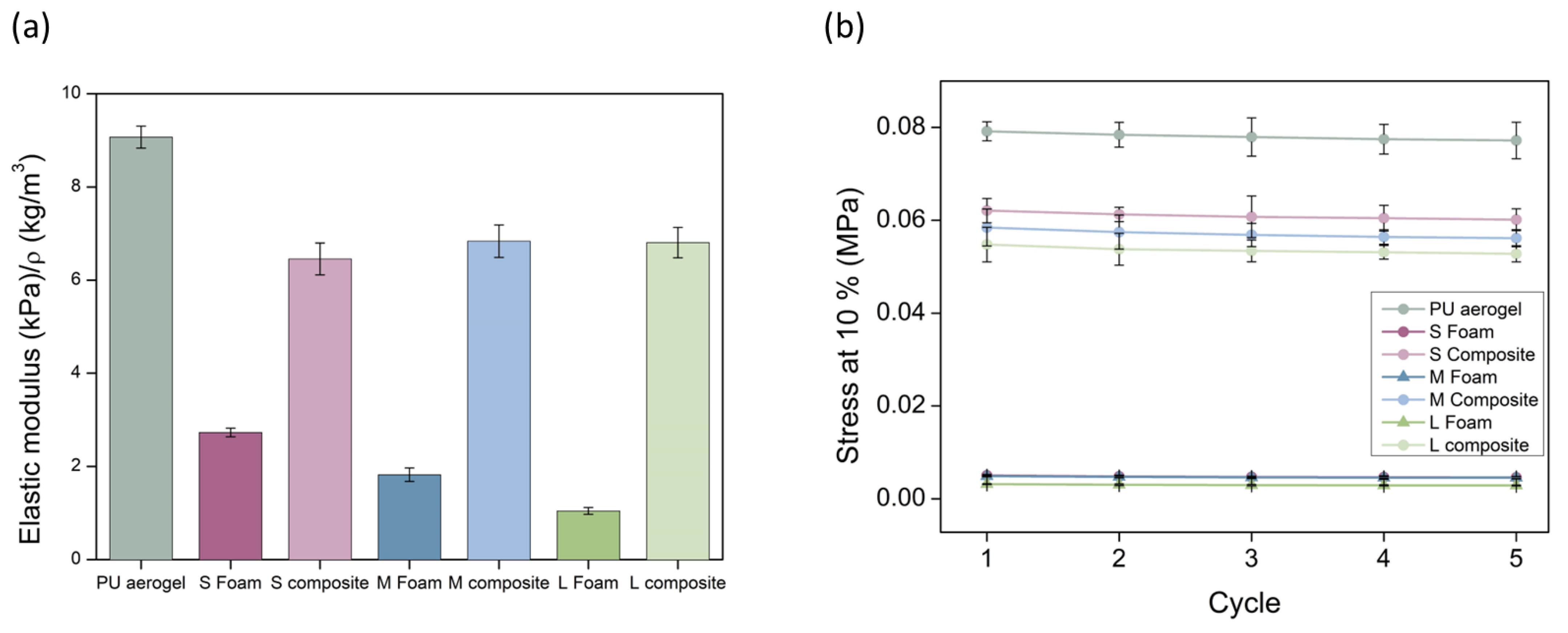
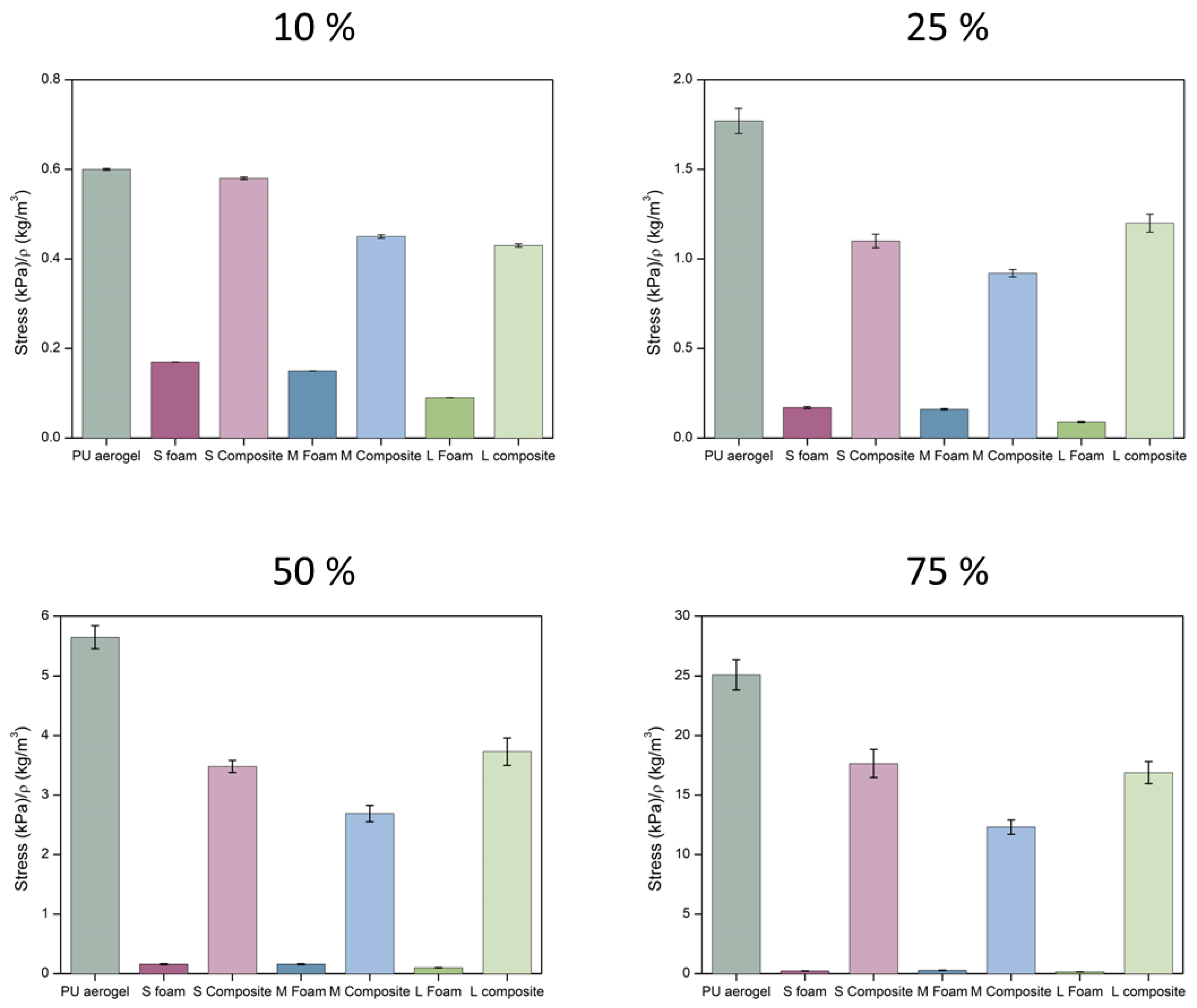
Stiffness and Strength
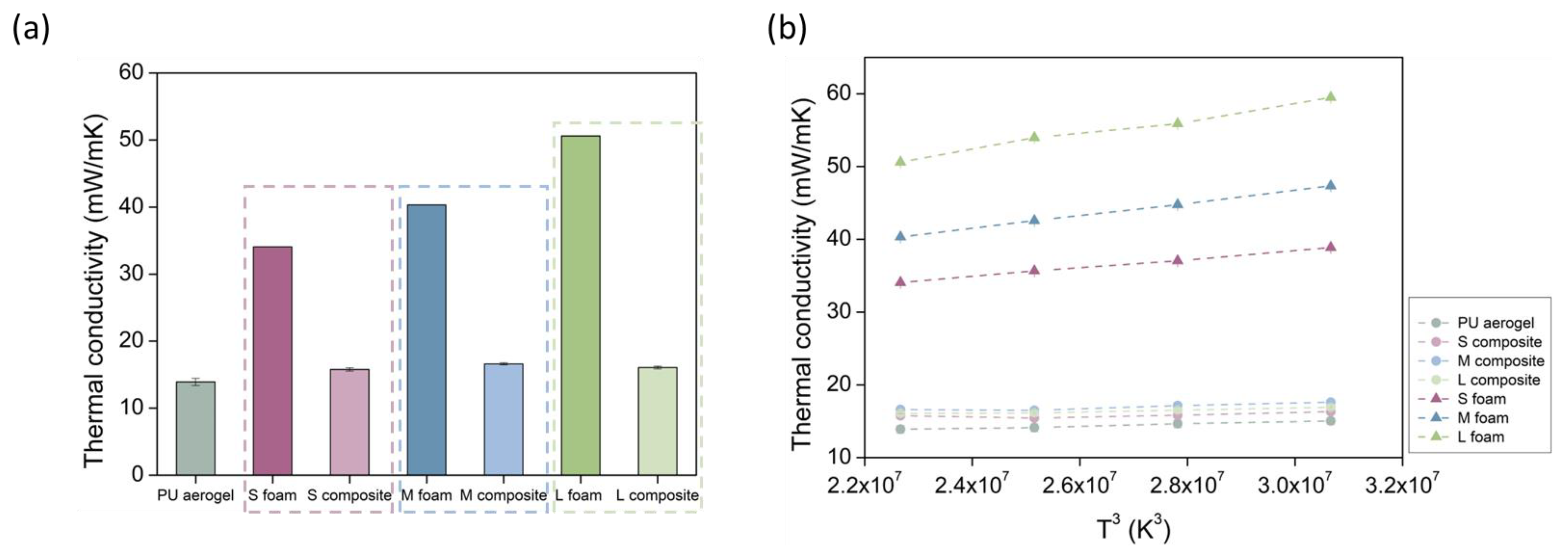
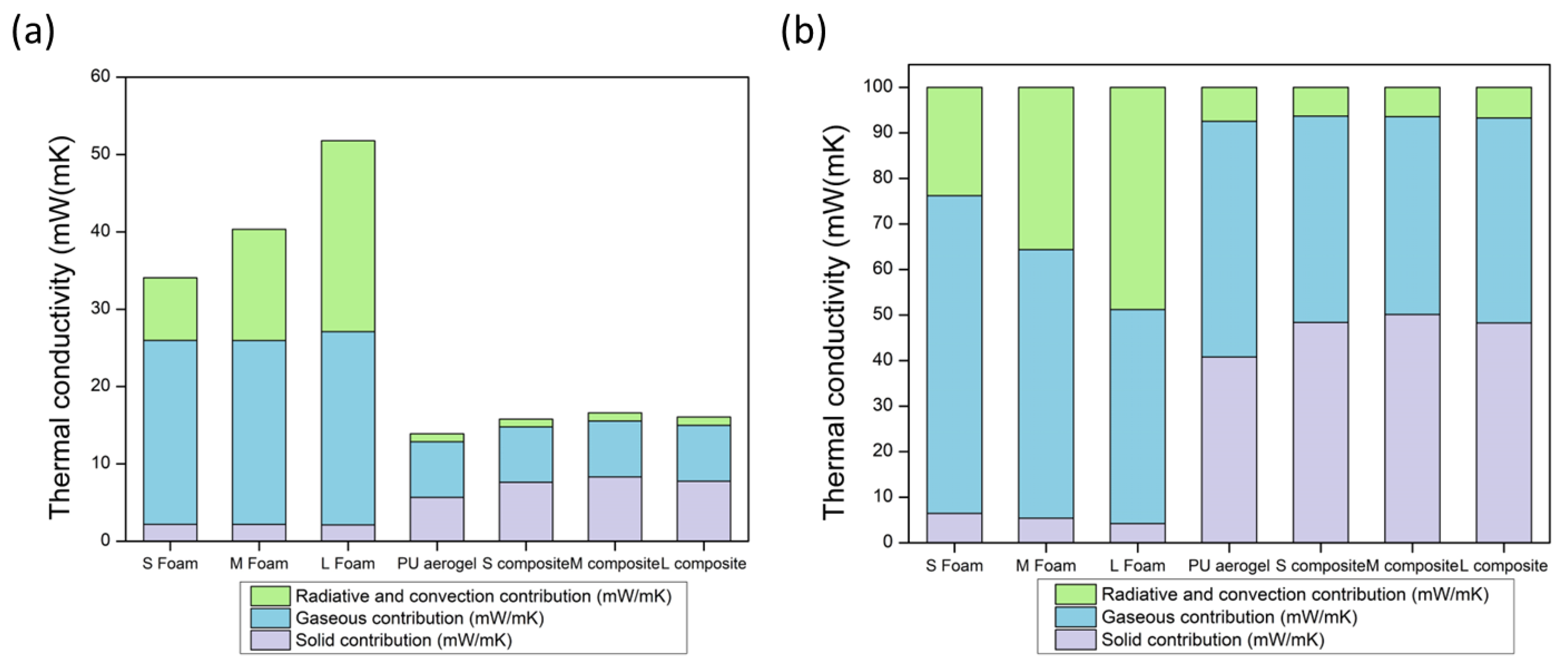
3.2.4. Thermal Conductivity
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Meier-westhues, U.; Squiller, E.P. Polyurethanes: Coatings, Adhesives and Sealants; Vincentz Network GmbH & Co. KG: Hannover, Germany, 2019; ISBN 9783866307889. [Google Scholar]
- Szycher, M. Szycher’s Handbook of Polyurethanes, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2012; ISBN 9781439863138. [Google Scholar]
- Jelle, B.P. Traditional, state-of-the-art and future thermal building insulation materials and solutions-Properties, requirements and possibilities. Energy Build. 2011, 43, 2549–2563. [Google Scholar] [CrossRef] [Green Version]
- Burgaz, E. Polyurethane Insulation Foams for Energy and Sustainability; Springer: Cham, Switzerland, 2019; Volume 111, ISBN 978-3-030-19557-1. [Google Scholar]
- Directive 2010/31/EU of the European Parliament and of the Council on the Energy Performance of Buildings; European Council: Brussels, Belgium, 2010.
- Widya, T.; Macosko, C.W. Nanoclay-modified rigid polyurethane foam. J. Macromol. Sci.-Phys. 2005, 44B, 897–908. [Google Scholar] [CrossRef]
- Pardo-Alonso, S.; Solórzano, E.; Estravís, S.; Rodríguez-Perez, M.A.; De Saja, J.A. In situ evidence of the nanoparticle nucleating effect in polyurethane-nanoclay foamed systems. Soft Matter 2012, 8, 11262–11270. [Google Scholar] [CrossRef]
- Wang, Y.; Cui, K.; Fang, B.; Wang, F. Cost-Effective Fabrication of Modified Palygorskite-Reinforced Rigid Polyurethane Foam Nanocomposites. Nanomaterials 2022, 12, 609. [Google Scholar] [CrossRef] [PubMed]
- Dolomanova, V.; Jens, C.M.R.; Jensen, L.R.; Pyrz, R.; Timmons, A.B. Mechanical properties and morphology of nano-reinforced rigid PU foam. J. Cell. Plast. 2011, 47, 81–93. [Google Scholar] [CrossRef]
- Bernal, M.M.; Lopez-Manchado, M.A.; Verdejo, R. In situ foaming evolution of flexible polyurethane foam nanocomposites. Macromol. Chem. Phys. 2011, 212, 971–979. [Google Scholar] [CrossRef] [Green Version]
- Visco, A.; Quattrocchi, A.; Nocita, D.; Montanini, R.; Pistone, A. Polyurethane foams loaded with carbon nanofibers for oil spill recovery: Mechanical properties under fatigue conditions and selective absorption in oil/water mixtures. Nanomaterials 2021, 11, 735. [Google Scholar] [CrossRef]
- Li, A.; Zhang, C.; Zhang, Y. Thermal conductivities of PU composites with graphene aerogels reduced by different methods. Compos. Part A Appl. Sci. Manuf. 2017, 103, 161–167. [Google Scholar] [CrossRef]
- Xie, H.; Yang, W.; Chun, A.; Yuen, Y.; Xie, C.; Xie, J.; Lu, H. Study on flame retarded flexible polyurethane foam / alumina aerogel composites with improved fire safety. Chem. Eng. J. 2017, 311, 310–317. [Google Scholar] [CrossRef]
- Hamamizadeh, E.; Mahabadi, H.A.; Khavanin, A. Investigating the Mechanical, Morphological, and Acoustic Properties of the Phenolic Aerogel/Flexible Polyurethane Foam Composite. J. Polym. Environ. 2022, 30, 2483–2492. [Google Scholar] [CrossRef]
- Kim, J.H.; Ahn, J.H.; Kim, J.D.; Lee, D.H.; Kim, S.K.; Lee, J.M. Influence of silica-aerogel on mechanical characteristics of polyurethane-based composites: Thermal conductivity and strength. Materials 2021, 14, 1790. [Google Scholar] [CrossRef] [PubMed]
- Nazeran, N.; Moghaddas, J. Synthesis and characterization of silica aerogel reinforced rigid polyurethane foam for thermal insulation application. J. Non-Cryst. Solids 2017, 461, 1–11. [Google Scholar] [CrossRef]
- Ahn, J.; Kim, J.; Kim, J.; Kim, S.; Hyun, K.; Park, S.; Lee, J. Enhancement of Mechanical and Thermal Characteristics of Polyurethane-Based Composite with Silica Aerogel. Mater. Sci. Forum. 2019, 951, 63–67. [Google Scholar] [CrossRef]
- Santiago-Calvo, M.; Tirado-Mediavilla, J.; Ruiz-Herrero, J.L.; Rodríguez-Pérez, M.Á.; Villafañe, F. The effects of functional nanofillers on the reaction kinetics, microstructure, thermal and mechanical properties of water blown rigid polyurethane foams. Polymer 2018, 150, 138–149. [Google Scholar] [CrossRef]
- Cimavilla-Román, P.; Pérez-Tamarit, S.; Santiago-Calvo, M.; Rodríguez-Pérez, M.Á. Influence of silica aerogel particles on the foaming process and cellular structure of rigid polyurethane foams. Eur. Polym. J. 2020, 135, 109884. [Google Scholar] [CrossRef]
- Maddalena, L.; Gomez, J.; Fina, A.; Carosio, F. Effects of graphite oxide nanoparticle size on the functional properties of layer-by-layer coated flexible foams. Nanomaterials 2021, 11, 266. [Google Scholar] [CrossRef]
- Ye, X.; Chen, Z.; Ai, S.; Hou, B.; Zhang, J.; Zhou, Q.; Wang, F.; Liu, H.; Cui, S. Microstructure characterization and thermal performance of reticulated SiC skeleton reinforced silica aerogel composites. Compos. Part B 2019, 177, 107409. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, Z.; Zhang, J.; Ai, S.; Tang, H. Ultralight and thermal insulation carbon foam/SiO2 aerogel composites. J. Porous Mater. 2019, 26, 1305–1312. [Google Scholar] [CrossRef]
- Merillas, B.; Martín-de León, J.; Villafañe, F.; Rodríguez-Pérez, M.A. Transparent Polyisocyanurate-Polyurethane-Based Aerogels: Key Aspects on the Synthesis and Their Porous Structures. ACS Appl. Polym. Mater. 2021, 3, 4607–4615. [Google Scholar] [CrossRef]
- ASTM D1622-08; Standard Test Method for Apparent Density of Rigid Cellular Plastics. ASTM International: West Conshohocken, PA, USA, 2008.
- Barrett, E.P.; Joyner, L.G.; Halenda, P.P. The Determination of Pore Volume and Area Distributions in Porous Substances. I. Computations from Nitrogen Isotherms. J. Am. Chem. Soc. 1951, 73, 373–380. [Google Scholar] [CrossRef]
- Pinto, J.; Solórzano, E.; Rodriguez-Perez, M.A.; De Saja, J.A. Characterization of the cellular structure based on user-interactive image analysis procedures. J. Cell. Plast. 2013, 49, 555–575. [Google Scholar] [CrossRef]
- ASTM D1621–00; Standard Test Method for Compressive Properties of Rigid Cellular Plastics. ASTM International: West Conshohocken, PA, USA, 1991; pp. 1621–1673.
- ISO 291:2005; Plastics—Standard Atmospheres for Conditioning and Testing. International Organization for Standardization: Geneva, Switzerland, 2005.
- Bhinder, J.; Agnihotri, P.K. Effect of carbon nanotube doping on the energy dissipation and rate dependent deformation behavior of polyurethane foams. J. Cell. Plast. 2020, 57, 287–311. [Google Scholar] [CrossRef]
- ASTM C518; Standard Test Method for Steady-State Thermal Transmission Properties by Means of the Heat Flow Meter Apparatus. ASTM International: West Conshohocken, PA, USA, 2017.
- ISO 8301:1991; Thermal Insulation—Determination of Steady-State Thermal Resistance and Related Properties—Heat Flow Meter Apparatus. International Organization for Standardization: Geneva, Switzerland, 1991.
- Sánchez-Calderón, I.; Merillas, B.; Bernardo, V.; Rodríguez-Pérez, M.Á. Methodology for measuring the thermal conductivity of insulating samples with small dimensions by heat flow meter technique. J. Therm. Anal. Calorim. 2022. [Google Scholar]
- Notario, B.; Pinto, J.; Solorzano, E.; De Saja, J.A.; Dumon, M.; Rodríguez-Pérez, M.A. Experimental validation of the Knudsen effect in nanocellular polymeric foams. Polymer 2015, 56, 57–67. [Google Scholar] [CrossRef]
- Merillas, B.; Martín-de León, J.; Villafañe, F.; Rodríguez-Pérez, M.Á. Optical Properties of Polyisocyanurate–Polyurethane Aerogels: Study of the Scattering Mechanisms. Nanomaterials 2022, 12, 1522. [Google Scholar] [CrossRef]
- Reignier, J.; Méchin, F.; Sarbu, A. Chemical gradients in PIR foams as probed by ATR-FTIR analysis and consequences on fire resistance. Polym. Test. 2021, 93, 106972. [Google Scholar] [CrossRef]
- Linul, E.; Marsavina, L.; Voiconi, T.; Sadowski, T. Study of factors influencing the mechanical properties of polyurethane foams under dynamic compression. J. Phys. Conf. Ser. 2013, 451, 012002. [Google Scholar] [CrossRef] [Green Version]
- Andersons, J.; Kirpluks, M.; Stiebra, L.; Cabulis, U. Anisotropy of the stiffness and strength of rigid low-density closed-cell polyisocyanurate foams. Mater. Des. 2016, 92, 836–845. [Google Scholar] [CrossRef]
- Chidambareswarapattar, C.; Mccarver, P.M.; Luo, H.; Lu, H.; Sotiriou-leventis, C.; Leventis, N. Fractal Multiscale Nanoporous Polyurethanes: Flexible to Extremely Rigid Aerogels from Multifunctional Small Molecules. Chem. Mater. 2013, 25, 3205–3224. [Google Scholar] [CrossRef]
- Rodríguez-Pérez, M.A.; González-Peña, J.I.; Witten, N.; De Saja, J.A. The effect of cell size on the physical properties of crosslinked closed cell polyethylene foams produced by a high pressure nitrogen solution process. Cell. Polym. 2002, 21, 165–194. [Google Scholar] [CrossRef]
- Aegerter, M.A.; Leventis, N.; Koebel, M.M. Aerogels Handbook; Springer: Berlin, Germany, 2011; ISBN 9781441974778. [Google Scholar]
- Zhang, Z.; Ouyang, Y.; Cheng, Y.; Chen, J.; Li, N.; Zhang, G. Size-dependent phononic thermal transport in low-dimensional nanomaterials. Phys. Rep. 2020, 860, 1–26. [Google Scholar] [CrossRef]
- Song, S.; Yovanovich, M.M.; Goodman, F.O. Thermal gap conductance of conforming surfaces in contact. J. Heat Transf. 1993, 115, 533–540. [Google Scholar] [CrossRef]
- Forest, C.; Chaumont, P.; Cassagnau, P.; Swoboda, B.; Sonntag, P. Polymer nano-foams for insulating applications prepared from CO2 foaming. Prog. Polym. Sci. 2015, 41, 122–145. [Google Scholar] [CrossRef]
- Hilyard, N.C.; Cunningham, A. Low Density Cellular Plastics: Physical Basis of Behaviour; Chapman and Hall: London, UK, 1994; ISBN 9789401045476. [Google Scholar]
- Hrubesh, L.W.; Pekala, R.W. Thermal properties of organic and inorganic aerogels. J. Mater. Res. 1994, 9, 731–738. [Google Scholar] [CrossRef]
- Kurańska, M.; Pinto, J.A.; Salach, K.; Barreiro, M.F.; Prociak, A. Synthesis of thermal insulating polyurethane foams from lignin and rapeseed based polyols: A comparative study. Ind. Crops Prod. 2020, 143, 111882. [Google Scholar] [CrossRef]
- Jarfelt, U.; Ramnäs, O. Thermal conductivity of polyurethane foam-best performance. In Proceedings of the 10th International Symposium on District Heating and Cooling, Reykjavik, Iceland, 3–5 September 2006; p. 12. [Google Scholar]
- Merillas, B.; Vareda, J.P.; Martín-de León, J.; Rodríguez-Pérez, M.Á.; Durães, L. Thermal Conductivity of Nanoporous Materials: Where Is the Limit? Polymers 2022, 14, 2556. [Google Scholar] [CrossRef]

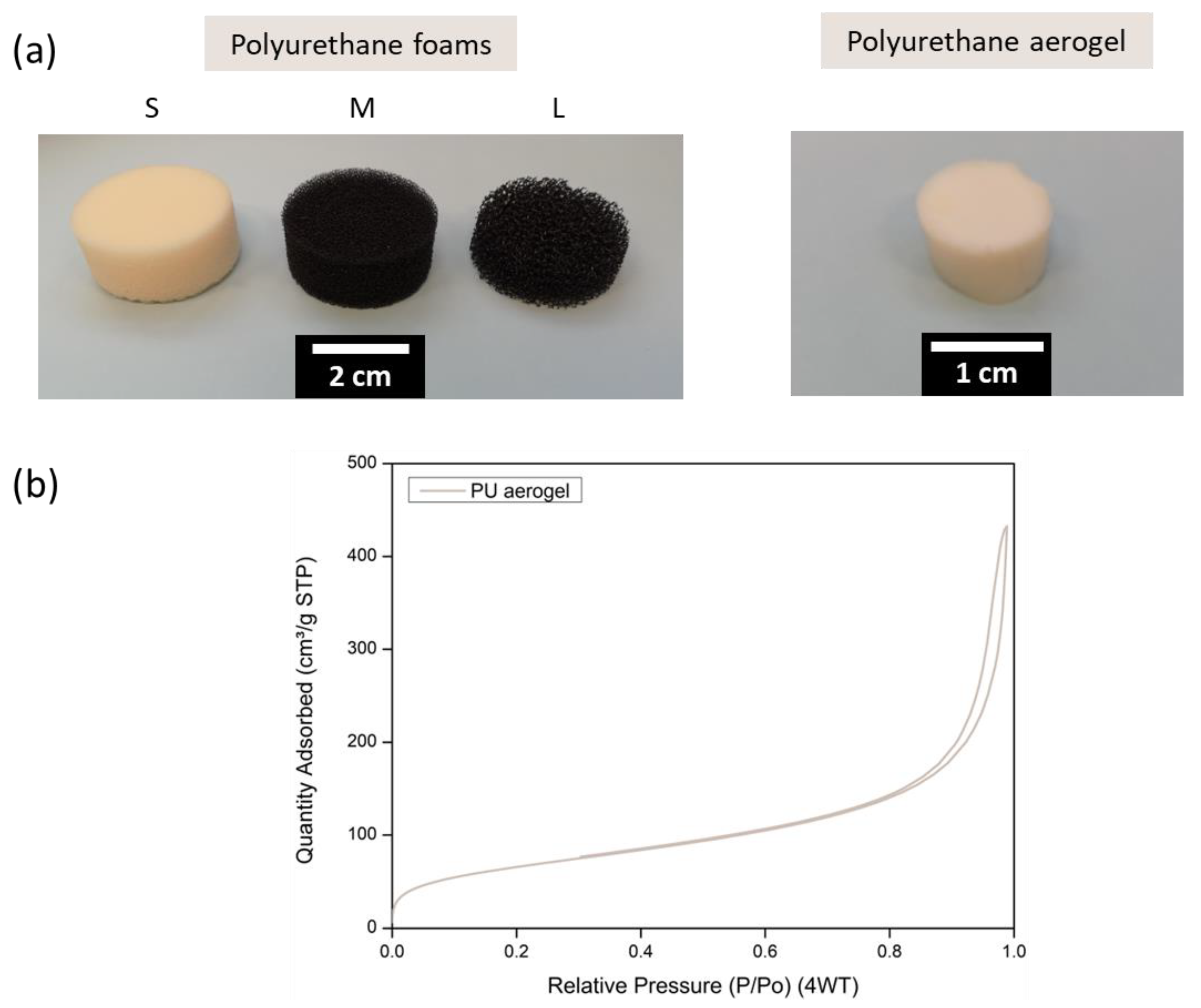

| Sample | Density (kg/m3) | SBET (m2/g) | φ | Λ (mW/m K) |
|---|---|---|---|---|
| PU aerogel | 129 ± 1.5 | 242.1 ± 12.1 | 114 nm | 13.90 ± 0.54 |
| S foam | 29.4 ± 0.7 | - | 0.44 mm | 34.07 ± 0.01 |
| M foam | 29.2 ± 0.4 | - | 1.4 mm | 40.33 ± 0.01 |
| L foam | 28.5 ± 0.5 | - | 4.3 mm | 50.60 ± 0.01 |
| Sample | Density (kg/m3) | Aerogel Mass (%) | Sv (%) | Aerogel Density (kg/m3) | Aerogel Porosity (%) |
|---|---|---|---|---|---|
| PU aerogel | 129 ± 1.5 | - | 66.3 | - | 88.87 |
| S foam | 29.4± 0.7 | - | - | - | - |
| S composite | 134 ± 0.3 | 81.95 | 9.6 | 107.9 ± 5.3 | 90.70 |
| M foam | 29.2 ± 0.4 | - | - | - | - |
| M composite | 125 ± 1.9 | 80.90 | 8.9 | 99.0 ± 4.7 | 91.46 |
| L foam | 28.5 ± 0.5 | - | - | - | - |
| L composite | 123 ± 0.9 | 80.78 | −1.6 | 97.6 ± 4.2 | 91.59 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Merillas, B.; Villafañe, F.; Rodríguez-Pérez, M.Á. Improving the Insulating Capacity of Polyurethane Foams through Polyurethane Aerogel Inclusion: From Insulation to Superinsulation. Nanomaterials 2022, 12, 2232. https://doi.org/10.3390/nano12132232
Merillas B, Villafañe F, Rodríguez-Pérez MÁ. Improving the Insulating Capacity of Polyurethane Foams through Polyurethane Aerogel Inclusion: From Insulation to Superinsulation. Nanomaterials. 2022; 12(13):2232. https://doi.org/10.3390/nano12132232
Chicago/Turabian StyleMerillas, Beatriz, Fernando Villafañe, and Miguel Ángel Rodríguez-Pérez. 2022. "Improving the Insulating Capacity of Polyurethane Foams through Polyurethane Aerogel Inclusion: From Insulation to Superinsulation" Nanomaterials 12, no. 13: 2232. https://doi.org/10.3390/nano12132232
APA StyleMerillas, B., Villafañe, F., & Rodríguez-Pérez, M. Á. (2022). Improving the Insulating Capacity of Polyurethane Foams through Polyurethane Aerogel Inclusion: From Insulation to Superinsulation. Nanomaterials, 12(13), 2232. https://doi.org/10.3390/nano12132232









