Elastic Properties of Single-Walled Phosphide Nanotubes: Numerical Simulation Study
Abstract
:1. Introduction
2. Materials and Methods
2.1. Atomic Structure of Phosphide Nanotubes
2.2. Molecular Mechanics of Phosphide Nanotubes and Equivalent Continuum Properties of Interatomic Bonds
2.2.1. Force Field Constants
2.2.2. Equivalent Properties of Elastic Beams
2.3. Geometrical Characteristics of Phosphide Nanotubes and FE Analysis
3. Results and Discussion
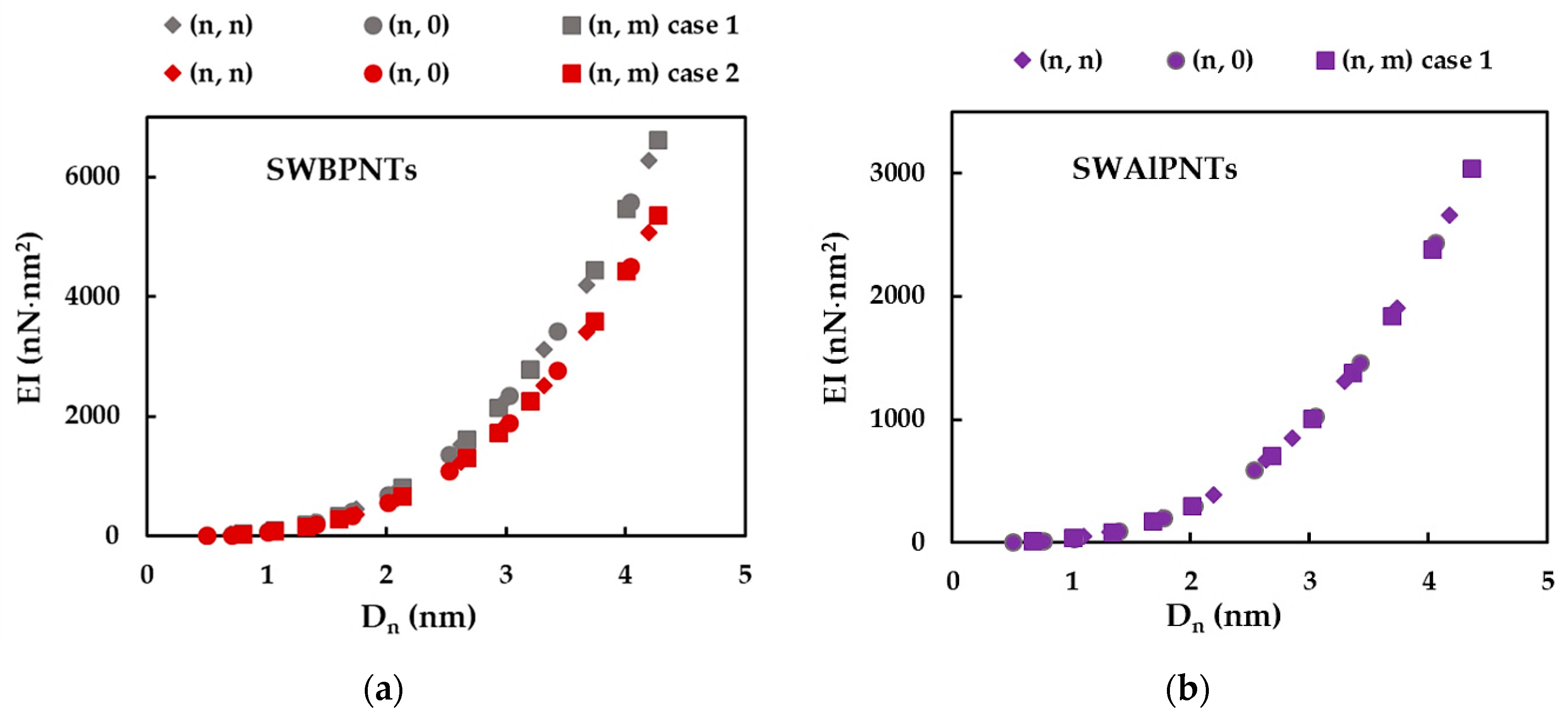
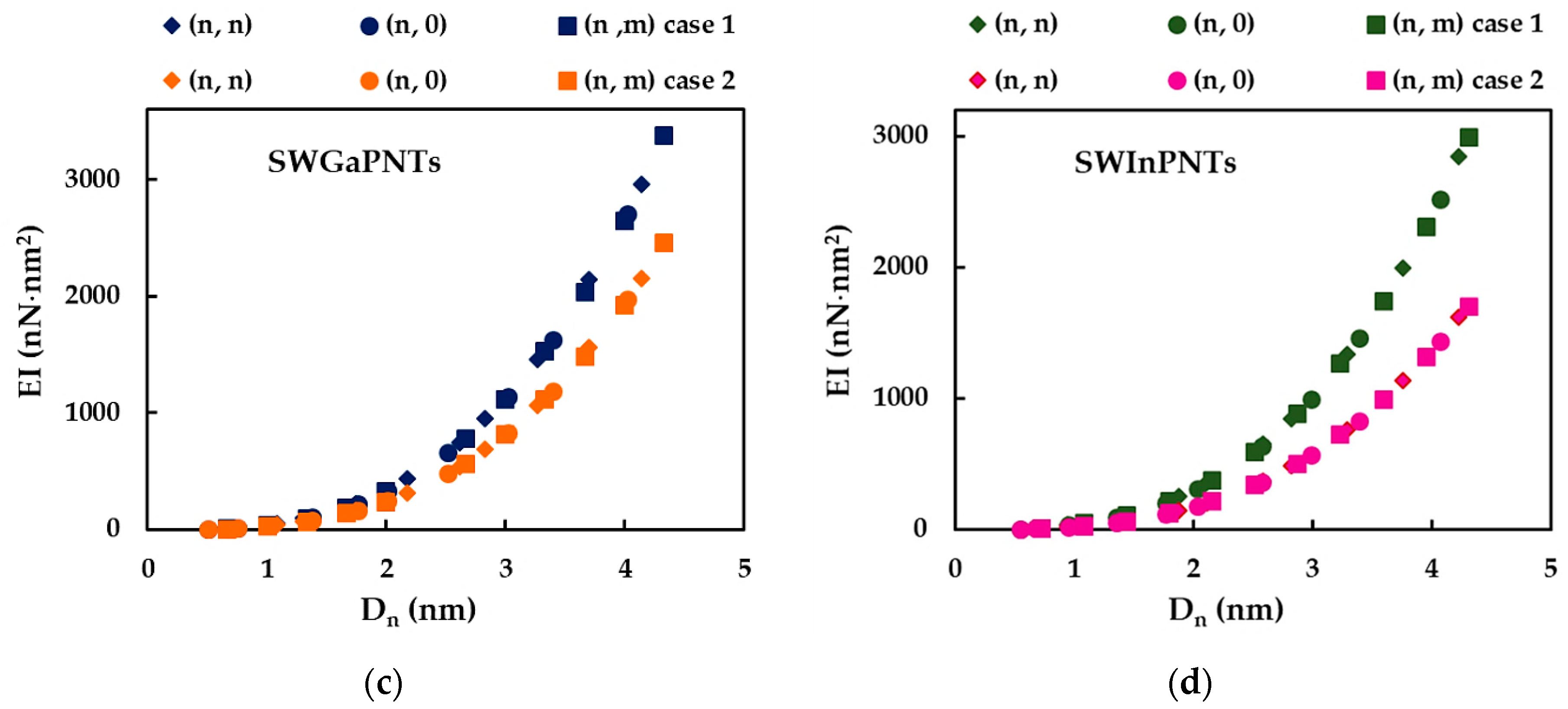
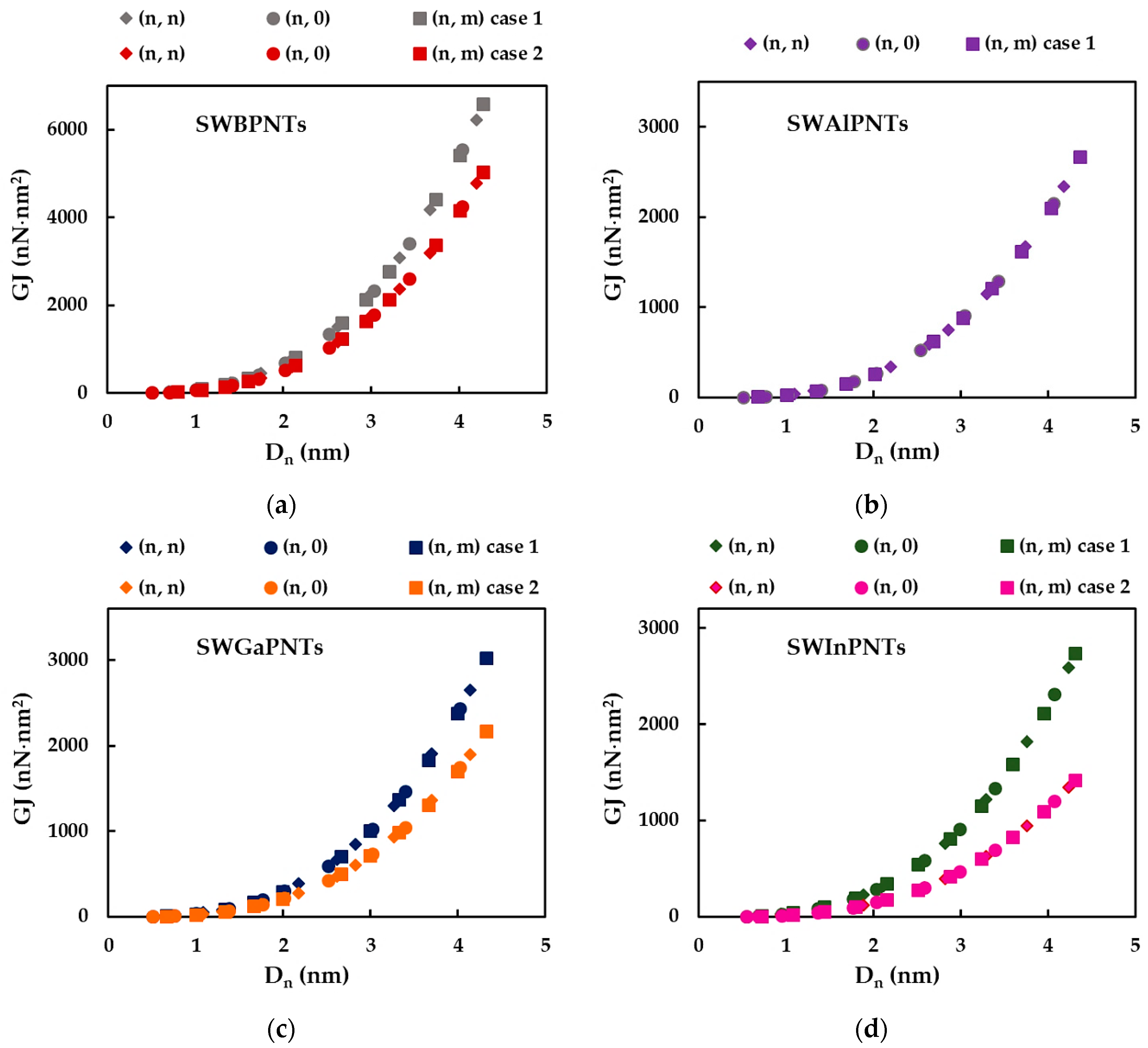
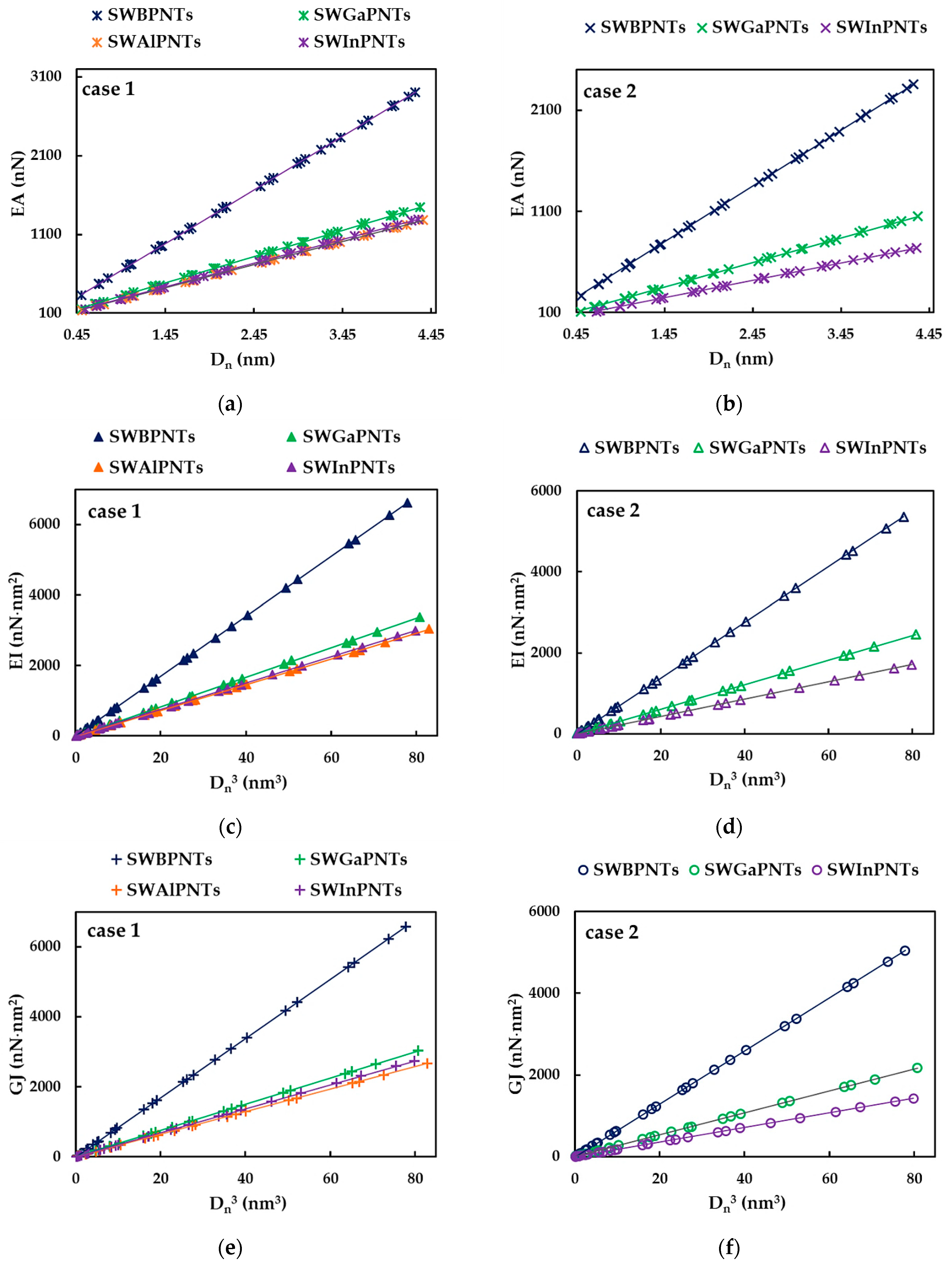
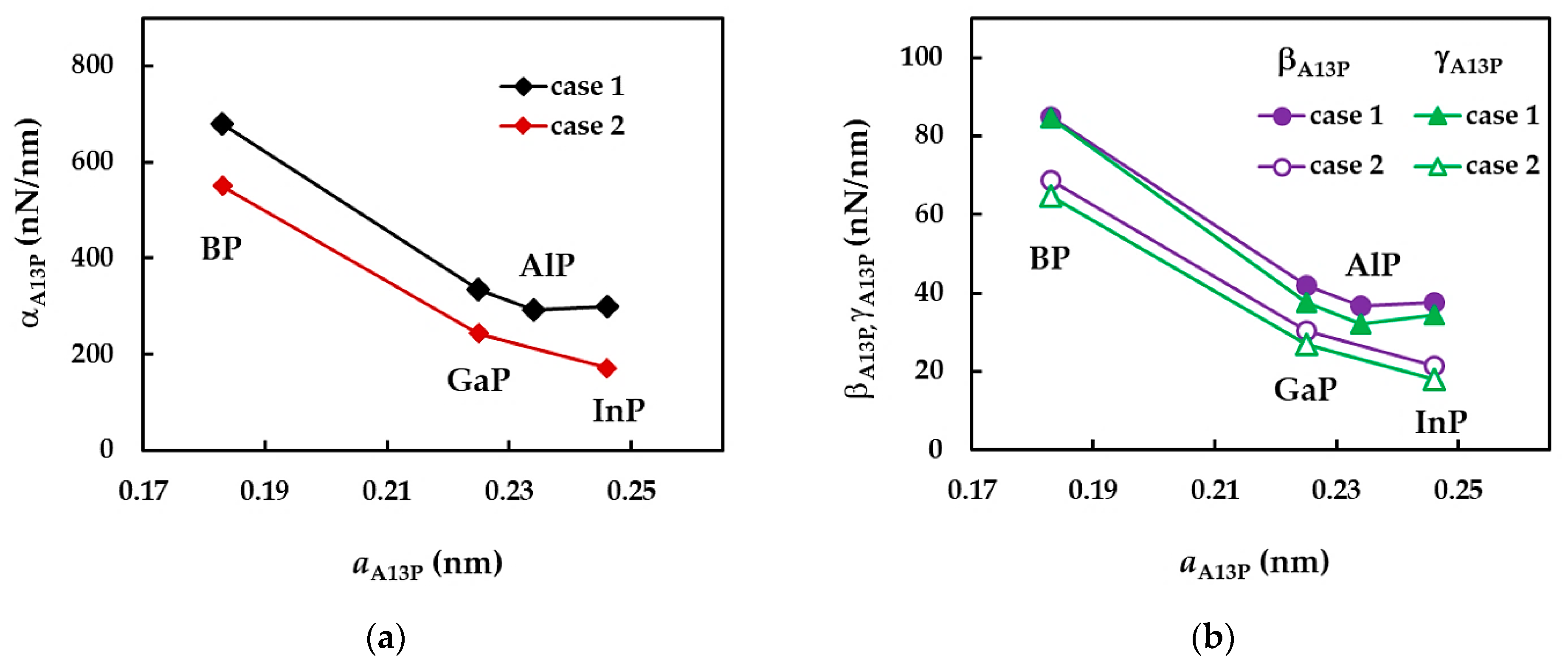
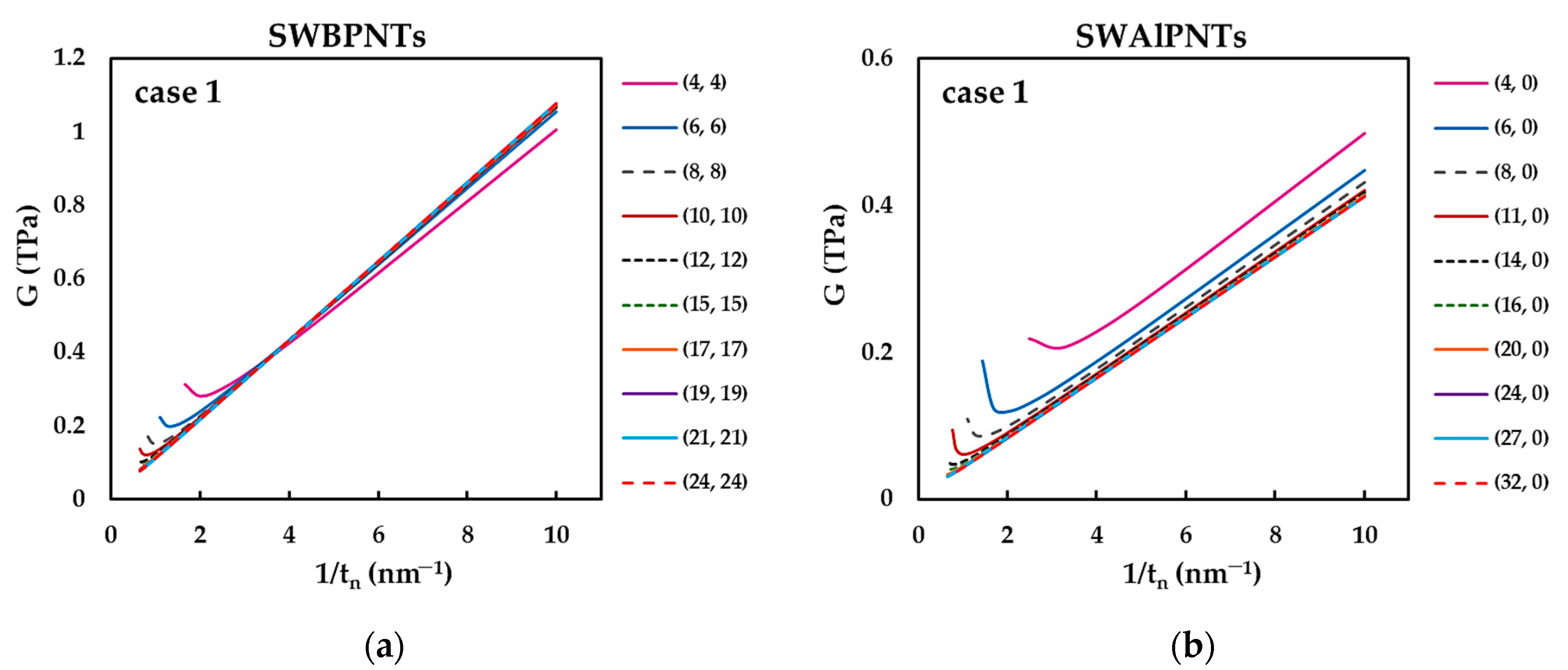
3.1. Rigidities of SWBPNTs, SWAlPNTs, SWGaPNTs and SWInPNTs
3.2. Elastic Moduli of SWBPNTs, SWAlPNTs, SWGaPNTs and SWInPNTs
3.2.1. Effect of Nanotube Wall Thickness on the Calculation of Elastic Moduli
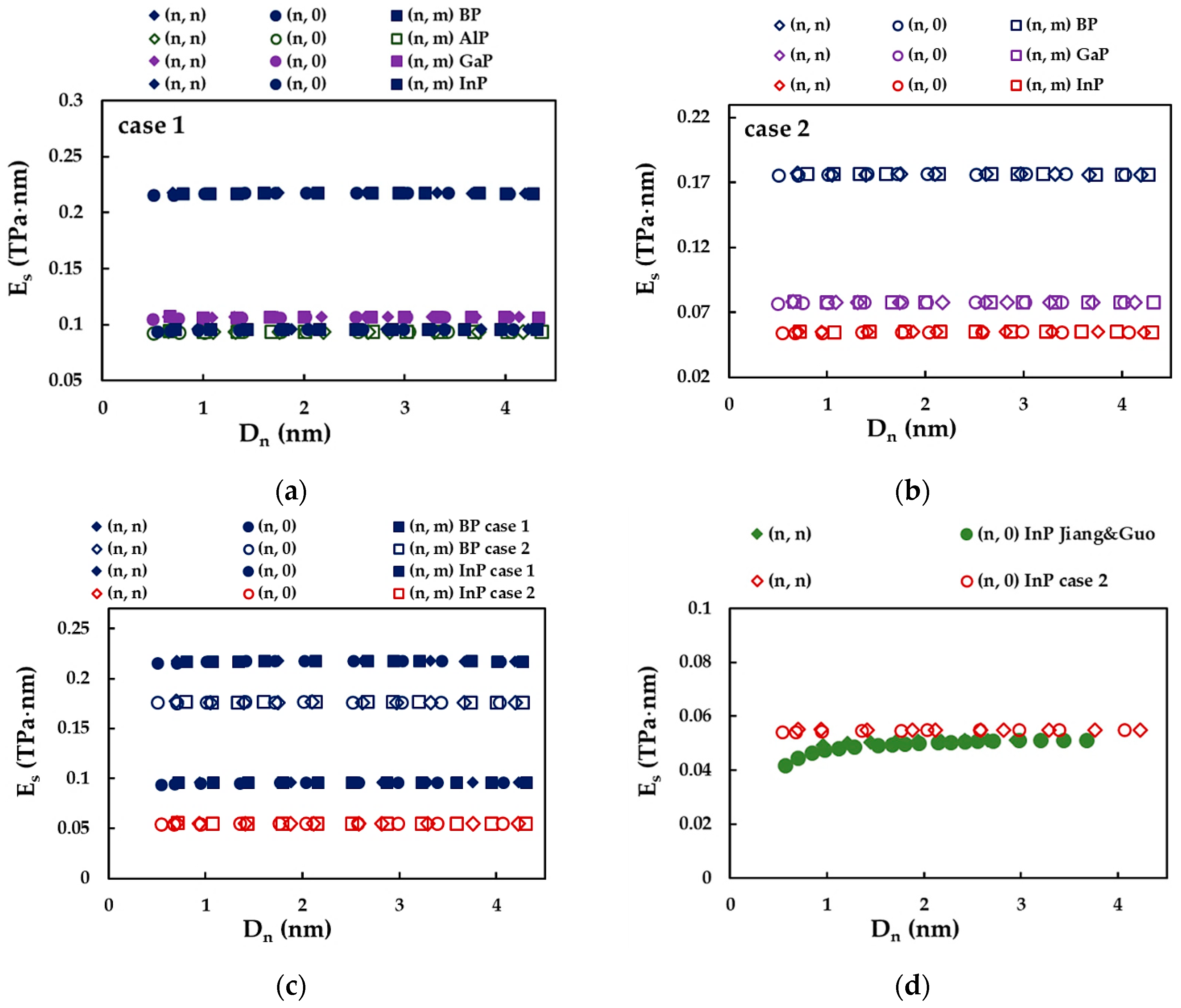
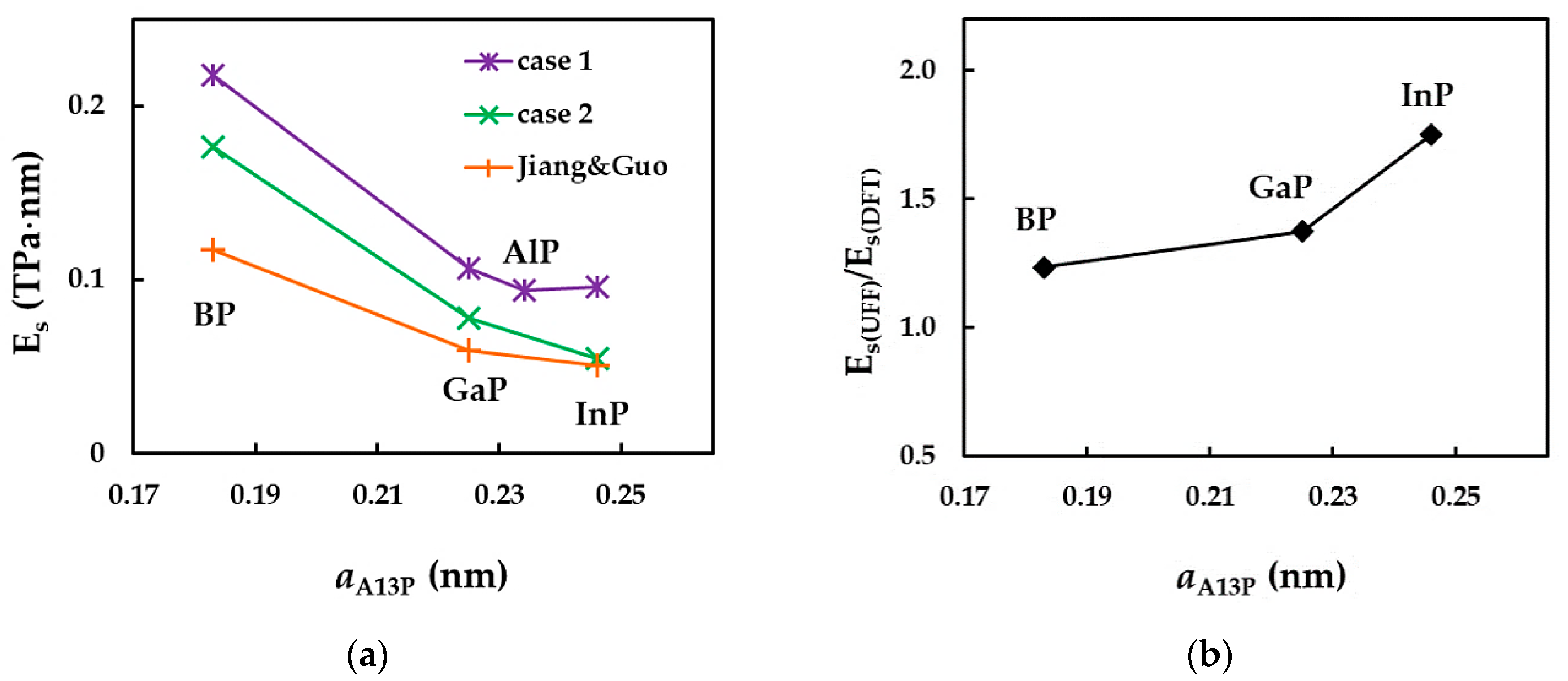
3.2.2. Surface Young’s Modulus of Phosphide Nanotubes
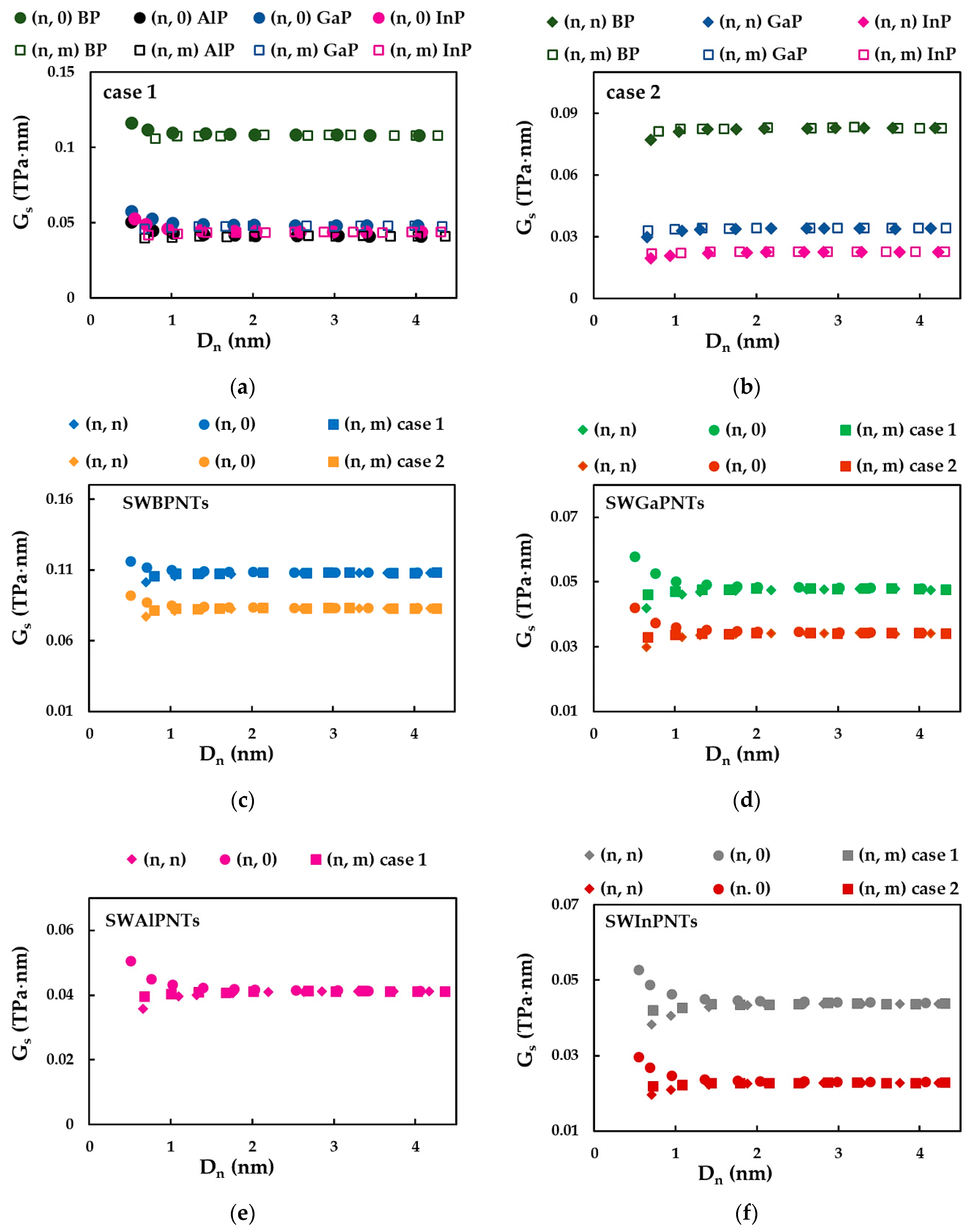
3.2.3. Surface Shear Modulus of Phosphide Nanotubes
3.3. Poisson’s Ratio of SWBPNTs, SWAlPNTs, SWGaPNTs and SWInPNTs
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kim, Y.H.; Jun, Y.W.; Jun, B.H.; Lee, S.M.; Cheon, J. Sterically induced shape and crystalline phase control of GaP nanocrystals. J. Am. Chem. Soc. 2002, 124, 13656–13657. [Google Scholar] [CrossRef] [PubMed]
- Wu, Q.; Hu, Z.; Liu, C.; Wang, X.; Chen, Y.; Lu, Y. Synthesis and optical properties of gallium phosphide nanotubes. J. Phys. Chem. B 2005, 109, 19719–19722. [Google Scholar] [CrossRef] [PubMed]
- Corbridge, D.E.C. Phosphorus: An Outline of Its Chemistry, Biochemistry, and Technology, 5th ed.; Elsevier: Amsterdam, The Netherlands, 1995. [Google Scholar]
- Duan, X.; Huang, Y.; Cui, Y.; Wang, J.; Lieber, C.M. Indium phosphide nanowires as building blocks for nanoscale electronic and optoelectronic devices. Nature 2001, 409, 66–68. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.F.; Gudiksen, M.S.; Duan, X.; Cui, Y.; Lieber, C.M. Highly polarized photoluminescence and photodetection from single indium phosphide nanowires. Science 2001, 293, 1455–1457. [Google Scholar] [CrossRef] [Green Version]
- Bjork, M.T.; Ohlsson, B.J.; Sass, T.; Persson, A.I.; Thelander, C.; Magnusson, M.H.; Deppert, K.; Wallenberg, L.R.; Samuelson, L. One-dimensional heterostructures in semiconductor nanowhiskers. Appl. Phys. Lett. 2002, 80, 1058–1060. [Google Scholar] [CrossRef]
- Gudiksen, M.S.; Lauhon, L.J.; Wang, J.; Smith, D.C.; Lieber, C.M. Growth of nanowire superlattice structures for nanoscale photonics and electronics. Nature 2002, 415, 617–620. [Google Scholar] [CrossRef]
- Bakkers, E.P.A.M.; Verheijen, M.A. Synthesis of InP Nanotubes. J. Am. Chem. Soc. 2003, 125, 3440–3441. [Google Scholar] [CrossRef]
- Law, M.; Goldberger, J.; Yang, P. Semiconductor nanowires and nanotubes. Annu. Rev. Mater. Res. 2004, 34, 83–122. [Google Scholar] [CrossRef] [Green Version]
- Yin, L.W.; Bando, Y.; Golberg, D.; Li, M.S. Template-free synthesis on single-crystalline InP nanotubes. Appl. Phys. Lett. 2004, 85, 3869–3871. [Google Scholar] [CrossRef]
- Palit, M.; Chowdhury, B.N.; Sikdar, S.; Sarkar, K.; Banerji, P.; Chattopadhyay, S. Band splitting induced by momentum-quantization in semiconductor nanostructures: Observation of emission lines in Indium Phosphide (InP) nanotubes. Phys. Lett. A 2021, 388, 127056. [Google Scholar] [CrossRef]
- Morales, A.M.; Lieber, C.M. A laser ablation method for the synthesis of crystalline semiconductor nanowires. Science 1998, 279, 208–211. [Google Scholar] [CrossRef] [PubMed]
- Mirzaei, M.; Giahi, M. Computational studies on boron nitride and boron phosphide nanotubes: Density functional calculations of boron-11 electric field gradient tensors. Phys. E 2010, 42, 1667–1669. [Google Scholar] [CrossRef]
- Mirzaei, M.; Meskinfam, M. Computational studies of effects of tubular lengths on the NMR properties of pristine and carbon decorated boron phosphide nanotubes. Solid State Sci. 2011, 13, 1926–1930. [Google Scholar] [CrossRef]
- Sayyad-Alangi, S.Z.; Hashemian, S.; Baei, M.T. Covalent functionalization of pristine and Ga-doped boron phosphide nanotubes with imidazole. Phosphorus Sulfur Silicon Relat. Elem. 2014, 189, 453–464. [Google Scholar] [CrossRef]
- Sayyad-Alangi, S.Z.; Baei, M.T.; Hashemian, S. Adsorption and electronic structure study of thiazole on the (6,0) zigzag single-walled boron phosphide nanotube. J. Sulphur Chem. 2013, 34, 407–420. [Google Scholar] [CrossRef]
- Lisenkov, S.V.; Vinogradov, G.A.; Lebedev, N.G. New class of non-carbon AlP nanotubes: Structure and Electronic Properties. JETP Lett. 2005, 81, 185–189. [Google Scholar] [CrossRef]
- Mirzaei, M.; Mirzaei, M. Aluminum phosphide nanotubes: Density functional calculations of aluminum-27 and phosphorus-31 chemical shielding parameters. J. Mol. Struct. THEOCHEM 2010, 951, 69–71. [Google Scholar] [CrossRef]
- Mirzaei, M.; Mirzaei, M. A computational study of gallium phosphide nanotubes. Phys. E 2011, 43, 1343–1345. [Google Scholar] [CrossRef]
- Kamal, C.; Chakrabarti, A.; Banerjee, A.; Deb, S.K. Ab initio studies of effect of intercalation on the properties of single walled carbon and gallium phosphide nanotubes. Phys. E 2013, 54, 273–280. [Google Scholar] [CrossRef]
- Srivastava, A.; Jain, S.K.; Khare, P.S. Ab-initio study of structural, electronic, and transport properties of zigzag GaP nanotubes. J. Mol. Model. 2014, 20, 2171. [Google Scholar] [CrossRef]
- Erkoç, S. Semi-empirical SCF-MO calculations for the structural and electronic properties of single-wall InP nanotubes. J. Mol. Struct. THEOCHEM 2004, 676, 109–113. [Google Scholar] [CrossRef]
- Muhsen, H.O.; Kadhim, B.B.; Almayyali, A.O.M. Electronic properties of InPNT drug carrier. J. Chem. Pharm. Sci. 2017, 10, 1414–1418. [Google Scholar]
- Mirzaei, M.; Yousefi, M.; Meskinfam, M. Studying (n, 0) and (m, m) GaP nanotubes (n = 3–10 and m = 2–6) through DFT calculations of Ga-69 quadrupole coupling constants. Solid State Sci. 2012, 14, 801–804. [Google Scholar] [CrossRef]
- Mirzaei, M.; Mirzaei, M. DFT calculations of NMR properties for GaP nanotubes. Monatsh. Chem. 2011, 142, 111–114. [Google Scholar] [CrossRef]
- Kochaev, A. Elastic properties of noncarbon nanotubes as compared to carbon nanotubes. Phys. Rev. B 2017, 96, 155428. [Google Scholar] [CrossRef]
- Jiang, L.; Guo, W. Analytical solutions for elastic binary nanotubes of arbitrary chirality. Acta Mech. Sin. 2016, 32, 1046–1057. [Google Scholar] [CrossRef]
- Şahin, H.; Cahangirov, S.; Topsakal, M.; Bekaroglu, E.; Akturk, E.; Senger, R.T.; Ciraci, S. Monolayer honeycomb structures of group-IV elements and III-V binary compounds: First-principles calculations. Phys. Rev. B 2009, 80, 155453. [Google Scholar] [CrossRef] [Green Version]
- Huber, K.P.; Hertzberg, G. Molecular Spectra and Molecular Siructure: IV. Constants of Diatomic Molecules, 1st ed.; Van Nostrand Reinhold Company: New York, NY, USA, 1979. [Google Scholar]
- Mayo, S.L.; Barry, D.; Olafson, B.D.; Goddard, W.A. DREIDING: A generic force field for molecular simulations. J. Phys. Chem. 1990, 94, 8897–8909. [Google Scholar] [CrossRef]
- Rappé, A.K.; Casewit, C.J.; Colwell, K.S.; Goddard, W.A.; Skid, W.M. UFF, a full periodic table force field for molecular mechanics and molecular dynamics simulations. J. Am. Chem. Soc. 1992, 114, 10024–10039. [Google Scholar] [CrossRef]
- Jiang, L.; Guo, W. A molecular mechanics study on size-dependent elastic properties of single-walled boron nitride nanotubes. J. Mech. Phys. Solids 2011, 59, 1204–1213. [Google Scholar] [CrossRef]
- Genoese, A.; Genoese, A.; Rizzi, N.L.; Salerno, G. Force constants of BN, SiC, AlN and GaN sheets through discrete homogenization. Meccanica 2018, 53, 593–611. [Google Scholar] [CrossRef]
- Ansari, R.; Rouhi, S.; Mirnezhad, M.; Aryayi, M. Stability characteristics of single-walled boron nitride nanotubes. Arch. Civ. Mech. Eng. 2015, 15, 162–170. [Google Scholar] [CrossRef]
- Tapia, A.; Cab, C.; Hernández-Pérez, A.; Villanueva, C.; Peñuñuri, F.; Avilés, F. The bond force constants and elastic properties of boron nitride nanosheets and nanoribbons using a hierarchical modeling approach. Phys. E 2017, 89, 183–193. [Google Scholar] [CrossRef]
- Kudin, K.N.; Scuseria, G.E.; Yakobson, B.I. C2F, BN, and C nanoshell elasticity from ab initio computations. Phys. Rev. B 2001, 64, 235406–235416. [Google Scholar] [CrossRef]
- Li, C.; Chou, T.W. A structural mechanics approach for the analysis of carbon nanotubes. Int. J. Solids Struct. 2003, 40, 2487–2499. [Google Scholar] [CrossRef]
- Chang, T.; Gao, H. Size-dependent elastic properties of a single-walled carbon nanotube via a molecular mechanics model. J. Mech. Phys. Solids 2003, 51, 1059–1074. [Google Scholar] [CrossRef]
- Sakharova, N.A.; Antunes, J.M.; Pereira, A.F.G.; Chaparro, B.M.; Fernandes, J.V. On the determination of elastic properties of single-walled boron nitride nanotubes by numerical simulation. Materials 2021, 14, 3183. [Google Scholar] [CrossRef]
- Sakharova, N.A.; Pereira, A.F.G.; Antunes, J.M.; Brett, C.M.A.; Fernandes, J.V. Mechanical characterization of single-walled carbon nanotubes. Numerical simulation study. Compos. B-Eng. 2015, 75, 73–85. [Google Scholar] [CrossRef]
- Pereira, A.F.G.; Antunes, J.M.; Fernandes, J.V.; Sakharova, N.A. Shear modulus and Poisson’s ratio of single-walled carbon nanotubes: Numerical evaluation. Phys. Status Solidi B 2016, 253, 366–376. [Google Scholar] [CrossRef]
- Antunes, J.M.; Pereira, A.F.G.; Sakharova, N.A. Overview on the Evaluation of the Elastic Properties of Non-Carbon Nanotubes by Theoretical Approaches. Materials 2022, 15, 3325. [Google Scholar] [CrossRef]


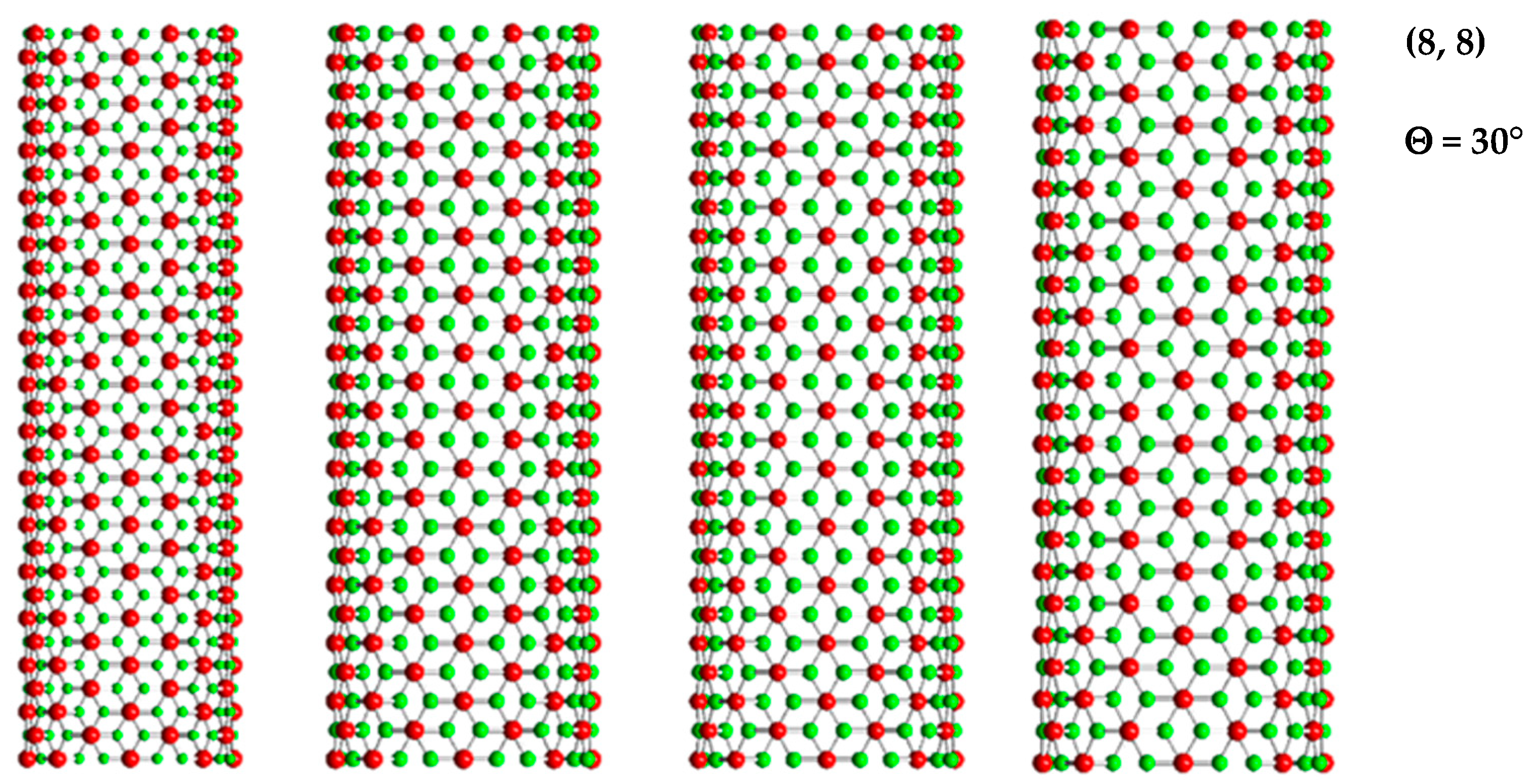

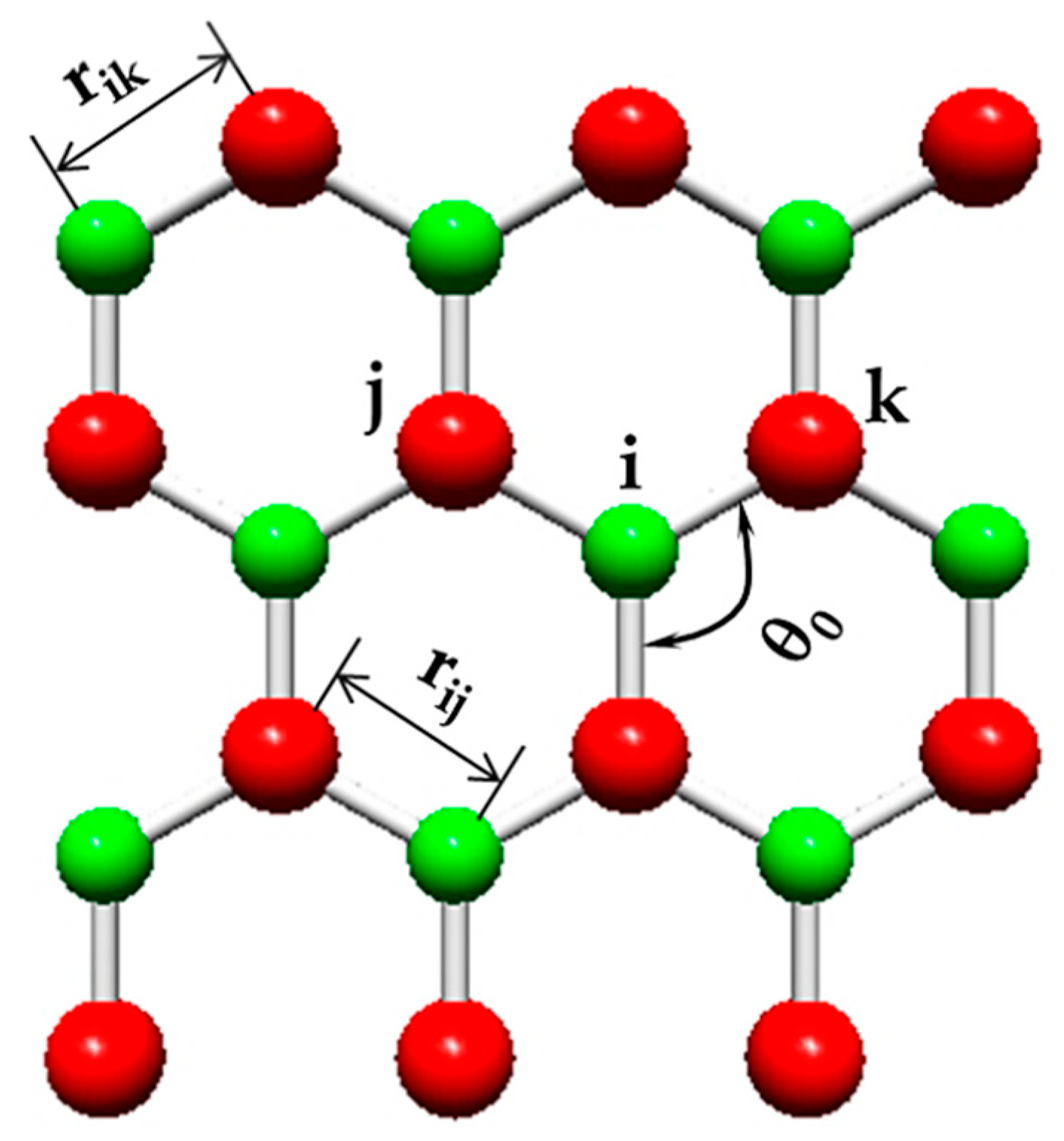
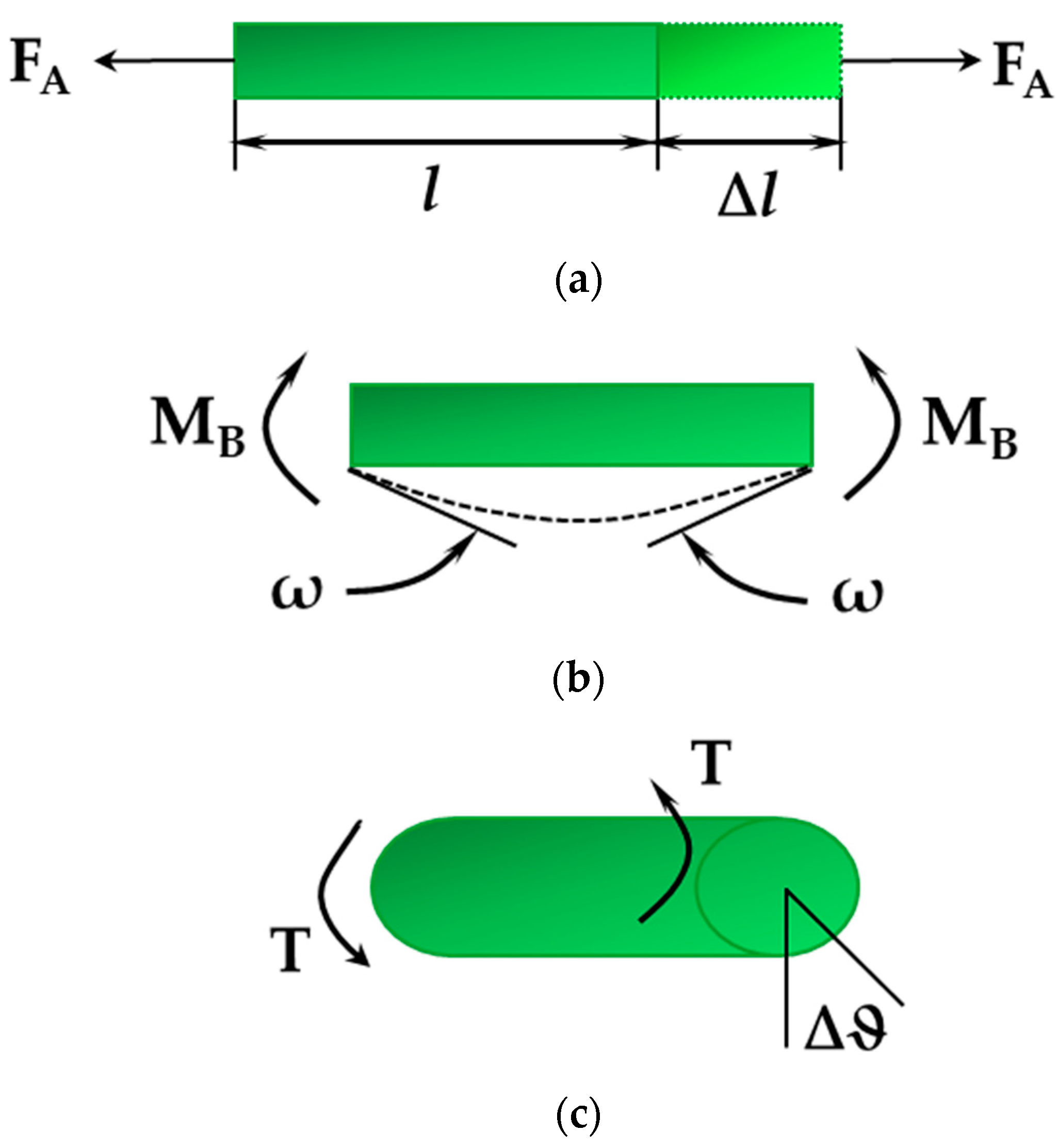



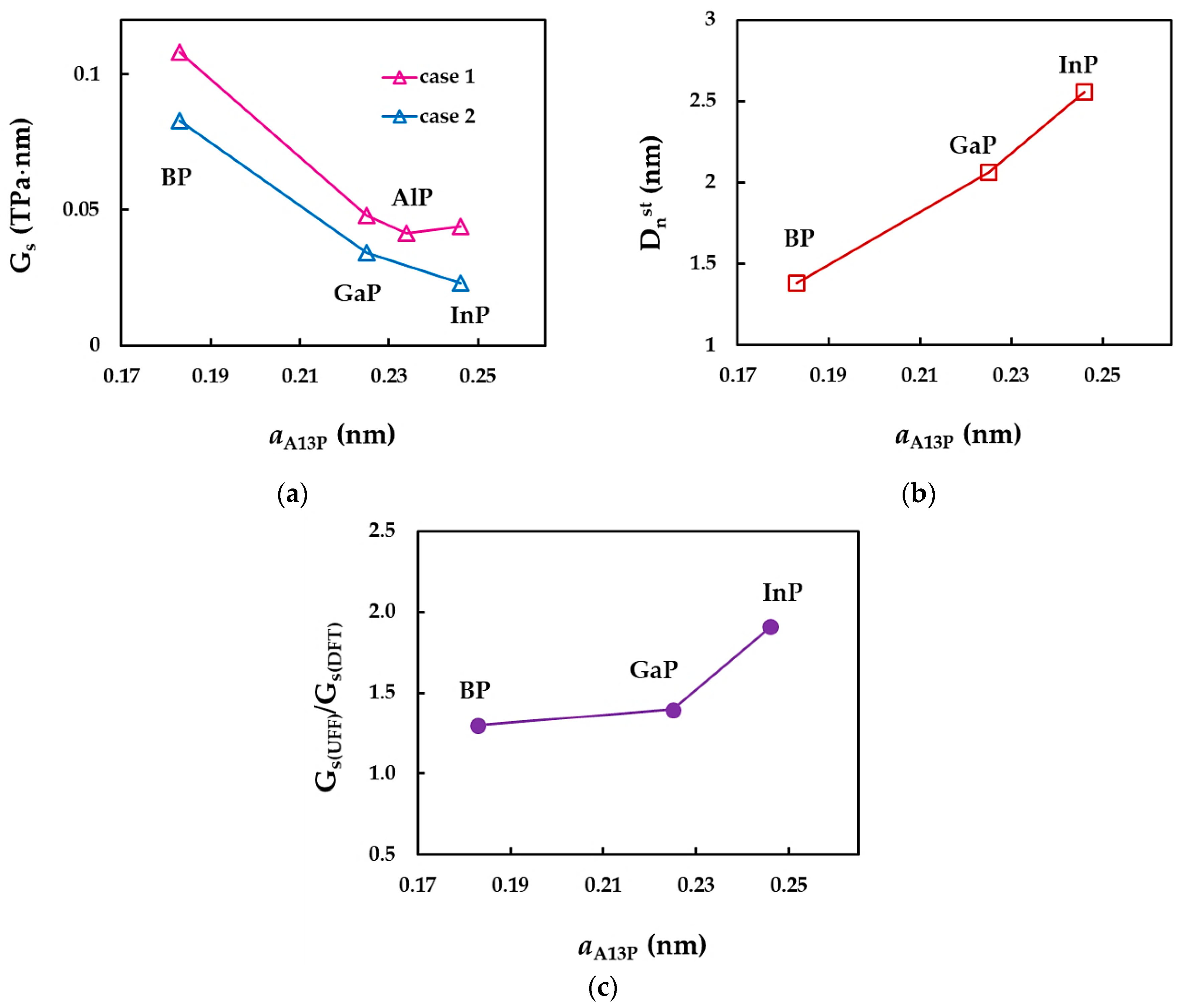



| BP | AlP | GaP | InP | |
|---|---|---|---|---|
| , nm | 0.183 [28] 0.193 [29] | 0.234 [17] 0.240 [26] | 0.220 [26] 0.225 [28] 0.229 [25] 0.236 [29] | 0.246 [28] 0.256 [29] |
| Compound | Atom 1 | Atom 2 | , nm | Es, nN/nm [28] | [28] | ||
|---|---|---|---|---|---|---|---|
| Charge [31] | Charge [31] | ||||||
| BP | B | P | 1.755 | 2.863 | 0.183 [28] | 135 | 0.28 |
| AlP | Al | 1.792 | 0.234 [17] | – | – | ||
| GaP | Ga | 1.821 | 0.225 [28] | 59 | 0.35 | ||
| InP | In | 2.070 | 0.246 [28] | 39 | 0.43 |
| Compound | Case 1 | ||||
|---|---|---|---|---|---|
| BP | 1 | 379 | 1.486 | 0.558 | 0.625 |
| 2 | 325 | 1.031 | 0.387 | ||
| AlP | 1 | 185 | 0.711 | 0.278 | |
| 2 | – | – | – | ||
| GaP | 1 | 211 | 0.853 | 0.345 | |
| 2 | 157 | 0.599 | 0.242 | ||
| InP | 1 | 184 | 0.852 | 0.446 | |
| 2 | 119 | 0.391 | 0.204 |
| Compound | Case 1 | l, nm [17,28] | d, nm Equation (26) | Eb, GPa Equation (27) | Gb, GPa Equation (28) | νb Equation (29) |
|---|---|---|---|---|---|---|
| BP | 1 | 0.183 | 0.2078 | 2042 | 624 | 0.21 |
| 2 | 0.1869 | 2165 | 954 | 0.28 | ||
| GaP | 1 | 0.225 | 0.2130 | 1335 | 696 | 0.33 |
| 2 | 0.2069 | 1052 | 782 | 0.35 | ||
| AlP | 1 | 0.234 | 0.2069 | 1287 | 813 | 0.38 |
| 2 | - | - | - | - | ||
| InP | 1 | 0.246 | 0.2377 | 1019 | 491 | 0.32 |
| 2 | 0.2004 | 924 | 722 | 0.43 |
| NT type | SWBPNTs | SWAlPNTs | SWGaPNTs | SWInPNTs | ||||
|---|---|---|---|---|---|---|---|---|
| (n, m) | Dn, nm | (n, m) | Dn, nm 1 | (n, m) | Dn, nm 1 | (n, m) | Dn, nm | |
| armchair (n, n), Θ = 30° | (4, 4) | 0.699 | (3, 3) | 0.659 | (3, 3) | 0.653 | (3, 3) | 0.705 |
| (6, 6) | 1.049 | (5, 5) | 1.098 | (5, 5) | 1.089 | (4, 4) | 0.940 | |
| (8, 8) | 1.398 | (6, 6) | 1.318 | (6, 6) | 1.306 | (6, 6) | 1.409 | |
| (10, 10) | 1.748 | (8, 8) | 1.757 | (8, 8) | 1.742 | (8, 8) | 1.879 | |
| (12, 12) | 2.097 | (10, 10) | 2.196 | (10, 10) | 2.177 | (9, 9) | 2.114 | |
| (15, 15) | 2.621 | (12, 12) | 2.636 | (12, 12) | 2.613 | (11, 11) | 2.584 | |
| (17, 17) | 2.971 | (13, 13) | 2.855 | (13, 13) | 2.830 | (12, 12) | 2.819 | |
| (19, 19) | 3.320 | (15, 15) | 3.295 | (15, 15) | 3.266 | (14, 14) | 3.289 | |
| (21, 21) | 3.670 | (17, 17) | 3.734 | (17, 17) | 3.701 | (16, 16) | 3.759 | |
| (24, 24) | 4.194 | (19, 19) | 4.173 | (19, 19) | 4.137 | (18, 18) | 4.228 | |
| zigzag (n, 0), Θ = 0° | (5, 0) | 0.504 | (4, 0) | 0.507 | (4, 0) | 0.503 | (4, 0) | 0.543 |
| (7, 0) | 0.706 | (6, 0) | 0.761 | (6, 0) | 0.754 | (5, 0) | 0.678 | |
| (10, 0) | 1.009 | (8, 0) | 1.014 | (8, 0) | 1.006 | (7, 0) | 0.949 | |
| (14, 0) | 1.413 | (11, 0) | 1.395 | (11, 0) | 1.383 | (10, 0) | 1.356 | |
| (17, 0) | 1.715 | (14, 0) | 1.775 | (14, 0) | 1.760 | (13, 0) | 1.763 | |
| (20, 0) | 2.018 | (16, 0) | 2.029 | (16, 0) | 2.011 | (15, 0) | 2.034 | |
| (25, 0) | 2.522 | (20, 0) | 2.536 | (20, 0) | 2.514 | (19, 0) | 2.577 | |
| (30, 0) | 3.027 | (24, 0) | 3.043 | (24, 0) | 3.017 | (22, 0) | 2.984 | |
| (34, 0) | 3.430 | (27, 0) | 3.424 | (27, 0) | 3.394 | (25, 0) | 3.391 | |
| (40, 0) | 4.036 | (32, 0) | 4.058 | (32, 0) | 4.022 | (30, 0) | 4.069 | |
| chiral (n, m), Θ = 19.1° | (6, 3) | 0.801 | (4, 2) | 0.671 | (4, 2) | 0.665 | (4, 2) | 0.718 |
| (8, 4) | 1.068 | (6, 3) | 1.006 | (6, 3) | 0.998 | (6, 3) | 1.077 | |
| (10, 5) | 1.335 | (8, 4) | 1.342 | (8, 4) | 1.330 | (8, 4) | 1.435 | |
| (12, 6) | 1.602 | (10, 5) | 1.677 | (10, 5) | 1.663 | (10, 5) | 1.794 | |
| (16, 8) | 2.136 | (12, 6) | 2.013 | (12, 6) | 1.995 | (12, 6) | 2.153 | |
| (20, 10) | 2.669 | (16, 8) | 2.684 | (16, 8) | 2.661 | (14, 7) | 2.512 | |
| (22, 11) | 2.936 | (18, 9) | 3.019 | (18, 9) | 2.993 | (16, 8) | 2.871 | |
| (24, 12) | 3.203 | (20, 10) | 3.355 | (20, 10) | 3.326 | (18, 9) | 3.230 | |
| (28, 14) | 3.737 | (22, 11) | 3.690 | (22, 11) | 3.658 | (20, 10) | 3.588 | |
| (30, 15) | 4.004 | (24, 12) | 4.026 | (24, 12) | 3.991 | (22, 11) | 3.947 | |
| (32, 16) | 4.271 | (26, 13) | 4.361 | (26, 13) | 4.324 | (24, 12) | 4.306 | |
| NTs | Case | |||
|---|---|---|---|---|
| BP | 1 | 680.40 | 84.99 | 84.44 |
| 2 | 550.96 | 68.80 | 64.75 | |
| AlP | 1 | 292.99 | 36.60 | 32.17 |
| 2 | – | – | – | |
| GaP | 1 | 334.07 | 41.78 | 37.43 |
| 2 | 243.15 | 30.40 | 26.81 | |
| InP | 1 | 300.5 | 37.54 | 34.29 |
| 2 | 171.35 | 21.39 | 17.84 |
| NTs | Case | Mean Difference, % | ||
| EA, nN | EI, nN·nm2 | GJ, nN·nm2 | ||
| BP | 1 | 0.34 | 0.60 | 0.37 |
| 2 | 0.25 | 0.65 | 0.43 | |
| AlP | 1 | 0.53 | 0.83 | 0.51 |
| 2 | – | – | – | |
| GaP | 1 | 0.38 | 0.82 | 0.55 |
| 2 | 0.40 | 0.85 | 0.52 | |
| InP | 1 | 0.39 | 0.77 | 0.43 |
| 2 | 0.39 | 0.82 | 0.50 | |
| Reference | NT Type | Es, TPa | Comments | |||
|---|---|---|---|---|---|---|
| SWBPNTs | SWAlPNTs | SWGaPNTs | SWInPNTs | |||
| Kochaev [26] | (n, n) | - | 0.228 | 0.161 | - | maximum value |
| (n, 0) | 0.208 | 0.139 | ||||
| (n, n) | 0.050 | 0.050 | minimum value | |||
| (n, 0) | 0.072 | 0.025 | ||||
| Jiang and Guo [27] | (n, n) | 0.118 | - | 0.060 | 0.051 | converged average value |
| (n, 0) | 0.117 | 0.059 | 0.051 | |||
| Present study | (n, n) | 0.218 1 | 0.094 1 | 0.107 1 | 0.096 1 | average value |
| 0.176 2 | - | 0.078 2 | 0.055 2 | |||
| (n, 0) | 0.218 1 | 0.094 1 | 0.107 1 | 0.096 1 | ||
| 0.176 2 | - | 0.078 2 | 0.055 2 | |||
| Elastic Moduli | SWCNTs | SWBNNTs | SWBPNTs | SWAlPNTs | SWGaPNTs | SWInPNTs |
|---|---|---|---|---|---|---|
| , TPa·nm | 0.361 [39,40] | 0.335 [39] | 0.218 1 | 0.094 1 | 0.107 1 | 0.096 1 |
| 0.176 2 | 0.078 2 | 0.055 2 | ||||
| , TPa·nm | 0.171 [39,41] | 0.165 [39] | 0.108 1 | 0.041 1 | 0.048 1 | 0.044 1 |
| 0.083 2 | 0.034 2 | 0.023 2 |
| Reference | NT Type | ν | Comments | |||
|---|---|---|---|---|---|---|
| SWBPNTs | SWAlPNTs | SWGaPNTs | SWInPNTs | |||
| Kochaev [26] | (10, 10) | - | 0.51 | 0.51 | - | - |
| (10, 0) | 0.51 | 0.52 | ||||
| Jiang and Guo [27] | (n, n) | 0.36 | - | 0.43 | 0.46 | converged average value |
| (n, 0) | 0.36 | 0.44 | 0.46 | |||
| Present study | (n, n) | 0.01 1 | 0.14 1 | 0.12 1 | 0.10 1 | converged average value |
| 0.06 2 | - | 0.14 2 | 0.055 2 | |||
| (n, 0) | 0.01 1 | 0.13 1 | 0.11 1 | 0.09 1 | ||
| 0.06 2 | - | 0.12 2 | 0.19 2 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sakharova, N.A.; Antunes, J.M.; Pereira, A.F.G.; Chaparro, B.M.; Fernandes, J.V. Elastic Properties of Single-Walled Phosphide Nanotubes: Numerical Simulation Study. Nanomaterials 2022, 12, 2360. https://doi.org/10.3390/nano12142360
Sakharova NA, Antunes JM, Pereira AFG, Chaparro BM, Fernandes JV. Elastic Properties of Single-Walled Phosphide Nanotubes: Numerical Simulation Study. Nanomaterials. 2022; 12(14):2360. https://doi.org/10.3390/nano12142360
Chicago/Turabian StyleSakharova, Nataliya A., Jorge M. Antunes, André F. G. Pereira, Bruno M. Chaparro, and José V. Fernandes. 2022. "Elastic Properties of Single-Walled Phosphide Nanotubes: Numerical Simulation Study" Nanomaterials 12, no. 14: 2360. https://doi.org/10.3390/nano12142360
APA StyleSakharova, N. A., Antunes, J. M., Pereira, A. F. G., Chaparro, B. M., & Fernandes, J. V. (2022). Elastic Properties of Single-Walled Phosphide Nanotubes: Numerical Simulation Study. Nanomaterials, 12(14), 2360. https://doi.org/10.3390/nano12142360






