Sensitivity Analysis of the Catalysis Recombination Mechanism on Nanoscale Silica Surfaces
Abstract
:1. Introduction

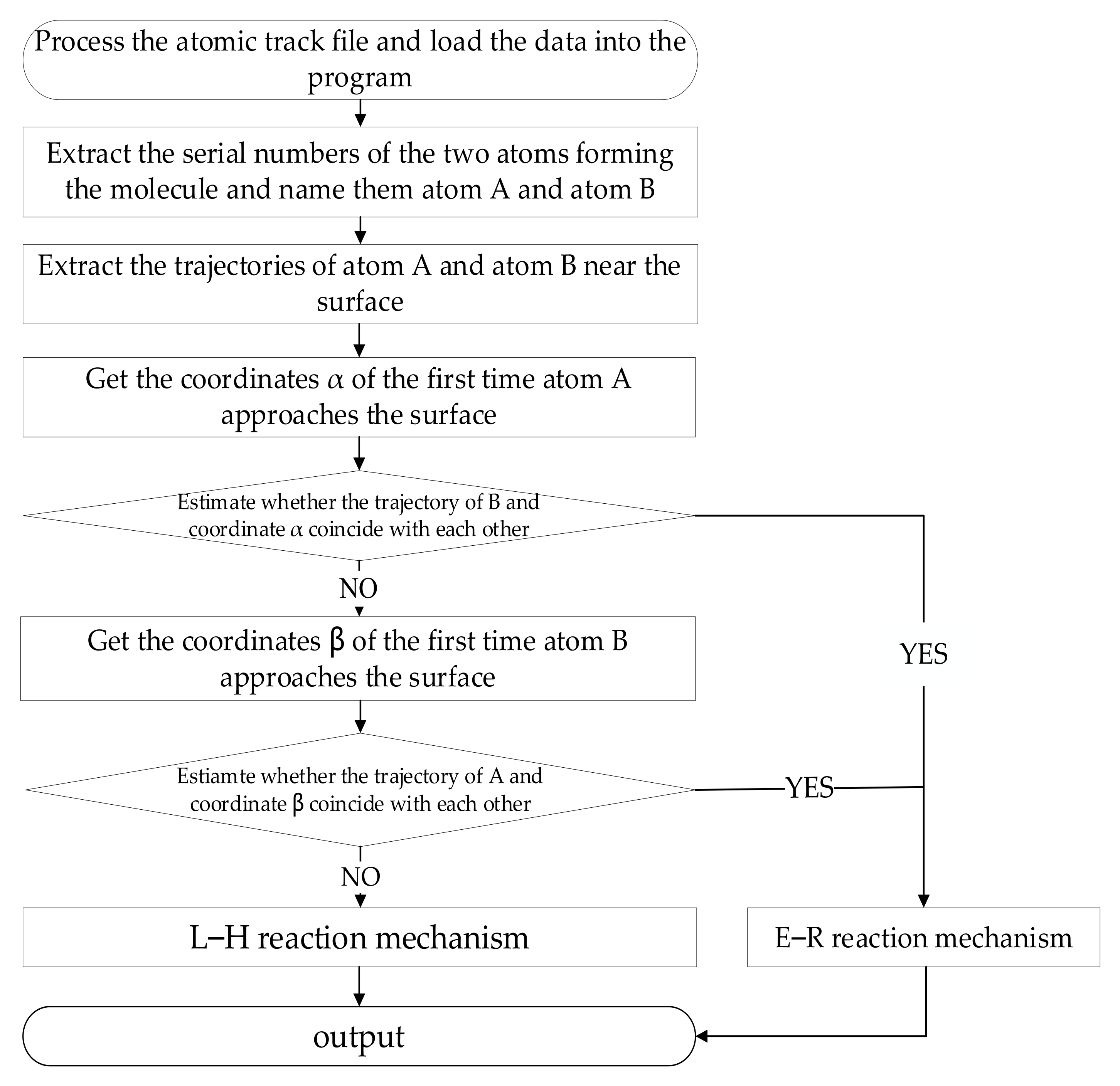
2. Materials and Methods
3. Results and Discussion
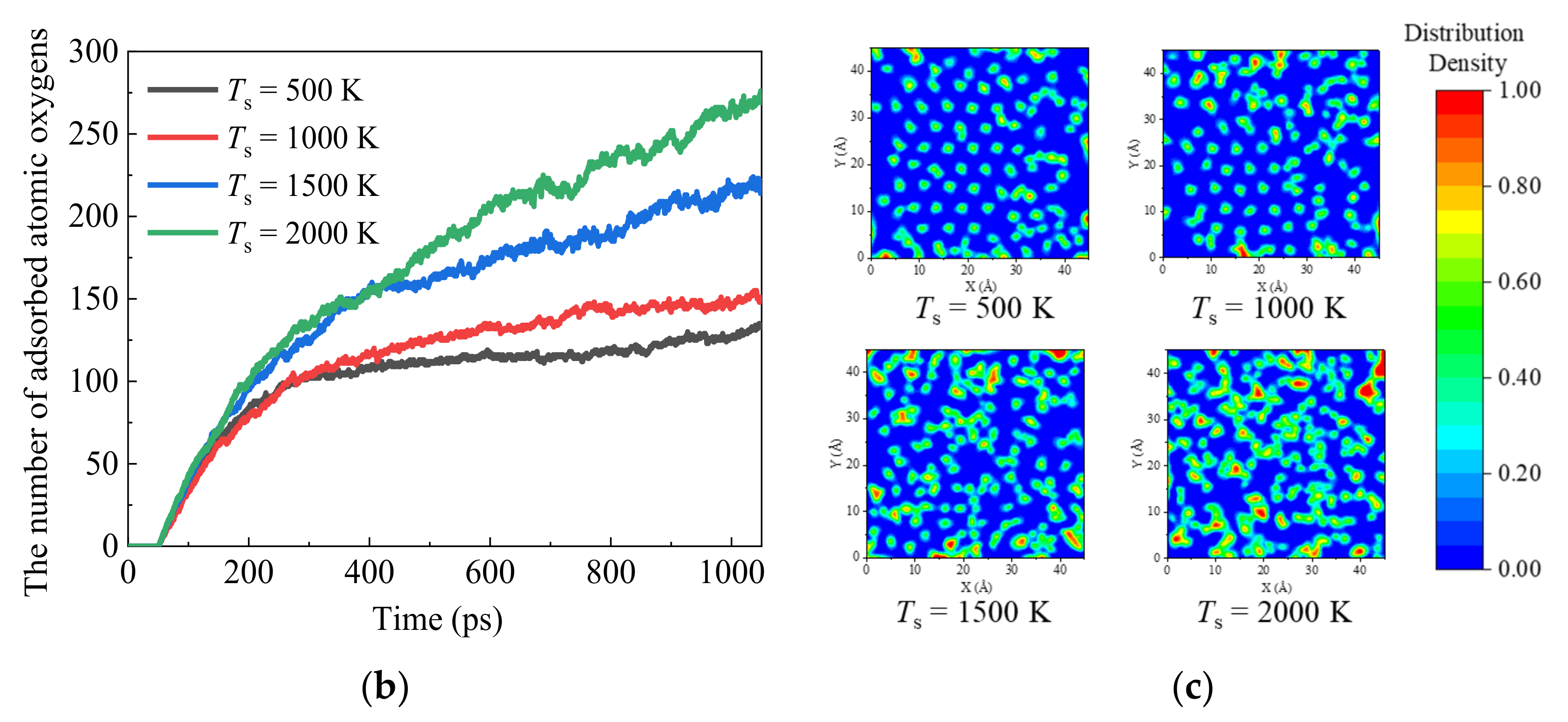
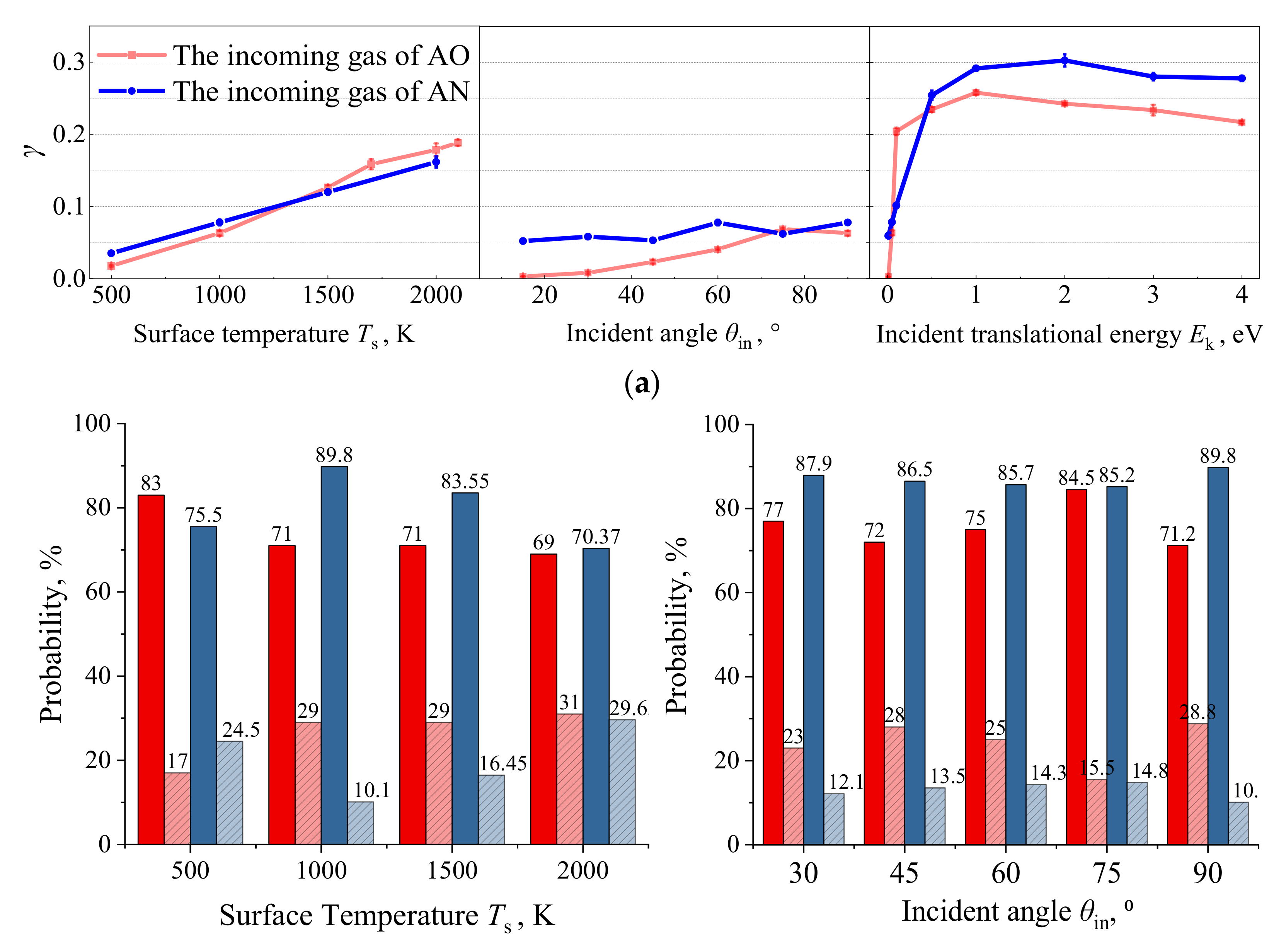
3.1. Validation and Temperature-Dependent Silica Surface Catalysis Characteristics
3.2. Effects of AO Incident Angle and Translational Energy
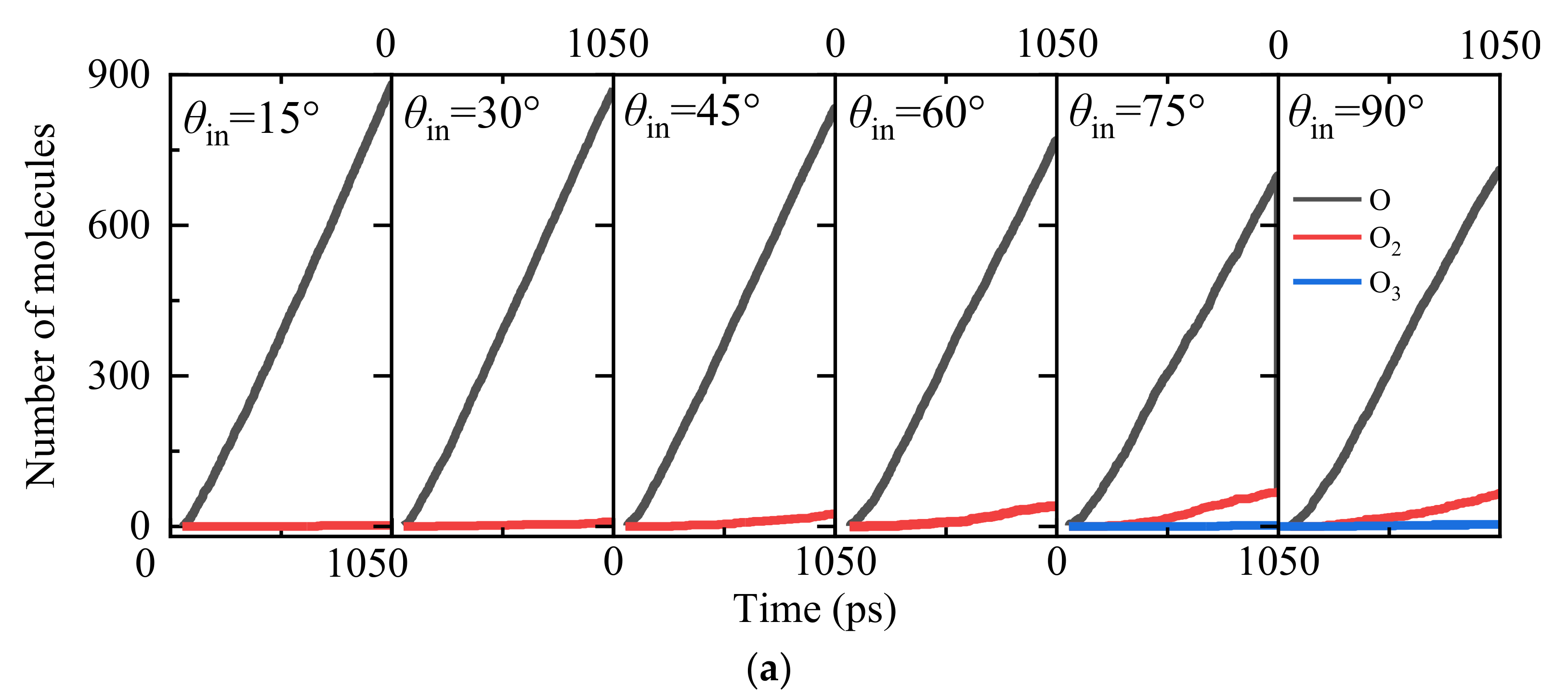
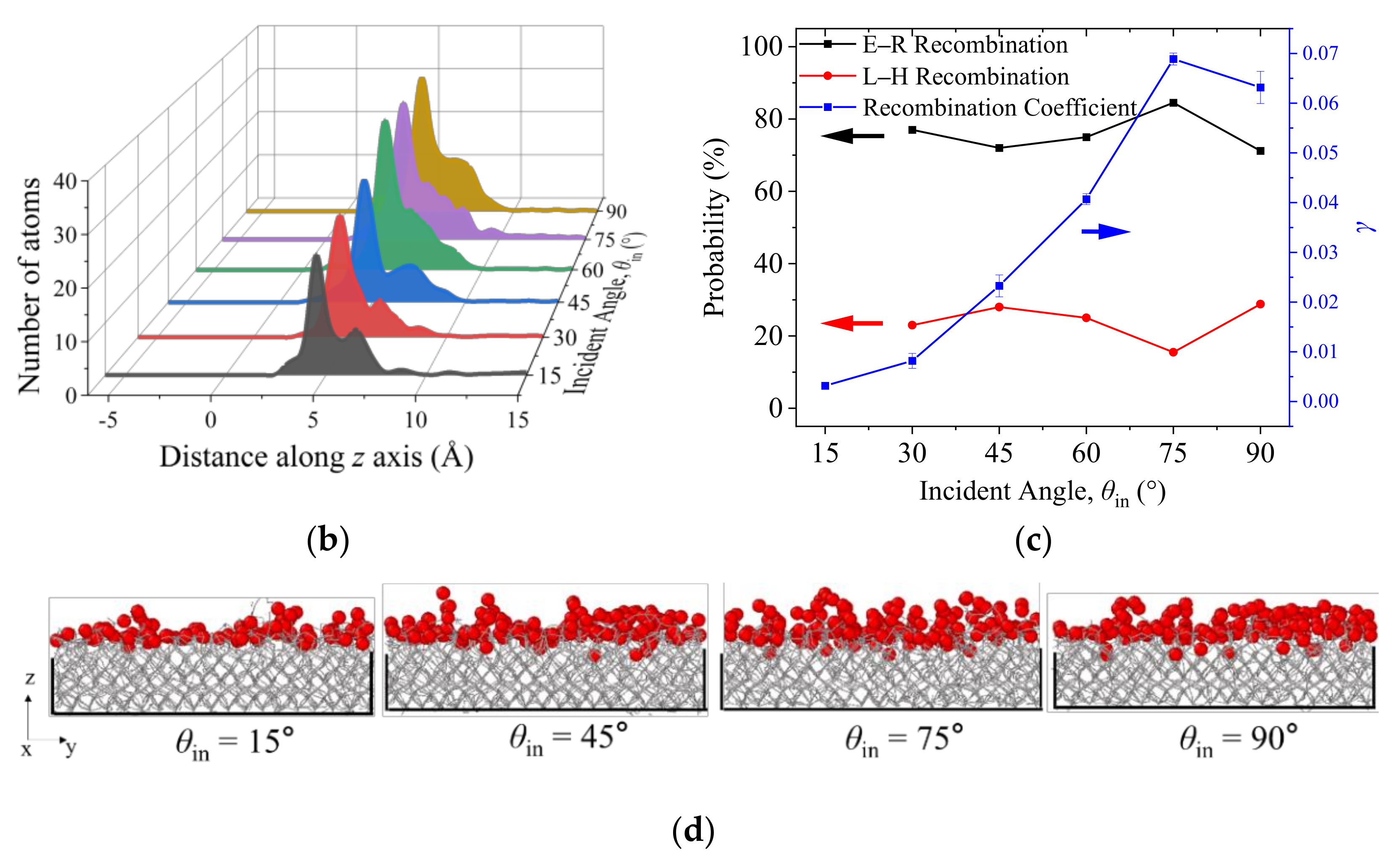
3.2.1. The Effect of AO Incident Angle
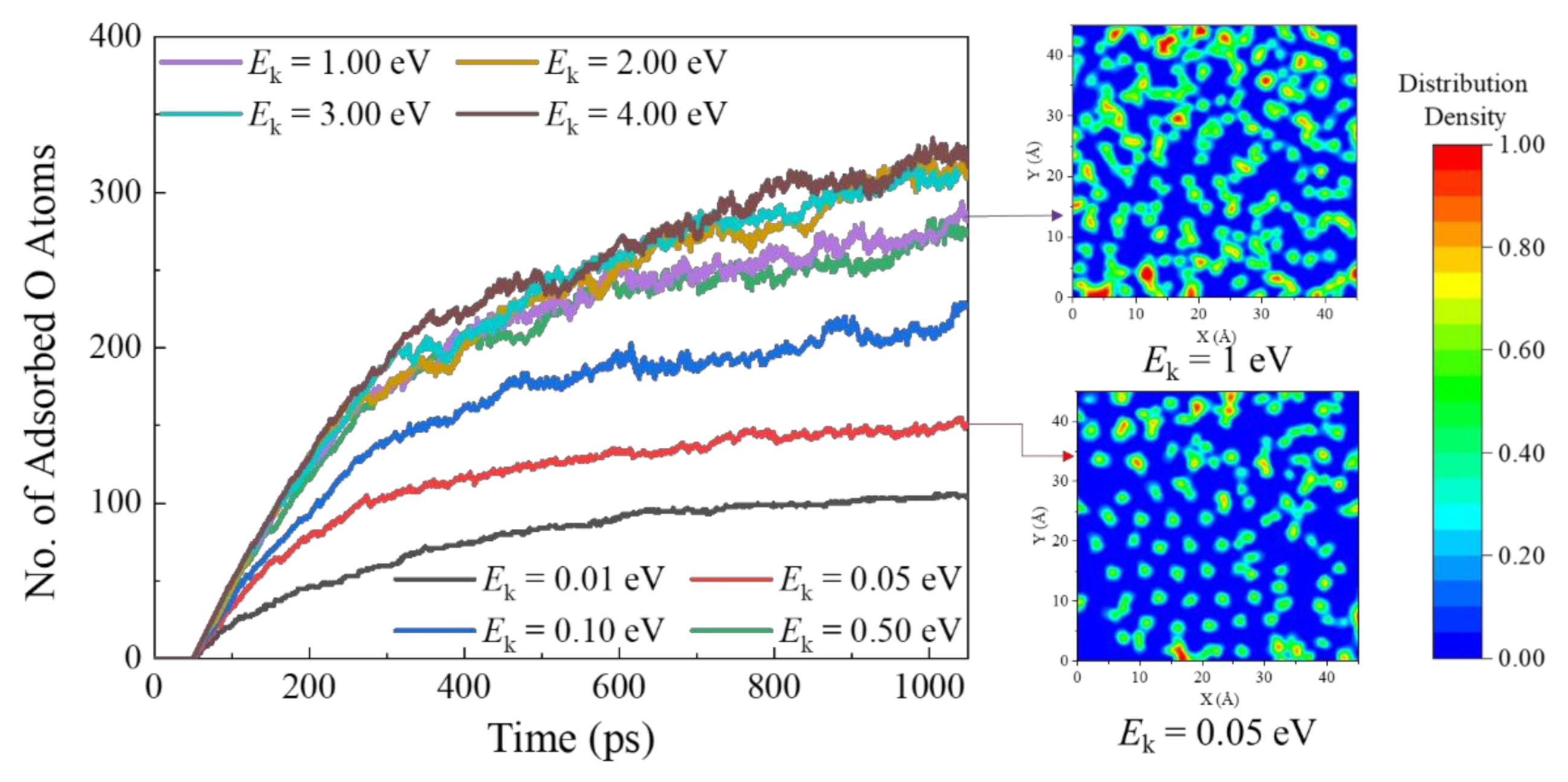
3.2.2. The Effect of Incoming AO Translational Energy
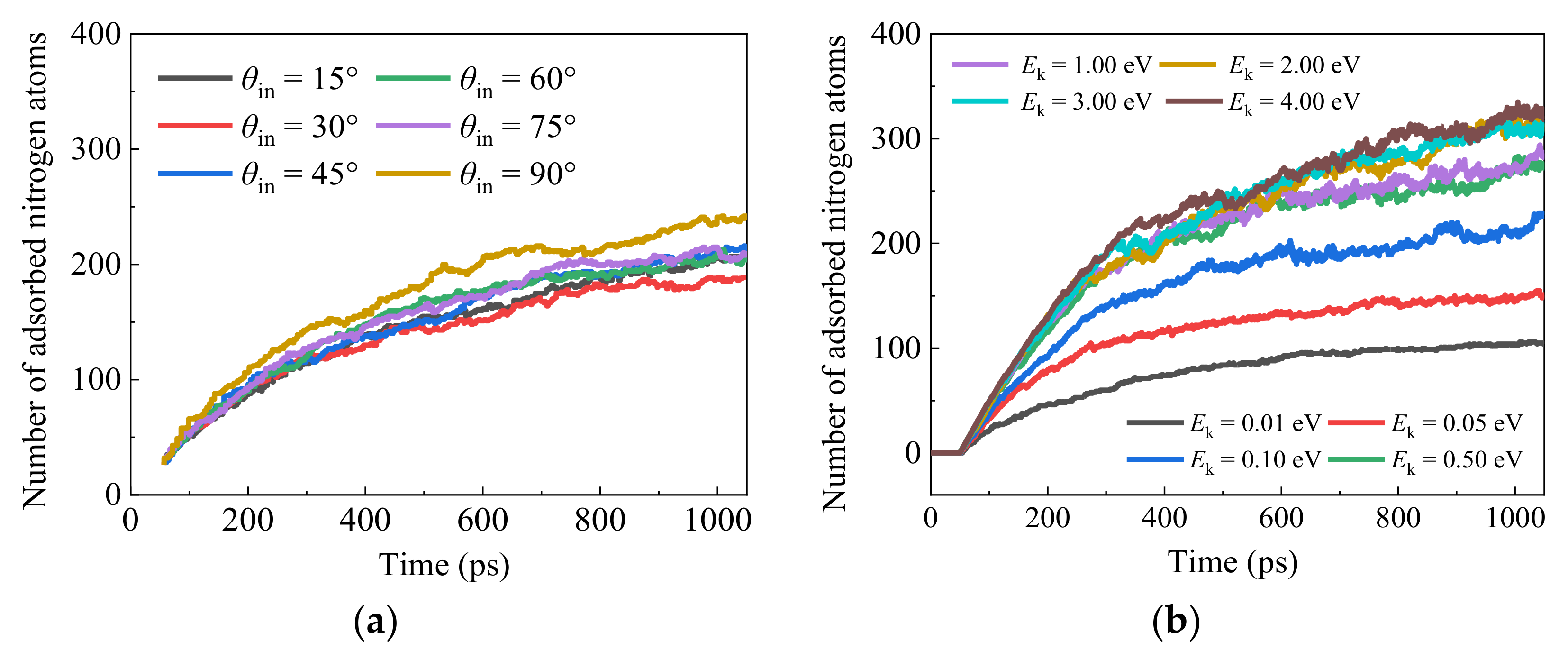
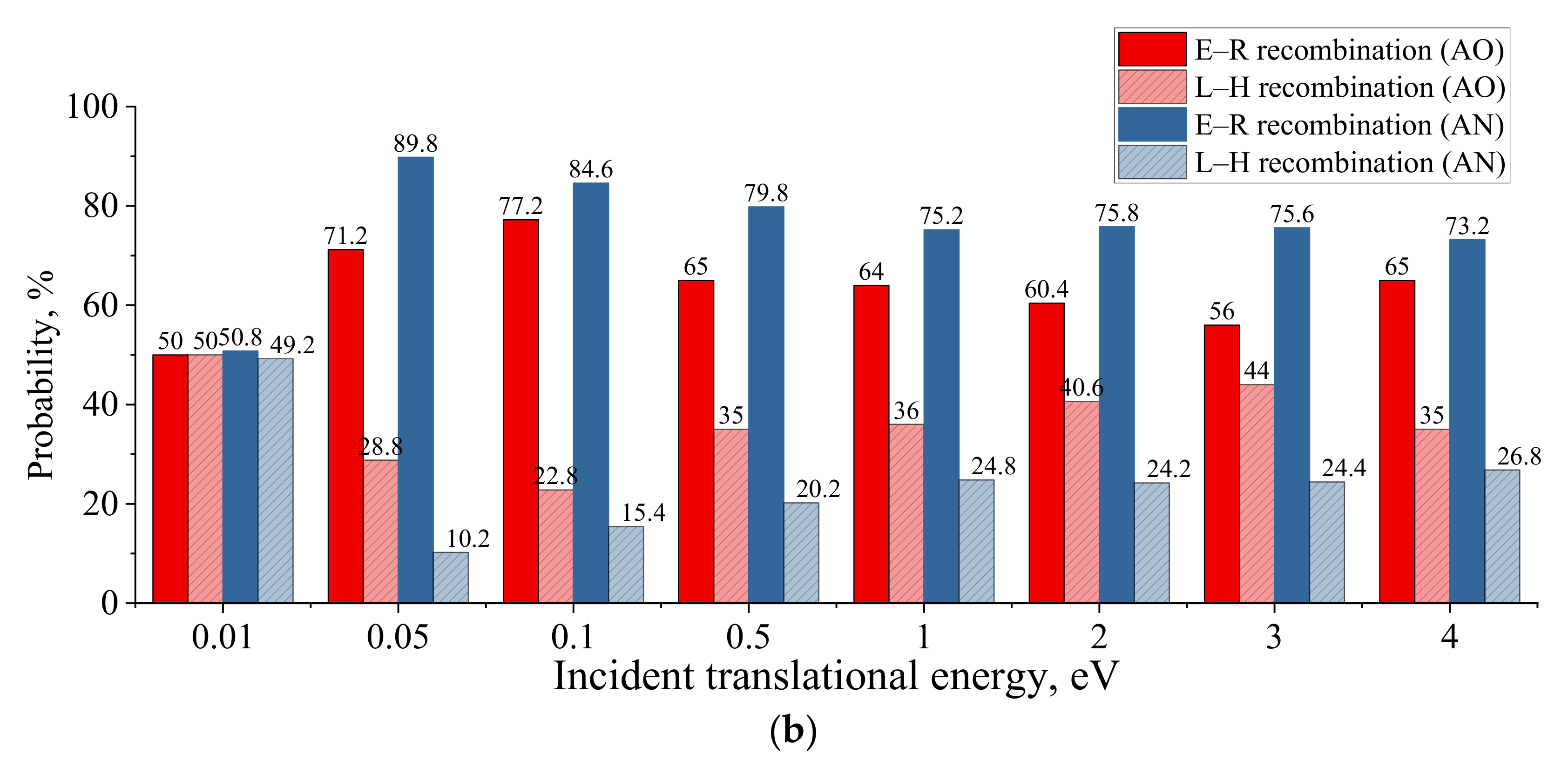
3.3. The AN Gas Effect on the Silica Surface Catalysis
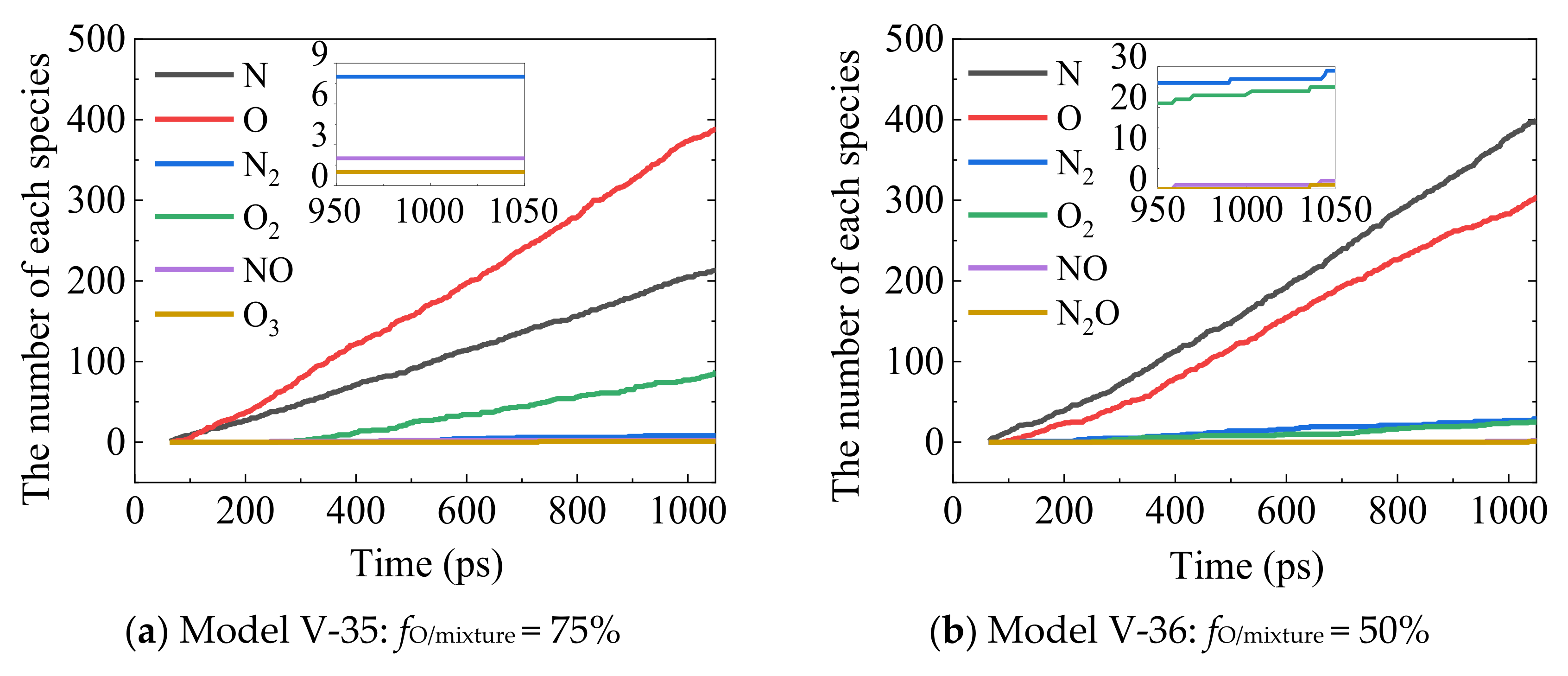
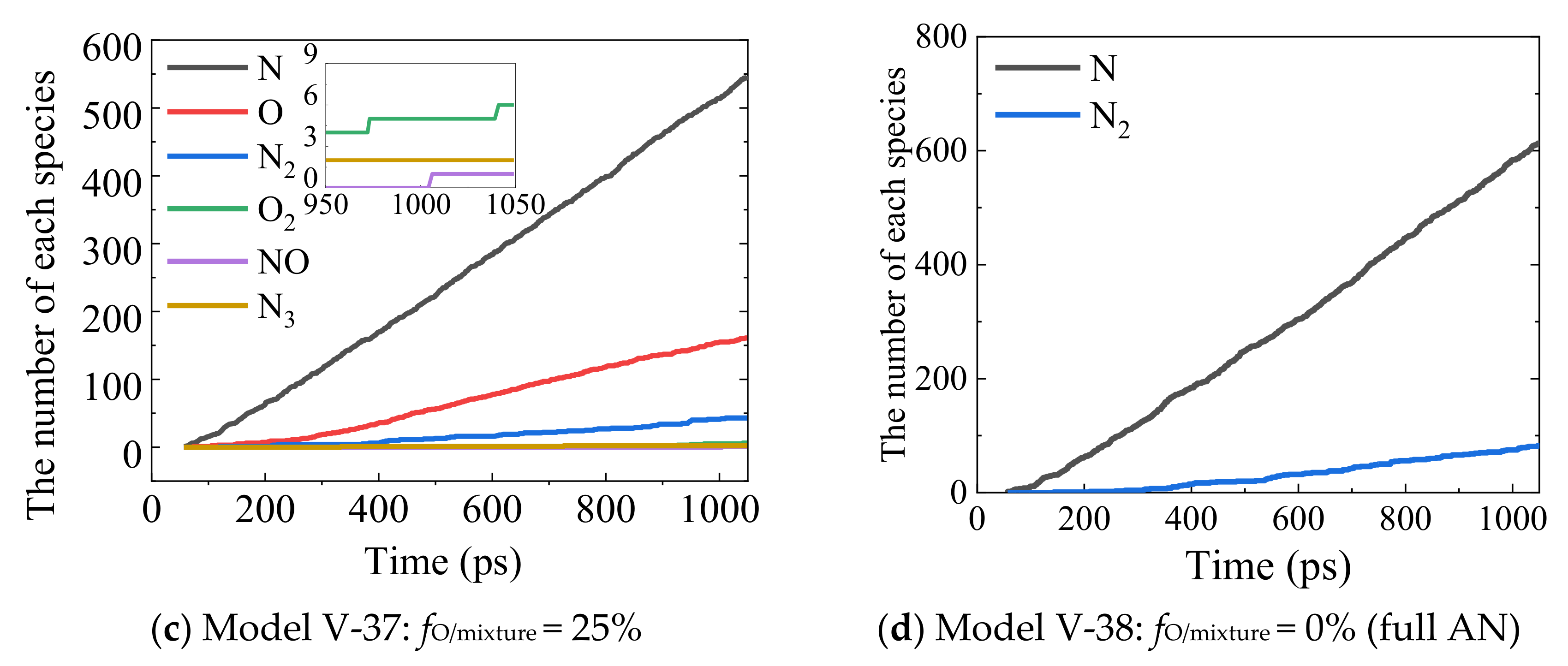
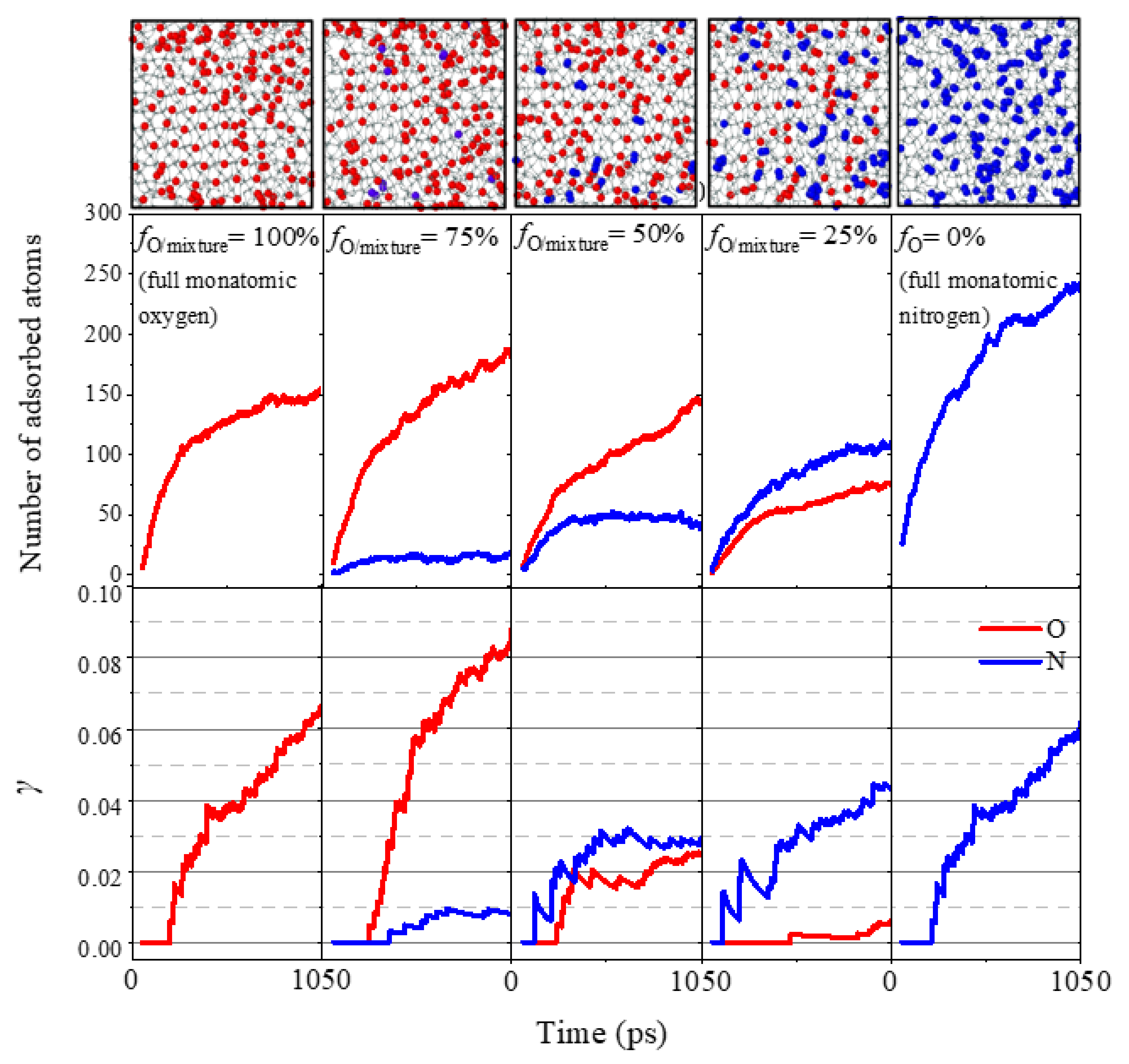
3.4. Effects of Bicomponent AO/AN Gas Mixture
4. Conclusions
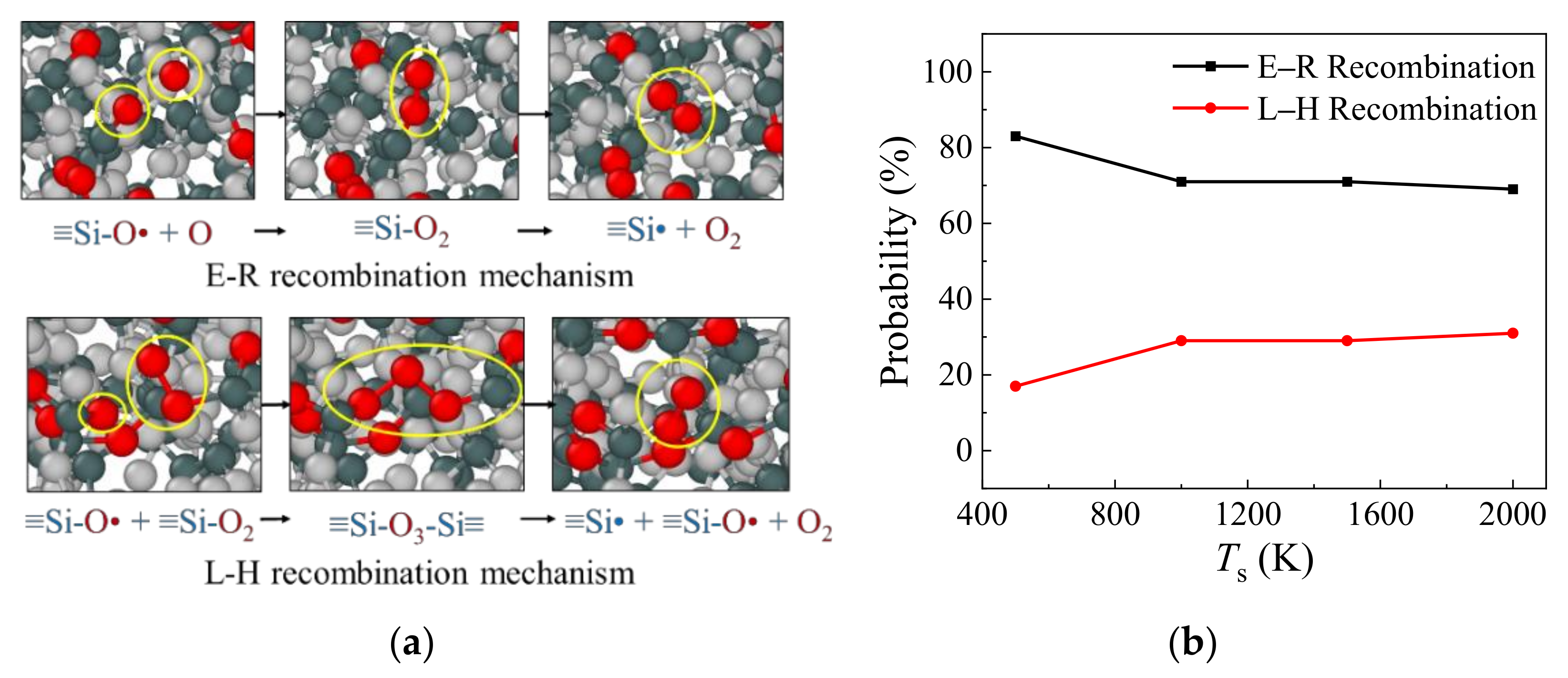
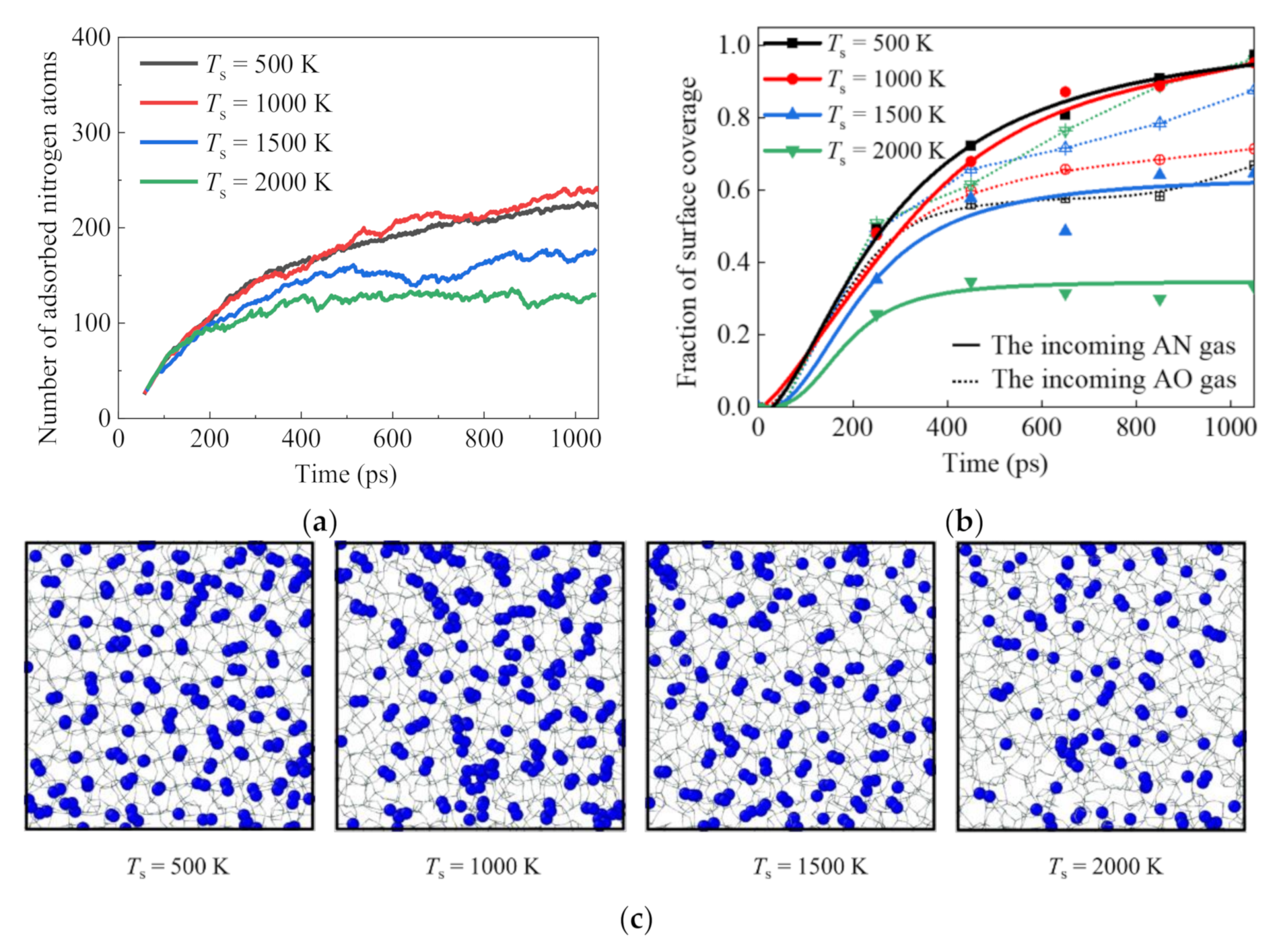
- With the increase in the silica surface temperature Ts from 500 K to 2000 K, the surface coverage fraction of AO becomes greater due to the increased number of active sites on the silica surface, which enhances the percentages of L–H recombination reactions with diffusion recombination mechanisms for molecular oxygen formation.
- The catalysis recombination coefficient γ under hyperthermal AO impacts is sensitive to the solid surface temperature, the gas angles of attack, and the incidence translational energy. A non-linear increasing pattern of γO2 with the increase in AO incident angle θin and translational energy Ek is observed. There is an optimal relationship between the incident angle and translational energy to achieve the highest surface catalytic recombination rate. High vertical-component kinetic energy may lead AO to directly bounce away from the surface without participating in the surface catalysis recombination reactions.
- Compared with the surface catalysis under hyperthermal AO impact, the AN surface adsorption fraction shows an inverse trend with the increase in surface temperature, which suggests the potential inadequacy of the traditional proportional-relationship assumption between the surface adsorption concentration and the surface catalysis recombination coefficient for other species, except the AO.
- For bi-component AO/AN gas mixtures, the corresponding surface catalysis coefficient is not the simple superposition of the effects of individual gases but is affected by both the intramolecular bond energies of O2 and N2 and intermolecular energies (e.g., Si/N, Si/O), potentially varying the surface adsorption fraction and distribution.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Weidner, J.P. Hypersonic propulsion-Breaking the thermal barrier. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 1993, 207, 47–59. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, R.; Zhang, G.; Jiang, P.; Song, J.; Chu, X. Toughening mechanism of thermal barrier coatings. Int. J. Thermophys. 2021, 42, 69. [Google Scholar] [CrossRef]
- Zhong, X. High-order finite-difference schemes for numerical simulation of hypersonic boundary-layer transition. J. Comput. Phys. 1998, 144, 662–709. [Google Scholar] [CrossRef] [Green Version]
- Takahashi, Y.; Yamada, K. Aerodynamic heating of inflatable aeroshell in orbital reentry. Acta Astronaut. 2018, 152, 437–448. [Google Scholar] [CrossRef]
- Mankodi, T.K.; Myong, R. Quasi-classical trajectory-based non-equilibrium chemical reaction models for hypersonic air flows. Phys. Fluids A 2019, 31, 106102. [Google Scholar] [CrossRef]
- Borrelli, R.; Riccio, A.; Tescione, D.; Gardi, R.; Marino, G. Thermo-structural behaviour of an UHTC made nose cap of a reentry vehicle. Acta Astronaut. 2009, 65, 442–456. [Google Scholar] [CrossRef]
- Maus, J.; Griffith, B.; Szema, K.; Best, J. Hypersonic Mach number and real gas effects on Space Shuttle Orbiteraerodynamics. J. Spacecr. Rocket. 1984, 21, 136–141. [Google Scholar] [CrossRef]
- Satheesh Chandran, M.; Sunitha, K.; Uday Bhaskar, N.; Santhosh Kumar, K.; Sreenivas, N.; Mathew, D. Low Density Syntactic Foam Composites as Ablative TPS Material for High Heat Flux Conditions for Reentry Missions. Trans. Indian Natl. Acad. Eng. 2021, 6, 153–158. [Google Scholar] [CrossRef]
- Barbato, M.; Giordano, D.; Muylaert, J.; Bruno, C.R. Comparison of catalytic wall conditions for hypersonic flow. J. Spacecr. 1996, 33, 620–627. [Google Scholar] [CrossRef]
- Behrens, B.; Mueller, M. Technologies for thermal protection systems applied on re-usable launcher. Acta Astronaut. 2004, 55, 529–536. [Google Scholar] [CrossRef]
- Yi, J.; He, X.; Sun, Y.; Li, Y. Electron beam-physical vapor deposition of SiC/SiO2 high emissivity thin film. Appl. Surf. Sci. 2007, 253, 4361–4366. [Google Scholar] [CrossRef]
- Kim, I.; Lee, S.; Kim, J.G.; Park, G. Analysis of nitrogen recombination activity on silicon dioxide with stagnation heat-transfer. Acta Astronaut. 2020, 177, 386–397. [Google Scholar] [CrossRef]
- Paterna, D.; Monti, R.; Savino, R.; Esposito, A. Experimental and numerical investigation of Martian atmosphere entry. J. Spacecr. Rocket. 2002, 39, 227–236. [Google Scholar] [CrossRef]
- Serpico, M.; Monti, R.; Savino, R. Heat flux on partially catalytic surfaces in hypersonic flows. J. Spacecr. Rocket. 1998, 35, 9–15. [Google Scholar] [CrossRef]
- Armenise, I.; Barbato, M.; Capitelli, M.; Kustova, E.; Transfer, H. State-to-State Catalytic Models, Kinetics, and Transport in Hypersonic Boundary Layers. J. Thermophys. Heat Transf. 2006, 20, 465–476. [Google Scholar] [CrossRef]
- Nasuti, F.; Barbato, M.; Bruno, C. Material-dependent catalytic recombination modeling for hypersonic flows. J. Thermophys. Heat Transf. 1996, 10, 131–136. [Google Scholar] [CrossRef]
- Herdrich, G.; Auweter-Kurtz, M.; Lhle, S.; Pidan, S.; Laux, T. Oxidation Behavior of SiC-based Thermal Protection System Materials Using Newly Developed Probe Techniques. In Proceedings of the 37th AIAA Thermophysics Conference, Portland, OR, USA, 28 June–1 July 2004. [Google Scholar]
- Park, C.; Jaffe, R.L.; Partridge, H. Chemical-kinetic parameters of hyperbolic earth entry. J. Thermophys. Heat Transf. 2001, 15, 76–90. [Google Scholar] [CrossRef]
- Li, K.; Liu, J.; Liu, W. A new surface catalytic model for silica-based thermal protection material for hypersonic vehicles. Chin. J. Aeronaut. 2015, 28, 1355–1361. [Google Scholar] [CrossRef] [Green Version]
- Yang, X.; Gui, Y.; Tang, W.; Du, Y.; Wei, D.; Xiao, G.; Liu, L. Surface chemical effects on hypersonic nonequilibrium aeroheating in dissociated carbon–oxygen mixture. J. Spacecr. Rocket. 2018, 55, 687–697. [Google Scholar] [CrossRef]
- Scott, C. Catalytic recombination of nitrogen and oxygen on iron-cobalt-chromia spinel. In Proceedings of the 21st Aerospace Sciences Meeting, Reno, NV, USA, 10–13 January 1983. [Google Scholar]
- Carandente, V.; Savino, R.; Esposito, A.; Zuppardi, G.; Caso, V. Experimental and numerical simulation, by an arc-jet facility, of hypersonic flow in Titan’s atmosphere. Exp. Therm. Fluid Sci. 2013, 48, 97–101. [Google Scholar] [CrossRef]
- Balat-Pichelin, M.; Badie, J.M.; Berjoan, R.; Boubert, P. Recombination coefficient of atomic oxygen on ceramic materials under earth re-entry conditions by optical emission spectroscopy. Chem. Phys. 2003, 291, 181–194. [Google Scholar] [CrossRef]
- Dickens, P.G.; Sutcliffe, M.B. Recombination of oxygen atoms on oxide surfaces. Part 1—Activation energies of recombination. Trans. Faraday Soc. 1964, 60, 1272–1285. [Google Scholar] [CrossRef]
- Stewart, D. Determination of surface catalytic efficiency for thermal protection materials-Room temperature to their upper use limit. In Proceedings of the 31st Thermophysics Conference, New Orleans, LA, USA, 18–20 June 1996. [Google Scholar]
- Kim, Y.C.; Boudart, M. Recombination of oxygen, nitrogen, and hydrogen atoms on silica: Kinetics and mechanism. Langmuir 1991, 7, 2999–3005. [Google Scholar] [CrossRef]
- Carleton, K.L.; Marinelli, W.J. Spacecraft thermal energy accommodation from atomic recombination. J. Thermophys. Heat Transf. 1992, 6, 650–655. [Google Scholar] [CrossRef]
- Rutigliano, M.; Cacciatore, M. Recombination of Oxygen Atoms on Silica Surface: New and More Accurate Results. J. Thermophys. Heat Transf. 2015, 30, 247–250. [Google Scholar] [CrossRef]
- Miller, J.H.; Tennehill, J.C.; Wadawadigi, G.; Edwards, T.A.; Lawrence, S.L. Computation of hypersonic flows with finite catalytic walls. J. Thermophys. Heat Transf. 1995, 9, 486–493. [Google Scholar] [CrossRef]
- Nguyen, V.T.; Fang, T.H. Material removal and wear mechanism in abrasive polishing of SiO2/SiC using molecular dynamics. Ceram. Int. 2020, 46, 21578–21595. [Google Scholar] [CrossRef]
- Chen, J.; Shi, J.; Chen, Z.; Zhang, M.; Peng, W.; Fang, L.; Sun, K.; Han, J. Mechanical properties and deformation behaviors of surface-modified silicon: A molecular dynamics study. J. Mater. Sci. 2019, 54, 3096–3110. [Google Scholar] [CrossRef]
- Li, W.; Garofalini, S.H. Molecular dynamics simulation of lithium diffusion in Li2O-Al2O3-SiO2 glasses. Solid State Ion. 2004, 166, 365–373. [Google Scholar] [CrossRef]
- Van Duin, A.C.; Dasgupta, S.; Lorant, F.; Goddard, W.A. ReaxFF: A Reactive Force Field for Hydrocarbons. J. Phys. Chem. A 2001, 105, 9396–9409. [Google Scholar] [CrossRef] [Green Version]
- Chenoweth, K.; Van Duin, A.C.; Goddard, W.A. ReaxFF Reactive Force Field for Molecular Dynamics Simulations of Hydrocarbon Oxidation. J. Phys. Chem. A 2008, 112, 1040–1053. [Google Scholar] [CrossRef] [Green Version]
- Raymand, D.; van Duin, A.C.; Baudin, M.; Hermansson, K. A reactive force field (ReaxFF) for zinc oxide. Surf. Sci. 2008, 602, 1020–1031. [Google Scholar] [CrossRef]
- Wang, N.; Feng, Y.; Guo, X. Atomistic mechanisms study of the carbonation reaction of CaO for high-temperature CO2 capture. Appl. Surf. Sci. 2020, 532, 147425. [Google Scholar] [CrossRef]
- Feng, M.; Jiang, X.Z.; Luo, K.H. A reactive molecular dynamics simulation study of methane oxidation assisted by platinum/graphene-based catalysts. Proc. Combust. Inst. 2019, 37, 5473–5480. [Google Scholar] [CrossRef]
- Hasan, R.M.M.; Politano, O.; Luo, X. ReaxFF molecular dynamics simulation study of nanoelectrode lithography oxidation process on silicon (100) surface. Appl. Surf. Sci. 2019, 496, 143679. [Google Scholar] [CrossRef]
- Cui, Z.; Yao, G.; Zhao, J.; Zhang, J.; Wen, D. Atomistic-scale investigations of hyperthermal oxygen–graphene interactions via reactive molecular dynamics simulation: The gas effect. Phys. Fluids 2021, 33, 052107. [Google Scholar] [CrossRef]
- Cui, Z.; Zhao, J.; He, L.; Jin, H.; Zhang, J.; Wen, D. A reactive molecular dynamics study of hyperthermal atomic oxygen erosion mechanisms for graphene sheets. Phys. Fluids 2020, 32, 112110. [Google Scholar] [CrossRef]
- Bačová, P.; Li, W.; Behbahani, A.F.; Burkhart, C.; Polińska, P.; Doxastakis, M.; Harmandaris, V. Coupling between Polymer Conformations and Dynamics Near Amorphous Silica Surfaces: A Direct Insight from Atomistic Simulations. Nanomaterials 2021, 11, 2075. [Google Scholar] [CrossRef]
- Gai, L.; Shin, Y.K.; Raju, M.; Van Duin, A.C.T.; Raman, S. Atomistic Adsorption of Oxygen and Hydrogen on Platinum Catalysts by Hybrid Grand Canonical Monte Carlo/Reactive Molecular Dynamics. J. Phys. Chem. C 2016, 120, 9780–9793. [Google Scholar] [CrossRef]
- Jeon, B.; Sankaranarayanan, S.K.R.S.; Van Duin, A.C.T.; Ramanathan, S. Influence of surface orientation and defects on early-stage oxidation and ultrathin oxide growth on pure copper. Philos. Mag. 2011, 91, 4073–4088. [Google Scholar] [CrossRef]
- Valentini, P.; Schwartzentruber, T.E.; Cozmuta, I. Molecular dynamics simulation of O2 sticking on Pt(111) using the ab initio based ReaxFF reactive force field. J. Chem. Phys. 2010, 133, 084703. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Liu, Y.; Chen, X.; Zhai, Z.; Xu, F.; Liu, Y. Micromechanism of oxygen transport during initial stage oxidation in Si(100) surface: A ReaxFF molecular dynamics simulation study. Appl. Surf. Sci. 2017, 406, 178–185. [Google Scholar] [CrossRef]
- Cao, H.; Pamungkas, M.A.; Kim, B.H.; Lee, K.R. A Molecular Dynamics Simulation Study on the Initial Stage of Si(001) Oxidation Under Biaxial Strain. J. Nanosci. Nanotechnol. 2013, 13, 1074–1077. [Google Scholar] [CrossRef] [PubMed]
- Newsome, D.A.; Sengupta, D.; Foroutan, H.; Russo, M.F.; Van Duin, A.C.T. Oxidation of Silicon Carbide by O2 and H2O: A ReaxFF Reactive Molecular Dynamics Study, Part I. J. Phys. Chem. C 2012, 116, 16111–16121. [Google Scholar] [CrossRef]
- Gamallo, P.; Prats, H.; Sayos, R. ReaxFF molecular dynamics simulations of CO collisions on an O-preadsorbed silica surface. J. Mol. Model. 2014, 20, 2160. [Google Scholar] [CrossRef]
- Mao, Q.; Van Duin, A.C.T.; Luo, K.H. Investigation of methane oxidation by palladium-based catalyst via ReaxFF Molecular Dynamics simulation. Proc. Combust. Inst. 2017, 36, 4339–4346. [Google Scholar] [CrossRef] [Green Version]
- Khalilov, U.; Pourtois, G.; Van Duin, A.C.T.; Neyts, E.C. Hyperthermal Oxidation of Si(100)2x1 Surfaces: Effect of Growth Temperature. J. Phys. Chem. C 2012, 116, 8649–8656. [Google Scholar] [CrossRef]
- Khalilov, U.; Pourtois, G.; Huygh, S.; Van Duin, A.C.T.; Neyts, E.C.; Bogaerts, A. New Mechanism for Oxidation of Native Silicon Oxide. J. Phys. Chem. C 2013, 117, 9819–9825. [Google Scholar] [CrossRef]
- Khalilov, U.; Neyts, E.C.; Pourtois, G.; Van Duin, A.C.T. Can We Control the Thickness of Ultrathin Silica Layers by Hyperthermal Silicon Oxidation at Room Temperature? J. Phys. Chem. C 2011, 115, 24839–24848. [Google Scholar] [CrossRef]
- Yang, Y.; Peddakotla, S.A.; Kumar, R.; Park, G. Microscopic Analysis of Catalytic Recombination Process. In Proceedings of the 2019 World Congress on Advances in Nano, Bio, Robotics and Energy (ANBRE19), Jeju Island, Korea, 17–21 September 2019. [Google Scholar]
- Cozmuta, I. Molecular mechanisms of gas surface interactions in hypersonic flow. In Proceedings of the 39th AIAA Thermophysics Conference, Miami, FL, USA, 25–28 June 2007. [Google Scholar]
- Norman, P.E. Modeling Air-Silica Surface Catalysis in Hypersonic Environments Using ReaxFF Molecular Dynamics. Master’s Thesis, University of Minnesota, Minneapolis, MN, USA, May 2010. [Google Scholar]
- Norman, P.; Schwartzentruber, T.; Cozmuta, I. Modeling Air-SiO2 Surface Catalysis under Hypersonic Conditions with ReaxFF Molecular Dynamics. In Proceedings of the 10th AIAA/ASME Joint Thermophysics and Heat Transfer Conference, Chicago, IL, USA, 28 June–1 July 2010. [Google Scholar]
- Norman, P.; Schwartzentruber, T.E.; Leverentz, H.; Luo, S.; Meana-Pañeda, R.; Paukku, Y.; Truhlar, D.G. The structure of silica surfaces exposed to atomic oxygen. J. Phys. Chem. C 2013, 117, 9311–9321. [Google Scholar] [CrossRef]
- Norman, P.; Schwartzentruber, T. A Finite-Rate Model for Oxygen-Silica Catalysis through Computational Chemistry Simulation. In Proceedings of the 28th International Symposium on Rarefied Gas Dynamics, Zaragoza, Spain, 9–13 July 2012. [Google Scholar]
- Cui, Z.; Jin, Z.; Yao, G.; Jun, Z.; Li, Z.; Tang, Z.; Wen, D. Competing effects of surface catalysis and ablation in hypersonic reentry aerothermodynamic environment. Chin. J. Aeronaut. 2021; in press. [Google Scholar] [CrossRef]
- Plimpton, S.; Crozier, P.; Thompson, A. LAMMPS-large-scale atomic/molecular massively parallel simulator. Sandia Natl. Lab. 2007, 18, 43. [Google Scholar]
- Kulkarni, A.D.; Truhlar, D.G.; Goverapet Srinivasan, S.; Van Duin, A.C.; Norman, P.; Schwartzentruber, T.E. Oxygen interactions with silica surfaces: Coupled cluster and density functional investigation and the development of a new ReaxFF potential. J. Phys. Chem. C 2013, 117, 258–269. [Google Scholar] [CrossRef]
- Pastor, R.W.; Brooks, B.R.; Szabo, A. An analysis of the accuracy of Langevin and molecular dynamics algorithms. Mol. Phys. 1988, 65, 1409–1419. [Google Scholar] [CrossRef]
- Deutschmann, O.; Riedel, U.; Warnatz, J. Modeling of nitrogen and oxygen recombination on partial catalytic surfaces. J. Heat Transf. 1995, 117, 495–501. [Google Scholar] [CrossRef]






| Type | Model | Surface Temperature, Ts | Incident Angle, θin | Incident Translational Energy, Ek | Incident Components, fO/mixture |
|---|---|---|---|---|---|
| Benchmarking Case | 1 (Standard) | 1000 K | 90° | 0.05 eV | 100%—full AO |
| Set I: Effect of silica surface temperature Ts | 2–4 | 500 K, 1500 K, and 2000 K | 90° | 0.05 eV | 100%—full AO |
| Set II: Effect of AO incident angle θin | 5–9 | 1000 K | 15°, 30°, 45°, 60°, and 75° | 0.05 eV | 100%—full AO |
| Set III: Effect of AO incident translational energy Ek | 10–16 | 1000 K | 90° | 0.01, 0.10, 0.50, 1.00, 2.00, 3.00, and 4.00 eV | 100%—full AO |
| Set IV: Effect of Ts, θin, and Ek on silica surface catalysis performance with AN bombardment | 17–20 | 500 K, 1000 K, 1500 K, and 2000 K | 90° | 0.05 eV | full AN |
| 21–26 | 1000 K | 15°, 30°, 45°, 60° 75°, and 90° | 0.05 eV | full AN | |
| 27–34 | 1000 K | 90° | 0.01, 0.05, 0.10, 0.50, 1.00, 2.00, 3.00, and 4.00 eV | full AN | |
| Set V: Bicomponent monatomic gas mixture effect | 35–38 | 1000 K | 90° | 0.05 eV | 75% (AO: 75%; AN: 25%), 50% (AO: 50%; AN: 50%), 25% (AO: 25%; AN: 75%) and 0% (AO: 0%; AN: 100%) |
| Reaction | Mechanism |
|---|---|
| O + (s) → O(s) | Adsorption |
| N + (s) → N(s) | |
| O(s) → O + (s) | Desorption |
| N(s) → N + (s) | |
| O2(s) → O2 + (s) | |
| N2(s) → N2 + (s) | |
| NO(s) → NO + (s) | |
| O + O(s) → O2 + (s) | E–R mechanism |
| N + N(s) → N2 + (s) | |
| N + O(s) → NO + (s) | |
| O + N(s) → NO + (s) | |
| O(s) + O(s) → O2 + (s) | L–H mechanism |
| N(s) + N(s) → N2 + (s) | |
| N(s) + O(s) → NO + (s) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, L.; Cui, Z.; Sun, X.; Zhao, J.; Wen, D. Sensitivity Analysis of the Catalysis Recombination Mechanism on Nanoscale Silica Surfaces. Nanomaterials 2022, 12, 2370. https://doi.org/10.3390/nano12142370
He L, Cui Z, Sun X, Zhao J, Wen D. Sensitivity Analysis of the Catalysis Recombination Mechanism on Nanoscale Silica Surfaces. Nanomaterials. 2022; 12(14):2370. https://doi.org/10.3390/nano12142370
Chicago/Turabian StyleHe, Lichao, Zhiliang Cui, Xiangchun Sun, Jin Zhao, and Dongsheng Wen. 2022. "Sensitivity Analysis of the Catalysis Recombination Mechanism on Nanoscale Silica Surfaces" Nanomaterials 12, no. 14: 2370. https://doi.org/10.3390/nano12142370
APA StyleHe, L., Cui, Z., Sun, X., Zhao, J., & Wen, D. (2022). Sensitivity Analysis of the Catalysis Recombination Mechanism on Nanoscale Silica Surfaces. Nanomaterials, 12(14), 2370. https://doi.org/10.3390/nano12142370








