Modeling and X-ray Analysis of Defect Nanoclusters Formation in B4C under Ion Irradiation
Abstract
:1. Introduction
2. Experimental Section
3. Swift Heavy Ion Irradiation: X-ray Diffraction
O• + B → [BO]•
[BO]• + O2 → [BO2]• + O•
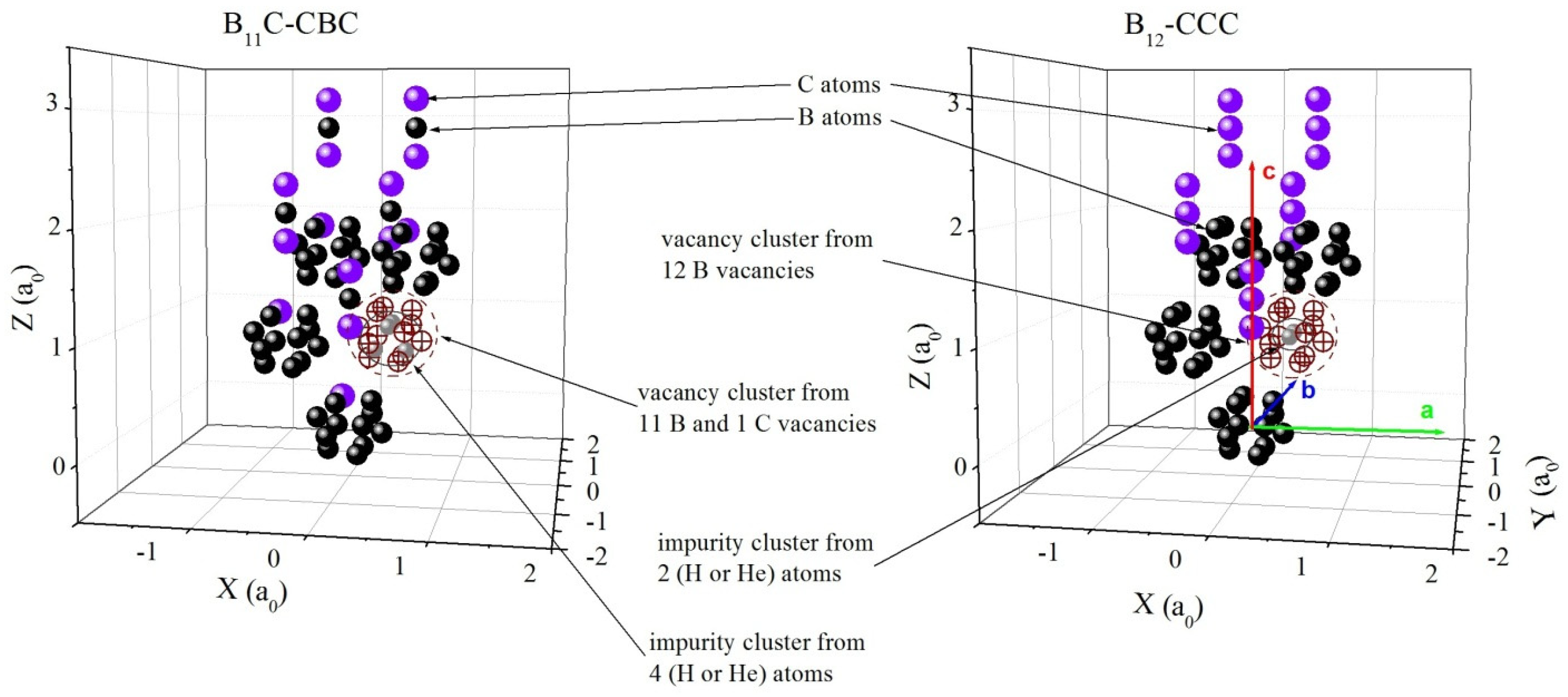
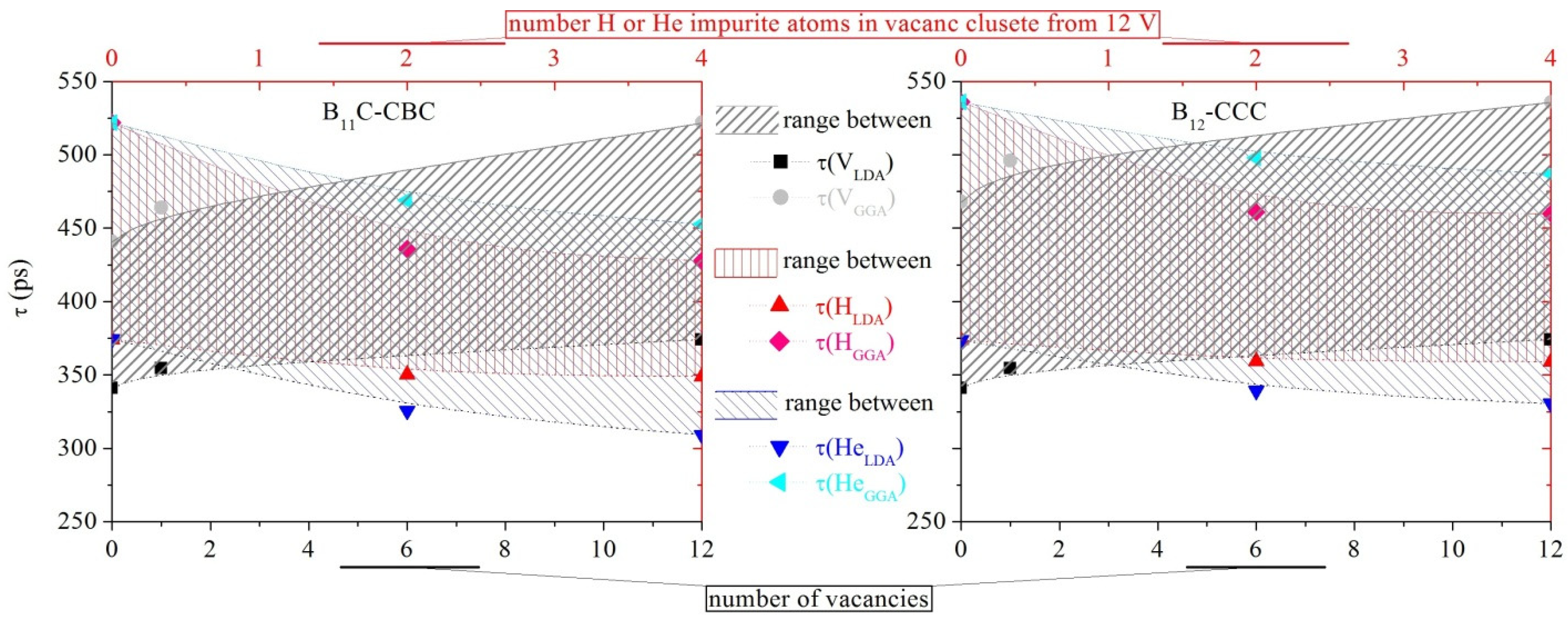
4. Model Calculations of PLT
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Luo, X.; Yang, J.; Liu, H.; Wu, X.; Wang, Y.; Ma, Y.; Wei, S.H.; Gong, X.; Xiang, H. Predicting two-dimensional boron-carbon compounds by the global optimization method. J. Am. Chem. Soc. 2011, 133, 16285–16290. [Google Scholar] [CrossRef] [PubMed]
- Arita, Y.; Nishi, Y.; Amaya, M.; Matsui, T. Isotope effects on thermal diffusivity of boron carbide. Thermochim. Acta 2000, 352–353, 39–42. [Google Scholar] [CrossRef]
- Gosset, D. Basic properties of boron carbide. Compr. Nucl. Mater. Second Ed. 2020, 7, 539–553. [Google Scholar] [CrossRef]
- Thévenot, F. Boron carbide—A comprehensive review. J. Eur. Ceram. Soc. 1990, 6, 205–225. [Google Scholar] [CrossRef]
- Pipon, Y.; Victor, G.; Moncoffre, N.; Gutierrez, G.; Miro, S.; Douillard, T.; Rapaud, O.; Pradeilles, N.; Sainsot, P.; Toulhoat, N.; et al. Structural modifications of boron carbide irradiated by swift heavy ions. J. Nucl. Mater. 2021, 546, 152737. [Google Scholar] [CrossRef]
- Domnich, V.; Reynaud, S.; Haber, R.A.; Chhowalla, M. Boron carbide: Structure, properties, and stability under stress. J. Am. Ceram. Soc. 2011, 94, 3605–3628. [Google Scholar] [CrossRef]
- Subramanian, C.; Suri, A.; Murthy, T.S.R.C. Development of Boron-based materials for nuclear applications. BARC Newsletter 2010, 45, 14–22. [Google Scholar]
- Raucoules, R.; Vast, N.; Betranhandy, E.; Sjakste, J. Mechanical properties of icosahedral boron carbide explained from first principles. Phys. Rev. B 2011, 84, 014112. [Google Scholar] [CrossRef]
- Mishra, A.; Sahoo, R.K.; Singh, S.K.; Mishra, B.K. Synthesis of low carbon boron carbide powder using a minimal time processing route: Thermal plasma. J. Asian Ceram. Soc. 2015, 3, 373–376. [Google Scholar] [CrossRef]
- Gunjishima, I.; Akashi, T.; Goto, T. Thermoelectric properties of single crystalline B4C prepared by a floating zone method. Mater. Trans. 2001, 42, 1445–1450. [Google Scholar] [CrossRef]
- Trukhanov, A.V.; Turchenko, V.O.; Bobrikov, I.A.; Trukhanov, S.V.; Kazakevich, I.S.; Balagurov, A.M. Crystal structure and magnetic properties of the BaFe12−xAlxO19 (x=0.1–1.2) solid solutions. J. Magn. Magn. Mater. 2015, 393, 253–259. [Google Scholar] [CrossRef]
- Trukhanov, S.V.; Trukhanov, A.V.; Turchenko, V.A.; Kostishyn, V.G.; Panina, L.V.; Kazakevich, I.S.; Balagurov, A.M. Structure and magnetic properties of BaFe11.9In0.1O19 hexaferrite in a wide temperature range. J. Alloys Compd. 2016, 689, 383–393. [Google Scholar] [CrossRef]
- Turchenko, V.A.; Trukhanov, S.V.; Kostishin, V.G.; Damay, F.; Porcher, F.; Klygach, D.S.; Vakhitov, M.G.; Matzui, L.Y.; Yakovenko, O.S.; Bozzo, B.; et al. Impact of In3+ cations on structure and electromagnetic state of M-type hexaferrites. J. Energy Chem. 2022, 69, 667–676. [Google Scholar] [CrossRef]
- Mirzayev, M.N.; Jabarov, S.H.; Asgerov, E.B.; Mehdiyeva, R.N.; Thabethe, T.T.; Biira, S.; Tiep, N.V. Crystal structure changes and weight kinetics of silicon-hexaboride under gamma irradiation dose. Results Phys. 2018, 10, 541–545. [Google Scholar] [CrossRef]
- Cataldo, F.; Iglesias-Groth, S.; Hafez, Y. Neutron bombardment of boron carbide B12C3: A FT-IR, calorimetric (DSC) and ESR study. Fuller. Nanotub. Carbon Nanostr. 2017, 25, 371–378. [Google Scholar] [CrossRef]
- Mirzayev, M.N.; Popov, E.; Demir, E.; Abdurakhimov, B.A.; Mirzayeva, D.M.; Skuratov, V.A.; Mutali, A.K.; Tiep, V.N.; Biira, S.; Tashmetov, M.Y.; et al. Thermophysical behavior of boron nitride and boron trioxide ceramics compounds with high energy electron fluence and swift heavy ion irradiated. J. Alloys Compd. 2020, 834, 155119. [Google Scholar] [CrossRef]
- Mirzayev, M.N.; Mammadov, K.F.; Skuratov, V.A.; Demir, E.; Jabarov, S.H.; Ismayilova, N.A.; Biira, S.; Abdurakhimov, B.; Popov, E. Oxidation kinetics and thermophysical properties of gamma irradiated silicon hexaboride. J. Alloys Compd. 2019, 801, 151–157. [Google Scholar] [CrossRef]
- Mirzayev, M.N. Oxidation kinetics of boron carbide ceramic under high gamma irradiation dose in the high temperature. Ceram. Int. 2020, 46, 2816–2822. [Google Scholar] [CrossRef]
- Mirzayev, M.N.; Demir, E.; Mammadov, K.F.; Skuratov, V.A.; Jabarov, S.H.; Biira, S.; Asgerov, E.B.; Abdurakhimov, B.A.; Tuğrul, A.B. Amorphisation of boron carbide under gamma irradiation. Pramana J. Phys. 2020, 94, 1–8. [Google Scholar] [CrossRef]
- Mirzayev, M.N. Simultaneous measurements of heat flow rate and thermal properties of nano boron trioxide under neutron irradiation at the low and high temperature. Vacuum 2020, 173, 109162. [Google Scholar] [CrossRef]
- Mirzayev, M.N. Heat transfer of hexagonal boron nitride (h-BN) compound up to 1 MeV neutron energy: Kinetics of the release of wigner energy. Radiat. Phys. Chem. 2021, 180, 109244. [Google Scholar] [CrossRef]
- Yamamoto, H.; Asaoka, H. Formation of binary clusters by molecular ion irradiation. Appl. Surf. Sci. 2001, 169–170, 305–309. [Google Scholar] [CrossRef]
- Sekimura, N.; Shirao, Y.; Yamaguchi, H.; Yonamine, S.; Arai, Y. Defect cluster formation in vanadium irradiated with heavy ions. J. Nucl. Mater. 1999, 271–272, 63–67. [Google Scholar] [CrossRef]
- Almessiere, M.A.; Trukhanov, A.V.; Slimani, Y.; You, K.Y.; Trukhanov, S.V.; Trukhanova, E.L.; Esa, F.; Sadaqat, A.; Chaudhary, K.; Zdorovets, M.; et al. Correlation between composition and electrodynamics properties in nanocomposites based on hard/soft ferrimagnetics with strong exchange coupling. Nanomaterials 2019, 9, 202. [Google Scholar] [CrossRef]
- Yakovenko, O.S.; Matzui, L.Y.; Vovchenko, L.L.; Oliynyk, V.V.; Zagorodnii, V.V.; Trukhanov, S.V.; Trukhanov, A.V. Electromagnetic properties of carbon nanotubes / BaFe12-xGaxO19 / epoxy composites with random and oriented filler distribution. Nanomaterials 2021, 11, 2873. [Google Scholar] [CrossRef]
- Mostafa, M.; Saleh, O.; Henaish, A.M.; El-Kaream, S.A.A.; Ghazy, R.; Hemeda, O.M.; Dorgham, A.M.; Al-Ghamdi, H.; Almuqrin, A.H.; Sayyed, M.I.; et al. Structure, morphology and electrical/magnetic properties of Ni-Mg nano-ferrites from a new perspective. Nanomaterials 2022, 12, 1045. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, H.; Saito, T.; Asaoka, H. Silicon cluster formation by molecular ion irradiation—relationship between irradiated ion species and cluster yield. Appl. Surf. Sci. 2001, 178, 127–133. [Google Scholar] [CrossRef]
- Mirzayev, M.N.; Mammadov, K.F.; Garibov, R.G.; Askerov, E.B. Thermophysical properties of boron carbide Irradiated by ionizing radiation. High Temp. 2018, 56, 374–377. [Google Scholar] [CrossRef]
- Aryal, S.; Rulis, P.; Ching, W.Y. Mechanism for amorphization of boron carbide B4C under uniaxial compression. Phys. Rev. B 2011, 84, 184112. [Google Scholar] [CrossRef]
- Gosset, D.; Miro, S.; Doriot, S.; Moncoffre, N. Amorphisation of boron carbide under slow heavy ion irradiation. J. Nucl. Mater. 2016, 476, 198–204. [Google Scholar] [CrossRef]
- Mirzayev, M.N.; Jabarov, S.H.; Asgerov, E.B.; Mehdiyeva, R.N.; Thabethe, T.T.; Biira, S.; Tiep, N.V. X-ray diffraction and thermodynamics kinetics of SiB6 under gamma irradiation dose. Silicon 2018, 11, 2499–2504. [Google Scholar] [CrossRef]
- Evans, B.R.; Lian, J.; Ji, W. Evaluation of shielding performance for newly developed composite materials. Ann. Nucl. Energy 2018, 116, 1–9. [Google Scholar] [CrossRef]
- Azizov, E.; Barsuk, V.; Begrambekov, L.; Buzhinsky, O.; Evsin, A.; Gordeev, A.; Grunin, A.; Klimov, N.; Kurnaev, V.; Mazul, I.; et al. Boron carbide (B4C) coating. Deposition and testing. J. Nucl. Mater. 2015, 463, 792–795. [Google Scholar] [CrossRef]
- Demir, E.; Gledenov, Y.M.; Tuğrul, A.B.; Mirzayev, M.N.; Islamov, A.K.; Turchenko, V.A.; Yılmaz, O.; Büyük, B.; Sansarbayar, E.; Öveçoğlu, M.L. Structural and morphological studies under small-angle neutron scattering of tungsten alloys. Moscow Univ. Phys. Bull. 2019, 74, 509–513. [Google Scholar] [CrossRef]
- Gillet, K.; Roma, G.; Crocombette, J.P.; Gosset, D. The influence of irradiation induced vacancies on the mobility of helium in boron carbide. J. Nucl. Mater. 2018, 512, 288–296. [Google Scholar] [CrossRef]
- Itoh, H.; Matsudaira, T.; Naka, S.; Hamamoto, H.; Obayashi, M. Formation process of tungsten borides by solid state reaction between tungsten and amorphous boron. J. Mater. Sci. 1987, 22, 2811–2815. [Google Scholar] [CrossRef]
- Khor, K.A.; Yu, L.G.; Sundararajan, G. Formation of hard tungsten boride layer by spark plasma sintering boriding. Thin Solid Films 2005, 478, 232–237. [Google Scholar] [CrossRef]
- Yazici, S.; Derin, B. Production of tungsten boride from CaWO4 by self-propagating high-temperature synthesis followed by HCl leaching. Int. J. Refract. Met. Hard Mater. 2011, 29, 90–95. [Google Scholar] [CrossRef]
- Barış, M.; Şimşek, T.; Gökmeşe, H.; Akkurt, A. Characterization of W2B nanocrystals synthesized by mechanochemical method. Boron 2016, 1, 45–51. [Google Scholar]
- Windsor, C.G.; Astbury, J.O.; Davidson, J.J.; McFadzean, C.J.; Morgan, J.G.; Wilson, C.L.; Humphry-Baker, S.A. Tungsten boride shields in a spherical tokamak fusion power plant. Nucl. Fusion 2021, 61, 086018. [Google Scholar] [CrossRef]
- Windsor, C.G.; Marshall, J.M.; Morgan, J.G.; Fair, J.; Smith, G.D.; Rajczyk-Wryk, A.; Tarragó, J.M. Design of cemented tungsten carbide and boride-containing shields for a fusion power plant. Nucl. Fusion 2018, 58, 076014. [Google Scholar] [CrossRef]
- Rodríguez-Carvajal, J. Recent advances in magnetic structure determination by neutron powder diffraction. Phys. B Condens. Matter 1993, 192, 55–69. [Google Scholar] [CrossRef]
- Ekambaram, S.; Patil, K.C.; Maaza, M. Synthesis of lamp phosphors: Facile combustion approach. J. Alloys Compd. 2005, 393, 81–92. [Google Scholar] [CrossRef]
- Sologub, O.L.; Michiue, Y.; Mori, T. Boron carbide, B13-xC2-y (x = 0.12, y = 0.01). Acta Crystallogr. Sect. E Struct. Reports Online 2012, 68, i67. [Google Scholar] [CrossRef] [PubMed]
- Heller, G. The System Boron—Oxygen BT-B Boron Compounds: 4th Supplement Volume 2, Boron and Oxygen; Heller, G., Faust, J., Niedenzu, K., Eds.; Springer: Berlin/Heidelberg, Germany, 1993; pp. 1–297. [Google Scholar]
- Burkholder, T.R.; Andrews, L. Reactions of boron atoms with molecular oxygen. Infrared spectra of BO, BO2, B2O2, B2O3, and BO-2 in solid argon. J. Chem. Phys. 1998, 95, 8697–8709. [Google Scholar] [CrossRef]
- Liu, C.H. Positron spectroscopy of boron carbide containing metal impurity. Mater. Trans. JIM 2000, 41, 1293–1296. [Google Scholar] [CrossRef]
- Li, M.; Hou, Q.; Cui, J.; Fu, B. Molecular dynamics simulations of tungsten bombard- ments on tungsten nanoparticles. Nucl. Instr. Meth. Phys. Res. Sect. B Beam Interact. Mater. At. 2019, 450, 47–50. [Google Scholar] [CrossRef]
- Granberg, F.; Byggmästar, J.; Nordlund, K. Molecular dynamics simulations of high-dose damage production and defect evolution in tungsten. J. Nucl. Mater. 2021, 556, 153158. [Google Scholar] [CrossRef]
- Mirzayev, M.N. Study thermodynamic assessment of the B-C and B-Si binary systems with swift heavy ions and high intense electron beam irradiation at the low temperature. Mod. Phys. Lett. B 2020, 34, 2050395. [Google Scholar] [CrossRef]
- Gosset, D.; Miro, S.; Doriot, S.; Victor, G.; Motte, V. Evidence of amorphisation of B4C boron carbide under slow, heavy ion irradiation. Nucl. Instr. Meth. Phys. Res. Sect. B Beam Interact. Mater. At. 2015, 365, 300–304. [Google Scholar] [CrossRef]
- Desgranges, L.; Escleine, J.M.; Bienvenu, P.; Roure, I.; Gosset, D.; Boffy, R.; Köster, U. A new methodology for studying neutron absorber materials: First results with boron carbide. Nucl. Instr. Meth. Phys. Res. Sect. B Beam Interact. Mater. At. 2018, 432, 42–47. [Google Scholar] [CrossRef]
- Victor, G.; Pipon, Y.; Bérerd, N.; Toulhoat, N.; Moncoffre, N.; Djourelov, N.; Miro, S.; Baillet, J.; Pradeilles, N.; Rapaud, O.; et al. Structural modifications induced by ion irradiation and temperature in boron carbide B4C. Nucl. Instr. Meth. Phys. Res. Sect. B Beam Interact. Mater. At. 2015, 365, 30–34. [Google Scholar] [CrossRef]
- Alin, M.; Kozlovskiy, A.L.; Zdorovets, M.V.; Uglov, V.V. Study of the mechanisms of the t-ZrO2 → c-ZrO2 type polymorphic transformations in ceramics as a result of irradiation with heavy Xe22+ ions. Solid State Sci. 2022, 123, 106791. [Google Scholar] [CrossRef]
- Makkonen, I.; Hakala, M.; Puska, M.J. Modeling the momentum distributions of annihilating electron-positron pairs in solids. Phys. Rev. B 2006, 73, 035103. [Google Scholar] [CrossRef]
- Boroński, E.; Nieminen, R.M. Electron-positron density-functional theory. Phys. Rev. B 1986, 34, 3820–3831. [Google Scholar] [CrossRef] [PubMed]
- Puska, M.J.; Nieminen, R.M. Defect spectroscopy with positrons: A general calculational method. J. Phys. F Met. Phys. 1983, 13, 333–346. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous electron gas. Phys. Rev. 1964, 136, B864. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-consistent equations including exchange and correlation effects. Phys. Rev. 1965, 140, A1133. [Google Scholar] [CrossRef]
- Hu, Y.; Murthy, G.; Rao, S.; Jain, J.K. Kohn-Sham density functional theory of Abelian anyons. Phys. Rev. B 2021, 103, 035124. [Google Scholar] [CrossRef]
- Jacobsohn, L.G.; Nastasi, M.; Daemen, L.L.; Jenei, Z.; Asoka-Kumar, P. Positron annihilation spectroscopy of sputtered boron carbide films. Diam. Relat. Mater. 2005, 14, 201–205. [Google Scholar] [CrossRef]
- May-Tal Beck, S.; Butterling, M.; Anwand, W.; Beck, A.; Wagner, A.; Brauer, G.; Ocherashvili, A.; Israelashvili, I.; Hen, O. Study of neutron induced defects in ceramics using the GiPS facility. J. Phys. Conf. Ser. 2013, 443, 012076. [Google Scholar] [CrossRef]
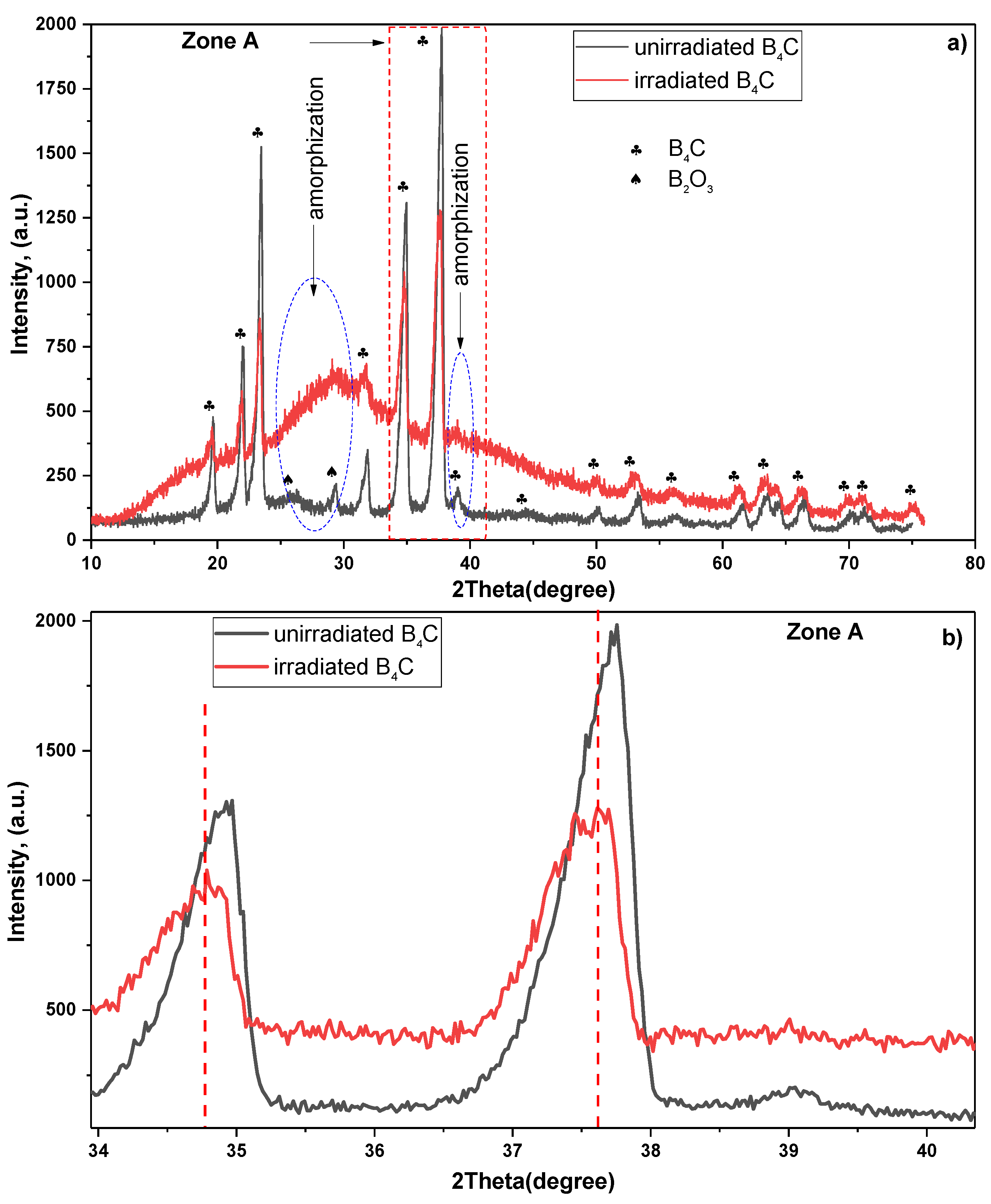
| K | λ(Å) | Peak Position (2θ) of Unirradiated Boron Carbide Sample | Peak Position (2θ) of Irradiated Boron Carbide Sample | FWHM of Unirradiated Boron Carbide Sample | FWHM of Irradiated Boron Carbide Sample | Crystallite Size of Unirradiated Boron Carbide Sample, L (nm) | Crystallite Size of Irradiated Boron Carbide Sample, L (nm) |
|---|---|---|---|---|---|---|---|
| 0.94 | 1.54178 | 19.61(2) | ←19.51(2) | 0.31(3) | 0.42(3)↑ | 26.78(2) | 19.84(2)↓ |
| 21.97(2) | ←21.86(2) | 0.33(3) | 0.37(3)↑ | 25.45(2) | 22.56(2)↓ | ||
| 23.40(2) | ←23.32(2) | 0.34(3) | 0.39(3)↑ | 24.82(2) | 21.56(2)↓ | ||
| 29.29(2) | - | 0.39(3) | - | 21.86(2) | - | ||
| 31.77(2) | ←31.49(2) | 0.51(3) | 1.98(3)↑ | 17.02(2) | 4.36(2)↓ | ||
| 34.82(2) | ←34.69(2) | 0.49(3) | 0.52(3)↑ | 17.92(2) | 16.76(2)↓ | ||
| 37.63(2) | ←37.53(2) | 0.51(3) | 0.56(3)↑ | 17.32(2) | 15.65(2)↓ | ||
| 38.00(2) | - | 0.59(3) | - | 14.89(2) | - | ||
| 50.05(2) | - | 0.76(3) | - | 12.12(2) | - | ||
| 53.25(2) | ←53.02(2) | 0.79(3) | 1.01(3)↑ | 11.74(2) | 9.23(2)↓ | ||
| 61.51(2) | ←61.32(2) | 0.81(3) | 0.63(3)↓ | 11.94(2) | 15.32(2)↑ | ||
| 63.48(2) | ←63.33(2) | 1.03(3) | 1.54(3)↑ | 9.52(2) | 6.34(2)↓ | ||
| 66.38(2) | ←66.21(2) | 1.04(3) | 1.06(3)↑ | 9.51(2) | 9.35(2)↓ |
| B11C-CBC | B12-CCC | ||||
|---|---|---|---|---|---|
| Novacancies and Impurity Atoms | LDA τ (ps) | GGA τ (ps) | Novacancies and Impurity Atoms | LDA τ (ps) | GGA τ (ps) |
| bulk | 341 | 441 | bulk | 348 | 468 |
| 3H interstitials | 286 | 334 | 3H interstitials | 312 | 386 |
| 3He interstitials | 258 | 355 | 3He interstitials | 280 | 394 |
| 1VB | 354 | 464 | 1VB | 363 | 496 |
| 1VC | 354 | 464 | 1VC | 363 | 496 |
| 1VB in center of the chain | 354 | 464 | 1VC in center of the chain | 363 | 496 |
| 1VC + 11VB | 374 | 522 | 12 VB | 374 | 536 |
| 1VC + 11VB 1H nearly to C atom | 373 | 516 | 12 VB 1H nearly to C atom | 373 | 580 |
| 1VC + 11VBwith 2H in center cluster | 350 | 436 | 12 VB with 2H in center cluster | 359 | 461 |
| 1VC + 11VB 2H nearly to C | 372.2 | 515 | 12 VB 2H nearly to C | 372.7 | 532 |
| 1VC + 11VBwith 4H in center cluster | 349 | 428 | 12 VB with 4H in center cluster | 359 | 460 |
| 1VC + 11VBwith 2He in center cluster | 326 | 469 | 12 VB with 2 He in center cluster | 339 | 498 |
| 1VC + 11VBwith 4He in center cluster | 309 | 453 | 12 VB with 4 He in center cluster | 330 | 487 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mirzayev, M.N.; Donkov, A.A.; Popov, E.A.; Demir, E.; Jabarov, S.H.; Chkhartishvili, L.S.; Adeojo, S.A.; Doroshkevich, A.S.; Sidorin, A.A.; Asadov, A.G.; et al. Modeling and X-ray Analysis of Defect Nanoclusters Formation in B4C under Ion Irradiation. Nanomaterials 2022, 12, 2644. https://doi.org/10.3390/nano12152644
Mirzayev MN, Donkov AA, Popov EA, Demir E, Jabarov SH, Chkhartishvili LS, Adeojo SA, Doroshkevich AS, Sidorin AA, Asadov AG, et al. Modeling and X-ray Analysis of Defect Nanoclusters Formation in B4C under Ion Irradiation. Nanomaterials. 2022; 12(15):2644. https://doi.org/10.3390/nano12152644
Chicago/Turabian StyleMirzayev, Matlab N., Alexander A. Donkov, Evgeni A. Popov, Ertugrul Demir, Sakin H. Jabarov, Levan S. Chkhartishvili, Samuel A. Adeojo, Aleksandr S. Doroshkevich, Alexey A. Sidorin, Asif G. Asadov, and et al. 2022. "Modeling and X-ray Analysis of Defect Nanoclusters Formation in B4C under Ion Irradiation" Nanomaterials 12, no. 15: 2644. https://doi.org/10.3390/nano12152644
APA StyleMirzayev, M. N., Donkov, A. A., Popov, E. A., Demir, E., Jabarov, S. H., Chkhartishvili, L. S., Adeojo, S. A., Doroshkevich, A. S., Sidorin, A. A., Asadov, A. G., Thabethe, T. T., Khandaker, M. U., Alamri, S., Osman, H., Trukhanov, A. V., & Trukhanov, S. V. (2022). Modeling and X-ray Analysis of Defect Nanoclusters Formation in B4C under Ion Irradiation. Nanomaterials, 12(15), 2644. https://doi.org/10.3390/nano12152644








