High-Temperature Quantum Hall Effect in Graphite-Gated Graphene Heterostructure Devices with High Carrier Mobility
Abstract
:1. Introduction
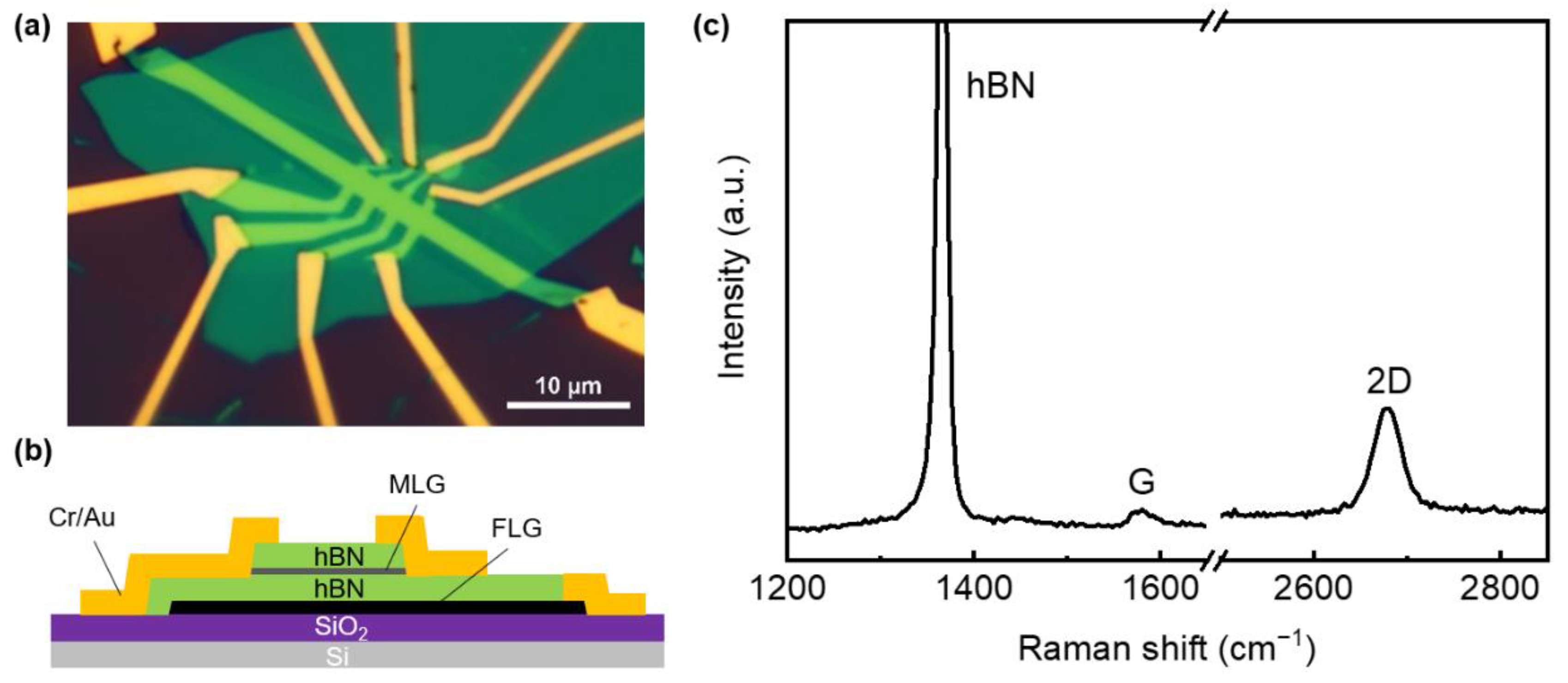
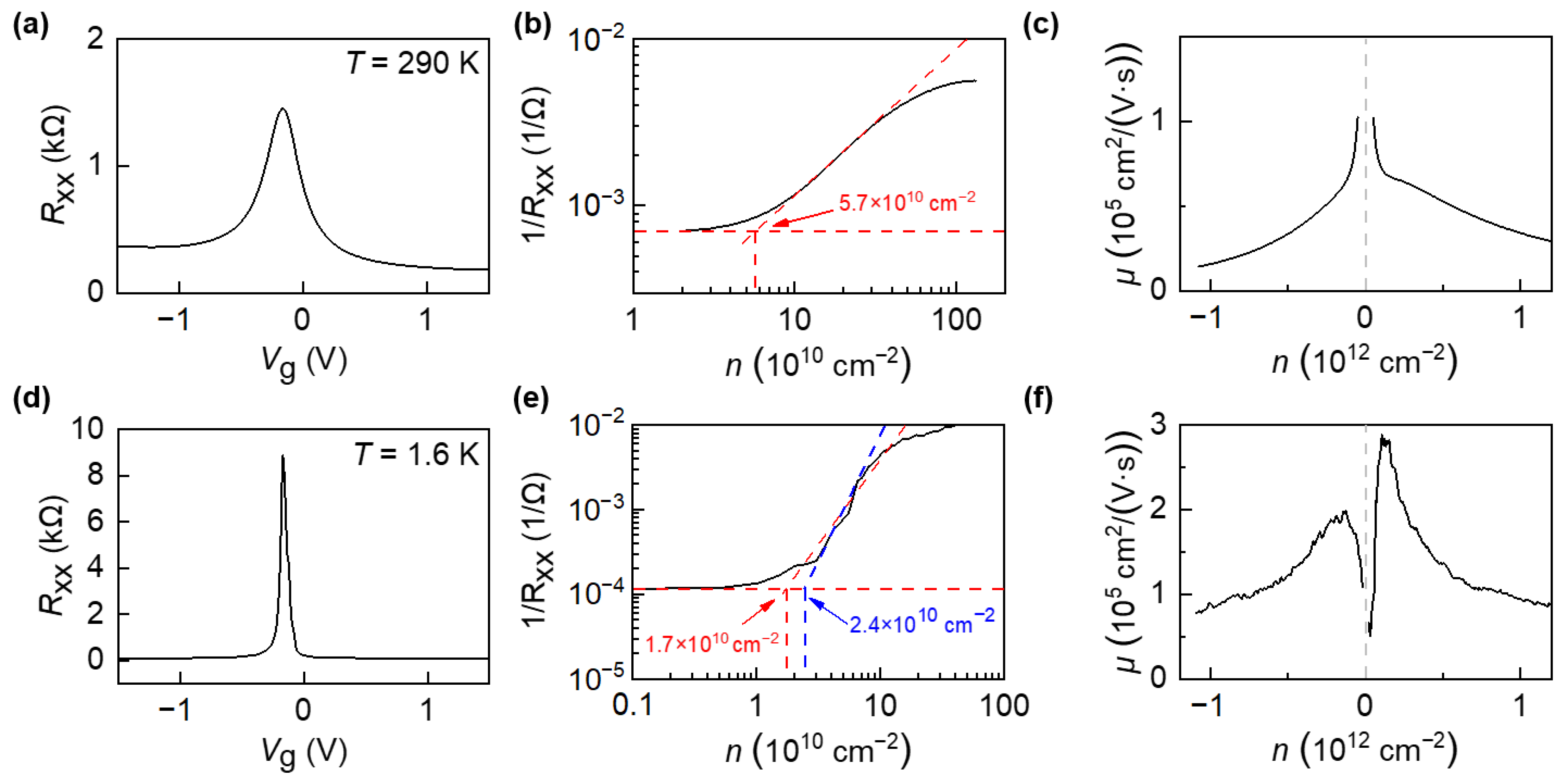
2. Results and Discussions
3. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Klitzing, K.V.; Dorda, G.; Pepper, M. New method for high-accuracy determination of the fine-structure constant based on quantized Hall resistance. Phys. Rev. Lett. 1980, 45, 494–497. [Google Scholar] [CrossRef] [Green Version]
- Halperin, B.I. Quantized Hall conductance, current-carrying edge states, and the existence of extended states in a two-dimensional disordered potential. Phys. Rev. B 1982, 25, 2185–2190. [Google Scholar] [CrossRef]
- Delahaye, F.; Jeckelmann, B. Revised technical guidelines for reliable dc measurements of the quantized Hall resistance. Metrologia 2003, 40, 217. [Google Scholar] [CrossRef]
- D’iorio, M.; Wood, B.M. Temperature dependence of the quantum Hall resistance. Surf. Sci. 1986, 170, 233–237. [Google Scholar] [CrossRef]
- Matthews, J.; Cage, M.E. Temperature Dependence of the Hall and Longitudinal Resistances in a Quantum Hall Resistance Standard. J. Res. Natl. Inst. Stand. Technol. 2005, 110, 497–510. [Google Scholar] [CrossRef]
- Yu, R.; Zhang, W.; Zhang, H.J.; Zhang, S.C.; Dai, X.; Fang, Z. Quantized anomalous Hall effect in magnetic topological insulators. Science 2010, 329, 61–64. [Google Scholar] [CrossRef] [Green Version]
- Chang, C.-Z.; Zhang, J.; Feng, X.; Shen, J.; Zhang, Z.; Guo, M.; Li, K.; Ou, Y.; Wei, P.; Wang, L.-L.; et al. Experimental Observation of the Quantum Anomalous Hall Effect in a Magnetic Topological Insulator. Science 2013, 340, 167–170. [Google Scholar] [CrossRef] [Green Version]
- Chang, C.-Z.; Zhao, W.; Kim, D.Y.; Zhang, H.; Assaf, B.A.; Heiman, D.; Zhang, S.-C.; Liu, C.; Chan MH, W.; Moodera, J.S. High-precision realization of robust quantum anomalous Hall state in a hard ferromagnetic topological insulator. Nat. Mater. 2015, 14, 473–477. [Google Scholar] [CrossRef] [Green Version]
- Bestwick, A.J.; Fox, E.J.; Kou, X.; Pan, L.; Wang, K.L.; Goldhaber-Gordon, D. Precise quantization of the anomalous Hall effect near zero magnetic field. Phys. Rev. Lett. 2015, 114, 187201. [Google Scholar] [CrossRef] [Green Version]
- Vonsovskii, S.V. Magnetism, 2nd ed.; John Wiley and Sons: New York, NY, USA, 1974. [Google Scholar]
- Bedyaev, A.V.; Voloshinskii, A.N.; Granovskii, A.B.; Ryzhanova, N.V. Anomalous hall effect in disordered ferromagnetic alloys of the transition metals. Sov. Phys. J. 1987, 30, 1. [Google Scholar] [CrossRef]
- Cohen, M.H.; Jortner, J. Effective medium theory for the Hall effect in disordered materials. Phys. Rev. Lett. 1973, 30, 696. [Google Scholar] [CrossRef]
- Götz, M.; Fijalkowski, K.M.; Pesel, E.; Hartl, M.; Schreyeck, S.; Winnerlein, M.; Grauer, S.; Scherer, H.; Brunner, K.; Gould, C.; et al. Precision measurement of the quantized anomalous Hall resistance at zero magnetic field. Appl. Phys. Lett. 2018, 112, 72102. [Google Scholar] [CrossRef] [Green Version]
- Fox, E.J.; Rosen, I.T.; Yang, Y.; Jones, G.R.; Elmquist, R.E.; Kou, X.; Pan, L.; Wang, K.L.; Goldhaber-Gordon, D. Part-per-million quantization and current-induced breakdown of the quantum anomalous Hall effect. Phys. Rev. B 2018, 98, 75145. [Google Scholar] [CrossRef] [Green Version]
- Okazaki, Y.; Oe, T.; Kawamura, M.; Yoshimi, R.; Nakamura, S.; Takada, S.; Mogi, M.; Takahashi, K.S.; Tsukazaki, A.; Kawasaki, M.; et al. Precise resistance measurement of quantum anomalous Hall effect in magnetic heterostructure film of topological insulator. Appl. Phys. Lett. 2020, 116, 143101. [Google Scholar] [CrossRef]
- Okazaki, Y.; Oe, T.; Kawamura, M.; Yoshimi, R.; Nakamura, S.; Takada, S.; Mogi, M.; Takahashi, K.S.; Tsukazaki, A.; Kawasaki, M.; et al. Quantum anomalous Hall effect with a permanent magnet defines a quantum resistance standard. Nat. Phys. 2022, 18, 25–29. [Google Scholar] [CrossRef]
- Rigosi, A.F.; Elmquist, R.E. The quantum Hall effect in the era of the new SI. Semicond. Sci. Technol. 2019, 34, 93004. [Google Scholar] [CrossRef]
- Milton, M.J.; Davis, R.; Fletcher, N. Towards a new SI: A review of progress made since 2011. Metrologia 2014, 51, R21. [Google Scholar] [CrossRef]
- Janssen TJ, B.M.; Rozhko, S.; Antonov, I.; Tzalenchuk, A.; Williams, J.M.; Melhem, Z.; He, H.; Lara-Avila, S.; Kubatkin, S.; Yakimova, R. Operation of graphene quantum Hall resistance standard in a cryogen-free table-top system. 2D Mater. 2015, 2, 35015. [Google Scholar] [CrossRef] [Green Version]
- Kruskopf, M.; Bauer, S.; Pimsut, Y.; Chatterjee, A.; Patel, D.K.; Rigosi, A.F.; Elmquist, R.E.; Pierz, K.; Pesel, E.; Götz, M.; et al. Graphene Quantum Hall Effect Devices for AC and DC Electrical Metrology. IEEE Trans. Electron Devices 2021, 68, 3672–3677. [Google Scholar] [CrossRef]
- Zhang, Y.; Tan, Y.W.; Stormer, H.L.; Kim, P. Experimental observation of the quantum Hall effect and Berry’s phase in graphene. Nature 2005, 438, 201–204. [Google Scholar] [CrossRef]
- Ribeiro-Palau, R.; Lafont, F.; Brun-Picard, J.; Kazazis, D.; Michon, A.; Cheynis, F.; Couturaud, O.; Consejo, C.; Jouault, B.; Poirier, W.; et al. Quantum Hall resistance standard in graphene devices under relaxed experimental conditions. Nat. Nanotechnol. 2015, 10, 965–971. [Google Scholar] [CrossRef] [Green Version]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Katsnelson, M.I.; Grigorieva, I.V.; Dubonos, S.V.; Firsov, A.A. Two-dimensional gas of massless Dirac fermions in graphene. Nature 2005, 438, 197–200. [Google Scholar] [CrossRef] [Green Version]
- Pezzini, S.; Mišeikis, V.; Pace, S.; Rossella, F.; Watanabe, K.; Taniguchi, T.; Coletti, C. High-quality electrical transport using scalable CVD graphene. 2D Mater. 2020, 7, 041003. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Jiang, Z.; Zhang, Y.; Morozov, S.V.; Stormer, H.L.; Zeitler, U.; Maan, J.C.; Boebinger, G.S.; Kim, P.; Geim, A.K. Room-Temperature Quantum Hall Effect in Graphene. Science 2007, 315, 1379. [Google Scholar] [CrossRef] [Green Version]
- Geim, A.K.; Grigorieva, I.V. Van der Waals heterostructures. Nature 2013, 499, 419–425. [Google Scholar] [CrossRef] [Green Version]
- Dean, C.R.; Young, A.F.; Meric, I.; Lee, C.; Wang, L.; Sorgenfrei, S.; Watanabe, K.; Taniguchi, T.; Kim, P.; Shepard, K.L.; et al. Boron nitride substrates for high-quality graphene electronics. Nat. Nanotechnol. 2010, 5, 722–726. [Google Scholar] [CrossRef]
- Wang, L.; Meric, I.; Huang, P.Y.; Gao, Q.; Gao, Y.; Tran, H.; Taniguchi, T.; Watanabe, K.; Campos, L.M.; Muller, D.A.; et al. One-Dimensional Electrical Contact to a Two-Dimensional Material. Science 2013, 342, 614–617. [Google Scholar] [CrossRef] [Green Version]
- Gorbachev, R.V.; Geim, A.K.; Katsnelson, M.I.; Novoselov, K.S.; Tudorovskiy, T.; Grigorieva, I.V.; MacDonald, A.H.; Morozov, S.V.; Watanabe, K.; Taniguchi, T.; et al. Strong Coulomb drag and broken symmetry in double-layer graphene. Nat. Phys. 2012, 8, 896–901. [Google Scholar] [CrossRef]
- Ponomarenko, L.A.; Gorbachev, R.V.; Yu, G.L.; Elias, D.C.; Jalil, R.; Patel, A.A.; Mishchenko, A.; Mayorov, A.S.; Woods, C.R.; Wallbank, J.R.; et al. Cloning of Dirac fermions in graphene superlattices. Nature 2013, 497, 594–597. [Google Scholar] [CrossRef] [Green Version]
- Dean, C.R.; Wang, L.; Maher, P.; Forsythe, C.; Ghahari, F.; Gao, Y.; Katoch, J.; Ishigami, M.; Moon, P.; Koshino, M.; et al. Hofstadter’s butterfly and the fractal quantum Hall effect in moiré superlattices. Nature 2013, 497, 598–602. [Google Scholar] [CrossRef]
- Hunt, B.; Sanchez-Yamagishi, J.D.; Young, A.F.; Yankowitz, M.; LeRoy, B.J.; Watanabe, K.; Taniguchi, T.; Moon, P.; Koshino, M.; Jarillo-Herrero, P.; et al. Massive Dirac Fermions and Hofstadter Butterfly in a van der Waals Heterostructure. Science 2013, 340, 1427–1430. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Britnell, L.; Gorbachev, R.V.; Jalil, R.; Belle, B.D.; Schedin, F.; Mishchenko, A.; Georgiou, T.; Katsnelson, M.I.; Eaves, L.; Morozov, S.V.; et al. Field-Effect Tunneling Transistor Based on Vertical Graphene Heterostructures. Science 2012, 335, 947–950. [Google Scholar] [CrossRef] [Green Version]
- Poirier, W.; Schopfe, F. Can graphene set new standards? Nat. Nanotechnol. 2010, 5, 171. [Google Scholar] [CrossRef] [PubMed]
- Schaefer, B.T.; Wang, L.; Jarjour, A.; Watanabe, K.; Taniguchi, T.; McEuen, P.L.; Nowack, K.C. Magnetic field detection limits for ultraclean graphene Hall sensors. Nat. Commun. 2020, 11, 4163. [Google Scholar] [CrossRef] [PubMed]
- Kretinin, A.V.; Cao, Y.; Tu, J.S.; Yu, G.L.; Jalil, R.; Novoselov, K.S.; Haigh, S.J.; Gholinia, A.; Mishchenko, A.; Lozada, M.; et al. Electronic Properties of Graphene Encapsulated with Different Two-Dimensional Atomic Crystals. Nano Lett. 2014, 14, 3270–3276. [Google Scholar] [CrossRef] [Green Version]
- Zibrov, A.A.; Kometter, C.; Zhou, H.; Spanton, E.M.; Taniguchi, T.; Watanabe, K.; Zaletel, M.P.; Young, A.F. Tunable interacting composite fermion phases in a half-filled bilayer-graphene Landau level. Nature 2017, 549, 360–364. [Google Scholar] [CrossRef] [Green Version]
- Rhodes, D.; Chae, S.H.; Ribeiro-Palau, R.; Hone, J. Disorder in van der Waals heterostructures of 2D materials. Nat. Mater. 2019, 18, 541–549. [Google Scholar] [CrossRef]
- Zeng, Y.; Li JI, A.; Dietrich, S.A.; Ghosh, O.M.; Watanabe, K.; Taniguchi, T.; Hone, J.; Dean, C.R. High-Quality Magnetotransport in Graphene Using the Edge-Free Corbino Geometry. Phys. Rev. Lett. 2019, 122, 137701. [Google Scholar] [CrossRef] [Green Version]
- Giovannetti GA KP, A.; Khomyakov, P.A.; Brocks, G.; Karpan, V.V.; van den Brink, J.; Kelly, P.J. Doping graphene with metal contacts. Phys. Rev. Lett. 2008, 101, 026803. [Google Scholar] [CrossRef]
- Xia, F.; Perebeinos, V.; Lin, Y.M.; Wu, Y.; Avouris, P. The origins and limits of metal–graphene junction resistance. Nat. Nanotechnol. 2011, 6, 179–184. [Google Scholar] [CrossRef]
- Russo, S.; Craciun, M.F.; Yamamoto, M.; Morpurgo, A.F.; Tarucha, S. Contact resistance in graphene-based devices. Phys. E Low-Dimens. Syst. Nanostruct. 2010, 42, 677–679. [Google Scholar] [CrossRef] [Green Version]
- Smith, J.T.; Franklin, A.D.; Farmer, D.B.; Dimitrakopoulos, C.D. Reducing contact resistance in graphene devices through contact area patterning. ACS Nano 2013, 7, 3661–3667. [Google Scholar] [CrossRef] [PubMed]
- Robinson, J.A.; LaBella, M.; Zhu, M.; Hollander, M.; Kasarda, R.; Hughes, Z.; Trumbull, K.; Cavalero, R.; Snyder, D. Contacting graphene. Appl. Phys. Lett. 2011, 98, 053103. [Google Scholar] [CrossRef]
- Yamaguchi, J.; Hayashi, K.; Sato, S.; Yokoyama, N. Passivating chemical vapor deposited graphene with metal oxides for transfer and transistor fabrication processes. Appl. Phys. Lett. 2013, 102, 143505. [Google Scholar] [CrossRef]
- Lindvall, N.; Kalabukhov, A.; Yurgens, A. Cleaning graphene using atomic force microscope. J. Appl. Phys. 2012, 111, 64904. [Google Scholar] [CrossRef]
- Schmitz, M.; Ouaj, T.; Winter, Z.; Rubi, K.; Watanabe, K.; Taniguchi, T.; Zeitler, U.; Beschoten, B.; Stampfer, C. Fractional quantum Hall effect in CVD-grown graphene. 2D Mater. 2020, 7, 041007. [Google Scholar] [CrossRef]
- Tanabe, S.; Sekine, Y.; Kageshima, H.; Nagase, M.; Hibino, H. Half-integer quantum Hall effect in gate-controlled epitaxial graphene devices. Appl. Phys. Express 2010, 3, 75102. [Google Scholar] [CrossRef]
- Du, X.; Skachko, I.; Barker, A.; Andrei, E.Y. Approaching ballistic transport in suspended graphene. Nat. Nanotechnol. 2008, 3, 491–495. [Google Scholar] [CrossRef] [Green Version]
- Bolotin, K.I.; Sikes, K.J.; Hone, J.; Stormer, H.L.; Kim, P. Temperature-Dependent Transport in Suspended Graphene. Phys. Rev. Lett. 2008, 101, 096802. [Google Scholar] [CrossRef] [Green Version]
- Mayorov, A.S.; Elias, D.C.; Mukhin, I.S.; Morozov, S.V.; Ponomarenko, L.A.; Novoselov, K.S.; Geim, A.K.; Gorbachev, R.V. How Close Can One Approach the Dirac Point in Graphene Experimentally? Nano Lett. 2012, 12, 4629–4634. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhang, Y.; Tan, Y.W.; Stormer, H.L.; Kim, P. Quantum Hall effect in graphene. Solid State Commun. 2007, 143, 14–19. [Google Scholar] [CrossRef]
- Li, L.; Yang, F.; Ye, G.J.; Zhang, Z.; Zhu, Z.; Lou, W.; Zhou, X.; Li, L.; Watanabe, K.; Taniguchi, T.; et al. Quantum Hall effect in black phosphorus two-dimensional electron system. Nat. Nanotechnol. 2016, 11, 593–597. [Google Scholar] [CrossRef] [PubMed]
- Banszerus, L.; Schmitz, M.; Engels, S.; Dauber, J.; Oellers, M.; Haupt, F.; Watanabe, K.; Taniguchi, T.; Beschoten, B.; Stampfer, C. Ultrahigh-mobility graphene devices from chemical vapor deposition on reusable copper. Sci. Adv. 2015, 1, e1500222. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kozlov, D.A.; Kvon, Z.D.; Mikhailov, N.N.; Dvoretskii, S.A.; Weishäupl, S.; Krupko, Y.; Portal, J.C. Quantum Hall effect in HgTe quantum wells at nitrogen temperatures. Appl. Phys. Lett. 2014, 105, 132102. [Google Scholar] [CrossRef]
- Kim, K.; Yankowitz, M.; Fallahazad, B.; Kang, S.; Movva, H.C.P.; Huang, S.; Larentis, S.; Corbet, C.M.; Taniguchi, T.; Watanabe, K.; et al. van der Waals Heterostructures with High Accuracy Rotational Alignment. Nano Lett. 2016, 16, 1989–1995. [Google Scholar] [CrossRef] [PubMed]
- Grenadier, S.; Li, J.; Lin, J.; Jiang, H. Dry etching techniques for active devices based on hexagonal boron nitride epilayers. J. Vac. Sci. Technol. A Vac. Surf. Film. 2013, 31, 061517. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, S.; Zhu, M.; Liu, Q.; Xiao, Y.; Cui, Z.; Guo, C. High-Temperature Quantum Hall Effect in Graphite-Gated Graphene Heterostructure Devices with High Carrier Mobility. Nanomaterials 2022, 12, 3777. https://doi.org/10.3390/nano12213777
Zhou S, Zhu M, Liu Q, Xiao Y, Cui Z, Guo C. High-Temperature Quantum Hall Effect in Graphite-Gated Graphene Heterostructure Devices with High Carrier Mobility. Nanomaterials. 2022; 12(21):3777. https://doi.org/10.3390/nano12213777
Chicago/Turabian StyleZhou, Siyu, Mengjian Zhu, Qiang Liu, Yang Xiao, Ziru Cui, and Chucai Guo. 2022. "High-Temperature Quantum Hall Effect in Graphite-Gated Graphene Heterostructure Devices with High Carrier Mobility" Nanomaterials 12, no. 21: 3777. https://doi.org/10.3390/nano12213777
APA StyleZhou, S., Zhu, M., Liu, Q., Xiao, Y., Cui, Z., & Guo, C. (2022). High-Temperature Quantum Hall Effect in Graphite-Gated Graphene Heterostructure Devices with High Carrier Mobility. Nanomaterials, 12(21), 3777. https://doi.org/10.3390/nano12213777






