Surface Coverage Simulation and 3D Plotting of Main Process Parameters for Molybdenum and Vanadium Adsorption onto Ferrihydrite
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials Synthesis and Characterization
2.2. Adsorption Experiments
2.3. Calculation of the Surface Sites Coverage
2.4. 3D Plotting of the Adsorption Parameters
3. Results
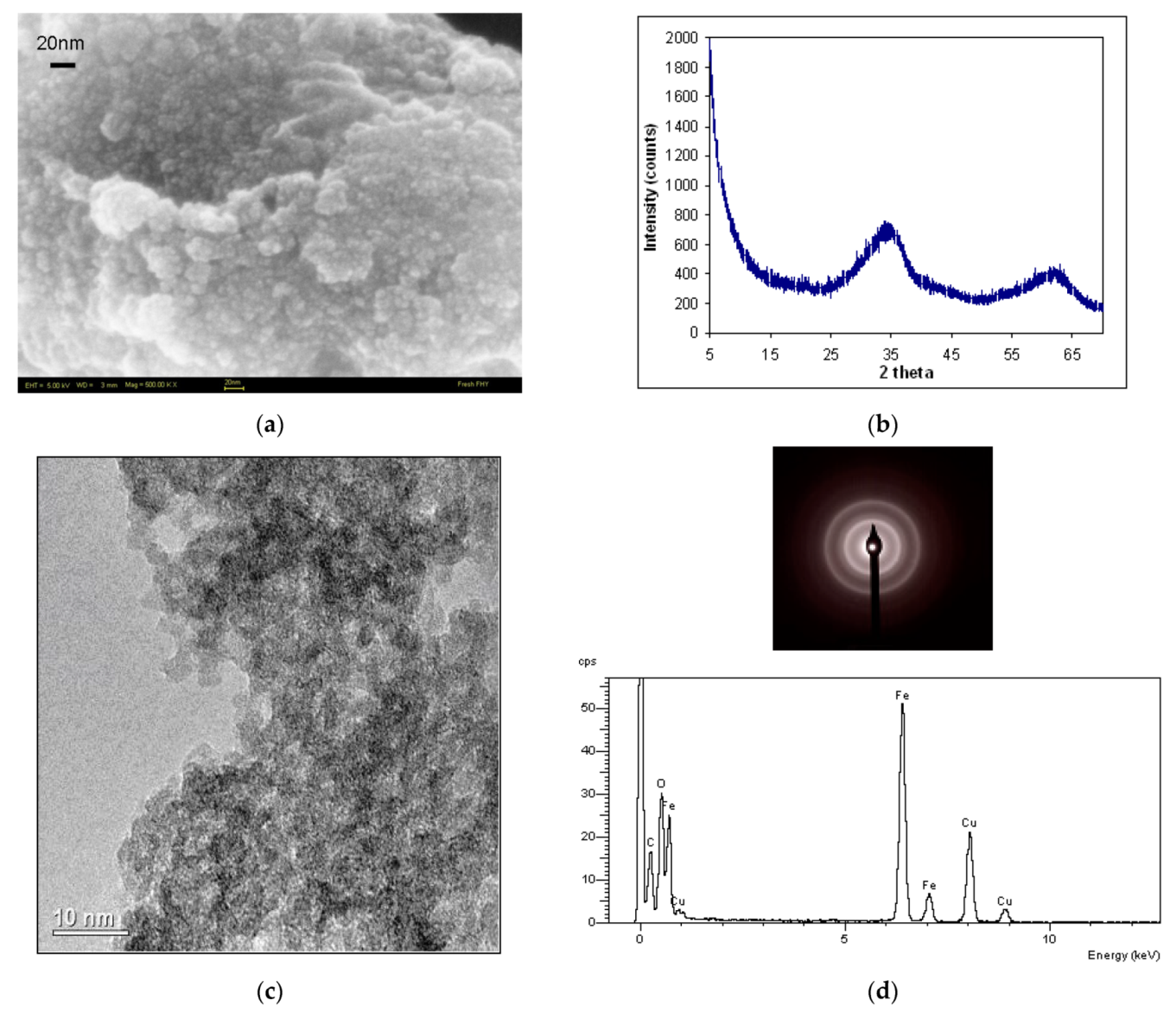
3.1. Ferrihydrite Characterization
3.1.1. High-Resolution Microscopy and X-ray Diffraction
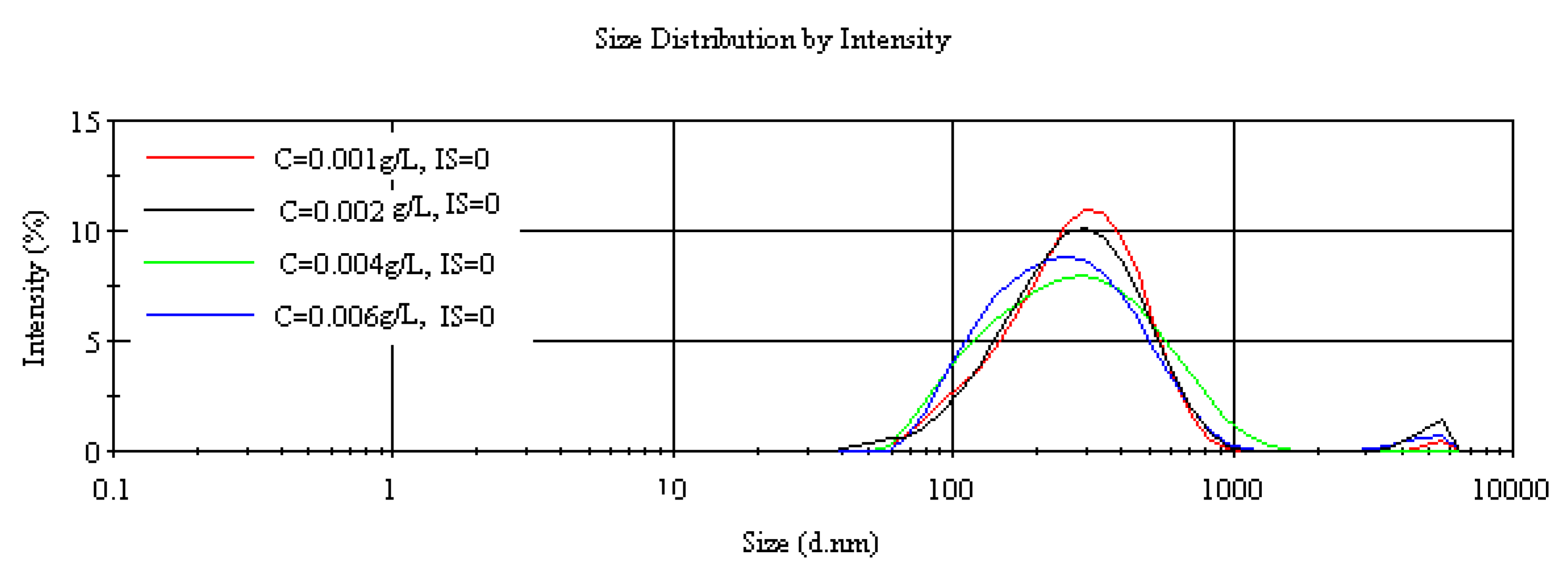
3.1.2. Dynamic Light Scattering and-Particle Size Measurement
3.1.3. Potentiometric Titration–Surface Charge Characterization
3.1.4. BET-Surface Area Measurements
3.2. Adsorption Results
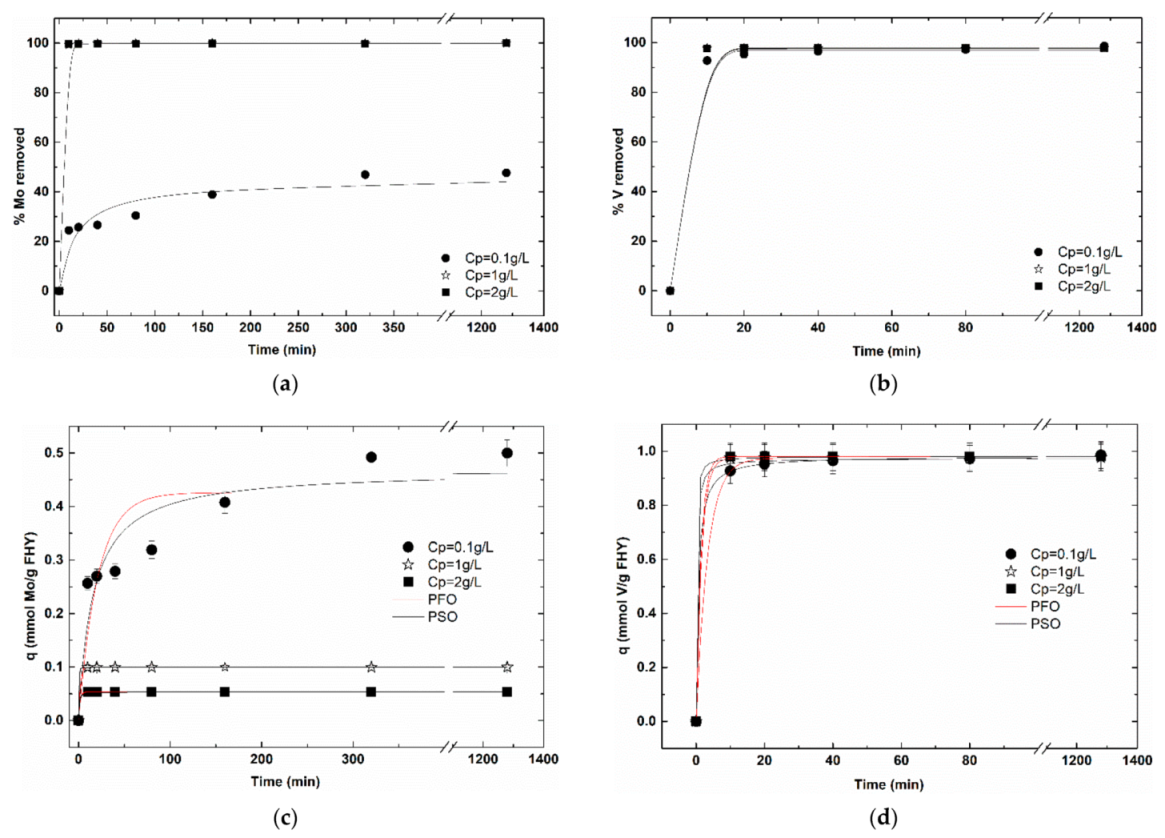
3.2.1. Particle Concentration Effect
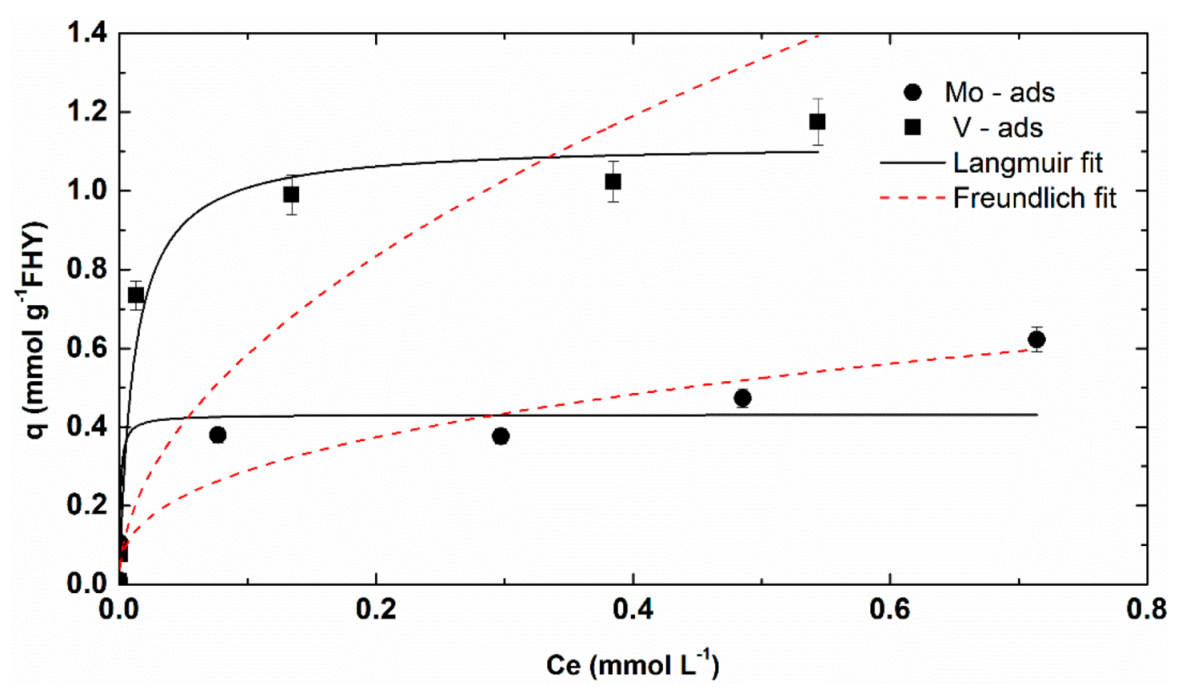
3.2.2. Metals Concentration Effect and Adsorption Isotherms
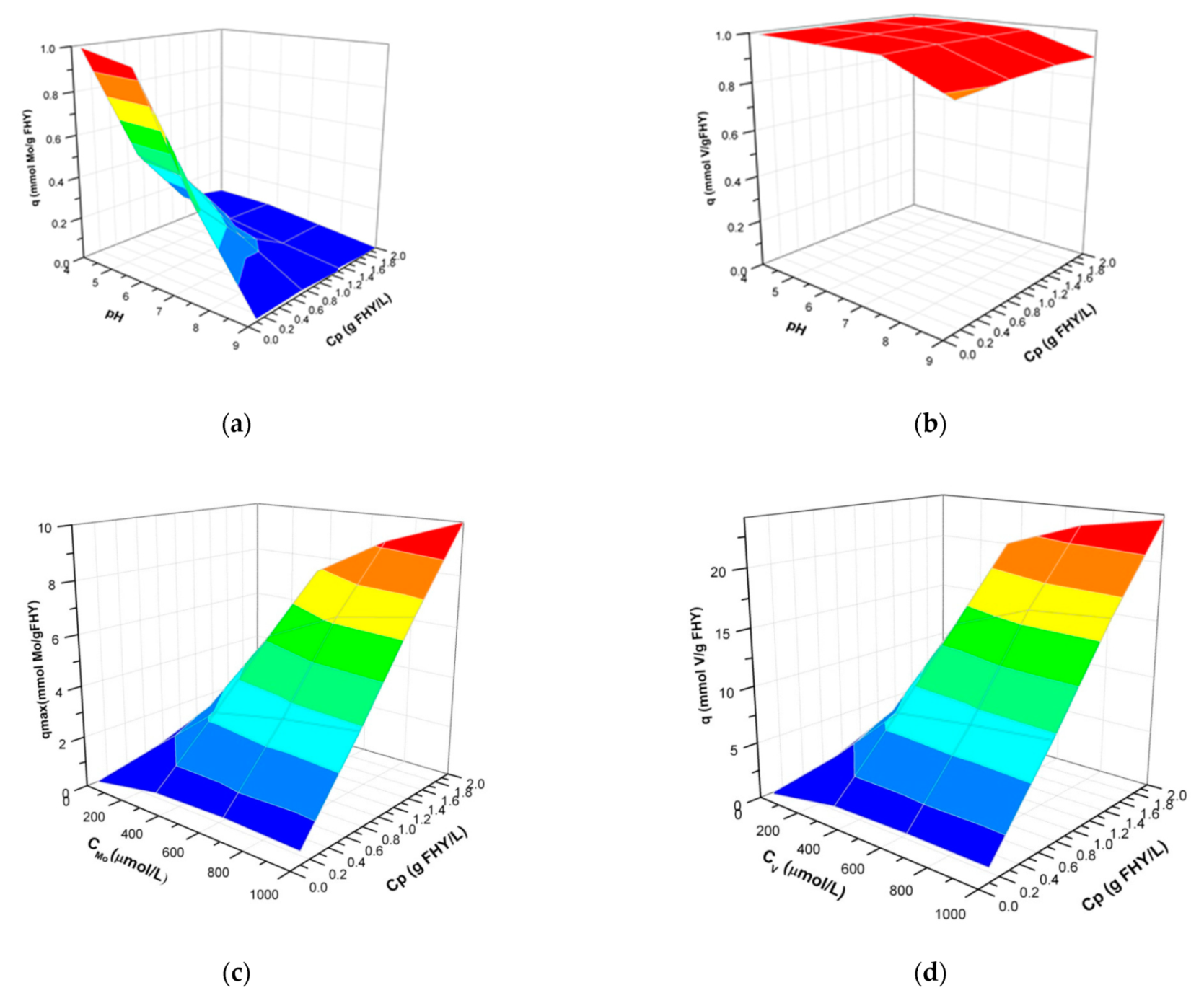
3.3. 3D Plotting of the Adsorption Parameters
4. Discussion
4.1. Ferrihydrite Characterization
4.2. Adsorption Studies
4.3. Simulation Outputs
4.4. Implications and Applications of the Results
5. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cornell, R.M.; Schwertmann, U. The Iron Oxides: Structure, Proprieties, Reactions, Occurances and Uses; Wiley-VCH GmbH&KGaA: Weinheim, Germany, 2003. [Google Scholar]
- Cornell, R.M.; Schwertmann, U. The Iron Oxides: Structure, Properties, Reactions, Occurrences and Uses; John Wiley & Sons: Hoboken, NJ, USA, 2006. [Google Scholar]
- United-States-Environmental-Protection-Agency. Drinking Water Contaminants—Standards and Regulations; Drinking Water Contaminant Candidate List 5; Washington, DC, USA, 2021. Available online: https://www.federalregister.gov/documents (accessed on 8 December 2021).
- Guvernul-României. NTPA-001/2002, Normativul Privind Stabilirea Limitelor de încărcare cu Poluanți a Apelor Uzate Industriale și Orășenești la Evacuarea în Receptorii Naturali. Monitorul Oficial, Partea I nr. 187 din 20 Martie 2002. Available online: http://legislatie.just.ro/Public/DetaliiDocumentAfis/98311 (accessed on 8 December 2021).
- Brinza, L.; Benning, L.G.; Statham, P.J. Adsorption studies of Mo and V onto ferrihydrite. Mineral. Mag. 2008, 72, 385–388. [Google Scholar] [CrossRef]
- Brinza, L.; Vu, H.P.; Neamtu, M.; Benning, L.G. Experimental and simulation results of the adsorption of Mo and V onto ferrihydrite. Sci. Rep. 2019, 9, 1365. [Google Scholar] [CrossRef] [PubMed]
- Das, S.; Essilfie-Dughan, J.; Jim Hendry, M. Sequestration of molybdate during transformation of 2-line ferrihydrite under alkaline conditions. Appl. Geochem. 2016, 73, 70–80. [Google Scholar] [CrossRef]
- Arai, Y. X-ray Absorption Spectroscopic Investigation of Molybdenum Multinuclear Sorption Mechanism at the Goethite—Water Interface. Environ. Sci. Technol. 2010, 44, 8491–8496. [Google Scholar] [CrossRef]
- Vessey, C.J.; Schmidt, M.P.; Abdolahnezhad, M.; Peak, D.; Lindsay, M.B.J. Adsorption of (Poly)vanadate onto Ferrihydrite and Hematite: An In Situ ATR–FTIR Study. ACS Earth Space Chem. 2020, 4, 641–649. [Google Scholar] [CrossRef]
- Larsson, M.A.; Persson, I.; Sjöstedt, C.; Gustafsson, J.P. Vanadate complexation to ferrihydrite: X-ray absorption spectroscopy and CD-MUSIC modelling. Environ. Chem. 2017, 14, 141–150. [Google Scholar] [CrossRef]
- Görn, M.G.; Bolanz, R.M.; Parry, S.; Göttlicher, J.; Steininger, R.; Majzlan, J. Incorporation of Mo6+ in ferrihydrite, goethite, and hematite. Clays Clay Miner. 2021, 69, 188–204. [Google Scholar] [CrossRef]
- Brinza, L.; Vu, H.P.; Shaw, S.; Mosselmans, J.F.W.; Benning, L.G. Effect of Mo and V on the Hydrothermal Crystallization of Hematite from Ferrihydrite: An in Situ Energy Dispersive X-ray Diffraction and X-ray Absorption Spectroscopy Study. Cryst. Growth Des. 2015, 15, 4768–4780. [Google Scholar] [CrossRef] [Green Version]
- Gustafsson, J.P. Modelling molybdate and tungstate adsorption to ferrihydrite. Chem. Geol. 2003, 200, 105–115. [Google Scholar] [CrossRef] [Green Version]
- United-States-Environmental-Protection-Agency. Understanding Variation in Partition Coefficient, Kd, Values. Volume I: The Kd Model, Methods of Measurement, and Application of Chemical Reaction Codes; Office of Radiation and Indoor Air, Office of Solid Waste and Emergency Response, U.S. Environmental Protection Agency; Office of Environmental Restoration, U.S. Department of Energy: Washington, DC, USA, 1999; p. 212. [Google Scholar]
- United-States-Environmental-Protection-Agency. Understanding Variation in Partition Coefficient, Kd, Values. Volume II: Review of Geochemistry and Available Kd Values for Cadmium, Cesium, Chromium, Lead, Plutonium, Radon, Strontium, Thorium, Tritium (3H), and Uranium; Office of Radiation and Indoor Air, Office of Solid Waste and Emergency Response, U.S. Environmental Protection Agency; Office of Environmental Restoration, U.S. Department of Energy: Washington DC, USA, 1999; p. 341. [Google Scholar]
- Hartzog, O.K.; Loganathan, V.A.; Kanel, S.R.; Jeppu, G.P.; Barnett, M.O. Normalization, comparison, and scaling of adsorption data: Arsenate and goethite. J. Colloid. Interface Sci. 2009, 333, 6–13. [Google Scholar] [CrossRef]
- Cornell, R.M.; Schwertmann, U. The Oxides in the Laboratory; Wiley-VCH: Weinheim, Germany, 2000. [Google Scholar]
- Polihrohiade, A. Absorbtia—Adsorbtia; Editura Tehnica: Bucuresti, Romania, 1967. [Google Scholar]
- Lagergren, S. Zur theorie der sogenannten adsorption gelöster stoffe, Kungliga Svenska Vetenskapsakademiens. Handlingar 1886, 24, 1–39. [Google Scholar]
- Ho, Y.S.; McKay, G. A Comparison of Chemisorption Kinetic Models Applied to Pollutant Removal on Various Sorbents. Process Saf. Environ. Prot. 1998, 76, 332–340. [Google Scholar] [CrossRef] [Green Version]
- Ho, Y.-S. Review of second-order models for adsorption systems. J. Hazard. Mater. 2006, 136, 681–689. [Google Scholar] [CrossRef] [Green Version]
- Abbas, M.S.; Ahmad, R. Equilibrium, kinetic and thermodynamic study of acid yellow-34 adsorption onto Cedrus deodara sawdust. Desalin. Water Treat. 2016, 57, 18175–18181. [Google Scholar] [CrossRef]
- Armagan, B.; Turan, M.; Karadag, D. Adsorption of Different Reactive Dyes onto Surfactant-Modified Zeolite: Kinetic and Equilibrium Modeling. In Survival and Sustainability: Environmental Concerns in the 21st Century; Gökçekus, H., Türker, U., LaMoreaux, J.W., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 1237–1254. [Google Scholar] [CrossRef]
- Benkaddour, S.; Slimani, R.; Hiyane, H.; El Ouahabi, I.; Hachoumi, I.; El Antri, S.; Lazar, S. Removal of reactive yellow 145 by adsorption onto treated watermelon seeds: Kinetic and isotherm studies. Sustain. Chem. Pharm. 2018, 10, 16–21. [Google Scholar] [CrossRef]
- Kaparapu, J.; Prasad, M.K. Equilibrium, kinetics and thermodynamic studies of cadmium(II) biosorption on Nannochloropsis oculata. Appl. Water Sci. 2018, 8, 9. [Google Scholar] [CrossRef] [Green Version]
- Prabakaran, R.; Arivoli, S. Adsorption kinetics, equilibrium and thermodynamic studies of Nickel adsorption onto Thespesia Populnea bark as biosorbent from aqueous solutions. Eur. J. Appl. Eng. Sci. Res. 2012, 1, 134–142. [Google Scholar]
- Al-Ghouti, M.A.; Da’ana, D.A. Guidelines for the use and interpretation of adsorption isotherm models: A review. J. Hazard. Mater. 2020, 393, 22. [Google Scholar] [CrossRef] [PubMed]
- Appelo, C.A.J.; Van Der Weiden, M.J.J.; Tournassat, C.; Charlet, L. Surface Complexation of Ferrous Iron and Carbonate on Ferrihydrite and the Mobilization of Arsenic. Environ. Sci. Technol. 2002, 36, 3096–3103. [Google Scholar] [CrossRef]
- Origin Pro, 8; Origin Lab, Northampton, MA, USA. Available online: http://www.originlab.com/ (accessed on 8 December 2021).
- Remy, N.; Boucher, A.; Wu, J. Applied Geostatistics with SGeMS: A User’s Guide; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar] [CrossRef]
- Gomes, M.V.C.; Bogle, I.D.L.; Biscaia, E.C.; Odloak, D. Using kriging models for real-time process optimisation. In Computer Aided Chemical Engineering; Braunschweig, B., Joulia, X., Eds.; Elsevier: Amsterdam, The Netherlands, 2008; Volume 25, pp. 361–366. [Google Scholar]
- Kleijnen, J. Kriging Metamodeling in Simulation: A Review. Eur. J. Oper. Res. 2007, 192, 707–716. [Google Scholar] [CrossRef] [Green Version]
- Quirante, N.; Javaloyes, J.; Ruiz-Femenia, R.; Caballero, J. Optimization of Chemical Processes Using Surrogate Models Based on a Kriging Interpolation. Comput. Aided Chem. Eng. 2015, 37, 179–184. [Google Scholar]
- Trivedi, P.; Dyer, J.A.; Sparks, D.L. Lead sorption onto ferrihydrite. 1. A macroscopic and spectroscopic assessment. Environ. Sci. Technol. 2003, 37, 908–914. [Google Scholar]
- Mendez, J.C.; Hiemstra, T. Surface area of ferrihydrite consistently related to primary surface charge, ion pair formation, and specific ion adsorption. Chem. Geol. 2020, 532, 119304. [Google Scholar] [CrossRef]
- Dyer, J.A.; Trivedi, P.; Scrivner, N.C.; Sparks, D.L. Lead sorption onto ferrihydrite. 2. Surface complexation modeling. Environ. Sci. Technol. 2003, 37, 915–922. [Google Scholar] [PubMed]
- Dyer, J.A.; Trivedi, P.; Scrivner, N.C.; Sparks, D.L. Surface complexation modeling of zinc sorption onto ferrihydrite. J. Colloid Interface Sci. 2004, 270, 56–65. [Google Scholar] [CrossRef]
- Alongi, K.S.; Shields, G.C. Chapter 8—Theoretical Calculations of Acid Dissociation Constants: A Review Article. In Annual Reports in Computational Chemistry; Wheeler, R.A., Ed.; Elsevier: Amsterdam, The Netherlands, 2010; Volume 6, pp. 113–138. [Google Scholar]
- Conover, W. Chemistry, 8th Edition (by Stephen S. Zumdahl and Susan A. Zumdahl). J. Chem. Educ. 2009, 86, 1273. [Google Scholar] [CrossRef]
- Zumdahl, S.A. Chemistry, 6th ed.; Houghton Mifflin: London, UK; Hi Marketing [distributor]: Boston, MA, USA, 2003. [Google Scholar]
- Rout, K.; Mohapatra, M.; Anand, S. 2-line ferrihydrite: Synthesis, characterization and its adsorption behaviour for removal of Pb(II), Cd(II), Cu(II) and Zn(II) from aqueous solutions. Dalton Trans. 2012, 41, 3302–3312. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Zhang, T.; Li, K. One-step synthesis of mesoporous two-line ferrihydrite for effective elimination of arsenic contaminants from natural water. Dalton Trans. 2011, 40, 2062–2066. [Google Scholar] [CrossRef]
- Macera, L.; Taglieri, G.; Daniele, V.; Passacantando, M.; D’Orazio, F. Nano-Sized Fe(III) Oxide Particles Starting from an Innovative and Eco-Friendly Synthesis Method. Nanomaterials 2020, 10, 323. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Trivedi, P.; Lisa, A. Ni and Zn sorption to amorphous versus crystalline iron oxides: Macroscopic studies. J. Colloid Interface Sci. 2001, 244, 221–229. [Google Scholar] [CrossRef]
- Dzombak, D.A.; Morel, F.M.M. Surface Complexation Modeling: Hydrous Ferric Oxide; John Wiley & Sons: Toronto, ON, Canada, 1990. [Google Scholar]
- Janney, D.E.; Cowley, J.M.; Buseck, P.R. Structure of synthetic 2-line ferrihydrite by electron nanodiffraction. Am. Mineral. 2000, 85, 1180–1187. [Google Scholar] [CrossRef]
- Janney, D.E.; Cowley, J.M.; Buseck, P.R. Transmission electron microscopy of synthetic 2- and 6-line ferrihydrite. Clays Clay Miner. 2000, 48, 111–119. [Google Scholar] [CrossRef]
- Raiswell, R.; Benning, L.G.; Davidson, L.; Tranter, M. Nanoparticulate bioavailable iron minerals in icebergs and glaciers. Mineral. Mag. 2008, 72, 345–348. [Google Scholar] [CrossRef]
- Macera, L.; Daniele, V.; Mondelli, C.; Capron, M.; Taglieri, G. New Sustainable, Scalable and One-Step Synthesis of Iron Oxide Nanoparticles by Ion Exchange Process. Nanomaterials 2021, 11, 798. [Google Scholar] [CrossRef]
- Scheinost, A.C.; Abend, S.; Pandya, K.I.; Sparks, D.L. Kinetic Controls on Cu and Pb Sorption by Ferrihydrite. Environ. Sci. Technol. 2001, 35, 1090–1096. [Google Scholar] [CrossRef]
- Bosch, J.; Heister, K.; Hofmann, T.; Meckenstock, R.U. Nanosized Iron Oxide Colloids Strongly Enhance Microbial Iron Reduction. Appl. Environ. Microbiol. 2010, 76, 184–189. [Google Scholar] [CrossRef] [Green Version]
- Du, H.; Xu, Z.; Hu, M.; Zhang, H.; Peacock, C.L.; Liu, X.; Nie, N.; Xue, Q.; Lei, M.; Tie, B. Natural organic matter decreases uptake of W(VI), and reduces W(VI) to W(V), during adsorption to ferrihydrite. Chem. Geol. 2020, 540, 119567. [Google Scholar] [CrossRef]
- Xue, Q.; Ran, Y.; Tan, Y.; Peacock, C.L.; Du, H. Arsenite and arsenate binding to ferrihydrite organo-mineral coprecipitate: Implications for arsenic mobility and fate in natural environments. Chemosphere 2019, 224, 103–110. [Google Scholar] [CrossRef] [PubMed]
- Mendez, J.C.; Hiemstra, T. Carbonate Adsorption to Ferrihydrite: Competitive Interaction with Phosphate for Use in Soil Systems. ACS Earth Space Chem. 2019, 3, 129–141. [Google Scholar] [CrossRef]
- Song, Y.; Swedlund, P.J.; Singhal, N. Copper(II) and Cadmium(II) Sorption onto Ferrihydrite in the Presence of Phthalic Acid: Some Properties of the Ternary Complex. Environ. Sci. Technol. 2008, 42, 4008–4013. [Google Scholar] [CrossRef] [PubMed]
- Vu, H.P.; Shaw, S.; Brinza, L.; Benning, L.G. Crystallization of Hematite (α-Fe2O3) under Alkaline Condition: The Effects of Pb. Cryst. Growth Des. 2010, 10, 1544–1551. [Google Scholar] [CrossRef]
- Raiswell, R.; Vu, H.P.; Brinza, L.; Benning, L.G. The determination of labile Fe in ferrihydrite by ascorbic acid extraction: Methodology, dissolution kinetics and loss of solubility with age and de-watering. Chem. Geol. 2010, 278, 70–79. [Google Scholar] [CrossRef]
- Trefry, J.H.; Metz, S. Role of hydrothermal precipitates in the geochemical cycling of vanadium. Lett. Nat. 1989, 432, 531–534. [Google Scholar] [CrossRef]
- Belova, D.A.; Lakshtanov, L.Z.; Stipp, S.L.S. Experimental study of Ni adsorption on chalk: Preliminary results. Mineral. Mag. 2008, 72, 377–379. [Google Scholar] [CrossRef]
- Sannino, F.; De Martino, A.; Pigna, M.; Violante, A.; Di Leo, P.; Mesto, E.; Capasso, R. Sorption of arsenate and dichromate on polymerin, Fe(OH)(x)-polymerin complex and ferrihydrite. J. Hazard. Mater. 2009, 166, 1174–1179. [Google Scholar] [CrossRef]
- Aldmour, S.T.; Burke, I.T.; Bray, A.W.; Baker, D.L.; Ross, A.B.; Gill, F.L.; Cibin, G.; Ries, M.E.; Stewart, D.I. Abiotic reduction of Cr(VI) by humic acids derived from peat and lignite: Kinetics and removal mechanism. Environ. Sci. Pollut. Res. 2019, 26, 4717–4729. [Google Scholar] [CrossRef] [Green Version]
- Burke, I.T.; Mayes, W.M.; Peacock, C.L.; Brown, A.P.; Jarvis, A.P.; Gruiz, K. Speciation of Arsenic, Chromium, and Vanadium in Red Mud Samples from the Ajka Spill Site, Hungary. Environ. Sci. Technol. 2012, 46, 3085–3092. [Google Scholar] [CrossRef] [PubMed]
- He, Q.; Si, S.; Zhao, J.; Yan, H.; Sun, B.; Cai, Q.; Yu, Y. Removal of vanadium from vanadium-containing wastewater by amino modified municipal sludge derived ceramic. Saudi J. Biol. Sci. 2018, 25, 1664–1669. [Google Scholar] [CrossRef] [Green Version]
- Gupta, A. Heavy Metal and Metalloid Contamination of Surface and Underground Water: Environmental, Policy and Ethical Issues; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar]
- Chester, R. Marine Geochemistry; Unwin Hyman Ltd.: London, UK, 1990. [Google Scholar]
- Ferreira, S.L.C.; Queiroz, A.S.; Fernandes, M.S.; Santos, H.C.D. Application of factorial designs and Doehlert matrix in optimization of experimental variables associated with the preconcentration and determination of vanadium and copper in seawater by inductively coupled plasma optical emission spectrometry. Spectrochim. Acta Part B 2002, 57, 1939–1950. [Google Scholar] [CrossRef] [Green Version]
- Kashiwabara, T.; Takahashi, Y.; Tanimizu, M.; Usui, A. Molecular-scale mechanisms of distribution and isotopic fractionation of molybdenum between seawater and ferromanganese oxides. Geochim. Cosmochim. Acta 2011, 75, 5762–5784. [Google Scholar] [CrossRef]
- Collier, R.W. Molybdenum in the ortheast Pacific Ocean. Limnol. Oceanogr. 1985, 30, 1351–1354. [Google Scholar] [CrossRef]
- Kashiwabara, T.; Takahashi, Y.; Tanimizu, M. XAFS study on the mechanism of isotopic fractionation of molybdenum during its adsorption on ferromanganese oxides. Geochem. J. 2009, 43, e31–e36. [Google Scholar] [CrossRef] [Green Version]
- Feely, R.A.; Massoth, G.J.; Baker, E.T.; Lebon, G.T.; Geiselman, T.L. Tracking the dispersal of hydrothermal plumes from the Juan de Fuca Ridge using suspended matter compositions. J. Geophys. Res. Solid Earth 1992, 97, 3457–3468. [Google Scholar] [CrossRef]
- Metz, S.; Trefry, J.H. Scavenging of vanadium by Iron Oxides in Hydrotermal Plumes. Eos 1988, 69, 148. [Google Scholar]

| System | Surface Sites Available (Mols Site L−1) | Th. Surf. Coverage | % th ads | % pr ads | q th (mmol g−1 FHY) | q pr (mmol g−1 FHY) | Pr R2 | Calculated Kd (L g−1) |
|---|---|---|---|---|---|---|---|---|
| V-Cp = 0.1 g L−1 | 7.54 × 10−5 | 100% | 75% | 100% | 0.75 | 0.98 ± 0.001 | 0.999 | 749.323 |
| V-Cp = 1 g L−1 | 7.54 × 10−4 | 13% | 100% | 100% | 0.1 | 0.974 ± 0.0019 | 0.999 | 453.820 |
| V-Cp = 2 g L−1 | 15.08 × 10−4 | 7% | 100% | 100% | 0.05 | 0.98 ± 0.00007 | 1 | 443.667 |
| Mo-Cp = 0.1 g L−1 | 7.54 × 10−5 | 100% | 75% | 40% | 0.750 | 0.468 ± 0.035 | 0.883 | 9.107 |
| Mo-Cp = 1 g L−1 | 7.54 × 10−4 | 13% | 100% | 100% | 0.1 | 0.0994 ± 0.00002 | 1 | 2983.375 |
| Mo-Cp = 2 g L−1 | 15.08 × 10−4 | 7% | 100% | 100% | 0.05 | 0.053 ± 0.00002 | 0.999 | 634.735 |
| Mo/V Initial Concentration | RL | Kd (L g−1) | ||
|---|---|---|---|---|
| V | Mo | V | Mo | |
| 1 | 0.720 | 1.000 | 236.775 | 539.686 |
| 10 | 0.205 | 1.000 | 71.983 | 380.90 |
| 100 | 0.025 | 0.999 | 55.956 | 4.925 |
| 250 | 0.010 | 0.997 | 7.377 | 1.265 |
| 500 | 0.005 | 0.993 | 2.660 | 0.974 |
| 750 | 0.003 | 0.990 | 2.161 | 0.871 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brinza, L. Surface Coverage Simulation and 3D Plotting of Main Process Parameters for Molybdenum and Vanadium Adsorption onto Ferrihydrite. Nanomaterials 2022, 12, 304. https://doi.org/10.3390/nano12030304
Brinza L. Surface Coverage Simulation and 3D Plotting of Main Process Parameters for Molybdenum and Vanadium Adsorption onto Ferrihydrite. Nanomaterials. 2022; 12(3):304. https://doi.org/10.3390/nano12030304
Chicago/Turabian StyleBrinza, Loredana. 2022. "Surface Coverage Simulation and 3D Plotting of Main Process Parameters for Molybdenum and Vanadium Adsorption onto Ferrihydrite" Nanomaterials 12, no. 3: 304. https://doi.org/10.3390/nano12030304
APA StyleBrinza, L. (2022). Surface Coverage Simulation and 3D Plotting of Main Process Parameters for Molybdenum and Vanadium Adsorption onto Ferrihydrite. Nanomaterials, 12(3), 304. https://doi.org/10.3390/nano12030304






