An Innovative Polarisation-Insensitive Perfect Metamaterial Absorber with an Octagonal-Shaped Resonator for Energy Harvesting at Visible Spectra
Abstract
1. Introduction
2. Materials and Design Technique
3. Results and Discussion
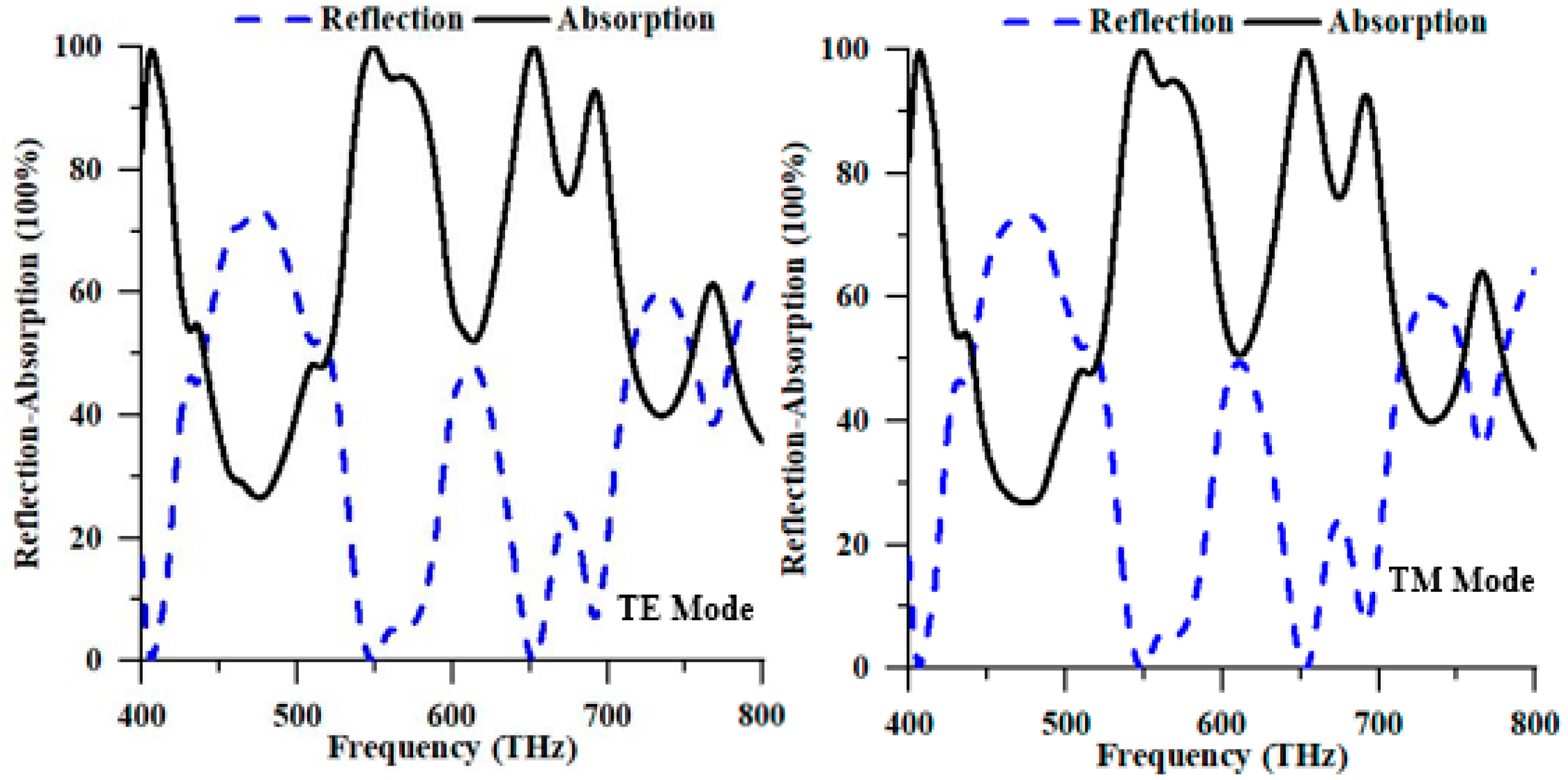
3.1. Absorption Characteristics
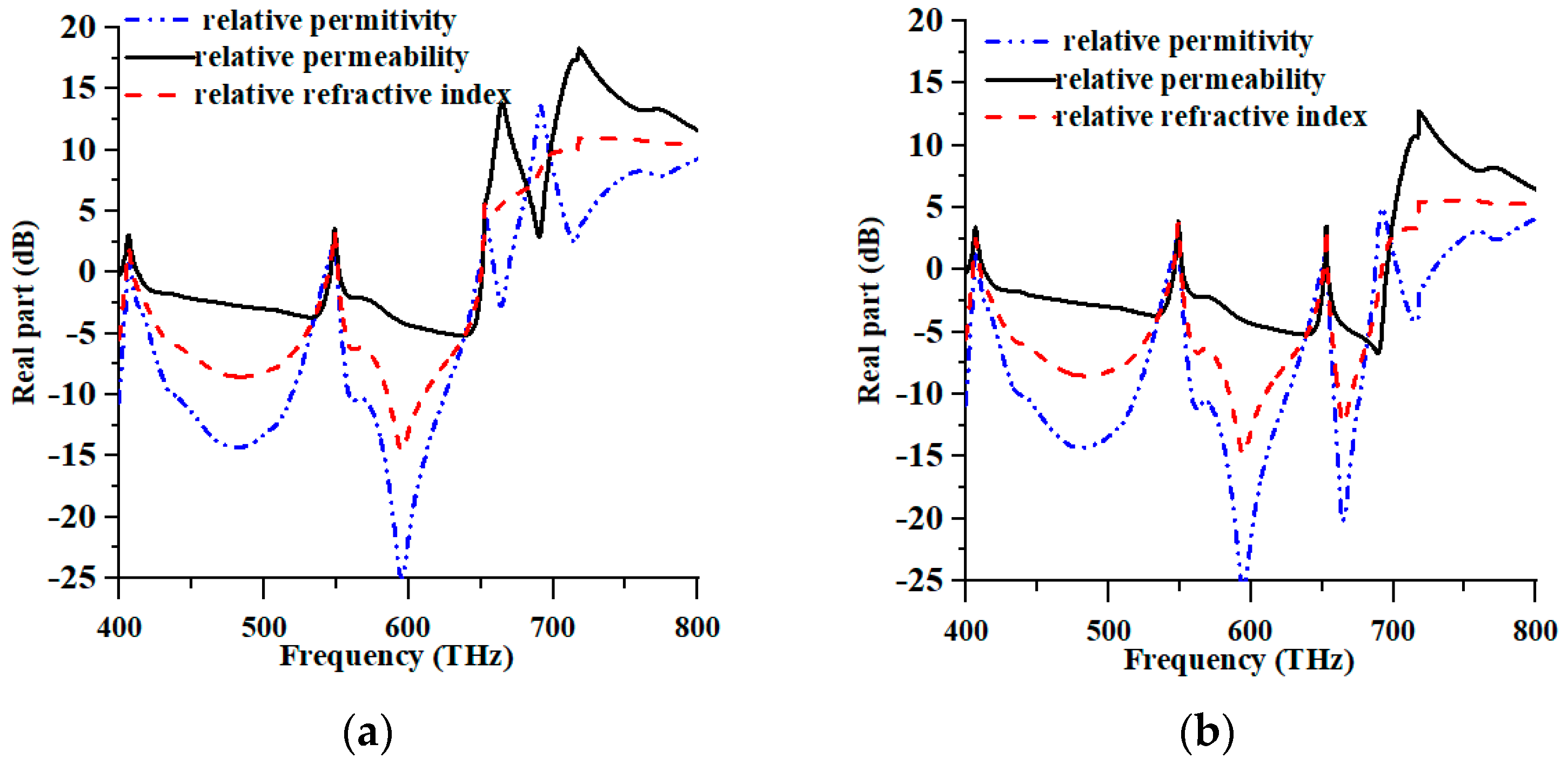
3.2. Metamaterial Characteristics
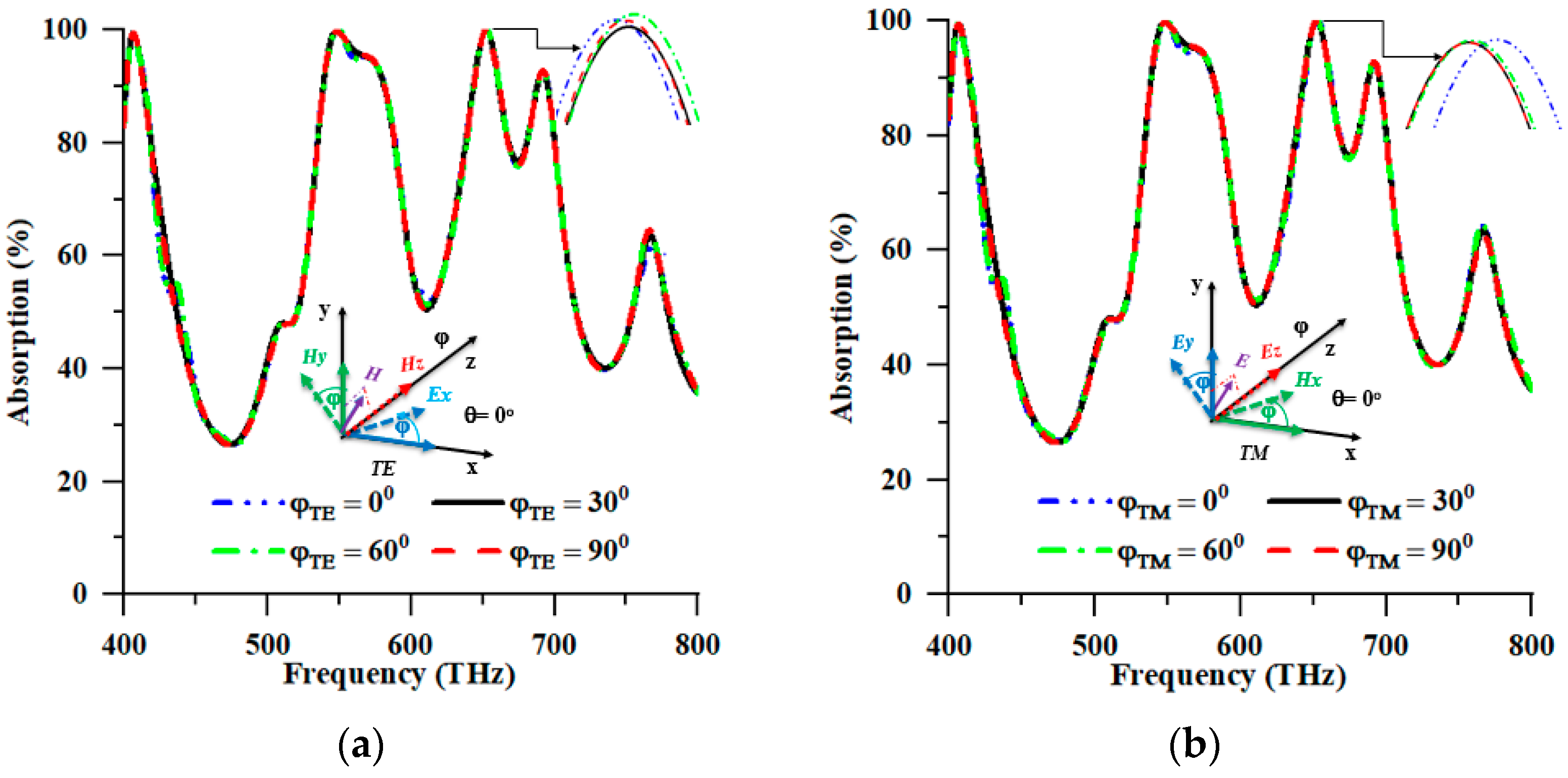
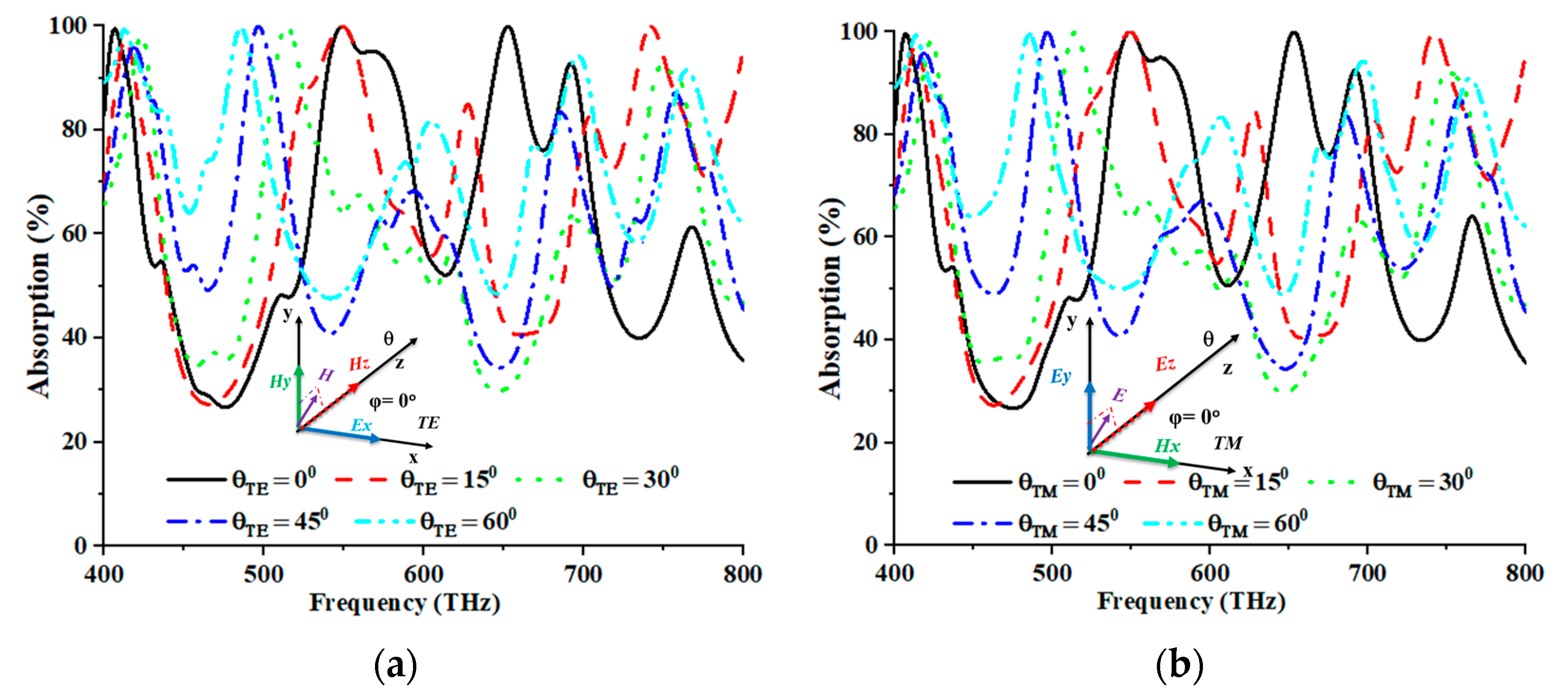
3.3. Polarisation Insensitivity and Incident Angle Stability
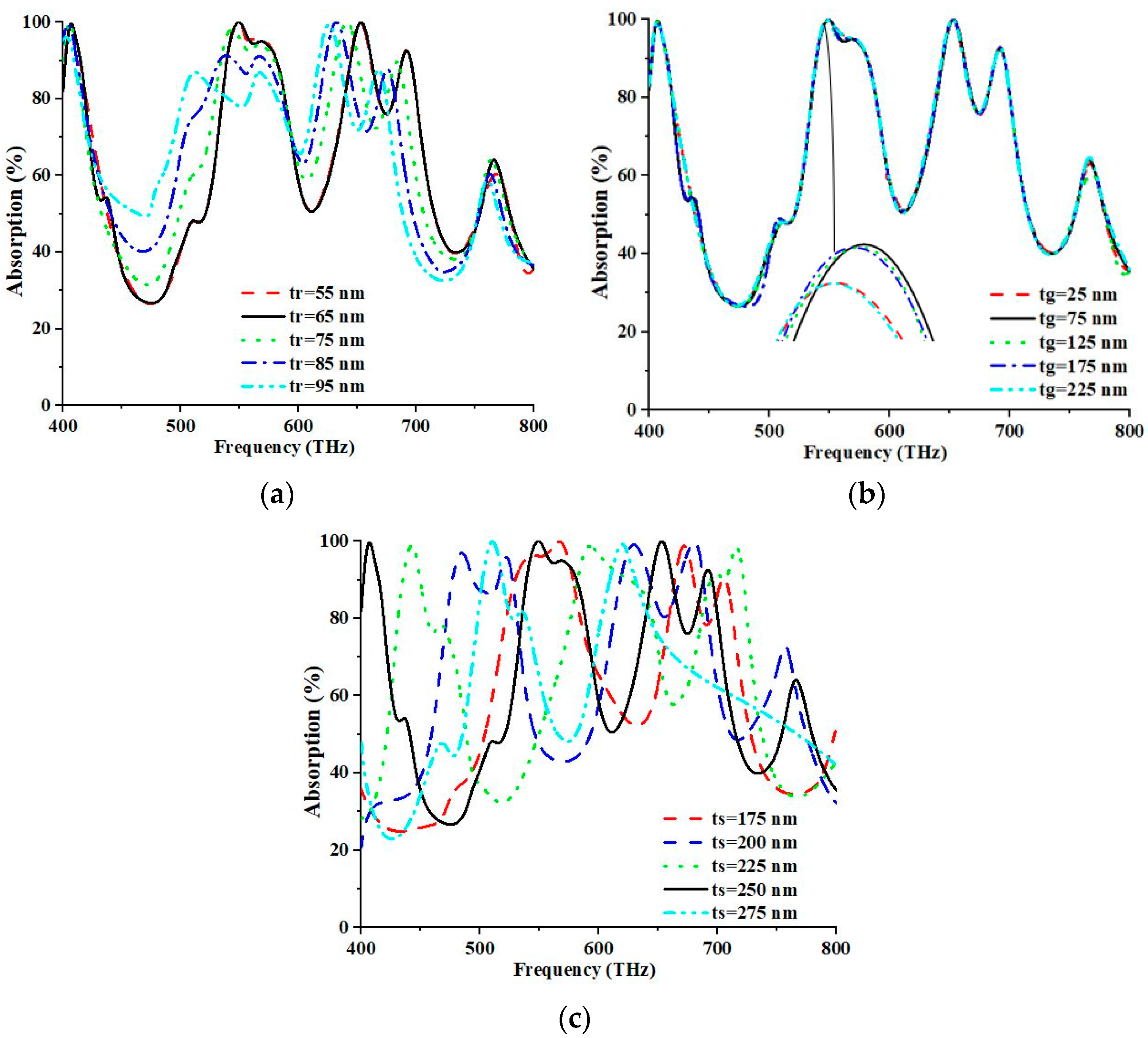
4. Parametric Sweep
4.1. Sweep of Top, Ground Resonator, and Substrate Thickness
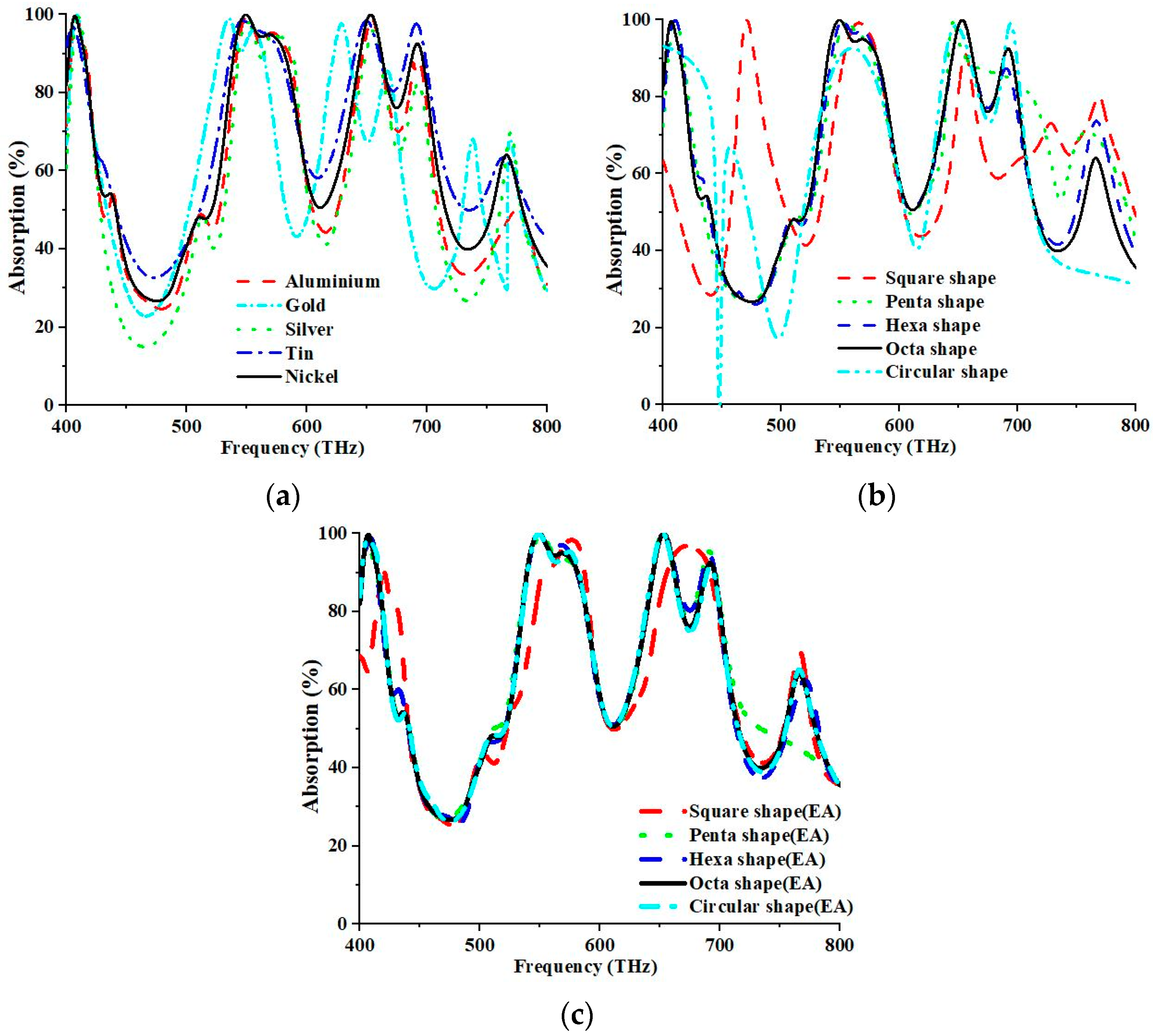
4.2. Sweep of Resonator’s Material and Resonator Shape
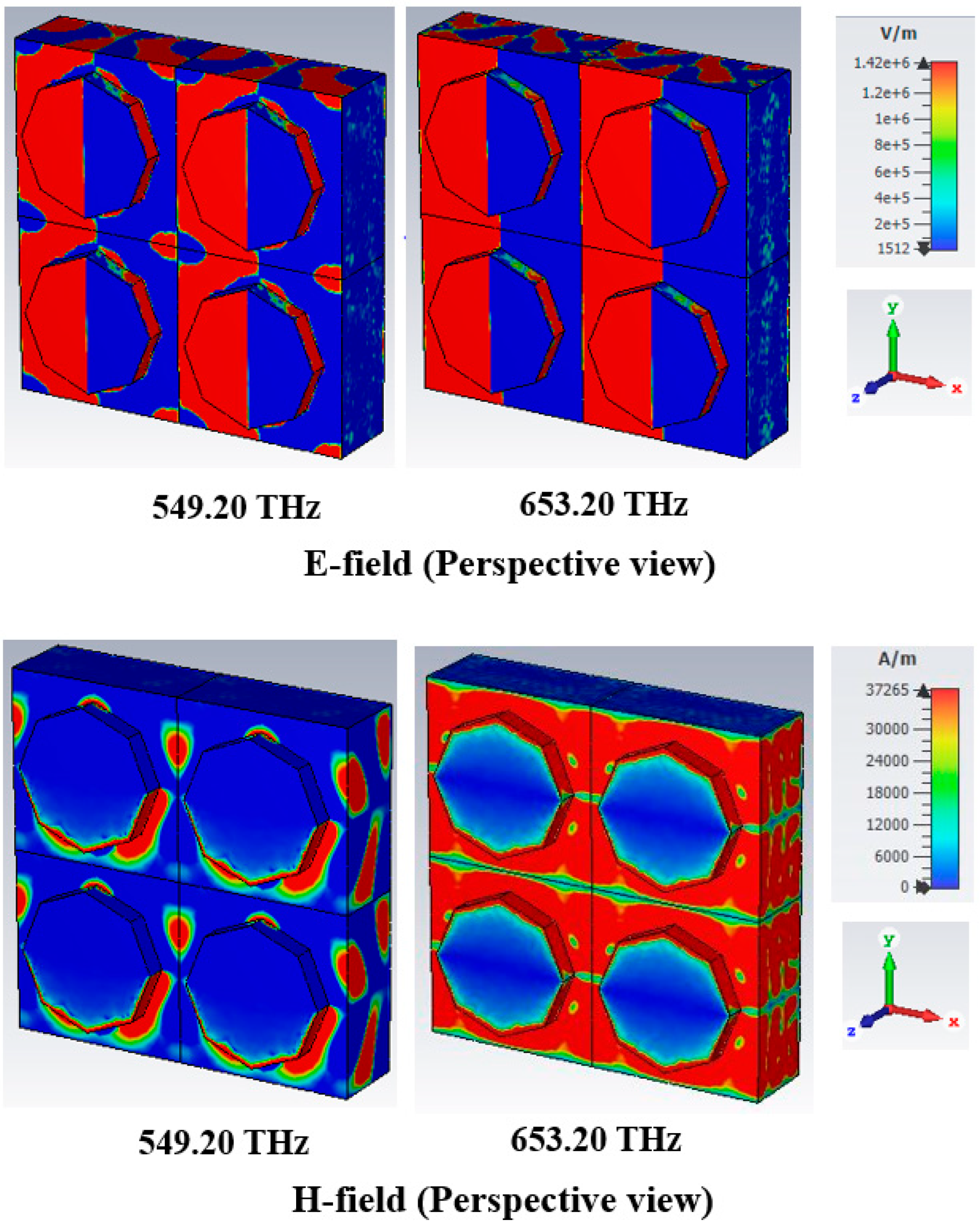
4.3. Response of Electric Field and Magnetic Field
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mitzi, D.B.; Gunawan, O.; Todorov, T.K.; Barkhouse, D.A.R. Prospects and performance limitations for Cu-Zn-Sn-S-Se photovoltaic technology. Philos. Trans. R. Soc. A 2013, 371, 20110432. [Google Scholar] [CrossRef]
- Kumar, N.S.; Naidu, K.C.B.; Banerjee, P.; Babu, T.A.; Reddy, B.V.S. A review on metamaterials for device applications. Crystals 2021, 11, 518. [Google Scholar] [CrossRef]
- Landy, N.I.; Sajuyigbe, S.; Mock, J.J.; Smith, D.R.; Padilla, W.J. Perfect metamaterial absorber. Phys. Rev. Lett. 2008, 100, 207402. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Sun, X.; Ma, C.; Li, J.; Li, X.; Guan, B.O.; Chen, K. Ultra-narrow-band metamaterial perfect absorber based on surface lattice resonance in a WS 2 nanodisk array. Opt. Express 2021, 29, 27084–27091. [Google Scholar] [CrossRef]
- Kumar, R.; Singh, B.K.; Pandey, P.C. Broadband metamaterial absorber in the visible region using a petal-shaped resonator for solar cell applications. Phys. E 2022, 142, 115327. [Google Scholar] [CrossRef]
- Huang, Y.; Liu, L.; Pu, M.; Li, X.; Ma, X.; Luo, X. A refractory metamaterial absorber for ultra-broadband, omnidirectional and polarization-independent absorption in the UV-NIR spectrum. Nanoscale 2018, 10, 8298–8303. [Google Scholar] [CrossRef] [PubMed]
- Abou Houran, M.; Alsharai, M.; Baqir, M.A.; Saqlain, M.; Dhasarathan, V. Polarization-Insensitive and Wide-Angle Absorber Operating in the Visible and Near-infrared Regimes. Optik 2023, 283, 170915. [Google Scholar] [CrossRef]
- Liu, Y.; Ma, W.Z.; Wu, Y.C.; Meng, D.; Dou, C.; Cheng, Y.Y.; Chen, Y.S.; Liu, J.; Gu, Y. A metamaterial absorber with a multi-layer metal-dielectric grating structure from visible to near-infrared. Opt. Commun. 2023, 542, 129588. [Google Scholar] [CrossRef]
- Shi, Y.; Du, J.; Qiu, J. A novel double 3D continuous phase composite with ultra-broadband wave absorption from gigahertz to UV–vis-NIR for extremely cold environment. Chem. Eng. J. 2022, 436, 135220. [Google Scholar] [CrossRef]
- Huang, Y.; Luo, J.; Pu, M.; Guo, Y.; Zhao, Z.; Ma, X.; Li, X.; Luo, X. Catenary electromagnetics for ultra-broadband lightweight absorbers and large-scale flat antennas. Adv. Sci. 2019, 6, 1801691. [Google Scholar] [CrossRef]
- Contractor, R.; D’Aguanno, G.; Menyuk, C. Ultra-broadband, polarization-independent, wide-angle absorption in impedance-matched metamaterials with anti-reflective moth-eye surfaces. Opt. Express 2018, 26, 24031–24043. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Luo, C.; Yao, G.; Yue, J.; Ji, J.; Yao, J.; Ling, F. Design of a concise and dual-band tunable metamaterial absorber. Chin. Opt. Lett. 2016, 14, 102303. [Google Scholar]
- Mulla, B.; Sabah, C. Multiband metamaterial absorber design based on plasmonic resonances for solar energy harvesting. Plasmonics 2016, 11, 1313–1321. [Google Scholar] [CrossRef]
- Ferraro, A.; Lio, G.E.; Hmina, A.; Palermo, G.; Djouda, J.M.; Maurer, T.; Caputo, R. Tailoring of plasmonic functionalized metastructures to enhance local heating release. Nanophotonics 2021, 10, 3907–3916. [Google Scholar] [CrossRef]
- Hossain, M.J.; Faruque, M.R.I.; Ahmed, R.; Alam, J.; Islam, M.T. Polarization-insensitive infrared-visible perfect metamaterial absorber and permittivity sensor. Results Phys. 2019, 14, 102429. [Google Scholar] [CrossRef]
- Mahmud, S.; Islam, S.S.; Mat, K.; Chowdhury, M.E.H.; Rmili, H.; Islam, M.T. Design and parametric analysis of a wide-angle polarization-insensitive metamaterial absorber with a star shape resonator for optical wavelength applications. Results Phys. 2020, 18, 103259. [Google Scholar] [CrossRef]
- Zhu, P.; Guo, L.J. High performance broadband absorber in the visible band by engineered dispersion and geometry of a metal-dielectric-metal stack. Appl. Phys. Lett. 2012, 101, 241116. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, X.; Huang, S.; Pan, P.; Chen, J.; Liu, G.; Gu, G. Automatically Acquired Broadband Plasmonic-Metamaterial Black Absorber during the Metallic Film-Formation. ACS Appl. Mater. Interfaces 2015, 7, 4962–4968. [Google Scholar] [CrossRef]
- Butun, S.; Aydin, K. Structurally tunable resonant absorption bands in ultrathin broadband plasmonic absorbers. Opt. Express 2014, 22, 19457–19468. [Google Scholar] [CrossRef]
- Hoa, N.T.Q.; Tung, P.D.; Lam, P.H.; Dung, N.D.; Quang, N.H. Numerical Study of an Ultrabroadband, Wide-Angle, Polarization-Insensitivity Metamaterial Absorber in the Visible Region. J. Electron. Mater. 2018, 47, 2634–2639. [Google Scholar] [CrossRef]
- Luo, M.; Shen, S.; Zhou, L.; Wu, S.; Zhou, Y.; Chen, L. Broadband, wide-angle, and polarization-independent metamaterial absorber for the visible regime. Opt. Express 2017, 25, 16715–16724. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Chen, J.; Liu, H.; Liu, Y.; Zhu, L. Numerical study of an ultra-broadband and polarization independence metamaterial cross-shaped fractal absorber. Plasmonics 2020, 15, 1517–1524. [Google Scholar] [CrossRef]
- Liang, Q.; Duan, H.; Zhu, X.; Chen, X.; Xia, X. Solar thermal absorber based on dielectric filled two-dimensional nickel grating. Opt. Mater. Express 2019, 9, 3193. [Google Scholar] [CrossRef]
- Azad, A.K.; Kort-Kamp, W.J.; Sykora, M.; Weisse-Bernstein, N.R.; Luk, T.S.; Taylor, A.J.; Chen, H.T. Metasurface broadband solar absorber. Sci. Rep. 2016, 6, 20347. [Google Scholar] [CrossRef]
- Gamaly, E.G.; Rode, A.V. Transient optical properties of dielectrics and semiconductors excited by an ultrashort laser pulse. J. Opt. Soc. Am. B 2014, 31, C36–C43. [Google Scholar] [CrossRef]
- Cen, C.; Chen, Z.; Xu, D.; Jiang, L.; Chen, X.; Yi, Z.; Wu, P.; Li, G.; Yi, Y. High quality factor, high sensitivity metamaterial graphene—Perfect absorber based on critical coupling theory and impedance matching. Nanomaterials 2020, 10, 95. [Google Scholar] [CrossRef]
- Zhu, W. Electromagnetic metamaterial absorbers: From narrowband to broadband. In Metamaterials and Metasurfaces; IntechOpen: London, UK, 2018. [Google Scholar]
- Yu, P.; Yang, H.; Chen, X.; Yi, Z.; Yao, W.; Chen, J.; Yi, Y.; Wu, P. Ultra-wideband solar absorber based on refractory titanium metal. Renew. Energy 2020, 158, 227–235. [Google Scholar]
- Katrodiya, D.; Jani, C.; Sorathiya, V.; Patel, S.K. Metasurface based broadband solar absorber. Opt. Mater. 2019, 89, 34–41. [Google Scholar] [CrossRef]
- Wu, B.; Liu, Z.; Liu, G.; Liu, X.; Tang, P.; Du, G.; Yuan, W.; Liu, M. An ultra-broadband, polarization and angle-insensitive metamaterial light absorber. J. Phys. D Appl. Phys. 2020, 53, 095106. [Google Scholar] [CrossRef]
- Jiao, S.; Li, Y.; Yang, H.; Xu, S. Numerical study of ultra-broadband wide-angle absorber. Results Phys. 2021, 24, 104146. [Google Scholar] [CrossRef]
- Qin, F.; Chen, Z.; Chen, X.; Yi, Z.; Yao, W.; Duan, T.; Wu, P.; Yang, H.; Li, G.; Yi, Y. A tunable triple-band near-infrared metamaterial absorber based on Au nano-cuboids array. Nanomaterials 2020, 10, 207. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, Z.; Xu, D.; Yi, Z.; Chen, X.; Chen, J.; Tang, Y.; Wu, P.; Li, G.; Yi, Y. Triple-band perfect metamaterial absorber with good operating angle polarization tolerance based on split ring arrays. Results Phys. 2020, 16, 102951. [Google Scholar] [CrossRef]
- Wu, P.; Zhang, C.; Tang, Y.; Liu, B.; Lv, L. A perfect absorber based on similar fabry-perot four-band in the visible range. Nanomaterials 2020, 10, 488. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Ni, B.; Liu, F.; Chen, L.; Wang, R. Design of a Visible Broadband Metamaterial Absorber Based on Nickel Metal. J. Russ. Laser Res. 2022, 43, 600–606. [Google Scholar] [CrossRef]
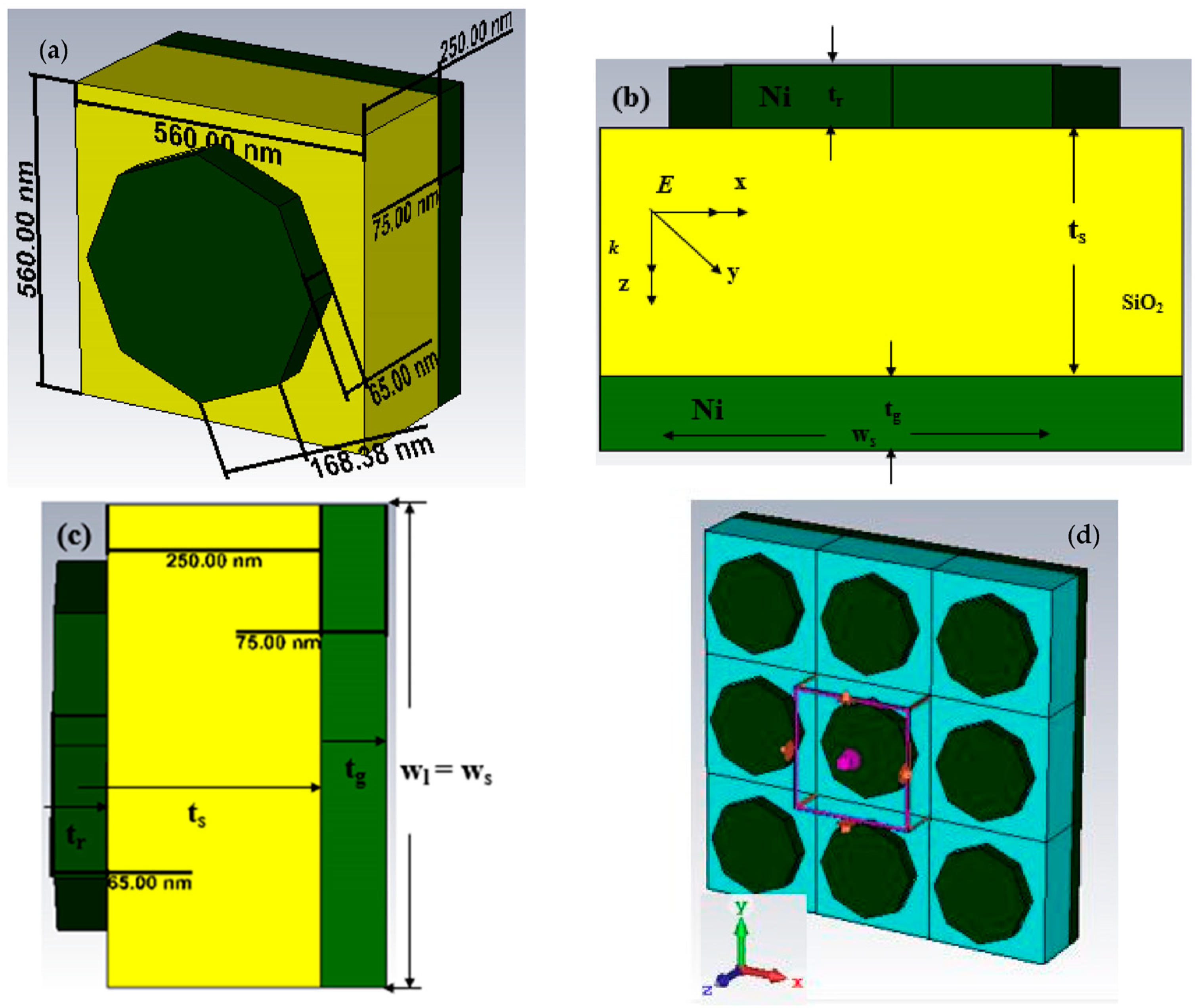



| Parameters | Value (nm) | Parameters | Value (nm) |
|---|---|---|---|
| Substrate width, ws | 560 | Resonator’s sidearm, s | 168.38 |
| Substrate length, ls | 560 | Resonator’s thickness, tr | 65 |
| Substrate height, hs | 250 | Ground plane’s thickness, tg | 75 |
| Resonator’s radius, Rr | 220 |
| Mode | Polarisation Angle (φ) | Absorption Peak at 549.20 THz | Absorption Peak | Frequency |
|---|---|---|---|---|
| TE | 0° | 99.979% | 99.993% | 652.40 THz |
| 30° | 99.667% | 99.984% | ||
| 60° | 99.966% | 99.987% | ||
| 90° | 99.646% | 99.989% | ||
| TM | 0° | 99.987% | 99.997% | 653.20 THz |
| 30° | 99.676% | 99.922% | ||
| 60° | 99.966% | 99.941% | ||
| 90° | 99.646% | 99.921% |
| Mode | Incident Angle (θ) | Absorption Peak | Frequency at THz | Absorption Peak | Frequency at THz |
|---|---|---|---|---|---|
| TE | 0° | 99.979% | 549.20 | 99.993% | 652.40 |
| 15° | 99.992% | 548.80 | 99.939% | 742.00 | |
| 30° | 99.922% | 514.40 | 92.292% | 748.80 | |
| 45° | 99.534% | 498.00 | 83.467% | 685.60 | |
| 60° | 99.539% | 485.20 | 91.479% | 764.00 | |
| TM | 0° | 99.987% | 549.20 | 99.997% | 653.20 |
| 15° | 99.994% | 549.20 | 84.766% | 627.60 | |
| 30° | 99.857% | 514.40 | 92.179% | 751.20 | |
| 45° | 99.928% | 496.40 | 83.633% | 686.00 | |
| 60° | 99.489% | 485.20 | 94.232% | 696.80 |
| Ref. | Dimension (nm3) | Materials | Bandwidth (nm) | Polarisation Insensitivity (φ) and Incident Stability (θ) | Average Absorption | Peak Absorption |
|---|---|---|---|---|---|---|
| [28] | 500 × 500 × 450 | W, SiO2, Au | 1759.80 | N/A | 93.17% | 98.63% |
| [29] | 1000 × 1000 × 310 | Au, SiO2 | 1234 | N/A | 80.24% | 96.40% |
| [30] | 400 × 400 × 295 | Ti, SiO2 | 1376 | 94.60% | 97.7% | |
| [31] | 1000 × 1000 × 320 | Ti, Al2O3, W | 1300 | 94.00% | 99.99% | |
| [32] | 600 × 600 × 550 | Au, SiO2, Au | 1500 | 98.53 | 99.60% | |
| [33] | 800 × 800 × 300 | Cu, SiO2, Cu | 700 | 95.53 | 99.90% | |
| [34] | 1000 × 1000 × 530 | Au, SiO2, Au | 350 | 97.30% | 99.90% | |
| [35] | 500 × 500 × 130 | Ni, SiO2, Ni | 370 | Yes, No | 95.76% | 99.93% |
| Proposed | 560 × 560 × 75 | Ni, SiO2, Ni | 375 | 99.992% | 99.997% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hossain, M.J.; Rahman, M.H.; Faruque, M.R.I. An Innovative Polarisation-Insensitive Perfect Metamaterial Absorber with an Octagonal-Shaped Resonator for Energy Harvesting at Visible Spectra. Nanomaterials 2023, 13, 1882. https://doi.org/10.3390/nano13121882
Hossain MJ, Rahman MH, Faruque MRI. An Innovative Polarisation-Insensitive Perfect Metamaterial Absorber with an Octagonal-Shaped Resonator for Energy Harvesting at Visible Spectra. Nanomaterials. 2023; 13(12):1882. https://doi.org/10.3390/nano13121882
Chicago/Turabian StyleHossain, Mohammad Jakir, Md. Habibur Rahman, and Mohammad Rashed Iqbal Faruque. 2023. "An Innovative Polarisation-Insensitive Perfect Metamaterial Absorber with an Octagonal-Shaped Resonator for Energy Harvesting at Visible Spectra" Nanomaterials 13, no. 12: 1882. https://doi.org/10.3390/nano13121882
APA StyleHossain, M. J., Rahman, M. H., & Faruque, M. R. I. (2023). An Innovative Polarisation-Insensitive Perfect Metamaterial Absorber with an Octagonal-Shaped Resonator for Energy Harvesting at Visible Spectra. Nanomaterials, 13(12), 1882. https://doi.org/10.3390/nano13121882







