Semi-Empirical Pseudopotential Method for Graphene and Graphene Nanoribbons
Abstract
:1. Introduction
2. Calculation Methods
2.1. B-Splines
2.2. Kinetic and Overlap Matrix Elements within Planar Basis
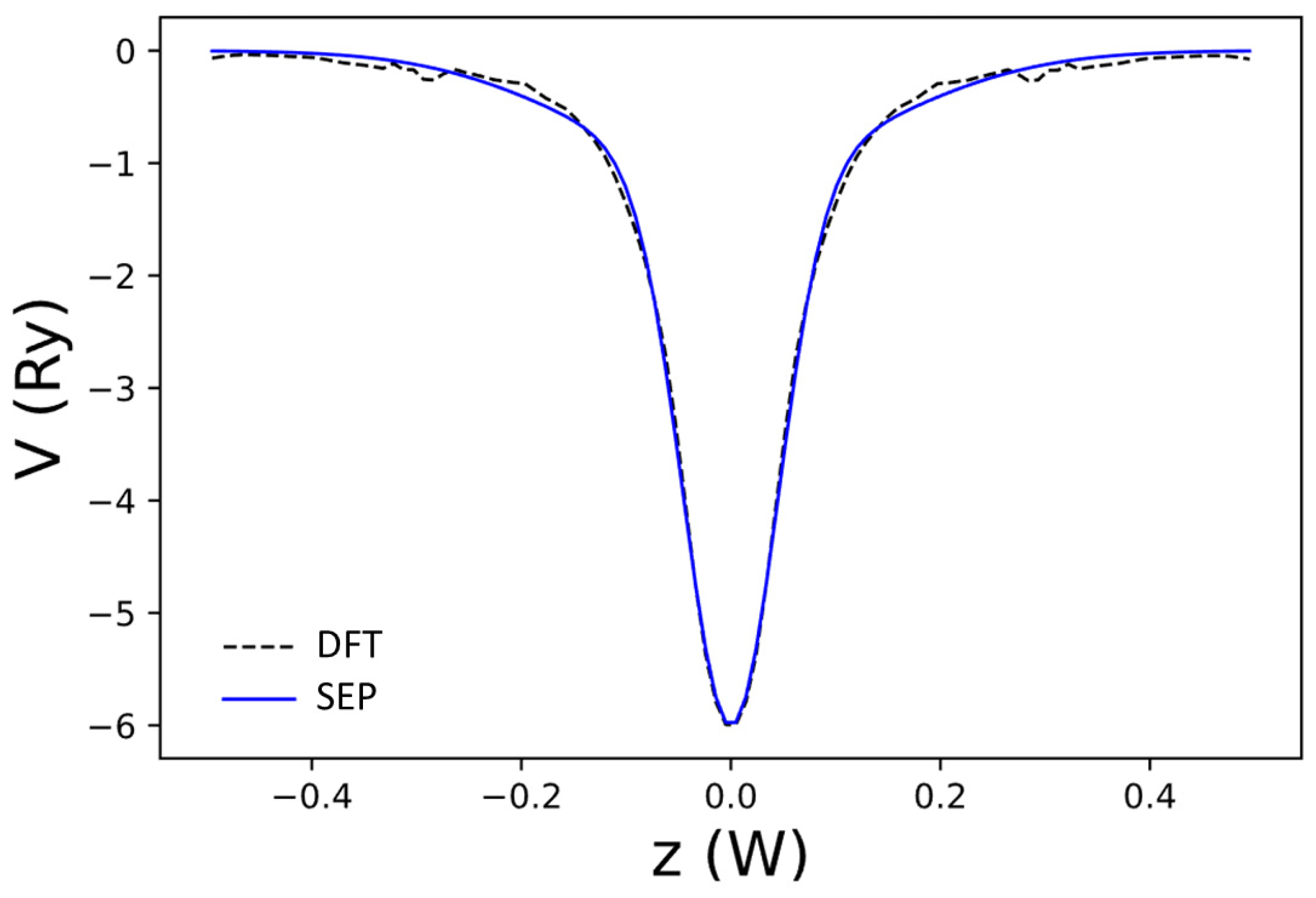
2.3. Implementation of the Semi-Empirical Local Pseudopotential for Graphene
2.4. Fitting of the Non-Local Pseudopotential for Graphene
2.5. Matrix Elements of Local and Nonlocal Pseudopotential
2.6. Nonlocal Corrections in Overlap and Potential
3. Results and Discussions
3.1. Band Structure of Graphene
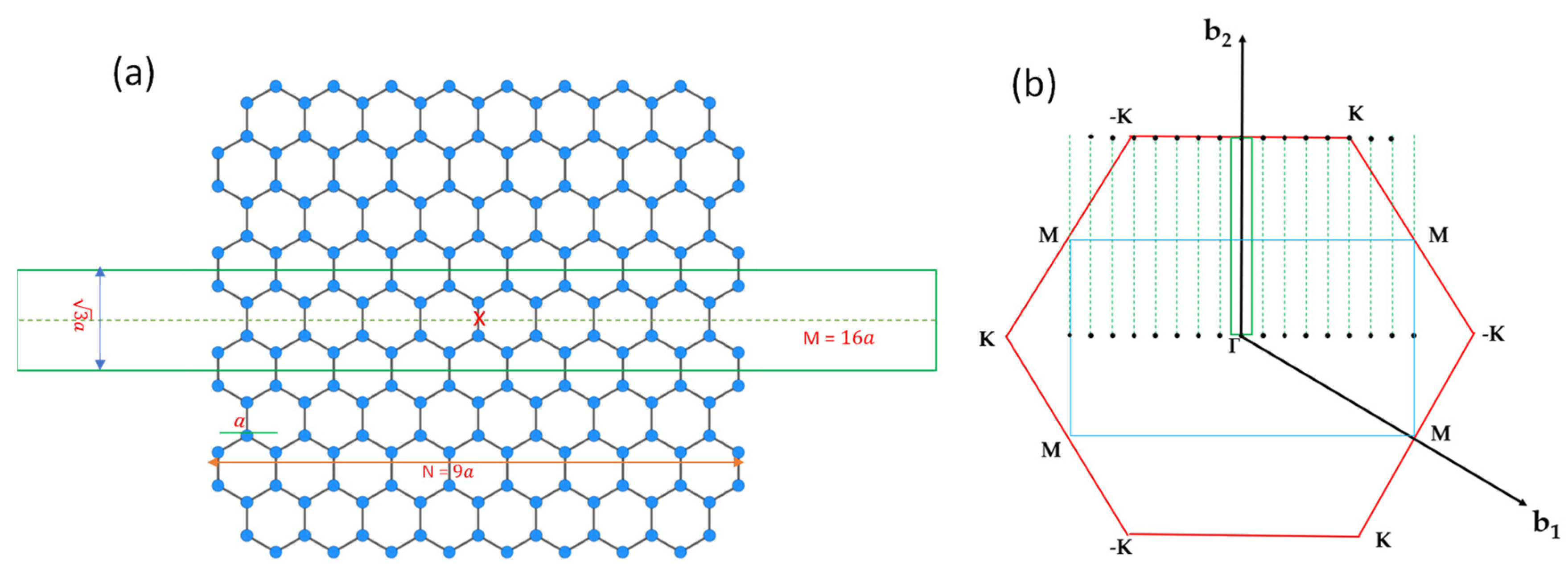
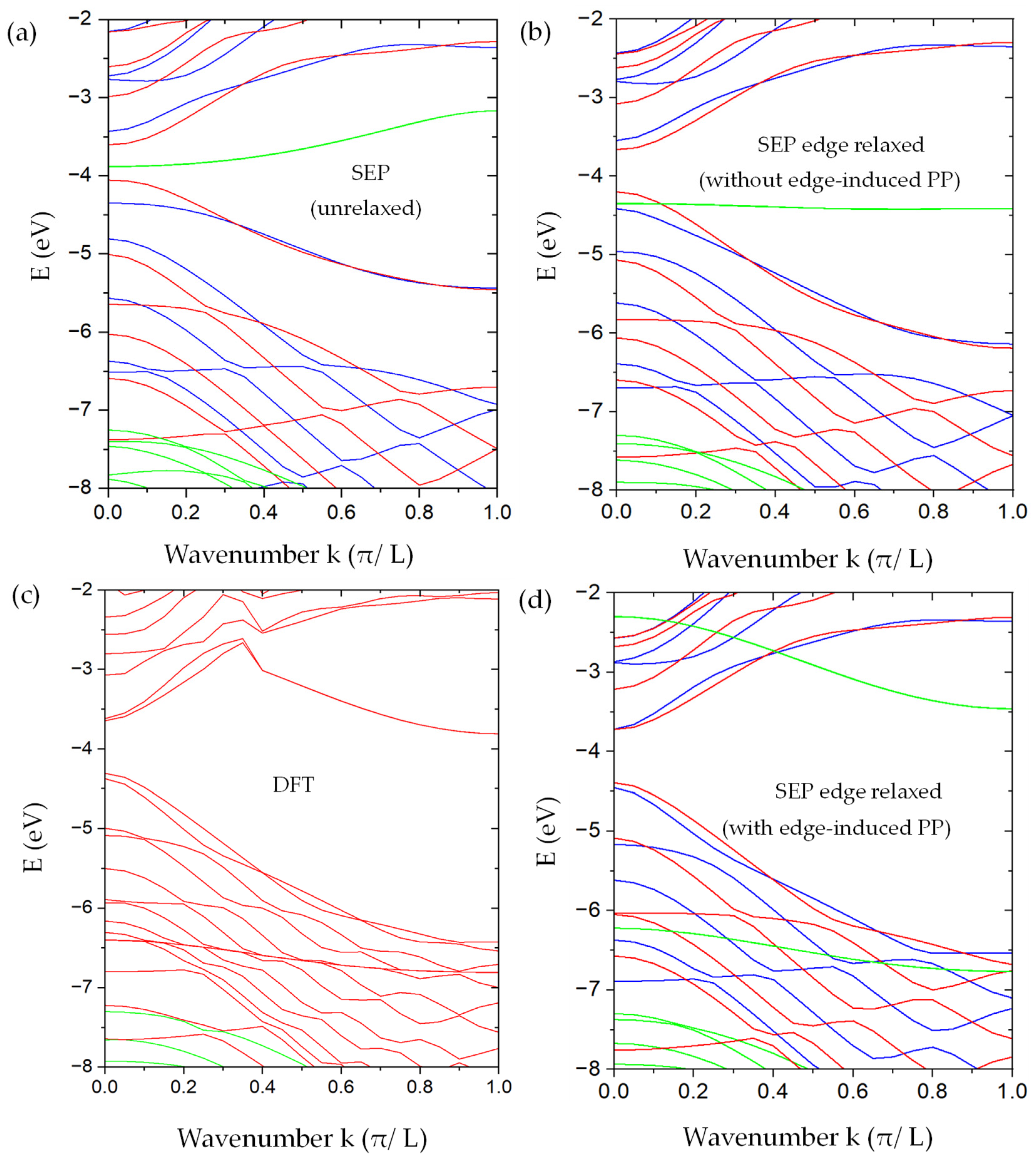
3.2. Band Structure of Armchair Graphene Nanoribbon
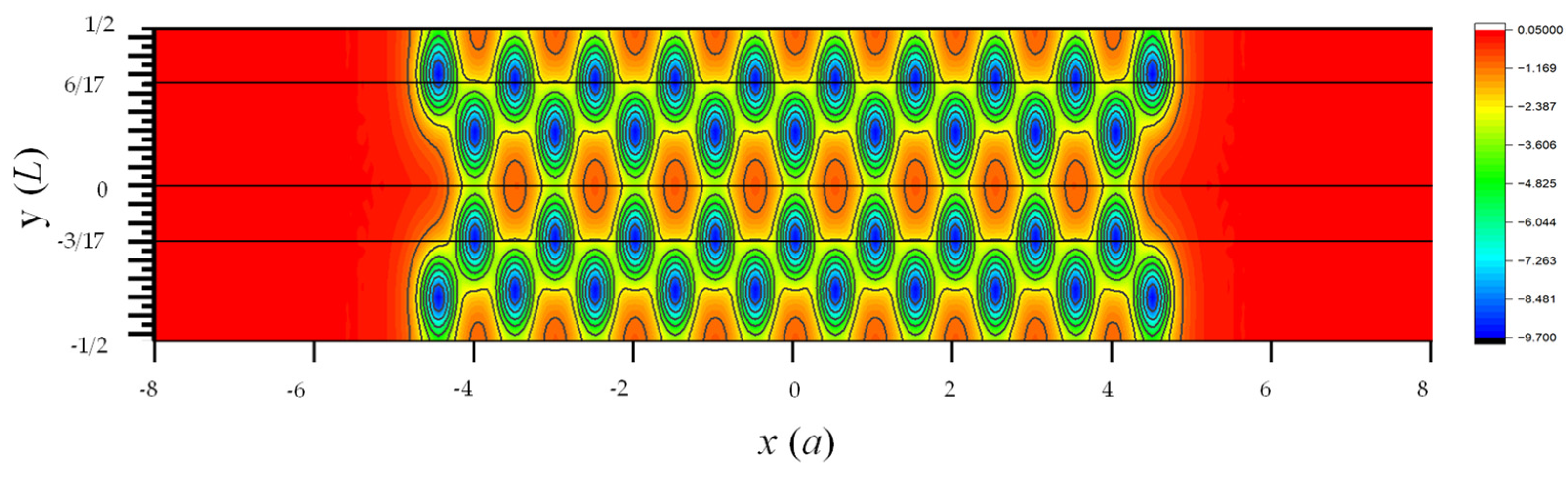
3.3. Modification of Pseudopotential for Edge Atoms of Armchair Graphene Nanoribbon
3.4. Comparison with Experiments
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Matrix Elements of Nonlocal Corrections in Overlap and Potential for Graphene
Appendix B
Matrix Elements for the Hamiltonian of Armchair Graphene Nanoribbon
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [Green Version]
- Mak, K.F.; Lee, C.G.; Hone, J.; Shan, J.; Heinz, T.F. Atomically thin MoS2: A new direct-gap semiconductor. Phys. Rev. Lett. 2010, 105, 136805. [Google Scholar] [CrossRef] [Green Version]
- Hohenberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, B864. [Google Scholar] [CrossRef] [Green Version]
- Castro Neto, A.H.; Guinea, F.; Peres, N.M.R.; Novoselov, K.S.; Geim, A.K. The electronic properties of graphene. Rev. Mod. Phys. 2009, 81, 109–162. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.; Li, Z.Y.; Duan, W.H. Thermal and thermoelectric properties of graphene. Small 2014, 10, 2182. [Google Scholar] [CrossRef] [Green Version]
- Qiu, D.Y.; da Jordana, F.H.; Louie, S.G. Optical Spectrum of MoS2: Many-Body Effects and Diversity of Exciton States. Phys. Rev. Lett. 2013, 111, 216805. [Google Scholar] [CrossRef] [Green Version]
- Hybertsen, M.S.; Louie, S.G. Electron correlation in semiconductors and insulators: Band gaps and quasiparticle energies. Phys. Rev. B 1986, 34, 5390. [Google Scholar] [CrossRef]
- Li, G.W.; Chang, Y.C. Planar-basis pseudopotential calculations of the Si(001)2×1 surface with and without hydrogen passivation. Phys. Rev. B 1993, 48, 12032. [Google Scholar] [CrossRef]
- Li, G.W.; Chang, Y.C. Electronic structure of As/Si(001)2×1 and Sb/Si(001)2×1 surfaces. Phys. Rev. B 1994, 50, 8675. [Google Scholar] [CrossRef]
- Chang, Y.C.; Li, G.W. Planar-basis pseudopotential method and planar Wannier functions for surfaces and heterostructures. Comput. Phys. Commun. 1996, 95, 158. [Google Scholar] [CrossRef]
- Ren, C.Y.; Hsue, C.S.; Chang, Y.C. A Mixed Basis Density Functional Approach for Low-Dimensional Systems with B-splines. Comput. Phys. Commun. 2015, 188, 94. [Google Scholar] [CrossRef] [Green Version]
- Ren, C.Y.; Hsue, C.S.; Chang, Y.C. A Mixed Basis Density Functional Approach for One dimensional Systems with B-splines. Comput. Phys. Commun. 2016, 202, 188. [Google Scholar] [CrossRef] [Green Version]
- Ren, C.Y.; Chang, Y.C. Density functional calculations of atomic structure, charging effect, and static dielectric constant of two-dimensional systems based on B-splines. Phys. E 2022, 140, 115203. [Google Scholar] [CrossRef]
- Cohen, M.L.; Bergstresser, T.K. Band Structure and Pseudopotential Form Factors for Fourteen Semiconductors of the Diamond and Zinc-blende Structure. Phys. Rev. 1966, 141, 789. [Google Scholar] [CrossRef]
- Chelikowsky, J.R.; Cohen, M.L. Nonlocal pseudopotential calculations for the electronic structure of eleven diamond and zinc-blende semiconductors. Phys. Rev. B 1976, 14, 556. [Google Scholar] [CrossRef]
- Vanderbilt, D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Phys. Rev. B 1990, 41, 7892. [Google Scholar] [CrossRef]
- Son, Y.W.; Cohen, M.L.; Louie, S.G. Energy Gaps in Graphene Nanoribbons. Phys. Rev. Lett. 2006, 97, 216803. [Google Scholar] [CrossRef] [Green Version]
- Son, Y.-W.; Cohen, M.L.; Louie, S.G. Half-metallic graphene nanoribbons. Nature 2007, 446, 342. [Google Scholar] [CrossRef] [Green Version]
- Fischetti, M.V.; Kim, J.; Narayanan, S.; Ong, Z.Y.; Sachs, C.; Ferry, D.K.; Aboud, S.J. Pseudopotential-based studies of electron transport in graphene and graphene nanoribbons. J. Phys. Condens. Matter 2013, 25, 473202. [Google Scholar] [CrossRef]
- Nakada, K.; Fujita, M.; Dresselhaus, G.; Dresselhaus, M.S. Edge state in graphene ribbons: Nanometer size effect and edge shape dependence. Phys. Rev. B 1996, 54, 17954. [Google Scholar] [CrossRef] [Green Version]
- Cai, J.; Ruffieux, P.; Jaafar, R.; Bieri, M.; Braun, T.; Blankenburg, S.; Muoth, M.; Seitsonen, A.P.; Saleh, M.; Feng, X.; et al. Atomically precise bottom-up fabrication of graphene nanoribbons. Nature 2010, 466, 470. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.C.; Cao, T.; Chen, C.; Pedramrazi, Z.; Haberer, D.; de Oteyza, D.G.; Fischer, F.R.; Louie, S.G.; Crommie, M.F. Molecular bandgap engineering of bottom-up synthesized graphene nanoribbon heterojunctions. Nat. Nanotechnol. 2015, 10, 156–160. [Google Scholar] [CrossRef] [Green Version]
- Yan, L.H.; Liljeroth, P. Engineered electronic states in atomically precise artificial lattices and graphene nanoribbons. Adv. Phys. X 2019, 4, 1651672. [Google Scholar] [CrossRef] [Green Version]
- Rizzo, D.J.; Veber, G.; Jiang, J.W.; McCurdy, R.; Cao, T.; Bronner, C.; Chen, T.; Louie, S.G.; Fischer, F.R.; Crommie, M.F. Inducing metallicity in graphene nanoribbons via zero-mode superlattices. Science 2020, 369, 1597. [Google Scholar] [CrossRef] [PubMed]
- Sevincli, H.; Topsakal, M.; Ciraci, S. Superlattice structures of graphene-based armchair nanoribbons. Phys. Rev. B 2008, 78, 245402. [Google Scholar] [CrossRef]
- Llinas, J.P.; Fairbrother, A.; Borin Barin, G.; Shi, W.; Lee, K.; Wu, S.; Choi, B.Y.; Braganza, R.; Lear, J.; Kau, N.; et al. Short-channel field-effect transistors with 9-atom and 13-atom wide graphene nanoribbons. Nat. Commun. 2017, 8, 633. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Wang, H.S.; Ma, C.; Chen, L.; Jiang, C.; Chen, C.; Xie, X.; Li, A.P.; Wang, X. Graphene nanoribbons for quantum electronics. Nat. Rev. Phys. 2021, 3, 791–802. [Google Scholar] [CrossRef]
- Xia, F.; Mueller, T.; Lin, Y.; Garcia, A.V.; Avouris, P. Ultrafast graphene photodetector. Nat. Nanotech 2009, 4, 839–843. [Google Scholar] [CrossRef] [Green Version]
- Do, T.-N.; Gumbs, G.; Huang, D.; Hoi, B.D.; Shih, P.-H. Role Played by Edge-Defects in the Optical Properties of Armchair Graphene Nanoribbons. Nanomaterials 2021, 11, 3229. [Google Scholar] [CrossRef]
- Sun, Q.; Yan, Y.; Yao, X.L.; Mullen, K.; Narita, A.; Fasel, R.; Ruffieux, P. Evolution of the topological energy band in graphene nanoribbons. J. Phys. Chem. Lett. 2021, 12, 8679. [Google Scholar] [CrossRef]
- Rizzo, D.J.; Jiang, J.W.; Joshi, D.; Veber, G.; Bronner, C.; Durr, R.A.; Jacobse, P.H.; Cao, T.; Kalayjian, A.; Rodriguez, H.; et al. Rationally designed topological quantum dots in bottom-up graphene nanoribbons. ACS Nano 2021, 15, 20633. [Google Scholar] [CrossRef] [PubMed]
- Groning, O.; Wang, S.; Yao, X.; Pignedoli, C.A.; Barin, G.B.; Daniels, C.; Cupo, A.; Meunier, V.; Feng, X.; Narita, A. Engineering of robust topological quantum phases in graphene nanoribbons. Nature 2018, 560, 209. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lin, K.S.; Chou, M.Y. Topological properties of gapped graphene nanoribbons with spatial symmetries. Nano Lett. 2018, 8, 7254. [Google Scholar] [CrossRef] [Green Version]
- Wakabayashi, K.; Fujita, M.; Ajiki, H.; Sigrist, M. Electronic and magnetic properties of nanographite ribbons. Phys. Rev. B 1999, 59, 8271. [Google Scholar] [CrossRef] [Green Version]
- Topsakal, M.; Sevincli, H.; Ciraci, S. Spin confinement in the superlattices of graphene ribbons. Appl. Phys. Lett. 2008, 92, 173118. [Google Scholar] [CrossRef]
- Kuo, D.M.T.; Chang, Y.C. Contact effects on thermoelectric properties of textured graphene nanoribbons. Nanomaterials 2022, 12, 3357. [Google Scholar] [CrossRef]
- Darancet, P.; Olevano, V.; Mayou, D. Coherent electronic transport through graphene constrictions: Subwavelength regime and optical analogy. Phys. Rev. Lett. 2009, 102, 136803. [Google Scholar] [CrossRef] [Green Version]
- Zuev, Y.M.; Chang, W.; Kim, P. Thermoelectric and magnetothermoelectric transport measurements of graphene. Phys. Rev. Lett. 2009, 102, 096807. [Google Scholar] [CrossRef] [Green Version]
- Carl, D.B. A Practical Guide to Splines; Springer: New York, NY, USA, 1987. [Google Scholar]
- Wei, P.; Bao, W.Z.; Pu, Y.; Lau, C.N.; Shi, J. Anomalous thermoelectric transport of Dirac particles in graphene. Phys. Rev. Lett. 2009, 102, 166808. [Google Scholar] [CrossRef]
- Wang, L.W.; Zunger, A. Pseudopotential calculations of nanoscale CdSe quantum dots. Phys. Rev. B 1996, 53, 9579. [Google Scholar] [CrossRef] [Green Version]
- Sanchez, A.M.; Cristobal, A.G.; Bester, G. Semiempirical pseudopotential approach for nitride-based nanostructured and ab initio-based passivation of free surfaces. Phys. Rev. B 2012, 86, 205430. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef] [PubMed]
- Ceperley, D.M.; Alder, B.J. Ground State of the Electron Gas by a Stochastic Method. Phys. Rev. Lett. 1980, 45, 566. [Google Scholar] [CrossRef] [Green Version]
- Perdew, J.P.; Zunger, A. Self-interaction correction to density-functional approximations for many-electron systems. Phys. Rev. B 1981, 23, 5048. [Google Scholar] [CrossRef] [Green Version]
- Shewchuk, J.R. An Introduction to the Conjugate Gradient Method Without the Agonizing Pain; Carnegie-Mellon University, Department of Computer Science: Pittsburgh, PA, USA, 1994. [Google Scholar]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys. Rev. 1965, 140, A1133. [Google Scholar] [CrossRef] [Green Version]
- Chang, Y.C. Theory of Electronic Properties of Si(111) 7×7 Surface. J. Vac. Sci. Technol. 1983, 1, 709. [Google Scholar] [CrossRef]
- Bonilla, L.L.; Teitsworth, S.W. Nonlinear Wave Methods for Charge Transport; Wiley-VCH: Weinheim, Germany, 2010. [Google Scholar]
- Carlstrom, J.E.; Plambeck, R.L.; Thornton, D.D. A Continuously Tunable 65-115 GHz Gunn Oscillator. IEEE 1985, 33, 610–619. [Google Scholar]
- Tapasztó, L.; Dobrik, G.; Lambin, P.; Biro, L.P. Tailoring the atomic structure of graphene nanoribbons by scanning tunnelling microscope lithography. Nat. Nanotechnol. 2008, 3, 397–401. [Google Scholar] [CrossRef] [Green Version]
- Ma, C.; Xiao, Z.; Puretzky, A.A.; Wang, H.; Mohsin, A.; Huang, J.; Liang, L.; Luo, Y.; Lawrie, B.J.; Gu, G.; et al. Engineering edge states of graphene nanoribbons for narrow-band photoluminescence. ACS Nano 2020, 14, 5090–5098. [Google Scholar] [CrossRef]
- Talirz, L.; Söde, H.; Dumslaff, T.; Wang, S.; Sanchez-Valencia, J.R.; Liu, J.; Shinde, P.; Pignedoli, C.A.; Liang, L.; Meunier, V.; et al. On-Surface Synthesis and Characterization of 9-Atom Wide Armchair Graphene Nanoribbons. ACS Nano 2017, 11, 1380–1388. [Google Scholar] [CrossRef]
- Rizzo, D.J.; Veber, G.; Cao, T.; Bronner, C.; Chen, T.; Zhao, F.; Rodriguez, H.; Louie, S.G.; Crommie, M.F.; Fischer, F.R. Topological band engineering of graphene nanoribbons. Nature 2018, 560, 204–208. [Google Scholar] [CrossRef] [Green Version]
- Wang, S.; Kharche, N.; Costa Girão, E.; Feng, X.; Mullen, K.; Meunier, V.; Fasel, R.; Ruffieux, P. Quantum dots in graphene nanoribbons. Nano Lett. 2017, 17, 4277–4283. [Google Scholar] [CrossRef]
- Merino-Díez, N.; Garcia-Lekue, A.; Carbonell-Sanromà, E.; Li, J.; Corso, M.; Colazzo, L.; Sedona, F.; Sánchez-Portal, D.; Pascual, J.I.; de Oteyza, D.G. Width-Dependent Band Gap in Armchair Graphene Nanoribbons Reveals Fermi Level Pinning on Au(111). ACS Nano 2017, 11, 11661–11668. [Google Scholar] [CrossRef]
- Ma, C.; Liang, L.; Xiao, Z.; Puretzky, A.A.; Hong, K.; Lu, W.; Meunier, V.; Bernholc, J.; Li, A.P. Seamless staircase electrical contact to semiconducting graphene nanoribbons. Nano Lett. 2017, 17, 6241–6247. [Google Scholar] [CrossRef]
- Franc, G.; Gourdon, A. Covalent networks through on-surface chemistry in ultra-high vacuum: State-of-the-art and recent developments. Phys. Chem. Chem. Phys. 2011, 13, 14283–14292. [Google Scholar] [CrossRef]
- Kimouche, A.; Ervasti, M.M.; Drost, R.; Halonen, S.; Harju, A.; Joensuu, P.M.; Sainio, J.; Liljeroth, P. Ultra-narrow metallic armchair graphene nanoribbons. Nat. Commun. 2015, 6, 10177. [Google Scholar] [CrossRef] [Green Version]
- Han, P.; Akagi, K.; Canova, F.; Shimizu, R.; Oguchi, H.; Shiraki, S.; Weiss, P.S.; Asao, N.; Hitosugi, T. Self-assembly strategy for fabricating connected graphene nanoribbons. ACS Nano 2015, 9, 12035–12044. [Google Scholar] [CrossRef] [Green Version]
- Senkovskiy, B.V.; Usachov, D.Y.; Fedorov, A.V.; Haberer, D.; Ehlen, N.; Fischer, F.R.; Grüneis, A. Finding the hidden valence band of N= 7 armchair graphene nanoribbons with angle-resolved photoemission spectroscopy. 2D Mater. 2018, 5, 035007. [Google Scholar] [CrossRef] [Green Version]
- Zheng, H.; Wang, Z.F.; Luo, T.; Shi, Q.W.; Chen, J. Analytical study of electronic structure in armchair graphene nanoribbons. Phys. Rev. B 2007, 75, 165414. [Google Scholar] [CrossRef] [Green Version]
- Liang, L.; Meunier, V. Electronic structure of assembled graphene nanoribbons: Substrate and many-body effects. Phys. Rev. B 2012, 86, 195404. [Google Scholar] [CrossRef]


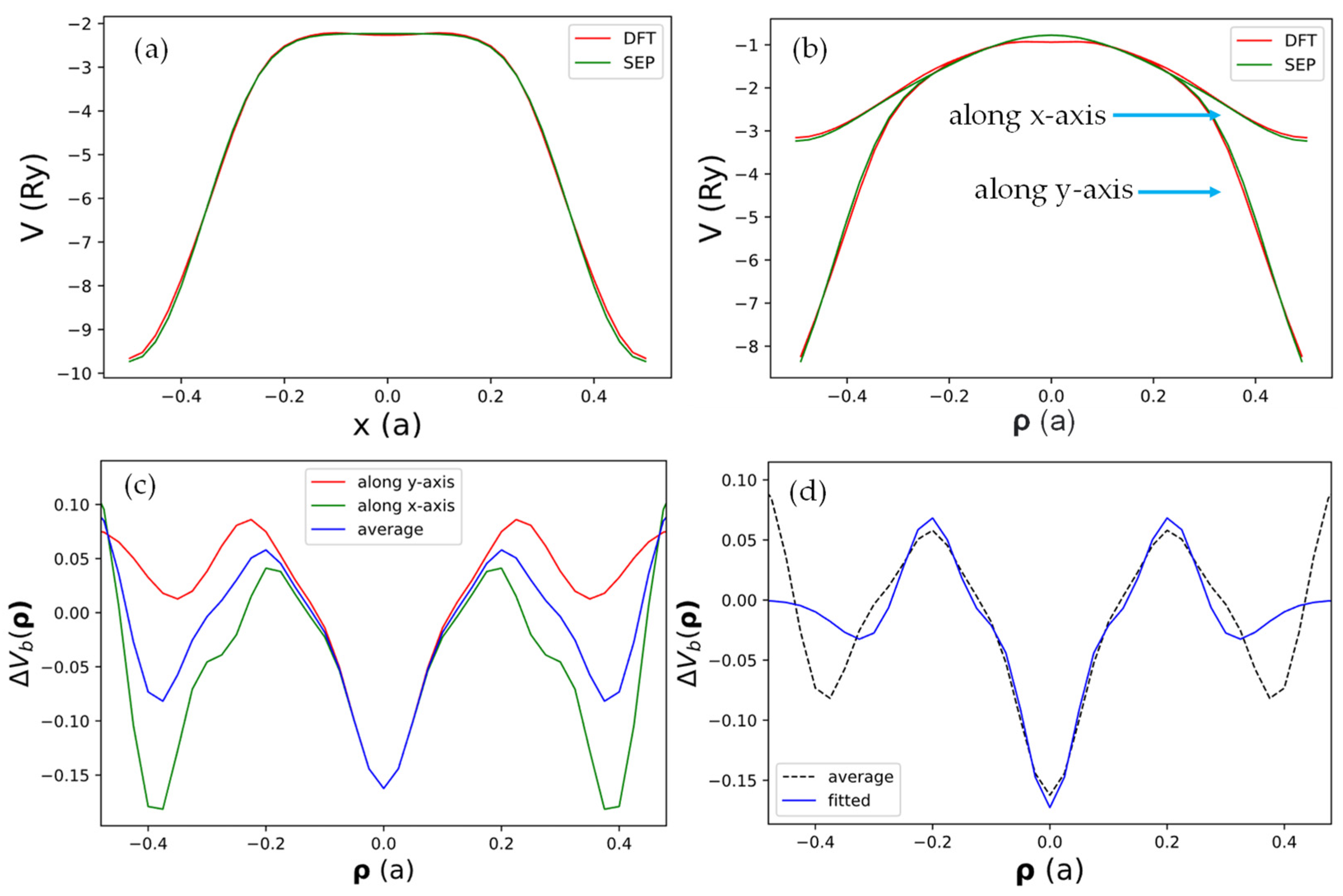
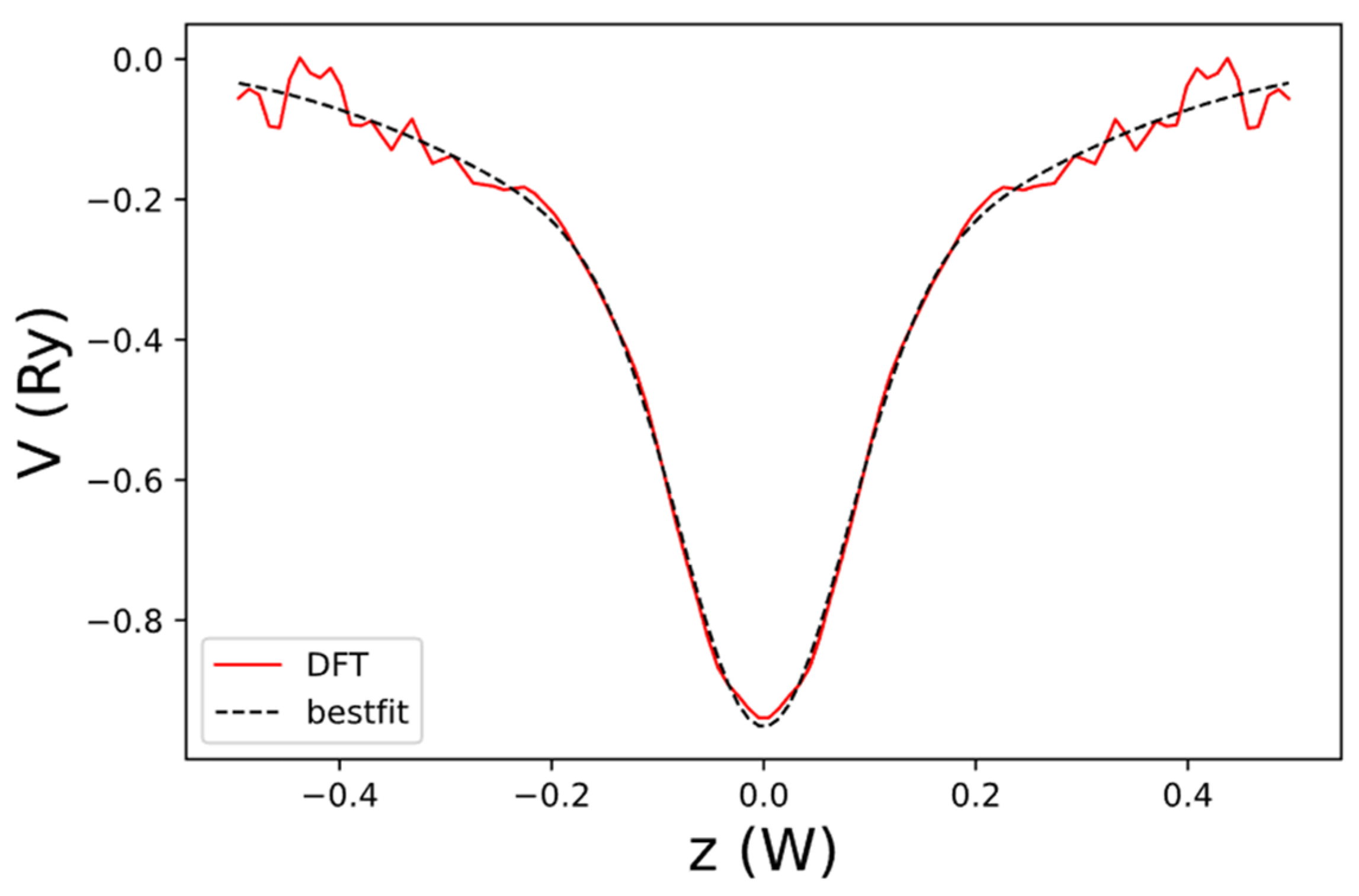
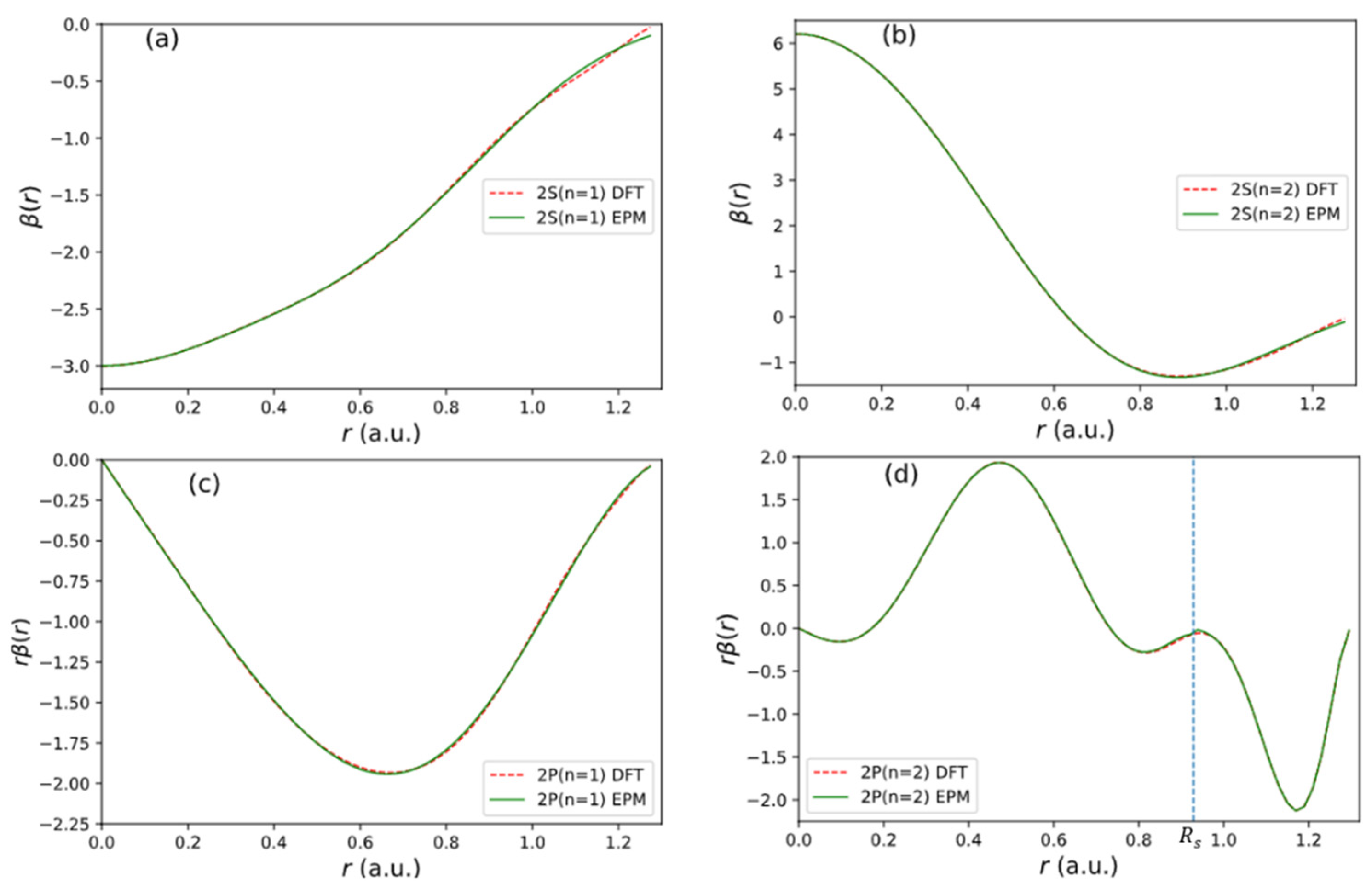
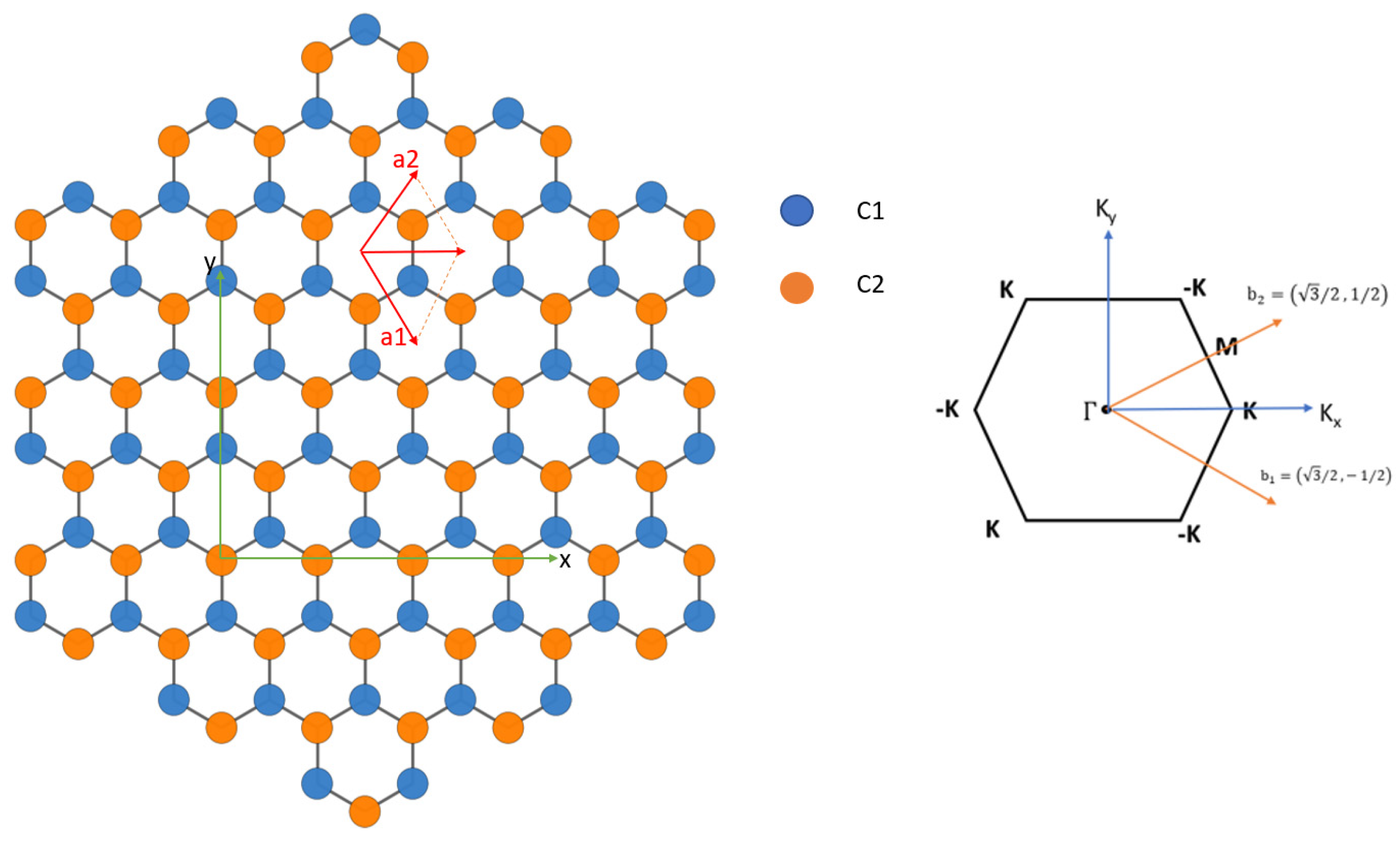




| Exponents | Coefficients | ||||
|---|---|---|---|---|---|
| 0.0396 | 1.4100 | 0.3461 | −0.3682 | −1.7360 | −1.5710 |
| Type | Exponent | |||||||
|---|---|---|---|---|---|---|---|---|
| Short Range (S) | 2.07 | 2.0372 | −16.164 | 13.912 | −2.8969 | 0.04494 | −0.00574 | 0.000224 |
| Long Range (L) | 2.07 | 2.6251 | −5.6668 | 2.1280 | 1.0239 | −0.1650 | 0.03132 | −0.002615 |
| Exponents in Equations (25) and (28) | Coefficients in Equation (25) | |||||||
|---|---|---|---|---|---|---|---|---|
| 3.0053 | 0.3601 | 0.0383 | −0.1727 | 1.5253 | −6.4817 | 11.5249 | −5.0681 | 0.6930 |
| Orbitals | |||||||
|---|---|---|---|---|---|---|---|
| 2 | 2.747 | 1.3174 | −2.999 | −4.209 | −11.95 | 7.612 | 0 |
| 2 | 2.171 | 1.3174 | 6.206 | −9.434 | −21.03 | 14.21 | 0 |
| 2 | 0.5104 | 1.3174 | −3.941 | −1.411 | 5.485 | −1.939 | 0 |
| 2 | 1.134 | 0.9228 | −2.492 | 94.68 | −365.4 | 480.9 | −209.8 |
| 2 | 0.0 | 1.2953 | −0.9771 | −350.7 | 4210 | −5711 | −4377 |
| n | n’ | l | ||
|---|---|---|---|---|
| 1 | 1 | 0 | 3.490422 | −0.449056 |
| 1 | 2 | 0 | 0.207297 | 0.344889 |
| 2 | 2 | 0 | −2.748230 | −0.212785 |
| 3 | 3 | 1 | 2.474918 | 1.236379 |
| 3 | 4 | 1 | −5.902130 | −0.938122 |
| 4 | 4 | 1 | 9.289400 | 0.631727 |
| −0.25 | −0.34 | 0.10 | −0.42 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paudel, R.K.; Ren, C.-Y.; Chang, Y.-C. Semi-Empirical Pseudopotential Method for Graphene and Graphene Nanoribbons. Nanomaterials 2023, 13, 2066. https://doi.org/10.3390/nano13142066
Paudel RK, Ren C-Y, Chang Y-C. Semi-Empirical Pseudopotential Method for Graphene and Graphene Nanoribbons. Nanomaterials. 2023; 13(14):2066. https://doi.org/10.3390/nano13142066
Chicago/Turabian StylePaudel, Raj Kumar, Chung-Yuan Ren, and Yia-Chung Chang. 2023. "Semi-Empirical Pseudopotential Method for Graphene and Graphene Nanoribbons" Nanomaterials 13, no. 14: 2066. https://doi.org/10.3390/nano13142066
APA StylePaudel, R. K., Ren, C. -Y., & Chang, Y. -C. (2023). Semi-Empirical Pseudopotential Method for Graphene and Graphene Nanoribbons. Nanomaterials, 13(14), 2066. https://doi.org/10.3390/nano13142066







